Lattice Boltzmann Numerical Study on Mesoscopic Seepage Characteristics of Soil–Rock Mixture Considering Size Effect
Abstract
:1. Introduction
2. Materials and Methods
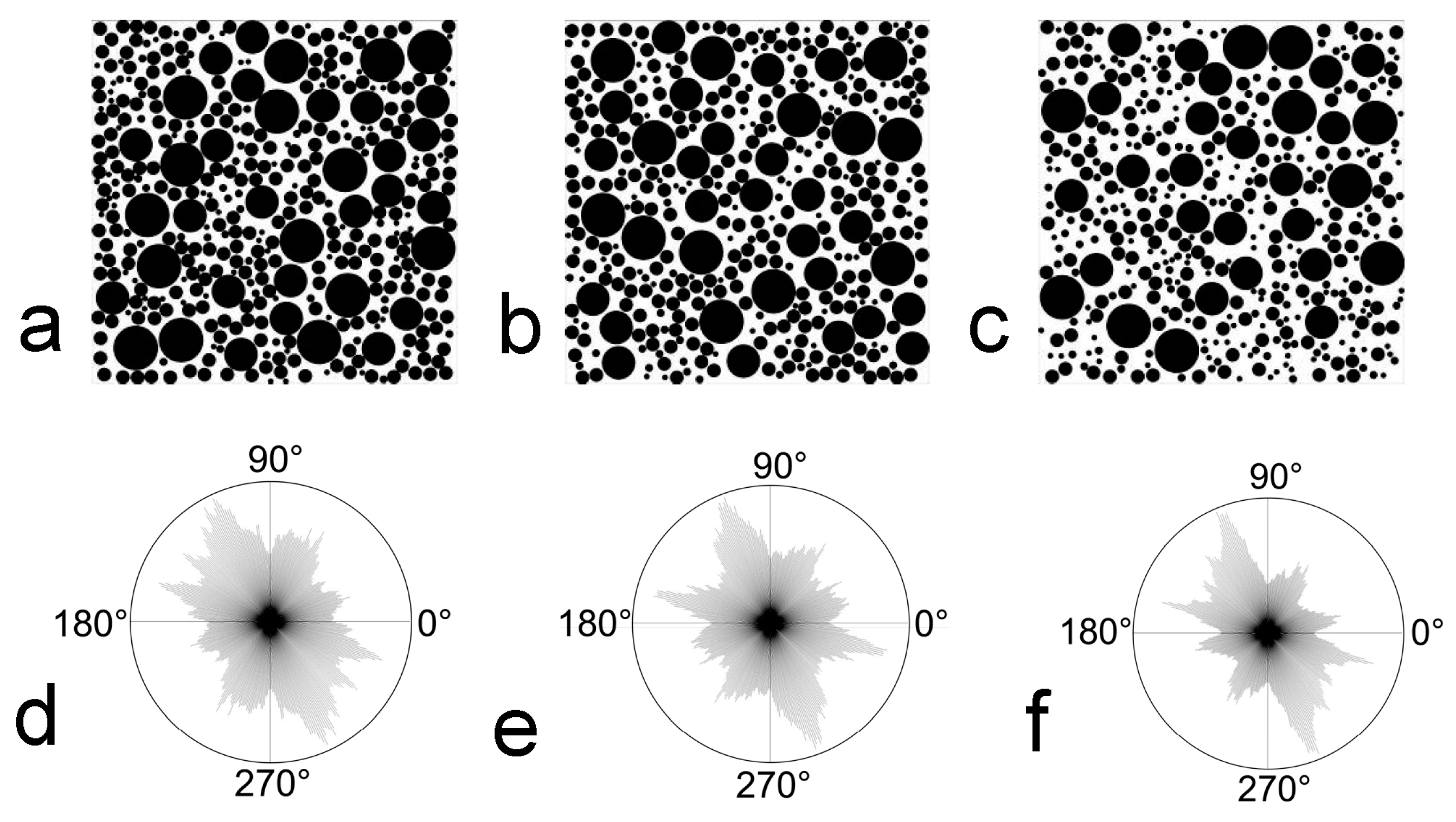
2.1. Discrete Models of Soil–Rock Mixture
2.2. Theoretical Part
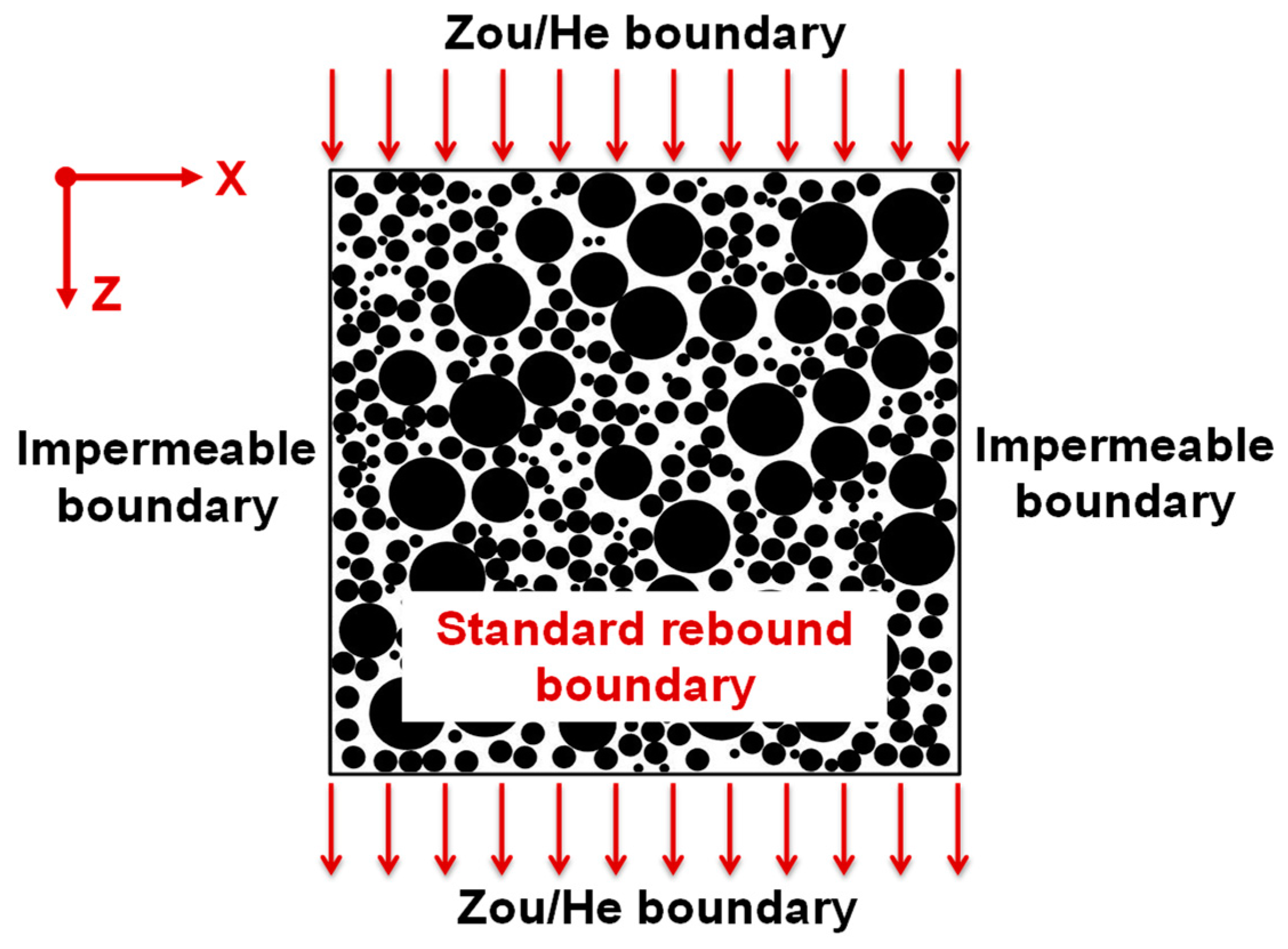
2.2.1. Lattice Boltzmann Theory and Boundary Conditions
2.2.2. Conversion of Lattice Unit and Physical Unit
2.2.3. Soil/Rock Particle Size Threshold
2.2.4. Size Feature Parameters
- (1)
- Model resolution (R) is the term used to describe the amount of data stored in a model image, which is typically expressed as the pixel density per inch (ppi) [28]. The output quality of an image is determined by resolution. The size of the model is determined by the image resolution and image size combined. The more significant the value, the more precise the model and image are.
- (2)
- Model feature size (S) is defined as the arithmetic square root of the product of the numerical model’s length l and width b. S represents the average length of the numerical model size.
- (3)
- The feature length ratio (F), which is defined as the ratio of the rock feature particle size (, refers to the particle size of the m-th type of rock in the SRM) to S, characterizes the relationship between the rock particle size and the model size in the SRM model.
- (4)
- The soil/rock particle size feature ratio (P), which is defined as the ratio of the soil feature particle size (, refers to the particle size of the m-th type of soil in the SRM) to Dr, characterizes the relationship between the soil/rock particle size feature in the SRM model.
2.2.5. Permeability Calculation Theory
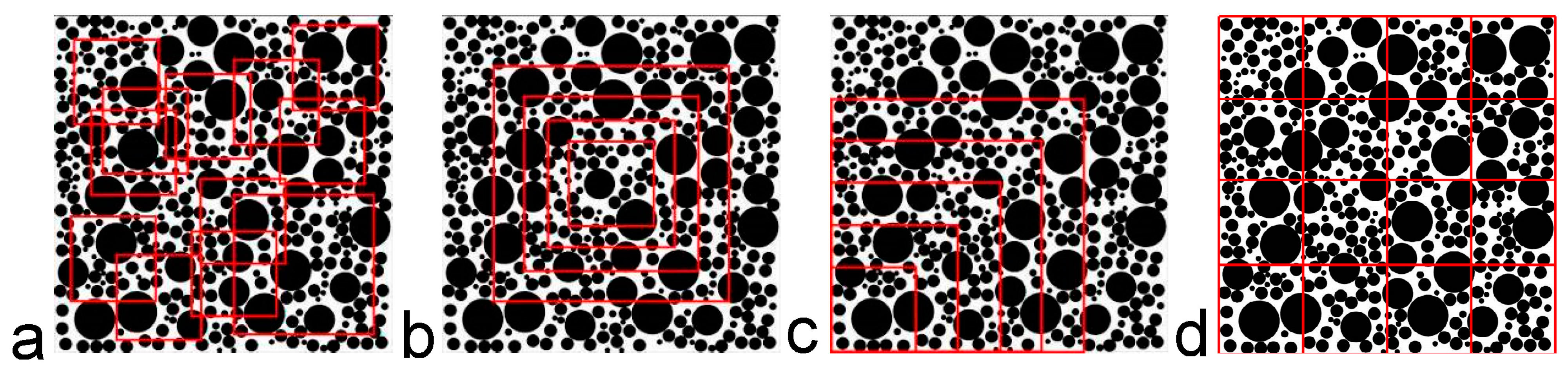
2.3. Model Size Segmentation
3. Results
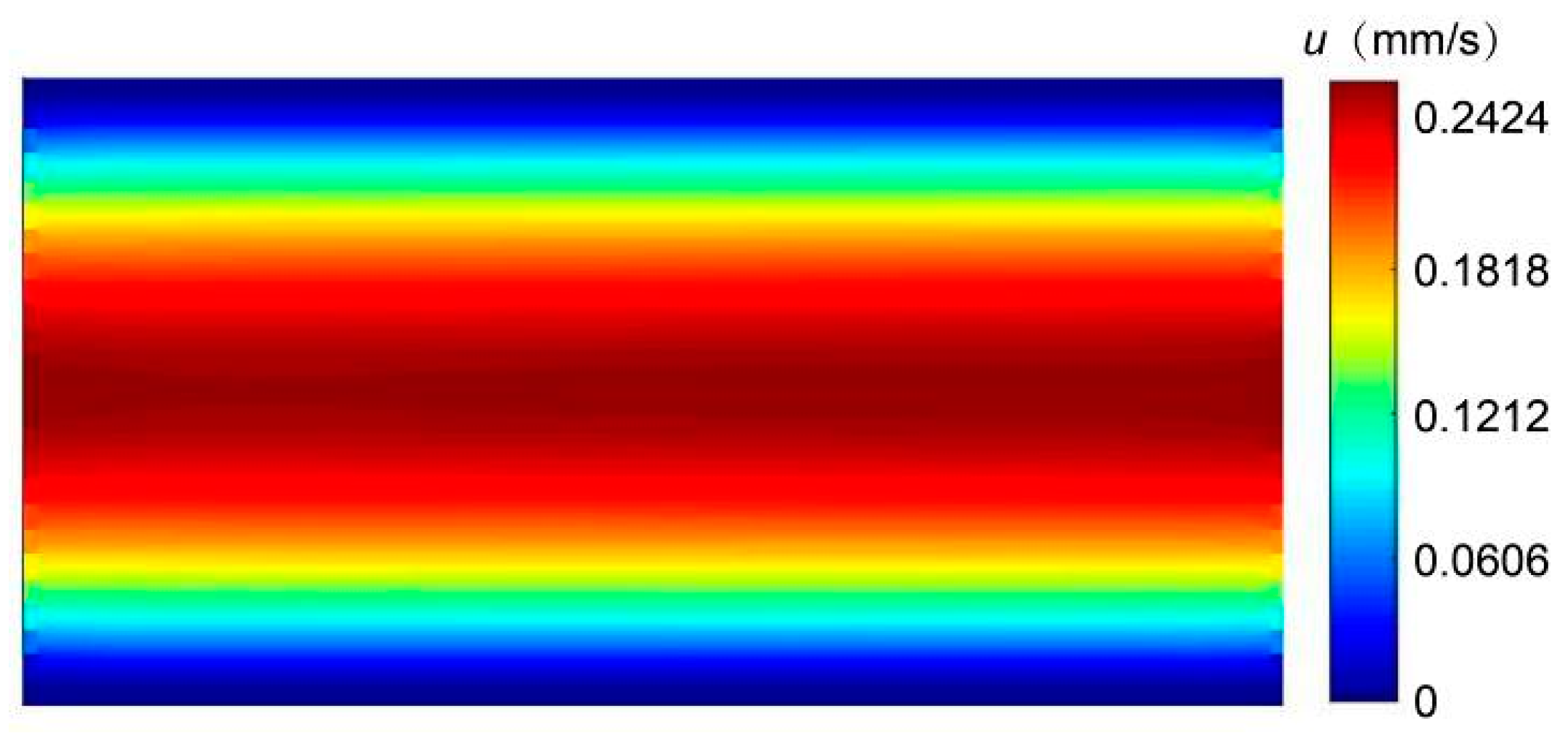
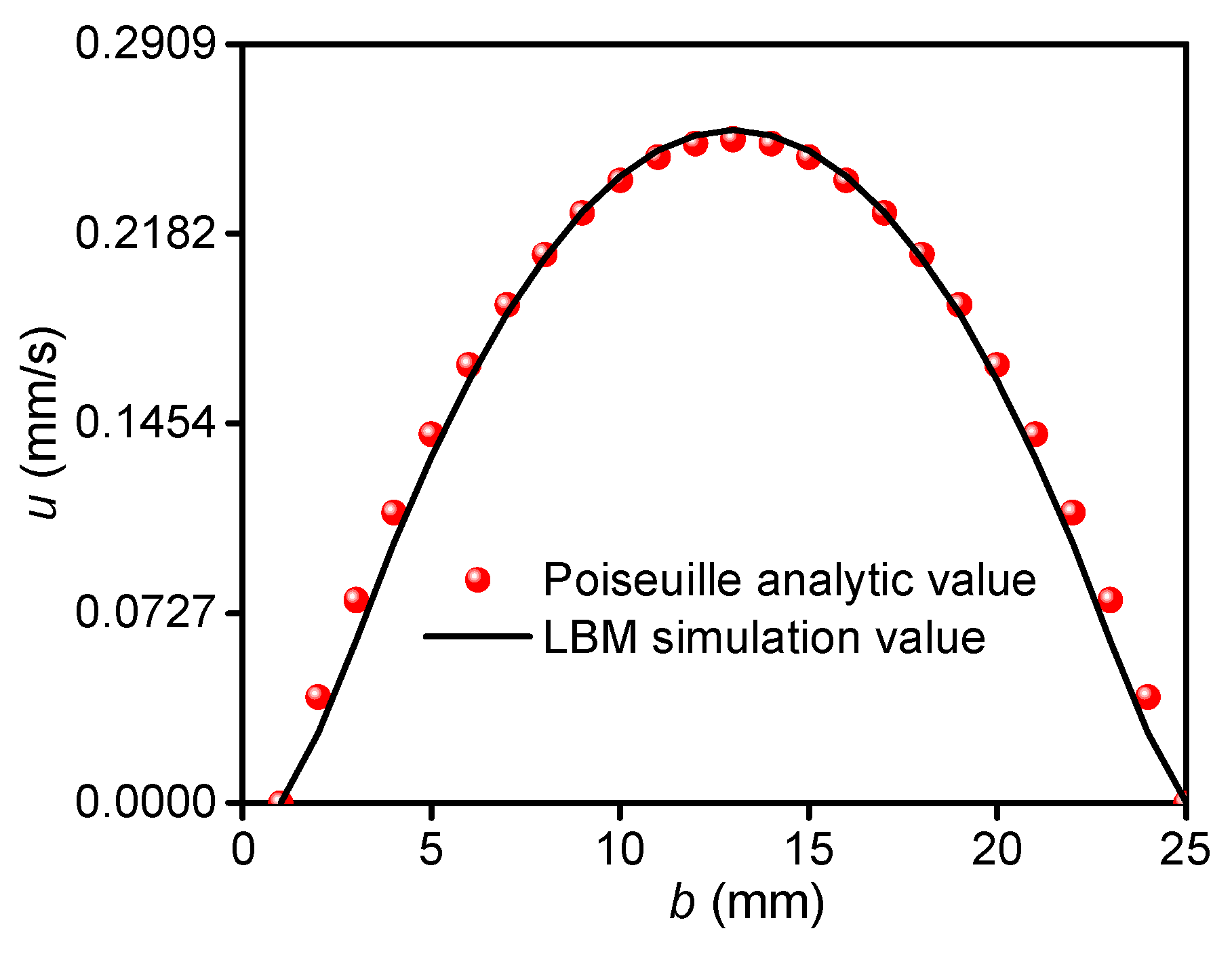
3.1. Numerical Model Validation
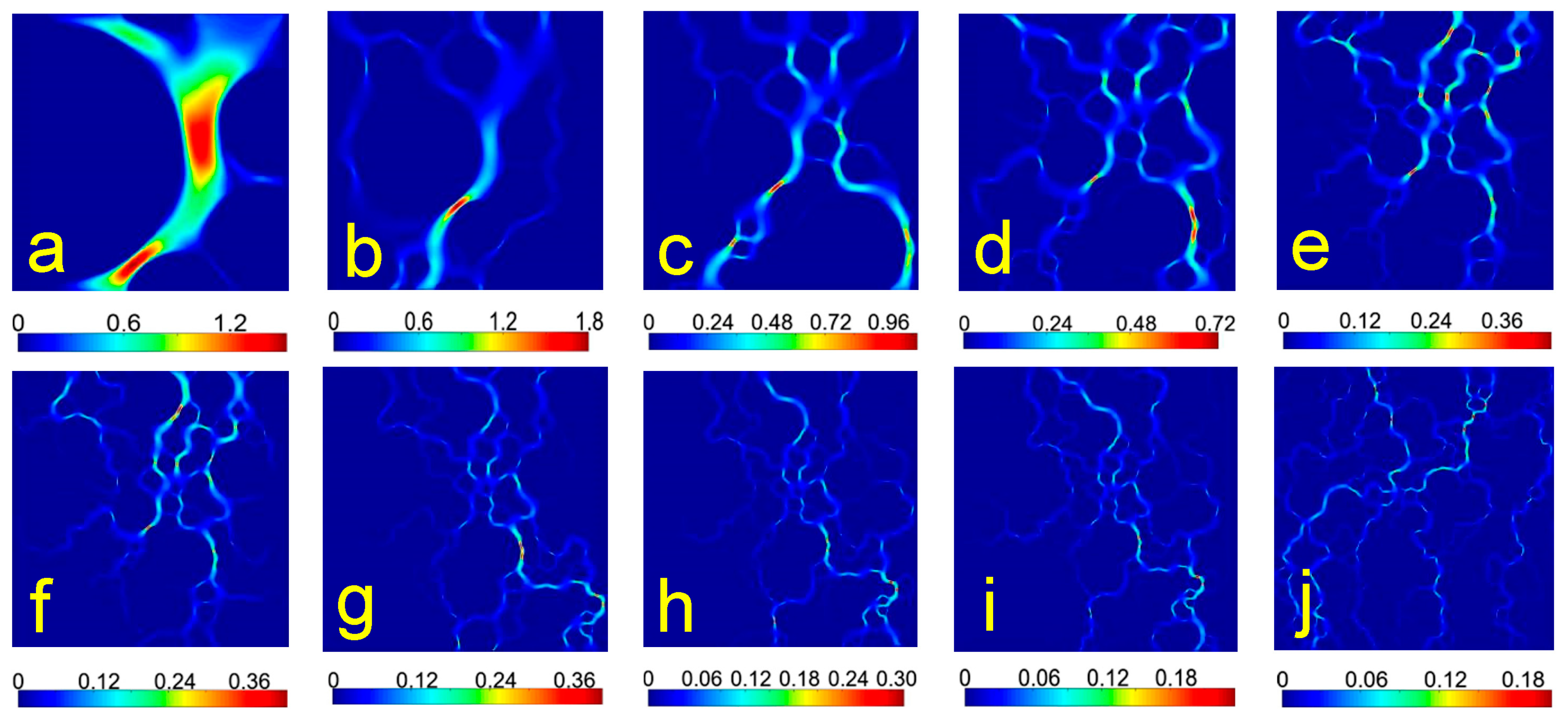
3.2. Influence of Size Effect on Permeability
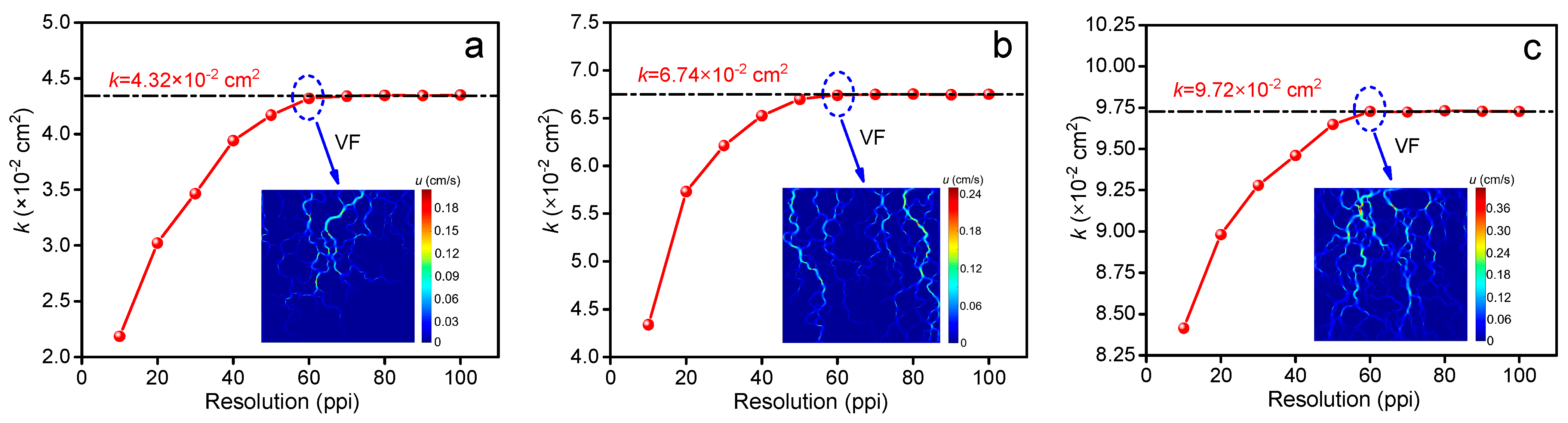
3.2.1. Resolution R
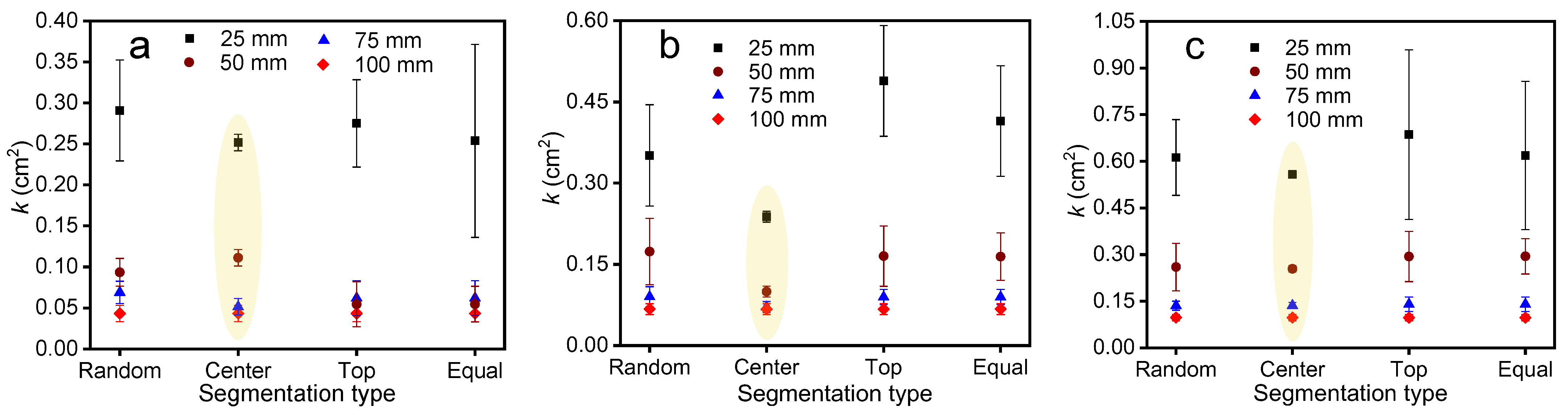
3.2.2. Segmentation Type
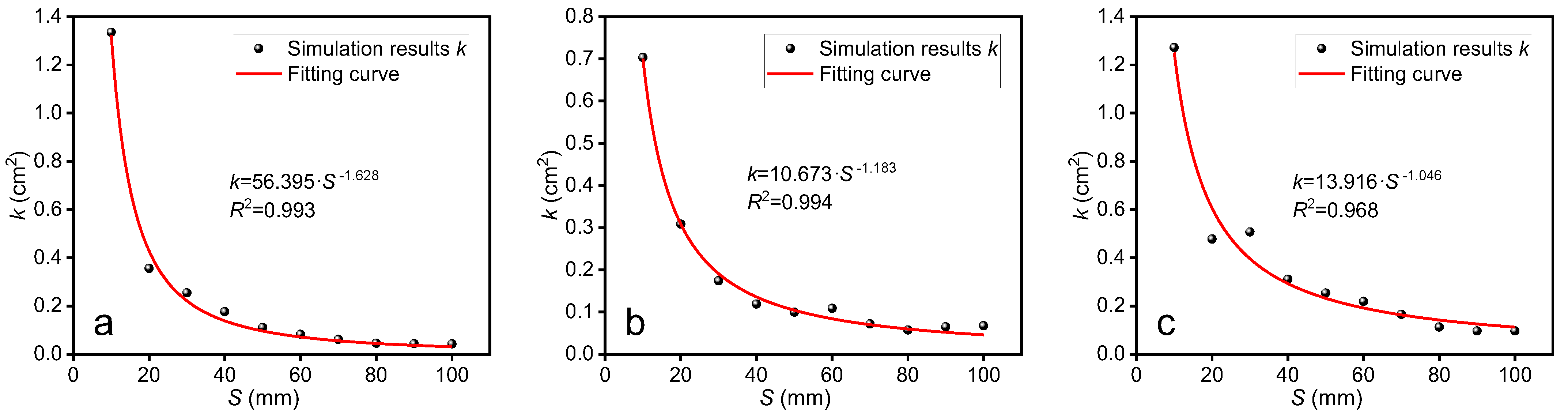
3.2.3. Model Feature Size S
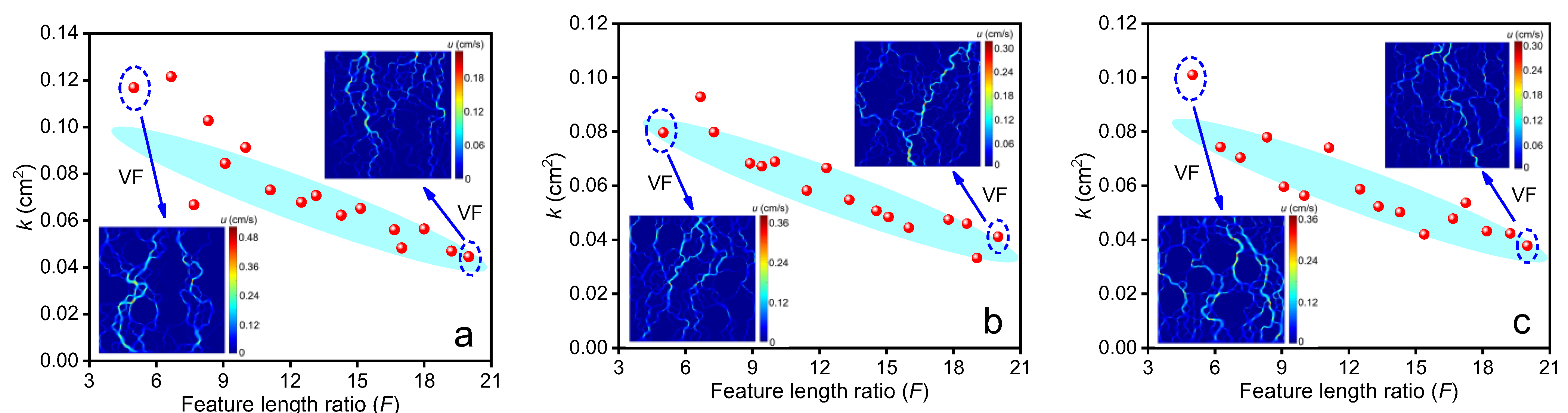
3.2.4. Feature Length Ratio F
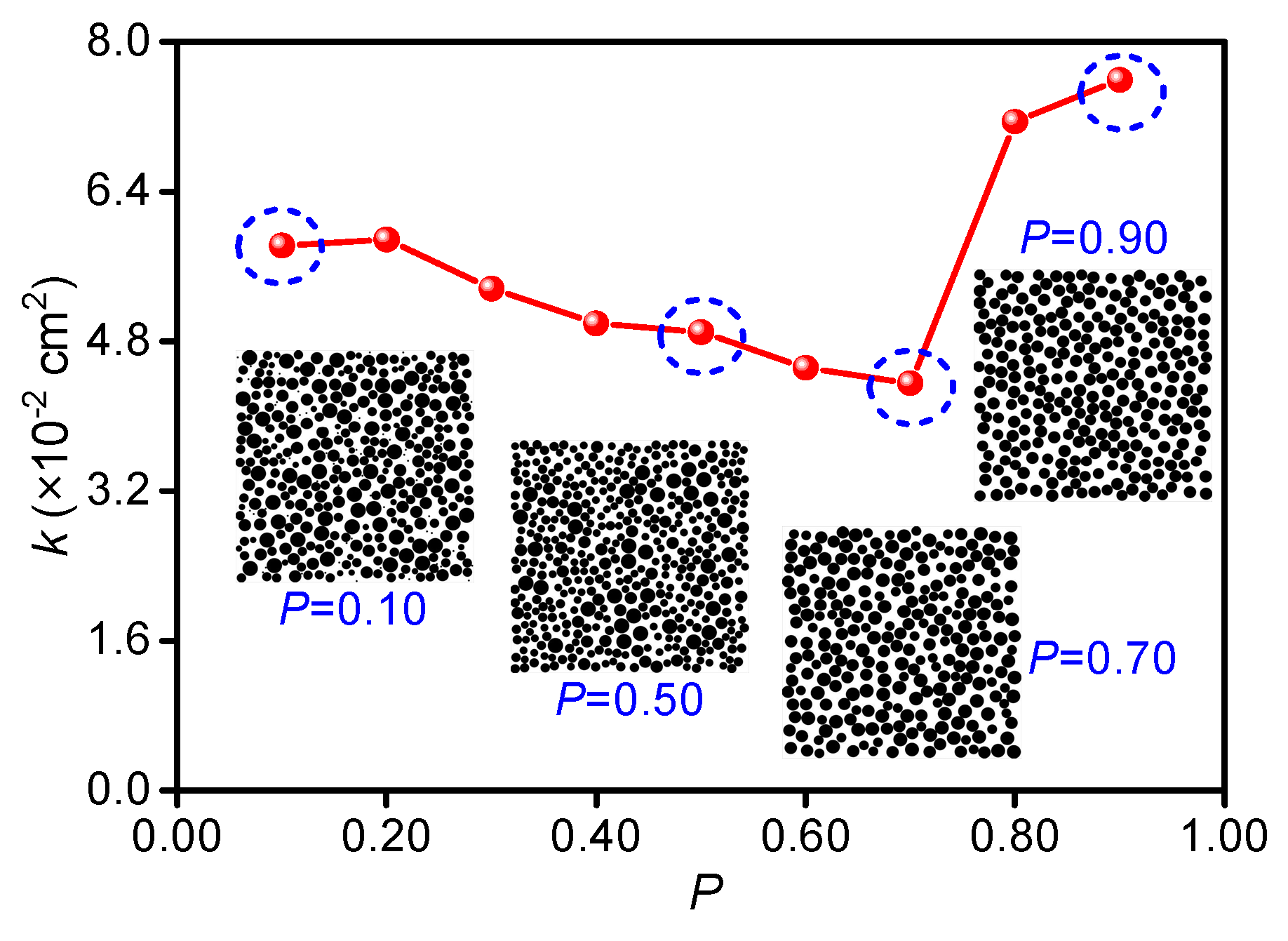
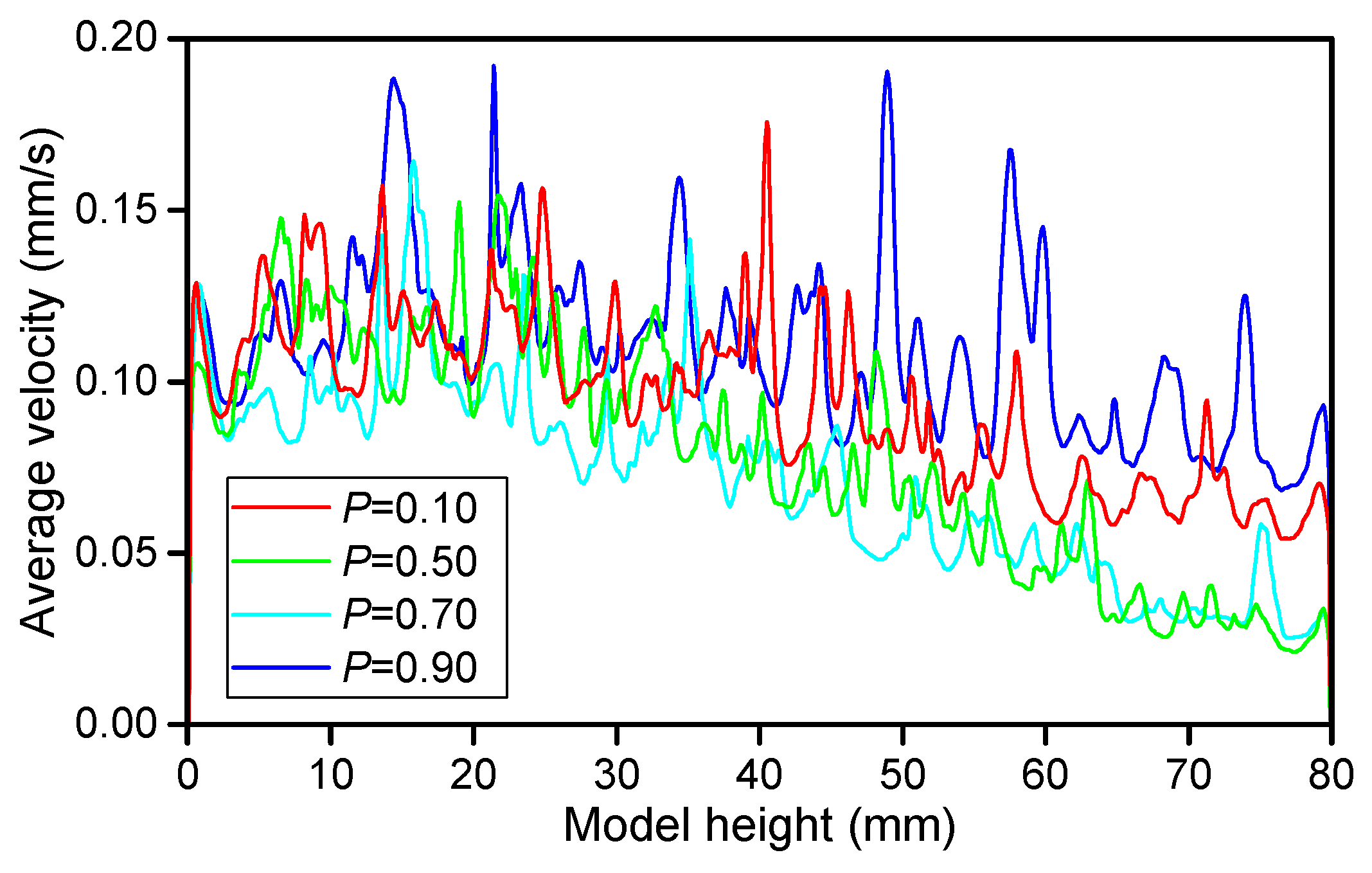
3.2.5. Soil/Rock Particle Size Feature Ratio P
3.3. Discussion
4. Conclusions
- (1)
- As R increases, the permeability of the SRM gradually rises and tends to stabilize when R reaches 60 ppi. The model’s porosity and rock content also have only a minor impact on the correlation between resolution and permeability.
- (2)
- The four segmentation types–center segmentation, random segmentation, equal segmentation, and top segmentation–are in order of decreasing dispersion in the permeability of the model obtained under the same S. The permeability of the model increases with S when using the same segmentation type, exhibiting a high degree of mutual anisotropy. The results for permeability obtained using the top and equal segmentation types are particularly noteworthy.
- (3)
- The permeability of the SRM model decreases gradually as S increases, satisfying the dimensionless mathematical model and tending to be stable at S = 80 mm. The permeability of the SRM increases in a linear “zonal” distribution as F increases, and as S increases, the dispersion in the permeability value distribution decreases, particularly when F ≥ 12. The permeability of the SRM decreases gradually and then sharply as P increases, and it is important in the grading and structural composition of the SRM.
- (4)
- In the current study, the conditions of R = 60 ppi, center segmentation type, S = 80 mm, F ≥ 12, and P determined by specific need can be used to select and generate the optimal REV numerical calculation model size of the SRM.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tao, M.; Ren, Q.; Bian, H.; Cao, M.; Jia, Y. Mechanical Properties of Soil-Rock Mixture Filling in Fault Zone Based on Mesostructure. Comput. Model. Eng. Sci. 2022, 132, 681–705. [Google Scholar] [CrossRef]
- Gao, W.-W.; Gao, W.; Hu, R.-L.; Xu, P.-F.; Xia, J.-G. Microtremor survey and stability analysis of a soil-rock mixture landslide: A case study in Baidian town, China. Landslides 2018, 15, 1951–1961. [Google Scholar] [CrossRef]
- Liu, L.; Mao, X.; Xiao, Y.; Wang, T.; Nie, M. Influence of water and rock particle contents on the shear behaviour of a SRM. Transp. Saf. Environ. 2020, 2, 29–43. [Google Scholar] [CrossRef]
- Iqbal, J.; Thomasson, J.A.; Jenkins, J.N.; Owens, P.R.; Whisler, F.D. Spatial Variability Analysis of Soil Physical Properties of Alluvial Soils. Soil. Sci. Soc. Am. J. 2005, 69, 1338–1350. [Google Scholar] [CrossRef]
- Wang, Y.; Mao, X.; Wu, Q.; Dai, Z. Study on the Influence of Seepage Conditions with Different Rainfall Intensities on the Structural Evolution of Soil-Rock Mixture Filler. Geofluids 2022, 2022, 7694663. [Google Scholar] [CrossRef]
- Zhou, Y.; Sheng, G.; Qiao, S.; Zhou, L.; Cai, J.; Xu, H. A determination method for the shear strength of soil-rock mixture considering the size effect and its application. Front. Mater. 2022, 9, 1075310. [Google Scholar] [CrossRef]
- Ke, L.; Takahashi, A. Strength reduction of cohesionless soil due to internal erosion induced by one-dimensional upward seepage flow. Soils Found. 2012, 52, 698–711. [Google Scholar] [CrossRef]
- Rong, G.; Zhou, C.B.; Wang, E.Z. Preliminary study on permeability tensor calcution of fractured rock mass and its representative elementary volume. J. Rock Mech. Eng. 2007, 26, 740–746. [Google Scholar]
- Chen, J.; Fang, Y.; Gu, R.; Shu, H.; Ba, L.; Li, W. Study on pore size effect of low permeability clay seepage. Arab. J. Geosci. 2019, 12, 238. [Google Scholar] [CrossRef]
- Wang, L. Two-Dimensional Numerical Experiment Based on the Permeability of Soil-Rock Mixture Microstructure and Its Size Effect. Master’s Thesis, China Three Gorges University, Sanxia, China, 2020. [Google Scholar] [CrossRef]
- Liu, Q.; Li, H.; Zhang, Y.B. Scale effect in permeability characteristics of rock mass using boundary element method. J. Univ. Jinan 2015, 29, 210–215. [Google Scholar] [CrossRef]
- Xu, W.J. Study on Meso-Structural Mechanics of Soil-Rock Mixture and Its Slope Stability. Ph.D. Thesis, Institute of Geology and Geophysics, Chinese Academy of Sciences, Beijing, China, 2008. Available online: http://ir.iggcas.ac.cn/handle/132A11/11061 (accessed on 5 January 2023).
- McNamara, G.R.; Zanetti, G. Use of the Boltzmann Equation to Simulate Lattice-Gas Automata. Phys. Rev. Lett. 1988, 61, 2332–2335. [Google Scholar] [CrossRef] [PubMed]
- Zhou, D.; Tan, Z. On the Application of the Lattice Boltzmann Method to Predict Soil Meso Seepage Characteristics. Fluid. Dyn. Mater. Process. 2020, 16, 903–917. [Google Scholar] [CrossRef]
- Kim, P.; Kim, Y.G.; Paek, C.-H.; Ma, J. Lattice Boltzmann method for consolidation analysis of saturated clay. J. Ocean. Eng. Sci. 2019, 4, 193–202. [Google Scholar] [CrossRef]
- He, Y.L.; Wang, Y.; Li, Q. Lattice Boltzmann Method: Theory and Application; Science Press: Beijing, China, 2009; Available online: https://onlinetoolsland.com/books/3352346 (accessed on 9 April 2023).
- Li, J.J.; Jin, L.; Chen, T. Numerical Simulation of Mesoscopic Seepage Field of Soil-rock Mixture Based on Lattice Boltzmann Method. Sci. Technol. Eng. 2019, 19, 235–241. [Google Scholar]
- Cai, P.C.; Que, Y.; Jiang, Z.L.; Yang, P.F. Lattice Boltzmann meso-seepage research of reconstructed soil based on the quartet structure generation set. Hydrogeol. Eng. Geol. 2022, 49, 33–42. [Google Scholar] [CrossRef]
- Jin, L.; Zeng, Y.W.; Cheng, T.; Li, J.J. Seepage characteristics of soil-rock mixture based on lattice Boltzmann method. Chin. J. Geotech. Eng. 2022, 44, 669–677. [Google Scholar] [CrossRef]
- Gao, J.; Xing, H.; Tian, Z.; Muhlhaus, H. Lattice Boltzmann modeling and evaluation of fluid flow in heterogeneous porous media involving multiple matrix constituents. Comput. Geosci. 2014, 62, 198–207. [Google Scholar] [CrossRef]
- Chen, T.; Yang, Y.; Zheng, H.; Wu, Z. Numerical determination of the effective permeability coefficient of soil–rock mixtures using the numerical manifold method. Int. J. Numer. Anal. Methods Géoméch. 2018, 43, 381–414. [Google Scholar] [CrossRef]
- Wang, T.; Yan, C.; Zheng, Y.; Jiao, Y.-Y.; Zou, J. Numerical study on the effect of meso-structure on hydraulic conductivity of soil-rock mixtures. Comput. Geotech. 2022, 146, 104726. [Google Scholar] [CrossRef]
- You, X.H. Stochastic structural model of soil rock mixture and its application. Chin. J. Rock Mech. Eng. 2002, 11, 1748. Available online: https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200211040.htm (accessed on 7 January 2023).
- Ding, X.; Shi, X.; Zhou, W.; Luan, B. Experimental Study on the Permeability of a Soil-Rock Mixture Based on the Threshold Control Method. Adv. Civ. Eng. 2019, 2019, 8987052. [Google Scholar] [CrossRef]
- Feng, Y.T.; Han, K.; Owen, D.R.J. Coupled lattice Boltzmann method and discrete element modelling of particle transport in turbulent fluid flows: Computational issues. Int. J. Numer. Methods Eng. 2007, 72, 1111–1134. [Google Scholar] [CrossRef]
- Succi, S. Lattice Boltzmann Equation for Fluid Dynamics and Beyond; Clarendon Press: Oxford, UK, 2001. [Google Scholar]
- Medley, E. The Engineering Characterization of Melanges and Similar Block-In-Matrix Rocks (Bimrocks). Ph.D. Thesis, University of California at Berkeley, San Francisco, CA, USA, 1994. Available online: https://www.researchgate.net/publication/35292215 (accessed on 15 January 2023).
- Yin, P.; Song, H.; Ma, H.; Yang, W.; He, Z.; Zhu, X. The modification of the Kozeny-Carman equation through the lattice Boltzmann simulation and experimental verification. J. Hydrol. 2022, 609, 127738. [Google Scholar] [CrossRef]
- Hager, A.; Kloss, C.; Pirker, S.; Goniva, C. Parallel Resolved Open Source CFD-DEM: Method, Validation and Application. J. Comput. Multiph. Flows 2014, 6, 13–27. [Google Scholar] [CrossRef]
- GB/T 50123-2019; Standard for Geotechnical Testing Method. Ministry of Water Resources of the People’s Republic of China, Ministry of Housing and Urban-Rural Development of the People’s Republic of China, State Administration of Market Supervision and Administration: Beijing, China, 2019. Available online: https://www.gb-gbt.cn/PDF.aspx/GBT50123-2019 (accessed on 25 January 2023).
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock. Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Yang, B.; Jiao, Y.; Lei, S. A study on the effects of microparameters on macroproperties for specimens created by bonded particles. Eng. Comput. 2006, 23, 607–631. [Google Scholar] [CrossRef]
- Schöpfer, M.P.; Abe, S.; Childs, C.; Walsh, J.J. The impact of porosity and crack density on the elasticity, strength and friction of cohesive granular materials: Insights from DEM modelling. Int. J. Rock. Mech. Min. Sci. 2008, 46, 250–261. [Google Scholar] [CrossRef]
- Jin, L.; Cheng, T.; Zhang, Y.; Li, J. Three-dimensional lattice Boltzmann simulation of the permeability of soil-rock mixtures and comparison with other prediction models. Int. J. Numer. Anal. Methods Géoméch. 2021, 45, 1067–1090. [Google Scholar] [CrossRef]
- Sheikh, B.; Pak, A. Numerical investigation of the effects of porosity and tortuosity on soil permeability using coupled three-dimensional discrete-element method and lattice Boltzmann method. Phys. Rev. E 2015, 91, 053301. [Google Scholar] [CrossRef]
- Wang, P.F.; Li, C.H.; Ma, X.W.; Li, Z.J.; Liu, J.J.; Wu, Y.F. Experimental study of seepage characteristics of soil-rock mixture with different rock contents in fault zone. Rock Soil Mech. 2018, 39, 53–61. [Google Scholar] [CrossRef]
- Chen, Z.H.; Chen, S.J.; Chen, J.; Sheng, Q.; Min, H.; Hu, W. In-situ Double-Ring Infiltration Test of Soil-Rock Mixture. J. Yangtze River Sci. Res. Inst. 2012, 29, 52–56. Available online: http://119.78.100.198/handle/2S6PX9GI/14025 (accessed on 8 April 2023).
- Zhou, Z.; Fu, H.L.; Liu, B.C.; Tan, H.H.; Long, W.X. Orthogonal tests on permeability of soil-rock-mixture. Chin. J. Geotech. Eng. 2006, 28, 1134–1138. [Google Scholar]
- Bear, J. Dynamics of Fluids in Porous Media; Elsevier: New York, NY, USA, 1972. [Google Scholar] [CrossRef]
- Zhou, Z.; Sun, J.; Lai, Y.; Wei, C.; Hou, J.; Bai, S.; Huang, X.; Liu, H.; Xiong, K.; Cheng, S. Study on size effect of jointed rock mass and influencing factors of the REV size based on the SRM method. Tunn. Undergr. Space Technol. 2022, 127, 104613. [Google Scholar] [CrossRef]
- Walsh, S.D.; Burwinkle, H.; Saar, M.O. A new partial-bounceback lattice-Boltzmann method for fluid flow through heterogeneous media. Comput. Geosci. 2009, 35, 1186–1193. [Google Scholar] [CrossRef]
- Lautenschlaeger, M.P.; Weinmiller, J.; Kellers, B.; Danner, T.; Latz, A. Homogenized lattice Boltzmann model for simulating multi-phase flows in heterogeneous porous media. Adv. Water Resour. 2022, 170, 104320. [Google Scholar] [CrossRef]



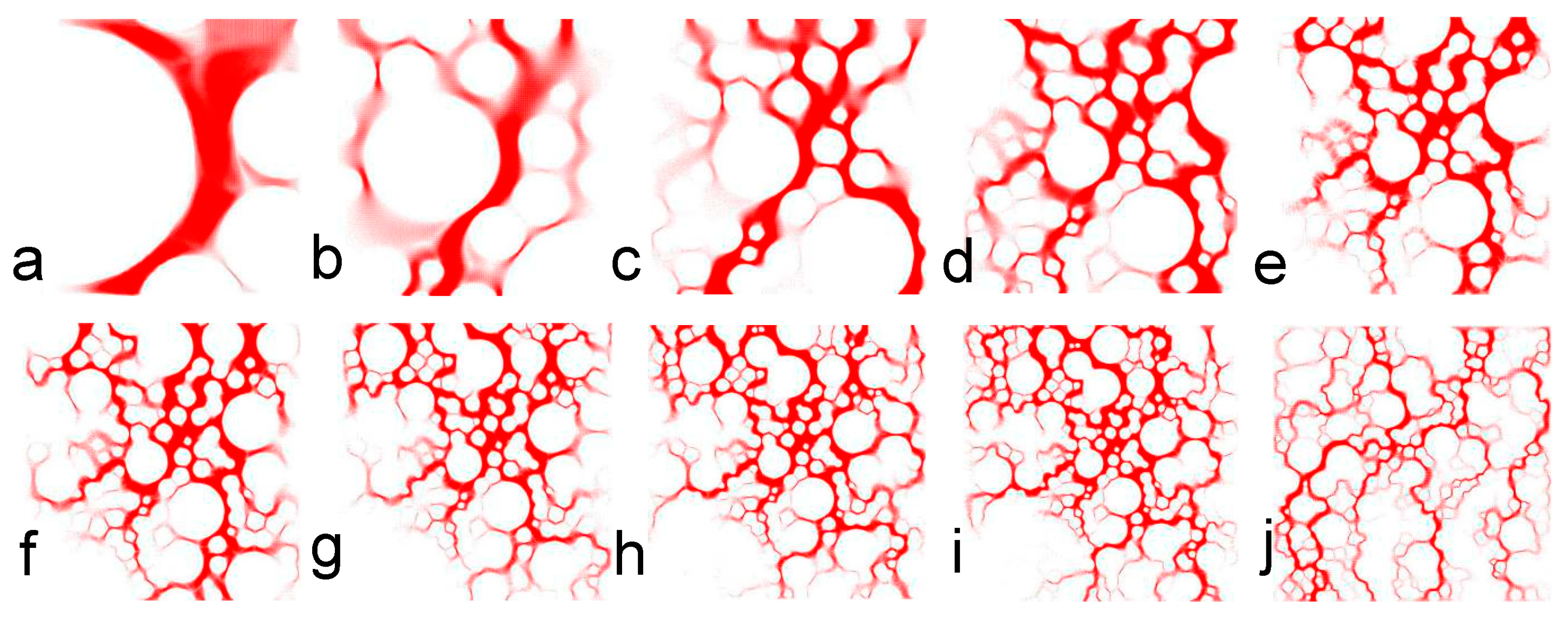

| Projects | Model | Segmentation Type | Basic Information | S (mm) | Number of Test Conditions |
|---|---|---|---|---|---|
| Resolution | SRM–1/SRM–2/SRM–3 | – | r–10, r–20, r–30, r–40, r–50 r–60, r–70, r–80, r–90, r–100 | 100 | 30 |
| Segmentation type | SRM–1/SRM–2/SRM–3 | Random/Center/Top/Equal | sj–25/jz–25/dd–25/df–25 | 25 | 225 |
| sj–50/jz–50/dd–50/df–50 | 50 | ||||
| sj–75/jz–75/dd–75/df–75 | 75 | ||||
| sj–100/jz–100/dd–100/df–100 | 100 | ||||
| Model feature size | SRM–1/SRM–2/SRM–3 | Center | jz–10, jz–20, jz–30, jz–40, jz–50, jz–60, jz–70, jz–80, jz–90, jz–100 | 10, 20, 30, 40, 50, 60, 70, 80, 90, 100 | 30 |
| Feature length ratio | SRM–1/SRM–2/SRM–3 SRM–add | Center | F = 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20 | 50, 80, 100 | 48 |
| Soil/rock particle size feature ratio | SRM–1/SRM–2/SRM–3 SRM–add | Center | P = 0.1, 0.2, 0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9 | 80 | 9 |
| l (mm) | b (mm) | t (s) | μ (Pa·s) | ρ (kg·m−3) | T (°C) | Δp (Pa) |
|---|---|---|---|---|---|---|
| 50 | 25 | 1.65×10−3 | 1.01×10−3 | 1000 | 20.0 | 3.67 × 10−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, P.; Mao, X.; Lou, K.; Yun, Z. Lattice Boltzmann Numerical Study on Mesoscopic Seepage Characteristics of Soil–Rock Mixture Considering Size Effect. Mathematics 2023, 11, 1968. https://doi.org/10.3390/math11081968
Cai P, Mao X, Lou K, Yun Z. Lattice Boltzmann Numerical Study on Mesoscopic Seepage Characteristics of Soil–Rock Mixture Considering Size Effect. Mathematics. 2023; 11(8):1968. https://doi.org/10.3390/math11081968
Chicago/Turabian StyleCai, Peichen, Xuesong Mao, Ke Lou, and Zhihui Yun. 2023. "Lattice Boltzmann Numerical Study on Mesoscopic Seepage Characteristics of Soil–Rock Mixture Considering Size Effect" Mathematics 11, no. 8: 1968. https://doi.org/10.3390/math11081968
APA StyleCai, P., Mao, X., Lou, K., & Yun, Z. (2023). Lattice Boltzmann Numerical Study on Mesoscopic Seepage Characteristics of Soil–Rock Mixture Considering Size Effect. Mathematics, 11(8), 1968. https://doi.org/10.3390/math11081968







