Optimal Investment Consumption Choices under Mispricing and Habit Formation
Abstract
:1. Introduction
2. Model Formulation
2.1. The Financial Market
2.2. Consumption Habits and Wealth Process
2.3. Optimization Problem
3. Solution of the Optimization Problems
3.1. Optimal Consumption and Investment Strategies
3.2. Special Cases
3.2.1. No Mispricing
3.2.2. Delta-Neutral Arbitrage Strategy
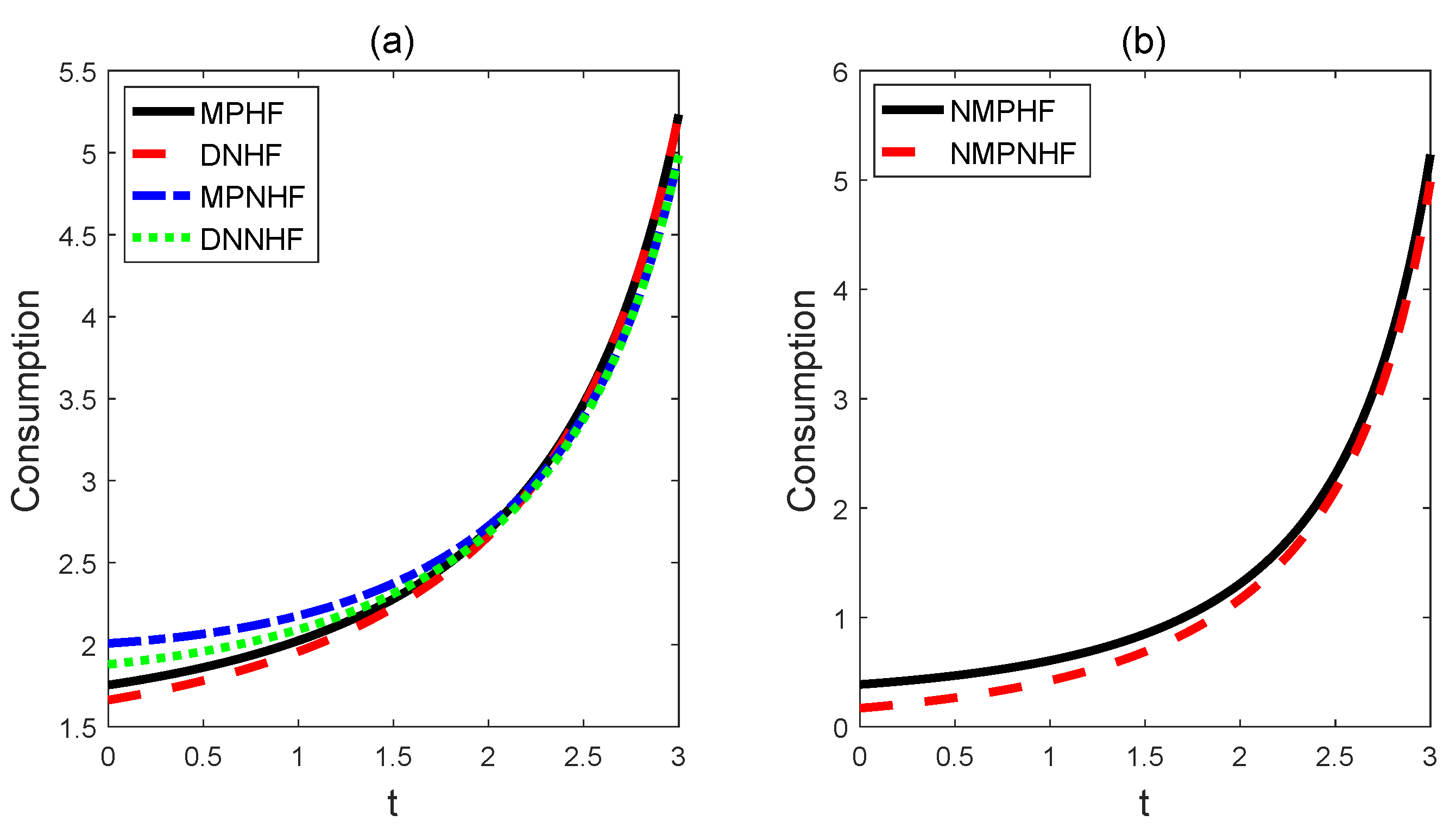
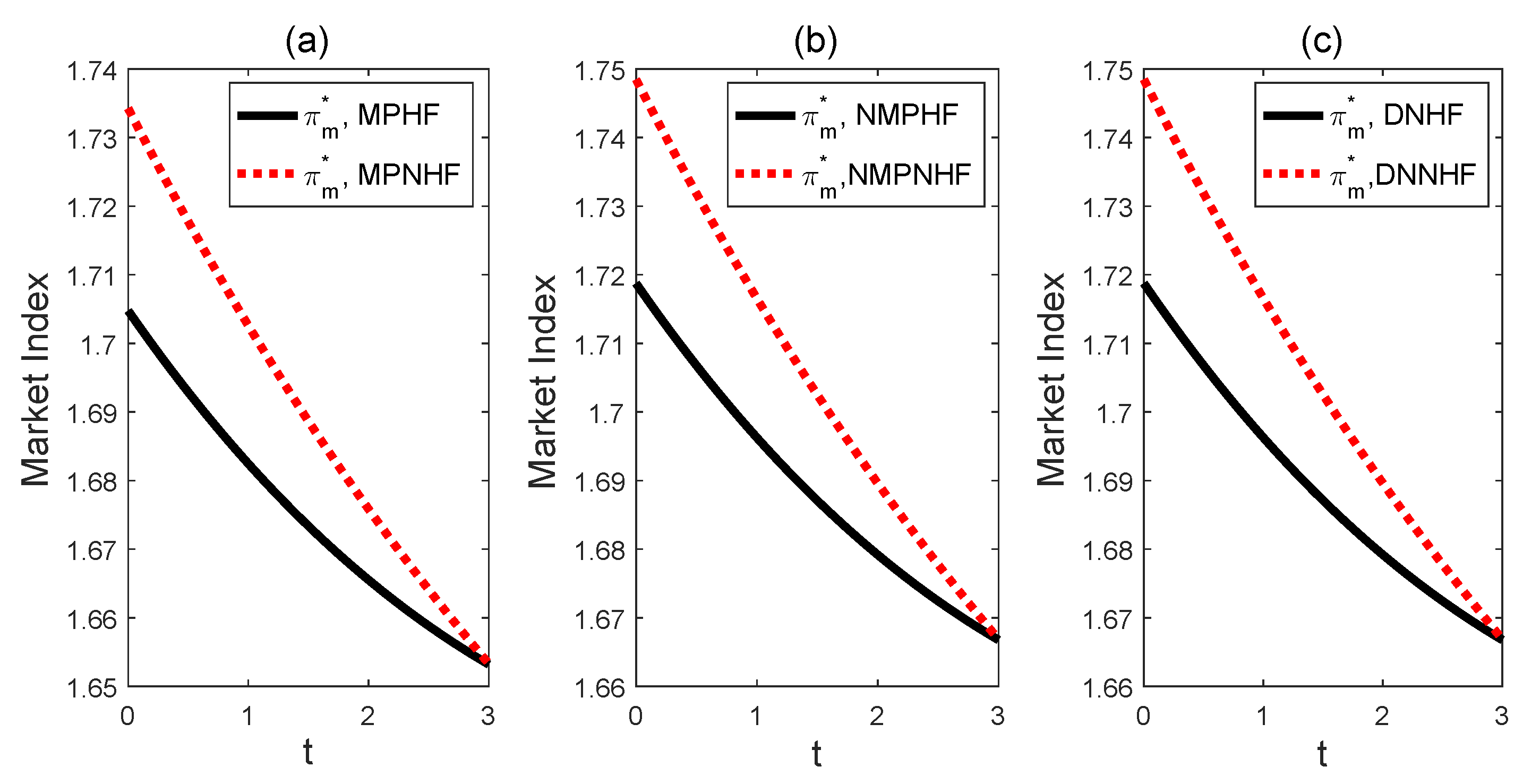
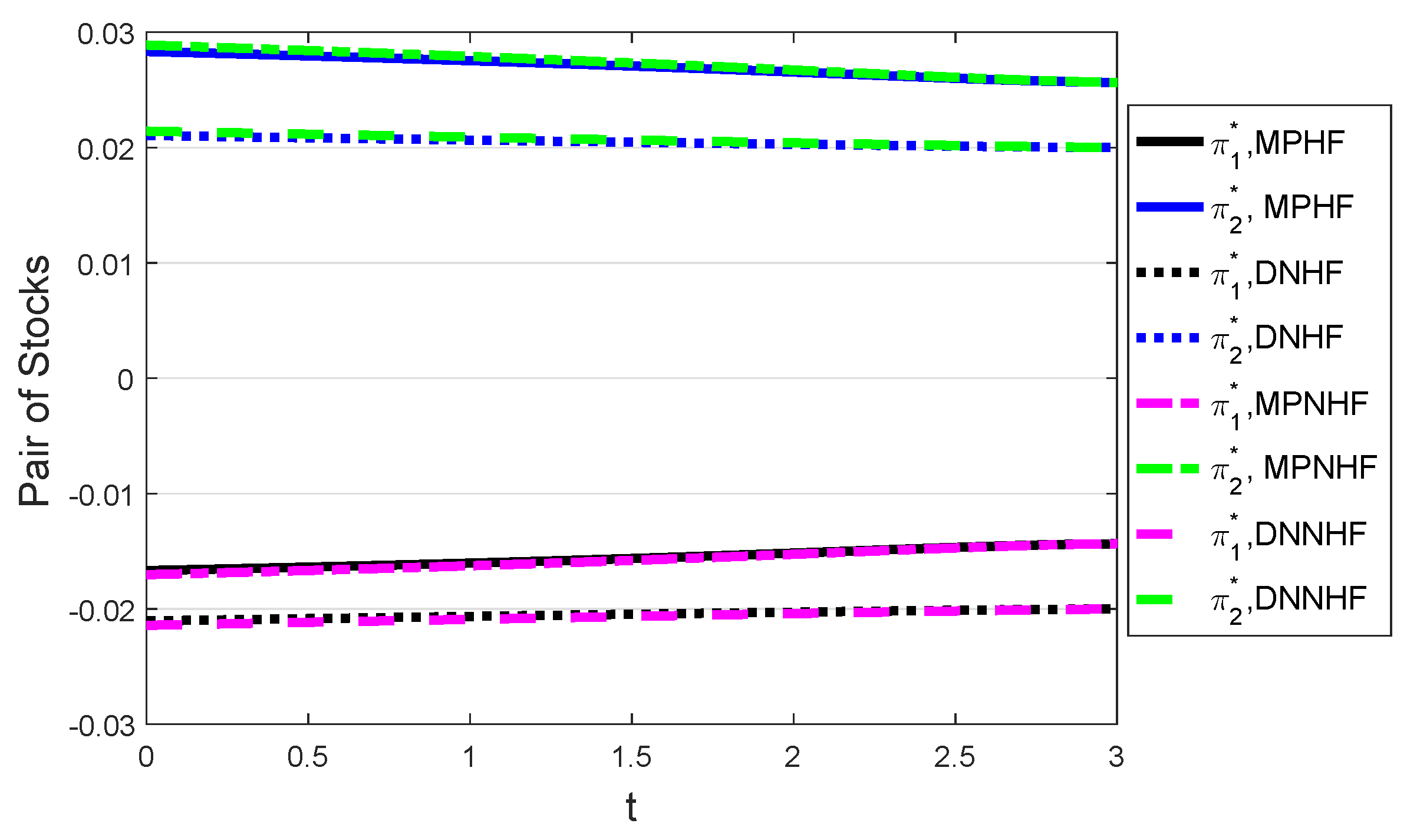
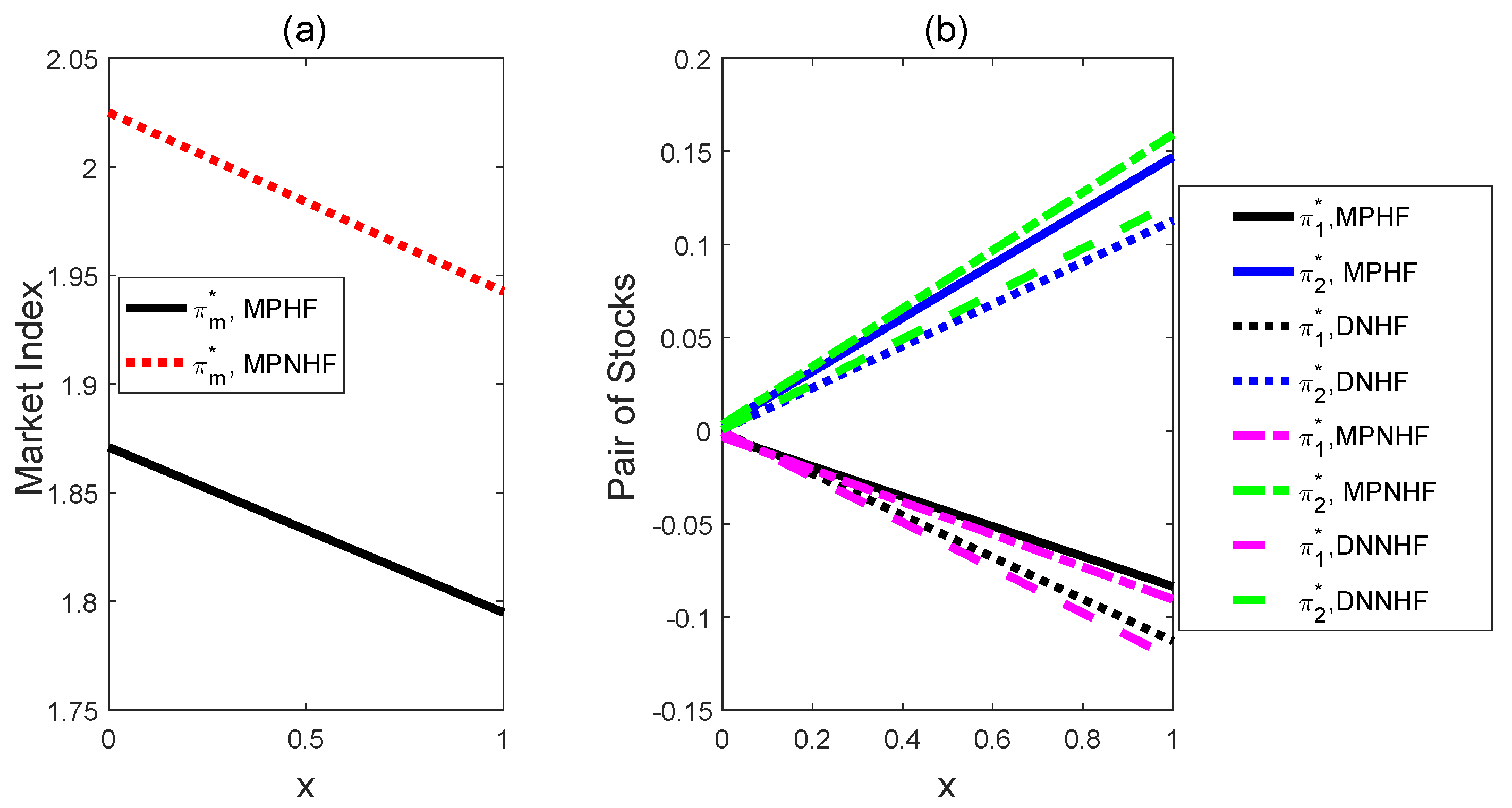
4. Numerical Illustrations
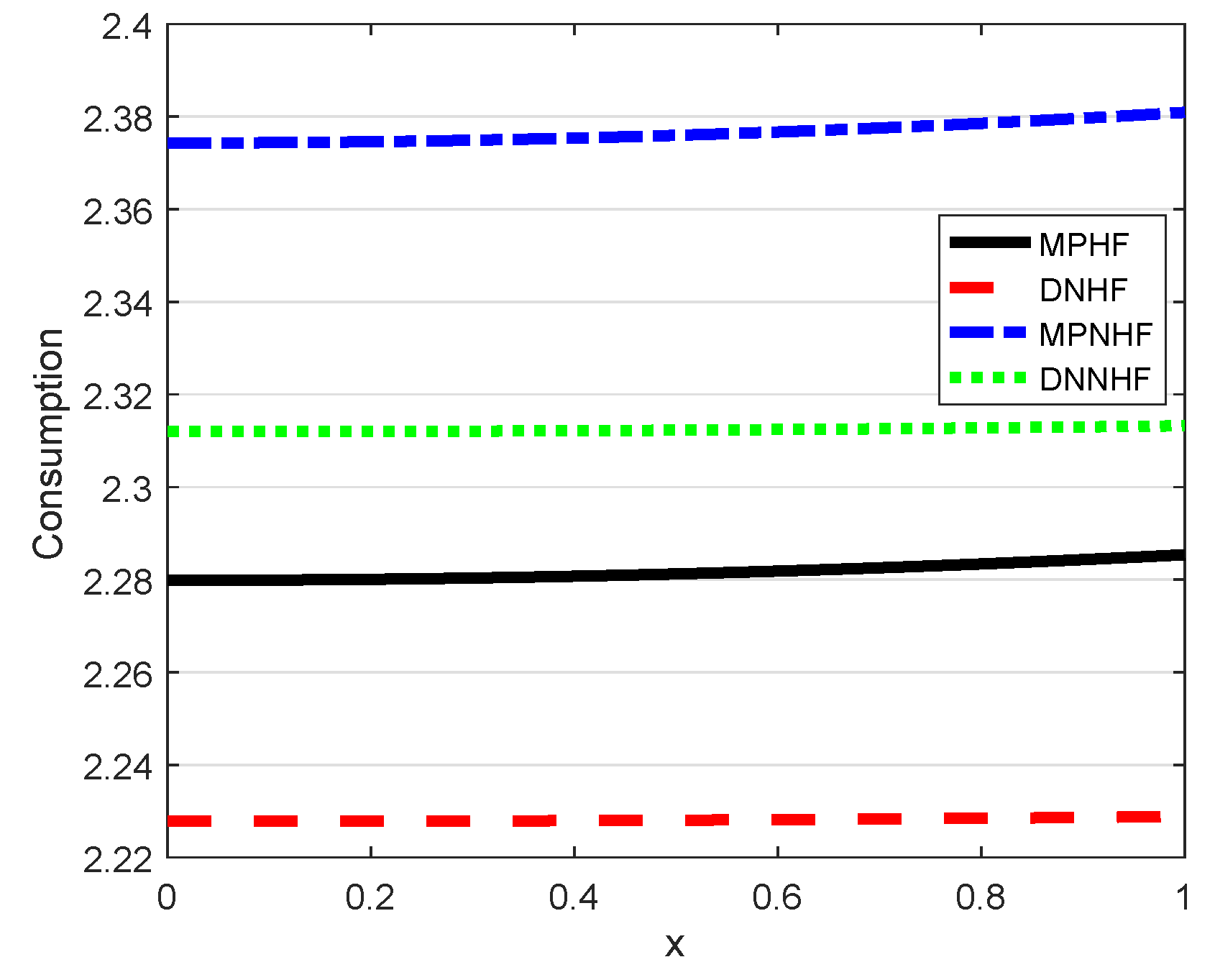
4.1. The Effects on Consumption and Investment Strategies
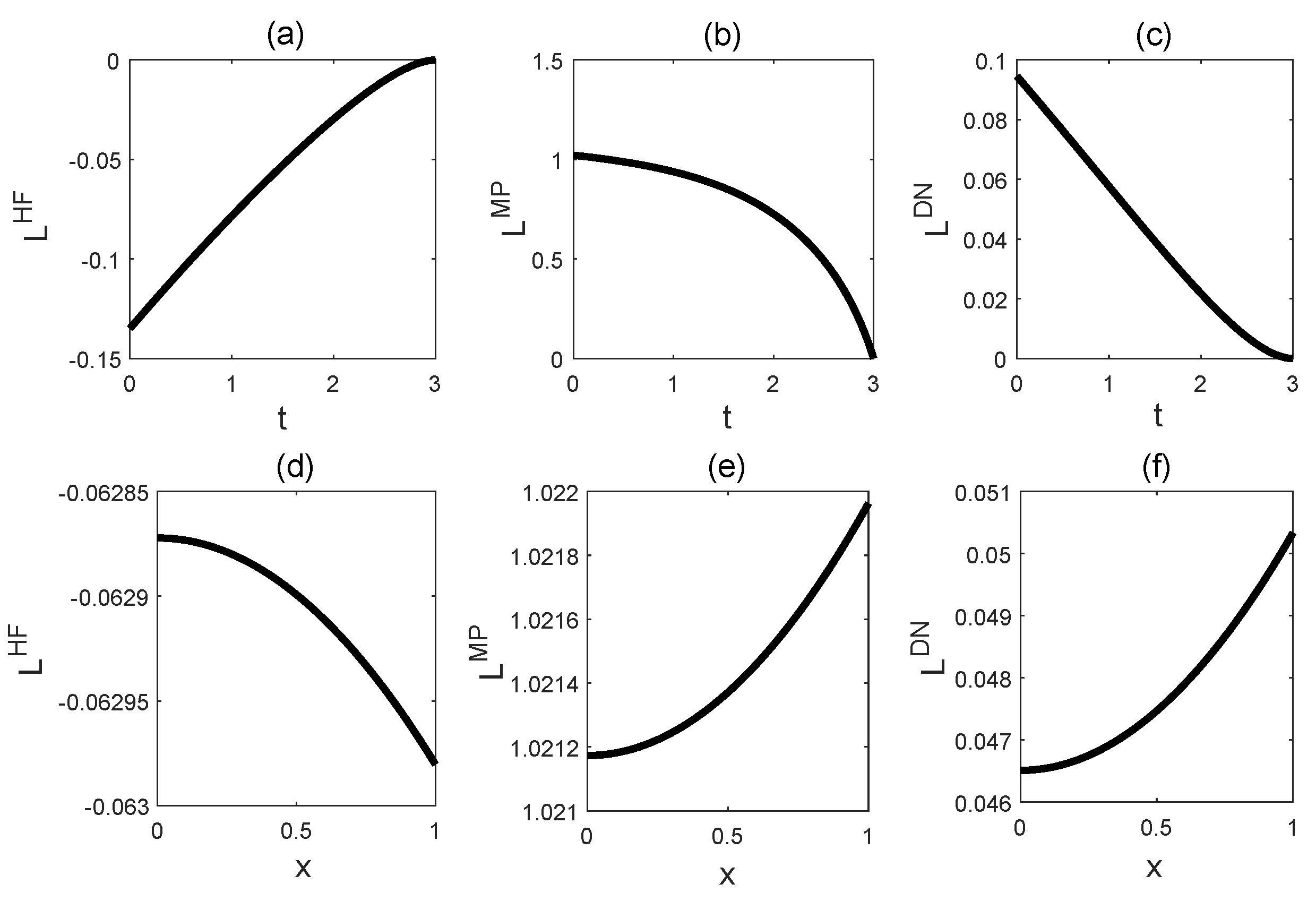
4.2. Wealth-Equivalent Utility Loss
5. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Merton, R.C. Lifetime portfolio selection under uncertainty: The continuous-time case. Rev. Econ. Stat. 1969, 51, 247–257. [Google Scholar] [CrossRef]
- Bellalah, M.; Zhang, D.; Zhang, P. An optimal portfolio and consumption problem with a benchmark and partial information. Math. Financ. Econ. 2023, 17, 127–152. [Google Scholar] [CrossRef]
- Cocco, J.F.; Gomes, F.J.; Maenhout, P.J. Consumption and portfolio choice over the life cycle. Rev. Financ. Stud. 2005, 18, 491–533. [Google Scholar] [CrossRef]
- Bilsen, S.V.; Laeven, R.J. Dynamic consumption and portfolio choice under prospect theory. Insur. Math. Econ. 2020, 91, 224–237. [Google Scholar] [CrossRef]
- Lichtenstern, A.; Shevchenko, P.V.; Zagst, R. Optimal life-cycle consumption and investment decisions under age-dependent risk preferences. Math. Financ. Econ. 2021, 15, 275–313. [Google Scholar] [CrossRef]
- Gu, A.; Viens, F.G.; Bo, Y. Optimal reinsurance and investment strategies for insurers with mispricing and model ambiguity. Insur. Math. Econ. 2017, 72, 235–249. [Google Scholar] [CrossRef]
- Liu, J.; Longstaff, F. 2004. Losing money on arbitrage: Optimal dynamic portfolio choice in markets with arbitrage opportunities. Rev. Financ. Stud. 2004, 17, 611–641. [Google Scholar] [CrossRef]
- Liu, J.; Timmermann, A. Optimal convergence trade strategies. Rev. Financ. Stud. 2013, 26, 1048–1086. [Google Scholar] [CrossRef]
- Yi, B.; Viens, F.; Law, B.; Li, Z. Dnynamic portfolio selection with mispricing and model ambiguity. Ann. Financ. 2015, 11, 37–75. [Google Scholar] [CrossRef]
- Gu, A.; Viens, F.G.; Yao, H. Optimal robust reinsurance-investment strategies for insurers with mean reversion and mispricing. Insur. Math. Econ. 2018, 80, 93–109. [Google Scholar] [CrossRef]
- Constantinides, G.M. Habit formation: A resolution of the equity premium puzzle. J. Political Econ. 1990, 98, 519–543. [Google Scholar] [CrossRef]
- Browning, M.; Collado, D. Habits and heterogeneity in demands: A panel data analysis. J. Appl. Econom. 2007, 22, 625–640. [Google Scholar] [CrossRef]
- Munk, C. Portfolio and consumption choice with stochastic investment opportunities and habit formation in preferences. J. Econ. Dyn. Control 2008, 32, 3560–3589. [Google Scholar] [CrossRef]
- Li, W.; Tan, K.S.; Wei, P. Demand for non-life insurance under habit formation. Insur. Math. Econ. 2020, 101, 38–54. [Google Scholar] [CrossRef]
- Shi, A.; Li, X.; Li, Z. Optimal portfolio selection with life insurance under subjective survival belief and habit formation. J. Ind. Manag. Optim. 2023, 19, 2464–2484. [Google Scholar] [CrossRef]
- Detemple, J.B.; Zapatero, F. Asset prices in an exchange economy with habit formation. Econometrica 1991, 59, 1633–1657. [Google Scholar] [CrossRef]
- Kraft, H.; Munk, C.; Wagner, S. Housing habits and their implications for life-cycle consumption and investment. Rev. Financ. 2018, 22, 1737–1762. [Google Scholar] [CrossRef]
- Kraft, H.; Munk, C. Optimal housing, consumption, and investment decisions over the life cycle. Manag. Sci. 2011, 57, 1025–1041. [Google Scholar] [CrossRef]
- Branger, N.; Larsen, L.S.; Munk, C. Robust portfolio choice with ambiguity and learning about return predictability. J. Bank. Financ. 2013, 37, 1397–1411. [Google Scholar] [CrossRef]

| r | b | ||||||||
| 0.03 | 0.05 | 1.2 | 0.1 | 0.3 | 1 | 0.2 | 0.4 | 3 | 0.2 |
| T | |||||||||
| 0.1 | 0.174 | 0.07 | 20 | 0.5 | 0.253 | 4 | 0.2 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, A.; Sun, J.; Liu, B. Optimal Investment Consumption Choices under Mispricing and Habit Formation. Mathematics 2024, 12, 2248. https://doi.org/10.3390/math12142248
Shi A, Sun J, Liu B. Optimal Investment Consumption Choices under Mispricing and Habit Formation. Mathematics. 2024; 12(14):2248. https://doi.org/10.3390/math12142248
Chicago/Turabian StyleShi, Ailing, Jingyun Sun, and Botao Liu. 2024. "Optimal Investment Consumption Choices under Mispricing and Habit Formation" Mathematics 12, no. 14: 2248. https://doi.org/10.3390/math12142248





