One-Bit Function Perturbation Impact on Robust Set Stability of Boolean Networks with Disturbances
Abstract
:1. Introduction
2. Preliminaries
2.1. Semi-Tensor Product of Matrices
2.2. Algebraic Representations of BNs with Disturbances
3. Main Results
- (i)
- ,
- (ii)
- , .
- (i)
- , which implies that set is reachable from and there exists no path from to set containing , simultaneously.
- (ii)
- , which implies that set is reachable from and there exists at least one path from to set containing , simultaneously.
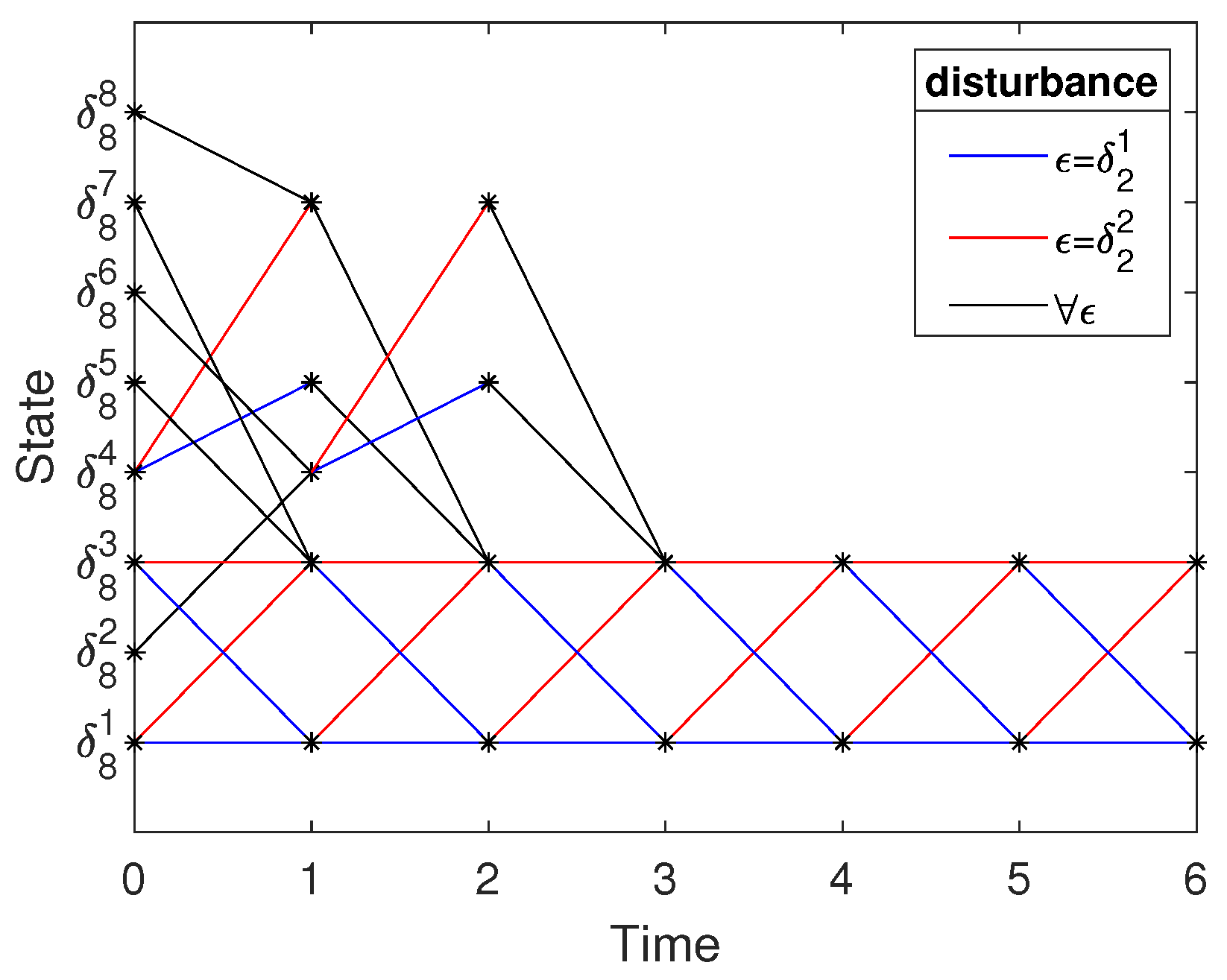
4. Illustrative Example
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kauffman, S.A. Metabolic stability and epigenesis in randomly constructed genetic nets. J. Theor. Biol. 1969, 22, 437–467. [Google Scholar] [CrossRef] [PubMed]
- Nazi, A.; Raj, M.; Di Francesco, M.; Ghosh, P.; Das, S.K. Deployment of robust wireless sensor networks using gene regulatory networks: An isomorphism-based approach. Pervasive Mob. Comput. 2014, 13, 246–257. [Google Scholar] [CrossRef]
- Wang, L.; Pichler, E.E.; Ross, J. Oscillations and chaos in neural networks: An exactly solvable model. Proc. Natl. Acad. Sci. USA 1990, 87, 9467–9471. [Google Scholar] [CrossRef] [PubMed]
- Guo, P.; Wang, Y.; Li, H. Algebraic formulation and strategy optimization for a class of evolutionary networked games via semi-tensor product method. Automatica 2013, 49, 3384–3389. [Google Scholar] [CrossRef]
- Li, H.; Wang, S.; Li, X.; Zhao, G. Perturbation analysis for controllability of logical control networks. SIAM J. Control Optim. 2020, 58, 3632–3657. [Google Scholar] [CrossRef]
- Zhong, J.; Yu, Z.; Li, Y.; Lu, J. State estimation for probabilistic Boolean networks via outputs observation. IEEE Trans. Neural Netw. Learn. Syst. 2021, 33, 4699–4711. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Feng, G.; Cao, J. Robust stochastic stability analysis of genetic regulatory networks with disturbance attenuation. Neurocomputing 2012, 79, 39–49. [Google Scholar] [CrossRef]
- KR, A.G.; Mahapatra, S.; Ranjan Mahapatro, S. Design of a decentralized control law for variable area coupled tank systems using H∞ complimentary sensitivity function. Asian J. Control 2024, 26, 1540–1552. [Google Scholar]
- Sun, Q.; Li, H.; Gao, H. Lebesgue sampling approach to robust stabilization of Boolean control networks with external disturbances. J. Frankl. Inst. 2023, 360, 2794–2810. [Google Scholar] [CrossRef]
- Li, H.; Xie, L.; Wang, Y. On robust control invariance of Boolean control networks. Automatica 2016, 68, 392–396. [Google Scholar] [CrossRef]
- Achu Govind, K.; Mahapatra, S.; Mahapatro, S.R. Enhanced decentralised fractional-order control using tree seed optimisation and singular value analysis. Int. J. Syst. Sci. 2024, 55, 1437–1464. [Google Scholar] [CrossRef]
- Li, L.; Zhang, A.; Lu, J. Robust set stability of probabilistic Boolean networks under general stochastic function perturbation. Inf. Sci. 2022, 582, 833–849. [Google Scholar] [CrossRef]
- Cheng, D.; Qi, H.; Li, Z. Analysis and Control of Boolean Networks; Springer: London, UK, 2011. [Google Scholar]
- Zhong, J.; Ho, D.W.; Lu, J.; Xu, W. Global robust stability and stabilization of Boolean network with disturbances. Automatica 2017, 84, 142–148. [Google Scholar] [CrossRef]
- Wang, J.; Liu, W.; Fu, S.; Xia, J. On robust set stability and set stabilization of probabilistic Boolean control networks. Appl. Math. Comput. 2022, 422, 126992. [Google Scholar] [CrossRef]
- Li, H.; Yang, X.; Wang, S. Robustness for stability and stabilization of Boolean networks with stochastic function perturbations. IEEE Trans. Autom. Control 2020, 66, 1231–1237. [Google Scholar] [CrossRef]
- Li, F.; Tang, Y. Pinning controllability for a Boolean network with arbitrary disturbance inputs. IEEE Trans. Cybern. 2019, 51, 3338–3347. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Feng, J. Detectability of Boolean networks with disturbance inputs. Syst. Control Lett. 2020, 145, 104783. [Google Scholar] [CrossRef]
- Chen, H.; Liang, J. Local synchronization of interconnected Boolean networks with stochastic disturbances. IEEE Trans. Neural Netw. Learn. Syst. 2019, 31, 452–463. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhao, X.; Fu, S.; Xia, J. Robust Output Tracking of Boolean Control Networks over Finite Time. Mathematics 2022, 10, 4078. [Google Scholar] [CrossRef]
- Li, F.; Tang, Y. Set stabilization for switched Boolean control networks. Automatica 2017, 78, 223–230. [Google Scholar] [CrossRef]
- Wang, J.; Fu, S.; De Leone, R.; Xia, J.; Qiao, L. On robust control invariance and robust set stabilization of mix-valued logical control networks. Int. J. Robust Nonlinear Control 2022, 32, 10347–10357. [Google Scholar] [CrossRef]
- Fu, S.; Pan, Y.; Feng, J.; Zhao, J. Strategy optimisation for coupled evolutionary public good games with threshold. Int. J. Control 2022, 95, 562–571. [Google Scholar] [CrossRef]
- Chen, R.; Eshleman, J.; Brodsky, R.; Medof, M. Glycophosphatidylinositol-anchored protein deficiency as a marker of Mutator phenotypes in cancer. Cancer Res. 2001, 61, 654–658. [Google Scholar] [PubMed]
- Xiao, Y.; Dougherty, E.R. The impact of function perturbations in Boolean networks. Bioinformatics 2007, 23, 1265–1273. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Liu, Y.; Ruan, Q.; Lou, J. Robust stability of Switched Boolean Networks with function perturbation. Nonlinear Anal. Hybrid Syst. 2022, 46, 101216. [Google Scholar] [CrossRef]
- Deng, L.; Fu, S.; Wang, J.; Zhang, F. Function Perturbation Impact on Robust Stability and Stabilization of Boolean Networks with Disturbances. IEEE Access 2023, 11, 84514–84521. [Google Scholar] [CrossRef]
- Li, H.; Yang, X. Robust optimal control of logical control networks with function perturbation. Automatica 2023, 152, 110970. [Google Scholar] [CrossRef]
- Wang, S.; Li, H. Graph-based function perturbation analysis for observability of multivalued logical networks. IEEE Trans. Neural Netw. Learn. Syst. 2020, 32, 4839–4848. [Google Scholar] [CrossRef]
- Chen, Q.; Li, H. Robust weak detectability analysis of Boolean networks subject to function perturbation. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 5004–5008. [Google Scholar] [CrossRef]


| Notations | Definitions |
|---|---|
| the set of positive integers | |
| the set of real matrices | |
| the transpose of matrix A | |
| the i-th column of matrix A | |
| the set of columns of matrix A | |
| the set of logical matrices | |
| the set of Boolean matrices | |
| -element of matrix A |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, L.; Cao, X.; Zhao, J. One-Bit Function Perturbation Impact on Robust Set Stability of Boolean Networks with Disturbances. Mathematics 2024, 12, 2258. https://doi.org/10.3390/math12142258
Deng L, Cao X, Zhao J. One-Bit Function Perturbation Impact on Robust Set Stability of Boolean Networks with Disturbances. Mathematics. 2024; 12(14):2258. https://doi.org/10.3390/math12142258
Chicago/Turabian StyleDeng, Lei, Xiujun Cao, and Jianli Zhao. 2024. "One-Bit Function Perturbation Impact on Robust Set Stability of Boolean Networks with Disturbances" Mathematics 12, no. 14: 2258. https://doi.org/10.3390/math12142258
APA StyleDeng, L., Cao, X., & Zhao, J. (2024). One-Bit Function Perturbation Impact on Robust Set Stability of Boolean Networks with Disturbances. Mathematics, 12(14), 2258. https://doi.org/10.3390/math12142258




