Transverse Compression of a Thin Inhomogeneous Elastic Layer
Abstract
:1. Introduction
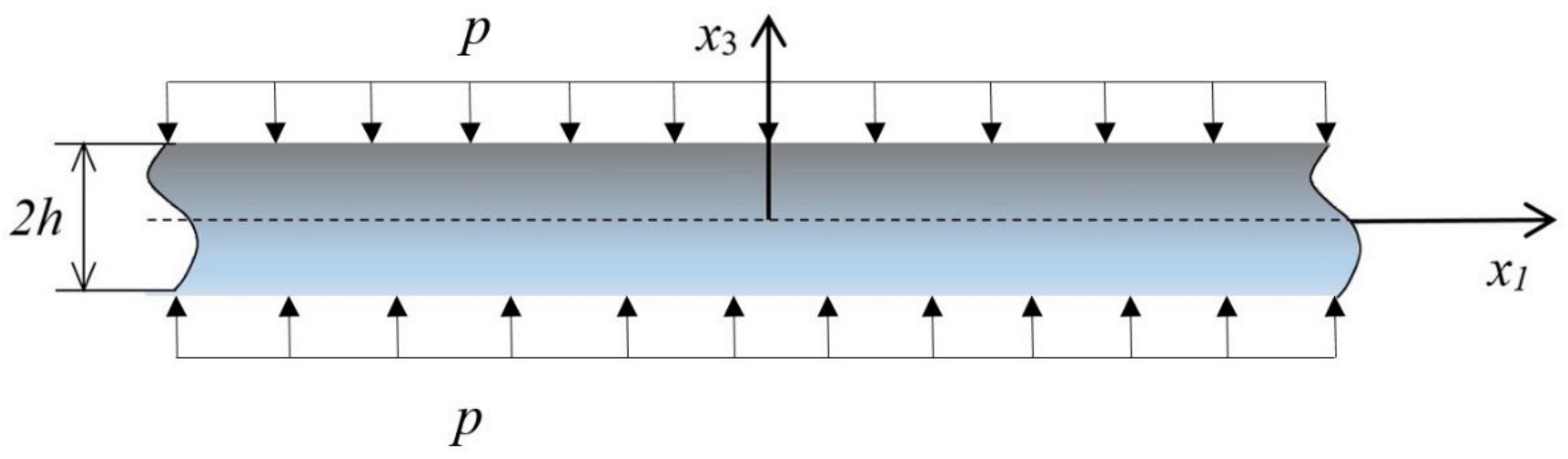
2. Statement of the Problem
3. Asymptotic Solution
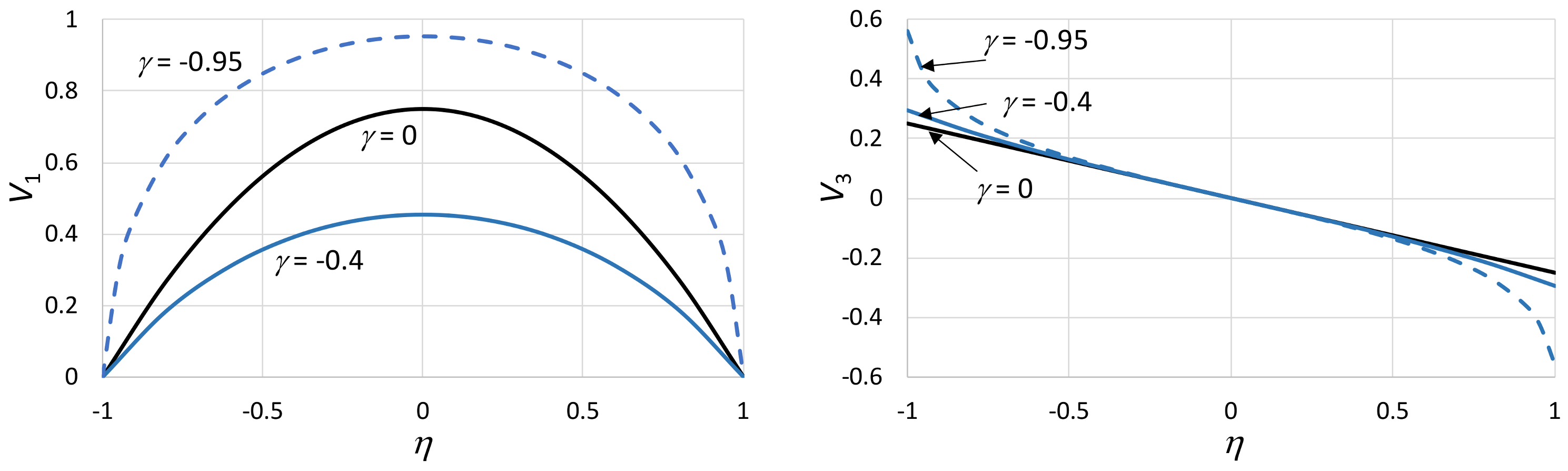
4. Particular Cases
5. Prescribed Transverse Displacements
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chalhoub, M.S.; Kelly, J.M. Effect of bulk compressibility on the stiffness of cylindrical base isolation bearings. Int. J. Solids Struct. 1990, 26, 743–760. [Google Scholar] [CrossRef]
- Gent, A.; Lindley, P. The compression of bonded rubber blocks. Proc. Inst. Mech. Eng. 1959, 173, 111–122. [Google Scholar] [CrossRef]
- Koh, C.G.; Lim, H.L. Analytical solution for compression stiffness of bonded rectangular layers. Int. J. Solids Struct. 2001, 38, 445–455. [Google Scholar] [CrossRef]
- Pinarbasi, S.; Akyuz, U.; Mengi, Y. A new formulation for the analysis of elastic layers bonded to rigid surfaces. Int. J. Solids Struct. 2006, 43, 4271–4296. [Google Scholar] [CrossRef]
- Pinarbasi, S.; Mengi, Y.; Akyuz, U. Compression of solid and annular circular discs bonded to rigid surfaces. Int. J. Solids Struct. 2008, 45, 4543–4561. [Google Scholar] [CrossRef]
- Tsai, H.C.; Lee, C.C. Compressive stiffness of elastic layers bonded between rigid plates. Int. J. Solids Struct. 1998, 35, 3053–3069. [Google Scholar] [CrossRef]
- Tsai, H.C. Compression behavior of annular elastic layers bonded between rigid plates. J. Mech. 2012, 1, 1–7. [Google Scholar] [CrossRef]
- Brady, B.T. An exact solution to the radially end-constrained circular cylinder under triaxial loading. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1971, 8, 165–178. [Google Scholar] [CrossRef]
- Qiao, S.; Lu, N. Analytical solutions for bonded elastically compressible layers. Int. J. Solids Struct. 2015, 58, 353–365. [Google Scholar] [CrossRef]
- Alzaidi, A.S.M.; Kaplunov, J.; Nikonov, A.; Zupančič, B. Transverse compression of a thin elastic disc. Z. Angew. Math. Phys. 2024, 75, 116. [Google Scholar] [CrossRef]
- Miyamoto, Y.; Kaysser, W.A.; Rabin, B.H.; Kawasaki, A.; Ford, R.G. (Eds.) Functionally Graded Materials: Design, Processing and Applications; Springer Science+Business Media: New York, NY, USA, 2013. [Google Scholar]
- Gupta, A.; Talha, M. Recent development in modeling and analysis of functionally graded materials and structures. Prog. Aerosp. Sci. 2015, 79, 1–14. [Google Scholar] [CrossRef]
- Adıyaman, G.; Öner, E.; Yaylacı, M.; Birinci, A. A study on the contact problem of a layer consisting of functionally graded material (FGM) in the presence of body force. J. Mech. Mater. Struct. 2023, 18, 125–141. [Google Scholar] [CrossRef]
- Ege, N.; Erbaş, B.; Kaplunov, J.; Noori, N. Low-frequency vibrations of a thin-walled functionally graded cylinder (plane strain problem). Mech. Adv. Mater. Struct. 2023, 30, 1172–1180. [Google Scholar] [CrossRef]
- Kaplunov, J.; Erbaş, B.; Ege, N. Asymptotic derivation of 2D dynamic equations of motion for transversely inhomogeneous elastic plates. Int. J. Eng. Sci. 2022, 178, 103723. [Google Scholar] [CrossRef]
- Le, K.C. An asymptotically exact first-order shear deformation theory for functionally graded plates. Int. J. Eng. Sci. 2023, 190, 103875. [Google Scholar] [CrossRef]
- Akhmedov, N.K.; Sofiyev, A.H. Asymptotic analysis of three-dimensional problem of elasticity theory for radially inhomogeneous transversally-isotropic thin hollow spheres. Thin-Walled Struct. 2019, 139, 232–241. [Google Scholar] [CrossRef]
- Huang, Q.; Gao, Y.; Hua, F.; Fu, W.; You, Q.; Gao, J.; Zhou, X. Free vibration analysis of carbon-fiber plain woven reinforced composite conical-cylindrical shell under thermal environment with general boundary conditions. Compos. Struct. 2023, 322, 117340. [Google Scholar] [CrossRef]
- Akhmedov, N.K.; Gasanova, N.S. Asymptotic behavior of the solution of an axisymmetric problem of elasticity theory for a sphere with variable elasticity modules. Math. Mech. Solids. 2020, 25, 2231–2251. [Google Scholar] [CrossRef]
- Argatov, I.; Mishuris, G. (Eds.) Contact Mechanics of Articular Cartilage Layers: Asymptotic Models; Springer: Cham, Switzerland, 2015; 335p. [Google Scholar]
- Goldenveizer, A.L. Algorithms for the asymptotic construction of a linear two-dimensional theory of thin shells and the Saint-Venant principle. PMM J. Appl. Math. Mech. 1994, 58, 1039–1050. [Google Scholar] [CrossRef]
- Gregory, R.D.; Wan, F.Y.M. On plate theories and Saint-Venant’s principle. Int. J. Solids Struct. 1985, 21, 1005–1024. [Google Scholar] [CrossRef]
- Aghalovyan, L.A. Asymptotic Theory of Anisotropic Plates and Shells; World Scientific: Singapore, 2015. [Google Scholar]
- Goldenveizer, A.L. Theory of Thin Elastic Shells; Nauka: Moscow, Russia, 1976. [Google Scholar]
- Goldenveizer, A.L. The general theory of elastic bodies (shells, coatings and linings). Mech. Solids 1992, 3, 3–17. [Google Scholar]
- Kaplunov, J.D.; Kossovich, L.J.; Nolde, E.V. Dynamics of Thin Walled Elastic Bodies; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- Erbaş, B.; Kaplunov, J.; Rajagopal, K.R. Elastic bending and transverse compression of a thin plate with density-dependent Young’s modulus. Int. J. Non-Linear Mech. 2024, 160, 104651. [Google Scholar] [CrossRef]
- Arumugam, J.; Alagappan, P.; Bird, J.; Moreno, M.; Rajagopal, K.R. A new constitutive relation to describe the response of bones. Int. J. Non-Linear Mech. 2024, 161, 104664. [Google Scholar] [CrossRef]
- Abd Aziz, A.U.; Ammarullah, M.I.; Ng, B.W.; Gan, H.S.; Abdul Kadir, M.R.; Ramlee, M.H. Unilateral external fixator and its biomechanical effects in treating different types of femoral fracture: A finite element study with experimental validated model. Heliyon 2024, 10, e26660. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alzaidi, A.S.M.; Kaplunov, J.; Zupančič, B.; Nikonov, A. Transverse Compression of a Thin Inhomogeneous Elastic Layer. Mathematics 2024, 12, 2502. https://doi.org/10.3390/math12162502
Alzaidi ASM, Kaplunov J, Zupančič B, Nikonov A. Transverse Compression of a Thin Inhomogeneous Elastic Layer. Mathematics. 2024; 12(16):2502. https://doi.org/10.3390/math12162502
Chicago/Turabian StyleAlzaidi, Ahmed S. M., Julius Kaplunov, Barbara Zupančič, and Anatolij Nikonov. 2024. "Transverse Compression of a Thin Inhomogeneous Elastic Layer" Mathematics 12, no. 16: 2502. https://doi.org/10.3390/math12162502





