Nonlinear Medical Ultrasound Tomography: 3D Modeling of Sound Wave Propagation in Human Tissues
Abstract
1. Introducion
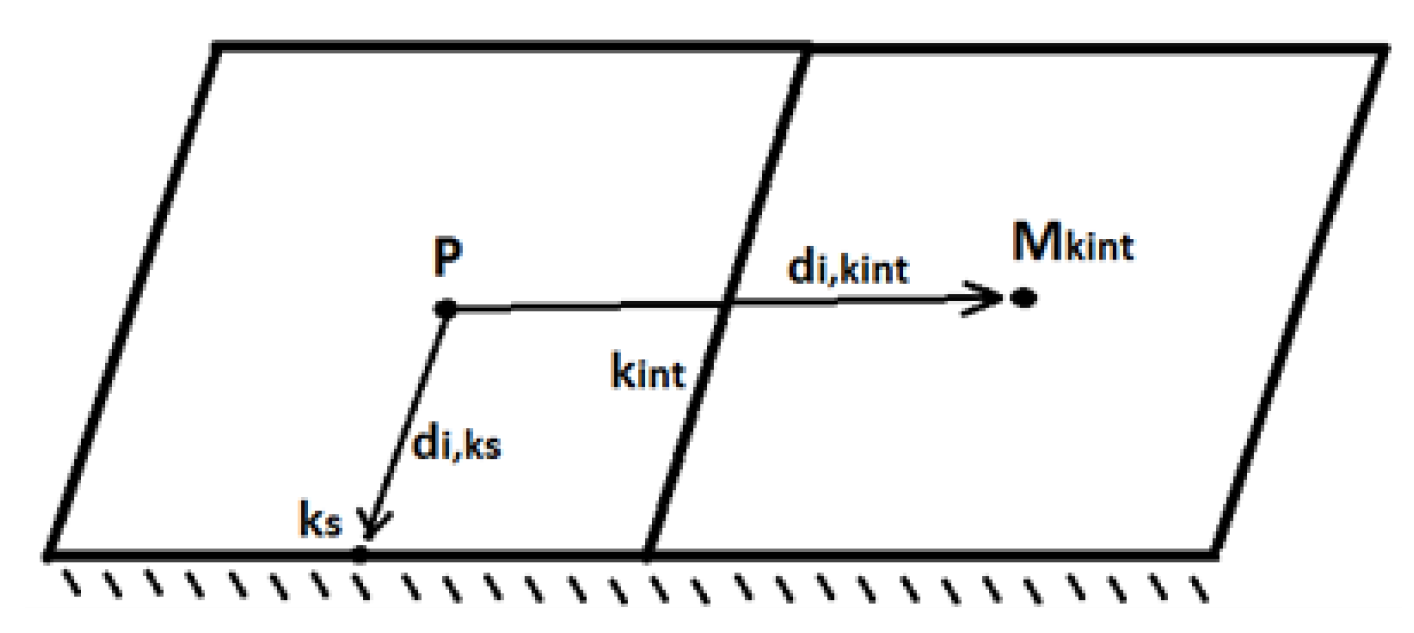
2. Governing Equations and the Numerical Method
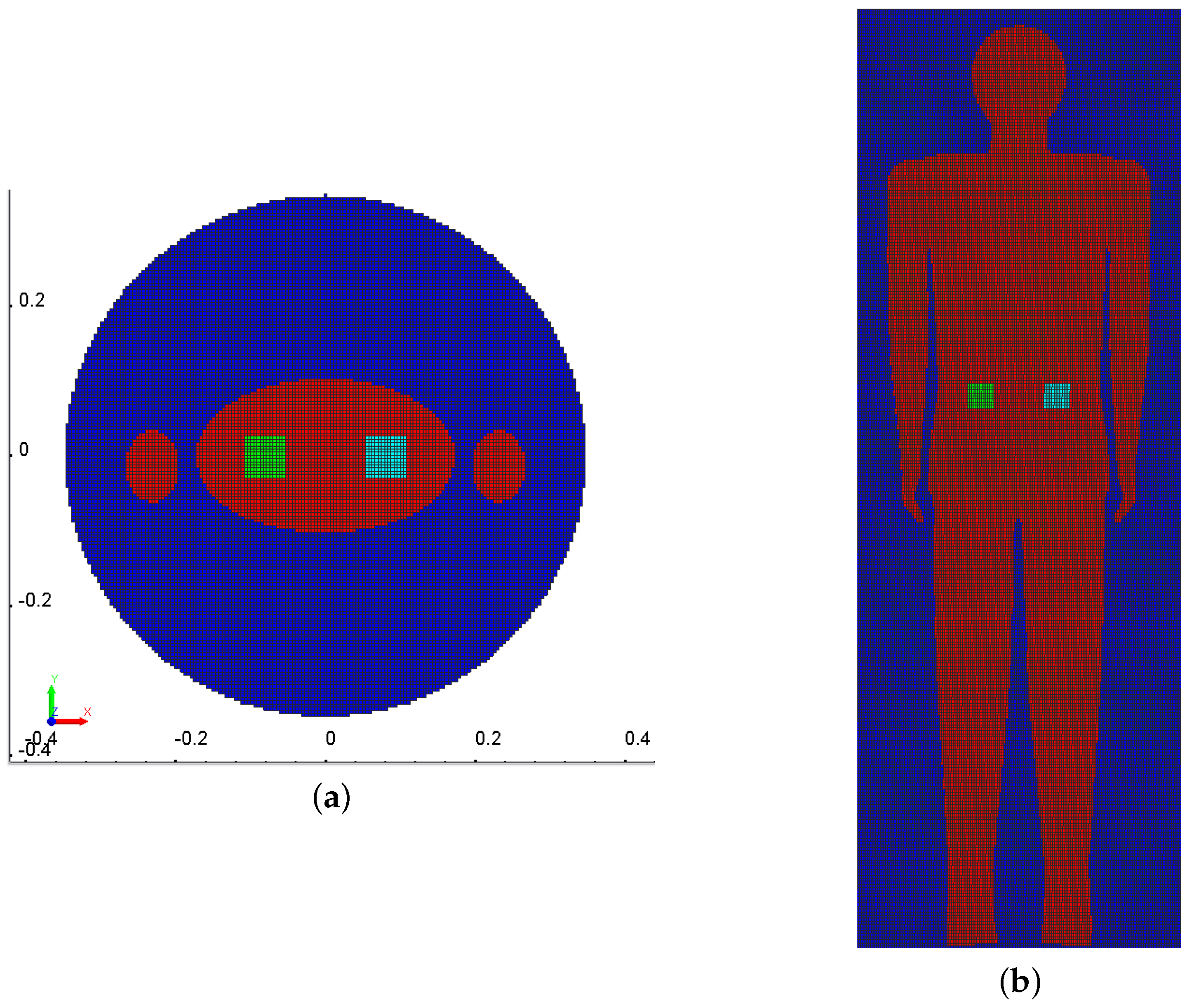
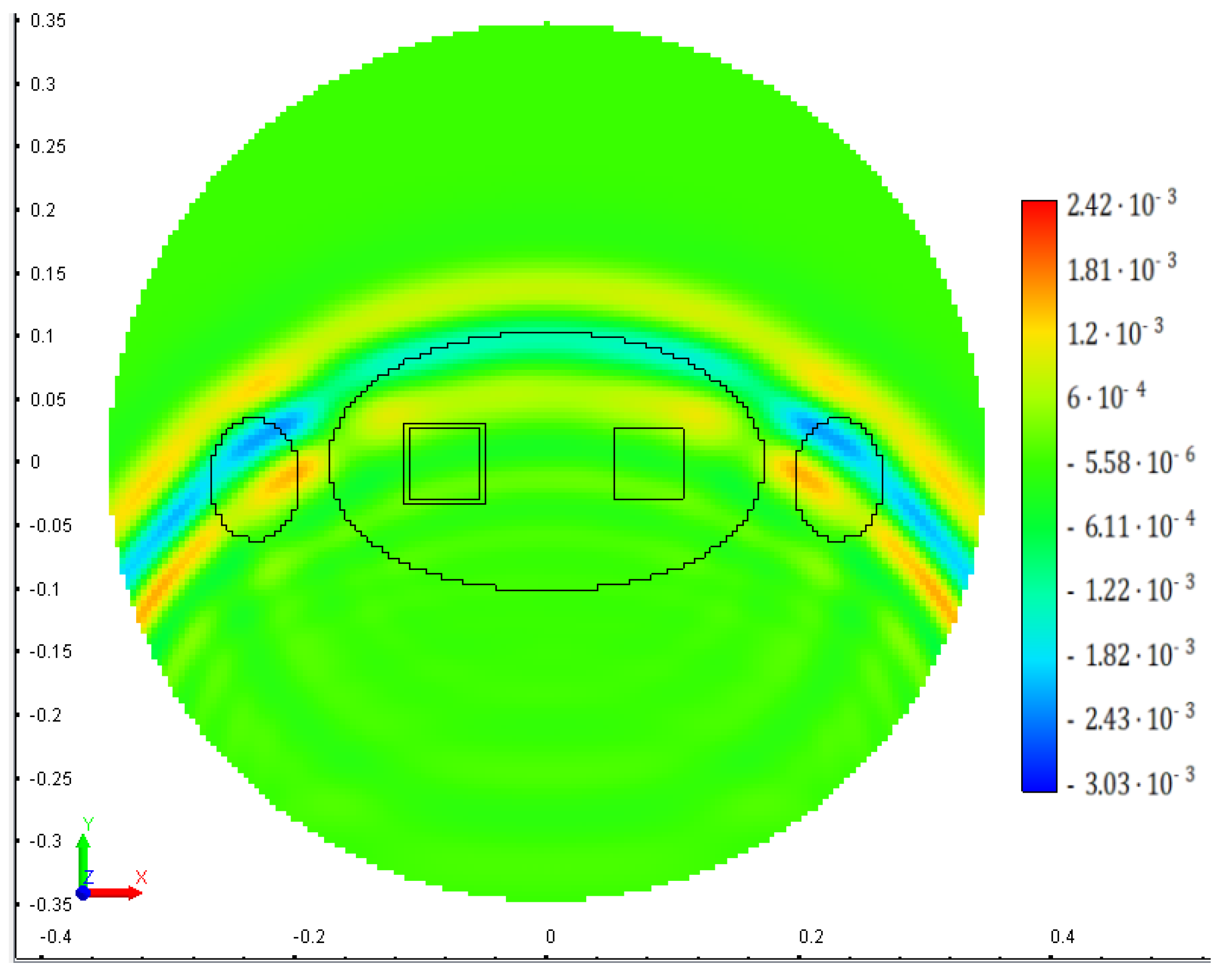
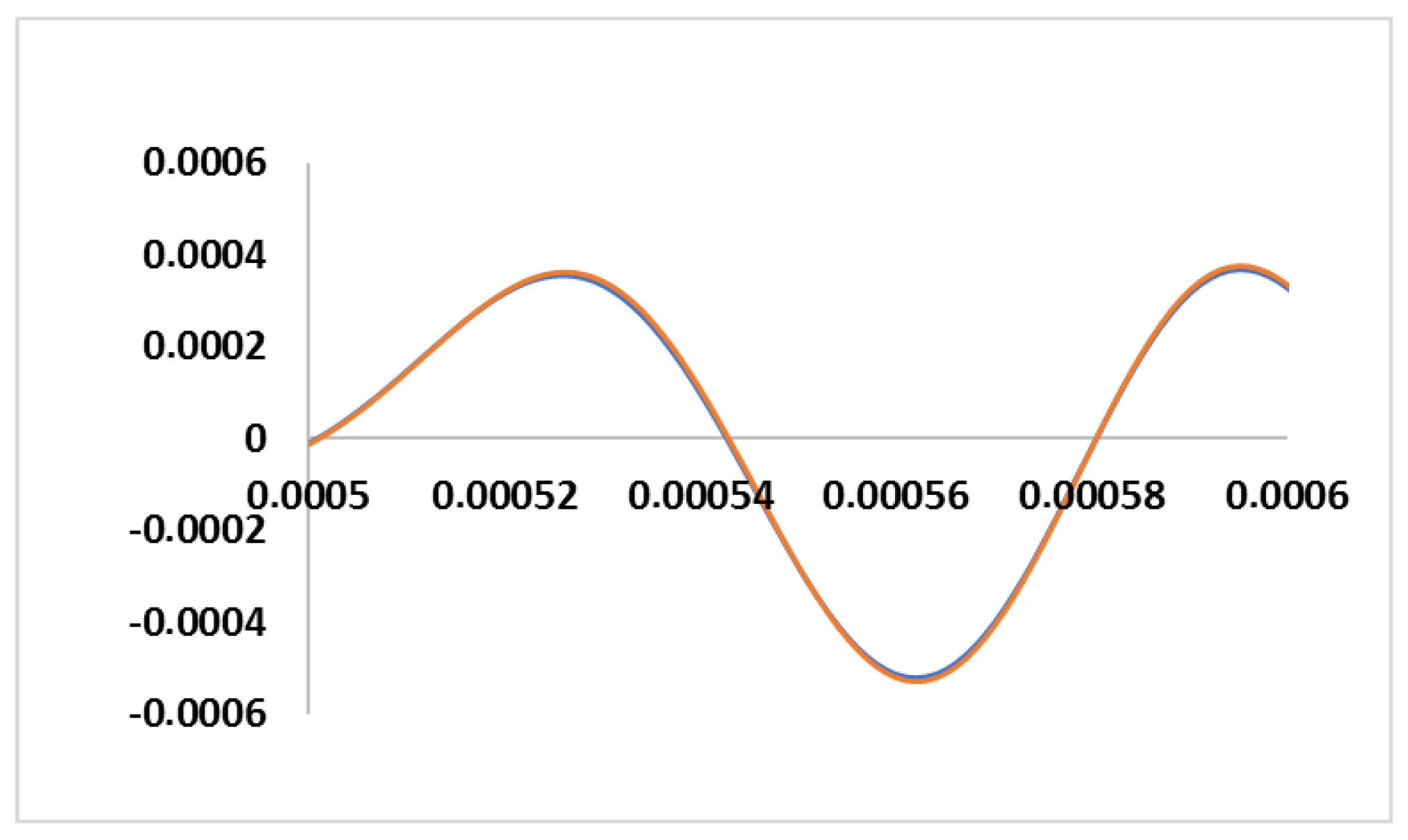
3. Numerical Experiments
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Strutt, J.W. The Theory of Sound; Cambridge University Press: Cambridge, UK, 2011; Volume 1. [Google Scholar]
- Strutt, J.W. The Theory of Sound; Cambridge University Press: Cambridge, UK, 2011; Volume 2. [Google Scholar]
- Li, S.; Jackowski, M.; Dione, D.P.; Varslot, T.; Staib, L.H.; Mueller, K. Refraction corrected transmission ultrasound computed tomography for application in breast imaging. Med. Phys. 2010, 37, 2233–2246. [Google Scholar] [CrossRef] [PubMed]
- Huthwaite, P.; Simonetti, F. High-resolution imaging without iteration: A fast and robust method for breast ultrasound tomography. J. Acoust. Soc. Am. 2011, 130, 1721–1734. [Google Scholar] [CrossRef] [PubMed]
- Duric, N.; Littrup, P.; Li, C.; Roy, O.; Schmidt, S.; Janer, R.; Cheng, X.; Goll, J.; Rama, O.; Bey-Knight, L.; et al. Breast ultrasound tomography: Bridging the gap to clinical practice. Proc. SPIE 2012, 8320, 832000. [Google Scholar]
- Jirik, R.; Peterlik, I.; Ruiter, N.; Fousek, J.; Dapp, R.; Zapf, M.; Jan, J. Sound-speed image reconstruction in sparse-aperture 3D ultrasound transmission tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 254–264. [Google Scholar] [CrossRef] [PubMed]
- Marmot, M.G.; Altman, D.G.; Cameron, D.A.; Dewar, J.A.; Thompson, S.G.; Wilcox, M. The benefits and harms of breast cancer screening: An independent review. Br. J. Cancer 2013, 108, 2205–2240. [Google Scholar] [CrossRef] [PubMed]
- Birk, M.; Dapp, R.; Ruiter, N.V.; Becker, J. GPU-based iterative transmission reconstruction in 3D ultrasound computer tomography. J. Parallel Distrib. Comput. 2014, 74, 1730–1743. [Google Scholar] [CrossRef]
- Burov, V.A.; Zotov, D.I.; Rumyantseva, O.D. Reconstruction of the sound velocity and absorption spatial distributions in soft biological tissue phantoms from experimental ultrasound tomography data. Acoust. Phys. 2015, 61, 231–248. [Google Scholar] [CrossRef]
- Sandhu, G.Y.; Li, C.; Roy, O.; Schmidt, S.; Duric, N. Frequency domain ultrasound waveform tomography: Breast imaging using a ring transducer. Phys. Med. Biol. 2015, 60, 5381–5398. [Google Scholar] [CrossRef]
- Liu, H.; Uhlmann, G. Determining both sound speed and internal source in thermo- and photo-acoustic tomography. Inverse Probl. 2015, 31, 105005. [Google Scholar] [CrossRef]
- Huang, L.; Shin, J.; Chen, T.; Lin, Y.; Gao, K.; Intrator, M.; Hanson, K. Breast ultrasound tomography with two parallel transducer arrays. In Medical Imaging 2016: Physics of Medical Imaging (International Society for Optics and Photonics); SPIE: Bellingham, WA, USA, 2016; Volume 9783, p. 97830C. [Google Scholar]
- Matthews, T.P.; Wang, K.; Li, C.; Duric, N.; Anastasio, M.A. Regularized dual averaging image reconstruction for full-wave ultrasound computed tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2017, 64, 811–825. [Google Scholar] [CrossRef]
- Opieliński, K.J.; Pruchnicki, P.; Szymanowski, P.; Szepieniec, W.K.; Szweda, H.; Świś, E.; Jóźwik, M.; Tenderenda, M.; Bułkowski, M. Multimodal ultrasound computer-assisted tomography: An approach to the recognition of breast lesions. Comput. Med. Imaging Graph. 2018, 65, 102–114. [Google Scholar] [CrossRef]
- Malik, B.; Terry, R.; Wiskin, J.; Lenox, M. Quantitative transmission ultrasound tomography: Imaging and performance characteristics. Med. Phys. 2018, 45, 3063–3075. [Google Scholar] [CrossRef] [PubMed]
- Guo, R.; Lu, G.; Qin, B.; Fei, B. Ultrasound imaging technologies for breast cancer detection and management: A review. Ultrasound Med. Biol. 2018, 44, 37–70. [Google Scholar] [CrossRef] [PubMed]
- Wiskin, J.; Malik, B.; Natesan, R.; Lenox, M. Quantitative assessment of breast density using transmission ultrasound tomography. Med. Phys. 2019, 46, 2610–2620. [Google Scholar] [CrossRef] [PubMed]
- Sood, R.; Rositch, A.F.; Shakoor, D.; Ambinder, E.; Pool, K.L.; Pollack, E.; Mollura, D.J.; Mullen, L.A.; Harvey, S.C. Ultrasound for breast cancer detection globally: A systematic review and meta-analysis. J. Glob. Oncol. 2019, 5, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Vourtsis, A. Three-dimensional automated breast ultrasound: Technical aspects and first results. Diagn. Intervent. Radiol. 2019, 100, 579–592. [Google Scholar] [CrossRef]
- Park, C.K.S.; Xing, S.; Papernick, S.; Orlando, N.; Knull, E.; Toit, C.D.; Bax, J.S.; Gardi, L.; Barker, K.; Tessier, D.; et al. Spatially tracked whole-breast three-dimensional ultrasound system toward point-of-care breast cancer screening in high-risk women with dense breasts. Med. Phys. 2022, 49, 3944–3962. [Google Scholar] [CrossRef]
- Wang, K.; Matthews, T.; Anis, F.; Li, C.; Duric, N.; Anastasio, M.A. Waveform inversion with source encoding for breast sound speed reconstruction in ultrasound computed tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 475–493. [Google Scholar] [CrossRef]
- Bernard, S.; Monteiller, V.; Komatitsch, D.; Lasaygues, P. Ultrasonic computed tomography based on full-waveform inversion for bone quantitative imaging. Phys. Med. Biol. 2017, 62, 7011–7035. [Google Scholar] [CrossRef]
- Pérez-Liva, M.; Herraiz, J.L.; Udías, J.M.; Miller, E.; Cox, B.T.; Treeby, B.E. Time domain reconstruction of sound speed and attenuation in ultrasound computed tomography using full wave inversion. J. Acoust. Soc. Am. 2017, 141, 1595–1604. [Google Scholar] [CrossRef]
- Filatova, V.; Danilin, A.; Nosikova, V.; Pestov, L. Supercomputer Simulations of the Medical Ultrasound Tomography Problem. Commun. Comput. Inf. Sci. 2019, 1063, 297–308. [Google Scholar]
- Kabanikhin, S.I.; Klyuchinskiy, D.V.; Novikov, N.S.; Shishlenin, M.A. Numerics of acoustical 2D tomography based on the conservation laws. J. Inverse Ill-Posed Probl. 2020, 28, 287–297. [Google Scholar] [CrossRef]
- Klyuchinskiy, D.; Novikov, N.; Shishlenin, M. Recovering density and speed of sound coefficients in the 2d hyperbolic system of acoustic equations of the first order by a finite number of observations. Mathematics 2021, 9, 199. [Google Scholar] [CrossRef]
- Filatova, V.; Pestov, L.; Poddubskaya, A. Detection of velocity and attenuation inclusions in the medical ultrasound tomography. J. Inverse Ill-Posed Probl. 2021, 29, 459–466. [Google Scholar] [CrossRef]
- Rumyantseva, O.D.; Shurup, A.S.; Zotov, D.I. Possibilities for separation of scalar and vector characteristics of acoustic scatterer in tomographic polychromatic regime. J. Inverse Ill-Posed Probl. 2021, 29, 407–420. [Google Scholar] [CrossRef]
- Shurup, A.S. Numerical comparison of iterative and functional-analytical algorithms for inverse acoustic scattering. Eurasian J. Math. Comput. Appl. 2022, 10, 79–99. [Google Scholar] [CrossRef]
- Lucka, F.; Pérez-Liva, M.; Treeby, B.E.; Cox, B.T. High resolution 3D ultrasonic breast imaging by time-domain full waveform inversion. Inverse Probl. 2022, 38, 025008. [Google Scholar] [CrossRef]
- Romanov, V.G.; Kabanikhin, S.I. Inverse Problems for Maxwell’s Equations; VSP: Utrecht, The Netherlands, 1994. [Google Scholar]
- Jafarzadeh, E.; Amini, M.H.; Sinclair, A.N. Spectral Shift Originating from Non-linear Ultrasonic Wave Propagation and Its Effect on Imaging Resolution. Ultrasound Med. Biol. 2021, 47, 1893–1903. [Google Scholar] [CrossRef]
- Campo-Valera, M.; Villó-Pérez, I.; Fernández-Garrido, A.; Rodríguez-Rodríguez, I.; Asorey-Cacheda, R. Exploring the Parametric Effect in Nonlinear Acoustic Waves. IEEE Access 2023, 11, 97221–97238. [Google Scholar] [CrossRef]
- Ichida, N.; Sato, T.; Linzer, M. Imaging the nonlinear ultrasonic parameter of a medium. Ultrason. Imaging 1983, 5, 295–299. [Google Scholar] [CrossRef]
- Lewin, P.A.; Nowicki, A. 16—Nonlinear acoustics and its application to biomedical ultrasonics. In Woodhead Publishing Series in Electronic and Optical Materials, Ultrasonic Transducers; Nakamura, K., Ed.; Woodhead Publishing: Cambridge, UK, 2012; pp. 517–544. [Google Scholar] [CrossRef]
- Meliani, M.; Nikolić, V. Analysis of General Shape Optimization Problems in Nonlinear Acoustics. Appl. Math. Optim. 2022, 86, 39. [Google Scholar] [CrossRef]
- Panfilova, A.; van Sloun, R.J.; Wijkstra, H.; Sapozhnikov, O.A.; Mischi, M. A review on B/A measurement methods with a clinical perspective. J. Acoust. Soc. Am. 2021, 149, 2200. [Google Scholar] [CrossRef]
- Tabak, G.; Oelze, M.L.; Singer, A.C. Effects of acoustic nonlinearity on communication performance in soft tissues. J. Acoust. Soc. Am. 2022, 152, 3583. [Google Scholar] [CrossRef]
- Kokumo, K.; Sharma, A.; Myers, K.; Ambinder, E.; Oluyemi, E.; Bell, M.A.L. Theoretical basis and experimental validation of harmonic coherence-based ultrasound imaging for breast mass diagnosis. In Medical Imaging 2023: Physics of Medical Imaging; SPIE: Bellingham, WA, USA, 2023; Volume 12463, pp. 95–104. [Google Scholar]
- Ferziger, J.H.; Peric, M. Computational Method for Fluid Dynamics; Springer: New York, NY, USA, 2002. [Google Scholar]
- Mozer, D.; Kim, J.; Mansour, N.N. DNS of Turbulent Channel Flow. J. Phys. Fluids 1999, 11, 943–945. [Google Scholar]
- Muir, T.G.; Carstensen, E.L. Prediction of nonlinear acoustic effects at biomedical frequencies and intensities. Ultrasound Med. Biol. 1980, 6, 345–357. [Google Scholar] [CrossRef] [PubMed]
- Okawai, H.; Kobayashi, K.; Nitta, S. An approach to acoustic properties of biological tissues using acoustic micrographs of attenuation constant and sound speed. J. Ultrasound Med. 2001, 20, 891–907. [Google Scholar] [CrossRef] [PubMed]
- Duck, F.A. Nonlinear acoustics in diagnostic ultrasound. Ultrasound Med. Biol. 2002, 28, 1–18. [Google Scholar] [CrossRef]
- Kaltenbacher, B. Mathematics of nonlinear acoustics. Evol. Equ. Control. Theory 2015, 4, 447–491. [Google Scholar] [CrossRef]
- Yu, T.; Cai, W. Simultaneous reconstruction of temperature and velocity fields using nonlinear acoustic tomography. Appl. Phys. Lett. 2019, 115, 104104. [Google Scholar] [CrossRef]
- Deng, Y.; Li, J.; Liu, H. On Identifying Magnetized Anomalies Using Geomagnetic Monitoring Within a Magnetohydrodynamic model. Arch. Ration. Mech. Anal. 2020, 235, 691–721. [Google Scholar] [CrossRef]
- Gan, W.S. Nonlinear Acoustical Imaging; Springer: Singapore, 2021. [Google Scholar]
- Kozelkov, A.S.; Krutyakova, O.G.L.; Kurulin, V.V.; Strelets, D.Y.E.; Shishlenin, M.A. The accuracy of numerical simulation of the acoustic wave propagations in a liquid medium based on Navier-Stokes equations. Sib. Electron. Math. Rep. 2021, 18, 1238–1250. [Google Scholar] [CrossRef]
- Maev, R.G.; Seviaryn, F. Applications of non-linear acoustics for quality control and material characterization. J. Appl. Phys. 2022, 132, 161101. [Google Scholar] [CrossRef]
- Chen, P.; Pollet, A.M.; Panfilova, A.; Zhou, M.; Turco, S.; den Toonder, J.M.; Mischi, M. Acoustic characterization of tissue-mimicking materials for ultrasound perfusion imaging research. Ultrasound Med. Biol. 2022, 48, 124–142. [Google Scholar] [CrossRef]
- Gemmeke, H.; Hopp, T.; Zapf, M.; Kaiser, C.; Ruiter, N.V. 3D ultrasound computer tomography: Hardware setup, reconstruction methods and first clinical results. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2017, 873, 59–65. [Google Scholar] [CrossRef]
- Zhang, Y. Nonlinear acoustic imaging with damping. arXiv 2023, arXiv:2302.14174. [Google Scholar]
- Wiskin, J.; Malik, B.; Klock, J. Low frequency 3D transmission ultrasound tomography: Technical details and clinical implications. Z. Med. Phys. 2023, 33, 427–443. [Google Scholar] [CrossRef]
- Wiskin, J.; Malik, B.; Ruoff, C.; Pirshafiey, N.; Lenox, M.; Klock, J. Whole-Body Imaging Using Low Frequency Transmission Ultrasound. Acad. Radiol. 2023, 30, 2674–2685. [Google Scholar] [CrossRef]
- Lashkin, S.V.; Kozelkov, A.S.; Yalozo, A.V.; Gerasimov, V.Y.; Zelensky, D.K. Efficiency analysis of the parallel implementation of the simple algorithm on multiprocessor computers. J. Appl. Mech. Tech. Phys. 2017, 58, 1242–1259. [Google Scholar] [CrossRef]
- Kozelkov, A.; Tyatyushkina, E.; Kurkin, A.; Kurulin, V.; Kurkina, O.; Kochetkova, O. Fluid flow simulation in a T-connection of square pipes using modern approaches to turbulence modeling. Sib. Electron. Math. Rep. 2023, 20, 25–46. [Google Scholar]
- Kozelkov, A.; Kurkin, A.; Utkin, D.; Tyatyushkina, E.; Kurulin, V.; Strelets, D. Application of Non-Reflective Boundary Conditions in Three-Dimensional Numerical Simulations of Free-Surface Flow Problems. Geosciences 2022, 12, 427. [Google Scholar] [CrossRef]
- Kozelkov, A.S.; Lashkin, S.V.; Efremov, V.R.; Volkov, K.N.; Tsibereva, Y.A.; Tarasova, N.V. An implicit algorithm of solving Navier–Stokes equations to simulate flows in anisotropic porous media. Comput. Fluids 2018, 160, 164–174. [Google Scholar] [CrossRef]
- Chen, Z.J.; Przekwas, A.J. A coupled pressure-based computational method for incompressible/compressible flows. J. Comput. Phys. 2010, 229, 9150–9165. [Google Scholar] [CrossRef]
- Moukalled, F.; Darwish, M. Pressure-Based Algorithms for Multi-Fluid Flow at All Speeds—Part I: Mass Conservation Formulation, Numerical Heat Transfer. Part B Fundam. 2004, 45, 495–522. [Google Scholar] [CrossRef]
- Jasak, H. Error Analysis and Estimation for the Finite Volume Method with Applications to Fluid Flows. Ph.D. Thesis, Department of Mechanical Engineering, Imperial College of Science, London, UK, 1996. [Google Scholar]
- Jasak, H.; Weller, H.G.; Gosman, A.D. High resolution NVD differencing scheme for arbitrarily unstructured meshes. Int. J. Numer. Methods Fluids 1999, 31, 431–449. [Google Scholar] [CrossRef]
- Leonard, B.P. A stable and accurate convective modeling procedure based on quadratic upstream interpolation. Comput. Methods Appl. Mech./Eng. 1979, 19, 59–98. [Google Scholar] [CrossRef]
- Gaskell, P.H. Curvature-compensated convective-transport—SMART, A new boundedness-preserving transport algorithm. Int. J. Numer. Methods Fluids 1988, 8, 617–641. [Google Scholar] [CrossRef]
- Goss, S.A.; Johnston, R.L.; Dunn, F. Comprehensive compilation of empirical ultrasonic properties of mammalian tissues. J. Acoust. Soc. Am. 1978, 64, 423–457. [Google Scholar] [CrossRef]
- Mast, T.D. Empirical relationships between acoustic parameters in human soft tissues. Acoust. Res. Lett. 2000, 1, 37–42. [Google Scholar] [CrossRef]
- Klyuchinskiy, D.; Novikov, N.; Shishlenin, M. A Modification of gradient descent method for solving coefficient inverse problem for acoustics equations. Computation 2020, 8, 73. [Google Scholar] [CrossRef]
- Shishlenin, M.A.; Savchenko, N.A.; Novikov, N.S.; Klyuchinskiy, D.V. On the reconstruction of the absorption coefficient for the 2D acoustic system. Sib. Electron. Math. Rep. 2023, 20, 1474–1489. [Google Scholar]
- Klyuchinskiy, D.V.; Novikov, N.S.; Shishlenin, M.A. CPU-time and RAM memory optimization for solving dynamic inverse problems using gradient-based approach. J. Comput. Phys. 2021, 439, 110374. [Google Scholar] [CrossRef]
- Jenaliyev, M.T.; Bektemesov, M.A.; Yergaliyev, M.G. On an inverse problem for a linearized system of Navier–Stokes equations with a final overdetermination condition. J. Inverse Ill-Posed Probl. 2023, 31, 611–624. [Google Scholar] [CrossRef]
- Wang, Z.; Dulikravich, G. A numerical Method for Solving Cascade Inverse Problems Using Navier-Stokes Equations. 33rd Aerospace Sciences Meeting and Exhibit. 1995. Published Online: 22 August 2012. Available online: https://arc.aiaa.org/doi/abs/10.2514/6.1995-304 (accessed on 20 November 2023).
- Arridge, S.; Maass, P.; Öktem, O.; Schönlieb, C. Solving inverse problems using data-driven models. Acta Numer. 2019, 28, 1–174. [Google Scholar] [CrossRef]
- Basurto-Hurtado, J.A.; Cruz-Albarran, I.A.; Toledano-Ayala, M.; Ibarra-Manzano, M.A.; Morales-Hernandez, L.A.; Perez-Ramirez, C.A. Diagnostic Strategies for Breast Cancer Detection: From Image Generation to Classification Strategies Using Artificial Intelligence Algorithms. Cancers 2022, 14, 3442. [Google Scholar] [CrossRef]
- Fan, Y.; Wang, H.; Gemmeke, H.; Hopp, T.; Hesser, J. Model-data-driven image reconstruction with neural networks for ultrasound computed tomography breast imaging. Neurocomputing 2022, 467, 10–21. [Google Scholar] [CrossRef]






| Speed of Sound, m/s | Density, kg/m3 | |
|---|---|---|
| Muscular tissue | 1550 | 994 |
| Adipose tissue | 1460 | 904 |
| Liver | 1570 | 1083 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shishlenin, M.; Kozelkov, A.; Novikov, N. Nonlinear Medical Ultrasound Tomography: 3D Modeling of Sound Wave Propagation in Human Tissues. Mathematics 2024, 12, 212. https://doi.org/10.3390/math12020212
Shishlenin M, Kozelkov A, Novikov N. Nonlinear Medical Ultrasound Tomography: 3D Modeling of Sound Wave Propagation in Human Tissues. Mathematics. 2024; 12(2):212. https://doi.org/10.3390/math12020212
Chicago/Turabian StyleShishlenin, Maxim, Andrey Kozelkov, and Nikita Novikov. 2024. "Nonlinear Medical Ultrasound Tomography: 3D Modeling of Sound Wave Propagation in Human Tissues" Mathematics 12, no. 2: 212. https://doi.org/10.3390/math12020212
APA StyleShishlenin, M., Kozelkov, A., & Novikov, N. (2024). Nonlinear Medical Ultrasound Tomography: 3D Modeling of Sound Wave Propagation in Human Tissues. Mathematics, 12(2), 212. https://doi.org/10.3390/math12020212







