Loss-Averse Supply Chain Coordination with a Revenue-Sharing Contract
Abstract
:1. Background
- What are the equilibrium decisions in the game?
- Could the revenue sharing coordinate the supply chain under three different scenarios? And how?
- If yes, what are the effects of loss aversion on the two sides’ optimal decisions under the three game scenarios? And at the same time, does maximizing utility align with maximizing profits?
- How do risk aversion levels and revenue-sharing factors interact with each other under the three scenarios?
- It introduces decision-makers’ loss aversion into traditional supply chain revenue-sharing contracts, modifying the “economic man” assumption of suppliers or retailers to reduce prediction errors.
- It is based on game theory and explores the coordination process and equilibrium analysis between the retailer and the supplier, enriching the research on behavioral supply chains, and providing analytical methods and a theoretical basis for supply chain coordination research and enterprise management.
- We extend equilibrium decision analysis to show the impact of loss-averse behavior on the revenue-sharing contract.
2. Literature Review
2.1. Supply Chain Coordination
2.2. Supply Chain Contracts
2.3. Loss Aversion
3. Benchmark Model
Centralized and Decentralized Decision Models
- (i)
- Under decentralized decision making, the retailer’s profit maximizes at an optimal order quantity,. At this time, the order quantity is
- (ii)
- When the supplier’s wholesale price , the supply chain is coordinated under the revenue-sharing contract, i.e., .
4. Loss Aversion Model
4.1. The NA Scenario
- (i)
- Under decentralized decision making, there exists a unique optimal order quantity that maximizes the expected profit of the loss-averse retailer, . And means the retailer’s optimal order quantity when loss-averse is lower than when risk-neutral.
- (ii)
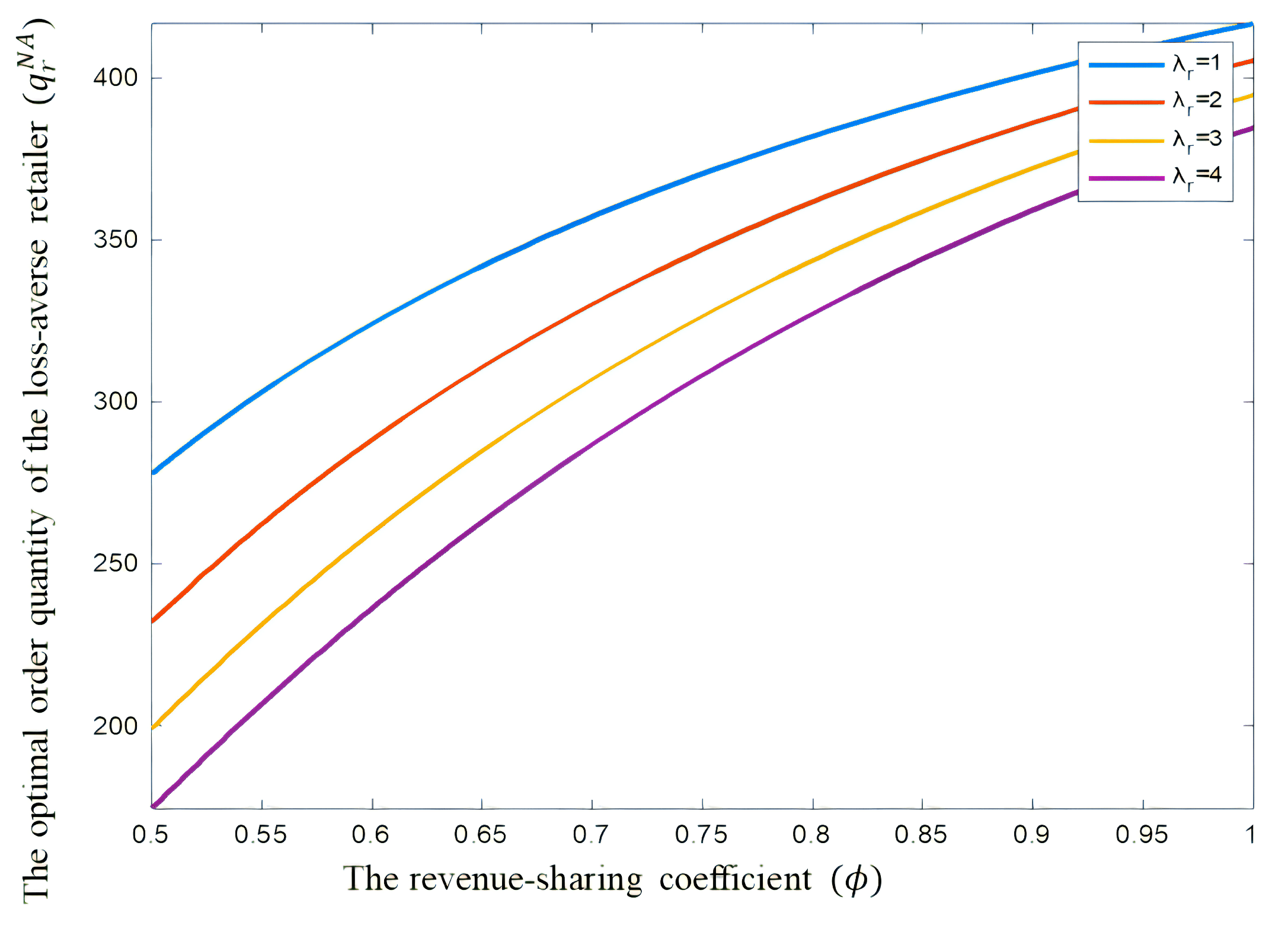
- When , , meaning the loss-averse retailer’s optimal order quantity equals their risk-neutral optimal order quantity. Thus, when , it indicates the retailer’s loss-averse attitude, leading to a reduction in order quantity. The optimal order quantity decreases with the supplier’s wholesale price w and the loss aversion coefficient but increases with the revenue-sharing coefficient ϕ. In a revenue-sharing contract, the loss-averse retailer’s expected utility function decreases with the supplier’s wholesale price w and the loss aversion coefficient .
- (iii)
- Under centralized decision making, the retailer’s optimal order quantity is .
- (iv)
- The supply chain is coordinated when and satisfy the setmoreover, .
4.2. The AN Scenario
- (i)
- Under centralized decision making, ; under decentralized decision making, .
- (ii)
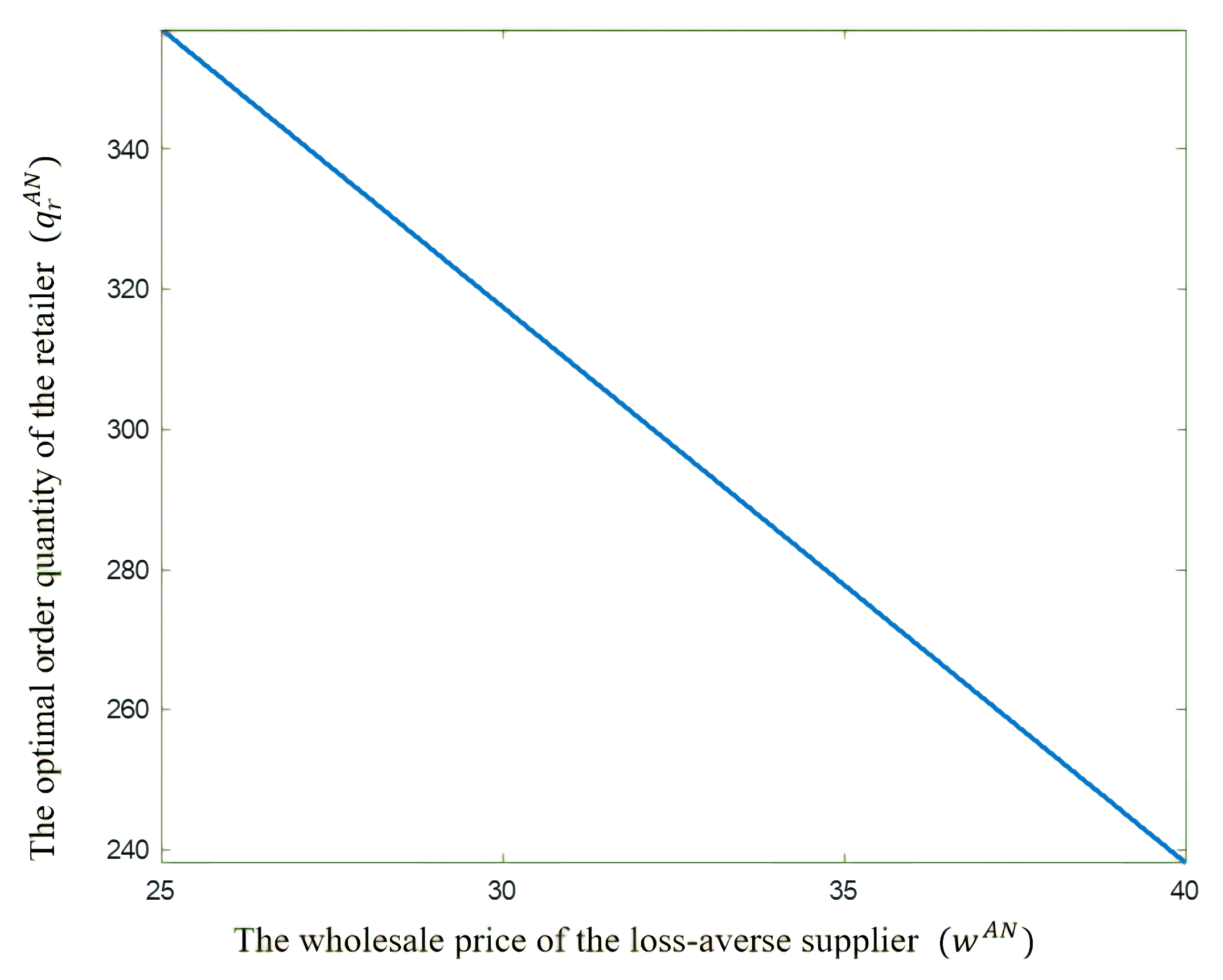
- When , . When , it indicates the supplier is loss-averse, and thus, .
- (iii)
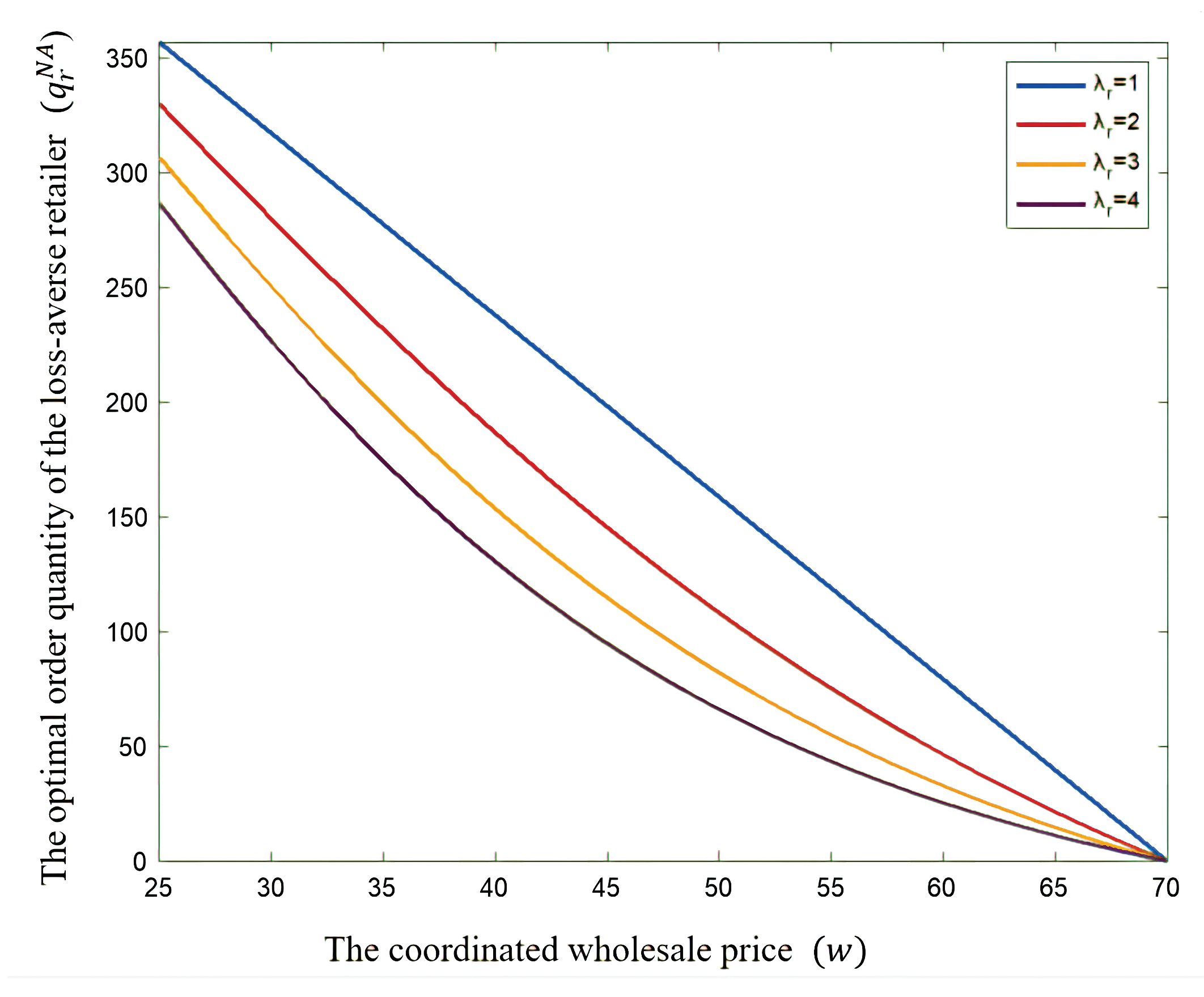
- Under decentralized decision making, the risk-neutral retailer’s optimal order quantity decreases with the wholesale price w but increases with the revenue-sharing coefficient ϕ. When ϕ satisfies , decreases with ϕ; otherwise, increases with ϕ.
- (iv)
- The supply chain system can be effectively coordinated when and satisfy the set
4.3. The AA Scenario
- (i)
- Under decentralized decision making, ; under centralized decision making,.
- (ii)
- When , the optimal order quantity increases with the supplier’s wholesale price w; otherwise, it is negatively correlated.
- (iii)
- When , decreases with the revenue-sharing coefficient ϕ; otherwise, increases with the revenue-sharing coefficient ϕ.
- (iv)
- The supply chain is coordinated if and satisfy the set
5. Comparison and Sensitivity Analysis
5.1. Comparison
- (i)
- The optimal wholesale price satisfies the relationship
- (ii)
- The optimal order quantity the relationship
5.2. Numerical Analysis in the NA Scenario
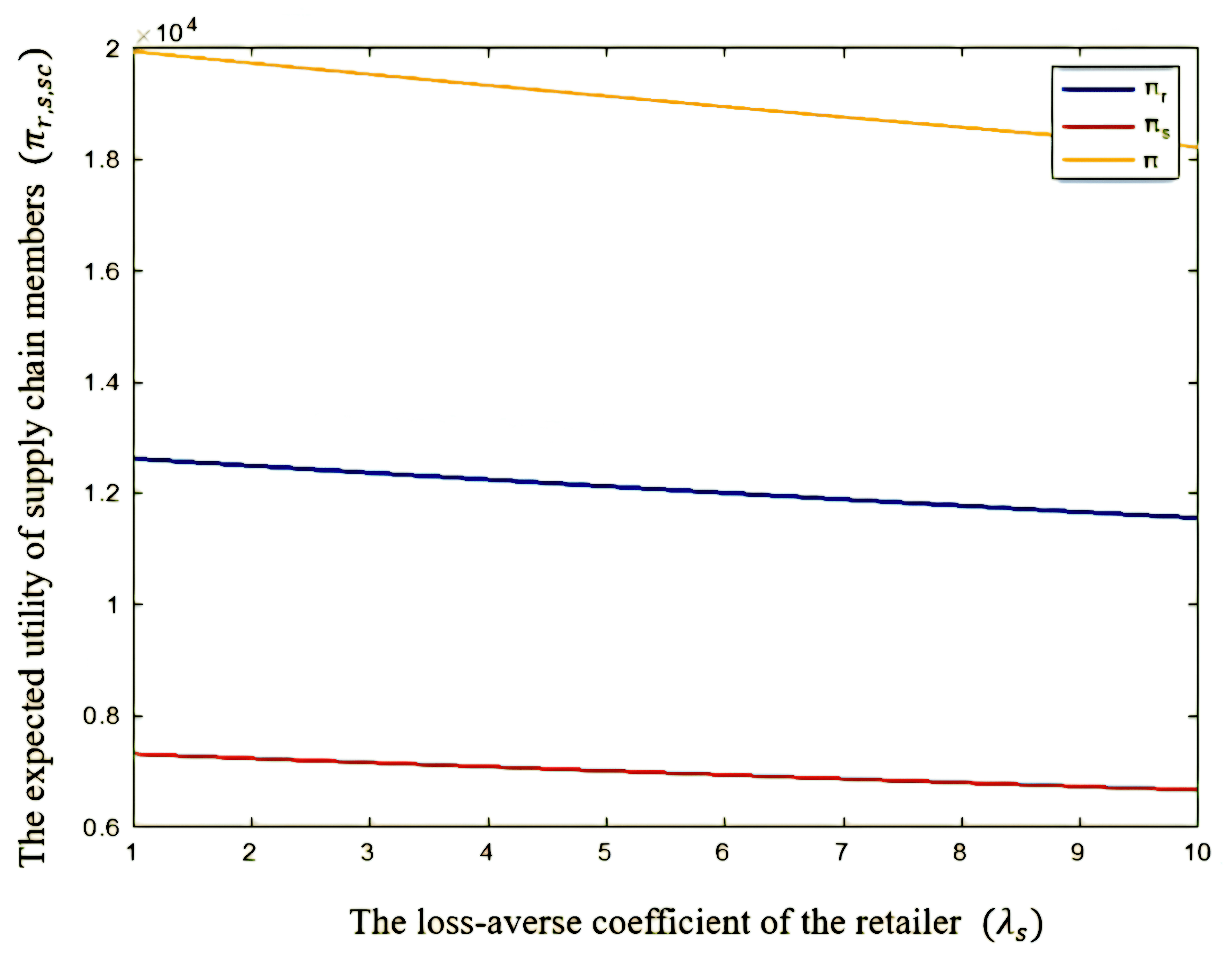
5.3. Numerical Analysis in the AN Scenario
6. Conclusions
6.1. Results Summary
6.2. Managerial Implications
6.3. Limitations and Further Study
- Considering more complex network-like supply chain structures.
- Comparing the impact of different loss avoidance methods on the supply chain system, other behavioral and preference factors can also be added to separately or jointly affect the revenue-sharing contract mechanism.
- Developing effective measures to address the loss avoidance behavior of enterprises, guiding system members to make optimal decisions.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1
Appendix A.2
Appendix A.3
Appendix A.4
Appendix A.5
Appendix A.6
Appendix A.7
References
- Webb, E.; Hernández-Quevedo, C.; Williams, G.; Scarpetti, G.; Reed, S.; Panteli, D. Providing health services effectively during the first wave of COVID-19: A cross-country comparison on planning services, managing cases, and maintaining essential services. Health Policy 2022, 126, 382–390. [Google Scholar] [CrossRef]
- Sachs, J.D.; Karim, S.S.A.; Aknin, L.; Allen, J.; Brosbøl, K.; Colombo, F.; Barron, G.C.; Espinosa, M.F.; Gaspar, V.; Gaviria, A.; et al. The lancet commission on lessons for the future from the COVID-19 pandemic. Lancet 2022, 400, 1224–1280. [Google Scholar] [CrossRef]
- Akgün, U.; Caffarra, C.; Etro, F.; Stillman, R. On the welfare impact of mergers of complements: Raising rivals’ costs versus elimination of double marginalization. Econ. Lett. 2020, 195, 109429. [Google Scholar] [CrossRef]
- Yang, J.; Fuss, S.; Johansson, D.J.; Azar, C. Investment dynamics in the energy sector under carbon price uncertainty and risk aversion. Energy Clim. Chang. 2023, 4, 100110. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect theory: An analysis of decision under risk. Econometrica 1979, 47, 263–291. [Google Scholar] [CrossRef]
- Liu, Z.; Chen, L.; Li, L.; Zhai, X. Risk hedging in a supply chain: Option vs. price discount. Int. J. Prod. Econ. 2014, 151, 112–120. [Google Scholar] [CrossRef]
- Hu, B.; Meng, C.; Xu, D.; Son, Y.-J. Three-echelon supply chain coordination with a loss-averse retailer and revenue sharing contracts. Int. J. Prod. Econ. 2016, 179, 192–202. [Google Scholar] [CrossRef]
- Bai, T.; Wu, M.; Zhu, S.X. Pricing and ordering by a loss averse newsvendor with reference dependence. Transp. Res. Part Logist. Transp. Rev. 2019, 131, 343–365. [Google Scholar] [CrossRef]
- Zhang, S.; Tong, X.; Jin, X. Contract design and comparison under the opportunity cost of working capital: Buyback vs. revenue sharing. Eur. J. Oper. Res. 2023, 309, 845–856. [Google Scholar] [CrossRef]
- Avinadav, T.; Chernonog, T.; Meilijson, I.; Perlman, Y. A consignment contract with revenue sharing between an app developer and a distribution platform. Int. J. Prod. Econ. 2022, 243, 108322. [Google Scholar] [CrossRef]
- Zhai, M.; Wang, X.; Zhao, X. The importance of online customer reviews characteristics on remanufactured product sales: Evidence from the mobile phone market on amazon.com. J. Retail. Consum. Serv. 2024, 77, 103677. [Google Scholar] [CrossRef]
- Jammernegg, W.; Kischka, P.; Silbermayr, L. Risk preferences, newsvendor orders and supply chain coordination using the mean-cvar model. Int. J. Prod. Econ. 2024, 270, 109171. [Google Scholar] [CrossRef]
- Liu, Y.; Tian, J.; Yu, N. Emergency supplies prepositioning via a government-led supply chain with a loss-averse supplier with anchoring. Comput. Ind. Eng. 2023, 182, 109362. [Google Scholar] [CrossRef]
- Yi, T.; Meiping, W.; Shaorui, Z. Pricing and contract preference in maritime supply chains with downstream competition impact of risk-aversion and contract unobservability. Ocean Coast. Manag. 2023, 242, 106691. [Google Scholar] [CrossRef]
- Liu, Z.; Hua, S.; Zhai, X. Supply chain coordination with risk-averse retailer and option contract: Supplier-led vs. retailer-led. Int. J. Prod. Econ. 2020, 223, 107518. [Google Scholar] [CrossRef]
- Zhang, J.; Sethi, S.P.; Choi, T.-M.; Cheng, T. Pareto optimality and contract dependence in supply chain coordination with risk-averse agents. Prod. Oper. Manag. 2022, 31, 2557–2570. [Google Scholar] [CrossRef]
- Barnes-Schuster, D.; Bassok, Y.; Anupindi, R. Coordination and flexibility in supply contracts with options. Manuf. Serv. Oper. Manag. 2002, 4, 171–207. [Google Scholar] [CrossRef]
- Malone, T.W. Modeling coordination in organizations and markets. In Readings in Distributed Artificial Intelligence; Elsevier: Amsterdam, The Netherlands, 1988; pp. 151–158. [Google Scholar]
- Arrow, K.J.; Harris, T.; Marschak, J. Optimal inventory policy. Econom. J. Econom. Soc. 1951, 19, 250–272. [Google Scholar] [CrossRef]
- Bernstein, F.; Federgruen, A. Decentralized supply chains with competing retailers under demand uncertainty. Manag. Sci. 2005, 51, 18–29. [Google Scholar] [CrossRef]
- Edgeworth, F.Y. The mathematical theory of banking. J. R. Stat. Soc. 1888, 51, 113–127. [Google Scholar]
- Giannoccaro, I.; Pontrandolfo, P. Supply chain coordination by revenue sharing contracts. Int. J. Prod. Econ. 2004, 89, 131–139. [Google Scholar] [CrossRef]
- Li, Y.; Wu, D.; Dolgui, A. Optimal trade credit coordination policy in dual-channel supply chain with consumer transfer. Int. J. Prod. Res. 2022, 60, 4641–4653. [Google Scholar] [CrossRef]
- Pasternack, B.A. Optimal pricing and return policies for perishable commodities. Mark. Sci. 1985, 4, 166–176. [Google Scholar] [CrossRef]
- Corbett, C.J.; DeGroote, X. A supplier’s optimal quantity discount policy under asymmetric information. Manag. Sci. 2000, 46, 444–450. [Google Scholar] [CrossRef]
- Dana, J.D., Jr.; Spier, K.E. Revenue sharing and vertical control in the video rental industry. J. Ind. Econ. 2001, 49, 223–245. [Google Scholar] [CrossRef]
- Hou, J.; Zeng, A.Z.; Zhao, L. Coordination with a backup supplier through buy-back contract under supply disruption. Transp. Res. Part Logist. Transp. Rev. 2010, 46, 881–895. [Google Scholar] [CrossRef]
- Zhang, Y.; Donohue, K.; Cui, T.H. Contract preferences and performance for the loss-averse supplier: Buyback vs. revenue sharing. Manag. Sci. 2016, 62, 1734–1754. [Google Scholar] [CrossRef]
- Du, N.; Yan, Y.; Qin, Z. Analysis of financing strategy in coopetition supply chain with opportunity cost. Eur. J. Oper. Res. 2023, 305, 85–100. [Google Scholar] [CrossRef]
- Liu, H.; Yan, Q. Revenue-sharing contract in a three-tier online supply chain under eb platform financing. Financ. Res. Lett. 2024, 59, 104773. [Google Scholar] [CrossRef]
- Simon, H.A. Rational choice and the structure of the environment. Psychol. Rev. 1956, 63, 129. [Google Scholar] [CrossRef]
- Zhang, L.; Song, S.; Wu, C. Supply chain coordination of loss-averse newsvendor with contract. Tsinghua Sci. Technol. 2005, 10, 133–140. [Google Scholar] [CrossRef]
- Shen, H.; Pang, Z.; Cheng, T. The component procurement problem for the loss-averse manufacturer with spot purchase. Int. J. Prod. Econ. 2011, 132, 146–153. [Google Scholar] [CrossRef]
- Ma, L.; Zhao, Y.; Xue, W.; Cheng, T.; Yan, H. Loss-averse newsvendor model with two ordering opportunities and market information updating. Int. J. Prod. Econ. 2012, 140, 912–921. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, Q.; Chen, X.; Wang, Z. Stackelberg game of buyback policy in supply chain with a risk-averse retailer and a risk-averse supplier based on cvar. PLoS ONE 2014, 9, e104576. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Hou, P.-W.; Chen, P.; Li, Q.-H. Pricing strategy and coordination in a dual channel supply chain with a risk-averse retailer. Int. J. Prod. Econ. 2016, 178, 154–168. [Google Scholar] [CrossRef]
- Vipin, B.; Amit, R. Loss aversion and rationality in the newsvendor problem under recourse option. Eur. J. Oper. Res. 2017, 261, 563–571. [Google Scholar] [CrossRef]
- Zhu, B.; Wen, B.; Ji, S.; Qiu, R. Coordinating a dual-channel supply chain with conditional value-at-risk under uncertainties of yield and demand. Comput. Ind. Eng. 2020, 139, 106181. [Google Scholar] [CrossRef]
- Bai, Q.; Xu, J.; Chauhan, S.S. Effects of sustainability investment and risk aversion on a two-stage supply chain coordination under a carbon tax policy. Comput. Ind. Eng. 2020, 142, 106324. [Google Scholar] [CrossRef]
- Peng, Q.; Wang, C.; Goh, M. Green innovation decision and coordination of supply chain under corporate social responsibility and risk preferences. Comput. Ind. Eng. 2023, 185, 109703. [Google Scholar] [CrossRef]
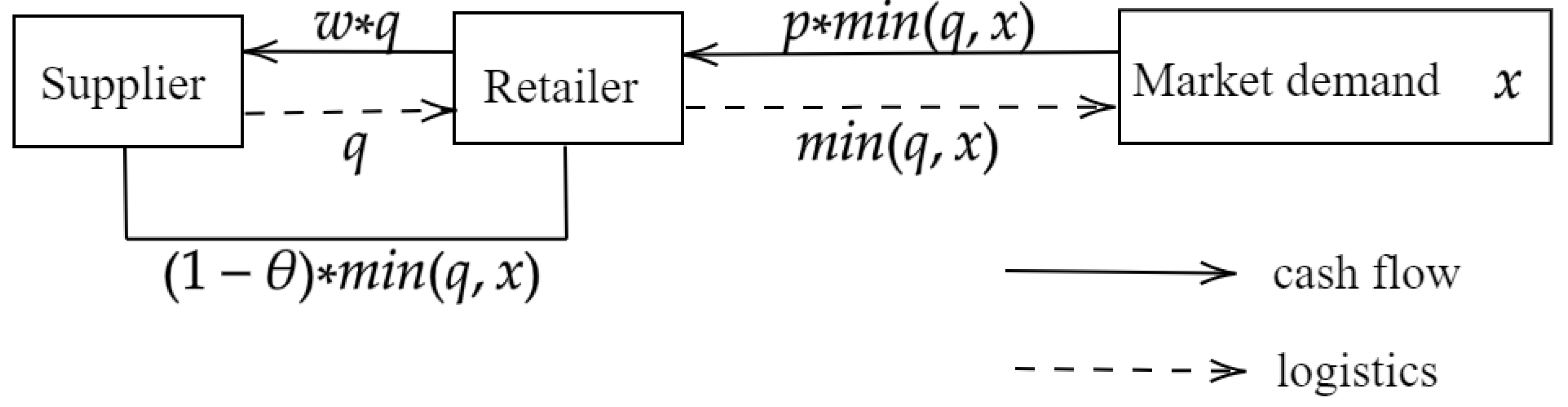


| Paper | Supply Chain Coordination | Revenue-Sharing Contract | Loss-Averse Supplier | Loss-Averse Retailer | Optimal Wholesale Price | Optimal Order Quantity |
|---|---|---|---|---|---|---|
| Zhou et al. (2014) [35] | ✓ | ✓ | ✓ | ✓ | ||
| Hu et al. (2016) [7] | ✓ | ✓ | ✓ | ✓ | ||
| Li et al. (2016) [36] | ✓ | ✓ | ✓ | |||
| Liu et al. (2020) [15] | ✓ | ✓ | ✓ | |||
| Bai et al. (2020) [39] | ✓ | ✓ | ✓ | ✓ | ||
| Zhang et al. (2022) [16] | ✓ | ✓ | ✓ | ✓ | ||
| Liu et al. (2023) [13] | ✓ | ✓ | ✓ | |||
| Yi et al. (2023) [14] | ✓ | ✓ | ||||
| This paper | ✓ | ✓ | ✓ | ✓ | ✓ | ✓ |
| Symbol | Description |
|---|---|
| Parameters: | |
| x | The market demand |
| The cumulative distribution function of demand | |
| The probability density function of demand | |
| c | Unit production cost of goods |
| p | Unit sales price of goods |
| v | Unit salvage value |
| Revenue share for the retailer | |
| Loss avoidance coefficient, i = r, s represents retailer, supplier, respectively | |
| Decision variables: | |
| w | Wholesale price per unit of goods |
| q | Order quantity of products |
| Revenue functions: | |
| The revenue of the retailer | |
| The revenue of the supplier | |
| Total revenue of the supply chain |
| 1 | 330 | 330 | 16,090.7 | 5337.8 | 21,428.5 |
| 2 | 307 | 307 | 14,854 | 4935 | 19,758 |
| 3 | 286 | 286 | 13,806 | 4581 | 18,387 |
| 4 | 269 | 269 | 12,894.3 | 4291.4 | 17,170.7 |
| 1 | 317 | 317 | 7331.1 | 12,781.6 | 20,112.7 |
| 2 | 302 | 302 | 7185.4 | 12,437.6 | 19,623 |
| 3 | 284 | 284 | 7024.1 | 12,174.6 | 19,198.7 |
| 4 | 263 | 263 | 6890.3 | 11,940.8 | 18,831.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, M.; Li, X.; Chen, Y. Loss-Averse Supply Chain Coordination with a Revenue-Sharing Contract. Mathematics 2024, 12, 844. https://doi.org/10.3390/math12060844
Wu M, Li X, Chen Y. Loss-Averse Supply Chain Coordination with a Revenue-Sharing Contract. Mathematics. 2024; 12(6):844. https://doi.org/10.3390/math12060844
Chicago/Turabian StyleWu, Ming, Xin Li, and Yuhao Chen. 2024. "Loss-Averse Supply Chain Coordination with a Revenue-Sharing Contract" Mathematics 12, no. 6: 844. https://doi.org/10.3390/math12060844
APA StyleWu, M., Li, X., & Chen, Y. (2024). Loss-Averse Supply Chain Coordination with a Revenue-Sharing Contract. Mathematics, 12(6), 844. https://doi.org/10.3390/math12060844






