Maxima of the A_α-Index of Non-Bipartite C₃-Free Graphs for 1/2 < α < 1

Abstract

In 2017, Nikiforov defined the $A_{\alpha }$-matrix of the graph G as $A_{\alpha }\left(G\right)=\alpha D\left(G\right)+(1-\alpha )A\left(G\right),0\le \alpha \le 1,$ which merges the diagonal degree matrix $D\left(G\right)$ and the adjacency matrix $A\left(G\right)$. In this paper, we characterize the graphs which attain the maximum $A_{\alpha }$-index among triangle-free non-bipartite graphs of order n for $1/2<\alpha <1$.

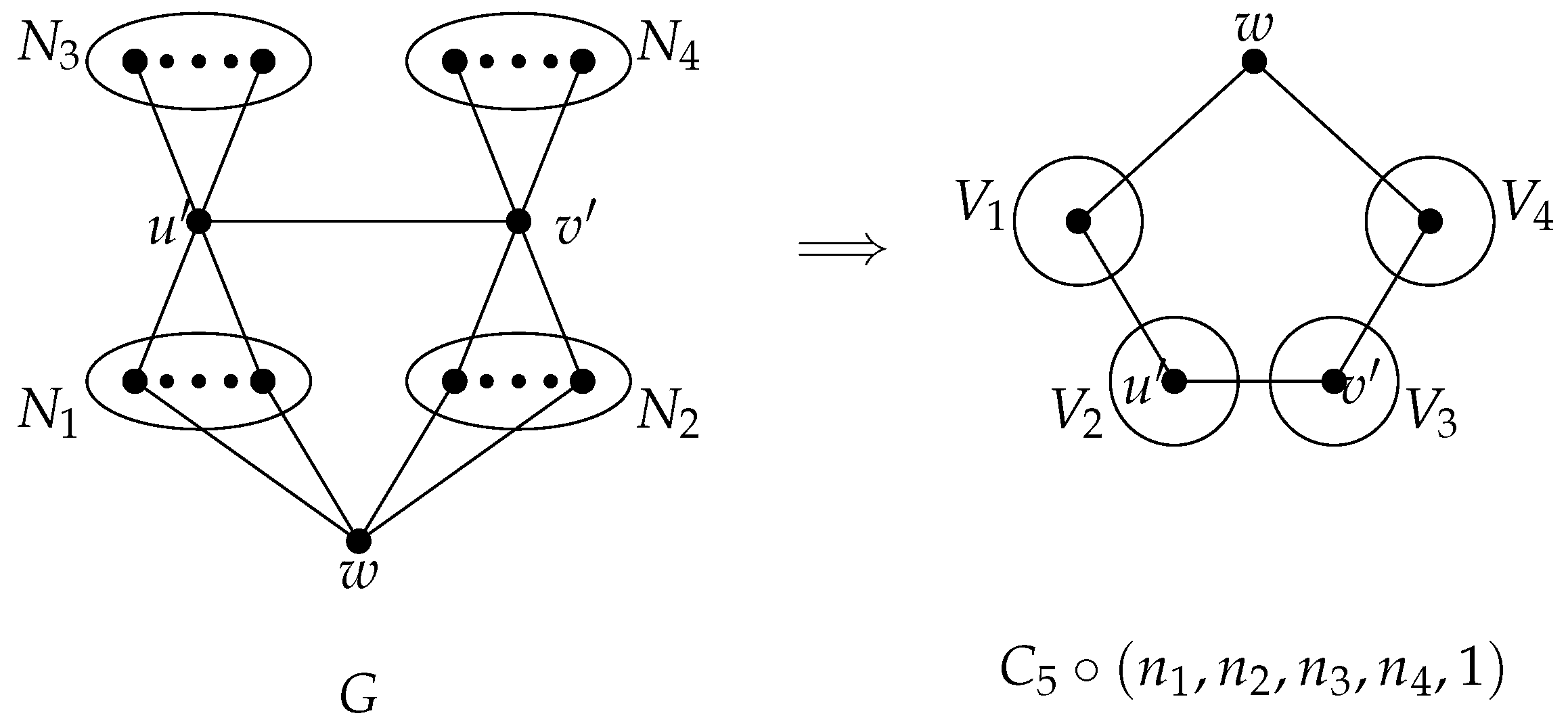
1. Introduction

Let $A\left(G\right)$ be the adjacency matrix and $D\left(G\right)$ be the diagonal degree matrix of a graph G. The matrix $A_{\alpha }\left(G\right)$ is defined in [1]

as

$$A_{\alpha }\left(G\right)=\alpha D\left(G\right)+(1-\alpha )A\left(G\right),0\le \alpha \le 1.$$

(1)

Obviously,

$$A\left(G\right)=A_0\left(G\right),Q\left(G\right)=2A_{1/2}\left(G\right),$$

where $Q\left(G\right)$ is the signless Laplacian matrix. Hence, the study of the family $A_{\alpha }\left(G\right)$ provides a new light for seeing the matrices $A\left(G\right)$ and $Q\left(G\right)$.

The largest eigenvalue of $A_{\alpha }\left(G\right)$, denoted by ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$, is called the $A_{\alpha }$ spectral radius or the $A_{\alpha }$-$index$ of G. For $0\le \alpha <1$, we know that $A_{\alpha }\left(G\right)$ is non-negative. From the Perron–Frobenius theorem, ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ has a positive unit eigenvector for every connected graph G, which is called the $Perron$$vector$ of $A_{\alpha }\left(G\right)$. Obviously, the $A_{\alpha }$-index does not change when G has isolated vertices. Therefore, in this paper, we only consider graphs without isolated vertices.

Let G be a graph with the vertex set $V\left(G\right)$ and the edge set $E\left(G\right)$. Let $n=\left|V\right(G\left)\right|$ and $m=\left|E\right(G\left)\right|$ be the order and the size of G, respectively. If H is not a subgraph of G, the graph G is called H-$free$. The Turán-type extremal problem is posed as follows (see [2]): what is the maximum size m of an H-free graph with the order n?

A spectral version of the Turán-type problem as shown in Problem 1 was formally posed by Nikiforov.

Problem 1

([3]). What is the maximum spectral radius of an H-free graph of order n or size m?

On the other hand, Freitas et al. [4] proposed a Q-spectral version of the Turán-type problem.

Problem 2

([4]). What is the maximum Q-index among all the H-free graphs with given order n or size m?

In the past two decades, much attention has been paid to Problems 1 and 2 for some specific graph H, such as the cycle $C_4$ [5], the cycle $C_6$ [6], even cycles [7], odd cycles [8], and $K_{r+1}$ [9,10]; other results are shown in related references ([11,12,13,14,15,16]).

Let H be a graph with the vertex set $V\left(H\right)=\{v_1,v_2,\dots ,v_k\}$ and $r=(n_1,n_2,\dots ,n_k)$, $n_i\ge 1$, $i=1,\dots ,k$. Replacing each vertex $v_i$ of H with a graph $n_i{K}_1$ (an independent set with $n_i$ vertices) and joining every vertex between $n_i{K}_1$ and $n_j{K}_1$ for $v_i{v}_j\in E\left(H\right)$, we obtain a new graph $H\circ r$, which is called a blow-up of H ([13]). For example, when $H\cong C_5$ with the vertex set $V\left(C_5\right)=\{v_1,v_2,v_3,v_4,,v_5\}$ and $r=(n-4,1,1,1,1)$, the graph $C_5\circ (n-4,1,1,1,1)$ is obtained, as shown in Figure 1.

Recently, Liu et al. [13] obtained the maximum Q-index ($q\left(G\right)$) in non-bipartite $C_3$-free graphs with n vertices.

Theorem 1

([13]). Let G be a non-bipartite graph of order n. If

$$q\left(G\right)\ge q(C_5\circ (n-4,1,1,1,1)),$$

then

G contains a triangle unless $G\cong C_5\circ (n-4,1,1,1,1)$.

Now, having the family $A_{\alpha }\left(G\right)$, Nikiforov [1] merged Problems 1 and 2 into one, namely, the $A_{\alpha }$-spectral version of the Turán-type problem.

Problem 3

([1]). Given a graph H, what is the maximum ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ of a graph G of order n or size m, with no subgraph isomorphic to H?

Nikiforov [1] also obtained the extremal graph in $K_{r+1}$-free ($r\ge 2$) graphs of order n for $0\le \alpha <1$. Inspired by Theorem 1 and the further discussion about the $A_{\alpha }$-spectral Turán-type problem, in this paper, we shall solve Problem 3 in non-bipartite triangle-free graphs of order n for $1/2<\alpha <1$.

Theorem 2.

Let G be a $C_3$-free non-bipartite graph of order n, $1/2<\alpha <1$. Then,

$${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le {\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right).$$

The equality holds if and only if $G\cong C_5\circ (n-4,1,1,1,1)$.

From Theorems 1 and 2, the extremal graph for Q-index is the same as that for $A_{\alpha }$-index ($1/2<\alpha <1$) among non-bipartite triangle-free graphs of order n.

2. Some Lemmas

We will give some useful lemmas in this section.

Let M be a real $n\times n$ matrix and G be a graph with n vertices. Given a partition $\Pi$: $V\left(G\right)=V_1\cup V_2\cup \cdots \cup V_k$, M can be correspondingly partitioned as

$$M=\left(\begin{array}{cccc}{M}_{11}& M_{12}& \cdots & M_{1k}\\ M_{21}& M_{22}& \cdots & M_{2k}\\ ⋮& ⋮& \ddots & ⋮\\ M_{k1}& M_{k2}& \cdots & M_{kk}\end{array}\right).$$

The quotient matrix of M with respect to $\Pi$ is defined as the $k\times k$ matrix $B_{\Pi }=\left(b_{i,j}\right)$, where $b_{i,j}$ is the average value of all row sums of $M_{i,j}$. Furthermore, if every row sum of $M_{i,j}$ is equal to $b_{i,j}$, the matrix $B_{\Pi }$ is called the equitable quotient matrix.

Lemma 1

([17,18]). Let M be a real symmetric matrix and M have the largest eigenvalue $\lambda \left(M\right)$. Let $B_{\Pi }$ be an equitable quotient matrix of M. Then, the eigenvalues of $B_{\Pi }$ are also eigenvalues of M. Furthermore, if M is non-negative and irreducible, then $\lambda \left(M\right)=\lambda \left(B_{\Pi }\right)$.

Lemma 2

([1]). Let $\alpha \in [0,1)$ and let G be a graph with $A_{\alpha }\left(G\right)=A_{\alpha }$. Let $u,v,w\in V\left(G\right)$ and suppose that $uv\in E\left(G\right)$ and $uw\notin E\left(G\right)$. Let $H=G-uv+uw$ and $x:=(x_1,x_2,\dots ,x_n)$ be a unit eigenvector to ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ such that $x_u>0$ and $\langle A_{\alpha }\left(H\right)x,x\rangle \ge \langle A_{\alpha }\left(G\right)x,x\rangle$. Then, ${\lambda }_1\left(A_{\alpha }\left(H\right)\right)>{\lambda }_1\left(A_{\alpha }\left(G\right)\right)$.

Lemma 3.

Let $\alpha \in [0,1)$ and let G be a connected graph with $A_{\alpha }\left(G\right)=A_{\alpha }$. Let $u,v,w\in V\left(G\right)$ and suppose that $uv\in E\left(G\right)$ and $uw\notin E\left(G\right)$. Let $H=G-uv+uw$ and $x:=(x_1,x_2,\dots ,x_n)$ be the Perron vector of $A_{\alpha }\left(G\right)$ such that $x_w\ge x_v$. Then, ${\lambda }_1\left(A_{\alpha }\left(H\right)\right)>{\lambda }_1\left(A_{\alpha }\left(G\right)\right)$.

Proof. 

Note that

$$\begin{array}{cc}\hfill & \langle A_{\alpha }\left(H\right)x,x\rangle -\langle A_{\alpha }\left(G\right)x,x\rangle \hfill \\ \hfill =& \sum _{uw\in E\left(H\right)}(\alpha x_u^2+2(1-\alpha )x_u{x}_w+\alpha x_w^2)-\sum _{uv\in E\left(G\right)}(\alpha x_u^2+2(1-\alpha )x_u{x}_v+\alpha x_v^2)\hfill \\ \hfill =& (x_w-x_v)[2(1-\alpha )x_u+\alpha (x_v+x_w)].\hfill \end{array}$$

Since G is connected, $x_u,x_w,x_v>0$. And $x_w\ge x_v$ implies that the above equality is not less than 0, that is to say, $\langle A_{\alpha }\left(H\right)x,x\rangle \ge \langle A_{\alpha }\left(G\right)x,x\rangle$. By Lemma 2, the result holds. □

Some degree-based bounds for ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ play very important roles in this survey. We list these bounds as Lemmas 4–6.

Lemma 4

([1]). If G is a graph with no isolated vertices, then

$${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le \underset{u\in V\left(G\right)}{max}\{\alpha d_u+(1-\alpha )m_u\},$$

where $m_u=\frac{1}{d_u}{\sum }_{uv\in E\left(G\right)}{d}_v$. If $1/2<\alpha <1$ and G is connected, the equality holds if and only if G is regular.

Lemma 5

([1]). For any graph G,

$${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le \underset{uv\in E\left(G\right)}{max}\{\alpha d_u+(1-\alpha )d_v\}.$$

Lemma 6

([1]). Let G be a graph with $\Delta \left(G\right)=\Delta$. If $1/2\le \alpha <1$, then

$${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\ge \alpha \Delta +{(1-\alpha )}^2/\alpha .$$

Next, we will discuss the $A_{\alpha }$-index of the graph $C_5\circ (n-4,1,1,1,1)$.

Lemma 7. 

Let ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)$ be the $A_{\alpha }$-index of the graph $C_5\circ (n-4,1,1,1,1)$, $n\ge 5$. Denote ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)$ by ${\lambda }_1$, then

(i) 

${\lambda }_1$ is the largest root of $x^3-(\alpha n+1)x^2+[{\alpha }^{2}n+5\alpha (n-3)-(2n-7)]x-2{\alpha }^2(2n-5)-2\alpha (n-5)+2(n-4)=0$;

(ii) 

$\alpha (n-3)+{(1-\alpha )}^2/\alpha \le {\lambda }_1$ for $1/2<\alpha <1$;

(iii) 

$\alpha (n-3)+(1-\alpha )<{\lambda }_1$ for $1/2<\alpha \le 2/3$;

(iv) 

$\alpha (n-2)<{\lambda }_1$ for $n\ge 6$ and $1/2<\alpha \le 2-\sqrt{2}$.

Proof. 

(i) The vertex set of $C_5\circ (n-4,1,1,1,1)$ can be partitioned as $\Pi :V_1\cup V_2\cup V_3$, where $V_2=\{v_2,v_5\}$, $V_3=\{v_3,v_4\}$ and $V_1=V(C_5\circ (n-4,1,1,1,1))\setminus \{V_2\cup V_3$} (see Figure 1). The equitable quotient matrix $B_{\Pi }$ of the matrix $A_{\alpha }(C_5\circ (n-4,1,1,1,1))$ is as follows:

$$B_{\Pi }=\left(\begin{array}{ccc}2\alpha & 2(1-\alpha )& 0\\ (n-4)(1-\alpha )& \alpha (n-3)& 1-\alpha \\ 0& 1-\alpha & 1+\alpha \end{array}\right).$$

Then, the characteristic polynomial of $B_{\Pi }$ is

$$\begin{array}{cc}\hfill f\left(x\right)=& |x{I}_3-B_{\Pi }|\hfill \\ \hfill =& x^3-(\alpha n+1)x^2+[{\alpha }^{2}n+5\alpha (n-3)-(2n-7)]x\hfill \\ \hfill & -2{\alpha }^2(2n-5)-2\alpha (n-5)+2(n-4).\hfill \end{array}$$

(2)

By Lemma 1, ${\lambda }_1$ is the largest real zero of $f\left(x\right)$. Hence, the result $\left(i\right)$ follows.

(ii) Since $\Delta (C_5\circ (n-4,1,1,1,1))=n-3$, Lemma 6 implies that ${\lambda }_1\ge \alpha (n-3)+{(1-\alpha )}^2/\alpha$ for $1/2<\alpha <1$.

(iii) Suppose that ${\lambda }_1=\alpha (n-3)+t$, then $t>0$ by Lemma 6. And we have $f\left(\alpha \right(n-3)+t)=0$ (for convenience, set $g\left(t\right)=f\left(\alpha \right(n-3)+t)$), i.e.,

$$\begin{array}{cc}\hfill g\left(t\right)=& t^3+t^2[\alpha (2n-9)-1]+t[{\alpha }^2(n^2-11n+27)+3\alpha (n-3)-(2n-7)]\hfill \\ \hfill & +{\alpha }^3(-2n^2+15n-27)+{\alpha }^2(4n^2-28n+46)\hfill \\ \hfill & -\alpha (2n^2-11n+11)+2(n-4)=0.\hfill \end{array}$$

After some algebra, we see that

$$\begin{array}{cc}\hfill g(1-\alpha )=& {\alpha }^3(-3n^2+28n-64)+{\alpha }^2(5n^2-46n+102)\hfill \\ \hfill & +\alpha (-2n^2+18n-37)-1\hfill \\ \hfill =& (1-\alpha )g_1\left(\alpha \right),\hfill \end{array}$$

where $g_1\left(\alpha \right)={\alpha }^2(3n^2-28n+64)-2\alpha (n^2-9n+19)-1$.

Next, we estimate the value $g(1-\alpha )$ in the case $1/2<\alpha \le 2/3$. If $n=5$, then $g_1\left(\alpha \right)=-{(1-\alpha )}^2<0$; if $n\ge 6$, $g_1\left(\alpha \right)$ is increasing for $1/2<\alpha \le 2/3$ and $g_1(2/3)=-\frac{1}{9}(4n-19)<0$. Thus, $g(1-\alpha )<0$. Furthermore, Lemma 5 implies that $t\le 2(1-\alpha )$, so $g\left(2\right(1-\alpha \left)\right)\ge 0$. Hence, $t>1-\alpha$ and ${\lambda }_1>\alpha (n-3)+(1-\alpha )$ for $1/2<\alpha \le 2/3$.

(iv) Note that $g\left(\alpha \right)=-(n-4)h\left(\alpha \right)$, where

$$\begin{array}{c}\hfill h\left(\alpha \right)={\alpha }^3(n-2)-{\alpha }^2(4n-9)+\alpha (2n-1)-2.\end{array}$$

It is easy to see that $h^{″}\left(\alpha \right)=6\alpha (n-2)-2(4n-9)<0$ for $1/2<\alpha \le 2-\sqrt{2}$. Thus, $h^{\prime }\left(\alpha \right)$ is decreasing and $h^{\prime }\left(\alpha \right)<h^{\prime }(1/2)=-\frac{1}{4}(5n-26)<0$ for $n\ge 6$. This implies that $h\left(\alpha \right)$ is decreasing and $h\left(\alpha \right)\ge h(2-\sqrt{2})=10-7\sqrt{2}>0$. Hence, $g\left(\alpha \right)<0$, combined with $g\left(2\right(1-\alpha \left)\right)\ge 0$, yields ${\lambda }_1>\alpha (n-2)$ for $1/2<\alpha \le 2-\sqrt{2}$. □

3. The ${\mathit{A}}_{\mathit{\alpha }}$-Index of ${\mathit{C}}_{\mathbf{5}}\circ ({\mathit{n}}_{\mathbf{1}},{\mathit{n}}_{\mathbf{2}},{\mathit{n}}_{\mathbf{3}},{\mathit{n}}_{\mathbf{4}},\mathbf{1})$

Throughout this section, $\alpha$ belongs to the interval $(1/2,1)$. Assume that G has the maximum ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ among all non-bipartite $C_3$-free graphs of order n. We will prove the following result for the extremal graph G.

Lemma 8.

Let G be a graph with the maximum $A_{\alpha }$-index among all non-bipartite $C_3$-free graphs of order n. Then, $G\cong C_5\circ (n_1,n_2,n_3,n_4,1)$.

Proof. 

Since G is $C_3$-free and non-bipartite, $n\ge 5$ holds. In fact, G must be connected. Otherwise, suppose that $G_1$ is a connected component of G with ${\lambda }_1\left(A_{\alpha }\left(G_1\right)\right)={\lambda }_1\left(A_{\alpha }\left(G\right)\right)$. Let $G_2=G_1+e$, where e is an edge by joining a vertex of $G_1$ to a vertex of any connected component of G. Obviously, $G_2$ is still non-bipartite and triangle-free. However, ${\lambda }_1\left(A_{\alpha }\left(G_2\right)\right)>{\lambda }_1\left(A_{\alpha }\left(G_1\right)\right)={\lambda }_1\left(A_{\alpha }\left(G\right)\right)$, a contradiction.

Let $u^{\prime }{v}^{\prime }$ be an edge of G with $\alpha d\left(u^{\prime }\right)+(1-\alpha )d\left(v^{\prime }\right)=max\{\alpha d\left(u\right)+(1-\alpha )d\left(v\right):uv\in E\left(G\right)\}$. Suppose that $d\left(u^{\prime }\right)+d\left(v^{\prime }\right)=t$, $d\left(u^{\prime }\right)=x$ and

$$b\left(x\right)=\alpha x+(1-\alpha )(t-x)=(2\alpha -1)x+(1-\alpha )t.$$

Since G is connected, $d\left(u^{\prime }\right)\ge 1$ and $d\left(v^{\prime }\right)\ge 1$.

Case 1. $d\left(v^{\prime }\right)=1$.

Obviously, $d\left(u^{\prime }\right)=t-1$ and $b(t-1)=\alpha (t-2)+1$. If $t\le n-3$, we see that $b(t-1)\le \alpha (n-5)+1<\alpha (n-3)$ for $1/2<\alpha <1$. From $\left(ii\right)$ of Lemmas 5 and 7, we obtain

$$\alpha (n-3)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\le {\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le b(t-1)<\alpha (n-3),$$

a contradiction. Hence, $t\ge n-2$.

Since G is $C_3$-free, there are no common neighbors between $u^{\prime }$ and $v^{\prime }$, which implies that $t\le n$. If $t=n-1$ or n, we easily know that G is a bipartite graph, a contradiction. If $t=n-2$, there exists two vertices $w_1$ and $w_2$ of G such that $w_1,w_2\notin N\left(u^{\prime }\right)\cup N\left(v^{\prime }\right)$. When $w_1$ is not adjacent to $w_2$, G is a bipartite graph, a contradiction. Hence, $w_1$ is adjacent to $w_2$. Whether $N\left(w_1\right)=\left\{w_2\right\}$ or $N\left(w_2\right)=\left\{w_1\right\}$, G is still bipartite, a contradiction. Hence, $N\left(w_1\right)\setminus \left\{w_2\right\}\ne \varnothing$, $N\left(w_2\right)\setminus \left\{w_1\right\}\ne \varnothing$ and $N\left(w_1\right)\cup N\left(w_2\right)\setminus \{w_1,w_2\}\subseteq N\left(u^{\prime }\right)\setminus \left\{v^{\prime }\right\}$. Suppose that there is a vertex $w\in N\left(u^{\prime }\right)\setminus \left\{v^{\prime }\right\}$ and $w\notin N\left(w_1\right)\cup N\left(w_2\right)\setminus \{w_1,w_2\}$, $G+w_{1}w$ is still non-bipartite triangle-free. However, ${\lambda }_1\left(A_{\alpha }(G+w_{1}w)\right)>{\lambda }_1\left(A_{\alpha }\left(G\right)\right)$, contrary to the assumption that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ is maximal. Therefore, G is the graph as shown in Figure 2. In fact, $G\cong C_5\circ (n_1,1,1,n_4,1)+u^{\prime }{v}^{\prime }$, $n_1+n_4=n-4$. Furthermore, $C_5\circ (n_1,1,1,n_4,1)+u^{\prime }{v}^{\prime }+v^{\prime }{w}_2\cong C_5\circ (n_1,1,1,n_4+1,1)$. Thus, ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,1,1,n_4+1,1))\right)$, a contradiction.

Case 2. $d\left(v^{\prime }\right)\ge 2$.

Obviously, $b\left(x\right)\le b(t-2)=\alpha (t-4)+2$. If $t\le n-2$, we have $b(t-2)\le \alpha (n-6)+2<\alpha (n-3)$ for $2/3<\alpha <1$. Thus, from $\left(ii\right)$ of Lemmas 5 and 7, we obtain

$$\alpha (n-3)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\le {\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le b(t-2)<\alpha (n-3),$$

a contradiction. Furthermore, $b(t-2)\le \alpha (n-6)+2<\alpha (n-3)+(1-\alpha )$ for $1/2<\alpha \le 2/3$. Applying $\left(iii\right)$ of Lemmas 5 and 7, we find that

$$\begin{array}{cc}\hfill \alpha (n-3)+(1-\alpha )& <{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\hfill \\ \hfill & \le {\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le b(t-2)<\alpha (n-3)+(1-\alpha ),\hfill \end{array}$$

a contradiction.

Thus, $t\ge n-1$ holds. The graph G is still a bipartite graph for $t=n$, a contradiction. Hence, $t=n-1$, namely, there is a vertex w of G such that $w\notin N\left(u^{\prime }\right)\cup N\left(v^{\prime }\right)$ (see Figure 3). The remaining part of the proof uses an idea borrowed from [13].

Define $N_1=\{v\in N\left(u^{\prime }\right):vw\in E\left(G\right)\}$, $N_2=\{v\in N\left(v^{\prime }\right):vw\in E\left(G\right)\}$, $N_3=N\left(u^{\prime }\right)\setminus (N_1\cup \left\{v^{\prime }\right\})$ and $N_4=N\left(v^{\prime }\right)\setminus (N_2\cup \left\{u^{\prime }\right\})$. Note that G is non-bipartite, we can obtain that $N_1\ne \varnothing$ and $N_2\ne \varnothing$. Furthermore, since G is triangle-free, both $N_1$ and $N_4$ are independent sets. And $G[N_1\cup N_4]$ is a complete bipartite graph. Suppose that there exist two vertices $v_1\in N_1$ and $v_2\in N_4$ such that $v_1{v}_2\notin E\left(G\right)$. Then, $G+v_1{v}_2$ is still non-bipartite triangle-free. However, ${\lambda }_1\left(A_{\alpha }(G+v_1{v}_2)\right)>{\lambda }_1\left(A_{\alpha }\left(G\right)\right)$, a contradiction. By the same analysis, $G[N_2\cup N_3]$ and $G[N_3\cup N_4]$ are also complete bipartite graphs (see Figure 3). Let $V_1=N_1$, $V_2=\left\{u^{\prime }\right\}\cup N_4$, $V_3=\left\{v^{\prime }\right\}\cup N_3$ and $V_4=N_2$. Then, ${\bigcup }_{i=1}^4{V}_i=V\left(G\right)\setminus \left\{w\right\}$. Set $n_i=\left|V_i\right|$, $i=1,\dots ,4$. Obviously, ${\sum }_{i=1}^4{n}_i=n-1$. Recall that $N_1\ne \varnothing$, $u^{\prime }\in V_2$, $v^{\prime }\in V_3$ and $N_2\ne \varnothing$. Hence, we have $n_i\ge 1$, where $1\le i\le 4$. It is easy to see that $G\cong C_5\circ (n_1,n_2,n_3,n_4,1)$ (see Figure 3). □

Next, we shall consider the $A_{\alpha }$-index of the graph $C_5\circ (n_1,n_2,n_3,n_4,1)$.

Lemma 9.

Let ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)$ be the $A_{\alpha }$-index of $C_5\circ (n_1,n_2,n_3,n_4,1)$, then ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)$ is the largest root of $c(n_1,n_2,n_3,n_4;x)=0$.

Proof. 

We partition the vertex set of $C_5\circ (n_1,n_2,n_3,n_4,1)$ as $\Pi :V_1\cup V_2\cup V_3\cup V_4\cup \left\{w\right\}$, (see Figure 3). The quotient matrix $B_{\Pi }$ of the matrix $A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))$ with respect to the partition $\Pi$ is as follows:

$$B_{\Pi }=\left(\begin{array}{ccccc}\alpha (n_1+n_4)& (1-\alpha )n_1& 0& 0& (1-\alpha )n_4\\ 1-\alpha & \alpha (1+n_2)& (1-\alpha )n_2& 0& 0\\ 0& (1-\alpha )n_1& \alpha (n_1+n_3)& (1-\alpha )n_3& 0\\ 0& 0& (1-\alpha )n_2& \alpha (n_2+n_4)& (1-\alpha )n_4\\ 1-\alpha & 0& 0& (1-\alpha )n_3& \alpha (1+n_3)\end{array}\right).$$

By computation, the characteristic polynomial of the quotient matrix $B_{\Pi }$ is

$$\begin{array}{cc}\hfill & c(n_1,n_2,n_3,n_4;x)=|x{I}_5-B_{\Pi }|\hfill \\ \hfill =& [x-\alpha (n_1+n_4)]d\left(x\right)-{(1-\alpha )}^2[e\left(x\right)+h\left(x\right)]+{(1-\alpha )}^{4}k\left(x\right),\hfill \end{array}$$

(3)

where

$$\begin{array}{cc}\hfill d\left(x\right)=& [x^2-\alpha (n_1+n_2+n_3+1)x+{\alpha }^2(1+n_2)(n_1+n_3)-{(1-\alpha )}^2{n}_1{n}_2]\hfill \\ \hfill & ·[x^2-\alpha (n_2+n_3+n_4+1)x+{\alpha }^2(1+n_3)(n_2+n_4)-{(1-\alpha )}^2{n}_3{n}_4],\hfill \\ \hfill e\left(x\right)=& n_2{n}_3[x-\alpha (n_1+n_4)][x-\alpha (1+n_2)][x-\alpha (1+n_3)],\hfill \\ \hfill h\left(x\right)=& [(n_1+n_4)x-\alpha (n_1+n_4)-\alpha (n_1{n}_3+n_2{n}_4)]\hfill \\ \hfill & ·[x^2-\alpha (n-1)x+{\alpha }^2(n_1{n}_2+n_3{n}_4+n_1{n}_4)+(2\alpha -1)n_2{n}_3],\hfill \\ \hfill k\left(x\right)=& n_1{n}_4[(n_2+n_3)x-\alpha {(n_2-n_3)}^2-\alpha (n_1{n}_3+n_2{n}_4)-2n_2{n}_3].\hfill \end{array}$$

Hence, the result holds. □

In the following, for the graph $C_5\circ (n_1,n_2,n_3,n_4,1)$, we always assume that $n_2\le n-3$. (Otherwise, $C_5\circ (n_1,n_2,n_3,n_4,1)\cong C_5\circ (n_4,n_3,n_2,n_1,1)$, where $n_3<n_2$.) In order to obtain the maximum ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)$, $n_1+n_2+n_3+n_4=n-1$, we will take some steps. First, when $n_1,n_4\ge 2$, ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right)$ has the larger value by Lemma 11 under certain conditions.

Lemma 10.

If $n_1,n_4\ge 2$ and $n_3\ge n_2$,

$$c^{\prime }(n_1,n_2,n_3,n_4;x)-c^{\prime }(n_1+n_4-1,n_2,n_3,1;x)>0$$

holds for $x>\alpha (n-3)$.

Proof. 

Through a direct calculation by (3), we have

$$\begin{array}{cc}\hfill & c(n_1,n_2,n_3,n_4;x)-c(n_1+n_4-1,n_2,n_3,1;x)\hfill \\ \hfill =& (n_4-1)\{[x-\alpha (n_1+n_4)]d_1\left(x\right)-\alpha {(1-\alpha )}^2{h}_1\left(x\right)+{(1-\alpha )}^4{k}_1\left(x\right)\},\hfill \end{array}$$

(4)

where

$$\begin{array}{cc}\hfill d_1\left(x\right)=& {\alpha }^2(n_1-1)x[x-\alpha (n_2+n_3+2)]\hfill \\ \hfill & +(2\alpha -1)(n_3-n_2)x[x-\alpha (n_2+n_3+1)]\hfill \\ \hfill & +\alpha {(1-\alpha )}^2(n_2+n_3)(n_1-1)x-{\alpha }^3(n_3-n_2)x\hfill \\ \hfill & +(n_1-1)[{\alpha }^4(1+n_2)(1+n_3)-{\alpha }^2{(1-\alpha )}^2(n_2+n_3+2n_2{n}_3)\hfill \\ \hfill & +{(1-\alpha )}^4{n}_2{n}_3]\hfill \\ \hfill & +(n_3-n_2)[{\alpha }^4(1+n_2)(1+n_3)-{\alpha }^2{(1-\alpha )}^2(n_2+n_3+n_2{n}_3)]\hfill \end{array}$$

$$\begin{array}{cc}\hfill h_1\left(x\right)=& \alpha (n_1+n_4)(n_1-1+n_3-n_2)(x-\alpha )\hfill \\ \hfill & +(n_3-n_2)[x^2-\alpha (n-1)x+(2\alpha -1)n_2{n}_3]\hfill \\ \hfill & -{\alpha }^2\{[{(n_3-n_2)}^2+n_2](n_1-1)+n_3{(n_1-1)}^2+(n_1{n}_2-n_3)n_4\},\hfill \\ \hfill k_1\left(x\right)=& (n_1-1)[(n_2+n_3)x-2n_2{n}_3]\hfill \\ \hfill & -\alpha \{[{(n_3-n_2)}^2+n_2](n_1-1)+n_3{(n_1-1)}^2+(n_1{n}_2-n_3)n_4\}.\hfill \end{array}$$

Note that

$$\begin{array}{cc}\hfill & [x-\alpha (n_1+n_4)]d_1^{\prime \prime }\left(x\right)+2d_1^{\prime }\left(x\right)-\alpha {(1-\alpha )}^2{h}_1^{\prime \prime }\left(x\right)+{(1-\alpha )}^4{k}_1^{\prime \prime }\left(x\right)\hfill \\ \hfill =& 2\{[{\alpha }^2(n_1-1)+(2\alpha -1)(n_3-n_2)][x-\alpha (n_1+n_4)]\hfill \\ \hfill & +(2\alpha -1)(n_3-n_2)[2x-\alpha (n_2+n_3+1)]\hfill \\ \hfill & +2{\alpha }^2(n_1-1)x-{\alpha }^3[(n_2+n_3+2)(n_1-1)+(n_3-n_2)]\hfill \\ \hfill & +\alpha {(1-\alpha )}^2[(n_2+n_3)(n_1-1)-(n_3-n_2)]\}\hfill \\ \hfill & >0\hfill \end{array}$$

for $x>\alpha (n-3)$.

Then,

$$d_1\left(x\right)+[x-\alpha (n_1+n_4)]d_1^{\prime }\left(x\right)-\alpha {(1-\alpha )}^2{h}_1^{\prime }\left(x\right)+{(1-\alpha )}^4{k}_1^{\prime }\left(x\right)$$

(5)

is increasing for $x>\alpha (n-3)$. Now, we need to estimate the value $x=\alpha (n-3)$ in (5); first, $d_1^{\prime }\left(\alpha (n-3)\right)$, $h_1^{\prime }\left(\alpha (n-3)\right)$, and $d_1\left(\alpha (n-3)\right)$ are given, respectively.

$$\begin{array}{cc}\hfill & d_1^{\prime }\left(\alpha (n-3)\right)\hfill \\ \hfill =& \alpha (2\alpha -1)(n_3-n_2)(n-6+n_1+n_4)+{\alpha }^3(n_1-1)(n-7+n_1+n_4)\hfill \\ \hfill & +\alpha {(1-\alpha )}^2(n_1-1)(n_3+n_2)-{\alpha }^3(n_3-n_2)\hfill \\ \hfill =& \alpha (2\alpha -1)(n_3-n_2)(n-5+n_4)+{\alpha }^3(n_3-n_2)(n_1-2)(\ge 0)\hfill \\ \hfill & +{\alpha }^3(n_1-1)(n-7+n_1+n_4)+2\alpha {(1-\alpha )}^2(n_1-1)n_2,\hfill \end{array}$$

$$\begin{array}{cc}\hfill & h_1^{\prime }\left(\alpha (n-3)\right)=\alpha (n_1+n_4)(n_1-1+n_3-n_2)+\alpha (n-5)(n_3-n_2),\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill & d_1\left(\alpha (n-3)\right)\hfill \\ \hfill =& {\alpha }^4(n-3)(n_1-1)(n_1+n_4-4)+{\alpha }^2(2\alpha -1)(n-3)(n_3-n_2)(n_1+n_4-3)\hfill \\ \hfill & +{\alpha }^2{(1-\alpha )}^2(n_1-1)(n-3)(n_2+n_3)-{\alpha }^4(n-3)(n_3-n_2)\hfill \\ \hfill & +(n_1-1)[{\alpha }^4+{\alpha }^2(2\alpha -1)(n_2+n_3+n_2{n}_3)-(2\alpha -1){(1-\alpha )}^2{n}_2{n}_3]\hfill \\ \hfill & +(n_3-n_2)[{\alpha }^4+{\alpha }^2(2\alpha -1)(n_2+n_3+n_2{n}_3)].\hfill \end{array}$$

It is easy to see that

$$\begin{array}{cc}\hfill & d_1\left(\alpha (n-3)\right)\hfill \\ \hfill >& {\alpha }^2(2\alpha -1)(n-3)(n_3-n_2)(n_1+n_4-3)\hfill \\ \hfill & +{\alpha }^2{(1-\alpha )}^2(n_1-1)(n-3)(n_3-n_2)+2{\alpha }^2{(1-\alpha )}^2{n}_2(n_1-1)(n-3)\hfill \\ \hfill & -{\alpha }^4(n-3)(n_3-n_2)-(2\alpha -1){(1-\alpha )}^2(n_1-1)n_2{n}_3\hfill \\ \hfill >& {\alpha }^2(2\alpha -1)(n-3)(n_3-n_2)(n_4-2)+{\alpha }^4(n-3)(n_3-n_2)(n_1-2)(\ge 0)\hfill \\ \hfill & +{\alpha }^2{(1-\alpha )}^2{n}_2(n_1-1)[2(n-3)-n_3].\hfill \end{array}$$

Combing the above results and $k_1^{\prime }(\alpha (n-3)>0$, we find that

$$\begin{array}{cc}\hfill \left(5\right)>& {\left(5\right)|}_{x=\alpha (n-3)}\hfill \\ \hfill >& {\alpha }^2{(1-\alpha )}^2{n}_2(n_1-1)[2(n-3)-n_3]-{\alpha }^2{(1-\alpha )}^2(n_1+n_4)(n_1-1)\hfill \\ \hfill & +{\alpha }^4(n_1-1)(n_2+n_3-2)(n_1+n_4)-{\alpha }^2{(1-\alpha )}^2(n_1+n_4)(n_3-n_2)\hfill \\ \hfill & +{\alpha }^4(n_1-1)(n_2+n_3-2)(n-7)-{\alpha }^2{(1-\alpha )}^2(n-7)(n_3-n_2)\hfill \\ \hfill & +2{\alpha }^2{(1-\alpha )}^2[(n_1-1)n_2(n_2+n_3-2)-(n_3-n_2)]>0.\hfill \end{array}$$

Hence, the result holds. □

Lemma 11.

If $n_3\ge n_2$, for $x>\alpha (n-3)$,

$$c(n_1,n_2,n_3,n_4;x)-c(n_1+n_4-1,n_2,n_3,1;x)>0$$

holds for the following four cases:

(i) 

$n_2=1$, $n_1,n_4\ge 3$ and $n_3\ge n_4$;

(ii) 

$n_2=1$, $n_4=2$ and $n_1,n_3\ge 3$, $n_3\ge n_4$;

(iii) 

$n_1=2$, $n_2,n_3,n_4\ge 2$;

(iv) 

$n_1\ge 3$, $n_2,n_3,n_4\ge 2$ and $n_3\ge n_4$.

Further, if ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)>\alpha (n-3)$,

$${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right).$$

holds.

Proof. 

Note that all of the below functions are the same with those in Lemma 10; we will not repeat again. For $x=\alpha (n-3)$, we give $h_1\left(\alpha (n-3)\right)$ and $k_1\left(\alpha (n-3)\right)$, respectively, i.e.,

$$\begin{array}{cc}\hfill & h_1\left(\alpha (n-3)\right)\hfill \\ \hfill =& {\alpha }^2(n-3)(n_3-n_2)(n_1+n_4-2)+{\alpha }^2(n-4)(n_1-1)(n_1+n_4)\hfill \\ \hfill & +(2\alpha -1)(n_3-n_2)n_2{n}_3\hfill \end{array}$$

(6)

$$\begin{array}{cc}\hfill & -{\alpha }^2(n_3-n_2)n_1-{\alpha }^2(n_3-n_2)n_4\hfill \\ \hfill & -{\alpha }^2\{[{(n_3-n_2)}^2+n_2](n_1-1)+n_3{(n_1-1)}^2+(n_1{n}_2-n_3)n_4\},\hfill \end{array}$$

(7)

and

$$\begin{array}{cc}\hfill & k_1\left(\alpha (n-3)\right)\hfill \\ \hfill =& (n_1-1)[\alpha (n-3)(n_2+n_3)-2n_2{n}_3](>0)\hfill \\ \hfill & -\alpha \{[{(n_3-n_2)}^2+n_2](n_1-1)+n_3{(n_1-1)}^2+(n_1{n}_2-n_3)n_4\}.\hfill \end{array}$$

(8)

In view of (4), we find that

$$\begin{array}{cc}\hfill & -\alpha {(1-\alpha )}^2\left(7\right)+{(1-\alpha )}^4\left(8\right)\hfill \\ \hfill >& \alpha {(1-\alpha )}^2{n}_4[{\alpha }^2(n_1{n}_2-n_2)-{(1-\alpha )}^2(n_1{n}_2-n_3)]>0,\hfill \end{array}$$

(9)

where the remaining part

$$\alpha (n_2+n_3-2)d_1\left(\alpha (n-3)\right)-\alpha {(1-\alpha )}^2\left(6\right)$$

(10)

can be reduced to

$$\begin{array}{cc}\hfill & {\alpha }^3(n_1-1)\{{\alpha }^2(n-3)(n_1+n_4-4)(n_2+n_3-2)\hfill \\ \hfill & +{(1-\alpha )}^2{n}_2(n-3-n_3)(n_2+n_3-2)\hfill \\ \hfill & -{(1-\alpha )}^2(n-4)(n_1+n_4)\}\hfill \\ \hfill & +{\alpha }^3(n-3)\{{\alpha }^2(n_1-2)(n_3-n_2)(n_2+n_3-2)\hfill \\ \hfill & +{(1-\alpha )}^2{n}_2(n_1-1)(n_2+n_3-2)\hfill \\ \hfill & -{(1-\alpha )}^2(n_3-n_2)(n_1+n_4-2)\}\hfill \\ \hfill & +\alpha (2\alpha -1)(n_3-n_2)\{{\alpha }^2(n_2+n_3+n_2{n}_3)(n_2+n_3-2)\hfill \\ \hfill & -{(1-\alpha )}^2{n}_2{n}_3\}.\hfill \end{array}$$

(11)

Now, we shall prove $\left(11\right)>0$. Obviously, $(n_2+n_3+n_2{n}_3)(n_2+n_3-2)>n_2{n}_3$ for $n_2+n_3\ge 3$. Next, we consider

$$\begin{array}{cc}\hfill & (n_2+n_3-2)[(n-3)(n_1+n_4-4)+n_2(n-3-n_3)]-(n-4)(n_1+n_4),\hfill \end{array}$$

(12)

$$(n_3-n_2)[(n_1-2)(n_2+n_3-2)-(n_1+n_4-2)]+n_2(n_1-1)(n_2+n_3-2).$$

(13)

For $n_2=1$, without loss of generality, we may assume that $n_3\ge n_4$ and analyze the following two cases (i) and (ii):

(i) $n_2=1$, $n_1,n_4\ge 3$ and $n_3\ge n_4$.

Since $n_1,n_4\ge 3$, $n_1+n_4\ge 6$. If $n_1+n_4\ge 8$,

$$\begin{array}{cc}\hfill \left(12\right)>& (n_4-1)(n-3)(n_1+n_4-4)-(n-4)(n_1+n_4)\hfill \\ \hfill \ge & [2(n-3)-(n-4)](n_1+n_4)-8(n-3)\ge 8>0.\hfill \end{array}$$

If $n_1+n_4=7$, the case $n_3\ge n_4$ implies that $n\ge 12$, and

$$\begin{array}{cc}\hfill \left(12\right)& =(n-10)[3(n-3)+6]-7(n-4)=3n^2-40n+58\ge 10>0.\hfill \end{array}$$

If $n_1+n_4=6$, the case $n_3\ge n_4$ implies that $n\ge 11$, and

$$\begin{array}{cc}\hfill \left(12\right)& =(n-9)[2(n-3)+5]-6(n-4)=2n^2-25n+33\ge 0.\hfill \end{array}$$

Furthermore, we see that

$$\begin{array}{cc}\hfill \left(13\right)\ge & (n_3-1)[(n_1-2)(n_4-1)+(n_1-1)-(n_1+n_4-2)]\hfill \\ \hfill =& (n_3-1)(n_1-3)(n_4-1)\ge 0.\hfill \end{array}$$

(ii) $n_2=1$, $n_4=2$ and $n_1,n_3\ge 3$, $n_3\ge n_4$.

Note that $n_1+n_3=n-4$ and suppose that $n_1=t$,

$$\begin{array}{cc}\hfill \left(12\right)& =(n-5-t)\left[\right(n-3\left)\right(t-2)+(t+1\left)\right]-(n-4)(t+2)\hfill \\ \hfill & =-(n-2)t^2+(n^2-6n+7)t-2n^2+15n-27.\hfill \end{array}$$

For $n\ge 11$, namely, $n_1+n_3\ge 7$, it is easy to see that $\left(12\right)>0$. Furthermore, $\left(13\right)>0$ holds with the same reason as (i). For $n=10$, i.e., $n_1=n_3=3$,

$$\begin{array}{cc}\hfill & (n_1-1)\left(12\right)+(n-3)\left(13\right)+(n_1-1)(n_3-n_2)\hfill \\ \hfill =& 2(2·7+2·4-6·5)+7(2·2+2·2-2·3)+2·2=2>0.\hfill \end{array}$$

This operation adds the term ${\alpha }^5(n_2+n_3-2)(n_1-1)$ to (11), their sum is still less than (10).

When $n_1\ge 2$, we discuss the following two cases (iii) and (iv):

(iii) $n_1=2$ and $n_2,n_3,n_4\ge 2$.

Note that $n_2+n_3+n_4=n-3$ and $n_2\ge 2$; letting $n_4=t$, we find that

$$\begin{array}{cc}\hfill & (n_1-1)\left(12\right)+(n-3)\left(13\right)\hfill \\ \hfill =& (n_2+n_3-2)[(n-3)(n_4-2)+(n-3)n_2+n_2(n_2+n_4)]\hfill \\ \hfill & -(n-4)(n_4+2)-n_4(n-3)(n_2+n_3-2-2n_2+2)\hfill \\ \hfill \ge & (n-5-t)\left[\right(n-3\left)\right(t-2)+2(n-3)+2(2+t)-(n-3\left)t\right]\hfill \\ \hfill & -(n-4)(t+2)+2t(n-3)\hfill \\ \hfill =& -2t^2+(3n-16)t+2n-12>0.\hfill \end{array}$$

holds for $2\le t\le n-7$.

(iv) $n_1\ge 3$, $n_2,n_3,n_4\ge 2$ and $n_3\ge n_4$.

If $n_3\ge 3$ and $n_1+n_4\ge 6$,

$$\begin{array}{cc}\hfill \left(12\right)\ge & (n-3)n_3(n_1+n_4-4)-(n-4)(n_1+n_4)\hfill \\ \hfill \ge & [3(n-3)-(n-4)](n_1+n_4)-12(n-3)\ge 6>0.\hfill \end{array}$$

If $n_3\ge 3$ and $n_1+n_4=5$, the case $n_2\ge 2$ implies that $n\ge 11$, and

$$\begin{array}{cc}\hfill \left(12\right)=& (n-8)[(n-3)+n_2(3+n_2)]-5(n-4)\hfill \\ \hfill \ge & n^2-16n+44+10n-80=n^2-6n-36\ge 19>0.\hfill \end{array}$$

If $n_3=2$, by $n_2,n_4\le n_3$, we have $n_2=n_4=2$ and

$$\begin{array}{cc}\hfill \left(12\right)=& 2[(n-3)(n-9)+2(n-5)]-(n-4)(n-5)=n^2-11n+14\ge 4>0.\hfill \end{array}$$

It is easy to know that

$$\begin{array}{cc}\hfill \left(13\right)>& (n_3-n_2)[(n_1-2)(n_2+n_3-2)-(n_1+n_4-2)+n_2(n_1-1)]\hfill \\ \hfill \ge & (n_3-n_2)[(n_1-2)n_4-(n_1+n_4-2)+2(n_1-1)]\hfill \\ \hfill =& (n_3-n_2)[(n_1-3)(n_4+1)+3]\ge 0.\hfill \end{array}$$

Note that ${\alpha }^2>{(1-\alpha )}^2$ for $1/2<\alpha <1$, then all the above results produce $\left(11\right)>0$. Hence, $\left(10\right)>0$, with respect to (9), Lemma 10 implies that

$$c(n_1,n_2,n_3,n_4;x)-c(n_1+n_4-1,n_2,n_3,1;x)>0$$

for $x>\alpha (n-3)$. Therefore, if ${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)>\alpha (n-3)$,

$${\lambda }_1\left(A_{\alpha }(C_5\circ (n_1,n_2,n_3,n_4,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right).$$

The proof is completed. □

Below are two complements for the second case $\left(ii\right)$ of Lemma 11:

(a) The case with $n_2=1$, $n_3=n_4=2$ and $n_1\ge 3$ is given in the following Lemma 12;

(b) The case with $n_2=1$ and $n_1=2$ is given in the following Lemma 13. Note that in this case, the graph $C_5\circ (n_1,n_2,n_3,n_4,1)\cong C_5\circ (1,n_2,n_3,1,2)$, $n_2+n_3=n-4$.

Lemma 12.

If $n\ge 7$, then

$${\lambda }_1\left(A_{\alpha }(C_5\circ (n-6,1,2,2,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right).$$

Proof. 

The quotient matrix of the matrix $A_{\alpha }(C_5\circ (n-6,1,2,2,1))$ is

$$\left(\begin{array}{ccc}2\alpha & 2(1-\alpha )& 0\\ (n-6)(1-\alpha )& \alpha (n-4)& 2(1-\alpha )\\ 0& 1-\alpha & 2+\alpha \end{array}\right).$$

Then, the corresponding characteristic polynomial is

$$\begin{array}{cc}\hfill f_1\left(x\right)=& x^3-[\alpha (n-1)+2]x^2+[{\alpha }^{2}n+6\alpha (n-4)-2(n-5)]x\hfill \\ \hfill & -4{\alpha }^2(n-2)-2\alpha (3n-20)+4(n-6).\hfill \end{array}$$

(14)

Lemma 1 implies that ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-6,1,2,2,1))\right)$ is the largest root of $f_1\left(x\right)=0$. And

$$f_1\left(x\right)-f\left(x\right)=-(1-\alpha )x^2+[\alpha (n-9)+3]x-2{\alpha }^2-2\alpha (2n-15)+2(n-8),$$

(15)

where $f\left(x\right)$ is the polynomial shown in (2). From Lemmas 5 and 6, we see that

$$\alpha (n-3)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\le \alpha (n-3)+2(1-\alpha )=\alpha (n-5)+2.$$

In view of (15), we have

$${\left(15\right)|}_{x=\alpha (n-3)}={\alpha }^3{(n-3)}^2-2{\alpha }^2(3n-8)-\alpha (n-21)+2(n-8),$$

(16)

$${\left(15\right)|}_{x=\alpha (n-5)+2}={\alpha }^3{(n-5)}^2-2{\alpha }^2-3\alpha (n-7)+2(n-7).$$

(17)

For $1/2<\alpha <1$, the right functions of (16) and (17) are both increasing and, therefore,

$${\left(16\right)>\left(16\right)|}_{\alpha =1/2}=\frac{1}{8}(n^2-6n-3)>0,$$

$${\left(17\right)>\left(17\right)|}_{\alpha =1/2}=\frac{1}{8}(n^2-6n-7)\ge 0.$$

Finally, $\left(15\right)>0$ holds for $\alpha (n-3)<x\le \alpha (n-3)+2(1-\alpha )$. Hence, ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-6,1,2,2,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)$. □

Lemma 13.

If $n_2,n_3\ge 2$ and $n_2\le n_3$, then for $x\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }$,

$$\tilde{c}(1,n_2,n_3,1,2;x)-\tilde{c}(1,1,n-5,1,2;x)>0.$$

Further, if ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,2))\right)\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{{\alpha }^2}$, then

$${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,2))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-5,1,2))\right).$$

Proof. 

The quotient matrix of the matrix $A_{\alpha }(C_5\circ (1,n_2,n_3,1,2))$ is

$$\left(\begin{array}{ccccc}2\alpha & 1-\alpha & 0& 0& 1-\alpha \\ 2(1-\alpha )& \alpha (2+n_2)& (1-\alpha )n_2& 0& 0\\ 0& (1-\alpha )& \alpha (1+n_3)& (1-\alpha )n_3& 0\\ 0& 0& (1-\alpha )n_2& \alpha (1+n_2)& 1-\alpha \\ 2(1-\alpha )& 0& 0& (1-\alpha )n_3& \alpha (2+n_3)\end{array}\right).$$

Then, the corresponding characteristic polynomial is

$$\tilde{c}(1,n_2,n_3,1,2;x)=(x-2\alpha )\tilde{d}\left(x\right)-{(1-\alpha )}^2[\tilde{e}\left(x\right)+2\tilde{h}\left(x\right)]+2{(1-\alpha )}^4\tilde{k}\left(x\right),$$

(18)

where

$$\begin{array}{cc}\hfill \tilde{d}\left(x\right)=& [x^2-\alpha (n-1)x+{\alpha }^2(2+n_2)(1+n_3)-{(1-\alpha )}^2{n}_2]\hfill \\ \hfill & ·[x^2-\alpha (n-1)x+{\alpha }^2(1+n_2)(2+n_3)-{(1-\alpha )}^2{n}_3],\hfill \\ \hfill \tilde{e}\left(x\right)=& n_2{n}_3(x-2\alpha )[x-\alpha (2+n_2)][x-\alpha (2+n_3)],\hfill \\ \hfill \tilde{h}\left(x\right)=& (2x-\alpha n)[x^2-\alpha (n-2)x+{\alpha }^2(n-3)+(2\alpha -1)n_2{n}_3],\hfill \\ \hfill \tilde{k}\left(x\right)=& (n-4)x-\alpha {(n_2-n_3)}^2-\alpha (n-4)-2n_2{n}_3.\hfill \end{array}$$

Applying (18), we have

$$\tilde{c}(1,n_2,n_3,1,2;x)-\tilde{c}(1,1,n-5,1,2;x)=(n_2-1)(n_3-1)\tilde{c}\left(x\right),$$

where

$$\begin{array}{cc}\hfill \tilde{c}\left(x\right)=& (x-2\alpha )\{2{\alpha }^2(x-\alpha )(x-\alpha (n-2))\hfill \\ \hfill & +{\alpha }^4(n_2{n}_3+n-2)+{\alpha }^2(2\alpha -1)(n-6)+{(1-\alpha )}^4\hfill \\ \hfill & -{(1-\alpha )}^2[(x-2\alpha )(x-\alpha (n-2))+{\alpha }^2(n_2{n}_3+n-5)]\}\hfill \\ \hfill & -2{(1-\alpha )}^2(2\alpha -1)[2x-\alpha n]+4{(1-\alpha )}^4(2\alpha -1).\hfill \end{array}$$

Note that

$${\tilde{c}}^{\prime \prime }\left(x\right)=2({\alpha }^2+2\alpha -1)[3x-\alpha n]-4\alpha (2\alpha -1)>{\tilde{c}}^{\prime \prime }\left(\alpha (n-3)\right)>0.$$

Then, ${\tilde{c}}^{\prime }\left(x\right)$ is increasing for $x>\alpha (n-3)$ and

$$\begin{array}{cc}\hfill {\tilde{c}}^{\prime }\left(x\right)=& [2{\alpha }^2(x-\alpha )-{(1-\alpha )}^2(x-2\alpha )](2x-\alpha n)\hfill \\ \hfill & +({\alpha }^2+2\alpha -1)(x-2\alpha )(x-\alpha (n-2))\hfill \\ \hfill & +3{\alpha }^4+{\alpha }^2(2\alpha -1)(n_2{n}_3+2n-11)+{(1-\alpha )}^4\hfill \\ \hfill >& {\tilde{c}}^{\prime }\left(\alpha (n-3)\right)>{\alpha }^4(n^2-10n+23)+{\alpha }^2(2\alpha -1)(n-5)(n-7)>0.\hfill \end{array}$$

Hence, $\tilde{c}\left(x\right)$ is increasing and the value $x=\alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }$ is larger than

$$\alpha (n-5)[2{\alpha }^4+{\alpha }^2(2\alpha -1)(n_2{n}_3-4)+(2\alpha -1){(1-\alpha )}^2(n-7)]>0.$$

Finally, for $x\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }$, we have

$$\tilde{c}(1,n_2,n_3,1,2;x)-\tilde{c}(1,1,n-5,1,2;x)>0.$$

Further, if ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,2))\right)\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{{\alpha }^2}$, then

$${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,2))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-5,1,2))\right).$$

We complete the proof. □

Next, the $A_{\alpha }$-index of $C_5\circ (1,n_2,n_3,1,1)$, $n_2+n_3=n-3$, will be shown as follows.

Lemma 14.

If $n_2,n_3\ge 2$ and $n_2\le n_3$, then

$${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right).$$

Proof. 

Clearly, $C_5\circ (1,1,n-4,1,1)\cong C_5\circ (n-4,1,1,1,1)$. Since $n_2,n_3\ge 2$, $n\ge 7$. The case $n=7$ needs to be considered separately. According to Lemma 12, we obtain ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,2,2,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (3,1,1,1,1))\right)$. When $n>7$, applying (3) ($n_1=n_4=1$), we see that

$$c(1,n_2,n_3,1;x)-c(1,1,n-4,1;x)=(n_2-1)(n_3-1)c\left(x\right),$$

(19)

where

$$\begin{array}{cc}\hfill c\left(x\right)=& (x-2\alpha )\{({\alpha }^2+2\alpha -1)(x-\alpha )[(x-\alpha (n-2)]+{\alpha }^2(2\alpha -1)(n_2{n}_3-1)\hfill \\ \hfill & +[{\alpha }^2(n-4)+(2\alpha -1)](4\alpha -2-{\alpha }^2)\}\hfill \\ \hfill & -{(1-\alpha )}^2(2\alpha -1)[x-\alpha (n-3)]+2{(1-\alpha )}^4(2\alpha -1).\hfill \end{array}$$

Clearly,

$$c^{\prime \prime }\left(x\right)=2({\alpha }^2+2\alpha -1)[3x-\alpha (n+1)]>c^{\prime \prime }\left(\alpha (n-3)\right)=4({\alpha }^2+2\alpha -1)\alpha (n-4)>0.$$

Therefore, $c^{\prime }\left(x\right)$ is increasing for $x>\alpha (n-3)$ and

$$\begin{array}{cc}\hfill c^{\prime }\left(x\right)=& ({\alpha }^2+2\alpha -1)\{(x-\alpha )[(x-\alpha (n-2)]+(x-2\alpha )[(x-\alpha (n-2)]\hfill \\ \hfill & +(x-\alpha )(x-2\alpha )\}\hfill \\ \hfill & +{\alpha }^2(2\alpha -1)(n_2{n}_3-1)+[{\alpha }^2(n-4)+(2\alpha -1)](4\alpha -2-{\alpha }^2)\hfill \\ \hfill & -{(1-\alpha )}^2(2\alpha -1)\hfill \\ \hfill >& c^{\prime }\left(\alpha (n-3)\right)>{\alpha }^4(n^2-12n+33)+{\alpha }^2(2\alpha -1)(n^2-11n+27)>0.\hfill \end{array}$$

Then, $c\left(x\right)$ is increasing for $x>\alpha (n-3)$.

Now, we consider the following four cases:

(i) $2/3<\alpha <1$.

Note that $3/4<\frac{2\alpha -1}{{\alpha }^2}<1$, we find that

$$\begin{array}{cc}\hfill & c\left(x\right)\ge c\left(\alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }\right)\hfill \\ \hfill =& {\alpha }^2(2\alpha -1)\left(\alpha (n-5)+\frac{{(1-\alpha )}^2}{\alpha }\right)\hfill \\ \hfill & ·[n_2{n}_3+n-7-(n-4)\frac{{\alpha }^2}{2\alpha -1}-(n-6)\frac{2\alpha -1}{{\alpha }^2}+\frac{{(2\alpha -1)}^2}{{\alpha }^4}]\hfill \\ \hfill & +(2\alpha -1){(1-\alpha )}^4(2-\frac{1}{\alpha })\hfill \\ \hfill >& {\alpha }^2(2\alpha -1)[2(n-5)+n-7-\frac{4}{3}(n-4)-\frac{3}{4}(n-6)]\hfill \\ \hfill =& \frac{{\alpha }^2(2\alpha -1)}{12}(11n-86)>0.\hfill \end{array}$$

Hence, $\left(19\right)>0$ for $x\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }$. And $\left(ii\right)$ of Lemma 7 implies that ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }$. Then, ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$.

(ii) $2-\sqrt{2}\le \alpha \le 2/3$.

By the monotonicity of $c\left(x\right)$ and $1/2\le \frac{2\alpha -1}{{\alpha }^2}\le 3/4$, we see that

$$\begin{array}{cc}\hfill & c\left(x\right)\ge c\left(\alpha \right(n-3)+(1-\alpha \left)\right)\hfill \\ \hfill =& {\alpha }^2(2\alpha -1)(\alpha (n-6)+1)\hfill \\ \hfill & ·[n_2{n}_3-1-(n-4)\frac{{\alpha }^2}{2\alpha -1}+(n-5)(\frac{1}{\alpha }-\alpha )+\frac{2\alpha -1}{{\alpha }^2}]\hfill \\ \hfill & -{(2\alpha -1)}^2{(1-\alpha )}^3\hfill \\ \hfill \ge & {\alpha }^3(2\alpha -1)[2n-11-2(n-4)+\frac{5}{6}(n-5)+\frac{1}{2}]-{(2\alpha -1)}^2{(1-\alpha )}^3\hfill \\ \hfill =& {\alpha }^3(2\alpha -1)\frac{5}{6}(n-8)-{(2\alpha -1)}^2{(1-\alpha )}^3>0.(n\ge 9)\hfill \end{array}$$

For $n=8$ ($n_2=2$, $n_3=3$), it is not hard to see that

$$\begin{array}{cc}\hfill & c\left(x\right)\ge c(4\alpha +1)\hfill \\ \hfill =& (2\alpha +1)(-10{\alpha }^4+13{\alpha }^3+5{\alpha }^2-7\alpha +1)-{(2\alpha -1)}^2{(1-\alpha )}^3>0.\hfill \end{array}$$

Hence, $\left(19\right)>0$ holds for $x\ge \alpha (n-3)+(1-\alpha )$. And $\left(iii\right)$ of Lemma 7 implies that ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)>\alpha (n-3)+(1-\alpha )$. Then, ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$.

For $1/2<\alpha <2-\sqrt{2}$, we rewrite $c\left(x\right)$ as the following form:

$$\begin{array}{cc}\hfill c\left(x\right)=& (x-2\alpha )\{{\alpha }^2(x-\alpha )[(x-\alpha (n-2)]+{\alpha }^2(n-3)(6\alpha -3-{\alpha }^2)\hfill \\ \hfill & +{\alpha }^2(2\alpha -1)(n_2{n}_3-n+2)+{(1-\alpha )}^2({\alpha }^2-4\alpha +2)\hfill \\ \hfill & +(2\alpha -1)(x-\alpha )\left[\right(x-\alpha (n-2)\left]\right\}\hfill \\ \hfill & -{(1-\alpha )}^2(2\alpha -1)[x-\alpha (n-3)]+2{(1-\alpha )}^4(2\alpha -1).\hfill \end{array}$$

(iii) $3-\sqrt{6}\le \alpha <2-\sqrt{2}$.

By the monotonicity of $c\left(x\right)$, we see that

$$\begin{array}{cc}\hfill c\left(x\right)\ge & c\left(\alpha \right(n-2\left)\right)\hfill \\ \hfill >& \alpha (n-4)[{\alpha }^2(n-3)(6\alpha -3-{\alpha }^2)+{(1-\alpha )}^2({\alpha }^2-4\alpha +2)]\hfill \\ \hfill & +(2\alpha -1)[{\alpha }^2(n_2{n}_3-n+2)+{(1-\alpha )}^2(2{\alpha }^2-5\alpha +2)]>0.\hfill \end{array}$$

Hence, $\left(19\right)>0$ holds for $x\ge \alpha (n-2)$. And $\left(iv\right)$ of Lemma 7 implies that ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)>\alpha (n-2)$. Then, ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$.

(iv) $1/2<\alpha <3-\sqrt{6}$.

Suppose that

$$c_1\left(x\right)=(x-\alpha )[(x-\alpha (n-2)]+(n-3)(6\alpha -3-{\alpha }^2),$$

where its largest real zero is denoted by $x_1$. Obviously, $\alpha (n-2)<x_1<\alpha (n-1)$, and at the same time,

$$\begin{array}{cc}\hfill & x_1^3=\alpha (n-1)x_1^2-[{\alpha }^2+3(2\alpha -1)(n-3)]x_1,\hfill \\ \hfill & x_1^2=\alpha (n-1)x_1-[{\alpha }^2+3(2\alpha -1)(n-3))].\hfill \end{array}$$

Put $x_1$ in (2) and apply the above two equations; we find that

$$\begin{array}{cc}\hfill f\left(x_1\right)=& (n-2)(1-2\alpha )x_1+{\alpha }^3+{\alpha }^2(2n-7)+\alpha (n+1)-n+1\hfill \\ \hfill <& {\alpha }^3-{\alpha }^2(2n^2-10n+15)+\alpha (n^2-3n+5)-n+1<0.\hfill \end{array}$$

So, $x_1<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$. For $x={\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$, clearly, $c\left(x\right)>0$. From (19), ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$. The proof is completed. □

4. Proof of Theorem 2

Now, we are in a position to present the proof of Theorem 2.

Proof. 

Suppose that G has the maximum $A_{\alpha }$-index in non-bipartite graphs of order n without triangle. By Lemma 8, we obtain $G\cong C_5\circ (n_1,n_2,n_3,n_4,1)$ as shown in Figure 3.

Case 1. $n_4=1$ or $n_1=1$.

Specially, when $n_1=n_4=1$, we have $G\cong C_5\circ (1,n_2,n_3,1,1)$. Further, $n_2=1$ or $n_3=1$ implies that $G\cong C_5\circ (n-4,1,1,1,1)$; otherwise, by Lemma 14, ${\lambda }_1\left(A_{\alpha }(C_5\circ (1,n_2,n_3,1,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-4,1,1))\right)$, a contradiction.

Next, we may assume that $n_4=1$ and $n_1\ge 2$. Let $V_4=\left\{v_4\right\}$ and $x:={\left(x_v\right)}_{v\in V\left(G\right)}$ be the Perron vector of $A_{\alpha }\left(G\right)$. Recall that $n_3\ge 1$, so we can choose a vertex $v_3\in V_3$. If $n_3\ge 2$, there exists at least one vertex $z\in V_3\setminus \left\{v_3\right\}$. Without loss of generality, suppose that $x_w\ge x_{v_4}$. Define a new graph $G^{\prime }=G-z{v}_4+zw$ for $z\in V_3\setminus \left\{v_3\right\}$; obviously, $G^{\prime }$ is still non-bipartite triangle-free. However, Lemma 3 implies that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }\left(G^{\prime }\right)\right)$, a contradiction. Hence, $n_3=1$. Further, if $n_2=1$, then $G\cong C_5\circ (n-4,1,1,1,1)$; otherwise, $G\cong C_5\circ (n_1,n_2,1,1,1)$, which is the same as $C_5\circ (1,n_1,n_2,1,1)$. However, Lemma 14 induces a contradiction.

Case 2. $n_2=1$ and $n_1,n_4\ge 2$ or $n_3=1$ and $n_1,n_4\ge 2$.

We may assume that $n_2=1$ and $n_1,n_4\ge 2$. If $n_3=1$, then $G\cong C_5\circ (n_1,1,1,n_4,1)$; obviously, $V_2=\left\{u^{\prime }\right\}$ and $V_3=\left\{v^{\prime }\right\}$. Let $x:={\left(x_v\right)}_{v\in V\left(G\right)}$ be the Perron vector of $A_{\alpha }\left(G\right)$. Without loss of generality, suppose that $x_{u^{\prime }}\ge x_{v^{\prime }}$. Let $G^{\prime }=G-v^{\prime }{v}_4+u^{\prime }{v}_4$ for $v_4\in V_4$, clearly $G^{\prime }$ is still non-bipartite triangle-free. However, Lemma 3 implies that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }\left(G^{\prime }\right)\right)$, a contradiction.

If $n_3\ge 2$, the condition $n_3\ge n_4$ can always be achieved by $n_2=1$. By $\left(ii\right)$ of Lemma 7,

$${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\ge {\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\ge \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }>\alpha (n-3).$$

Applying $\left(i\right)$ and $\left(ii\right)$ of Lemma 11 in the case $n_1,n_4\ge 3$ and the case $n_4=2$ with $n_1,n_3\ge 3$, respectively, we find that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right)$, contrary to the assumption that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ is maximal. For $n_3=n_4=2$ and $n_1\ge 3$, by Lemma 12, we know that ${\lambda }_1\left(A_{\alpha }(C_5\circ (n-6,1,2,2,1))\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)$, a contraction. For $n_1=2$, since $C_5\circ (2,1,n_3,n_4,1)\cong C_5\circ (1,n_4,n_3,1,2)$, by Lemma 13, we see that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (1,1,n-5,1,2))\right)$, which contradicts the maximality of ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$.

Case 3. $n_i\ge 2$ for $i=1,\dots ,4$.

If $n_1=2$, from $\left(iii\right)$ of Lemma 11, we see that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right)$, a contradiction with the maximum ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$.

Let $u^{*}$ be a vertex of G, which satisfies that $\alpha d\left(u^{*}\right)+(1-\alpha )m\left(u^{*}\right)=max\{\alpha d\left(u\right)+(1-\alpha )m\left(u\right),u\in V\left(G\right)\}$. From Lemma 4, we obtain ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le \alpha d\left(u^{*}\right)+(1-\alpha )m\left(u^{*}\right)$. It is not hard to see that $u^{*}\in V_2\cup V_3$, without loss of generality, suppose that $n_2\le n_3$. First, if $u^{*}\in V_2$, then

$$\begin{array}{cc}\hfill & \alpha d\left(u^{*}\right)+(1-\alpha )m\left(u^{*}\right)\hfill \\ \hfill =& \alpha (n_1+n_3)+(1-\alpha )\frac{n_1(1+n_2)+n_3(n_2+n_4)}{n_1+n_3}\hfill \\ \hfill =& \alpha (n_1+n_3)+(1-\alpha )\frac{(n_2+1)(n_1+n_3)+n_3(n_4-1)}{n_1+n_3}\hfill \\ \hfill =& \alpha \left(n_1+n_3-n_2-1-\frac{n_3(n_4-1)}{n_1+n_3}\right)+(n_2+1)+\frac{n_3(n_4-1)}{n_1+n_3}.\hfill \end{array}$$

And by Lemma 6 and $\left(ii\right)$ Lemma 7, we have

$$\begin{array}{cc}\hfill \alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }& \le {\lambda }_1\left(A_{\alpha }(C_5\circ (n-4,1,1,1,1))\right)\hfill \\ \hfill & \le {\lambda }_1\left(A_{\alpha }\left(G\right)\right)\le \alpha d\left(u^{*}\right)+(1-\alpha )m\left(u^{*}\right).\hfill \end{array}$$

Hence,

$$\alpha (n-3)+\frac{{(1-\alpha )}^2}{\alpha }\le \alpha (n_1+n_3-n_2-1-\frac{n_3(n_4-1)}{n_1+n_3})+(n_2+1)+\frac{n_3(n_4-1)}{n_1+n_3},$$

i.e.,

$$\alpha \left(2n_2-1+n_4+\frac{n_3(n_4-1)}{n_1+n_3}\right)+\frac{{(1-\alpha )}^2}{\alpha }\le (n_2+1)+\frac{n_3(n_4-1)}{n_1+n_3}.$$

Let

$$p\left(\alpha \right)=\alpha \left(2n_2-1+n_4+\frac{n_3(n_4-1)}{n_1+n_3}\right)+\frac{{(1-\alpha )}^2}{\alpha }$$

and for $1/2<\alpha <1$,

$$p^{\prime }\left(\alpha \right)=2n_2-1+n_4+\frac{n_3(n_4-1)}{n_1+n_3}+1-\frac{1}{{\alpha }^2}>2(n_2-2)+n_4+\frac{n_3(n_4-1)}{n_1+n_3}>0.$$

So, $p\left(\alpha \right)>p(1/2)$ and

$$\frac{1}{2}\left(2n_2+n_4+\frac{n_3(n_4-1)}{n_1+n_3}\right)<(n_2+1)+\frac{n_3(n_4-1)}{n_1+n_3},$$

namely, $n_1(n_4-2)<n_3$, which is equivalent to $n_4+(n_1-1)(n_4-2)-2<n_3$. If $n_1\ge 3$, $n_3\ge n_4$ holds. Furthermore, if $u^{*}\in V_3$, the proof works along the same lines as $u^{*}\in V_2$. We obtain $n_4(n_1-2)<n_2$, which implies that $n_3\ge n_2>n_4$ in the supposition $n_1\ge 3$. So, by $\left(iv\right)$ of Lemma 11, we know that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)<{\lambda }_1\left(A_{\alpha }(C_5\circ (n_1+n_4-1,n_2,n_3,1,1))\right)$, contrary to the assumption that ${\lambda }_1\left(A_{\alpha }\left(G\right)\right)$ is maximal.

Finally, only the graph $C_5\circ (n-4,1,1,1,1)$ has the maximal $A_{\alpha }$-index. The proof is completed. □

5. Concluding Remarks

In this paper, we explored the $A_{\alpha }$-index of a triangle-free non-bipartite graph with order n and obtained the extremal graph $C_5\circ (n-4,1,1,1,1)$, $1/2<\alpha <1$. For the case $0<\alpha <1/2$, we will undertake further discussion in our subsequent research.