Isometric Deformation of (m,n)-Type Helicoidal Surface in the Three Dimensional Euclidean Space
Abstract
:1. Introduction
2. Preliminaries
3. Helicoidal Surfaces of Value (m,n)
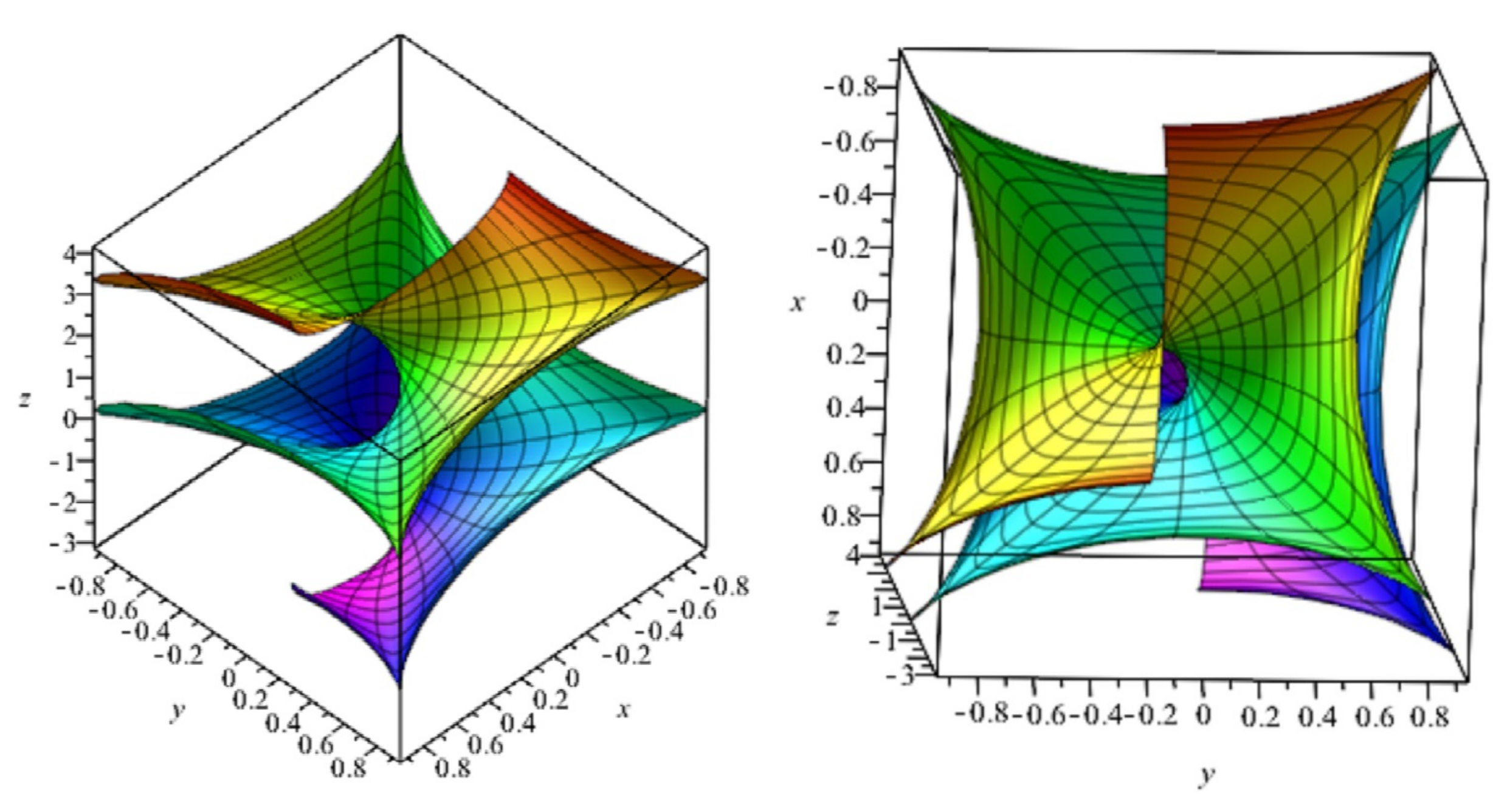
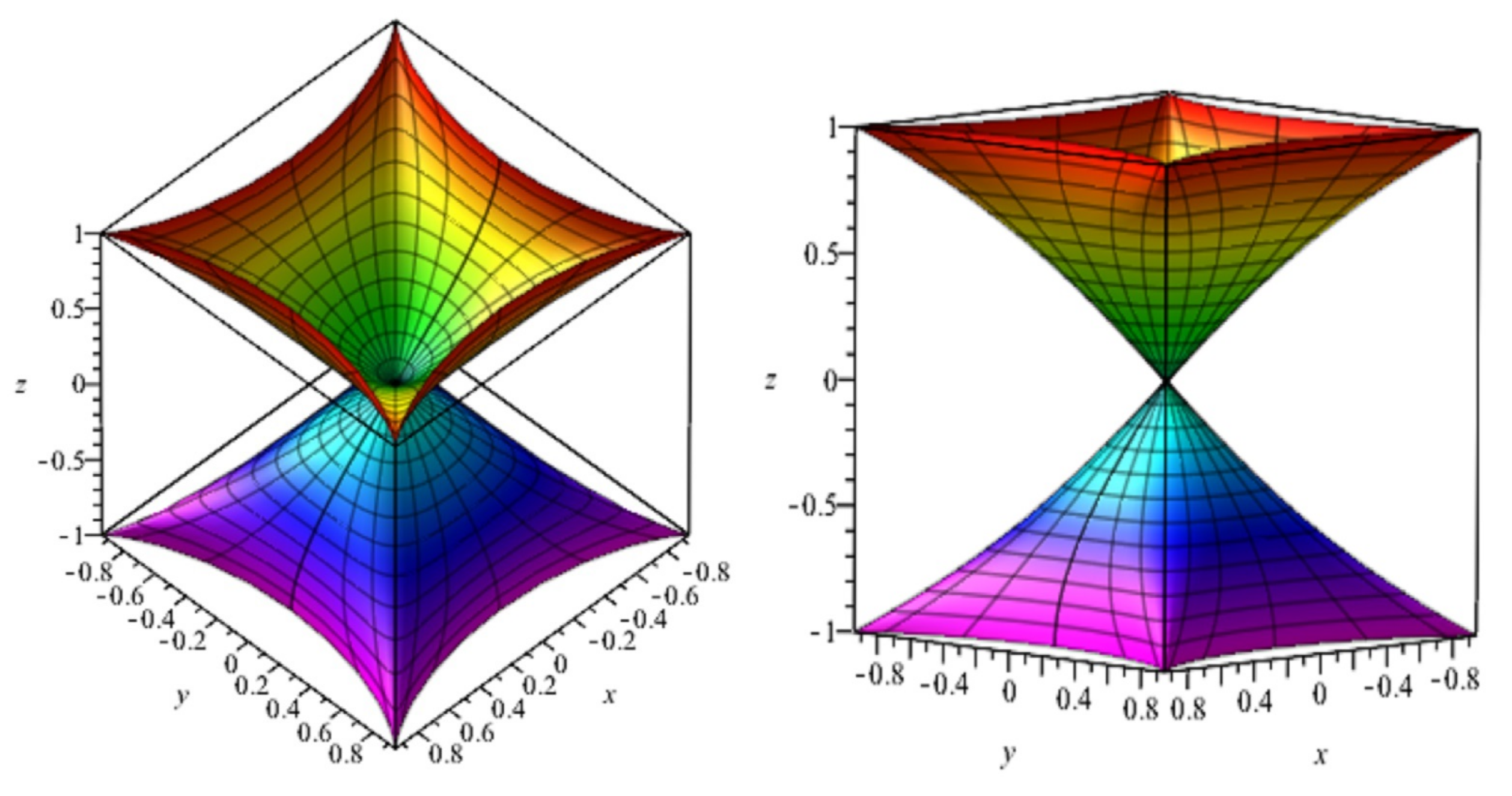
4. Helicoidal Surface of Value (0,1)
5. Laplace–Beltrami Operator
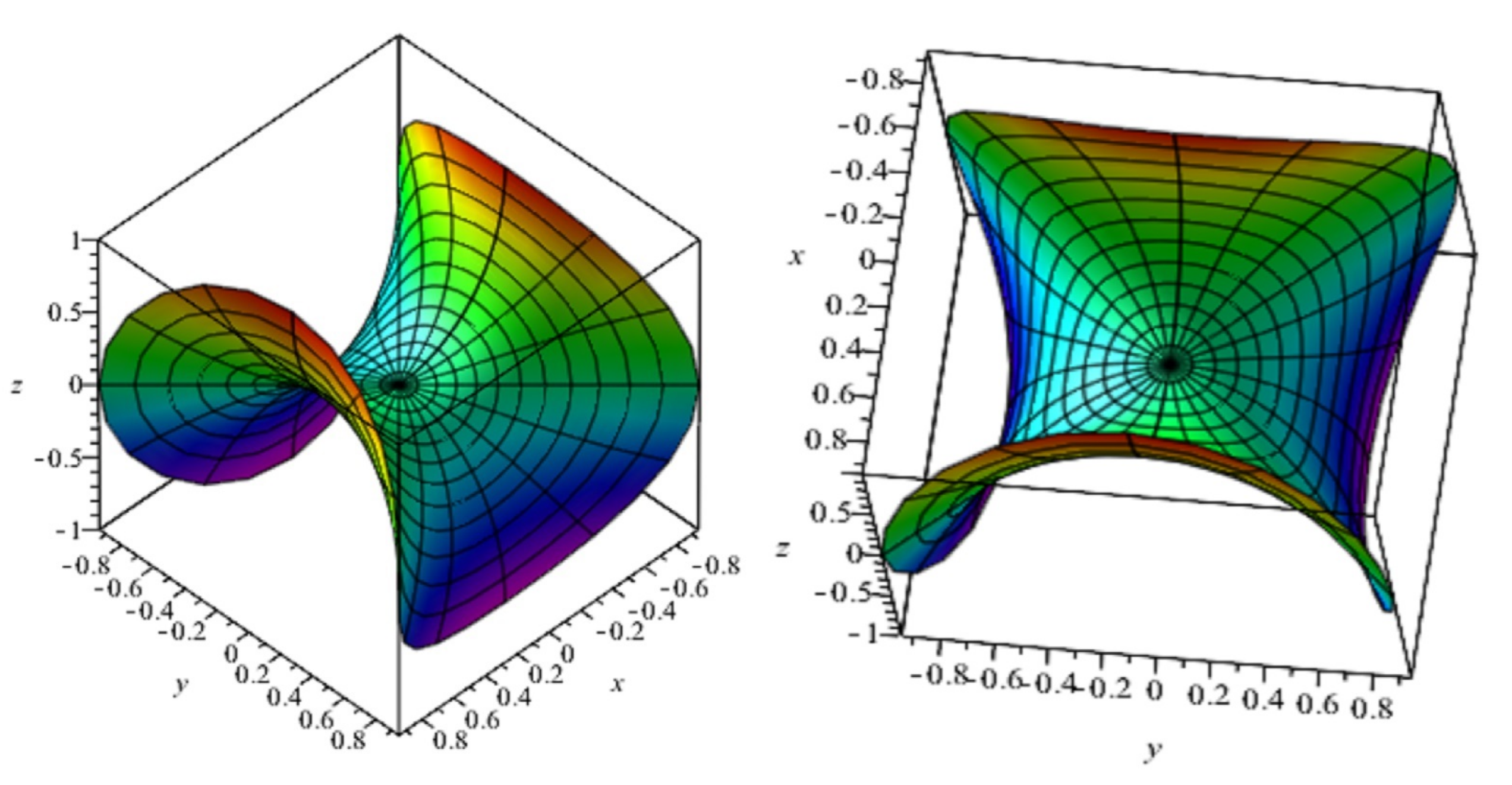
6. Rotational Surface Satisfying in 𝔼3
Author Contributions
Funding
Conflicts of Interest
References
- Chen, B.Y. Total Mean Curvature and Submanifolds of Finite Type; World Scientific: Singapore, 1984. [Google Scholar]
- Arslan, K.; Kılıç Bayram, B.; Bulca, B.; Öztürk, G. Generalized Rotation Surfaces in 𝔼4. Results Math. 2012, 61, 315–327. [Google Scholar] [CrossRef]
- Do Carmo, M.; Dajczer, M. Helicoidal surfaces with constant mean curvature. Tohoku Math. J. 1982, 34, 351–367. [Google Scholar] [CrossRef]
- Chen, B.Y.; Choi, M.; Kim, Y.H. Surfaces of revolution with pointwise 1-type Gauss map. Korean Math. Soc. 2005, 42, 447–455. [Google Scholar] [CrossRef]
- Choi, M.; Kim, Y.H. Characterization of the helicoid as ruled surfaces with pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2001, 38, 753–761. [Google Scholar]
- Choi, M.; Yoon, D.W. Helicoidal surfaces of the third fundamental form in Minkowski 3-space. Bull. Korean Math. Soc. 2015, 52, 1569–1578. [Google Scholar] [CrossRef]
- Dillen, F.; Pas, J.; Verstraelen, L. On surfaces of finite type in Euclidean 3-space. Kodai Math. J. 1990, 13, 10–21. [Google Scholar] [CrossRef]
- Dursun, U.; Turgay, N.C. Minimal and pseudo-umbilical rotational surfaces in Euclidean space 𝔼4. Mediterr. J. Math. 2013, 10, 497–506. [Google Scholar] [CrossRef]
- Kim, Y.H.; Turgay, N.C. Surfaces in 𝔼3 with L1-pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2013, 50, 935–949. [Google Scholar] [CrossRef]
- Ferrandez, A.; Garay, O.J.; Lucas, P. On a Certain Class of Conformally at Euclidean Hypersurfaces. In Global Analysis and Global Differential Geometry; Springer: Berlin, Germany, 1990; pp. 48–54. [Google Scholar]
- Güler, E.; Turgut Vanlı, A. Bour’s theorem in Minkowski 3-space. J. Math. Kyoto Univ. 2006, 46, 47–63. [Google Scholar] [CrossRef]
- Güler, E.; Yaylı, Y. Generalized Bour theorem. Kuwait J. Sci. 2015, 42, 79–90. [Google Scholar]
- Güler, E.; Yaylı, Y.; Hacı salihoğlu, H.H. Bour’s theorem on the Gauss map in 3-Euclidean space. Hacettepe J. Math. Stat. 2010, 39, 515–525. [Google Scholar]
- Ikawa, T. Bour’s theorem and Gauss map. Yokohama Math. J. 2000, 48, 173–180. [Google Scholar]
- Ikawa, T. Bour’s theorem in Minkowski geometry. Tokyo J. Math. 2001, 24, 377–394. [Google Scholar] [CrossRef]
- Ji, F.; Kim, Y.H. Mean curvatures and Gauss maps of a pair of isometric helicoidal and rotation surfaces in Minkowski 3-space. J. Math. Anal. Appl. 2010, 368, 623–635. [Google Scholar] [CrossRef]
- Ji, F.; Kim, Y.H. Isometries between minimal helicoidal surfaces and rotation surfaces in Minkowski space. Appl. Math. Comput. 2013, 220, 1–11. [Google Scholar] [CrossRef]
- The Hieu, D.; Ngoc Thang, N. Bour’s theorem in 4-dimensional Euclidean space. Bull. Korean Math. Soc. 2017, 54, 2081–2089. [Google Scholar]
- Ganchev, G.; Milousheva, V. General rotational surfaces in the 4-dimensional Minkowski space. Turk. J. Math. 2014, 38, 883–895. [Google Scholar] [CrossRef] [Green Version]
- Güler, E.; Magid, M.; Yaylı, Y. Laplace Beltrami operator of a helicoidal hypersurface in four space. J. Geom. Sym. Phys. 2016, 41, 77–95. [Google Scholar] [CrossRef]
- Güler, E.; Hacısalihoğlu, H.H.; Kim, Y.H. The Gauss Map and the third Laplace–Beltrami operator of the rotational hypersurface in 4-Space. Symmetry 2018, 10, 398. [Google Scholar] [CrossRef]
- Lawson, H.B. Lectures on Minimal Submanifolds, 2nd ed.; Mathematics Lecture Series, 9; Publish or Perish, Inc.: Wilmington, NC, USA, 1980; Volume I. [Google Scholar]
- Takahashi, T. Minimal immersions of Riemannian manifolds. J. Math. Soc. Jpn. 1966, 18, 380–385. [Google Scholar] [CrossRef]
- Senoussi, B.; Bekkar, M. Helicoidal surfaces with ΔJr = Ar in 3-dimensional Euclidean space. Stud. Univ. Babeş-Bolyai Math. 2015, 60, 437–448. [Google Scholar]
- Bour, E. Théorie de la déformation des surfaces. J. Êcole Imperiale Polytech. 1862, 22–39, 1–148. [Google Scholar]
- Kenmotsu, K. Surfaces of revolution with prescribed mean curvature. Tohôku Math. J. 1980, 32, 147–153. [Google Scholar] [CrossRef]
- Hitt, L.; Roussos, I. Computer graphics of helicoidal surfaces with constant mean curvature. An. Acad. Brasil. Ciênc. 1991, 63, 211–228. [Google Scholar]
- Güler, E. A new kind helicoidal surface of value m. Int. Elec. J. Geom. 2014, 7, 154–162. [Google Scholar]
- Eisenhart, L. A Treatise on the Differential Geometry of Curves and Surfaces; Palermo 41 Ginn and Company: Orland, MA, USA, 1909. [Google Scholar]
- Spivac, M. A Comprehensive Introduction to Differential Geometry III; Interscience: New York, NY, USA, 1969. [Google Scholar]
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Güler, E. Isometric Deformation of (m,n)-Type Helicoidal Surface in the Three Dimensional Euclidean Space. Mathematics 2018, 6, 226. https://doi.org/10.3390/math6110226
Güler E. Isometric Deformation of (m,n)-Type Helicoidal Surface in the Three Dimensional Euclidean Space. Mathematics. 2018; 6(11):226. https://doi.org/10.3390/math6110226
Chicago/Turabian StyleGüler, Erhan. 2018. "Isometric Deformation of (m,n)-Type Helicoidal Surface in the Three Dimensional Euclidean Space" Mathematics 6, no. 11: 226. https://doi.org/10.3390/math6110226
APA StyleGüler, E. (2018). Isometric Deformation of (m,n)-Type Helicoidal Surface in the Three Dimensional Euclidean Space. Mathematics, 6(11), 226. https://doi.org/10.3390/math6110226




