Constructions of Helicoidal Surfaces in a 3-Dimensional Complete Manifold with Density
Abstract
:1. Introduction
2. Preliminaries
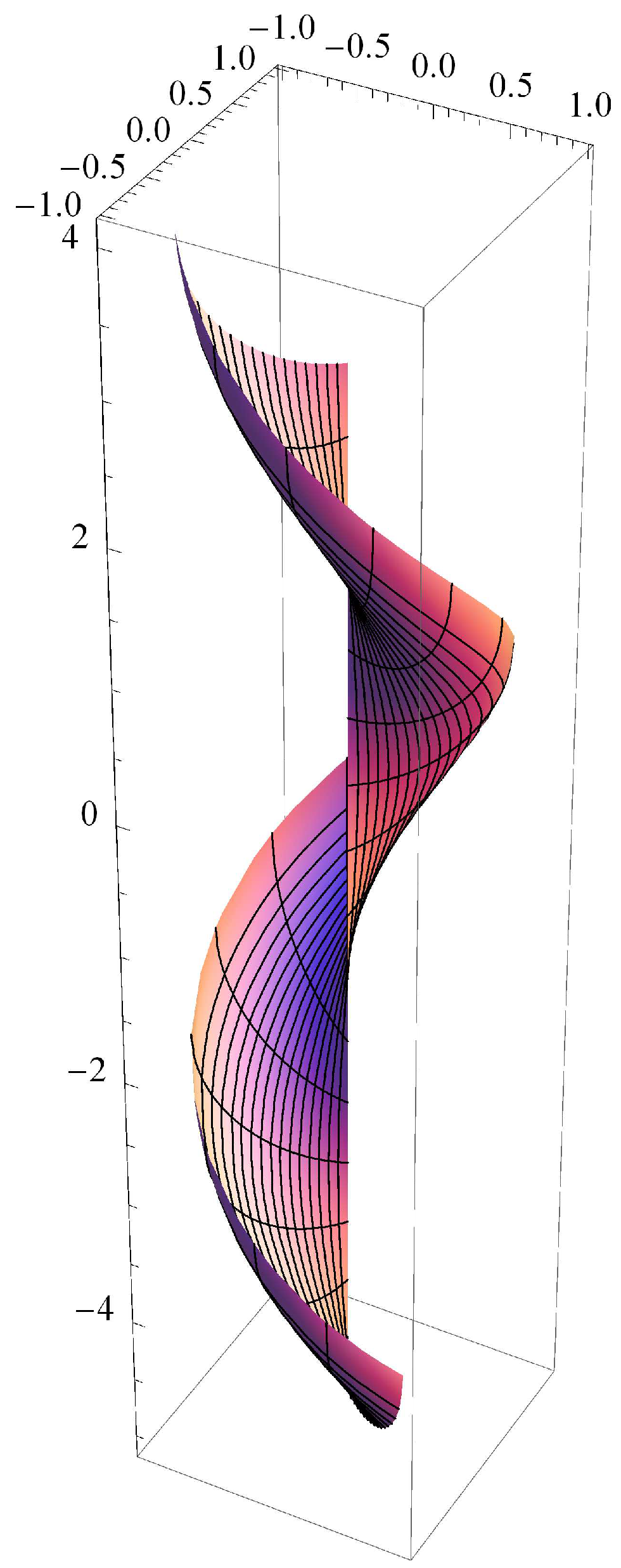
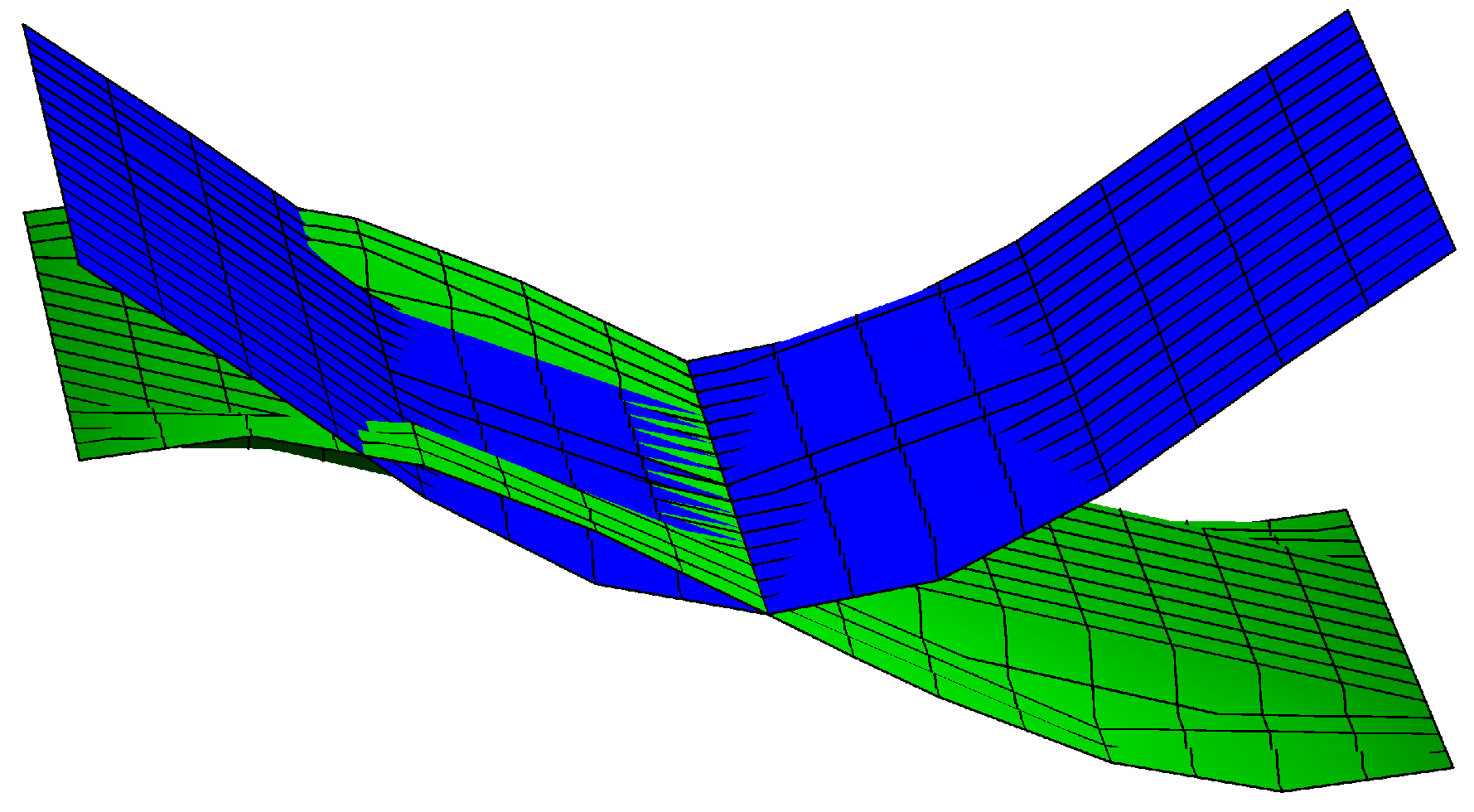
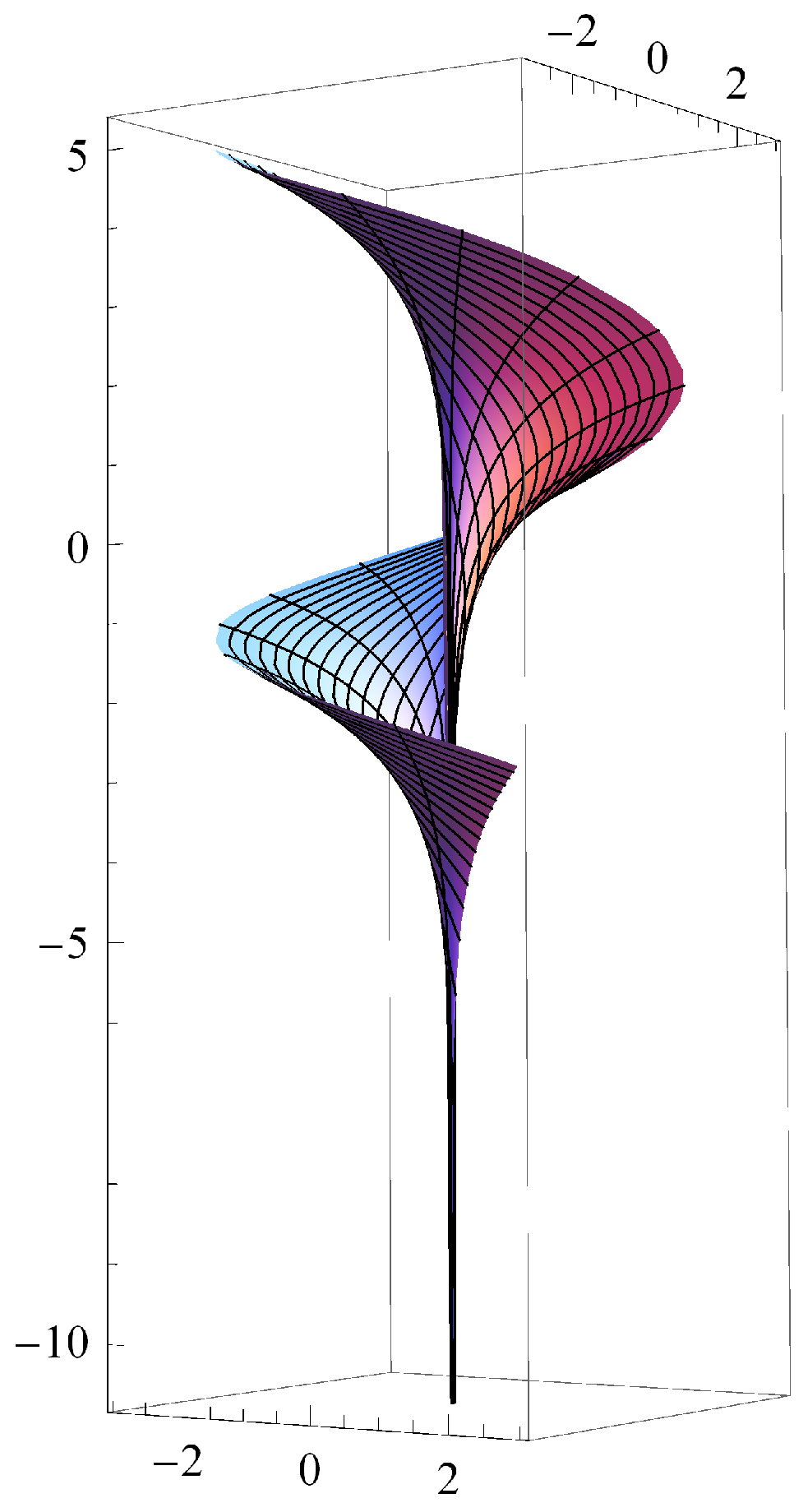
3. Helicoidal Surfaces with Prescribed Weighted Mean or Weighted Extrinsic Curvature
4. Conclusions and Future Work
Funding
Conflicts of Interest
References
- Araujo, K.O.; Cui, N.; Pina, R.D.S. Helicoidal minimal surfaces in a conformally flat 3-space. Bull. Korean Math. Soc. 2016, 53, 531–540. [Google Scholar] [CrossRef]
- Baba-Hamed, C.; Bekkar, M. Helicoidal surfaces in the three-dimensional Lorentz-Minkowski space satisfying ΔIIri = λiri. J. Geom. 2011, 100, 1. [Google Scholar] [CrossRef]
- Choi, M.K.; Kim, Y.H.; Liu, H.; Yoon, D.W. Helicoidal surfaces and their Gauss map in Minkowski 3-space. Bull. Korean Math. Soc. 2010, 47, 859–881. [Google Scholar] [CrossRef]
- Delaunay, C.H. Sur la surface de révolution dont la courbure moyenne est constante. J. Math. Appl. 1841, 6, 309–314. [Google Scholar]
- Do Carmo, M.P.; Dajczer, M. Helicoidal surfaces with constant mean curvature. Tohoku Math. J. 1982, 34, 425–435. [Google Scholar]
- Güler, E.; Turgut Vanli, A. On the mean, gauss, the second gaussıan and the second mean curvature of the Helicoidal surfaces with light-like axis in . Tsukuba J. Math. 2008, 32, 49–65. [Google Scholar] [CrossRef]
- Güler, E. A new kind helicoidal surface of value m. Int. Electron. J. Geom. 2014, 7, 154–162. [Google Scholar]
- Güler, E. Isometric deformation of (m,n)-type helicoidal surface in the three dimensional Euclidean Space. Mathematics 2018, 6, 226. [Google Scholar] [CrossRef]
- Ji, F.; Hou, Z.H. Helicoidal surfaces under the cubic screw motion in Minkowski 3-space. J. Math. Anal. Appl. 2006, 318, 634–647. [Google Scholar] [CrossRef]
- Lee, C.W.; Lee, J.W.; Yoon, D.W. On Helicoidal Surfaces in a Conformally Flat 3-Space. Mediterr. J. Math. 2017, 14, 164. [Google Scholar] [CrossRef]
- Rafael, L.; Demir, E. Helicoidal surfaces in Minkowski space with constant mean curvature and constant Gauss curvature. Open Math. 2014, 12, 1349–1361. [Google Scholar] [Green Version]
- Roussos, I.M. The helicoidal surfaces as Bonnet surfaces. Tohoku Math. J. Second Ser. 1988, 40, 485–490. [Google Scholar] [CrossRef]
- Baikoussis, C.; Koufogiorgos, T. Helicoidal surfaces with prescribed mean or Gaussian curvature. J. Geom. 1998, 63, 25–29. [Google Scholar] [CrossRef]
- Beneki, C.C.; Kaimakamis, G.; Papantoniou, B.J. Helicoidal surfaces in three-dimensional Minkowski space. J. Math. Anal. Appl. 2002, 275, 586–614. [Google Scholar] [CrossRef]
- Ji, F.; Hou, Z.H. A kind of helicoidal surfaces in 3-dimensional Minkowski space. J. Math. Anal. Appl. 2005, 304, 632–643. [Google Scholar] [CrossRef]
- Yoon, D.W.; Kim, D.S.; Kim, Y.H.; Lee, J.W. Constructions of Helicoidal Surfaces in Euclidean Space with Density. Symmetry 2017, 9, 173. [Google Scholar] [CrossRef]
- Yıldız, Ö.G.; Hızal, S.; Akyiğit, M. Type I+ Helicoidal Surfaces with Prescribed Weighted Mean or Gaussian Curvature in Minkowski Space with Density. Anal. Univ. “Ovidius” Constanta-Ser. Mat. 2018, 26, 1–11. [Google Scholar]
- Hıeu, D.T.; Hoang, N.M. Ruled minimal surfaces in with density ez. Pac. J. Math. 2009, 243, 277–285. [Google Scholar]
- Morgan, F. Geometric Measure Theory: A Beginner’s Guide; Academic Press: New York, NY, USA, 2016. [Google Scholar]
- Morgan, F. Manifolds with density. Not. AMS 2005, 52, 853–858. [Google Scholar]
- Morgan, F. Myers’ theorem with density. Kodai Math. J. 2006, 29, 455–461. [Google Scholar] [CrossRef]
- Morgan, F. Manifolds with Density and Perelman’s Proof of the Poincaré Conjecture. Am. Math. Mon. 2009, 116, 134–142. [Google Scholar] [CrossRef]
- Rayón, P.; Gromov, M. Isoperimetry of waists and concentration of maps. Geom. Funct. Anal. 2003, 13, 178–215. [Google Scholar] [CrossRef] [Green Version]
- Rosales, C.; Cañete, A.; Bayle, V.; Morgan, M. On the isoperimetric problem in Euclidean space with density. Calcul. Var. Partial Differ. Equ. 2008, 31, 27–46. [Google Scholar] [CrossRef]
- Yoon, D.W. Weighted minimal translation surfaces in Minkowski 3-space with density. Int. J. Geom. Methods Mod. Phys. 2017, 14, 1750178. [Google Scholar] [CrossRef]
- Pina, A.V.R.; Souza, M. Surfaces of rotation with constant extrinsic curvature in a conformally flat 3-space. Results Math. 2011, 60, 225. [Google Scholar]
- Corwin, I.; Hoffman, N.; Hurder, S.; Šešum, V.; Xu, Y. Differential geometry of manifolds with density. Rose-Hulman Undergrad. Math. J. 2006, 7, 1–15. [Google Scholar]


© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yıldız, Ö.G. Constructions of Helicoidal Surfaces in a 3-Dimensional Complete Manifold with Density. Mathematics 2019, 7, 27. https://doi.org/10.3390/math7010027
Yıldız ÖG. Constructions of Helicoidal Surfaces in a 3-Dimensional Complete Manifold with Density. Mathematics. 2019; 7(1):27. https://doi.org/10.3390/math7010027
Chicago/Turabian StyleYıldız, Önder Gökmen. 2019. "Constructions of Helicoidal Surfaces in a 3-Dimensional Complete Manifold with Density" Mathematics 7, no. 1: 27. https://doi.org/10.3390/math7010027
APA StyleYıldız, Ö. G. (2019). Constructions of Helicoidal Surfaces in a 3-Dimensional Complete Manifold with Density. Mathematics, 7(1), 27. https://doi.org/10.3390/math7010027




