Sculpture from Patchwise Modules
Abstract
:1. Introduction
2. Previous Work
3. Materials and Methods
3.1. Splines and Patches
3.2. Material Constraints
4. Results
4.1. Two-Patch Surfaces
4.2. Four-Patch Surfaces
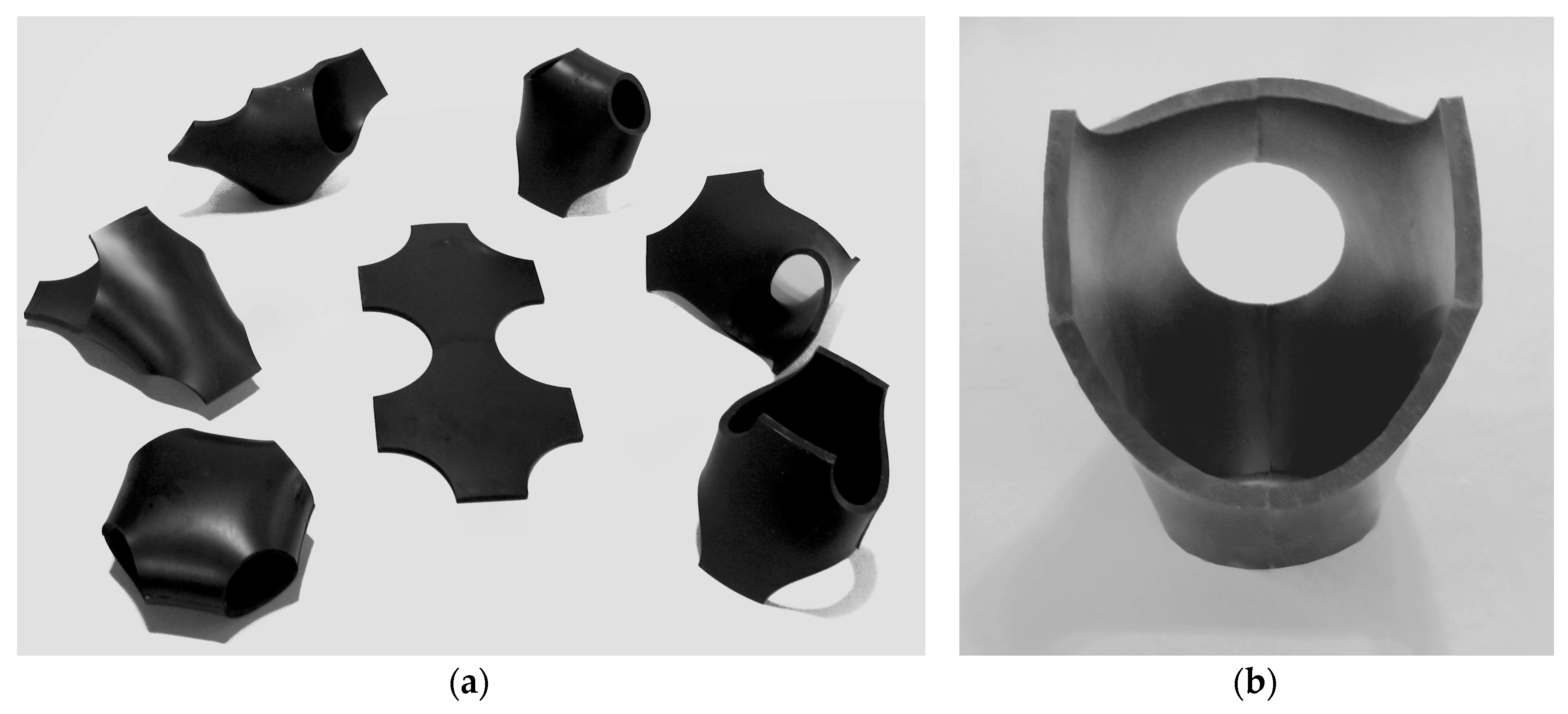
4.3. Six-Patch Surfaces
5. Discussion: Vented Torso
Funding
Conflicts of Interest
References
- Luecking, S. Mathematics Education and Early Abstract Art. In Proceedings of the Bridges: Mathematics, Music, Art, Architecture, Enschede, The Netherlands, 27–31 July 2013. [Google Scholar]
- Vierling-Claassen, A. Models of surfaces and abstract art in the early 20th century. In Bridges 2010; Springer: Berlin/Heidelberg, Germany, 2010; pp. 11–18. [Google Scholar]
- Berger, M.; Chave, A.C.; Kreutzer, M.; Norden, L.; Storr, R.; Hesse, E. Eva Hesse: A Retrospective; Yale University Art Gallery: New Haven, CT, USA, 1992. [Google Scholar]
- Friedman, N. Hypersculptures by Robert Morris, Tony Smith, Arthur Silverman, Richard Serra, and Charles Ginnever. Hyperseeing. Special Issue on ISAMA’07 Sixth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. May 2007, pp. 21–40. Available online: http://www.isama.org/hyperseeing/07/07-05.pdf (accessed on 15 January 2019).
- Oldenburg, C.; Celant, G.; Koepplin, D.; Rosenthal, M.L. Claes Oldenburg: An Anthology; Guggenheim Museum: New York, NY, USA, 1995. [Google Scholar]
- Ferguson, C.; Ferguson, H. Helaman Ferguson: Mathematics in Stone and Bronze; Meridian Creative Group: Erie, PA, USA, 1994. [Google Scholar]
- Helaman, F.; Rockwood, A.; Cox, J. Topological design of sculptured surfaces. Comput. Graph. 1992, 26, 149–156. [Google Scholar]
- Hart, G.W. Sculpture from Symmetrically Arranged Planar Components. In Meeting Alhambra, Proceedings of the ISAMA-Bridges, Granada, Spain, 23–25 July 2003; University of Granada: Granada, Spain, 2003; pp. 315–322. [Google Scholar]
- Hart, G.W. Icosahedral Constructions. In Bridges: Mathematical Connections in Art, Music and Science; Southwestern College: Winfield, KS, USA, 1998. [Google Scholar]
- Hart, G. Modular kirigami. In Bridges Donostia, 2007: Mathematical Connections in Art, Music and Science; Southwestern College: Winfield, KS, USA, 2007; pp. 1–8. [Google Scholar]
- Hart, G. Hyperseeing a Sculpture Based on the (10-3)-a Lattice. Hyperseeing, September 2008; pp. 1–4. Available online: http://www.isama.org/hyperseeing/08/08-e.pdf (accessed on 16 January 2019).
- Hart, G.W. Slide-together: Geometric paper constructions. In Proceedings of the 2004 Bridges Conference on Mathematical Connections in Art, Music, and Science, Winfield, KA, USA, 28 July–1 August 2004. [Google Scholar]
- Friedman, N. Hyperseeing, hypersculptures, and space curves. 1998. Available online: https://www.mi.sanu.ac.rs/vismath/friedman/index.html (accessed on 8 January 2019).
- Friedman, N. Fractal Form and Space. Hyperseeing, March 2007; pp. 7–11. Available online: http://www.isama.org/hyperseeing/07/07-03.pdf (accessed on 8 January 2019).
- Friedman, N. Form, Space and Light. Hyperseeing, July 2007; pp. 1–4. Available online: http://www.isama.org/hyperseeing/07/07-07.pdf (accessed on 3 December 2018).
- Friedman, N. Hypersculptures: Four Right Angles. Hyperseeing, 2011; pp. 13–15. Available online: http://www.isama.org/hyperseeing/11/11a.pdf (accessed on 11 January 2019).
- Friedman, N. Charles Perrry—Ribbed Forms. Hyperseeing, January 2007; pp. 1–3. Available online: http://www.isama.org/hyperseeing/07/07-01.pdf (accessed on 10 January 2019).
- Perry, C. On Mathematical Sculpture. Hyperseeing. Special Issue on ISAMA’08 Seventh Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2008, pp. 11–20. Available online: http://www.isama.org/hyperseeing/08/08-c.pdf (accessed on 12 January 2019).
- Perry, C.O. On the Edge of Science: The Role of the Artist’s Intuition in Science. Leonardo 1992, 25, 249–252. [Google Scholar] [CrossRef]
- Mallos, J. How to Weave a Basket of Arbitrary Shape. Hyperseeing. Special Issue on ISAMA’09 Eight Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2009, pp. 13–19. Available online: http://www.isama.org/hyperseeing/09/09b.pdf (accessed on 20 January 2019).
- Mallos, J. Unit-Weave the Cubic Polyhedra. Hyperseeing. Special Issue on ISAMA’09 Eight Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2009, pp. 113–117. Available online: http://www.isama.org/hyperseeing/09/09b.pdf (accessed on 21 January 2019).
- Mallos, J. Triangle-Strip Knitting. Hyperseeing. Special Issue on ISAMA’10 Nineth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2010, pp. 111–116. Available online: http://www.isama.org/hyperseeing/10/10b.pdf (accessed on 9 January 2019).
- Mallos, J. Extra Ways to See: An Artist’s Guide to Map Operations. Hyperseeing. Special Issue on ISAMA’11 Tenth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2011, pp. 111–121. Available online: http://www.isama.org/hyperseeing/11/11b.pdf (accessed on 13 January 2019).
- Mallos, J. Zip Crochet: Developing a self-sufficient fabric technique for CAD/CAM surfaces. Hyperseeing. Special Issue on SMI 2013/ISAMA2013, Shape Modeling International 2012 Fabrication and Sculpting Event. June 2013, pp. 51–60. Available online: http://www.isama.org/hyperseeing/13/13a.pdf (accessed on 23 January 2019).
- Segerman, H. Recent 3D Printed Sculptures. Hyperseeing, 2012; pp. 33–38. Available online: http://www.isama.org/hyperseeing/11/11c.pdf (accessed on 10 January 2019).
- Segerman, H. 3D printing for mathematical visualisation. Math. Intell. 2012, 34, 56–62. [Google Scholar] [CrossRef]
- Roelofs, R. Weaving with Concrete. Hyperseeing. Special Issue on SMI 2013/ISAMA2013, Shape Modeling International 2012 Fabrication and Sculpting Event. June 2013, pp. 67–74. Available online: http://www.isama.org/hyperseeing/13/13a.pdf (accessed on 14 January 2019).
- Roelofs, R. Two-and three-dimensional constructions based on Leonardo grids. In Nexus Network Journal; Birkhäuser: Basel, Switzerland, 2008; pp. 17–26. [Google Scholar]
- Roelofs, R. About weaving and helical holes. In Bridges 2010: Mathematics, Music, Art, Architecture, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2010; pp. 75–84. [Google Scholar]
- Roelofs, R. Connected Holes. In Proceedings of the 2008 Bridges Conference on Mathematical Connections in Art, Music, and Science, Leeuwarden, The Netherlands, 24–28 July 2008; pp. 30–38. [Google Scholar]
- Roelofs, R. Three-dimensional and dynamic constructions based on Leonardo grids. Int. J. Space Struct. 2007, 22, 191–200. [Google Scholar] [CrossRef]
- Roelofs, R. Elevations and Stellations. In Bridges 2014; Tessellations Publishing: Phoenix, AZ, USA, 2014; pp. 235–260. [Google Scholar]
- Reimann, D.A. Nonplanar expansions of polyhedral edges in platonic and archimedean solids. In Bridges 2015: Mathematics, Music, Art, Architecture, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2015; pp. 143–150. [Google Scholar]
- Reimann, D.A. Transforming Squares to Strips in Expanded Polyhedral Forms. In Bridges 2017: Mathematics, Music, Art, Architecture, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2017; pp. 435–438. [Google Scholar]
- Luecking, S. Zoomorphs: Hyparhedral and Taut Skin Surfaces in Sculpture. Hyperseeing. Special Issue on ISAMA’10 Nineth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2010, pp. 91–102. Available online: http://www.isama.org/hyperseeing/10/10b.pdf (accessed on 17 January 2019).
- Luecking, S. Creating Super-Spheres with Spline Modeling. Hyperseeing. Special Issue on ISAMA’07. Sixth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. May 2007, pp. 41–48. Available online: http://www.isama.org/hyperseeing/07/07-05.pdf (accessed on 15 January 2019).
- Luecking, S. Collapsed Orbs: Astroidal Sculptures from the Breakdown of the Sphere. Hyperseeing. Special Issue on ISAMA’09 Eight Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2009, pp. 57–62. Available online: http://www.isama.org/hyperseeing/09/09b.pdf (accessed on 22 January 2019).
- Luecking, S. Constructing and Tiling Hypar Kites. Hyperseeing. Special Issue on ISAMA’10 Nineth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2010, pp. 83–90. Available online: http://www.isama.org/hyperseeing/10/10b.pdf (accessed on 9 January 2019).
- Luecking, S. Octoids: Sculpting Between Octahedron and Sphere. Hyperseeing. Special Issue on ISAMA’11 Tenth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2011, pp. 89–91. Available online: http://www.isama.org/hyperseeing/11/11b.pdf (accessed on 11 January 2019).
- Luecking, S. Building a Scherk Surface from Paper Tiles. Hyperseeing. Special Issue on ISAMA’11 Tenth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2011, pp. 101–104. Available online: http://www.isama.org/hyperseeing/11/11b.pdf (accessed on 7 January 2019).
- Luecking, S. Sculpture from a Space Filling Saddle Pentahedron. Hyperseeing. Special Issue on ISAMA’11 Tenth Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2011, pp. 105–109. Available online: http://www.isama.org/hyperseeing/11/11b.pdf (accessed on 7 January 2019).
- Luecking, S. Contour Armatures and Faired Surfaces in Combinative Sculpture. Hyperseeing. Special Issue on SMI 2012, Shape Modeling International 2012 Fabrication and Sculpting Event. June 2012, pp. 51–60. Available online: http://www.isama.org/hyperseeing/12/12a.pdf (accessed on 5 January 2019).
- Luecking, S. Design and Construction of Sunder Planet: Spherical Polyhedrons from Pyramid Modules. Hyperseeing. Special Issue on SMI 2013/ISAMA2013, Shape Modeling International 2012 Fabrication and Sculpting Event. June 2013, pp. 9–17. Available online: http://www.isama.org/hyperseeing/13/13a.pdf (accessed on 4 January 2019).
- Luecking, S. Intuiting Topology: Sculptures of Bruce White. Hyperseeing, March 2007; pp. 4–7. Available online: http://www.isama.org/hyperseeing/07/07-03.pdf (accessed on 11 January 2019).
- Morris, R. Notes on sculpture. In Minimal Art: A Critical Anthology; University of California Press: Oakland, CA, USA, 1966; pp. 222–235. [Google Scholar]
- Friedman, N. Robert Longhurst: Three Sculptures. Hyperseeing, July 2007; pp. 8–12. Available online: http://www.isama.org/hyperseeing/07/07-07.pdf (accessed on 15 January 2019).
- Cantrell, A.; Longhurst, R.; Schwalbe, D.; Wagon, S.; Bruning, J. Rhapsody in White: A victory for mathematics. Math. Intell. 2000, 22, 37–40. [Google Scholar] [CrossRef]
- Friedman, N.; Luecking, S.; Kapoor, A. Cloud Gate, Hyperseeing: Journal of the 187 International Society for Art, Mathematics and Architecture. 7 July 2007, pp. 13–17. Available online: http://www.isama.org/hyperseeing/07/07-07.pdf (accessed on 18 January 2019).
- Jones, M. Kapoorian geographies of relationality: The Baroque, topological twists, phase space in action. Environ. Plan. A 2014, 46, 2585–2603. [Google Scholar] [CrossRef]
- Dodgson, N.A. Engineering Art and Telling Tales: Anish Kapoor at the Royal Academy. Interdiscipl. Sci. Rev. 2016, 41, 281–296. [Google Scholar] [CrossRef]
- Friedman, N. Larry Frazier: Topological Surfaces. Hyperseeing, July 2007; pp. 5–7. Available online: http://www.isama.org/hyperseeing/07/07-08.pdf (accessed on 15 January 2019).
- Friedman, N. Giant Steps by Charles Ginnever. Hyperseeing. Special Issue on ISAMA’08 Seventh Interdisciplinary Conference of the International Society of the Arts, Mathematics, and Architecture. June 2008, pp. 21–28. Available online: http://www.isama.org/hyperseeing/08/08-c.pdf (accessed on 12 January 2019).
- Friedman, N. Bathsheba Grossman at Shapeways. Hyperseeing, 2009; pp. 28–30. Available online: http://www.isama.org/hyperseeing/08/08-e.pdf (accessed on 12 January 2019).
- Grossman, B. Sculptor & Ubernerd. Available online: https://www.egconf.com/videos/bathsheba-grossman-sculptor-ubernerd-eg7 (accessed on 17 February 2019).
- Adler, S. Bathsheba’s Secrets. Available online: https://www.jckonline.com/editorial-article/bathshebas-secrets (accessed on 17 February 2019).
- Senie, H.F. Re-approaching Tony Smith. Sculpture 1998, 17, 8–43. [Google Scholar]
- Friedman, N. Serra Spaces. Hyperseeing, 2010; pp. 9–19. Available online: http://www.isama.org/hyperseeing/10/10a.pdf (accessed on 16/1 2019).
- Friedman, N.; Séquin, C. H Keizo Ushio’s Sculptrue: Split Tori and Moebius Bands. Available online: https://www. maths.ed.ac.uk/~v1ranick/papers/keizo.pdf (accessed on 17 February 2019).
- Séquin, C.H. 15 Years of Scherk-Collins Saddle Chains. Hyperseeing, 2010; pp. 1–8. Available online: http://www.isama.org/hyperseeing/10/10a.pdf (accessed on 19 January 2019).
- Séquin, C.H. Virtual prototyping of scherk-collins saddle rings. Leonardo 1997, 30, 89–96. [Google Scholar] [CrossRef]
- Séquin, C.H.; Meshkin, H.; Downs, L. Interactive generation of Scherk-Collins sculptures. In Proceedings of the 1997 Symposium on Interactive 3D Graphics, Providence, RI, USA, 27–30 April 1997. [Google Scholar]
- Séquin, C.H. To build a twisted bridge. In Bridges 2000: Mathematics, Art, Music, Architecture, Education, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2000; pp. 23–34. [Google Scholar]
- Séquin, C.H. Splitting Tori, Knots, and Möbius Bands. In Bridges 2005: Mathematics, Art, Music, Architecture, Education, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2005; pp. 245–254. [Google Scholar]
- Séquin, C.H. 2-Manifold Sculptures. In Bridges 2015: Mathematics, Art, Music, Architecture, Education, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2015; pp. 17–26. [Google Scholar]
- Akgün, T.; Kaya, I. Spiral Developable Sculptures of Ilhan Koman. In 2007 Bridges Conference in Art, Music, and Science; Tessellations Publishing: Phoenix, AZ, USA, 2007; pp. 47–52. [Google Scholar]
- Akgün, T.; Koman, A.; Akleman, E. Paper Sculptures with Vertex Deflection. In Bridges London: Mathematics, Music, Art, Architecture, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2006; pp. 639–640. [Google Scholar]
- Friedman, N. Eva Hild: Sculpture and Light. Hyperseeing, July 2007; pp. 1–5. Available online: http://www.isama.org/hyperseeing/07/07-08.pdf (accessed on 15 January 2019).
- Hild, E.; Schwalbe, D.; Seeley, R.; Seeley, B.H.; Wagon, S. Eva Hild’s Perpetual Motion. Hyperseeing, 2011; pp. 1–8. Available online: http://www.isama.org/hyperseeing/11/11a.pdf (accessed on 14 December 2018).
- Friedman, N. Eva Hild: Large-scale Sculptures. Hyperseeing, 2012; pp. 23–32. Available online: http://www.isama.org/hyperseeing/11/11c.pdf (accessed on 5 January 2019).
- Friedman, N. Eva Hild: Topological Sculpture from Life Experience. In Bridges London: Mathematics, Music, Art, Architecture, Culture; Tarquin Publications: St. Albans, UK, 2006; pp. 569–572. [Google Scholar]
- Swart, D.; Séquin, C.H.; Fenyvesi, K. Homage to Eva Hild. In Bridges 2017: Mathematics, Art, Music, Architecture, Education, Culture; Tessellations Publishing: Phoenix, AZ, USA, 2017; pp. 117–124. [Google Scholar]
- Hernandez, E.A.P.; Hu, S.; Kung, H.W.; Hartl, D.; Akleman, E. Towards building smart self-folding structures. Comput. Graph. 2013, 37, 730–742. [Google Scholar] [CrossRef]
- Hernandez, E.A.P.; Hartl, D.J.; Akleman, E.; Lagoudas, D.C. Modeling and analysis of origami structures with smooth folds. Comput.-Aided Des. 2016, 78, 93–106. [Google Scholar] [CrossRef]
- Miller, J.; Akleman, E. Edge-based intersected polyhedral paper sculptures constructed by interlocking slitted planar pieces. In Bridges Leeuwarden: Mathematics, Music, Art, Architecture, Culture; Tarquin Publications: St. Albans, UK, 2008; pp. 259–264. [Google Scholar]
- Akleman, E.; Ke, S.; Wu, Y. Physical mesh data structures. In ACM SIGGRAPH 2016 Talks; ACM: New York, NY, USA, 2016; p. 9. [Google Scholar]
- Akleman, E.; Ke, S.; Wu, Y.; Kalantar, N.; Borhani, A.; Chen, J. Construction with physical version of quad-edge data structures. Comput. Graph. 2016, 58, 172–183. [Google Scholar] [CrossRef]
- Ergun, A.; Ozener, O.; Yuksel, C. On a Family of Symmetric, Connected and High Genus Sculptures. In Proceedings of the Bridges. 2006. Available online: https://pdfs.semanticscholar.org/a310/b40382c3dacac4e1b081deb4344925cd9857.pdf (accessed on 21 December 2018).
- Bartels, R.H.; Beatty, J.C.; Barsky, B.A. An Introduction to Splines for Use in Computer Graphics and Geometric Modeling; Morgan Kaufmann: Burlington, MA, USA, 1995. [Google Scholar]
- Grandine, T.A. The Extensive Use of Splines at Boeing; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2005; Volume 38. [Google Scholar]










© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luecking, S. Sculpture from Patchwise Modules. Mathematics 2019, 7, 197. https://doi.org/10.3390/math7020197
Luecking S. Sculpture from Patchwise Modules. Mathematics. 2019; 7(2):197. https://doi.org/10.3390/math7020197
Chicago/Turabian StyleLuecking, Stephen. 2019. "Sculpture from Patchwise Modules" Mathematics 7, no. 2: 197. https://doi.org/10.3390/math7020197
APA StyleLuecking, S. (2019). Sculpture from Patchwise Modules. Mathematics, 7(2), 197. https://doi.org/10.3390/math7020197




