Solving Non-Linear Fractional Variational Problems Using Jacobi Polynomials
Abstract
:1. Introduction
2. Basic Preliminaries
3. Operational Matrices
4. Method of Solution
5. Error Analysis
6. Convergence Analysis
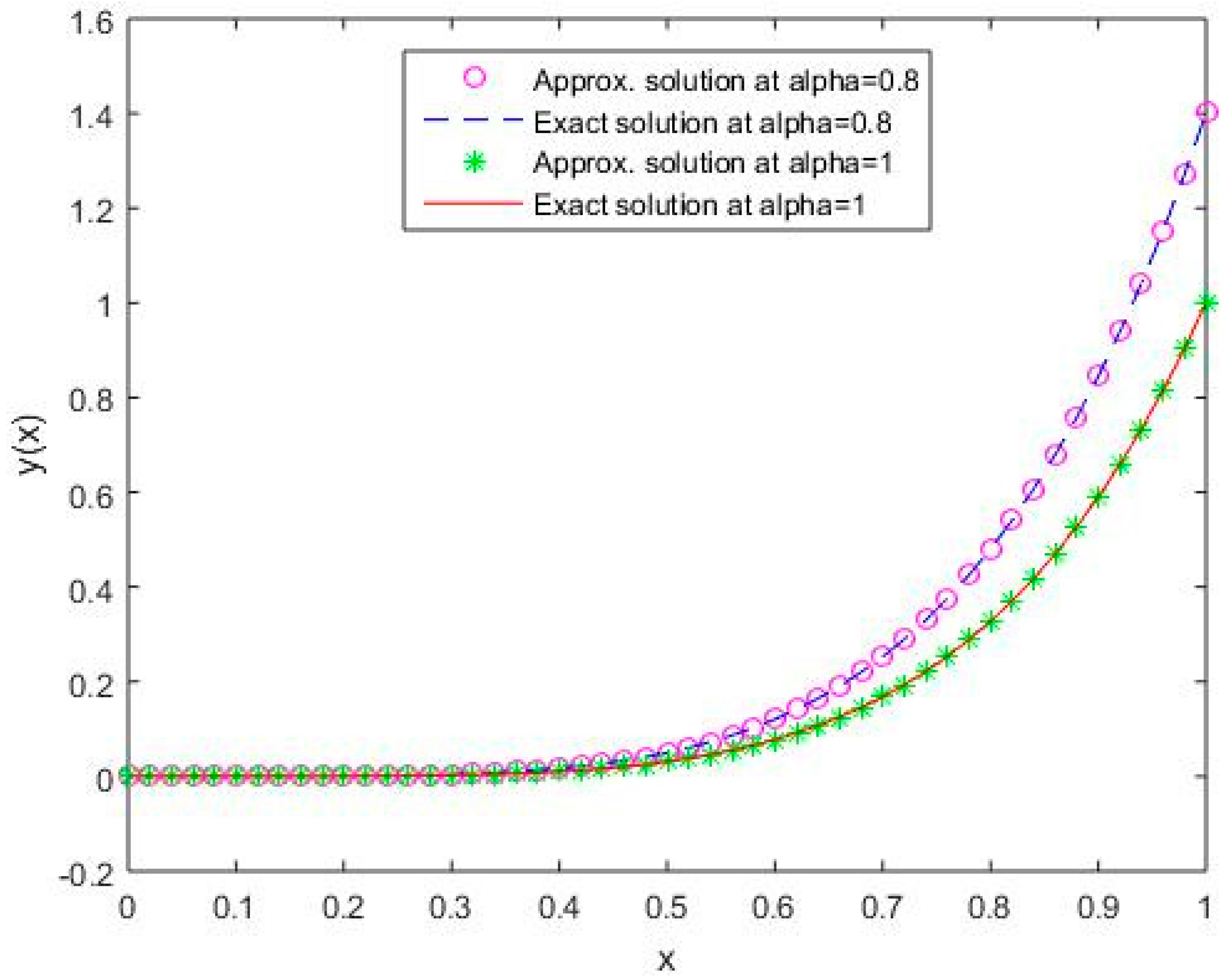
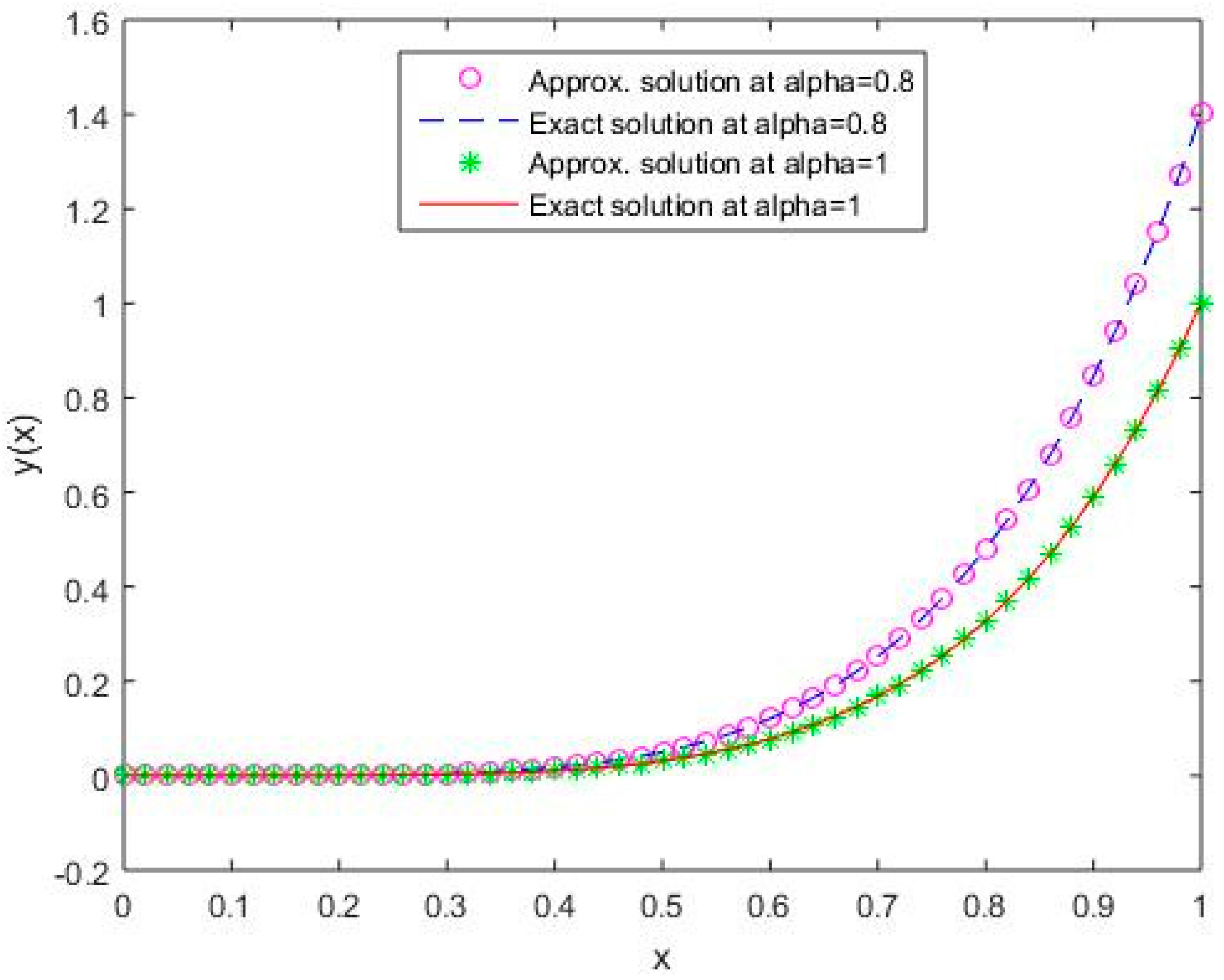
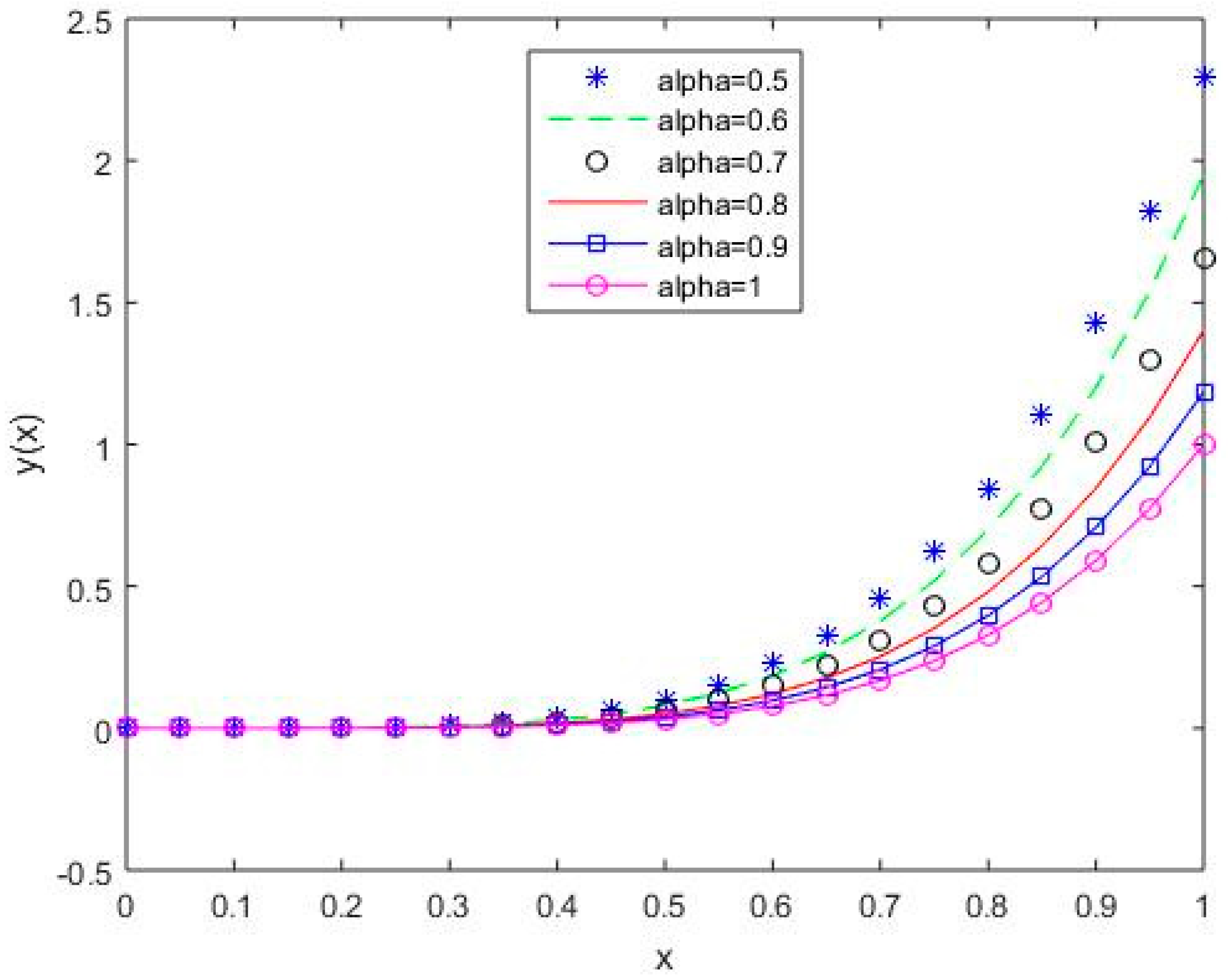
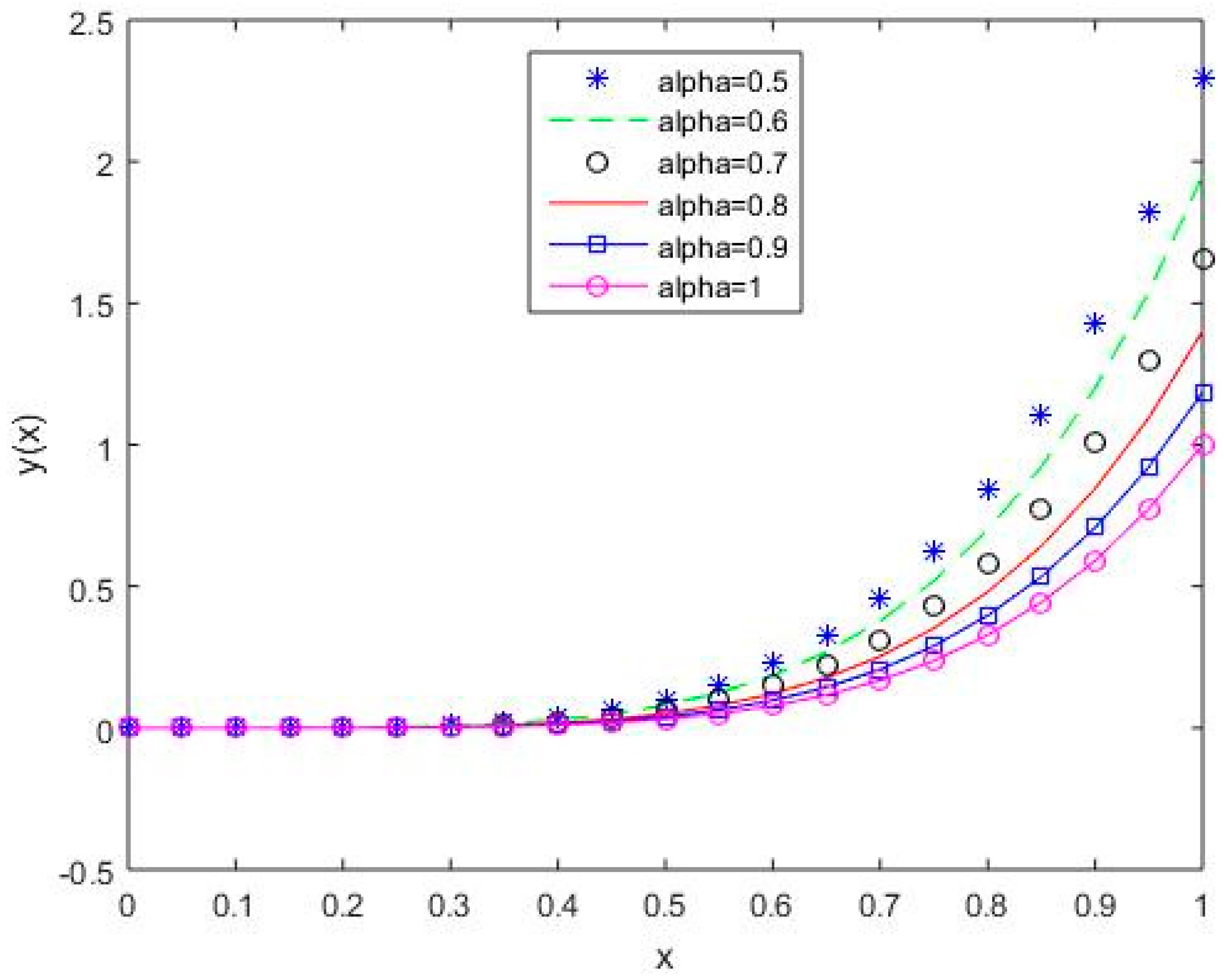
7. Numerical Results and Discussions
8. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Dym, C.L.; Shames, I.H. Solid Mechanics: A Variational Approach; McGraw-Hill: New York, NY, USA, 1973. [Google Scholar]
- Frederico, G.S.F.; Torres, D.F.M. Fractional conservation laws in optimal control theory. Nonlinear Dyn. 2008, 53, 215–222. [Google Scholar] [CrossRef]
- Pirvan, M.; Udriste, C. Optimal control of electromagnetic energy. Balk. J. Geom. Appl. 2010, 15, 131–141. [Google Scholar]
- Agrawal, O.P. A general finite element formulation for fractional variational problems. J. Math. Anal. Appl. 2008, 337, 1–12. [Google Scholar] [CrossRef]
- Dehghan, M.; Tatari, M. The use of Adomian decomposition method for solving problems in calculus of variations. Math. Probl. Eng. 2006, 2006, 1–12. [Google Scholar] [CrossRef]
- Singh, H. A new stable algorithm for fractional Navier-Stokes equation in polar coordinate. Int. J. Appl. Comp. Math. 2017, 3, 3705–3722. [Google Scholar] [CrossRef]
- Robinson, A.D. The use of control systems analysis in neurophysiology of eye movements. Ann. Rev. Neurosci. 1981, 4, 462–503. [Google Scholar] [CrossRef] [PubMed]
- Singh, H. Operational matrix approach for approximate solution of fractional model of Bloch equation. J. King Saud Univ.-Sci. 2017, 29, 235–240. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus a differential approach to the analysis of viscoelasticity damped structures. AIAA J. 1983, 21, 741–748. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus in the transient analysis of viscoelasticity damped structures. AIAA J. 1985, 23, 918–925. [Google Scholar] [CrossRef]
- Panda, R.; Dash, M. Fractional generalized splines and signal processing. Signal Process. 2006, 86, 2340–2350. [Google Scholar] [CrossRef]
- Magin, R.L. Fractional calculus in bioengineering. Crit. Rev. Biomed. Eng. 2004, 32, 1–104. [Google Scholar] [CrossRef] [PubMed]
- Bohannan, G.W. Analog fractional order controller in temperature and motor control applications. J. Vib. Control. 2008, 14, 1487–1498. [Google Scholar] [CrossRef]
- Novikov, V.V.; Wojciechowski, K.W.; Komkova, O.A.; Thiel, T. Anomalous relaxation in dielectrics. Equations with fractional derivatives. Mater. Sci. 2005, 23, 977–984. [Google Scholar]
- Agrawal, O.P. A new Lagrangian and a new Lagrange equation of motion for fractionally damped systems. J. Appl. Mech. 2013, 237, 339–341. [Google Scholar] [CrossRef]
- Mozyrska, D.; Torres, D.F.M. Minimal modified energy control for fractional linear control systems with the Caputo derivative. Carpath. J. Math. 2010, 26, 210–221. [Google Scholar]
- Almeida, R.; Malinowska, R.; Torres, D.F.M. A fractional calculus of variations for multiple integrals with application to vibrating string. J. Math. Phys. 2010, 51, 033503. [Google Scholar] [CrossRef]
- Lotfi, A.; Dehghan, M.; Yousefi, S.A. A numerical technique for solving fractional optimal control problems. Comput. Math. Appl. 2011, 62, 1055–1067. [Google Scholar] [CrossRef]
- Khosravian-Arab, H.; Almeida, R. Numerical solution for fractional variational problems using the Jacobi polynomials. Appl. Math. Modell. 2015. [Google Scholar] [CrossRef]
- Agrawal, O.P. Formulation of Euler-Lagrange equations for fractional variational problems. J. Math. Anal. Appl. 2002, 272, 368–379. [Google Scholar] [CrossRef]
- Doha, E.H.; Bhrawy, A.H.; Baleanu, D.; Ezz-Eldien, S.S.; Hafez, R.M. An efficient numerical scheme based on the shifted orthonormal Jacobi polynomials for solving fractional optimal control problems. Adv. Differ. Equ. 2015. [Google Scholar] [CrossRef]
- Ezz-Eldie, S.S.; Doha, E.H.; Baleanu, D.; Bhrawy, A.H. A numerical approach based on Legendre orthonormal polynomials for numerical solutions of fractional optimal control problems. J. Vib. Ctrl. 2015. [Google Scholar] [CrossRef]
- Osama, H.M.; Fadhel, S.F.; Zaid, A.M. Numerical solution of fractional variational problems using direct Haar wavelet method. Int. J. Innov. Res. Sci. Eng. Technol. 2014, 3, 12742–12750. [Google Scholar]
- Ezz-Eldien, S.S. New quadrature approach based on operational matrix for solving a class of fractional variational problems. J. Comp. Phys. 2016, 317, 362–381. [Google Scholar] [CrossRef]
- Bastos, N.; Ferreira, R.; Torres, D.F.M. Discrete-time fractional variational problems. Signal Process. 2011, 91, 513–524. [Google Scholar] [CrossRef]
- Wang, D.; Xiao, A. Fractional variational integrators for fractional variational problems. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 602–610. [Google Scholar] [CrossRef]
- Odzijewicz, T.; Malinowska, A.B.; Torres, D.F.M. Fractional variational calculus with classical and combined Caputo derivatives. Nonlinear Anal. 2012, 75, 1507–1515. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Ezz-Eldien, S.S. A new Legendre operational technique for delay fractional optimal control problems. Calcolo 2015. [Google Scholar] [CrossRef]
- Tavares, D.; Almeida, R.; Torres, D.F.M. Optimality conditions for fractional variational problems with dependence on a combined Caputo derivative of variable order. Optimization 2015, 64, 1381–1391. [Google Scholar] [CrossRef]
- Ezz-Eldien, S.S.; Hafez, R.M.; Bhrawy, A.H.; Baleanu, D.; El-Kalaawy, A.A. New numerical approach for fractional variational problems using shifted Legendre orthonormal polynomials. J. Optim. Theory Appl. 2017, 174, 295–320. [Google Scholar] [CrossRef]
- Almeida, R. Variational problems involving a Caputo-type fractional derivative. J. Optim. Theory Appl. 2017, 174, 276–294. [Google Scholar] [CrossRef]
- Pandey, R.K.; Agrawal, O.P. Numerical Scheme for Generalized Isoparametric Constraint Variational Problems with A-Operator. In Proceedings of the ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Portland, OR, USA, 4–7 August 2013. [Google Scholar] [CrossRef]
- Pandey, R.K.; Agrawal, O.P. Numerical scheme for a quadratic type generalized isoperimetric constraint variational problems with A.-operator. J. Comput. Nonlinear Dyn. 2015, 10, 021003. [Google Scholar] [CrossRef]
- Pandey, R.K.; Agrawal, O.P. Comparison of four numerical schemes for isoperimetric constraint fractional variational problems with A-operator. In Proceedings of the ASME 2015 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Boston, MA, USA, 2–5 August 2015. [Google Scholar] [CrossRef]
- Singh, H.; Srivastava, H.M.D.; Kumar, D. A reliable numerical algorithm for the fractional vibration equation. Chaos Solitons Fractals 2017, 103, 131–138. [Google Scholar] [CrossRef]
- Singh, O.P.; Singh, V.K.; Pandey, R.K. A stable numerical inversion of Abel’s integral equation using almost Bernstein operational matrix. J. Quant. Spec. Rad. Trans. 2012, 111, 567–579. [Google Scholar] [CrossRef]
- Zhou, F.; Xu, X. Numerical solution of convection diffusions equations by the second kind Chebyshev wavelets. Appl. Math. Comput. 2014, 247, 353–367. [Google Scholar] [CrossRef]
- Yousefi, S.A.; Behroozifar, M.; Dehghan, M. The operational matrices of Bernstein polynomials for solving the parabolic equation subject to the specification of the mass. J. Comput. Appl. Math. 2011, 235, 5272–5283. [Google Scholar] [CrossRef]
- Singh, H. A New Numerical Algorithm for Fractional Model of Bloch Equation in Nuclear Magnetic Resonance. Alex. Eng. J. 2016, 55, 2863–2869. [Google Scholar] [CrossRef]
- Khalil, H.; Khan, R.A. A new method based on Legendre polynomials for solutions of the fractional two dimensional heat conduction equations. Comput. Math. Appl. 2014, 67, 1938–1953. [Google Scholar] [CrossRef]
- Singh, C.S.; Singh, H.; Singh, V.K.; Singh, O.P. Fractional order operational matrix methods for fractional singular integro-differential equation. Appl. Math. Modell. 2016, 40, 10705–10718. [Google Scholar] [CrossRef]
- Rivlin, T.J. An Introduction to the Approximation of Functions; Dover Publication: New York, NY, USA, 1981. [Google Scholar]
- Kreyszig, E. Introductory Functional Analysis with Applications; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 1978. [Google Scholar]
- Bhrawy, A.H.; Tharwat, M.M.; Alghamdi, M.A. A new operational matrix of fractional integration for shifted Jacobi polynomials. Bull. Malays. Math. Sci. Soc. 2014, 37, 983–995. [Google Scholar]
- Behroozifar, M.; Sazmand, A. An approximate solution based on Jacobi polynomials for time-fractional convection–diffusion equation. Appl. Math. Comput. 2017, 296, 1–17. [Google Scholar] [CrossRef]









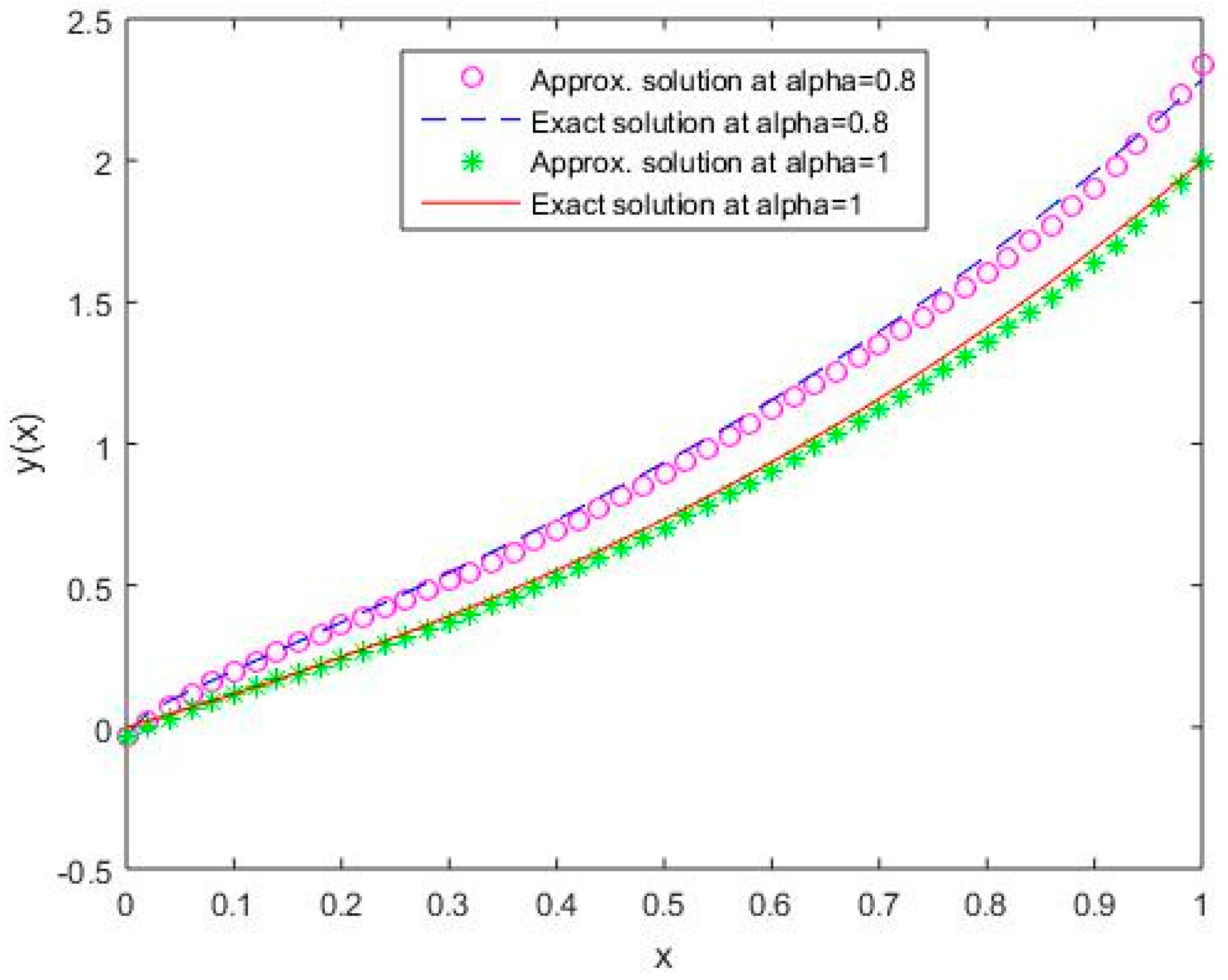





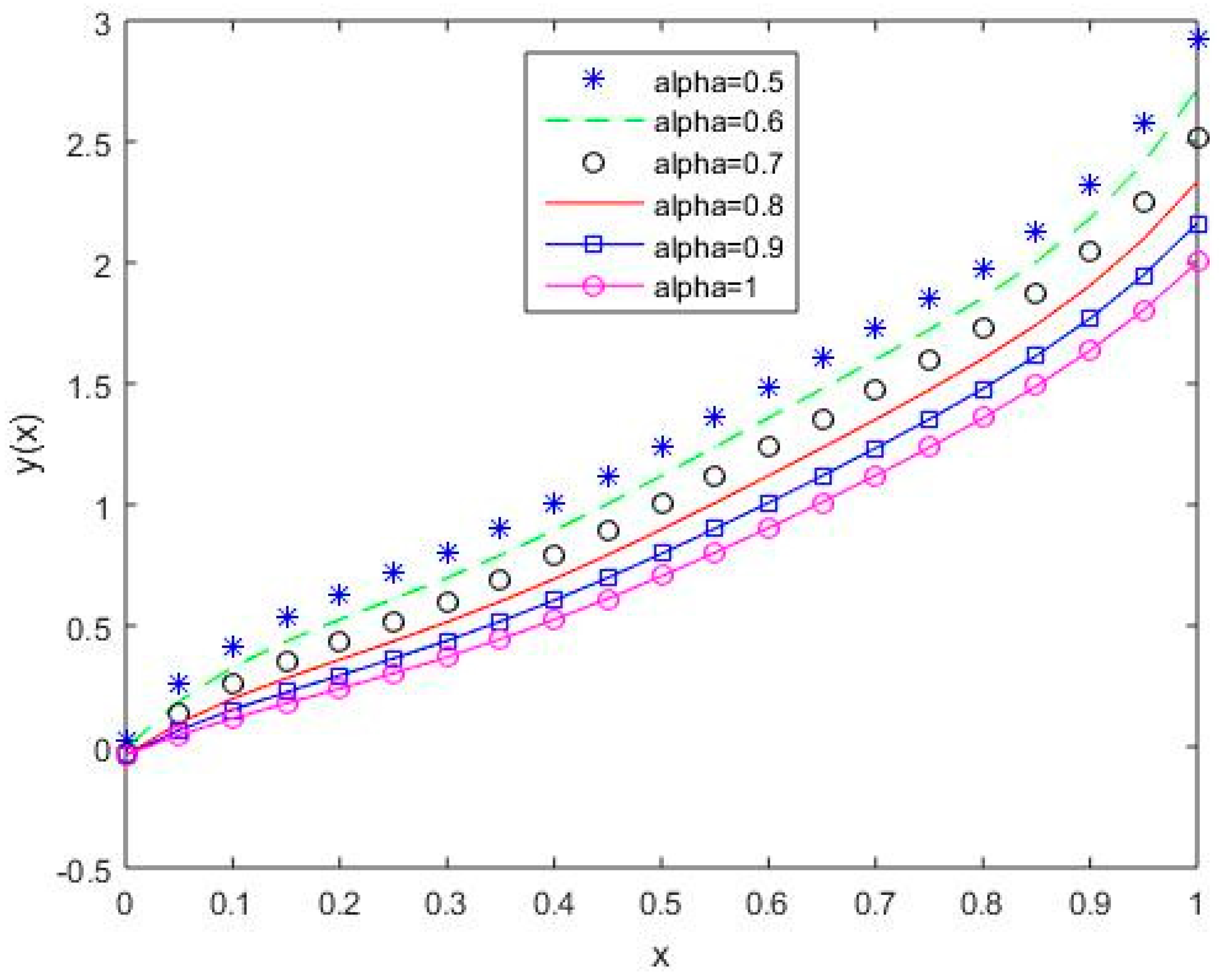
| Polynomials | Maximum Absolute Errors | Root-Mean-Square Errors | ||
|---|---|---|---|---|
| n = 2 | n = 6 | n = 2 | n = 6 | |
| S1 | 1.4584 × 10−1 | 1.8326 × 10−7 | 1.3923 × 10−2 | 2.4900 × 10−8 |
| S2 | 1.6154 × 10−1 | 4.2127 × 10−7 | 2.0960 × 10−2 | 7.1127 × 10−8 |
| S3 | 2.2296 × 10−1 | 3.6897 × 10−7 | 1.3179 × 10−2 | 3.3726 × 10−8 |
| S4 | 4.1764 × 10−1 | 1.0973 × 10−6 | 3.2039 × 10−2 | 1.7307 × 10−7 |
| S5 | 1.9055 × 10−1 | 3.1593 × 10−7 | 2.2138 × 10−2 | 4.2368 × 10−8 |
| Polynomials | Maximum Absolute Errors | Root-Mean-Square Errors | ||
|---|---|---|---|---|
| n = 2 | n = 6 | n = 2 | n = 6 | |
| S1 | 2.0407 × 10−2 | 1.4819 × 10−7 | 2.7038 × 10−3 | 1.5021 × 10−8 |
| S2 | 2.4295 × 10−2 | 1.3713 × 10−2 | 3.1356 × 10−3 | 1.6490 × 10−3 |
| S3 | 8.0010 × 10−2 | 4.8371 × 10−2 | 1.2590 × 10−2 | 8.3236 × 10−3 |
| S4 | 1.1193 × 10−1 | 5.0558 × 10−2 | 9.8251 × 10−3 | 6.8594 × 10−3 |
| S5 | 2.5349 × 10−2 | 1.9316 × 10−2 | 3.2343 × 10−3 | 2.4746 × 10−3 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, H.; Pandey, R.K.; Srivastava, H.M. Solving Non-Linear Fractional Variational Problems Using Jacobi Polynomials. Mathematics 2019, 7, 224. https://doi.org/10.3390/math7030224
Singh H, Pandey RK, Srivastava HM. Solving Non-Linear Fractional Variational Problems Using Jacobi Polynomials. Mathematics. 2019; 7(3):224. https://doi.org/10.3390/math7030224
Chicago/Turabian StyleSingh, Harendra, Rajesh K. Pandey, and Hari Mohan Srivastava. 2019. "Solving Non-Linear Fractional Variational Problems Using Jacobi Polynomials" Mathematics 7, no. 3: 224. https://doi.org/10.3390/math7030224
APA StyleSingh, H., Pandey, R. K., & Srivastava, H. M. (2019). Solving Non-Linear Fractional Variational Problems Using Jacobi Polynomials. Mathematics, 7(3), 224. https://doi.org/10.3390/math7030224







