Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine
Abstract
:1. Introduction
2. Numerical Details
2.1. Geometry and Grid
2.2. Governing Equations and Turbulence Model
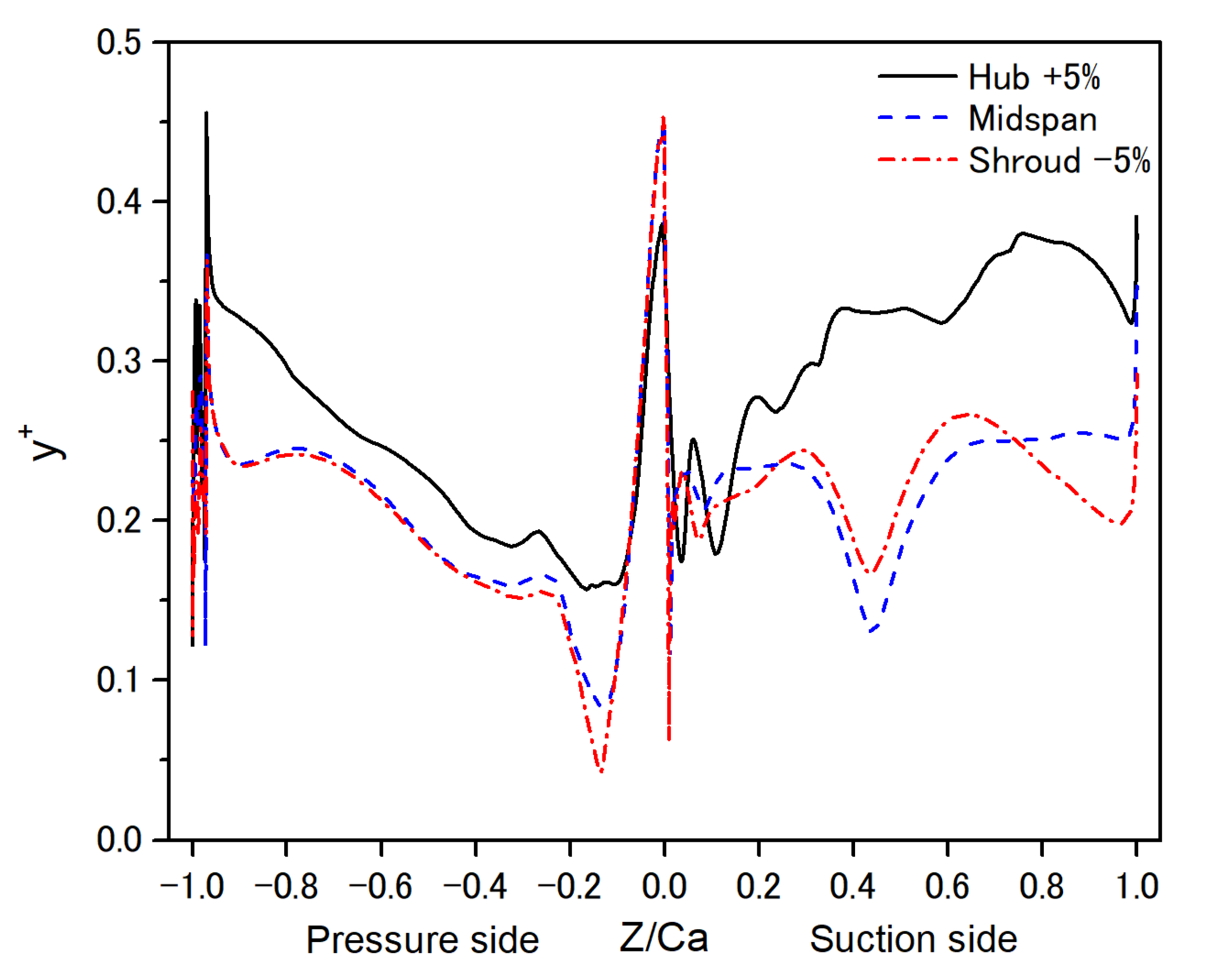
2.3. Boundary Conditions and Unsteady Simulation
3. Results and Discussion
3.1. Flow Characteristics
3.2. Heat Transfer Characteristics
3.3. Aerodynamic and Total-to-Total Efficiencies
4. Conclusions
- −
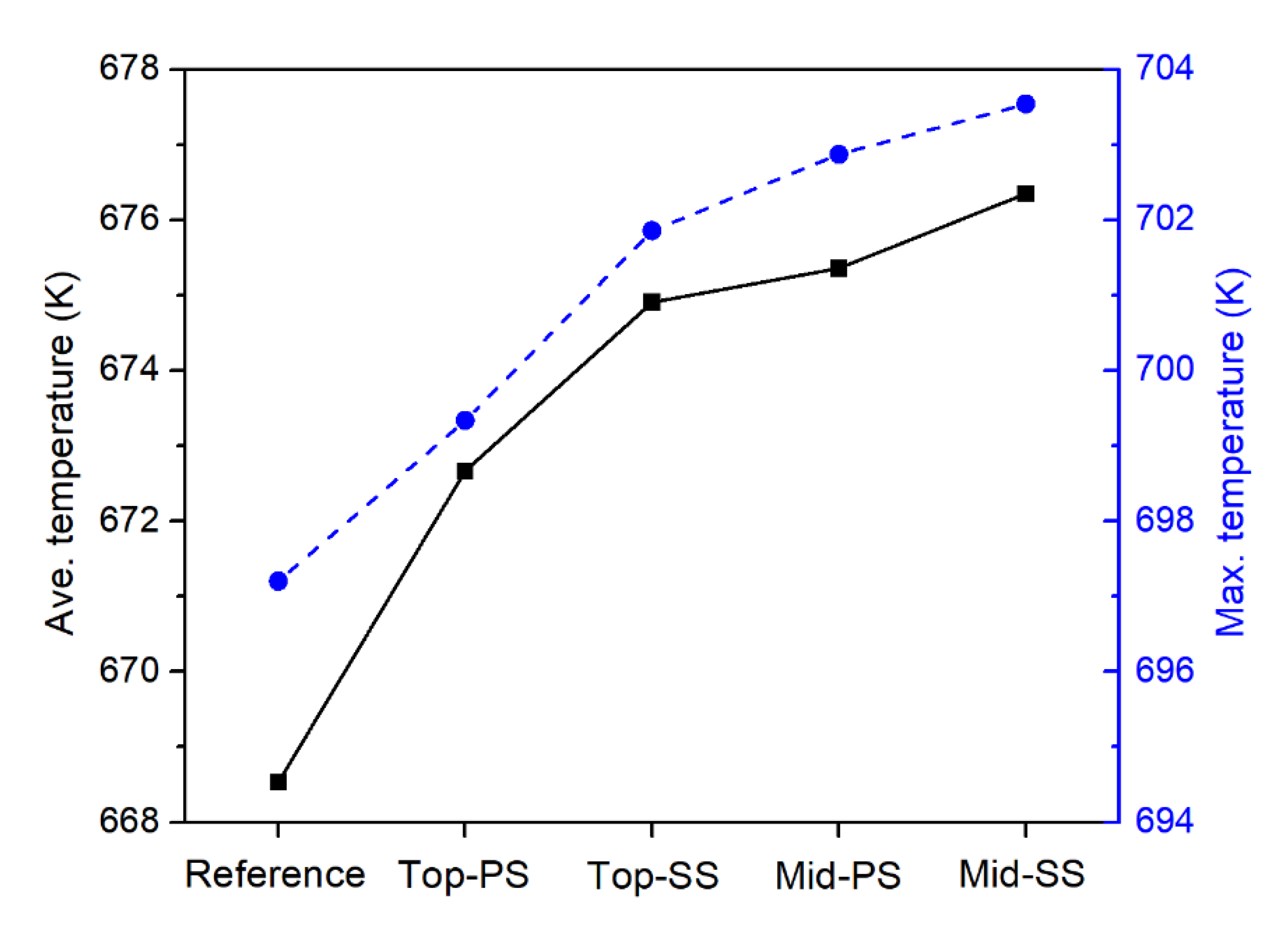
- The average and maximum temperatures on the R1 blade and S2 vane surfaces of the damaged blades were higher than those in the reference case. This was due to the effects of the altered flow field profiles on the damaged blades.
- −
- The tip leakage flow increased in the middle-damage cases but decreased in the top-damage cases, compared to the reference case.
- −
- The heat transfer on the blade tip in the middle-damage cases was remarkably higher than the one in the other cases.
- −
- The location of the damage had significant effects on the heat transfer characteristics on the blade and vane surfaces. On the R1 blade, the heat flux around the damage location exhibited a sudden increase. The heat flux on the S2 vane surface considerably increased around the mid-span on the pressure side and around the hub and tip on the suction side. This led to an increase in the local thermal stress, showing a potential reduction in the fatigue life of the blade and the vane which would increase the maintenance costs.
- −
- Moreover, the modifications to the top-damaged blades enhanced the aerodynamic and total-to-total efficiencies, while the same for the middle-damaged blades caused reductions in the efficiencies.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Fluid density (kg/ | Vorticity magnitude | ||
| u | Fluid velocity (m/s) | Critical Reynolds number | |
| P | Fluid pressure (Pa) | Stanton number | |
| Fluid viscosity (Pa.s) | Heat flux (W/ | ||
| E | Specific internal energy (J) | Temperature of the wall surface (K) | |
| Effective thermal conductivity [(W/m.K) | Average total temperature of the inlet flow (K) | ||
| Effective dynamic viscosity (Pa.s) | Specific heat of ideal air (J/kg.K) | ||
| Turbulence viscosity (Pa.s) | Average density of the inlet flow (kg/ | ||
| Blending functions | Average velocity of the inlet flow (m/s) | ||
| Turbulence kinetic energy (J/kg) | Average total pressure of inlet flow (Pa) | ||
| Eddy dissipation/ Angular velocity (rad/s) | Torque (N.m)/Temperature (K) | ||
| Turbulent Prandtl number for | Mass flow rate (kg/s) | ||
| Turbulent Prandtl number for | κ | Ratio of specific heat | |
| Intermittency | Lift force (N) | ||
| S | Strain rate magnitude () | Drag force (N) | |
| Empirical correlation | Aerodynamic efficiency | ||
| Total-to-total efficiency |
References
- Povey, T.; Qureshi, I. Developments in hot-streak simulators for turbine testing. J. Turbomach. 2009, 131, 031009-1–031009-15. [Google Scholar] [CrossRef]
- An, B.-T.; Liu, J.-J.; Jiang, H.-D. Numerical investigation on unsteady effects of hot streak on flow and heat transfer in a turbine stage. J. Turbomach. 2009, 131, 031015-1–031015-15. [Google Scholar] [CrossRef]
- Anzellini, S.; Monteseguro, V.; Bandiello, E.; Dewaele, A.; Burakovsky, L.; Errandonea, D. In situ characterization of the high pressure–high temperature melting curve of platinum. Sci. Rep. 2019, 9, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Errandonea, D.; Burakovsky, L.; Preston, D.L.; MacLeod, S.G.; Santamaría-Perez, D.; Chen, S.; Cynn, H.; Simak, S.I.; McMahon, M.I.; Proctor, J.E. Experimental and theoretical confirmation of an orthorhombic phase transition in niobium at high pressure and temperature. Commun. Mater. 2020, 1, 1–11. [Google Scholar] [CrossRef]
- Kaewbumrung, M.; Tangsopa, W.; Thongsri, J. Investigation of the trailing edge modification effect on compressor blade aerodynamics using SST k-ω turbulence model. Aerospace 2019, 6, 48. [Google Scholar] [CrossRef] [Green Version]
- Kumari, S.; Satyanarayana, D.; Srinivas, M. Failure analysis of gas turbine rotor blades. Eng. Fail. Anal. 2014, 45, 234–244. [Google Scholar] [CrossRef]
- Witek, L. Simulation of crack growth in the compressor blade subjected to resonant vibration using hybrid method. Eng. Fail. Anal. 2015, 49, 57–66. [Google Scholar] [CrossRef]
- Mazur, Z.; Hernandez-Rossette, A.; Garcia-Illescas, R.; Luna-Ramirez, A. Failure analysis of a gas turbine nozzle. Eng. Fail. Anal. 2008, 15, 913–921. [Google Scholar] [CrossRef]
- Choi, M.G.; Ryu, J. Numerical study of the axial gap and hot streak effects on thermal and flow characteristics in two-stage high pressure gas turbine. Energies 2018, 11, 2654. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Wang, D.; Liu, Z.; Feng, Z. Numerical analysis on effects of inlet pressure and temperature non-uniformities on aero-thermal performance of a HP turbine. Int. J. Heat Mass Transf. 2017, 104, 83–97. [Google Scholar] [CrossRef]
- Azad, G.S.; Han, J.-C.; Teng, S.; Boyle, R.J. Heat transfer and pressure distributions on a gas turbine blade tip. J. Turbomach. 2000, 122, 717–724. [Google Scholar] [CrossRef] [Green Version]
- Asgarshamsi, A.; Benisi, A.H.; Assempour, A.; Pourfarzaneh, H. Multi-objective optimization of lean and sweep angles for stator and rotor blades of an axial turbine. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2015, 229, 906–916. [Google Scholar] [CrossRef]
- Pogorelov, A.; Meinke, M.; Schröder, W. Large-eddy simulation of the unsteady full 3D rim seal flow in a one-stage axial-flow turbine. Flowturbulence Combust. 2019, 102, 189–220. [Google Scholar] [CrossRef]
- Sasao, Y.; Kato, H.; Yamamoto, S.; Satsuki, H.; Ooyama, H.; Ishizaka, K. F208 numerical and experimental investigations of unsteady 3-d flow through two-stage cascades in steam turbine model (Steam Turbine-3). In Proceedings of the International Conference on Power Engineering (ICOPE), Kobe, Japan, 16–20 November 2009. [Google Scholar]
- Timko, L. Energy Efficient Engine High Pressure Turbine Component Test Performance Report; Technical Report; National Aeronautics and Space Administration (NASA): Cincinnati, OH, USA, 1 January 1984.
- Arnone, A.; Benvenuti, E. Three-Dimensional Navier-Stokes Analysis of a Two-Stage Gas Turbine; American Society of Mechanical Engineers: New York, NY, USA, 1994; Volume 78835. [Google Scholar]
- ANSYS. ANSYS TurboGrid Tutorials, Release 15.0; ANSYS: Canonsburg, PA, USA, 2013. [Google Scholar]
- ANSYS. ANSYS CFX, 12.1 Ansys Cfx-Solver Theory Guide; ANSYS: Canonsburg, PA, USA, 2009. [Google Scholar]
- Celik, I.; Yavuz, I.; Smirnov, A. Large eddy simulations of in-cylinder turbulence for internal combustion engines: A review. Int. J. Engine Res. 2001, 2, 119–148. [Google Scholar] [CrossRef]
- Ryu, J.; Lele, S.; Viswanathan, K. Study of supersonic wave components in high-speed turbulent jets using an LES database. J. Sound Vib. 2014, 333, 6900–6923. [Google Scholar] [CrossRef]
- Ryu, J.; Livescu, D. Turbulence structure behind the shock in canonical shock–vortical turbulence interaction. J. Fluid Mech. 2014, 756, R1-1–R1-13. [Google Scholar] [CrossRef] [Green Version]
- Ngo, T.T.; Phu, N.M. Computational fluid dynamics analysis of the heat transfer and pressure drop of solar air heater with conic-curve profile ribs. J. Therm. Anal. Calorim. 2020, 139, 3235–3246. [Google Scholar] [CrossRef]
- Nguyen, M.P.; Ngo, T.T.; Le, T.D. Experimental and numerical investigation of transport phenomena and kinetics for convective shrimp drying. Case Stud. Therm. Eng. 2019, 14, 100465. [Google Scholar] [CrossRef]
- Hao, Z.-R.; Gu, C.-W.; Ren, X.-D. The application of discontinuous Galerkin methods in conjugate heat transfer simulations of gas turbines. Energies 2014, 7, 7857–7877. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.; Bak, J.; Kang, Y.; Cho, L.; Cho, J. A study on the numerical analysis methodology for thermal and flow characteristics of high pressure turbine in aircraft gas turbine engine. Ksfm J. Fluid Mach. 2014, 17, 46–51. [Google Scholar] [CrossRef]
- Hylton, L.; Mihelc, M.; Turner, E.; Nealy, D.; York, R. Analytical and Experimental Evaluation of the Heat Transfer Distribution over the Surfaces of Turbine Vanes; Technical Report; National Aeronautics and Space Administration (NASA): Indianapolis, IN, USA, 1 May 1983.
- Menter, F.; Rumsey, C. Assessment of two-equation turbulence models for transonic flows. In Proceedings of the Fluid Dynamics Conference, Colorado Springs, CO, USA, 20–23 June 1994; p. 2343. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. Aiaa J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- ANSYS. ANSYS Fluent Theory Guide; ANSYS, Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]
- Gentry, M.; Jacobi, A.M. Heat transfer enhancement by delta-wing-generated tip vortices in flat-plate and developing channel flows. J. Heat Transf. 2002, 124, 1158–1168. [Google Scholar] [CrossRef]
- Lee, J.; Lee, H.J.; Ryu, J.; Lee, S.H. Three-dimensional turbulent flow and heat transfer characteristics of longitudinal vortices embedded in turbulent boundary layer in bent channels. Int. J. Heat Mass Transf. 2018, 117, 958–965. [Google Scholar] [CrossRef]
- Touil, K.; Ghenaiet, A. Simulation and analysis of vane-blade interaction in a two-stage high-pressure axial turbine. Energy 2019, 172, 1291–1311. [Google Scholar] [CrossRef]
- Wei, Z.-J.; Qiao, W.-Y.; Chen, P.-P.; Liu, J. Formation and Transport of Secondary Flows Caused by Vortex-Blade Interaction in a High Pressure Turbine. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Montreal, QC, Canada, 15–19 June 2015; p. V02AT38A008. [Google Scholar]
- Yang, D.; Feng, Z. Tip leakage flow and heat transfer predictions for turbine blades. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Montreal, QC, Canada, 14–17 May 2007; pp. 589–596. [Google Scholar]
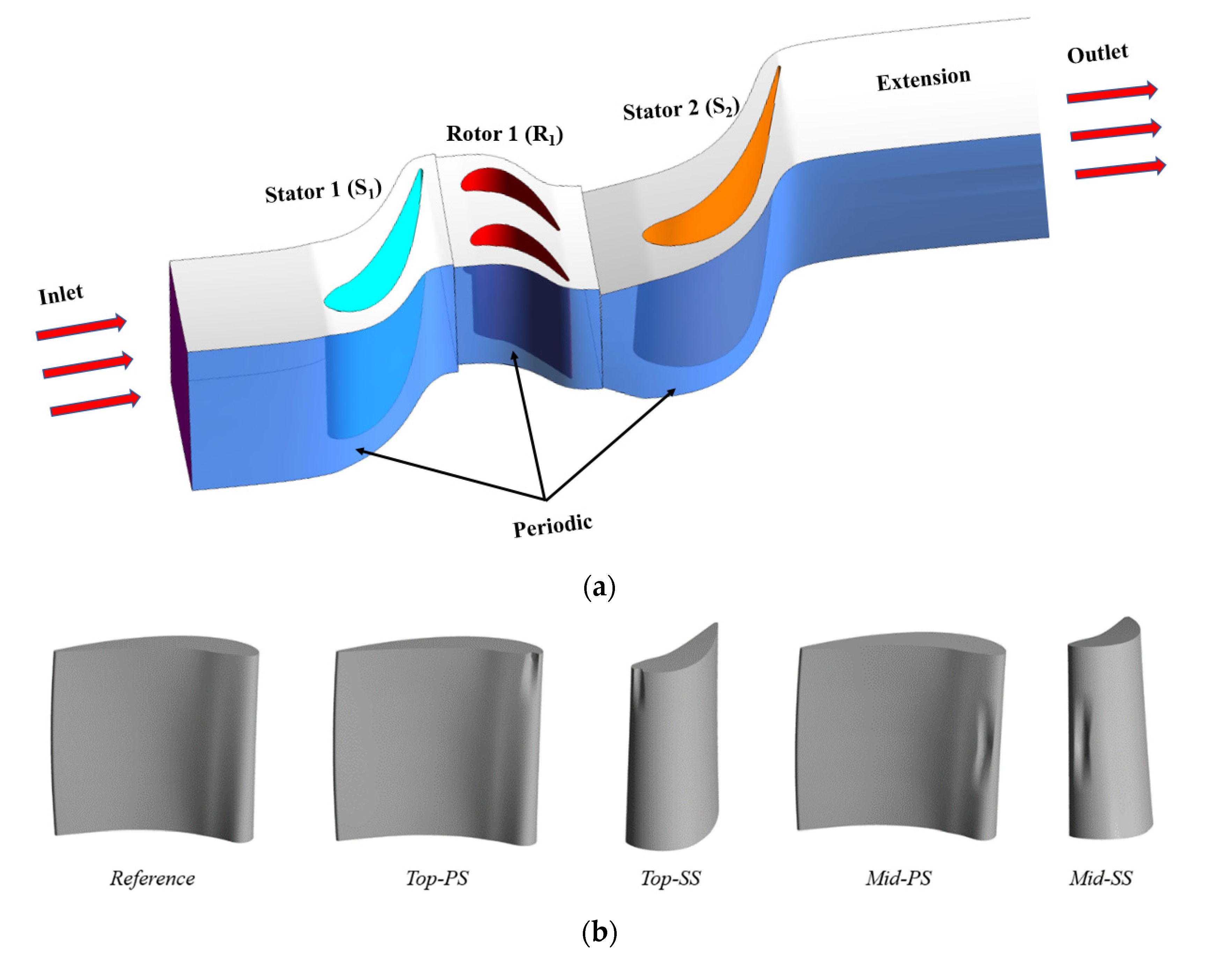
















| Average Pressure (kPa) (Relative Error) | |||
|---|---|---|---|
| Case 1 | 1 | 274.88 (0.76%) | 124.69 (1.17%) |
| Case 2 | 1.34 | 276.97 (1.06%) | 126.15 (0.69%) |
| Case 3 | 1.8 | 279.91 (0.82%) | 127.03 (0.58%) |
| Case 4 | 2.4 | 282.21 (0.43%) | 127.76 (0.23%) |
| Case 5 | 3.2 | 283.41 | 128.05 |
| Boundary conditions | |
| Inlet | Total pressure: 344,740 Pa Total temperature: 839 K Turbulence intensity: 5% |
| Outlet | Static pressure: 104,470 Pa |
| Simulation settings | |
| Wall conditions | Adiabatic or iso-thermal |
| Rotor blade conditions | Undamaged or damaged at PS and SS in top and middle of blade |
| Vane and blade interface | Transient rotor-stator (unsteady simulation) |
| Rotor speed | 3600 RPM |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mai, T.D.; Ryu, J. Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine. Mathematics 2020, 8, 2191. https://doi.org/10.3390/math8122191
Mai TD, Ryu J. Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine. Mathematics. 2020; 8(12):2191. https://doi.org/10.3390/math8122191
Chicago/Turabian StyleMai, Thanh Dam, and Jaiyoung Ryu. 2020. "Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine" Mathematics 8, no. 12: 2191. https://doi.org/10.3390/math8122191
APA StyleMai, T. D., & Ryu, J. (2020). Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine. Mathematics, 8(12), 2191. https://doi.org/10.3390/math8122191





