1. Introduction
Ordinary differential equations are one of the most frequently used mathematical tools for modeling and describing dynamical systems in general and growth phenomena in particular. In such models a quantitative variable evolves with time according to certain parameters and deterministic functions, and for their properties they are commonly applied to several fields of research.
Studying such models requires, on the one hand, understanding the evolving mechanisms of dynamical systems to properly explain their behavior. On the other, we must be able to include in them external information different from that supplied by the variables under study. In doing so we modify their behavior and exert external control on the evolution of the phenomenon under consideration.
However, in many situations, the resulting models do not adequately reproduce the observed phenomenon. The main reason is that deterministic models do not take into account certain disturbances that can greatly influence the final behavior. These disturbances may have multiple and various origins, which are not always quantifiable or may even be unknown. As stated by Li et al. [
1], “
noises are ubiquitous in both nature and human society, such as fire, earthquake, climate warming, financial crisis, etc.”. Many applications require the modeling of external elements whose nature can be considered stochastic, either because of the impossibility of making measurements or because said measurements present a high degree of uncertainty. It is, therefore, necessary to modify the deterministic model to introduce a random element.
Faced with that need, the design of models is obtained from the ordinary differential equation for the deterministic model, in which a random element is introduced, usually a function of a white noise. The result are stochastic models. Such modification requires considering stochastic differential equations (SDE) whose solutions are, under some conditions, diffusion processes. Not only does the use of these stochastic models provide a more realistic explanation of the variables under study, but it also allows us to study other important aspects (like inference or certain time variables that represent some features of the phenomenon).
As previously mentioned, ordinary differential equations are modified to give rise to a certain curve by introducing in them a white noise function. The choice of such function (usually motivated by specific characteristics of the studied phenomenon) can lead to a variety of stochastic processes, although they are related to the same deterministic curve. In addition, that choice can determine our specific approach to the stochastic solution process. In fact, even if the SDE has a solution (and sometimes it is not an explicit one), the resulting diffusion process is difficult to handle because the associated Kolmogorov equations have not yet been solved. For example, Feller pioneered this methodology by applying it to the case of the logistic equation and introducing an additional error term that was directly proportional to the square root of the variable. However, the backward and forward equations associated with the diffusion process solution of the SDE have never been solved (see Schurz [
2] for other possible choices of the error term and associated diffusion processes). Furthermore, and regarding the logistic case, in the few cases where the transition density has been calculated (see Tuckwell [
3]), it was impossible to find an explicit expression for certain important characteristics such as the mean of the process. This has led to theoretical works that analyze the existence of a solution for the SDE, study its stability properties (see Sun and Wang [
4]), or propose simulation techniques (see Hu and Wang [
5]). These considerations can be extended to other types of situations beyond the logistic case.
In light of the above, efforts have been made to address SDEs whose solutions are more manageable stochastic processes and also reproduce a certain pattern of observed behavior, i.e., their mean follows a given growth curve. This allows certain issues to be directly addressed, including inference or the study of temporal variables (such as first-passage-times), without having to resort to approximations such as those derived from the discretization of the SDE. The modification of certain ordinary differential equations by adding a multiplicative noise has given rise to processes whose mean is a growth curve. In this sense it is worth highlighting the role of the lognormal process with exogenous factors. As a matter of fact, concrete choices of such exogenous factors lead to processes by which we may study patterns of behavior modeled by a wide range of growth curves (see Román-Román et al. [
6,
7] and references therein). These references are related to the one-dimensional case, although there are extensions to multidimensionality such as the one proposed by Rupšys in [
8], where a 4-variate Bertalanffy-type SDE is considered.
Other lines of action within the scope of SDEs should be mentioned. For example, the inclusion of delays in the modeling of certain phenomena (see Rupšys [
9], Longtin [
10], Sakthivel et al. [
11]), the introduction of fractional Brownian motion and fractional calculus (Dung [
12]), or the consideration of both methodologies at once (Moghaddam et al. [
13]).
We must keep in mind that the ultimate objective of studying models such as these is modeling behavior patterns in real situations. Beyond the widely discussed classic growth curves (logistic, Gompertz, Bertalanffy, Richards, etc.), other interesting families of curves have been considered in recent times. Among them, the hyperbolastic functions of type I, II and III, introduced by Tabatabai et al. [
14], stand out. The main feature of this type of curves is the introduction of hyperbolic functions into known models, thus revealing mobile inflection points and increasing the capability of the models to fit real data. Some works have proved the usefulness of such curves in the description of dynamical phenomena. For example, Eby et al. [
15] used them to study the growth of the solid Ehrlich carcinoma under particular treatments, obtaining a more accurate representation than those yielded by other classic curves such as Gompertz or Weibull. Tabatabai et al. [
16] used the hyperbolastic type III model to describe the behavior of embryonic stem cells. Later, other models derived from hyperbolastic curves have been introduced, specifically the oscillabolastic and the T-model (Tabatabai et al. [
17,
18]). Focusing on the first one, the oscillabolastic curve was introduced as an appropriate model to describe dynamic phenomena that present oscillatory behaviors. The introduction of parameter-dependent trigonometric functions allows for oscillatory dynamics to be incorporated into the model and regulates both the amplitude and frequency of such oscillations. A progressive reduction in amplitude guarantees the asymptotic stability of the oscillatory behavior. In that paper the authors applied the oscillating model to two data sets: the first dealing with the oscillabolastic modeling of Ehrlich ascites tumor cells, and the second with the mean signal intensity of Hes1 gene expression in response to serum stimulation.
The main objective of this article is to present two diffusion processes associated with the oscillabolastic curve. Each will be obtained from a differential equation verified by the curve, and include an additive and a multiplicative error term, respectively. The rest of the paper is organized as follows: in
Section 2 the oscillabolastic deterministic model is introduced and studied from a classical as well as an alternative differential formulation. The adaptation of these two formulations to stochastic models takes place in
Section 3. To provide practical instances of application to the models presented,
Section 4 addresses the maximum likelihood estimation of the parameters of each model. The complexity of the models and their high number of parameters make the resolution of the likelihood equations somewhat difficult. The use of numerical methods will thus require the prior estimation of initial solutions for the system, which is why a methodology for its calculation is suggested at the end of that section.
Section 5 is a simulation study for both models that includes the generation of data for each process and the application of our proposed estimation methodology.
2. The Oscillabolastic Curve
The original formulation of the oscillabolastic curve is given by the function
,
where
and
are real parameters. Clearly, the function
is defined for all
t and
. As easily seen, for
one has
, so one can define
also at
. However, in the context of growth phenomena,
t is a time index and therefore we consider the curve defined in
,
.
To study the behavior of (
1), we start by observing that its expression is determined by two terms, say
, where
and
. It can therefore be seen that the behavior of the curve is governed mainly by
. This function summarizes the oscillatory trend of the model, as well as its evolution towards
, which we consider to be the population limit in a growth model (and not the maximum sustainable capacity that is typical of monotonous and positive growth models). Parameter
is responsible for the amplitude of the oscillations, which become larger as
grows. Furthermore, their frequency is directly proportional to
.
The flexibility of the oscillabolastic curve is clearly increased thanks to the influence of
. In this case, the hyperbolic arcsine allows
to be deformed, thus making the oscillatory pattern evolve along a curved path. This in turn has an impact on the sequence of local extremes of
(local minima and maxima at each oscillation), since one of the functions (
or
) will present a wider range at initial values than the other, which will remain closer to constant value
. In any case, both will eventually converge to
.
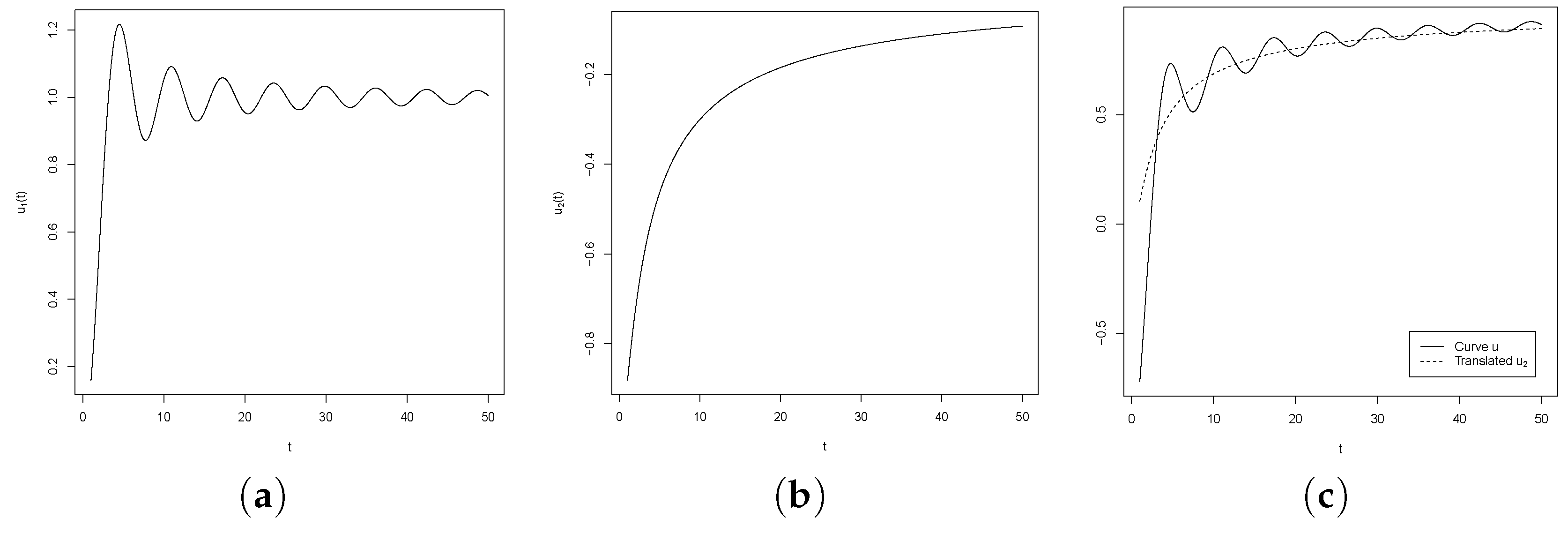
Figure 1 shows the two parts of an oscillabolastic curve for
and
, as well as the resulting curve
. It can be seen how
influences the final curve by modifying the behavior of
. This interaction is indicated by a dashed line representing translated function
, where
is the average difference between
and
.
Another fundamental aspect of is its ability to make the final curve smoother, and to partially compensate the amplitude of oscillations from . In this sense, high values of cause a deformation of the oscillations, increasing the difference between the increasing and decreasing phases.
In any case, the behavior of the oscillabolastic curve is characterized by four parameters. It is not easy to establish a clear pattern of influence for each without taking into account the others, as well as the time interval in which the curve is evaluated.
An important characteristic of the oscillabolastic curve is the presence of multiple inflections, a logical consequence of the oscillations of the model. Equation
leads to the following equation, verified for any inflection time
,
From an analytical approach, the function
satisfies, under the initial condition
, the linear ordinary differential equation
for
, being
a real function defined in the same domain as
and where
denotes the parametric vector
.
On the other hand, it is possible to reformulate the oscillabolastic curve as
where the relationship between the parameters of both formulations would be given by
and
.
In this case, it can be verified that the reformulated curve is the solution to the linear ordinary differential equation
with initial condition
, and where function
is expressed as
being
.
Both differential equations, (
3) and (
5), define deterministic models of evolution for the oscillabolastic curve. The most notable difference between them is their structure: in the first case we have a non-homogeneous linear differential equation with non-constant coefficients, while in the second it is reduced to a multiplicative model.
Given the potential applications of both models, their stochastic extensions will be addressed in the following sections. The main idea is to make the mean of the resulting stochastic process able to describe oscillabolastic-type behaviors, which opens the door for observed patterns of this type to be reproduced.
3. Oscillabolastic-Type Diffusion Processes
This section introduces stochastic extensions for the deterministic oscillabolastic model in light of the two differential equations described in the previous section. The starting points will be ordinary differential Equations (
3) and (
5). Although both are linear differential equations, the diffusion processes to which they give rise have different characteristics. The former is a Gaussian process (of the non-homogeneous Ornstein–Uhlenbeck type), while the second is related to the non-homogeneous geometric Brownian motion, or lognormal process with exogenous factors.
3.1. Oscillabolastic Gaussian Diffusion Process
This process is obtained by adding a random component to (
3). Specifically, a white noise with variance
is included in the model, where
is a parameter that marks the random influence on the model. Writing the resulting equation in the traditional form of stochastic differential equations, we have
where
is the standard Wiener process in
, which we consider independent from the initial variable
, whereas
is given by (
4).
Equation (
7) is a linear SDE verifying the conditions of existence and uniqueness of solution. In fact, functions
and
are measurable and bounded in every subinterval
, with
, of
, which guarantees the existence and uniqueness of a global solution (i.e., defined in
) for each initial condition
.
This solution is a stochastic diffusion process
, adapted to the natural filtration generated by the Borel sets in one dimension and characterized by drift
and constant infinitesimal variance
. The explicit formulation of the process is given in terms of the solution of ordinary differential equation
, leading to
Initial condition
is fundamental to establishing the nature of the process. Indeed, if
is a constant (degenerate) or a Gaussian random variable, then the solution of (
7) is a Gaussian diffusion process (from now on we will consider both scenarios). In addition, in the case where
is constant, the independence of the increments of the process is also guaranteed.
On the other hand, condition
guarantees the existence of the
p-th order moment for
. Specifically, the expressions of the mean and covariance functions are respectively
for
, being
. Here
denotes increasing operator
for a function
f, whereas
.
Regarding the mean function
, note that it can be expressed as
where
is the initial value of the oscillabolastic curve. Also note that when constructing the stochastic model, the main goal is for the mean function of the process to coincide with that of a prescribed oscillabolastic model, i.e.,
for all
t. Regarding the last expression, this condition holds when
. However, the asymptotic tendency of the process (the behavior of its mean at the limit) is similar to that of the oscillabolastic deterministic model. Indeed, the difference between the mean and the curve, for each time instant, decreases as
t tends to
∞, i.e.,
With regard to the transition density function, which is required to implement the inference procedures that will be described in the next section, for any time instants
the conditioned variable
is normal, with mean and variance given respectively by expressions
where
is the standard deviation of
(resp. for
s) and
is the correlation function. Both expressions can also be written in the form
and
from which the probability transition density function is given by
3.2. Oscillabolastic Diffusion Process Derived from the Non-Homogeneous Lognormal Diffusion Process
From Equation (
5), it is possible to obtain a stochastic model whose mean function coincides with the original deterministic model, i.e., the oscillabolastic curve. As a matter of fact, this differential equation represents a Malthusian model with a time-dependent fertility
given by (
6). The introduction of a white noise
with variance
in this fertility component, accomplished by replacing
with
, leads to the SDE
where
is the standard Wiener process, independent from
,
. Please note that the reformulation of the original curve leads to a model with one parameter less than the Gaussian model.
In this case, the existence and uniqueness of the solution is ensured by the continuity of the
function. Thus, the solution of (
9) is a stochastic diffusion process
characterized by drift
and infinitesimal variance
. Furthermore, a closed-form expression for the solution is given, for
, by
where
being
This process is a particular case of the non-homogeneous lognormal diffusion, or lognormal diffusion process with exogenous factors. The inclusion of the function in the drift justifies the terminology: the infinitesimal mean of the process can be affected by the inclusion of time-dependent external factors.
As regards the distribution of the process, if is distributed according to a lognormal distribution or is a degenerate random variable (), all the finite-dimensional distributions of the process are lognormal. Concretely, and , vector is distributed according to a n-dimensional lognormal distribution , where the components of the vector are , , being , , those of the matrix .
As far as the transition probability is concerned, it is obtained from that of
,
. Concretely,
whose density function is
Once the distribution of the process has been established, we may calculate a variety of its associated characteristics including the mean and conditioned mean functions, whose expressions are
and
respectively. Both functions are oscillabolastic curves of the type introduced in the previous section. Other characteristics of the process can be obtained from the results included in Román et al. [
6].
4. Estimation of the Parameters
The main useful application of the diffusion processes described in the previous section is the description of dynamic phenomena subject to random influences, in particular growth phenomena. For that reason, the estimation of the parameters included in the models is especially relevant. Among the procedures available we will employ the maximum likelihood estimation method. The estimation of the parameters involves solving a system of equations, which will require using adequate numerical techniques.
Let us consider d sample paths observed at time instants , . Please note that neither the sample sizes nor the times of observation have to be the same, although we will suppose that the first time of observation is common for all sample paths, i.e., , . Let be the vector containing the random variables of the i-th sample-path, i.e., , , and denote .
For simplicity, we consider a degenerate initial distribution, i.e., . The reason is that the parameters of the distribution in the non-degenerate case (normal or lognormal depending on the process) are usually considered functionally independent from the parameters involved in the transition distribution, so the estimation of these last parameters is not influenced by the estimation of the others. In any case, if were not degenerate its parameters could not be estimated from the values of the sample paths at the first time instant of observation.
In what follows, and for the sake of simplicity in the notation, we will not differentiate between the processes when noting the observed values. Obviously, the context determines the meaning in each case.
4.1. The Gaussian Case
Let us consider a fixed value
of
. From (
8) the likelihood function can be obtained, whose logarithm is
where
and
. Now operator
(applied to a generic function
f) denotes the increments
.
To obtain the maximum likelihood estimates, we must calculate the derivatives of
with respect to the parameters. In this sense, the derivatives with respect to the components of
are
where
On the other hand, the partial derivative with respect to
is
Finally, by using (
11) and (
12), and equating to zero the partial derivative of the loglikelihood, one gets:
The resolution of this system will be determined by the application of numerical techniques. Such procedures will require a set of initial solutions. This problem, common to the two models presented, will be addressed in
Section 4.3.
4.2. Lognormal Case
Regarding oscillabolastic process
, given by SDE (
9), the maximum likelihood estimation can be addressed by means of the procedure described in [
6]. This approach has already been used succesfully in the inferential treatment of the Gompertz multisigmoidal diffusion process [
7], as well as in the generalization of the classic Weibull model, in particular for the hyperbolastic type III diffusion process [
19].
In this case, and considering probability transition density function (
10), the logarithm of the likelihood function is given by
where
being
.
The estimate of vector
(see [
6] for details) follows by solving the system of equations
where
These functions are obtained from the partial derivatives of the
functions:
where
From these last expressions, and from those of
and
, the subsystem of Equation (
14) can be written in the form
with
On the other hand, and after some calculus, Equation (15) transforms into
being
and
.
As in the previous case, system of Equations (
16) and (
17) cannot be solved explicitly, and it is, therefore, necessary to use numerical methods such as Newton-Raphson’s, for which an initial solution is required. In the next subsection we present a strategy to provide such a solution based on the information provided by the sample data, and applicable to the two resulting systems of equations.
4.3. Initial Solutions
As already mentioned, the systems of likelihood equations obtained in the preceding subsections have a high degree of complexity due to the high number of parameters involved (although in the lognormal case this number is reduced) and to the presence of random data. Their resolution, therefore, will require the use of numerical techniques. For such procedures to be applied a prior choice must be made regarding initial solutions.
This section proposes a strategy based on the information provided by the sample data. The key to the procedure focuses on the sample mean function, which is calculated from the values of the sample paths at each time instant of observation
. Since the procedure needs to evaluate this function over the entire observation interval, a spline function is later fitted to the mean values of the observed sample paths. Furthermore, the procedure is applicable to the two processes introduced earlier. The goal is to make the theoretical mean functions of both processes approximate the oscillabolastic curve (
1), although in the Gaussian case this approach is asymptotic. We expose the strategy for the Gaussian case. For the lognormal-type process it is deduced by simply taking into account the relationship between the parameters which has been described in
Section 2.
The following is the suggested procedure for each parameter:
: This is the limit value of the model, and represents its asymptotic behavior when . However, due to oscillations, this value is not the maximum (which usually occurs in monotonous positive growth models, giving rise to the carrying capacity of the system under consideration). Since the curve approaches , a possible option to obtain an initial value is to take the last value of the sample mean function. However, this depends largely on the length of the time interval, as well as on the amplitude of the oscillations and/or of the curvature of the model. These effects can lead to estimates of far from its true value. For greater accuracy, an alternative approach would be to take the average value between the sequences of local extreme values (maxima and minima) of the sample mean function.
: The calculation of initial values for these parameters is quite complex. The starting point is to have an approximation for one of the instants of time () in which an inflection is observed in the mean of the process. To obtain it, a maximum of the derivative of the sample mean function is numerically approached.
On the other hand, it can be observed that (
1) is a linear function in
and
. Therefore, considering
and
, and the value
previously calculated, it is possible to obtain an expression of both parameters as a function of
and
from the system of equations
Please note that
and
can be obtained from the spline function previously adjusted to the mean of the sample paths. We shall denote by
and
the resulting values. Now the problem has been reduced to finding initial values for
and
. To this end we look for pairs of values
within a two-dimensional bounded region satisfying the inflection condition (
2) for a predefined error threshold, say
, i.e., it must hold
being
the inflection time instant previously obtained. We suggest using values of
between 0.0001 and 0.1, depending on the order of magnitude of the sample data. Initial values
and
will be the mean of the resulting values for each parameter, respectively. Finally, the initial values for
and
are obtained from
and
, respectively.
: The initial value for
is obtained from the estimation of the variance of the one-dimensional distributions of the processes. For instance, in the case of the Gaussian oscillabolastic process, the variance is (please remember that we have considered a degenerate initial distribution)
so, if we denote by
the sample variance at
, a linear regression model of the
values versus
(
) is considered in order to obtain the estimated value for
. It should be noted that the sample variance can sometimes present a rather volatile behavior. Therefore, in practice it is advisable to procure a smoothed version of said function before obtaining values for
.
For the other oscillabolastic-type diffusion process, for which (see
Section 3.2)
being
, we can avoid using the sample variance function. In point of fact, it is well-known that for a lognormal distribution
the quotient between the arithmetic mean and the geometric one provides an estimation of
. This leads, for each
, and when the initial distribution is degenerate, to an estimate of
in the distribution of
, say,
,
, where
and
are, respectively, the values of the mean and the geometric sample mean of the sample paths at
. Again, the initial value for
is obtained by performing a simple linear regression of
values against
.
Please note that if is not a degenerate random variable, provides an estimate of for each . In that case, can be previously estimated from the values of the sample paths at and then its values introduced in the regression function.
5. Simulation Study
In this section, a simulation study illustrates the behavior of the oscillabolastic process, as well as the application of the proposed methodology for estimating the parameters of the processes under consideration. One of its most interesting aspects is how initial solutions are obtained for the subsequent estimation of the parameters of the process, to which end we have applied the methodology proposed in the previous subsection.
5.1. Simulation in the Gaussian Case
Data from the oscillabolastic Gaussian process are obtained by simulating 20 sample paths in the time interval
, with degenerate initial distribution
. As for the values of the parameters, we have started by considering
and
. The parameter corresponding to the infinitesimal variance is, in this case,
. Simulated paths are shown in
Figure 2.
Following the method described in
Section 4.1, the system of maximum likelihood Equation (
13) is solved by numerical procedures. To do this, initial solutions to the parameters are obtained by applying the methodology described in
Section 4.3. The initial value for
is selected by taking the last value of the observed mean, in this case
. One inflection point is calculated from (
2) after fitting a spline function to the observed mean values, resulting in
, and being
the value of the curve at this point. With this information, initial values
and
for
and
, respectively, are found by holding the inflection condition with an error threshold
. The search region is determined by the square
. In fact, the number of oscillations of the observed data suggests that
must lie between 0.1 and 3 (and might even be 2). On the other hand, the absence of a pronounced curvature implies low values of
. Finally, the initial values are
and
. From these values, and using the expressions
and
obtained from (
18), we find the corresponding initial values for
and
, concretely
and
. Finally, the initial value for
is obtained from the estimation of the slope of the regression line of observed variability against time, resulting in
.
Once the initial values of the parameters have been determined, they are used to solve the system of equations. Detailed results for estimated values of each parameter are shown in
Table 1. Please note that in this case, initial values coincide with estimated ones, mainly due to low variability of the sample paths.
To evaluate the quality of the estimation of the process, we have considered the absolute relative errors between the sample mean of the simulated process and the estimated one, i.e.,
where
and
are the values of the sample mean function and the ones estimated at
,
.
In this case, the value of this error is
, which indicates a very good fit between the observed and estimated mean functions from the values found for the parameters. This can be visualized in
Figure 3, in which both mean functions are plotted.
A new simulation study has been carried out in order to check the process estimation for oscillatory behavior, for example when the parameter that influences the frequency of the oscillations is varied, and to accommodate for the variability of the sample paths. The simulation pattern is the same as the one considered in the previous case. Parameter takes values 2, 3, and 4. As previously mentioned, the number of oscillations in the data may determinate the range of values of . Also, the curvature of the trend followed by the oscillations may be a good indicator for the range of parameter . Initial values coming from this bounded region lead to the estimation of and .
Final results for different combinations of parameters are shown in
Table 2. A graphical comparison between simulated and estimated mean functions, for different parameters, is shown in
Figure 4. It is observed that despite the initial difference between the mean function and the original oscillabolastic curve, the Gaussian diffusion process performs well with the proposed methodology for the parameter estimation. Please note that the last two results are the same but for different regions of
. Results are the same up to the sign of
and
, due to the odd condition of the sine function.
Also, three simulations has been carried out for different values of the infinitesimal variance,
and
, with parameters
, and
from
to 10, starting at
. Please note that final performance is very good although, as expected, RAE increases with variability. Results are shown in
Table 3 and
Figure 5.
5.2. Simulation in the Lognormal Case
The simulation scheme applied to the lognormal oscillabolastic diffusion process is similar to the one used earlier. In this case, the initial value of the degenerate distribution is
. Original values for the parameters are
, and
.
Figure 6 displays the simulated paths (note that in the lognormal case, and in contrast to the Gaussian one, variability grows faster due to its dependence on the state).
The initial values for the parameters follow from the methodology used in the lognormal case. The search region for and is the same as in the Gaussian simulation example above. The number of oscillations (in relation to the time interval) and the curvature of the observations suggest that this may be a good choice. An inflection condition with , and error results in initial values and , respectively. Because of increasing variability, very low error thresholds are not recommended, as they would decrease performance.
Initial values of and have been calculated following the same methodology set out in the previous example, resulting in and . Finally, the initial value of infinitesimal variance follows from the regression analysis of the values obtained from the arithmetic and geometric mean of the observed mean values. In this case, is obtained.
Using these initial values in the numerical resolution of the maximum likelihood system of Equations (
16) and (
17) leads to
, and
.
Table 4 summarizes the results.
Figure 7 shows the simulated and estimated mean functions. Note how the adjustment provided by the mean function is good and is supported by the value of the RAE, which is
.
As for the Gaussian oscillabolastic diffusion process, another simulation study has been carried out by varying, in this case, parameters (which affects the frequency of the oscillations) and (responsible for the variability of the sample paths). Following the same methodology as in the Gaussian case, the ranges of and can be directly proposed from the observations.
In this case, paths are simulated for values of
. Final estimated values, as well as the search range for initial values of
are shown in
Table 5. Furthermore,
Figure 8 shows simulated and estimated mean functions for some combinations of parameters.
for every one of them is also shown in the table. These values suggest that the model and the proposed estimation methodology perform very well.
On the other hand,
Table 6 and
Figure 9 also show good performance for different values of variability, in particular
for parameters
and
for
with
.
6. Conclusions
Classic growth curves are generally described as arising from ordinary differential equations, which can be studied by themselves or as part of more sophisticated models. In the present paper, we have focused on the study of the oscillabolastic curve, which is particularly interesting for its ability to describe dynamic oscillatory phenomena.
Stochastic models are obtained from deterministic ones by including in them certain random fluctuations that can significantly influence the evolution of dynamic phenomena. This work considers two oscillabolastic stochastic models that retain the properties of the original curve and can be applied to practical situations. Two strategies have been proposed for managing the original differential equation and producing two different stochastic models. One of them is a Gaussian process while the other is a derivative of the lognormal process with exogenous factors.
One of the main problems derived from such models is the development of inference strategies. The estimation of the parameters by maximum likelihood implies the numerical resolution of two systems of equations. We have proposed a methodology to obtain initial solutions for the parameters based on the main traits of the model, namely the presence of an oscillabolastic trend (in the lognormal case) or an asymptotic oscillabolastic behavior (in the Gaussian case). To apply these procedures, a simulation study has been carried out taking into account a variety of error measures. This simulation study confirms the validity of the methodology proposed.