Some Notes on the Formation of a Pair in Pairs Trading

Abstract

The main goal of the paper is to introduce different models to calculate the amount of money that must be allocated to each stock in a statistical arbitrage technique known as pairs trading. The traditional allocation strategy is based on an equal weight methodology. However, we will show how, with an optimal allocation, the performance of pairs trading increases significantly. Four methodologies are proposed to set up the optimal allocation. These methodologies are based on distance, correlation, cointegration and Hurst exponent (mean reversion). It is showed that the new methodologies provide an improvement in the obtained results with respect to an equal weighted strategy.

1. Introduction

Efficient Market Hypothesis (EMH) is a well-known topic in finance. Implications of the weak form of efficiency is that information about the past is reflected in the market price of a stock and therefore, historical market data is not helpful for predicting the future. An investor in an efficient market will not be able to obtain a significant advantage over a benchmark portfolio or a market index trading based on historical data (for a review see Reference [1,2]).

On the opposite way, some researchers have shown that the use of historical data as well as trading techniques is sometimes possible due to temporal markets anomalies. Despite that most of economists consider that these anomalies are not compatible with an efficient market, recent papers have shown new perspectives called Fractal Market Hypothesis (FMH) and Adaptive Market Hypothesis (AMH), that tries to integrate market anomalies into the efficient market hypothesis.

The EMH was questioned by the mathematician Mandelbrot in 1963 and after the economist Fama showed his doubts about the Normal distribution of stock returns, essential point of the efficient hypothesis. Mandelbrot concluded that stock prices exhibit long-memory, and proposed a Fractional Brownian motion to model the market. Di Matteo [3,4] considered that investors can be distinguished by the investment horizons in which they operate. This consideration allows us to connect the idea of long memory and the efficiency hypothesis. In the context of an efficient market, the information is considered as a generic item. This means that the impact that public information has over each investor is similar. However, the FMH assumes that information and expectations affect in a different way to traders, which are only focused on short terms and long term investors [5,6].

The idea of a AMH has been recently introduced by Lo [7] to reflect an evolutionary perspective of the market. Under this new idea, markets show complex dynamics at different times which make that some arbitrage techniques perform properly in some periods and poorly in others.

In an effort of conciliation, Sanchez et al. [8] remarks that the market dynamic is the results of different investors interactions. In this way, scaling behavior patterns of a specific market can characterize it. Developed market price series usually show only short memory or no memory whereas emerging markets do exhibit long-memory properties. Following this line, in a recent contribution, Sanchez et al. [9] proved that pairs trading strategies are quite profitable in Latin American Stock Markets whereas in Nasdaq 100 stocks, it is only in high volatility periods. These results are in accordance with both markets hyphotesis. A similar result is obtained by Zhang and Urquhart [10] where authors are able to obtain a significant exceed return with a trading strategy across Mainland China and Hong kong but not when the trading is limited to one of the markets. The authors argue that this is because of the increasing in the efficiency of Mainland China stock market and the decreasing of the Hong Kong one because of the integration of Chinese stock markets and permission of short selling.

These new perspectives of market rules explain why statistical arbitrage techniques, such as pairs trading, can outperform market indexes if they are able to take advantage of market anomalies. In a previous paper, Ramos et al. [11] introduced a new pairs trading technique based on Hurst exponent which is the classic and well known indicator of market memory (for more details, References [8,12] contain an interesting review). For our purpose, the selection of the pair policy is to choose those pairs with the lowest Hurst exponent, that is, the more anti-persistent pairs. Then we use a reversion to the mean trading strategy with the more anti-persistent pairs according with the previously mentioned idea that developed market prices show short memory [3,13,14,15].

Pairs trading literature is extensive and mainly focused on the pair selection during the trading period as well as the developing of a trading strategy. The pioneer paper was Gatev et al. [16] where authors introduced the distance method with an application to the US market. In 2004, Vidyamurthy [17] presented the theoretical framework for pair selection using the cointegration method. Since then, different analysis have been carried out using this methodology in different markets, such us the European market [18,19], the DJIA stocks [20], the Brazilian market [21,22] or the STOXX 50 index [23]. Galenko et al. [24] made an application of the cointegration method to arbitrage in fund traded on different markets. Lin et al. [25] introduced the minimum profit condition into the trading strategy and Nath [26] used the cointegration method in intraday data. Elliott et al. [27] used Markov chains to study a mean reversion strategy based on differential predictions and calibration from market observations. The mean reversion approach has been tested in markets not considered efficient such us Asian markets [28] or Latin American stock markets [9]. A recent contribution of Ramos et al. [29] introduced a new methodology for testing the co-movement between assets and they tested it in statistical arbitrage. However, researchers did not pay attention to the amount of money invested in every asset, considering always a null dollar market exposition. This means that when one stock is sold, the same amount of the other stock is purchased. In this paper we propose a new methodology to improve pairs trading performance by developing new methods to improve the efficiency in calculating the ratio to invest in each stock that makes up the pair.

2. Pair Selection

One of the topics in pairs trading is how to find a suitable pair for pairs trading. Several methodologies have been proposed in the literature, but the more common ones are co-movement and the distance method.

2.1. Co-Movement

Baur [30] defines co-movement as the shared movement of all assets at a given time and it can be measured using correlation or cointegration techniques.

Correlation technique is quite simple, and the higher the correlation coefficient is, the greatest they move in sync. An important issue to be considered is that correlation is intrinsically a short-run measure, which implies that a correlation strategy will work better with a lower frequency trading strategy.

In this work, we will use the Spearman correlation coefficient, which is a nonparametric range statistic which measure the relationship between two variables. This coefficient is particularly useful when the relationship between the two variables is described by a monotonous function, and does not assume any particular distribution of the variables [31].

The Spearman correlation coefficient for a sample $A_i$, $B_i$ of size n can be described as follows: first, consider the ranks of the samples $rg{A}_i$, $rg{B}_i$, then the Spearman correlation coefficient $r_s$ is calculated as:

$$r_s={\rho }_{r{g}_A,r{g}_B}=\frac{cov(r{g}_A,r{g}_B)}{{\sigma }_{r{g}_A}\ast {\sigma }_{r{g}_B}},$$

(1)

where

• $\rho$ denotes the Pearson correlation coefficient, applied to the rank variables
• $cov(r{g}_A,r{g}_B)$, is the covariance of the rank variables.
• ${\sigma }_{r{g}_A}$ and ${\sigma }_{r{g}_B}$, are the standard deviations of the rank variables.

Cointegration approach was introduced by Engle and Granger [32] and it considers a different type of co-movement. In this case, cointegration refers to movements in prices, not in returns, so cointegration and correlation are related, but different concepts. In fact, cointegrated series can perfectly be low correlated.

Two stocks A and B are said to be cointegrated if there exists $\gamma$ such that $P_t^A-\gamma P_B^t$ is a stationary process, where $P_t^A$ and $P_B^t$ are the log-prices A and B, respectively. In this case, the following model is considered:

$$P_t^A-\gamma P_B^t=\mu +{ϵ}_t,$$

(2)

where

• $\mu$ is the mean of the cointegration model
• ${ϵ}_t$ is the cointegration residual, which is a stationary, mean-reverting process
• $\gamma$ is the cointegration coefficient.

We will use the ordinary least squares (OLS) method to estimate the regression parameters. Through the Augmented Dickey Fuller test, we will verify if the residual ${ϵ}_t$ is stationary or not, and with it we will check if the stocks are co-integrated.

2.2. The Distance Method

This methodology was introduced by Gatev et al. [16]. It is based on minimizing the sum of squared differences between somehow normalized price series:

$$ESD=\sum _t{(S_A\left(t\right)-S_B\left(t\right))}^2,$$

(3)

where $S_A\left(t\right)$ is the cumulative return of stock A at time t and $S_B\left(t\right)$ is the cumulative return of stock B at time t.

The best pair will be the pair whose distance between its stocks is the lowest possible, since this means that the stocks moves in sync and there is a high degree of co-movement between them.

An interesting contribution to this trading system was introduced by Do and Faff [33,34]. The authors replicated this methodology for the U.S. CRSP stock universe and an extended period. The authors confirmed a declining profitability in pairs trading as well as the unprofitability of the trading strategy due to the inclusion of trading costs. Do and Faff then refined the selection method to improve the pair selection. The authors restricted the possible combinations only within the 48 Fama-French industries and they looked for pairs with a high number of zero-crossings to favor the pairs with greatest mean-reversion behavior.

2.3. Pairs Trading Strategy Based on Hurst Exponent

Hurst exponent (H from now on) was introduced by Hurst in 1951 [35] to deal with the problem of reservoir control for the Nile River Dam. Until the beginning of the 21st century, the most common methodology to estimate H was the R/S analysis [36] and the DFA [37], but due to accuracy problems remarked by several studies (see for example References [38,39,40,41]), new algorithms were developed for a more efficient estimation of the Hurst exponent, some of them with its focus on financial time series. One of the most important methodologies is the GHE algorithm, introduced in Reference [42], which is a general algorithm with good properties.

The GHE is based on the scaling behavior of the statistic

$$K_q\left(\tau \right)=\frac{{<|X(t+\tau )-X\left(t\right)|}^q>}{{<\left|X\left(t\right)\right|}^q>}$$

which is given by

$$K_q\left(\tau \right)\propto {\tau }^{qH}·,$$

(4)

where $\tau$ is the scale (usually chosen between 1 and a quarter of the length of the series), H is the Hurst exponent, $<·>$ denotes the sample average on time t and q is the order of the moment considered. In this paper we will always use $q=1$.

The GHE is calculated by linear regression, taking logarithms in the expression contained in (4) for different values of $\tau$ [3,43].

The interpretation of H is as follow: when H is greater than $0.5$, the process is persistent, when H is less than $0.5$, it is anti persistent, while Brownian motion has a value of H equal to $0.5$.

With this technique, pairs with the lowest Hurst exponent has to be chosen in order to apply reversion to the mean strategies which is also the base of correlation and cointegration strategies.

2.4. Pairs Trading Strategy

Next, we describe the pairs trading strategy, which is taken from Reference [11]. As usual, we consider two periods. The first one is the formation period (one year), which is used for the pair selection. This is done using the four methods defined in this section (distance, correlation, cointegration and Hurst exponent). The second period is the execution period (six months), in which all selected pairs are traded as follows:

• In case $s>m+\sigma$ the pair will be sold. The position will be closed if $s<m$ or $s>m+2\sigma$.
• In case $s<m-\sigma$ the pair will be bought. The position will be closed if $s>m$ or $s<m-2\sigma$.

where m is a moving average of the series of the pair and s is a moving standard deviation of m.

3. Forming the Pair: Some New Proposals

As we remarked previously, all works assume that the amount purchased in a stock is equal to the amount sold in the other pair component. The main contribution of this paper is to analyse if not assuming an equal weight ratio in the formation of the pair improves the performance of the different pair trading strategies. In this section different methods are proposed.

When a pair is formed, we use two stocks A and B. This two stocks have to be normalized somehow, so we introduce a constant b such that stock A is comparable to stock $bB$. Then, to buy an amount T of the pair $AB$ means that we buy $\frac{1}{b+1}T$ of stock A and sell $\frac{b}{b+1}T$ of stock B, while to sell an amount T of the pair $AB$ means that we sell $\frac{1}{b+1}T$ of stock A and buy $\frac{b}{b+1}T$ of stock B.

We will denote by $p_X\left(t\right)$ the logarithm of the price of stock X in time t minus the logarithm of the price of stock X at time $t=0$, that is $p_X\left(t\right)=log\left(pric{e}_X\left(t\right)\right)-log\left(pric{e}_X\left(0\right)\right)$, and by $r_X\left(t\right)$ the log-return of stock X between times $t-1$ and t, $r_X\left(t\right)=p_X\left(t\right)-p_X(t-1)$.

In this paper we discuss the following ways to calculate the weight factor b:

1.

Equal weight ($EW$).

In this case $b=1$. This is the way used in most of the literature. In this case, the position in the pair is dollar neutral. This method was used in Reference [16], and since then, it has become the more popular procedure to fix b.

2.

Based on volatility.

Volatility of stock A is $std\left(r_A\right)$ and volatility of stock B is $std\left(r_B\right)$. If we want that A and $bB$ have the same volatility then $b=std\left(r_A\right)/std\left(r_B\right)$. This approach was used in Reference [11] and it is based on the idea that both stocks are normalized if they have the same volatility.

3.

Based on minimal distance of the log-prices.

In this case we minimize the function $f\left(b\right)={\sum }_t|p_A\left(t\right)-b{p}_B\left(t\right)|$, so we look for the weight factor b such that $p_A$ and $b{p}_B$ has the minimum distance. This approach is based on the same idea that the distance as a selection method. The closer is the evolution of the log-price of stocks A and $bB$, the more reverting to the mean properties the pair will have.

4

Based on correlation of returns.

If returns are correlated then $r_A$ is approximately equal to $b{r}_B$, where b is obtained by linear regression $r_A=b{r}_B$. In this case, if returns of stocks A and B are correlated, then the distribution of $r_A$ and $b{r}_B$ will be the same, so we can use this b to normalize both stocks.

5.

Based on cointegration of the prices.

If the prices (in fact, the log-prices) of both stocks A and B are cointegrated then $p_A-b{p}_B$ is stationary, whence b is obtained by linear regression $p_A=b{p}_B$. In this case, this value of b makes the pair series stationary so we can expect reversion to the mean properties of the pair series. Even if the stocks A and B are not perfectly cointegrated, this method for the calculation of b may be still valid, since, thought $p_A-b{p}_B$ may be not stationary, it can be somehow close to it or still have mean-reversion properties.

6.

Based on lowest Hurst exponent of the pair.

The series of the pair is defined as $s\left(b\right)\left(t\right)=p_A\left(t\right)-b{p}_B\left(t\right)$. In this case, we look for the weight factor b such that the series of the pair $s\left(b\right)$ has the lowest Hurst exponent, what implies that the series is as anti-persistent as possible. So we look for b which minimizes the function $f\left(b\right)=H\left(s\right(b\left)\right)$, where $H\left(s\right(b\left)\right)$ is the Hurst exponent of the pair series $s\left(b\right)$. The idea here is similar to the cointegration method, but from a theoretical point of view, we do not expect $p_A-b{p}_B$ to be stationary (which is quite difficult with real stocks), but to be anti-persistent, which is enough for our trading strategy.

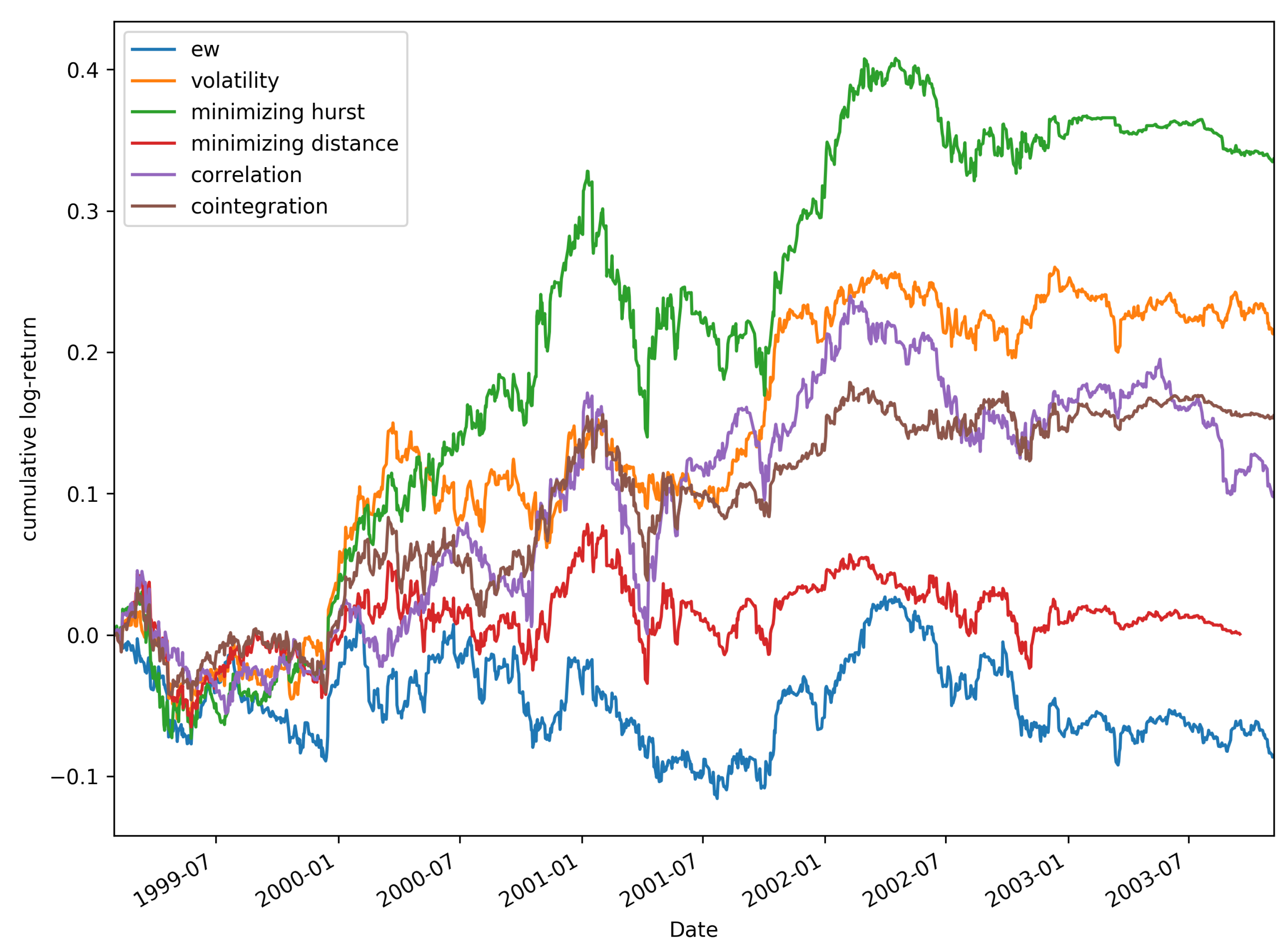
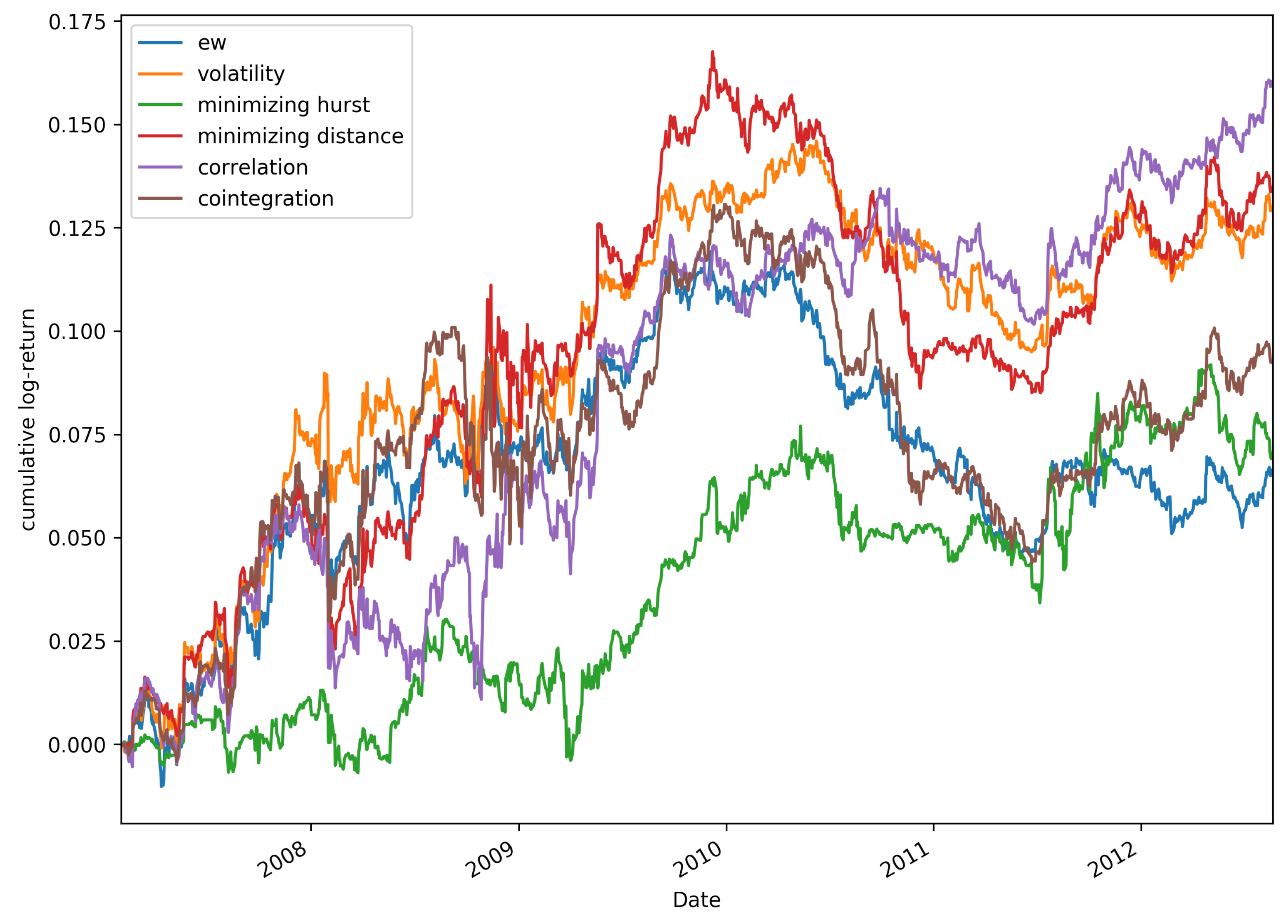
4. Experimental Results

For testing the results through the different models introduced in this paper, we will use the components of the Nasdaq 100 index technological sector (see

Table A1. The Technology Sector Nasdaq 100.

Ticker	Company
AAPL	Apple Inc.
ADBE	Adobe Systems Incorporated
ADI	Analog Devices, Inc.
ADP	Automatic Data Processing, Inc.
ADSK	Autodesk, Inc.
AMAT	Applied Materials, Inc.
ATVI	Activision Blizzard, Inc.
AVGO	Broadcom Limited
BIDU	Baidu, Inc.
CA	CA, Inc.
CERN	Cerner Corporation
CHKP	Check Point Software Technologies Ltd.
CSCO	Cisco Systems, Inc.
CTSH	Cognizant Technology Solutions Corporation
CTXS	Citrix Systems, Inc.
EA	Electronic Arts Inc.
FB	Facebook, Inc.
FISV	Fiserv, Inc.
GOOG	Alphabet Inc.
GOOGL	Alphabet Inc.
INTC	Intel Corporation
INTU	Intuit Inc.
LRCX	Lam Research Corporation
MCHP	Microchip Technology Incorporated
MSFT	Microsoft Corporation
MU	Micron Technology, Inc.
MXIM	Maxim Integrated Products, Inc.
NVDA	NVIDIA Corporation
QCOM	QUALCOMM Incorporated
STX	Seagate Technology plc
SWKS	Skyworks Solutions, Inc.
SYMC	Symantec Corporation
TXN	Texas Instruments Incorporated
VRSK	Verisk Analytics, Inc.
WDC	Western Digital Corporation
XLNX	Xilinx, Inc.

in Appendix A),for the period between January 1999 and December 2003, coinciding with the “dot.com” bubble crash and the period between January 2007 and December 2012, this period coincides with the financial instability caused by the “subprime” crisis. These periods are choosen based on the results showed by Sánchez et al. [9].

We use Pairs Trading traditional methods (Distance Method, Correlation and Cointegration) in addition to the method developed by Ramos et al. [11] based on the Hurst exponent.

In Appendix B, it is shown the results obtained for different selection methods and different ways to calculate b, for the two selected periods. In addition to the returns obtained for each portfolio of pairs, we include two indicators of portfolio performance and risk, the Sharpe Ratio and the maximum Drawdown.

In the first period analyzed, the $EW$ method to calculate b is never the best one. The best methods to calculate b seems to be the cointegration method and the minimization of the Hurst exponent. Also note that the Spearman correlation, the cointegration and the Hurst exponent selection methods provide strategies with high Sharpe ratios for several methods to calculate b.

In the second period analyzed, the $EW$ method to calculate b works fine with the cointegration selection method, but it is not so good with the other ones, while the correlation method to calculate b is often one of the best ones.

Note that, in both periods, the Sharpe ratio when we use $EW$ to calculate b are usually quite low with respect to the other methods.

Figure 1, Figure 2, Figure 3 and Figure 4 show the cumulative log-return of the strategy for different selection methods and different ways to calculate b.

Figure 1 shows the returns obtained for the period 1999–2003 using the co-integration approach as a selection method. We can observe that during the whole period, the best option is to choose to calculate the b factor by means of the lowest value of the Hurst exponent, while the $EW$ method is the worst.

Figure 2 represents the returns obtained for each of the b calculation methods for the 1999–2003 period, using the Hurst exponent method for the selection of pairs and a portfolio composed of 20 pairs. It can be observed that during the period studied, the results obtained using the $EW$ method are also negative, while the Hurst exponent method is again the best option.

For the period 2007–2012, for a portfolio composed of 20 pairs selected using the distance method, Figure 3 shows the cumulative returns for the different methods proposed. In this case we can highlight the methods of correlation, minimizing distance and cointegration, as the methods to calculate b that provide the highest returns. Again, we can observe that the worst options would be the $EW$ method together with the volatility one.

Figure 4 shows the results obtained using the different models to calculate the b factor for a portfolio of 10 pairs by selecting them using the Spearman model. We can observe that all returns are positive throughout the period studied (2007–2012). The most outstanding are the methods of correlation, minimum distance and volatility, which move in a very similar way during this period. On the contrary, the method of the lowest value of the Hurst exponent and the $EW$ one are the worst options during the whole period.

Finally, we complete our sensitivity analysis by analyzing the influence of the strategy considered in Section 2.4. We consider the Hurst exponent as the selection method, 20 pairs in the portfolio and the period 1999–2003. We change the strategy by using 1 (as before), 1.5 and 2 standard deviations. That is, we modify the strategy as follows:

• In case $s>m+k\sigma$ the pair will be sold. The position will be closed if $s<m$ or $s>m+2k\sigma$.
• In case $s<m-k\sigma$ the pair will be bought. The position will be closed if $s>m$ or $s<m-2k\sigma$.

where $k=1,1.5,2$.

Table A2. Comparison of results using the Hurst exponent selection method for the period 1999–2003 with 20 pairs and different numbers of standard deviations.

b Calculation Method	k ${}^1$	Sharpe ${}^2$	Profit TC ${}^3$
Cointegration	1.0	0.39	14.55%
Cointegration	1.5	0.60	26.00%
Cointegration	2.0	0.59	24.08%
Correlation	1.0	0.15	6.10%
Correlation	1.5	0.17	8.21%
Correlation	2.0	0.31	13.82%
EW	1.0	−0.28	−11.25%
EW	1.5	0.38	15.49%
EW	2.0	0.21	7.74%
Lowest Hurst Exponent	1.0	0.70	40.51%
Lowest Hurst Exponent	1.5	0.51	28.00%
Lowest Hurst Exponent	2.0	0.57	28.51%
Minimal Distance	1.0	0.03	0.05%
Minimal Distance	1.5	0.39	15.70%
Minimal Distance	2.0	0.31	11.48%
Volatility	1.0	0.49	18.22%
Volatility	1.5	0.41	16.37%
Volatility	2.0	0.25	9.12%

${}^1$ number of standard deviations; ${}^2$ Sharpe Ratio; ${}^3$ Profitability with transaction costs.

shows that the $EW$, correlation and minimal distance obtain the worst results, while cointegration and the Hurst exponent obtain robust and better results for the different values of k.

Discussion of the Results

In

Table A3. Comparison of results using the distance selection method for the period 1999–2003.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1375	0.40%	0.72%	0.05	13.70%
Correlation	10	1357	−0.60%	−4.36%	−0.07	18.60%
EW	10	1403	−1.30%	−7.30%	−0.15	19.60%
Minimal distancie	10	1389	−0.90%	−5.49%	−0.10	16.30%
Lowest Hurst Exponent	10	1352	−1.50%	−8.55%	−0.16	19.20%
Volatility	10	1370	−1.30%	−7.57%	−0.16	13.90%
Cointegration	20	2786	3.50%	16.31%	0.47	7.40%
Correlation	20	2630	2.80%	12.68%	0.36	9.20%
EW	20	2884	1.00%	3.36%	0.14	12.30%
Minimal distancie	20	2794	2.50%	11.00%	0.34	8.30%
Lowest Hurst Exponent	20	2685	0.60%	1.66%	0.08	12.00%
Volatility	20	2812	0.40%	0.39%	0.06	8.70%
Cointegration	30	4116	2.80%	12.83%	0.42	8.00%
Correlation	30	3830	2.00%	8.62%	0.27	12.60%
EW	30	4247	1.10%	4.18%	0.18	14.80%
Minimal distancie	30	4105	1.90%	7.93%	0.28	8.40%
Lowest Hurst Exponent	30	3861	0.30%	0.01%	0.04	11.80%
Volatility	30	4160	0,10%	-0,99%	0.01	9,20%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A4. Comparison of results using the distance selection method for the period 2007–2012.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1666	1.80%	8.73%	0.35	10.20%
Correlation	10	1594	3.50%	19.51%	0.55	12.10%
EW	10	1677	1.20%	5.42%	0.22	9.40%
Minimal distance	10	1649	2.80%	15.15%	0.56	8.80%
Lowest Hurst Exponent	10	1677	2.60%	13.42%	0.51	8.00%
Volatility	10	1684	1.20%	5.22%	0.24	11.90%
Cointegration	20	3168	2.60%	13.82%	0.60	6.50%
Correlation	20	2985	3.10%	16.91%	0.58	9.30%
EW	20	3219	1.70%	8.19%	0.36	4.20%
Minimal distance	20	3172	3.10%	17.01%	0.72	6.20%
Lowest Hurst Exponent	20	3116	2.20%	11.54%	0.51	7.20%
Volatility	20	3221	2.00%	9.89%	0.48	10.00%
Cointegration	30	4714	1.50%	7.33%	0.38	6.70%
Correlation	30	4453	1.40%	6.42%	0.29	10.90%
EW	30	4791	1.40%	6.50%	0.34	5.30%
Minimal distance	30	4709	1.70%	8.43%	0.44	5.90%
Lowest Hurst Exponent	30	4545	1.40%	6.48%	0.35	6.80%
Volatility	30	4785	1.60%	7.60%	0.43	9.00%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A5. Comparison of results using the Spearman correlation selection method for the period 1999–2003.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1274	4.10%	19.93%	0.50	14.30%
Correlation	10	1432	3.00%	13.67%	0.36	11.20%
EW	10	1400	4.30%	20.80%	0.56	9.30%
Minimal distance	10	1219	4.00%	19.68%	0.51	12.50%
Lowest Hurst Exponent	10	1103	5.70%	29.20%	0.64	10.70%
Volatility	10	1405	3.30%	15.39%	0.45	8.40%
Cointegration	20	2583	4.70%	23.41%	0.69	12.30%
Correlation	20	2833	3.90%	18.78%	0.55	10.90%
EW	20	2814	2.80%	12.69%	0.45	8.30%
Minimal distance	20	2538	4.40%	21.63%	0.65	12.50%
Lowest Hurst Exponent	20	2176	3.50%	16.71%	0.48	10.40%
Volatility	20	2781	2.50%	11.01%	0.41	9.30%
Cointegration	30	3776	4.90%	24.54%	0.79	8.10%
Correlation	30	4196	2.80%	12.90%	0.41	8.60%
EW	30	4168	0.40%	0.71%	0.08	9.90%
Minimal distance	30	3717	4.20%	20.76%	0.69	8.30%
Lowest Hurst Exponent	30	3236	4.20%	20.72%	0.56	8.20%
Volatility	30	4125	1.20%	4.52%	0.22	9.50%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A6. Comparison of results using the Spearman correlation selection method for the period 2007–2012.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1614	1.70%	8.09%	0.38	8.30%
Correlation	10	1653	2.90%	15.75%	0.75	4.60%
EW	10	1620	1.20%	5.28%	0.37	7.00%
Minimal distancie	10	1551	2.50%	13.05%	0.58	7.80%
Lowest Hurst Exponent	10	1117	1.30%	6.18%	0.42	4.20%
Volatility	10	1668	2.30%	12.13%	0.72	5.00%
Cointegration	20	3022	1.00%	4.09%	0.29	6.80%
Correlation	20	3268	2.60%	13.77%	0.75	4.00%
EW	20	3236	1.40%	6.78%	0.46	5.70%
Minimal distance	20	2944	1.10%	4.93%	0.34	7.80%
Lowest Hurst Exponent	20	1966	0.40%	1.12%	0.14	3.90%
Volatility	20	3282	1.30%	5.76%	0.42	4.70%
Cointegration	30	4342	0.80%	3.15%	0.27	5.80%
Correlation	30	4872	2.60%	13.58%	0.74	4.30%
EW	30	4814	1.60%	7.80%	0.57	4.90%
Minimal distance	30	4222	0.90%	3.69%	0.30	7.00%
Lowest Hurst Exponent	30	2718	0.60%	2.49%	0.26	2.80%
Volatility	30	4864	1.90%	9.28%	0.67	3.60%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A7. Comparison of results using the cointegration selection method for the period 1999–2003.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	998	5.30%	26.80%	0.58	12.40%
Correlation	10	1015	7.30%	39.38%	0.78	9.30%
EW	10	1369	4.30%	20.83%	0.41	10.30%
Minimal distance	10	945	4.00%	19.45%	0.47	9.40%
Lowest Hurst Exponent	10	1123	7.70%	41.68%	0.79	11.90%
Volatility	10	1376	6.90%	36.62%	0.68	11.00%
Cointegration	20	1984	5.50%	28.41%	0.78	9.00%
Correlation	20	1985	5.50%	28.31%	0.76	6.40%
EW	20	2718	2.90%	13.24%	0.36	9.90%
Minimal distance	20	1876	4.50%	22.36%	0.67	8.10%
Lowest Hurst Exponent	20	2031	6.90%	36.88%	0.90	7.70%
Volatility	20	2688	4.10%	19.76%	0.50	11.50%
Cointegration	30	2957	0.90%	3.51%	0.14	11.00%
Correlation	30	3132	2.40%	11.06%	0.36	10.30%
EW	30	4064	−0.10%	−1.85%	−0.01	12.20%
Minimal distance	30	2783	0.70%	2.67%	0.12	9.40%
Lowest Hurst Exponent	30	2924	3.60%	17.23%	0.50	7.50%
Volatility	30	4040	0.90%	3.25%	0.12	13.00%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A8. Comparison of results using the cointegration selection method for the period 2007–2012.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1516	−1.00%	−6.82%	−0.19	15.50%
Correlation	10	1512	−0.10%	−2.11%	−0.02	14.70%
EW	10	1604	1.40%	6.80%	0.30	9.60%
Minimal distance	10	1478	0.70%	2.32%	0.12	12.50%
Lowest Hurst Exponent	10	1502	−1.60%	−9.90%	−0.32	10.90%
Volatility	10	1635	−0.10%	−2.14%	−0.02	12.90%
Cointegration	20	2884	−0.70%	−5.44%	−0.19	9.90%
Correlation	20	2955	−0.70%	−5.28%	−0.16	11.70%
EW	20	3195	1.80%	8.90%	0.48	4.40%
Minimal distance	20	2709	0.20%	−0.15%	0.06	9.50%
Lowest Hurst Exponent	20	2666	−0.90%	−6.53%	−0.26	9.00%
Volatility	20	3189	0.50%	1.31%	0.14	8.90%
Cointegration	30	4142	0.00%	−1.38%	0.00	8.80%
Correlation	30	4373	0.20%	−0.56%	0.04	9.50%
EW	30	4720	2.70%	14.63%	0.75	4.90%
Minimal distance	30	3923	1.10%	4.69%	0.28	7.90%
Lowest Hurst Exponent	30	3694	−0.30%	−2.93%	−0.09	7.60%
Volatility	30	4742	1.30%	5.82%	0.36	7.00%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

,

Table A9. Comparison of results using the Hurst exponent selection method for the period 1999–2003.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1136	−0.60%	−3.94%	−0.06	15.60%
Correlation	10	1176	0.50%	1.32%	0.04	24.40%
EW	10	1334	2.80%	12.87%	0.29	12.20%
Minimal distance	10	1166	−1.20%	−6.87%	−0.12	13.50%
Lowest Hurst Exponent	10	1234	4.60%	22.77%	0.37	21.40%
Volatility	10	1401	7.40%	39.60%	0.72	14.40%
Cointegration	20	2104	3.10%	14.55%	0.39	11.10%
Correlation	20	2400	1.50%	6.10%	0.15	15.70%
EW	20	2695	-2.10%	−11.25%	−0.28	12.30%
Minimal distance	20	2093	0.20%	0.05%	0.03	10.60%
Lowest Hurst Exponent	20	2375	7.50%	40.51%	0.70	17.10%
Volatility	20	2755	3.80%	18.22%	0.49	8.90%
Cointegration	30	2984	3.10%	14.91%	0.48	7.80%
Correlation	30	3516	2.00%	8.83%	0.22	16.50%
EW	30	4066	−1.30%	−7.56%	−0.19	11.80%
Minimal distance	30	2915	2.70%	12.63%	0.41	6.50%
Lowest Hurst Exponent	30	3411	7.10%	37.86%	0.78	13.40%
Volatility	30	3994	4.40%	21.57%	0.63	6.50%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

and

Table A10. Comparison of results using the Hurst exponent selection method for the period 2007–2012.

b Calculation Method	N ${}^1$	Oper ${}^2$	AR ${}^3$	%Profit TC ${}^4$	Sharpe ${}^5$	Max Drawdown
Cointegration	10	1596	3.00%	16.40%	0.55	8.00%
Correlation	10	1587	3.70%	21.51%	0.57	9.80%
EW	10	1643	3.00%	16.26%	0.59	9.10%
Minimal distancie	10	1581	2.10%	11.02%	0.41	8.70%
Lowest Hurst Exponent	10	1649	4.80%	28.15%	0.83	8.30%
Volatility	10	1724	1.30%	5.98%	0.27	8.40%
Cointegration	20	2795	0.80%	3.40%	0.21	7.10%
Correlation	20	3001	2.60%	14.10%	0.50	7.50%
EW	20	3258	1.70%	8.27%	0.40	9.10%
Minimal distancie	20	2758	−0.40%	−3.48%	−0.10	10.60%
Lowest Hurst Exponent	20	3129	1.90%	9.84%	0.39	8.30%
Volatility	20	3204	0.40%	0.90%	0.11	6.40%
Cointegration	30	4100	−0.20%	−2.27%	−0.05	9.30%
Correlation	30	4418	2.00%	10.23%	0.43	8.10%
EW	30	4666	1.90%	9.34%	0.46	7.60%
Minimal distancie	30	4049	0.00%	−1.55%	−0.01	10.50%
Lowest Hurst Exponent	30	4248	0.40%	0.78%	0.09	9.20%
Volatility	30	4790	0.60%	1.50%	0.15	8.40%

${}^1$ Number of pairs; ${}^2$ Number of operations; ${}^3$ Annualised return; ${}^4$ Profitability with transaction costs; ${}^5$ Sharpe Ratio.

, the results obtained with a pair trading strategy are shown. In those tables, we have consider four different methods for the pair selection (distance, correlation, cointegration and Hurst exponent), three different number of pairs (10, 20 and 30 pairs) and two periods (1999–2003 and 2007–2012). Overall, if we focus on the Sharpe ratio of the results, in $58\%$ of the cases (14 out of 24) the $EW$ method for calculating b obtains one of the three (out of seven) worst results. If we compare the $EW$ method with the other methods proposed we obtain the following: minimal Hurst exponent is better than $EW$ in $58\%$ of the cases, minimal distance is better than $EW$ in $58\%$ of the cases, correlation is better than $EW$ in $67\%$ of the cases, cointegration is better than $EW$ in $58\%$ of the cases and volatility is better than $EW$ in $50\%$ of the cases. So, in general, the proposed methods (except the volatility one) tend to be better than the $EW$ one.

However, since we are considering stocks in the technology sector, if we focus in the dot.com bubble (that is, the period 1999–2003) which affected more drastically to the stocks in the portfolio, we have, considering the Sharpe ratio of the results, that in $83\%$ of the cases (10 out of 12) the $EW$ method for calculating b obtains one of the three (out of seven) worst results. In this period, if we compare the $EW$ method with the other methods proposed we obtain the following: minimal Hurst exponent is better than $EW$ in $75\%$ of the cases, minimal distance is better than $EW$ in $83\%$ of the cases, correlation is better than $EW$ in $83\%$ of the cases, cointegration is better than $EW$ in $83\%$ of the cases and volatility is better than $EW$ in $58\%$ of the cases. So, in general, the proposed methods (except the volatility one) tend to be much better than the $EW$ one in this period.

On the other hand, in the second period (2007–2012), the $EW$ performs much better than in the first period (1999–2003) and it does similarly or slightly better than the other methods.

Results show that these novel approaches used to calculate the factor b improve the results obtained compared with the classic $EW$ method for the different strategies and mainly in the first period considered (1999–2003). Therefore, it seems that the performance of pairs trading can be improved not only acting on the strategy, but also on the method for the allocation in each stock.

In this section we have tested different methods for the allocation in each stock of the pair. Though we have used the different allocation methods with all the selection methods analyzed, it is clear that some combinations make more sense than others. For example, if the selection of the pair is done by selecting the pair with a lower Hurst exponent, the allocation method based on the minimization of the Hurst exponent of the pair should work better than other allocation methods.

One of the main goal of this paper is to point out that the allocation in each stock of the pair can be improved in the pairs trading strategy and we have given some ways to make this allocation. However, further research is needed to asses which of the methods is the best for this purpose. Even better, which of the combinations of selection and allocation method is the best. Though this problem depends on many factors, and some of them changes, depending on investor preferences, a multi-criteria decision analysis (see, for example References [44,45,46]) seems to be a good approach to deal with it.

In fact, in future research it can be tested if the selection method can be improved if we take into account the allocation method. For example, for the distance selection method, we can use the allocation method based on the minimization of the distance to normalize the price of the stocks in a different way than in the classical distance selection method, taking into account the allocation in each stock. Not all selection methods can be improved in this way (for example, the correlation selection method will not improve), but some of them, including some methods which we have not analyzed in this paper or future selection methods, could be improved.

5. Conclusions

In pairs trading literature, researchers have focused their attention in increasing pairs trading performance proposing different methodologies for pair selection. However, in all cases it is assumed that the amount invested in each stock of a pair (b) must be equal. This technique is called Equally Weighted ($EW$).

This paper presents a novel approach to try to improve the performance of this statistical arbitrage technique through novel methodologies in the calculation of b. Any selection method can benefit from these new allocation methods. Depending on the selection method used, we prove that the new methodologies for calculating the factor b obtain a greater return than those used up to the present time.

Results show that the classic EW method does not performance as well as the others. Cointegration, correlation and Hurst exponent give excellent results when are used to calculate factor b.