On the Effect of the Absorption Coefficient in a Differential Game of Pollution Control
Abstract
:1. Introduction
2. Cooperative Differential Game in the form of the Characteristic Function
2.1. Differential Game in Normal Form
2.2. Different Methods of Characteristic Function Construction
2.2.1. -Characteristic Function
2.2.2. -Characteristic Function
2.2.3. -Characteristic Function
2.2.4. -Characteristic Function
3. Problem of Optimal Pollution Control
3.1. Problem Statement
3.2. Influence of the Absorption Coefficient on the Payoff
4. Game-Theoretical Model of Pollution Control
4.1. No Absorption Coefficient Model
4.2. Absorption Coefficient Model
5. Optimal Control of Pollution Emissions for the Irkutsk Region
Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Construction of Optimal Pollution Control
Appendix B. Computation of the Characteristic Functions
Appendix B.1. Nash Equilibrium
Appendix B.2. Cooperative Agreement
Appendix B.3. Construction of the α-Characteristic Function
Appendix B.4. Construction of the δ-Characteristic Function
Appendix B.5. Construction of the ζ-Characteristic Function
Appendix B.6. Construction of the η-Characteristic Function
Appendix C. Proofs of Superadditivity Characteristic Functions
Appendix C.1. Additional Statement
Appendix C.2. Superadditivity of the α-Characteristic Function
Appendix C.3. Superadditivity of the δ-Characteristic Function
Appendix C.4. Superadditivity of the ζ-Characteristic Function
Appendix C.5. Superadditivity of the η-Characteristic Function
References
- Petrosjan, L.; Zakharov, V. Mathematical Models in Ecology; Izdatelstvo Sankt-Peterburgskogo Universiteta: St. Petersburg, Russia, 1997. [Google Scholar]
- Mazalov, V.; Rettieva, A. Nash equilibrium in environmental problems. Math. Model. 2006, 18, 73–90. [Google Scholar]
- Rettieva, A. Cooperative incentive condition in bioresource sharing problem. Upravleniye Bolshimi Sistemami 2009, 26, 366–384. [Google Scholar]
- Breton, M.; Zaccour, G.; Zahaf, A. A differential game of joint implementation of environmental projects. Automatica 2005, 41, 1737–1749. [Google Scholar] [CrossRef]
- Dockner, E.; Van Long, N. International pollution control: Cooperative versus noncooperative strategies. J. Environ. Econ. Manag. 1993, 25, 13–29. [Google Scholar] [CrossRef] [Green Version]
- Haurie, A.; Zaccour, G. Differential game models of global environmental management. Ann. Int. Soc. Dyn. Games 1995, 2, 3–23. [Google Scholar]
- Petrosjan, L.; Zaccour, G. Time-consistent Shapley value allocation of pollution cost reduction. J. Econ. Dyn. Control 2003, 27, 381–398. [Google Scholar] [CrossRef]
- Greenberg, J. Coalition structures. In Handbook of Game Theory with Economic Applications; North Holland: Amsterdam, The Netherlands, 1994; Volume 2, pp. 1306–1337. [Google Scholar]
- Hajduková, J. Coalition formation games: A survey. Int. Game Theory Rev. 2006, 8, 613–641. [Google Scholar] [CrossRef]
- Shapley, L. A value for n-person games. In Contributions to the Theory of Games II; Princeton University Press: Princeton, NJ, USA, 1953; pp. 307–317. [Google Scholar]
- Winter, E.; Aumann, R. The Shapley value. In Handbook of Game Theory with Economic Applications; North Holland: Amsterdam, The Netherlands, 2002; Volume 3, pp. 1521–2351. [Google Scholar]
- Alvin, E. Introduction to the Shapley value. In The Shapley Value: Essays in Honor of Lloyd S. Shapley; Cambridge University Press: Cambridge, UK, 1988. [Google Scholar]
- Basar, T.; Olsder, G. Dynamic Noncooperative Game Theory, 2nd ed.; Society for Industrial and Applied Mathematics (SIAM): Philadelphia, PA, USA, 1999. [Google Scholar]
- Hull, D. Optimal Control Theory for Applications; Springer: New York, NY, USA, 2003. [Google Scholar]
- Moulin, H. Equal or proportional division of a surplus, and other methods. Int. J. Game Theory 1987, 16, 161–186. [Google Scholar] [CrossRef]
- Gromova, E.; Tur, A. On the optimal control of pollution emissions for the largest enterprises of the Irkutsk region of the Russian Federation. Matematicheskaya Teoriya Igr i Ee Prilozheniya 2018, 2, 150–152. [Google Scholar]
- Petrosjan, L.; Danilov, N. Cooperative Differential Games and Their Applications; Tomsk University Press: Tomsk, Russia, 1982. [Google Scholar]
- Krasovskii, N.; Subbotin, A. Game-Theoretical Control Problems; Springer: New York, NY, USA, 1989. [Google Scholar]
- Gromova, E.; Petrosyan, L. On an approach to constructing a characteristic function in cooperative differential games. Autom. Remote Control 2017, 78, 1680–1692. [Google Scholar] [CrossRef]
- von Neumann, J.; Morgenstern, O. Theory of Games and Economic Behavior; Princeton University Press: Princeton, NJ, USA, 1953. [Google Scholar]
- Reddy, P.; Shevkoplyas, E.; Zaccour, G. Time-consistent Shapley value for games played over event trees. Automatica 2013, 49, 1521–1527. [Google Scholar] [CrossRef]
- Gromova, E.; Marova, E. Coalition and anti-coalition interaction in cooperative differential games. IFAC PapersOnLine 2018, 51, 479–483. [Google Scholar] [CrossRef]
- Gromova, E.; Marova, E.; Gromov, D. A substitute for the classical Neumann-Morgenstern characteristic function in cooperative differential games. J. Dyn. Games 2020, 7, 105–122. [Google Scholar] [CrossRef] [Green Version]
- Petrosjan, L.; Danilov, N. Stability of solutions in non-zero sum differential games with transferable payoffs. Vestnik Leningradskogo Universiteta. Ser. Matematika Mekhanika Astronomiya 1979, 1, 52–59. [Google Scholar]
- Dockner, E.; Jorgensen, S.; van Long, N.; Sorger, G. Differential Games in Economics and Management Science; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Kostyunin, S.; Palestini, A.; Shevkoplyas, E. On a nonrenewable resource extraction game played by asymmetric firms. SIAM J. Optim. Theory Appl. 2014, 163, 660–673. [Google Scholar] [CrossRef]
- Mazalov, V.; Rettieva, A. Fish wars with many players. Int. Game Theory Rev. 2010, 12, 385–405. [Google Scholar] [CrossRef]
- Gromova, E.; Malahova, A.; Marova, E. On the superadditivity of a characteristic function in cooperative differential games with negative externalities. In Proceedings of the Constructive Nonsmooth Analysis and Related Topics, St. Petersburg, Russia, 22–27 May 2017; pp. 1–4. [Google Scholar]
- Petrosjan, L.; Gromova, E. Two-level cooperation in coalitional differential games. Trudy Instituta Matematiki i Mekhaniki UrO RAN 2014, 20, 193–203. [Google Scholar]
- Corless, R.M.; Gonnet, G.H.; Hare, D.E.G.; Knuth, D.E. On the Lambert W function. Adv. Comput. Math. 1996, 5, 329–359. [Google Scholar] [CrossRef]
- Gromova, E.; Marova, E. On the characteristic function construction technique in differential games with prescribed and random duration. Contrib. Game Theory Manag. 2018, 11, 53–65. [Google Scholar]
- Barsuk, P.; Gromova, E. Properties of one cooperative solution in a differential game of pollution control. In Proceedings of the IV Stability and Control Processes Conference 2020, Saint Petersburg, Russia, 20–24 April 2020. [Google Scholar]
- Gromova, E.; Tur, A.; Barsuk, P. A Pollution Control Problem for the Aluminum Production in Eastern Siberia: Differential Game Approach. 2020. Available online: https://arxiv.org/abs/2005.08260 (accessed on 15 April 2020).
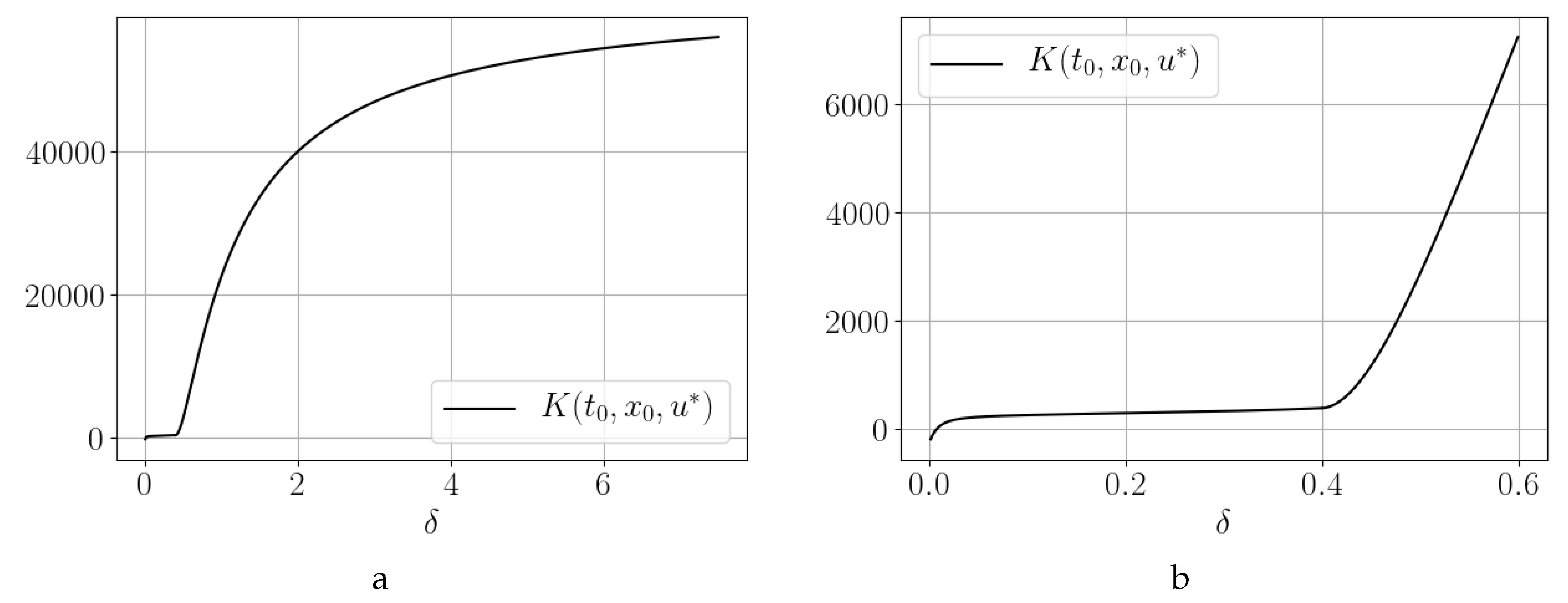
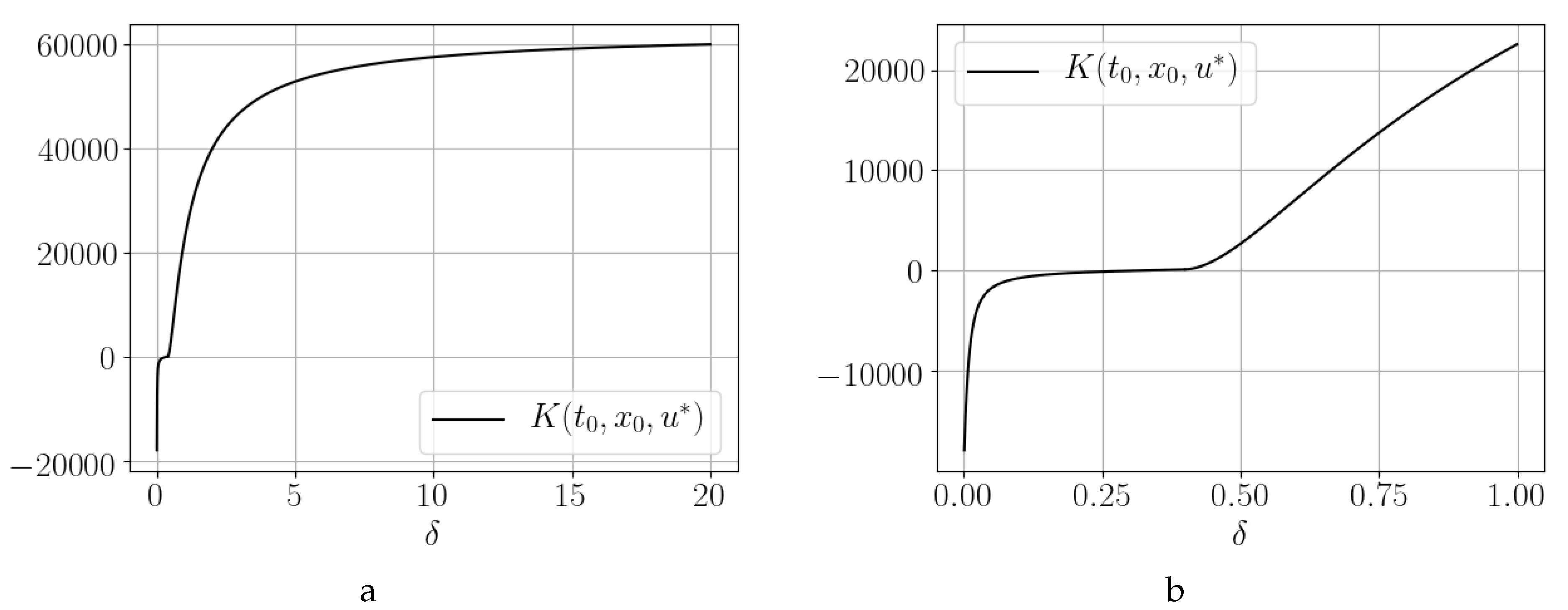
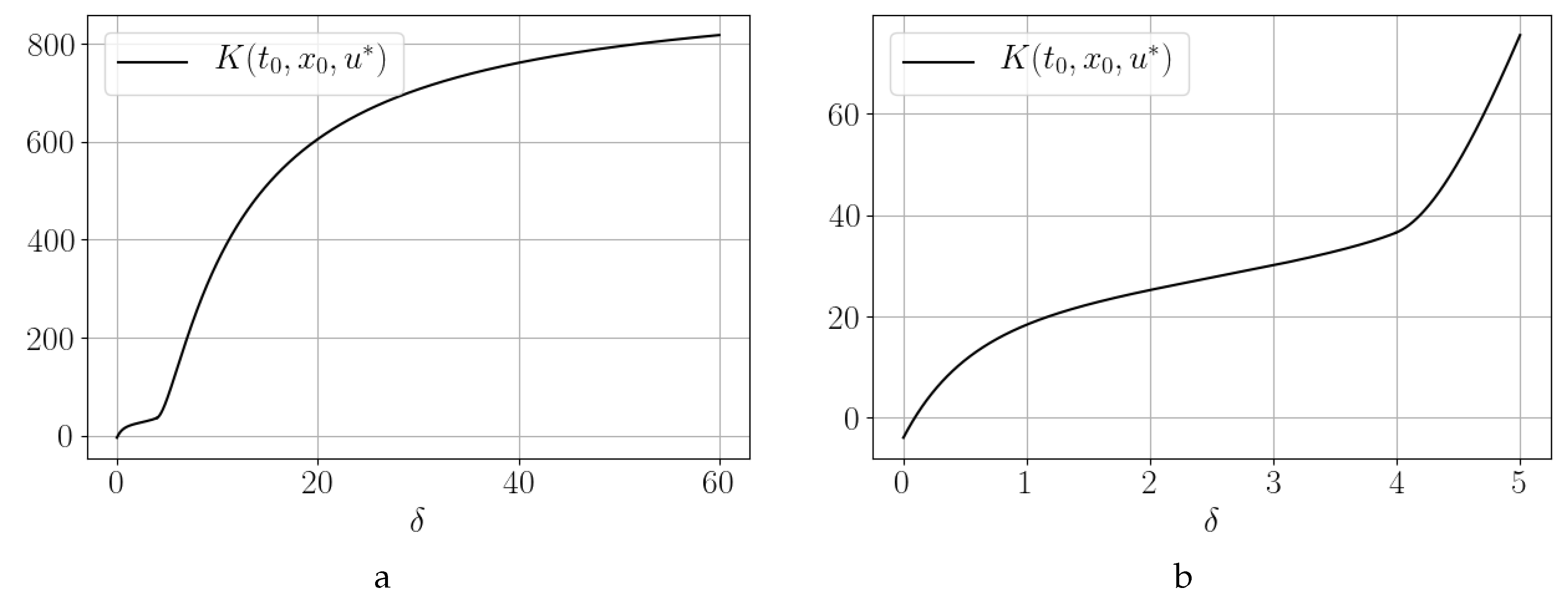
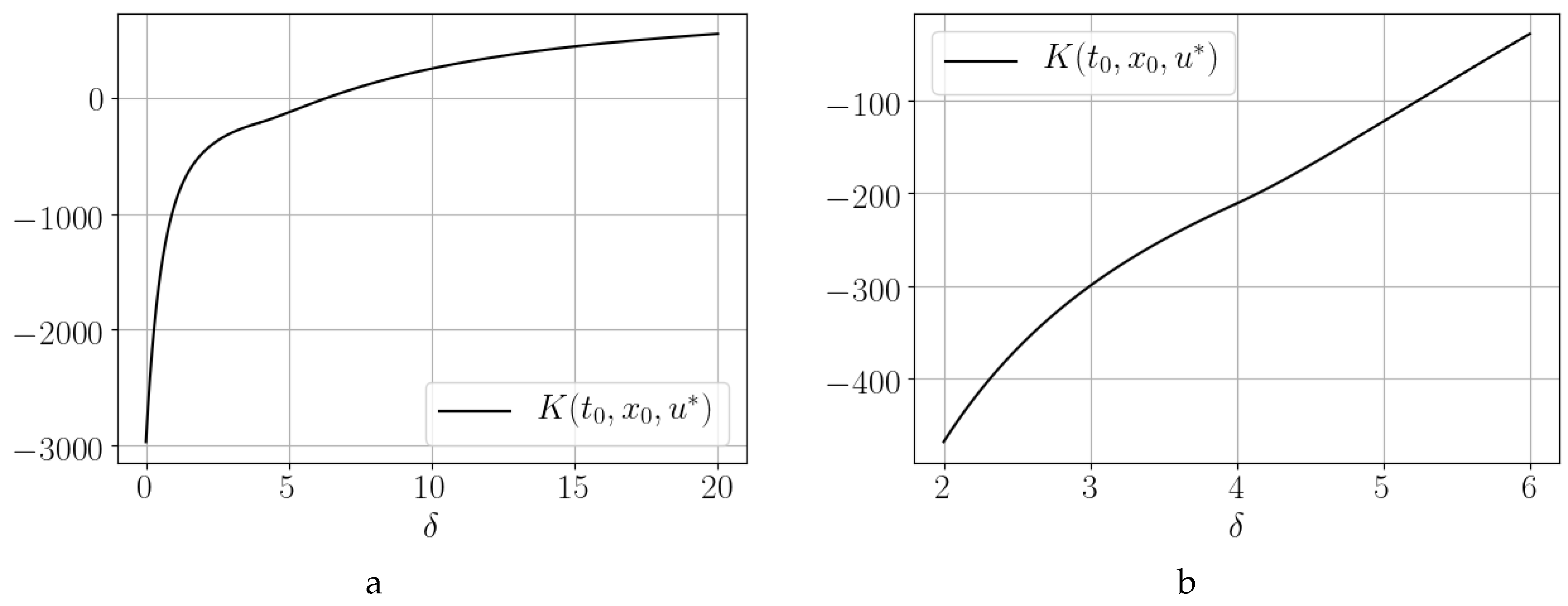

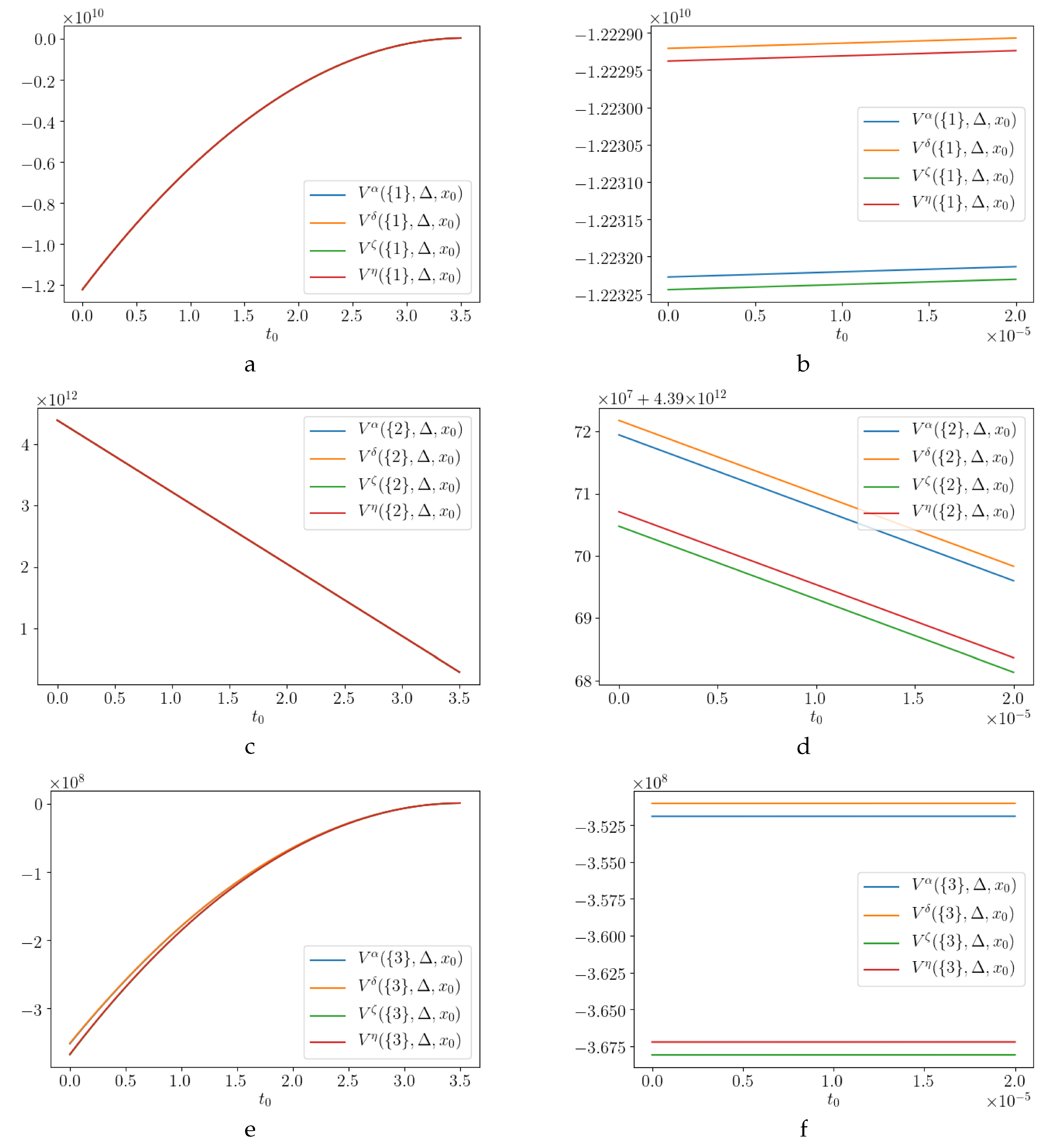
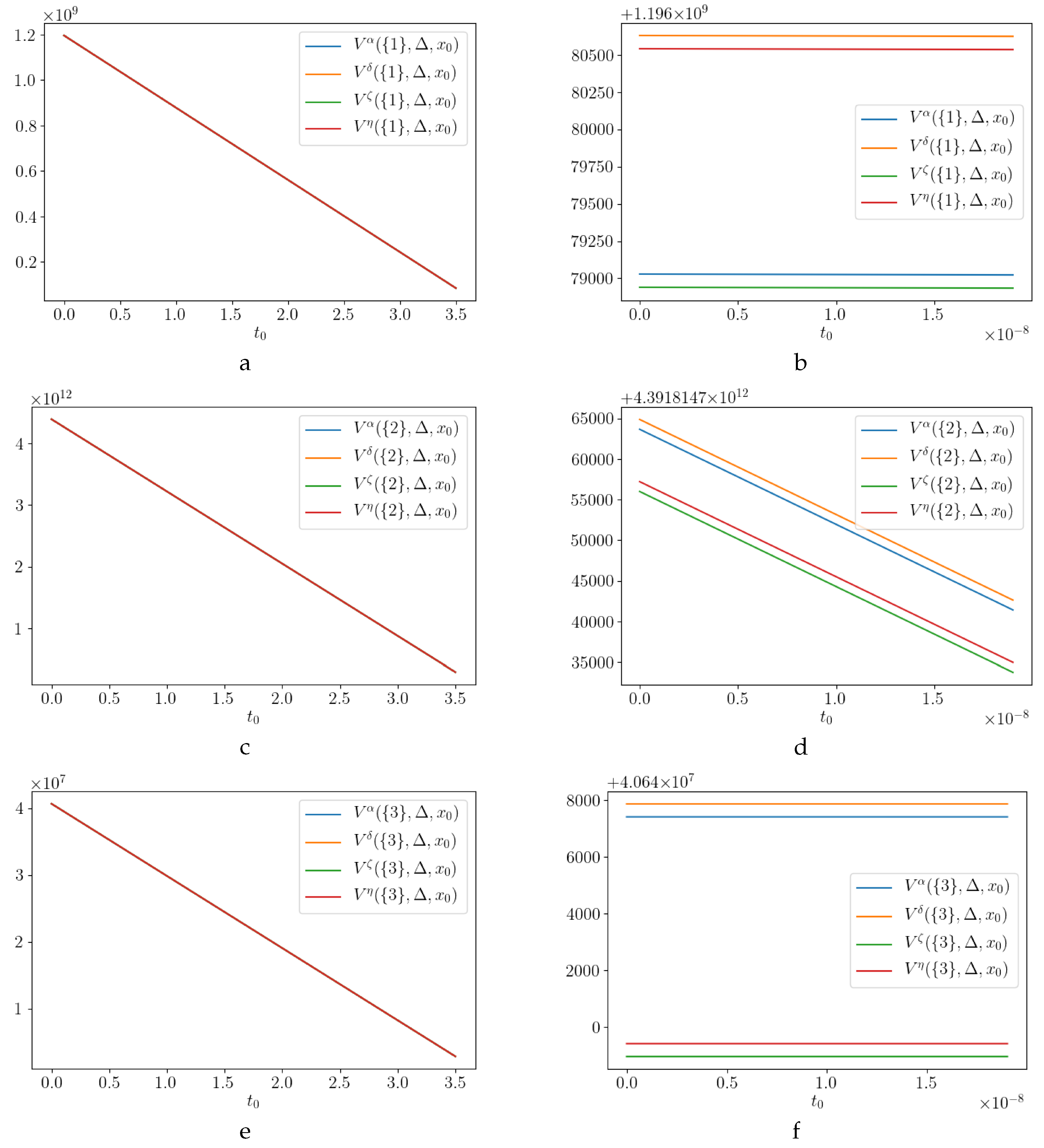

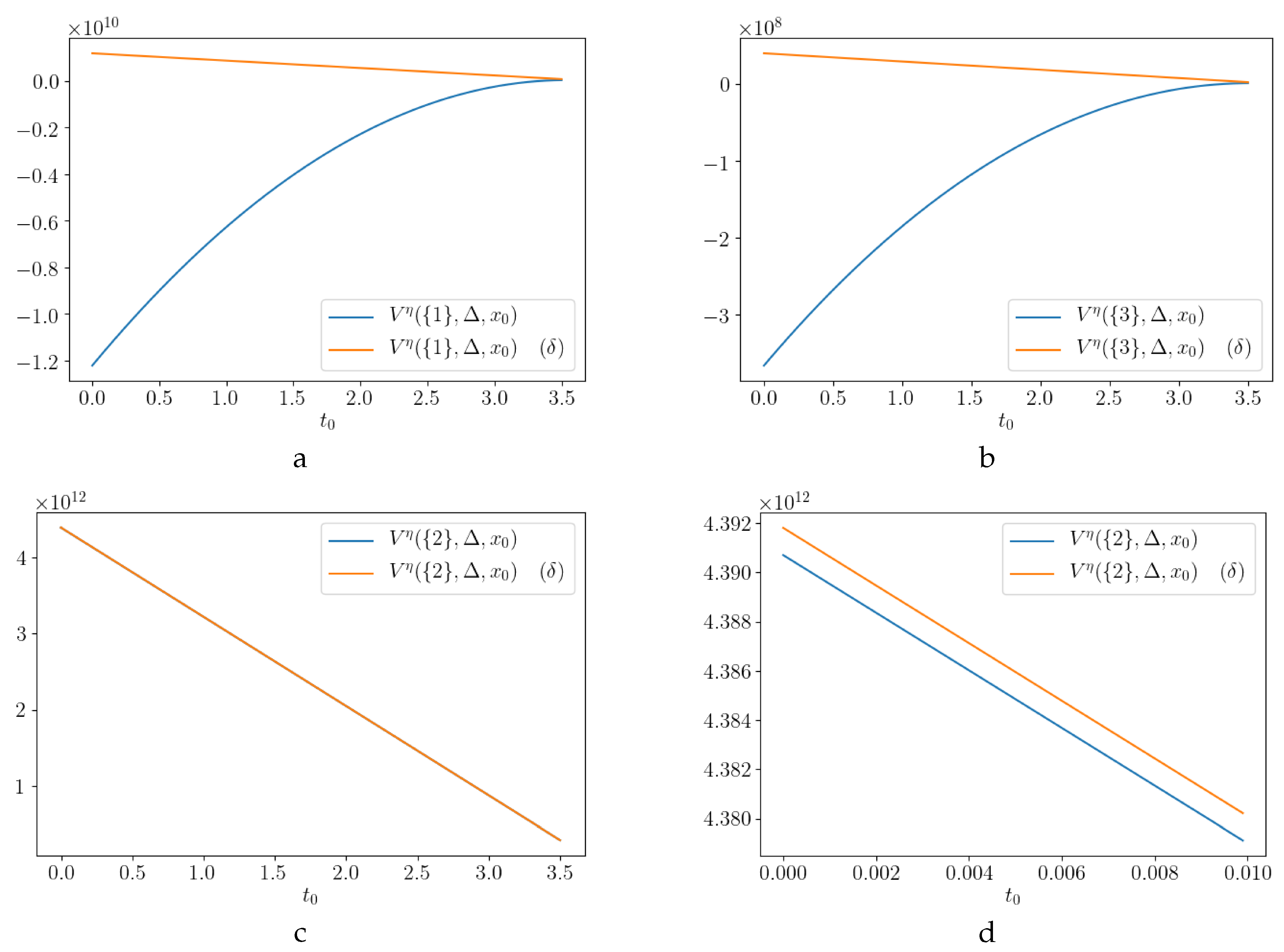
| Enterprise | ||
|---|---|---|
| OJSC «RUSAL Bratsk» | 28,838.01 | 1254.97 |
| OJSC «ILIM Group» | 1,530,463 | 102.27 |
| Units of OJSC «Irkutskenergo» | 5228.4 | 36.65 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marova, E.; Gromova, E.; Barsuk, P.; Shagushina, A. On the Effect of the Absorption Coefficient in a Differential Game of Pollution Control. Mathematics 2020, 8, 961. https://doi.org/10.3390/math8060961
Marova E, Gromova E, Barsuk P, Shagushina A. On the Effect of the Absorption Coefficient in a Differential Game of Pollution Control. Mathematics. 2020; 8(6):961. https://doi.org/10.3390/math8060961
Chicago/Turabian StyleMarova, Ekaterina, Ekaterina Gromova, Polina Barsuk, and Anastasia Shagushina. 2020. "On the Effect of the Absorption Coefficient in a Differential Game of Pollution Control" Mathematics 8, no. 6: 961. https://doi.org/10.3390/math8060961
APA StyleMarova, E., Gromova, E., Barsuk, P., & Shagushina, A. (2020). On the Effect of the Absorption Coefficient in a Differential Game of Pollution Control. Mathematics, 8(6), 961. https://doi.org/10.3390/math8060961





