1. Introduction
The problem of price-dependent consumption in electric power markets has recently acquired particular relevance associated with stimulating the consumer to reduce their load during peak hours and to equalize it with respect to the daily average. These are the well-known demand side management (DSM) problems. Currently, control algorithms are developed to target not only the consumers who have a control reserve of electrical appliances but also the prosumers who either generate their own electricity or have energy storage facilities. In our work, attention will be focused on methods of adopting effective incentives for consumers to optimize their loads [
1].
It is known that the allocation of several possible price alternatives for electricity increases the social welfare obtained from the trade. This is a kind of “voluntary” price discrimination, which, as a whole, is beneficial to both society and its individual participants (for example, Ramsey prices [
2,
3]). At the same time, an increase in social welfare will only occur if consumers “agree” to choose different tariff schemes, formed taking into account the features of the expected load of the consumers. The goal of the present work is to offer efficient pricing schemes for the electricity market. To this end, we use the well-known principles of the mechanism design theory.
This is justified by several considerations. In interaction, there is the problem of incomplete information. Here, the electricity supplier cannot know in advance what price the consumer will prefer or what type they will allocate themselves to. The supplier has only assumptions or knows the probabilities of a particular consumer action; therefore, the problem is associated with the correct “identification” of types. The mechanism created should encourage consumers to use a strategy of truthful communication of their preferences through the choice of certain prices intended specifically for them.
The ideas of efficient pricing are not new [
4]. They were designed to achieve the highest efficiency in electric power systems and are based on cost minimization while taking into account emissions of CO
2 into the atmosphere [
5,
6]. Researchers noted that consumers change their behavior over time and become more actively involved in load optimization programs (demand side management, DSM) [
7], including via instruments for hedging high price risks [
8].
The development of modern pricing schemes in the retail electricity market is directly related to game theory [
9]. The new feature of our approach is that we apply the mechanism design theory to determine the individual tariffs of individual consumers or groups of consumers. This requires understanding which parts of the mechanism design theory can be used. Despite the fact that in the retail market consumers do not explicitly form bids and their interaction with the supplier cannot be referred to as an auction, they have an option to choose from several proposed pricing schemes. Thus, the research shifts toward defining an entire price range, so that consumers have incentives to reduce the load at the right time. Such problems require special methods, including those based on the principles of identifying different types of participants and creating optimal mechanisms.
Recently, increasing researchers are addressing demand management issues. Among other things, they are driven by smart grid development, which enables communication with the consumer on the go. The development of online pricing schemes is becoming relevant, which is reflected in a number of works with game-theoretic formulations [
10,
11,
12,
13,
14,
15,
16]. Currently, there are several approaches. A number of researchers determined the same price for all consumers, taking into account their strategic behavior [
10,
12,
16].
In this case, an equilibrium similar to the Nash equilibrium is formed. Prices are set with different levels of detail, taking into account the electrical equipment that consumers have [
12,
17]. Some authors [
11] proposed methods of forming incentive prices with elements of optimal contracts where consumers truthfully disclose their usefulness.
They also considered the formation of a single price based on the utility function, which is a combination of individual consumer preferences, and analyzed the behavior strategies of individual consumers as part of an aggregated group. In contrast to the works listed above, we propose the formation of an equilibrium that will be close to the maximum social welfare, i.e., in our case, part of the consumer surplus is not lost. It is important that the pricing mechanism that we offer targets each individual consumer.
In the practice of pricing in the retail electricity market, it is common to offer several types of prices at the same time, assuming that different consumers will choose different prices. However, real markets often have the so-called “adverse selection”, when different consumers choose the same tariff [
18] and do not participate in incentive programs aimed to optimize the load, such as TOU (time-of-use pricing) [
19]. Recently, this problem has attracted attention in connection with the development of communications with an active consumer.
For example, the authors in [
20] presented a pricing model (contract design) in the retail electricity market, where there are several types of consumers described by different utility functions. The authors modeled a cost function that did not have the property of decreasing returns to scale. All functions were continuous with respect to time, which greatly complicates the analysis; however, the results can be used as a good theoretical foundation for the further development of pricing mechanisms. Our article proposes a mechanism that solves the adverse selection problem using design mechanism methods. Moreover, this mechanism can be easily implemented into pricing practices.
In [
21], the authors discuss optimal contracts in the electricity market under asymmetric information with detailed customer types and examine different possible outcomes for suppliers with different appetites for risk. As in our work, the distortion of equilibrium was shown in the case of asymmetric awareness of market participants about each other.
One of the tasks to be solved in this paper is the representation of the mechanism design problem in the retail electricity market in the form of an optimization problem with a set of constraints describing the specifics of the consumer choice of a certain tariff. In many works, even if the mechanisms or rules of the retail electricity market pricing implement a separating equilibrium, it remains the Nash equilibrium [
10,
22], which is far from maximum welfare.
Many research works ignore the fact that pricing may help achieve the maximum welfare and stability of the resulting equilibrium when the consumer chooses any of the tariffs offered by the supplier. In contrast to [
16], we build a mechanism with an additional motivational condition—Incentive Compatibility, ensuring a stable equilibrium—while the resulting equilibrium will provide the maximum welfare, and all participants will have no incentive to leave.
Our approach combines techniques for creating a convex optimization mechanism. The interaction mechanism we have created will be considered feasible if consumers voluntarily choose incentive tariffs and manage their consumption according to them.
This paper is organized as follows.
Section 2 and
Section 3 present a model that implements the optimal pricing mechanism in the retail market. First, in
Section 2, we formulate the base problem. Then, we describe the properties of the functions of consumers and suppliers of electricity, as well as the properties of the distribution of consumer types. Then, we provide possible pricing mechanisms and prove the imperfection (instability) of the Incentive Rationality mechanism, which implements the state of maximum social welfare.
Next, in
Section 3, the optimal mechanism is formulated in a situation of information asymmetry, and a solution that delivers the separating equilibrium is found. The important result of this part is the proof that the proposed optimal mechanism can be applied if we consider the interaction of players simultaneously in several periods. Moreover, in different periods, the ratio of types may change. In
Section 4, we build an algorithm that implements the optimal mechanism design. In
Section 5, this algorithm is applied to an electrical power system that consists of two, three, or more consumers. We compare the effect of different pricing schemes and demonstrate the optimality of the proposed algorithm.
4. Optimal Pricing Algorithm
4.1. The Problem of Using the Nash Equilibrium Prices and the Maximum
Social Welfare
The optimal mechanism developed above is based on the dominant strategies of the participants with additional restrictions on participation. As a result, a separating equilibrium is achieved. However, will the proposed the mechanism be better than the well-known Nash equilibrium or the search for the maximum social welfare [
13]? In this case, not only is the resulting solution important but also the timing of the pricing. Consumers are offered a list of tariffs from which they choose the one that suits them best in taking into account the expected load.
Let us briefly consider the results that can be obtained under these conditions during the formation of the Nash equilibrium and the maximum of social welfare. Then, we will use an example to compare the obtained outcomes with the solution delivered by the optical mechanism.
1. Nash Equilibrium. The model forms the Nash equilibrium under conditions of complete information, and the mechanism is applied in dominant strategies. The problem that each consumer
will solve has the form
Without a loss of generality, assume that
, where
is a price per unit of electricity offered to the consumer
in the period
. Then, in the equilibrium point, we have that, at the equilibrium point, the consumer has the FOC of maximizing their utility satisfied:
(The maximum will be unique since the utility function
is concave.). To find the Nash equilibrium, we must solve the following problem:
The created equilibrium provides different prices for consumers maximizing their utility and ensuring the supplier’s profit. In the equilibrium point, the supplies satisfy
where
is a characteristic of the utility and demand function, which affects the distortion of the resulting solution with respect to the maximum social welfare. On the other hand, the problem (
38) can be formulated through the definition of the Nash equilibrium, where the winning strategy for the consumer
at the moment
is the strategy of choosing such
that
This corresponds to the fulfillment of the Incentive Compatibility condition in the mechanism (
9). Despite the fact that we form a separating equilibrium in which no one is interested in leaving, this equilibrium provides a solution that is far from the socially optimal one. Later, we will illustrate this by an example.
2. The model for maximizing social welfare.
where
is the revenue from each consumer
in the period
. The solution is pricing in accordance with the FOC of the problem (
41):
In the context of imperfect information, it turns out to be advantageous for consumers with a higher utility to choose a contract where the prices will correspond to the lower type utility, since, in this case, when (
42) is fulfilled, they will be able to choose larger supplies than within their own contract. Therefore, the mechanism of Incentive Rationality or Max Welfare is not applicable in practice because it does not provide a separating equilibrium (Proposition 1) and, therefore, a social maximum.
The next paragraph describes the algorithm for applying the optimal mechanism, which will be implemented by each consumer to choose their contract while the maximum social welfare is achieved.
4.2. The Step-By-Step Algorithm of Optimal Pricing
Step 1. The input data is the characteristics of the electric power system, which includes the load curves of all consumers incorporated into the power system, and the characteristics of the supplier’s costs. Based on the average consumption for each user, the characteristics of the utility functions (or elastic demand) in different time periods are restored.
Step 2. Determine the sequence of the consumer type levels starting with the lowest one
, n is the number of types. Solve the problem of finding the maximum social welfare (
41). Contracts designed for
are checked to determine if they match the corresponding types:
– for each consumer, the profitability of their contract and someone else’s is calculated;
– the type of consumers , for which any change of contract yields negative profitability , is defined as the lowest;
– the next level is the type that profits from other contracts (the contract for ) more than from their own . Other contracts turn out to be non-profitable , ;
– then, the process continues and consumers are ranked by the profit they obtain by choosing contracts of other types.
Step 3. Based on the sorted levels of consumer types, active restrictions are determined in accordance with Proposition 2: for the lowest type, the participation restriction (
20) will be active, and, for the rest, the consistency restrictions will be by type with respect to the contract of the previous consumer type (
21).
Step 4. Solve the optimization problem (
19)–(
22) and obtain the optimal contract.
The next section provides an example of the optimal pricing mechanism for the electric power system.
5. An Example of Using the Optimal Mechanism
5.1. Data. Initializing the Cost and Utility Functions
Step 1. We used the data on real loads of several consumers of different types. All consumers belong to the same category with a load below 670 kW and a low voltage level. Load curves, which are taken as a basis, represent an estimate of the mathematical expectation for the consumption per month (December 2109) for several different consumers. These loads were recorded under a Flat tariff (constant prices during the day) that was used to restore the supplier’s cost function. All prices are given in Russian rubles and correspond to Russian prices as of December 2019. For the sake of generality, we may assume that these are conventional units. Several types of consumers are analyzed:
a dormitory with a load schedule similar to that of ordinary households (Consumer one),
a small business that only operates during the day (Consumer 2), and
several households with a low load (Consumer 3).
The total load changes insignificantly. The experiment will focus on redistributing the shares of different types of consumers, as well as on increasing the number of individual households within the same (approximate) total consumption.
Figure 3 and
Figure 4,
Table A1 in
Appendix A show the initial average loads of Consumer 1 (
) and Consumer 2 (
).
The supplier’s costs are defined as quadratic . They have the same characteristics in all periods . If necessary, these coefficients can be varied depending on time. For the current example, , .
The first stage implies initialization of the consumer utility functions based on real consumption for a Flat tariff. Electricity demand is traditionally described as linear functions with low elasticity.
This function satisfies the properties (
3)–(
5):
. More precisely,
Figure 2 shows indifference curves of the utility functions for consumers of different types in a certain period of time. They satisfy the Spence–Mirrlees single crossing conditions (
4) and (
5). Having the initial hourly load and pricing data and assuming that the consumer
maximizes their income
under these conditions (flat tariff, which, in this case, was
rubles), we can restore the main characteristics of the utility function (
44). For Consumers 1 and 2,
,
,
vary. The results of evaluating the characteristics of utility for some periods are presented in
Table 1. These characteristics are recalculated every time the composition of consumers changes, since the price depending on the total volume of consumption also changes.
5.2. Comparison of Different Pricing Schemes
Step 2. In this part, we will compare pricing schemes based on the Nash equilibrium principle and the maximization of social welfare (Incentive Rationality mechanism), taking into account imperfect information (optimal mechanism).
Let us solve two problems for the data presented in
Section 3.1:
finding the Nash equilibrium (
38) and (
39),
social welfare maximization (
41). Solving this problem corresponds to the application of the mechanism with Incentive Rationality and a solution to the problem (
13)–(
15).
Step 3. Based on the Incentive Rationality mechanism, we define the higher type of consumer and then form and solve (Step 4):
problem (
19)–(
22) implementing the optical mechanism.
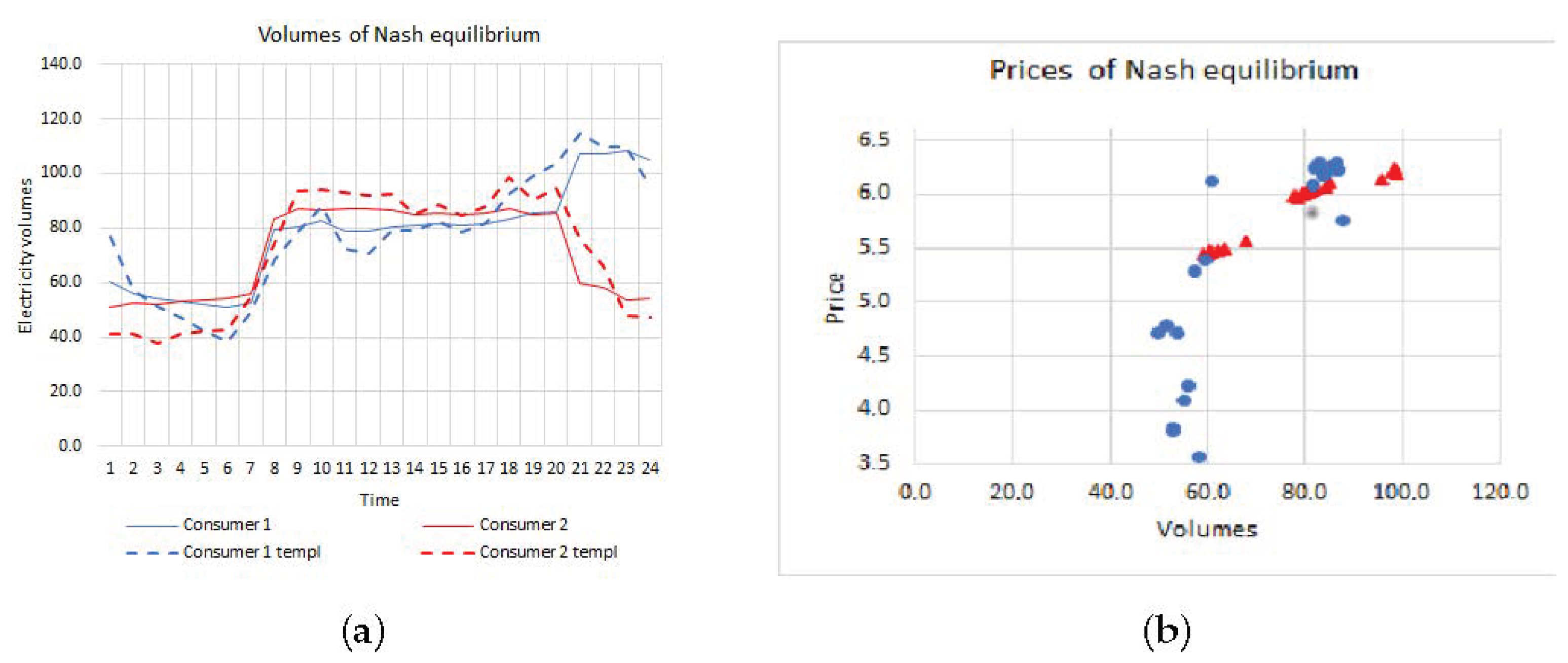
All pricing schemes that were formed as a result of solving these problems stimulate a reduction of the load during peak hours and align the schedule with respect to the average. The loads adjusted with respect to the initial state (
Figure 3) are shown in
Figure 5a,
Figure 6a and
Figure 7a, whereas the prices are given in
Figure 5b,
Figure 6b and
Figure 7b. The general characteristics of the results obtained can be seen in
Table 2. All results are given in rubles. Profits and the consumer surplus are calculated by month. For comparison, the
Table 2 in the first column shows the results for a Flat rate.
The pricing in all models is done in accordance with the incentive principle: the higher the consumption, the higher the price. This corresponds to the costs that grow with increasing consumption.
Here,
, and each consumer
chooses their contract
,
.
is the revenue of the consumer
due to selecting the contract
of the consumer
. The calculation results in the “Nash” and “Max Welfare” columns are carried out under the conditions of complete information, where the contract of the consumer
is used to calculate the prices of the contract of the consumer
only in terms of changes in the total marginal costs. After calculating the prices according to the “Nash” and “Max Welfare” rules (
Table 2), the consumers are ranked in accordance with Step 2:
- –
using the model of “Max Welfare”, compare the profit of the consumer ensured by their own contract (the profit is 922 rub.) with the profit delivered by choosing the contract of the consumer (the profit is 1163 rub.). Therefore, the strategy of the consumer is to choose someone else’s contract. The pricing in the “Nash” model does not give an incentive to switch to someone else’s contract, however, the consumer payoff in the “Nash” model is smaller than that in the model of “Max Welfare”;
- –
similarly, consider actions of the consumer in the “Max Welfare” model. If chooses their own contract, it yields the profit of 981 rub.. However, if chooses the contract of , the profit is 731 rub.. Therefore, the strategy of is to choose their own contract. The “Nash” model delivers the same result.
In the case considered above, the consumer
is of higher type and has the Incentive Compatibility as an active constraint, while the consumer
is constrained by the Incentive Rationality (
20) (Step 3) (Here, we do not provide the details of ranking consumers for each of the periods
. We carry this out when solving problems, but only give its aggregated version here.). Now, the optimization problem for creating the optimal mechanism is formulated and its solution is presented in the column “Opt mechanism”.
Analysis of the results.
1. The most effective pricing mechanism is the one that maximizes social welfare (line ). Here, , and it is assumed that each consumer chooses their own contract.
2. The Nash equilibrium delivers the largest profit to the supplier. The maximum social welfare pricing reduces profits, partially redistributing the surplus of the supplier in favor of the consumers. This effect is further enhanced when prices are set according to the optimal mechanism (line ).
3. The “Nash” mechanism implements an equilibrium that is stable in terms of the incentive to choose one’s contract, since the basic principle of its formation is the Incentive Compatibility condition. On the other hand, the resulting equilibrium differs significantly from the social maximum, especially in the case of a low elasticity of demand. (As is well-known, the demand for electricity has a low elasticity, which is defined in our model through a high marginal utility. As a result, the Nash equilibrium prices appear to be higher than the marginal cost by a significant amount due to the parameter .) This is what makes the “Nash” pricing model faulty.
4. The “Max Welfare” pricing model is not feasible in practice. The contracts it designs do not satisfy all consumers. Only the low type will choose their contract. It will be beneficial for a higher type to adhere to the contract of another consumer.
5. The optimal mechanism “Opt Mechanism” forms stable contracts in the sense that the consumer chooses “their own” contract.
Table 2 shows that the profit ensured by choosing their own contract (truthfully declaring their own type) is higher than when choosing someone else’s contract (for
and similarly for
).
6. Due to the asymmetry of information in the optimal mechanism, social welfare is partially lost in comparison with the “Max Welfare”. Pricing is efficiently done for the higher type consumers, and the lower type loses some of their profit in their favor. This is clearly seen from the last two rows of
Table 2. Here, we have the indicators of the mismatch between marginal costs and marginal utilities. For “Max Welfare”, the marginal utility
is equal to the marginal cost
. This does not happen in the optimal mechanism.
7. The optimal mechanism is as close as possible to the solution that delivers the maximum social welfare.
5.3. Features of Optimal Contracts for Various Configurations of the Electric
Power Systems
This part discusses several examples of different consumer compositions. In the first paragraph, there are two consumers, but of different sizes. This is different from the previous example, where the total load was approximately equal throughout the day. The second case considers three consumers, each assigned to their contract. The third case focuses on the situation with many small consumers.
5.3.1. Two Consumers of Different Sizes
We consider consumers with the same load configurations as before. The difference is that Consumer 1 is now larger and accounts for about 60% of the aggregated load, and Consumer 2 is, respectively, smaller.
Figure 8 shows the load graphs optimized by the optimal mechanism.
Figure 9 demonstrates the effect that such pricing has on the system as a whole. It also shows the aggregated load before and after applying incentive pricing. For the given example, the scatter was calculated with respect to the day average. The use of incentive prices for several consumers at the same time can reduce the the scatter around the average up to 32% for the given conditions.
Next, we present the results of numerical modeling of the equilibrium parameters when Consumer 1 (higher type) shifts from 0.5 to 0.9.
Figure 10 and
Figure 11 show the changes in the supplier’s profit, social welfare, consumer surplus for Consumers 1 and 2. A complete table of values is given in
Appendix A Table A2.
The optimal mechanism creates a separating equilibrium with incentives to reduce the load. With an increase in the share of higher-type consumers in the power system, the following occurs:
2. The supplier’s profit falls (
Figure 10, green line);
3. Growth of the overall welfare (
Figure 10, red line);
4. Consumer surplus among higher-type consumers grows (
Figure 11, green solid line) partly due to a decrease in consumer surplus of lower-type consumers (
Figure 11, red solid line) and partly due to the supplier’s profits.
5.3.2. Assigning Contracts to Three Different Consumers of the Power System
We considered a system that has three consumers of different supply. The graph of Consumer 3 represents a typical household load. Using the algorithm given in
Section 2.2, we determined the optimal loads and prices for each of the consumers.
Figure 12 and
Figure 13 show the main characteristics of the equilibrium obtained by the two models in comparison with the initial Flat tariff.
Table 3 summarizes the general equilibrium characteristics for the optimal mechanism. Price 1 denotes the prices offered to Consumer 1. It can be seen that consumers choose their own prices as the consumer surplus is maximal. For comparison,
Table 4 shows the results of calculations according to the welfare maximum model, where we have a mixing equilibrium, since it is profitable for Consumers 1 and 2 to switch to the contract of Consumer 3. In addition, if the prices of Consumer 3 are unavailable, Consumer 1 chooses the contract of Consumer 2.
By reacting to prices, electricity users regulate their load. For the given conditions, price-dependent optimization of the load by consumers decreases the variation in the electricity consumption with respect to the daily average by 16%. This is less than for two consumers, and is associated with the characteristics of the load of individual users.
The examples given above demonstrated the effectiveness of the proposed mechanism with two or three types of consumers. If we introduce more prices in the market, this will cause confusion for consumers and, therefore, such a situation is not considered here. At the same time, if the promising pricing schemes embedded in smart grid systems target individual consumers, then the proposed approach may also be relevant.
In our study, testing was carried out with a large number of users and two pricing schemes. We considered from 10 to 60 consumers that were divided into two types. We obtained regularities similar to
Section 5.3.1. The higher the type of consumers, the larger the supplier’s profit and the consumer surplus. The proposed optimal mechanism also proved to be effective, confirming possible scaling to any number of participants.
6. Conclusions
Electricity markets are actively regulated by the state as power supply systems are critical infrastructures for the economy and life. Therefore, price regulation methods are aimed at maximizing social welfare. This paper discusses the pricing method driven by welfare maximization models and reveals its inconsistency. We demonstrated that, in this case, there was a mixed equilibrium where all consumers tended to choose the same prices. As a result, the maximum social welfare was not achieved and the incentives to optimize the consumer’s load were reduced.
We propose an optimal mechanism based on the fulfillment of the Incentive Rationality and Incentive Compatibility conditions. We used this mechanism to set prices, and, as a result, we obtained a separating equilibrium, when each consumer was inclined to choose their own prices. The solution obtained was close to the maximum welfare. This also enabled optimization of the load schedule of the electric power system, which leads to more effective functioning (the scatter is reduced with respect to the daily average, and pronounced peaks are smoothed out). This is what constitutes the novelty and relevance of our study, which is in contrast to the available publications that propose to determine tariffs in accordance with the Nash equilibrium, as a result of which, a significant part of the social welfare is lost.
To formalize the model, a number of statements were proven. One of the key statements is the proposition that it is possible to use the Incentive Compatibility condition in certain periods to build a pricing mechanism for several periods. This will ensure the fulfillment of the Incentive Compatibility condition, which is crucial for the optimal mechanism during the entire time interval considered. The proposed mechanism will also work in a situation where, in one period of time, the first consumer receives a utility from a unit of electricity that is higher than the second consumer, and, in another period, they change roles. Then, the consumer types are not transitive with respect to each other over time.
The mechanism was demonstrated on various configurations of a multi-consumer power system. We compared pricing schemes according to the Nash, the maximum social welfare, and our mechanism. We demonstrated the effectiveness of the “Opt Mechanism” when compared to other schemes.
The use of smart meters enables the regulation of prices and consumption on the go. Electricity supply companies can use the proposed pricing mechanism in real-life problems. The mechanism is quite straightforward for implementation. and it will work successfully when planning for a day that is a week in advance, through providing incentives for the consumer to correctly disclose their types and optimize the load, which will ensure the effectiveness of the pricing scheme for the entire power system.
To develop the current task, we propose to study the issues of dividing a large number of consumers into several consistent groups for the optimal formation of the load schedule. In this study, this issue was resolved for individual consumers; the transition to a group is associated with additional difficulties in the formation of an aggregated utility function that will not violate the incentives for individual players to be in it.