To support correct interpretation of the data gathered by questionnaires (nominal measurements) the goodness of fit chi-square test (Pearson’s) was used to prove that the differences between the answers within the individual questions are significant. The expected observation counts are values of the same size (the probability is distributed equally). Thus we expect the observed frequencies statistically equal to expected frequencies to accept the null hypotheses for each test. The results of the survey are presented by the graphs (pie-charts) in the section “Questionnaire Data Presentation”. To provide the proof that the samples of the achievement test results in both groups were selected from the same complete sample, the Kolmogorov-Smirnov’s test was used. Even if it is more powerful for ordinal samples, we can carefully use it for our discrete values as well with the reliability limitation that it brings (, for the nearest tabulated critical value , where the values are tabulated values for and groups). From the results can be judged that both groups were acquired from the same complete sample.
The next step utilized Fisher–Snedecor’s F-test to discover whether there is no statistically significant difference between the data variance in both groups ( or the nearest tabulated , counted ). It was calculated that F is lower than F critical. It can be expressed that there is no significant difference of data variance inside of both groups; therefore analysis of variance can be performed as the next step. Fisher’s analysis of variance (ANOVA) was used to evaluate data for the achievement test results. This test will help to judge whether there is a significant difference between the results in the achievement tests of experimental and control group, especially:
6.1. Questionnaire Data Presentation
Every hypothesis for the affective area is verified based on data acquired from the questioner using Pearson’s goodness of fit chi-squared test and the following formula:
where
O is observed count,
E is expected count (asserted by null hypothesis). The significance level is 0.05 as already stated. Counted
will be compared to the critical value using an appropriate degrees of freedom (depends on the response categories). If the counted
is greater than the critical value we will reject the null hypothesis and accept the alternative hypothesis, otherwise we will not refuse the null hypotheses. The critical values will be presented in the form of:
. For example:
for significance level 0.05 and 1 level of freedom for two answer categories in the question.
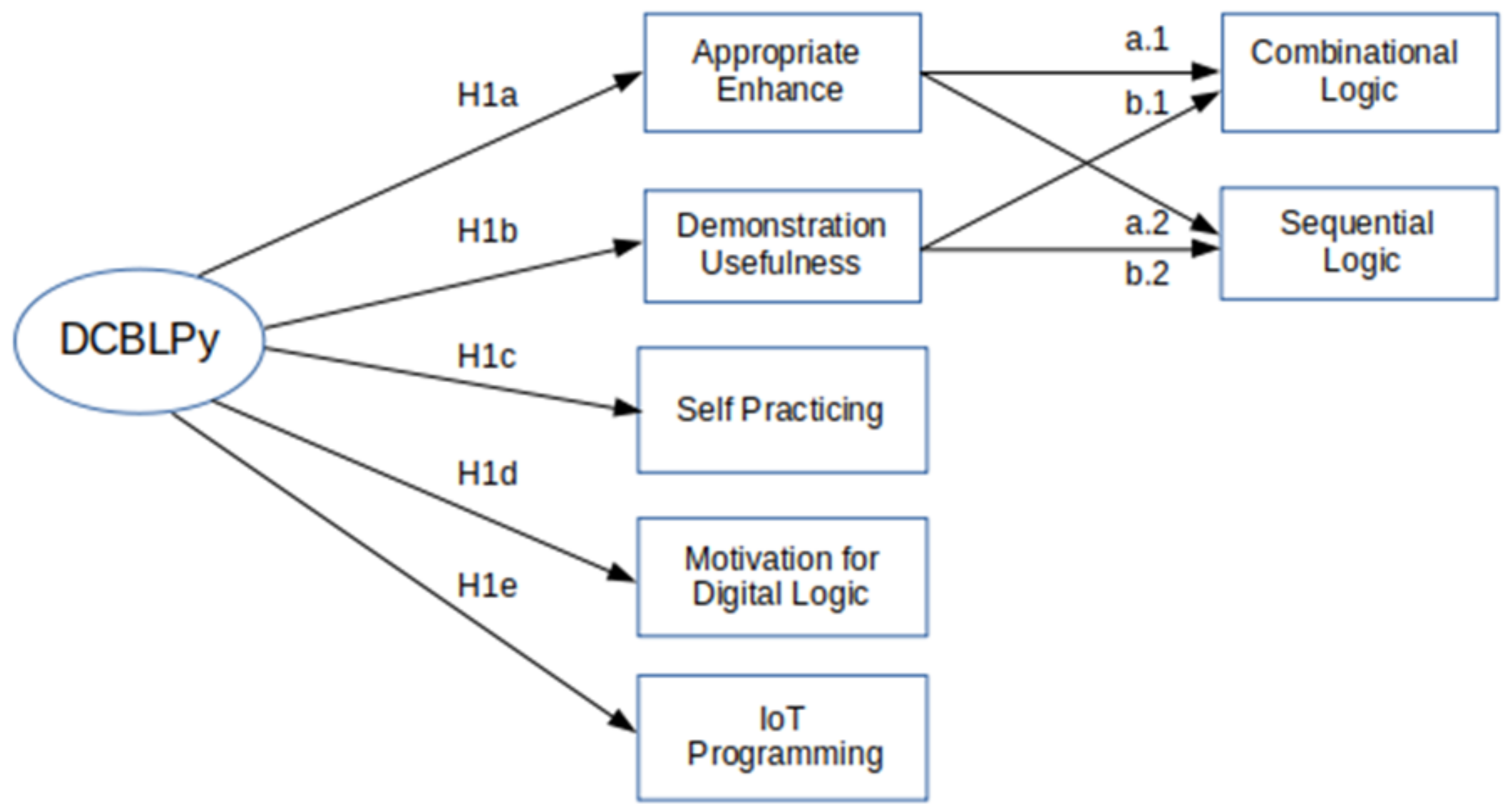
The graphs and statistical results are available to support the decision regarding each of the hypotheses. We start with the factual hypothesis H1a area (zero hypothesis H0 and alternative HA) and continue with H1b (zero, alternative), and so forth.
For the Area of Interest H1a—Appropriate Enhancement
We need to judge if the discourse is appropriately enhancing the digital logic education. There are three questions in the questionnaire related to this area of interest (
Table 1) that must be evaluated one by one before we judge.
- (A)
The scope of DCBLP integration into digital logic.
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no significant difference in responses sensing DCBLP.
Hypothesis A (HA). There is a significant difference in responses sensing DCBLP.
The questionnaire responses for answering the hypothesis are gathered in the
Table 4.
From the
Table 4 (and leftmost graph in
Figure 9 is obvious that there is a significant difference in sensing DCBLP (
). Thus
is rejected and alternative hypothesis
for the area of interest (H1a) can be accepted. It is possible to express that the positive attitude is observed.
Next, the differences between the learning areas of combinational and sequential logic are investigated. The results are visualized by the middle and right graphs in
Figure 9. It is now necessary to answer the question: “where is the significant positive impact (meaning the area of the training) when positive (question H1a-A) attitude has been expressed by the students?”
- (B)
Description of combinational logic by DCBLP
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no significant difference in responses regarding usefulness of DCBLP in relation to combinational logic programming.
Hypothesis A (HA). There is a significant difference in responses regarding usefulness of DCBLP in relation to combinational logic programming.
Calculation of the results are based on the same approach as for the question H1a-A. The frequencies of responses are: quite useful = 16, very useful = 4, rather not useful = 4. For the comparison calculated it is possible to conclude there is a significant difference between the individual answers thus rejecting and accepting . Since there is a difference, the comparison can continue. For the combinational logic can be expressed that the students evaluate it as “quite useful”. It is significantly more than expressing it as a “very useful” (). There is no student that evaluates it as a “totally bad”.
- (C)
Description of sequential logic by DCBLP
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no significant difference in responses regarding usefulness of DCBLP in relation to sequential logic programming.
Hypothesis A (HA). There is a significant difference in responses regarding usefulness of DCBLP in relation to sequential logic programming.
The frequencies of responses are: very useful = 1, quite useful = 16, rather not useful = 1, totally bad = 1. For the sequential logic the same amount of the students (the same students) as described in H1a-B expressed that DCBLP is “quite useful”. However, there is significantly less amount of the students that marked DCBLP as a “very useful” compared to combinational logic area (). There are also more students that stated DCBLP for sequential logic area as “rather not useful” compared to combinational logic. Thought the difference between these two statements (quite useful vs. rather not useful) is still statistically significant (. For the case of simplification (aggregating positive attitude, aggregating negative attitude) the difference is still significant (). We also compared the overall responses with the result , . Alternative hypothesis (with countable 5% error probability) can be accepted. Additionally, it is possible to express that in both of the areas (combinational and sequential logic) the discourse is statistically sensed by the students as “quite useful”.
Based on the counts of the responses for the questions A, B and the comparison of expected observation the whole area H1a can be expressed that a new discourse is sensed by the experimental group as appropriately enhancing the digital logic education.
For the Area of Interest H1b—Demonstration Usefulness
We need to judge whether the discourse is positively sensed during the demonstration of logic. There are two questions in the questionnaire related to this area of interest (
Table 1) that must be evaluated one by one before we judge. The null hypothesis can be verified using two questions in the survey (
Figure 10). The first one is direct: “In which (if any) area the demonstration of digital logic supported by DCBLP helped with the study?” The second is indirect: “What was the most useful aspect noticed during the training?”
- (A)
Demonstration of the digital logic supported by DCBLP
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no significant difference in responses regarding DCBLP demonstration usefulness.
Hypothesis A (HA). There is a significant difference in responses regarding DCBLP demonstration usefulness.
The frequencies of responses are: yes—for combinational logic = 4, yes—for sequential logic = 4, yes—both types = 16, did not help = 0. There is no need to utilize chi-square test to interpret the data gained for the first question as the difference between the amounts of answers is obvious (
Figure 10-left), however the calculation is as follows:
,
. Accepting the HA: there is a significant difference in responses regarding DCBLP demonstration usefulness. Based on the second question (
Figure 10-right) an additional hypothesis (B) can be expressed.
- (B)
What was the most helpful during the training
Hypothesis 0 (H0). There is no significant difference between demonstration based on DCBLP and other helpful aspects.
Hypothesis A (HA). There is a significant difference between demonstration based on DCBLP and other helpful aspects.
The students could select multiple answers, therefore the frequencies of responses are higher. The frequencies of responses are: nothing = 1, demonstration based on DCBLP = 10, own notes = 6, whiteboard writings = 19. Also for the question the chi-square has been calculated (). Although the answers are significantly different, after additional analysis comparing individual answers one to each other it cannot be confirmed that DCBLP acts as the most significant helpful aspect in the training. Null hypothesis () cannot be rejected in this case.
For the H1b area it is possible to conclude that the declared usefulness is mostly sensed under both areas of learning content, and in overall significantly more than declared unhelpfulness, however there is no significant difference between declared aspects that can help the most. Demonstration is not sensed as the most important aspect during the training.
For the Area of Interest H1c—Self Practicing
We need to judge if the discourse is positively sensed during the practicing or for practicing the logic. There are two questions in the questionnaire related to this area of interest (
Table 1) that must be evaluated one by one before we judge.
- (A)
Do you like to include practicing DCBLP in ICT labs?
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no declared (sensed) need for practicing DCBLP during the training.
Hypothesis A (HA). There is declared (sensed) need for practicing DCBLP during the training.
The responses are as follows: yes—I don’t have the possibility to train at home = 18, yes—need more practicing and feedback = 4, no—I doubt I can ever understand it = 1, no—it’s useless = 1. There is an obvious difference (
Figure 11-left) between the answers distribution (
). Based on data we can reject
and accept
: There exists students’ sensed need for practicing logic when using DCBLP.
- (B)
During the training I missed the most ...
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There are no declared differences between practicing needs.
Hypothesis A (HA). There are declared differences between practicing needs.
The responses for the answer are (multiple choices were allowed): learning materials = 18, more programming at school = 10, more tasks for home preparation = 7, more challenging programming tasks = 4, easier programming tasks = 9. Answers for missing aspects in training () show that there is a difference between preferences, however there is no significant difference between the needing of programming at home and programming at school () and also no significant difference between missing more challenging programming tasks and easier programming tasks ().
There is also no significant difference between the necessity of the learning materials and more tasks for programming (neither separated nor aggregated programming at school/home). However there is a strong needing for including practical programming into the training (aggregated for
Figure 11-left
), and has been revealed, there is also a strong need for learning materials that were not available during the period the research was in progress (as already mentioned in the section Training Content Enhancements in Details). Therefore null hypothesis is rejected and
is accepted.
For the H1c area it is possible to conclude that, based on the declared answers, there are significant differences in the answers in both subcategories. It has been investigated that there is a strong need to include practicing into the training and a strong need for the learning materials that were not available during the time of preliminary training.
For the Area of Interest H1d—Motivation for Digital Logic (Learning)
We need to judge if the discourse is sensed as motivating for the subject learning. There are two questions in the questionnaire related to this area of interest (
Table 1) that must be evaluated one by one before we judge.
- (A)
Including (inheriting) DCBLP into digital logic was for me ...
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no difference between answers regarding declared motivation.
Hypothesis A (HA). There is a difference between answers regarding declared motivation.
The count responses for the answer are: motivating for digital logic = 9, neutral = 10, demotivating = 3. There is a difference between answers when asking about motivation, but there is no significant difference between individual answers compared in overall (). There is no statistically measurable additional motivation aspect expressed by the students when compared the amount of answers in option “Motivating” to answers in option “Demotivating” (). Therefore cannot be rejected.
- (B)
Programming included in digital logic
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no difference between answers regarding declared popularity.
Hypothesis A (HA). There is a difference between answers regarding declared popularity.
The count responses for the answer are: dislike = 3, like = 16, neutral = 5. Although there is no strong motivation aspect measured, students do like the way of enhancing digital logic by proposed programming (
Figure 12-right). There is a significant difference (
) between the frequency of answers dislike/like/neutral. The popularity is the most frequent answer. The null hypothesis is rejected and the alternative hypothesis
is accepted.
For the area H1d is possible to express that there is no significant difference between declaration of motivation or demotivation. We cannot say that most of the students are motivated thanks to the discourse. However, it is possible to express that the students sensed the discourse as suitable and they like the inheritance into the training.
For the Area of Interest H1e—Iot Programming
We need to judge whether the discourse is sensed as motivating for the future programming on IoT devices. There is one question in the questionnaire related to this area of interest (
Table 1) that must be evaluated one by one before we judge.
- (A)
Motivation for the future programming on IoT
The statistical null hypothesis and alternative hypothesis are formulated:
Hypothesis 0 (H0). There is no difference between answers regarding declared motivation for future programming on IOT.
Hypothesis A (HA). There is a difference between answers regarding declared motivation for future programming on IOT.
The count responses for the answer are: very motivated = 4, slightly motivated = 14, slightly demotivated = 4, demotivated = 2. There is a significant difference () in distribution of the answers. When comparing only options “slightly motivated” and “very motivated”, the option “slightly motivated” must be selected as statistically significant ().The null hypothesis must be rejected and HA is accepted.
The students claim they are slightly motivated for future programming on IoT devices after completing the training although it cannot be assured that the only reason is a new discourse (
Figure 13).
There is an additional finding beside the verification of the proposed hypothesis. There ware more questions regarding the programming used in the educational process. It was not just about an impact of DCBLP on digital logic. It was about the students’ perception of the programming language support. It is clear from the graph below (
Figure 14) that there is no significant difference between the amounts of answers gathered for each option.
It partially corresponds to previous students’ claims regarding DCBLP included in digital logic. There are slight differences although they are not significant. The interesting matter can be revealed when joined with another question about the study program preferences. Of the respondents, 20% claimed they would prefer electronics study content instead of a focus on programming. Of these students, 80% also claimed that programming was not supportive for solving any tasks during the whole study program. Creating a contingency table and putting data in the test for these two areas (if there is a relation between preferences of study program and willingness to using the programming language) brought the result that there is a relation () between the study program preferences and willingness to use the programming language. It means that the students preferring electronics instead of programming also perceive the new discourse as not supportive. From this small sample cannot be concluded it is valid for whole population, however the students’ preferences regarding the study branch that they prefer should be taken into account.
6.2. Achievement Test Results Data Presentation
The achievement test provides data for the H2 related hypothesis. Part of the analysis is based on exploratory data analysis supported by box-plots and the decisions of significant differences are based on ANOVA. We compare the answers in the achievement tests in both groups (experimental/control). Training areas are discussed individually in details, supported by statistics and appropriate graphs:
First the overall performance comparison in the achievement test:
Hypothesis 0 (H0). There is no difference between students overall performance in the area of digital logic with and without a support of a new discourse.
Hypothesis A (HA). There is a difference between students overall performance in the area of digital logic with and without a support of a new discourse.
The calculation is based on summarized points (for all of the tasks) gained by each student (in the achievement test.). Based on that, average points for both groups have been calculated (
Figure 15-left). Standard deviance is also included in the graph. For better comparison of both groups the quartile deviance has been calculated as well (
Figure 15-right). The experimental group
(where
is median,
the first quartile,
is the third quartile), the lowest gained points equal 4.5 and the highest points gained equal 16. For the control group
, the lowest points gained equal 0, the highest points gained equal 14. The maximum points that could be gained by each student (for the whole test) is 16.
The points gained by each student are also used to calculate analysis of variance ANOVA (
Table 5)
, from which it is possible to accept alternative hypothesis
for the area of overall performance.
Additional investigation regarding hypothesis must be performed:
Hypothesis 2a0 (H2a0). The discourse has no measurable impact in the block of Boolean logic functions.
Hypothesis 2aA (H2aA). The discourse has a measurable impact in the block of Boolean logic functions.
Because of the different count of participants, the data cannot be directly compared by simple calculation and visualization of the amount (frequency) of correct answers. Therefore, this comparison (
Figure 16-left) is only illustrative. Better results comparison brings box-plots (quartile based graphs:
Figure 16-right). The experimental group
, the lowest gained points is 1.5 and the highest points gained is 7. For the control group
, the lowest points gained equal 0, the highest points gained = 7. Maximum possible points that could be gained by each students for this part = 7.
Analysis of variance brings the following results (
Table 6):
, rejecting H2a0 and accepting H2aA. There is a significant difference between the groups in the selected questions with the content of Boolean logic.
As next the null and alternative hypotheses are formulated for the combinational logic area:
Hypothesis 2b0 (H2b0). The discourse has no measurable impact in the block of combinational logic.
Hypothesis 2bA (H2bA). The discourse has a measurable impact in the block of combinational logic.
The experimental group
, the lowest gained points = 0 and the highest points gained = 2. For the control group
, the lowest points gained = 0, the highest points gained = 1. Maximum possible points that could be gained by each student for this part = 2 (
Figure 17).
Analysis of variance brings the following results (
Table 7):
, rejecting null hypothesis, accepting H2bA.
Next, the null hypotheses are formulated for the sequential logic area:
Hypothesis 2c0 (H2c0). The discourse has no measurable impact in the block of sequential logic.
Hypothesis 2cA (H2cA). The discourse has a measurable impact in the block of sequential logic.
The experimental group
, the lowest gained points is 0 and the highest points gained is 2. For the control group
, the lowest points gained equal 0, the highest points gained = 1. Maximum possible points that could be gained by each students for this part = 2 (
Figure 18).
Analysis of variance brings the following results (
Table 8):
, rejecting null hypothesis and accepting H2cA.
Next the null and alternative hypotheses are formulated for the programming area:
Hypothesis 2d0 (H2d0). It has no measurable impact in the programming language usage across the logic blocks.
Hypothesis 2dA (H2dA). It has the measurable impact in the programming language usage across the logic blocks.
All tasks/questions require the usage of a programming language. There was no necessity to use DCBLP in the answers for the question as the control group students have had no training in this new programming discourse. Although both groups went through previous training in the programming language (see
Figure 1 stage 1). Every piece of code using any programming language and leading to a task’s solution was acceptable. The graph on the left side of
Figure 19 shows the frequency (sum amount) of acceptable solutions per task, on the right are the quartile graphs for groups’ comparison. The experimental group
, the lowest gained points = 0 and the highest points gained = 5. For the control group
, the lowest points gained equal 0, the highest points gained = 4. Maximum possible points that could be gained by each students for this part = 5. Graphs for this part are available in
Figure 19.
Analysis of variance brings the following results (
Table 9):
, rejecting null hypothesis, accepting H2dA.
It is also possible to express that it has no impact on the previous hypotheses’ acceptance in certain areas.
The task no. 4 into Boolean logic tasks and functions: , still accepting H2aA;
The tasks no. 7, 8c, 9 into combinational logic tasks: , still accepting H2bA;
The task no. 12 into sequential tasks: , still accepting H2cA.
In all of the measured areas the achievements of the students in the experimental group were significantly better.