Analysis of the Use of a Wind Turbine as an Energy Recovery Device in Transport Systems
Abstract
:1. Introduction
2. Materials and Methods
2.1. First Driving Scenario
- Low velocity (589 s–9.18 min); maximum velocity (Vmax) of 56.5 km/h
- Medium velocity (433 s–7.22 min); Vmax of 76.6 km/h
- High velocity (455 s–7.58 min); Vmax of 97.4 km/h
- Extra-high velocity (323 s–5.38 min); Vmax of 131.3 km/h
2.2. Second Driving Scenario
2.3. Third Driving Scenario
2.4. Driving Modes and Forces Acting on the Vehicle
3. Results: Analysis of Power Consumed and Power Recovered
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Paris Agreement. 2015. Available online: https://ec.europa.eu/clima/policies/international/negotiations/paris_en (accessed on 15 December 2020).
- 2030 Climate and Energy Framework. Available online: https://ec.europa.eu/clima/policies/strategies/2030_en (accessed on 15 December 2020).
- Las Reservas de Petróleo en el Mundo. 2019. Available online: https://www.rinconeducativo.org/es/recursos-educativos/las-reservas-de-petroleo-en-el-mundo (accessed on 3 February 2021).
- European Environment Agency. 2019. Available online: https://www.eea.europa.eu/es/themes/transport (accessed on 20 January 2021).
- European Commission, Energy, Climate Change, Environment, Climate Action, EU Action, Climate Strategies and Targets, Long-Term Strategy for 2050. Available online: https://ec.europa.eu/clima/policies/strategies/2050_es#tab-0-1 (accessed on 20 January 2021).
- Commission on Science and Technology for Development, Economic and Social Council, United Nations. 2018. Available online: https://unctad.org/meetings/es/SessionalDocuments/ecn162018d2_es.pdf (accessed on 1 February 2021).
- Renovables: La respuesta está en el viento, BBVA Research. 2017. Available online: https://www.bbvaresearch.com/publicaciones/renovables-la-respuesta-esta-en-el-viento/ (accessed on 3 February 2021).
- Global Energy and CO2 Status Report: Renewables. International Energy Agency. 2019. Available online: https://www.iea.org (accessed on 3 February 2021).
- Mirzaei, P.A.; Rad, M. Toward design and fabrication of wind-driven vehicles: Procedure to optimize the threshold of driving forces. Appl. Math. Model. 2013, 37, 50–61. [Google Scholar] [CrossRef] [Green Version]
- Drela, M. Dead-Downwind Faster than the Wind (DFTTW) Analysis. Available online: https://www.lockhaven.edu/~dsimanek/museum/ddwfttw.htm (accessed on 3 February 2021).
- Gaunaa, M.; Øye, S.; Mikkelsen, R.F. Theory and Design of Flow Driven Vehicles Using Rotors for Energy Conversion. In Proceedings of the 2009 European Wind Energy Conference and Exhibition, Marseille, France, 16–19 March 2009. [Google Scholar]
- Khan, S.A.; Sufiyan, S.A.; George, J.T.; Ahmed, N. Analysis of Down-Wind Propeller Vehicle. Int. J. Sci. Res. Publ. 2013, 3, 1–4. [Google Scholar]
- Wind-Powered ‘Ventomobile’ Places First in Race; University of Stuttgart: Stuttgart, Germany, 2008; Available online: https://www.sciencedaily.com/ (accessed on 3 February 2021).
- Barry, K. For Sale: Record-Breaking Downwind Cart. Low Miles, Newer Propeller. 2013. Available online: https://www.wired.com/2013/06/downwind-cart-for-sale/ (accessed on 12 February 2021).
- Wind-Powered Car Breaks Record. BBC News. 27 March 2009. Available online: http://news.bbc.co.uk/2/hi/7968860.stm (accessed on 12 February 2021).
- Yuji, O.; Takashi, K. A Shrouded Wind Turbine Generating High Output Power with Wind-lens Technology. Energies 2010, 3, 634–649. [Google Scholar] [CrossRef] [Green Version]
- Hashfi, T.B.; Islam, M.M.; Shareef, H.; Khan, S.S. A Low Cost Method to Convert Automotive Alternator for Wind Electricity Generation. In Proceedings of the 2018 6th International Renewable and Sustainable Energy Conference (IRSEC); IEEE: Piscataway, NJ, USA, 2018; pp. 1–4. [Google Scholar] [CrossRef]
- Ventile. Available online: https://www.diariomotor.com/tecmovia/2011/07/10/ventile-un-concepto-amigo-del-viento/ (accessed on 12 February 2021).
- Lamborghini. Available online: https://forococheselectricos.com/2013/12/lamborghini-countach-electrico-y-eolica.html (accessed on 12 February 2021).
- Awal, M.R.; Jusoh, M.; Sakib, M.N.; Hossain, F.S.; Beson, M.R.C.; Aljunid, S.A. Design and implementation of vehicle mounted wind turbine. ARPN J. Eng. Appl. Sci. 2015, 10, 8699–8860. [Google Scholar]
- Diaz, J. Electrical Generator System for Capturing Wind Energy on a Moving Vehicle. U.S. Patent No. 8,618,683, 31 December 2013. [Google Scholar]
- Ripley, P.W. Wind Turbine for Electric Car. U.S. Patent No. 8,513,828, 20 August 2013. [Google Scholar]
- Yu, X. Portable Wind Power Apparatus for Electric Vehicles. U.S. Patent No. 6,897,575, 24 May 2005. [Google Scholar]
- Tesla T1. Available online: https://www.behance.net/gallery/41317559/TESLA-T1 (accessed on 12 February 2021).
- Chaudhary, Y.; Bangi, V.; Guduru, R.; Aung, K.; Reddy, G. Preliminary Investigation on Generation of Electricity Using Micro Wind Turbines Placed on A Car. Int. J. Renew. Energy Dev. 2017, 6, 75–81. [Google Scholar] [CrossRef] [Green Version]
- Tirapu-Manero, J.R. Generador eólico para vehículos. Patent U 201000802.
- Damron, P.C.; Damron, J.L.; Philip, C.D.; Jane, L. Propeller Wind Charging System for Electrical Vehicle. U.S. Patent No. 5,920,127, 6 July 1999. [Google Scholar]
- Fathabadi, H. Possibility of Utilizing Wind Turbine to Recover a Portion of the Kinetic Energy Losses of a Car. IEEE Trans. Veh. Technol. 2019, 68, 8663–8670. [Google Scholar] [CrossRef]
- Rubio, F.; Llopis-Albert, C. Viability of using wind turbines for electricity generation in electric vehicles. Multidiscip. J. Educ. Soc. Technol. Sci. 2019, 6, 115–126. [Google Scholar] [CrossRef]
| Phase | Low | Medium | High | Extra-High | Total |
|---|---|---|---|---|---|
| Duration (s) | 589 | 433 | 455 | 323 | 1800 |
| Stop duration (s) | 156 | 48 | 31 | 7 | 242 |
| Distance (m) | 3095 | 4756 | 7162 | 8254 | 23,267 |
| Percentage of stops (%) | 26.5 | 11.1 | 6.8 | 2.2 | |
| Maximum velocity (V_max) (km/h) | 56.5 | 76.6 | 97.4 | 131.3 | |
| Mean velocity without stops (V_max) (km/h) | |||||
| Mean velocity with stops (V_mean) (km/h) | |||||
| Minimum acceleration (A_min) (g) | −1.47 | −1.49 | −1.49 | −1.21 | |
| Maximun acceleration (A_max) (g) | 1.47 | 1.57 | 1.58 | 1.03 |
| Section | Time t (s) | Accumulated Time taccum(s) | Initial Velocity vi (km/h) | Final Velocity vf (km/h) | Power P (kW) |
|---|---|---|---|---|---|
| accel | 10 | 10 | 0 | 14 | 0.95850289 |
| 30% uphill gradient | 5 | 15 | 14 | 14 | 11.3855839 |
| v const | 10 | 25 | 14 | 14 | 0.44075916 |
| 30% uphill gradient | 10 | 35 | 14 | 14 | 11.3855839 |
| decel | 10 | 45 | 14 | 0 | −0.55384279 |
| stop | 30 | 75 | 0 | 0 | 0 |
| accel | 8 | 83 | 0 | 40 | 8.4046202 |
| v const | 25 | 108 | 40 | 40 | 1.96606653 |
| decel | 8 | 116 | 40 | 0 | −7.02747857 |
| stop | 29 | 145 | 0 | 0 | 0 |
| accel | 11 | 156 | 0 | 50 | 9.70328836 |
| v const | 25 | 181 | 50 | 50 | 2.96712213 |
| decel | 32 | 213 | 50 | 10 | −1.66810764 |
| accel | 32 | 245 | 10 | 50 | 4.1189294 |
| v const | 33 | 278 | 50 | 50 | 2.96712213 |
| decel | 10 | 288 | 50 | 0 | −8.71001131 |
| stop | 28 | 316 | 0 | 0 | 0 |
| accel | 21 | 337 | 0 | 30 | 2.13233817 |
| v const | 10 | 347 | 30 | 30 | 1.22541088 |
| decel | 28 | 375 | 30 | 0 | −0.76118035 |
| stop | 30 | 405 | 0 | 0 | 0 |
| accel | 14 | 419 | 0 | 20 | 1.40125475 |
| v const | 32 | 451 | 20 | 20 | 0.68857082 |
| decel | 16 | 467 | 20 | 0 | −0.6655442 |
| stop | 27 | 494 | 0 | 0 | 0 |
| accel | 12 | 506 | 0 | 17 | 1.17886267 |
| accel | 5 | 511 | 17 | 24 | 2.92595189 |
| accel | 7 | 518 | 24 | 50 | 12.3231589 |
| v const | 48 | 566 | 50 | 50 | 2.96712213 |
| decel | 11 | 577 | 50 | 0 | −7.83318751 |
| stop | 27 | 604 | 0 | 0 | 0 |
| accel | 6 | 610 | 0 | 17 | 2.10800362 |
| accel | 5 | 615 | 17 | 24 | 2.92595189 |
| accel | 7 | 622 | 24 | 50 | 12.3231589 |
| v const | 48 | 670 | 50 | 50 | 2.96712213 |
| decel | 11 | 681 | 50 | 0 | −7.83318751 |
| stop | 24 | 705 | 0 | 0 | 0 |
| accel | 14 | 719 | 0 | 50 | 7.93082554 |
| 5% uphill gradient | 19 | 738 | 50 | 50 | 9.76889127 |
| v const | 44 | 782 | 50 | 50 | 2.96712213 |
| 5% downhill gradient | 16 | 798 | 50 | 50 | −3.83910935 |
| decel | 4 | 802 | 50 | 40 | −6.28836806 |
| decel | 6 | 808 | 40 | 0 | −9.66762003 |
| accel | 7 | 815 | 0 | 14 | 1.28257697 |
| 30% uphill gradient | 5 | 820 | 14 | 14 | 11.3855839 |
| v const | 10 | 830 | 14 | 14 | 0.44075916 |
| 30% uphill gradient | 10 | 840 | 14 | 14 | 11.3855839 |
| decel | 10 | 850 | 14 | 0 | −0.55384279 |
| stop | 30 | 880 | 0 | 0 | 0 |
| accel | 11 | 891 | 0 | 50 | 9.70328836 |
| v const | 25 | 916 | 50 | 50 | 2.96712213 |
| decel | 32 | 948 | 50 | 20 | −0.96510347 |
| accel | 32 | 980 | 20 | 50 | 4.09855394 |
| v const | 33 | 1013 | 50 | 50 | 2.96712213 |
| stop | 30 | 1043 | 0 | 0 | 0 |
| accel | 11 | 1054 | 0 | 50 | 9.70328836 |
| v const | 25 | 1079 | 50 | 50 | 2.96712213 |
| decel | 32 | 1111 | 50 | 30 | 0.03705418 |
| accel | 32 | 1143 | 30 | 50 | 3.89507888 |
| v const | 33 | 1176 | 50 | 50 | 2.96712213 |
| decel | 4 | 1180 | 50 | 25 | −16.3257903 |
| decel | 8 | 1188 | 25 | 0 | −2.62842607 |
| stop | 27 | 1215 | 0 | 0 | 0 |
| Section | Time t (s) | Accumulated Time taccum (s) | Initial Velocity vi (km/h) | Final Velocity vf (km/h) | Power P (kW) |
|---|---|---|---|---|---|
| accel | 19 | 19 | 0 | 70 | 11.7250007 |
| accel | 17 | 36 | 70 | 120 | 34.1755318 |
| v const | 110 | 146 | 120 | 120 | 22.0187963 |
| 5% uphill gradient | 21 | 167 | 120 | 120 | 38.3412573 |
| v const | 600 | 767 | 120 | 120 | 22.0187963 |
| 5% downhill gradient | 17 | 784 | 120 | 120 | 5.68205581 |
| decel | 13 | 797 | 120 | 60 | 21.8040946 |
| accel | 8 | 805 | 60 | 100 | 39.2352298 |
| v const | 76 | 881 | 100 | 100 | 13.858852 |
| 5% uphill gradient | 20 | 901 | 100 | 20 | 6.47960103 |
| decel | 7 | 908 | 20 | 10 | 1.18731357 |
| accel | 19 | 927 | 10 | 60 | 8.82263626 |
| accel | 17 | 944 | 60 | 120 | 35.6766789 |
| v const | 400 | 1344 | 120 | 120 | 22.0187963 |
| 5% uphill gradient | 21 | 1365 | 120 | 120 | 38.3412573 |
| v const | 600 | 1965 | 120 | 120 | 22.0187963 |
| decel | 7 | 1972 | 120 | 90 | 19.3342737 |
| v const | 200 | 2172 | 90 | 90 | 10.7070313 |
| decel | 7 | 2179 | 90 | 60 | 18.0128431 |
| v const | 50 | 2229 | 60 | 60 | 4.28516204 |
| accel | 19 | 2248 | 60 | 110 | 26.9599013 |
| accel | 19 | 2267 | 110 | 120 | 24.4972599 |
| v const | 150 | 2417 | 120 | 120 | 22.0187963 |
| 5% downhill gradient | 30 | 2447 | 120 | 100 | −3.210079 |
| v const | 40 | 2487 | 100 | 100 | 13.858852 |
| 5% uphill gradient | 21 | 2508 | 100 | 120 | 40.8436589 |
| v const | 3600 | 6108 | 120 | 120 | 22.0187963 |
| decel | 7 | 6115 | 120 | 100 | 6.82720575 |
| decel | 20 | 6135 | 100 | 50 | 7.80658275 |
| decel | 9 | 6144 | 50 | 0 | 9.88813014 |
| Case 1: Acceleration on Flat |
 |
| Case 2: Deceleration on flat |
 |
| Case 3: Constant velocity on flat |
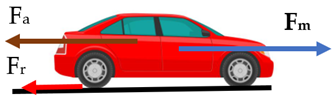 |
| Case 4: Acceleration uphill |
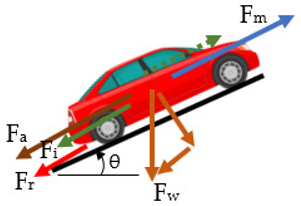 |
| Case 5: Constant velocity uphill |
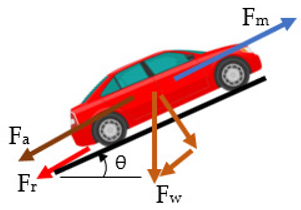 |
| Case 6: Deceleration uphill |
 |
| Case 7: Deceleration downhill |
 |
| Case 8: Constant velocity downhill |
 |
| Case 9: Acceleration downhill |
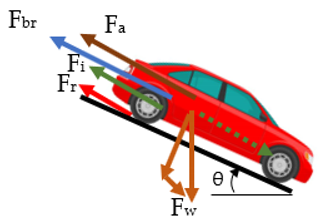 |
| A (m2) | C | ρ (kg/m3) | m (kg) | g (m/s2) | θ (rad) |
|---|---|---|---|---|---|
| 2.2 | 0.32 | 1.25 | 1000 | 9.81 | 0 |
| Regenerative Braking System | Scenario 1 | Scenario 2 | Scenario 3 |
|---|---|---|---|
| Time (s) | 1800 | 1215 | 6144 |
| Distance travelled (m) | 27,008.4 | 8840.2 | 198,787.5 |
| Theoretical | Theoretical | Theoretical | |
| Motor power (kW) | 607.76 | 183.93 | 479.12 |
| Braking power (kW) | 133.1597 | 75.32 | 94.55 |
| REGENERATIVE BRAKING SYSTEM + AERODYNAMIC FORCE | Scenario 1 | Scenario 2 | Scenario 3 |
| Theoretical | Theoretical | Theoretical | |
| Motor power (kW) | 607.76 | 183.93 | 479.12 |
| Braking power + Aerodyn. Power (kW) | 385.65 | 95.76 | 375.34 |
| Regenerative Device | Regenerative System + Use of Aerodynamics Force | |
|---|---|---|
| Theoretical % | Theoretical % | |
| Scenario 3 | 19.73 | 78.34 |
| Scenario 2 | 40.95 | 52.06 |
| Scenario 1 | 21.9 | 63.45 |
| Purely Regenerative System | Scenario 1 | Scenario 2 | Scenario 3 |
|---|---|---|---|
| Motor power (kW) | 607.76 | 183.93 | 479.12 |
| Braking power (kW) | 133.1597 | 75.32 | 94.55 |
| Power recovered (kW) | 22.54 | 2.09 | 23.82 |
| Regenerative system + use of aerodyn. force | Scenario 1 | Scenario 2 | Scenario 3 |
| Theoretical | Theoretical | Theoretical | |
| Motor power (kW) | 607.76 | 183.93 | 479.12 |
| Braking power + Aerodyn. power (kW) | 385.65 | 95.76 | 375.34 |
| Power recovered (kW) | 144.29 | 11.68 | 160.46 |
| Regenerative Braking System | Regenerative Braking System + Aerodynamics | |||
|---|---|---|---|---|
| Theoretical % | Real % | Theoretical % | Real % | |
| Scenario 1 (mixed) | 21.9 | 3.71 | 63.45 | 23.74 |
| Scenario 2 (urban) | 40.95 | 1.14 | 52.06 | 6.35 |
| Scenario 3 (interurban) | 19.73 | 4.97 | 78.34 | 33.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rubio, F.; Llopis-Albert, C. Analysis of the Use of a Wind Turbine as an Energy Recovery Device in Transport Systems. Mathematics 2021, 9, 2265. https://doi.org/10.3390/math9182265
Rubio F, Llopis-Albert C. Analysis of the Use of a Wind Turbine as an Energy Recovery Device in Transport Systems. Mathematics. 2021; 9(18):2265. https://doi.org/10.3390/math9182265
Chicago/Turabian StyleRubio, Francisco, and Carlos Llopis-Albert. 2021. "Analysis of the Use of a Wind Turbine as an Energy Recovery Device in Transport Systems" Mathematics 9, no. 18: 2265. https://doi.org/10.3390/math9182265
APA StyleRubio, F., & Llopis-Albert, C. (2021). Analysis of the Use of a Wind Turbine as an Energy Recovery Device in Transport Systems. Mathematics, 9(18), 2265. https://doi.org/10.3390/math9182265






