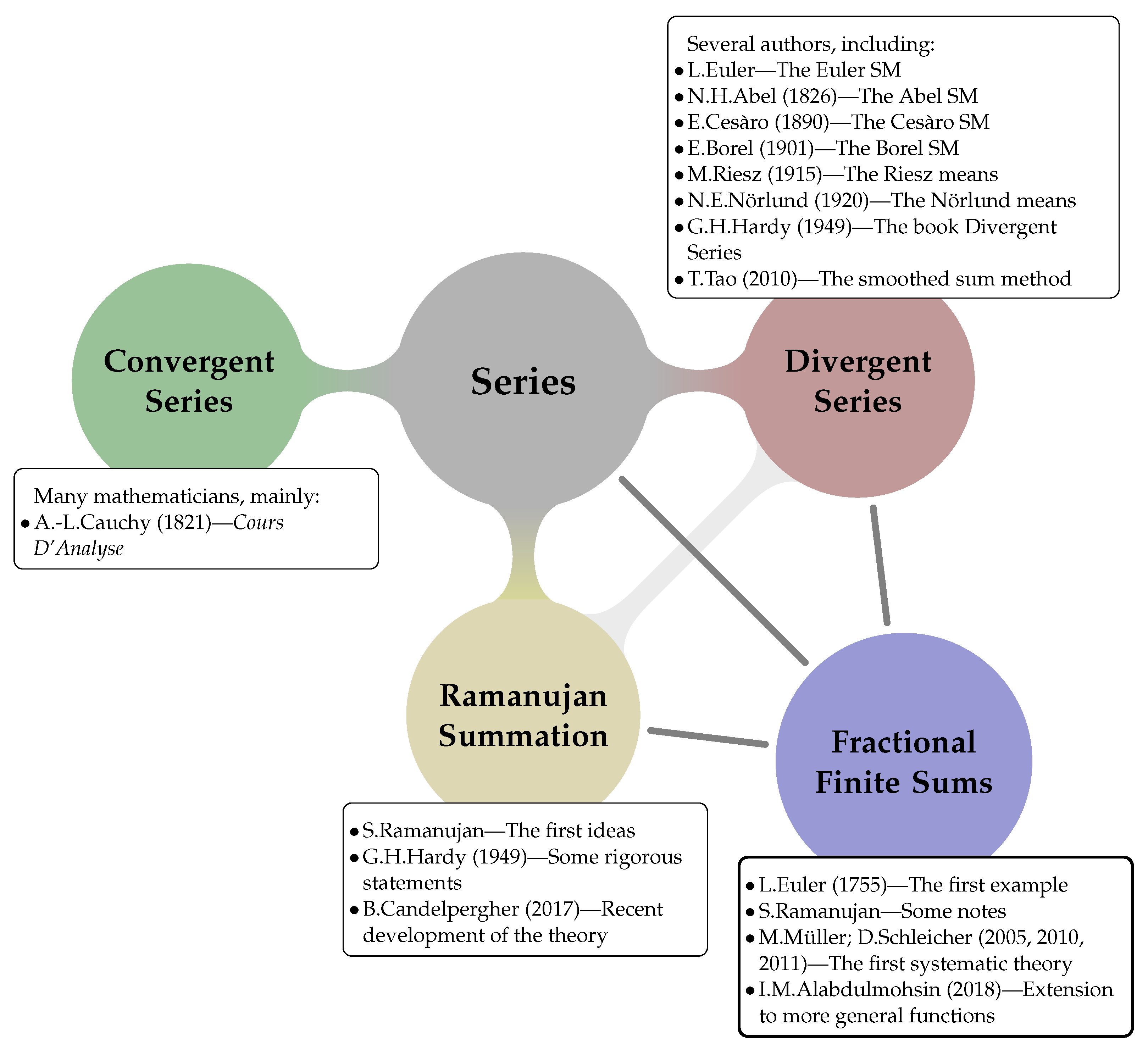
Overview in Summabilities: Summation Methods for Divergent Series, Ramanujan Summation and Fractional Finite Sums
Abstract
:1. Introduction
2. Divergent Series and Summation Formulae
2.1. About a General Summation Method
2.2. The Cesàro Summation Method
2.3. The Nörlund Means
2.4. The Abel Summation Method
2.5. The Euler Summation Method
2.6. The Borel Summation Methods
2.7. The Riesz Means
2.8. Some Examples
2.9. The Euler–Maclaurin Summation Formula
2.10. The Smoothed Sum Method
2.11. Additional Examples: Power Sums, Riemann Zeta Function, and Some Applications
3. Ramanujan Summation
3.1. Ramanujan Constant of a Series
3.2. The Definition of Ramanujan Summation
3.3. Some Properties of the Ramanujan Summation
3.4. About the Algebraic Framework
4. Fractional Finite Sums
4.1. Fractional Finite Sums, According to Ramanujan
4.2. Fractional Finite Sums, According to Müller and Schleicher
4.2.1. The Axioms for the Fractional Finite Sums
4.2.2. The Unique Possible Definition for the Fractional Finite Sums
4.2.3. Some Examples and Applications
4.3. Fractional Finite Sums for More General Functions
- Simple finite sum (SFS): sums of type
- Composite finite sum (CFS): sums of type
- Oscillatory simple finite sum (OSFS): sums of type
- Oscillatory composite finite sum (OCFS): sums of type
4.3.1. Simple Finite Sums
4.3.2. Composite Finite Sum
4.3.3. The Generalized Definition of Series
4.3.4. Oscillatory Simple Finite Sums
4.3.5. Oscillatory Composite Finite Sums
4.3.6. Methods to Evaluate Fractional Finite Sums
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CFS | Composite finite sum |
| EMSF | Euler–Maclaurin summation formula |
| EBSF | Euler–Boole summation formula |
| FSF | Fractional summable function |
| FFS | Fractional finite sum |
| FFSF | Fundamental fractional summation formula |
| OCFS | Oscillatory composite finite sum |
| OSFS | Oscillatory simple finite sum |
| RCS | Ramanujan constant of a series |
| RS | Ramanujan summation |
| SFS | Simple finite sum |
| SM | Summation method |
| WKB | Wentzel-Kramers-Brillouin |
References
- Euler, L. Institutiones Calculi Differentialis cum eius usu in Analysi Finitorum ac Doctrina Serierum; Academiae Imperialis Scientiarum Petropolitanae: St. Petersburg, Russia, 1755. [Google Scholar]
- Euler, L. Fondations of Differential Calculus; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Cajori, F. A History of Mathematical Notations: Two Volumes Bound As One; Dover: New York, NY, USA, 1993. [Google Scholar]
- Cauchy, A.L. Cours D’Analyse de L’École Royale Polytechnique; De L’Imprimerie Royale: Paris, France, 1821. [Google Scholar]
- Bradley, R.E.; Sandifer, C.E. Cauchy’s Cours d’analyse: An Annotated Translation; Sources and Studies in the History of Mathematics and Physical Sciences; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Fourier, J.B.J. La théorie analytique de la chaleur; Chez Firmin Didot, Père ed Fils: Paris, France, 1822. [Google Scholar]
- Graham, R.L.; Knuth, D.E.; Patashnik, O. Concrete Mathematics: A Foundation for Computer Science; Addison-Wesley Publishing Company: New York, NY, USA, 1994. [Google Scholar]
- Mariconda, C.; Tonolo, A. Discrete Calculu—Methods for Counting; Unitext; Springer: Berlin/Heidelberg, Germany, 2016; Volume 103. [Google Scholar]
- Tao, T. Compactness and Contradiction; American Mathematical Society: Providence, RI, USA, 2010. [Google Scholar]
- Ramanujan, S. Notebooks of Srinivasa Ramanujan; Tata Institute of Fundamental Research: Bombay, India, 1957; Volumes 1–2. [Google Scholar]
- Ramanujan, S. Collected Papers of Srinivasa Ramanujan; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Candelpergher, B. Ramanujan Summation of Divergent Series; Lectures Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 2017; Volume 2185. [Google Scholar]
- Müller, M.; Schleicher, D. How to add a non-integer number of terms, and how to produce unusual infinite summations. J. Comput. Appl. Math. 2005, 178, 347–360. [Google Scholar] [CrossRef] [Green Version]
- Müller, M.; Schleicher, D. Fractional sums and Euler-like identities. Ramanujan J. 2010, 21, 123–143. [Google Scholar] [CrossRef] [Green Version]
- Müller, M.; Schleicher, D. How to add a noninteger number of terms: From axioms to new identities. Am. Math. Mon. 2011, 118, 136–152. [Google Scholar] [CrossRef]
- Alabdulmohsin, I.M. Summability Calculus A Comprehensive Theory of Fractional Finite Sums; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Van Rooij, A.C.M. Non-archimedian Functional Analysis; Marcel Dekker: New York, NY, USA, 1978. [Google Scholar]
- Natarajan, P.N. An Introduction to Ultrametric Summability Theory, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Schikhof, W.H. Ultrametric Calculus: An Introduction to p-Adic Analysis; Cambridge University Press: Cambridge, UK, 1984. [Google Scholar]
- Vladimirov, V.S.; Volovich, I.V.; Zelenov, E.I. p-Adic Analysis and Mathematical Physics; Word Scientific: Singapore, 1994. [Google Scholar]
- Andree, R.V.; Petersen, G.M. Matrix methods of summation, regular for p-adic valuations. Proc. Am. Math. Soc. 1953, 7, 250–253. [Google Scholar]
- Hardy, G.H. Divergent Series; Oxford University Press: Oxford, UK, 1949. [Google Scholar]
- Fridy, J.A. Properties of Absolute Summability Matrices. Proc. Am. Math. Soc. 1970, 24, 583–585. [Google Scholar] [CrossRef]
- Fridy, J.A. Summability of Rearrangements of Sequences. Math. Z. 1975, 143, 187–192. [Google Scholar] [CrossRef]
- Keagy, T.A. Matrix transformations and absolute summability. Pac. J. Math. 1976, 63, 411–415. [Google Scholar] [CrossRef]
- Wilanski, A. Summability Through Functional Analysis; Elsevier: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Misra, U.K. An Introduction to Summability Methods. In Current Topics in Summability Theory and Applications; Dutta, H., Rhoades, B., Eds.; Springer: Singapore, 2016; Chapter 1; pp. 1–27. [Google Scholar]
- Ferraro, G. The first modern definition of the sum of a divergent series: An aspect of the rise of 20th Century mathematics. Arch. Hist. Exact Sci. 1999, 54, 101–135. [Google Scholar] [CrossRef]
- Cesàro, E. Sur la multiplication des series. Bull. Scie. Math. 1890, 14, 114–120. [Google Scholar]
- Fine, N.J. Cesaro summability of Walsh-Fourier series. Proc. Natl. Acad. Sci. USA 1955, 41, 588–591. [Google Scholar] [CrossRef] [Green Version]
- Waterman, D. On the summability of Fourier series of functions of Λ-bounded variation. Stud. Math. 1976, LV, 87–95. [Google Scholar] [CrossRef]
- Grigoryan, M.G.; Galoyan, L.N. On the uniform convergence of negative order Cesaro means of Fourier series. J. Math. Anal. Appl. 2016, 434, 554–567. [Google Scholar] [CrossRef]
- Hölder, O. Beweis des Satzes, dass eine eindeutige analytische Function in unendlicher Nähe einer wesentlich singulären Stelle jedem Werth beliebig nahe kommt. Math. Ann. 1882, 20, 138–143. [Google Scholar] [CrossRef]
- Chapman, S. On non-integral orders of summability of series and integrals. Proc. Lond. Math. Soc. 1911, 2, 369–409. [Google Scholar] [CrossRef]
- Erdélyi, A. Higher Transcendental Functions; McGraw-Hill: New York, NY, USA, 1953. [Google Scholar]
- Artin, E. The Gamma Function; Selected Topics in Mathematics; Holt, Rinehart and Winston: New York, NY, USA, 1964; p. 39. [Google Scholar]
- Nörlund, N.E. Sur une application des fonctions permutables. Lunds Universitet Arsskrift 1919, 16, 1–10. [Google Scholar]
- Mears, F.M. Absolute Regularity and the Norlund Mean. Ann. Math. 1937, 38, 594–601. [Google Scholar] [CrossRef]
- Abel, N.H. Untersuchungen über die Reihe: u.s. w. Crelles J. 1826, 1826, 311–339. [Google Scholar]
- Lindelöf, E. Le Calcul des Résidus et ses Applications à la Théorie des Fonctions; Gauthier-Villars: Paris, France, 1905. [Google Scholar]
- Mittag-Leffler, G. Sur la représentation analyitique d’une branche uniforme d’une function monogène. Acta Math. 1905, 29, 101–181. [Google Scholar] [CrossRef]
- Rajagopal, C.T. On Tauberian theorems for Abel-Cesàro summability. Proc. Glasg. Math. Assoc. 1958, 3, 176–181. [Google Scholar] [CrossRef] [Green Version]
- Badiozzaman, A.J.; Thorpe, B. Some best possible Tauberian results for Abel and Cesàro summability. Bull. Lond. Math. Soc. 1996, 28, 283–290. [Google Scholar] [CrossRef]
- Whittaker, J.M. The absolute summability of Fourier Series. Proc. Edimburgh Math. Soc. 1930, 2, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Zeidler, E. Quantum Field Theory II: Quantum Electrodynamics; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Euler, L. De seriebus divergentibus. Novi Comment. Acad. Sci. Petropolitanae 1760, 5, 205–237. [Google Scholar]
- Santander, M. Sumas de Potencias y Series Divergentes Un panorama sobre sumación de series, sumas suavizadas, números y polinomios de Bernoulli, la fórmula de Euler-Maclaurin y la función ζ de Riemann. University of Valladolid, Valladolid, Spain, 2017. Unpublished Notes. [Google Scholar]
- Borel, E. Leçons sur les Séries Divergentes, 2nd ed.; Gauthier-Villars: Paris, France, 1901. [Google Scholar]
- Aoki, T.; Yoshida, J. Microlocal reduction of ordinary differential operator with a large parameter. Publ. Res. Inst. Math. Sci. Kyoto Univ. 1993, 29, 959–975. [Google Scholar] [CrossRef] [Green Version]
- Bender, C.M.; Orszag, S.A. Advanced Mathematical Methods for Scientist and Engineers I, Asymptotic Methods and Perturbation Theory; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Voros, A. The return of the quartic osscilator. The complex WKB method. Annales L’Institut Henri Poincaré Sect. A 1983, 39, 211–338. [Google Scholar]
- Giller, S.; Milczarski, P. Borel summable solutions to one-dimensional Schrödinger equation. J. Math. Phys. 2001, 42, 608–640. [Google Scholar] [CrossRef] [Green Version]
- Costin, O. Asymptotics and Borel summability; Monographs and Surveys in Pure and Applied Mathematics; CRC Press: Boca Raton, FL, USA, 2008; Volume 141. [Google Scholar]
- Nemes, G. On the Borel summability of WKB solutions of certain Schrödinger-type differential equations. J. Approx. Theory 2021, 265, 105562. [Google Scholar] [CrossRef]
- Ecalle, J. Les Fonctions Resurgentes. Tome I; Publications Mathématiques d’Orsay: Paris, France, 1981. [Google Scholar]
- Ecalle, J. Les Fonctions Resurgentes. Tome II; Publications Mathématiques d’Orsay: Paris, France, 1981. [Google Scholar]
- Ecalle, J. Les Fonctions Resurgentes. Tome III; Publications Mathématiques d’Orsay: Paris, France, 1985. [Google Scholar]
- Delabaere, E.; Pham, F. Resurgent methods in semi-classical asymptotics. Annales L’Institut Henri Poincaré Section A 1999, 71, 1–94. [Google Scholar]
- Delabaere, E. Divergent Series, Summability and Resurgence III—Resurgence Methods and the First Painlevé Equation; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Hardy, G.H.; Riesz, M. The General Theory of Dirichlet’s Series; Cambridge Tracts in Mathematics and Mathematical Physics; Stechert-Hafner, Inc.: New York, NY, USA, 1964; Volume 18. [Google Scholar]
- Bernoulli, J. Ars Conjectandi, Opus Posthumum. Accedit Tractatus de Seriebus Infinitis, et Epistola Ballicé Scripta de Ludo Pilae Reticularis; Thurneysen Brothers: Basel, Switzerland, 1713. [Google Scholar]
- Gould, H.W. Formulas for Bernoulli Numbers. Am. Math. Mon. 1972, 79, 44–51. [Google Scholar] [CrossRef]
- Lehmer, D.H. A new approach to Bernoulli polynomials. Am. Math. Mon. 1988, 95, 905–911. [Google Scholar] [CrossRef]
- Boas, R.P. Partial Sums of Infinite Series, and How They Grow. Am. Math. Mon. 1977, 84, 237–258. [Google Scholar] [CrossRef]
- Euler, L. Methodus generalis summandi progressiones. Comment. Acad. Sci. Petropolitanae 1738, 6, 68–97. [Google Scholar]
- Euler, L. Methodus universalis series summandi ulterius promota. Comment. Acad. Sci. Petropolitanae 1736, 8, 147–158. [Google Scholar]
- Maclaurin, C. A Treatise of Fluxions; Knight and Compton Printers: Edinburgh, UK, 1742. [Google Scholar]
- Mills, S. The independent derivations by Leonhard Euler and Colin MacLaurin of the Euler-MacLaurin summation formula. Arch. Hist. Exact Sci. 1985, 33, 1–13. [Google Scholar] [CrossRef]
- Apostol, T.M. An elementary view of Euler’s summation formula. Am. Math. Mon. 1999, 106, 409–418. [Google Scholar] [CrossRef]
- Varadarajan, V.S. Euler and his work on infinite series. Bull. Am. Math. Soc. 2007, 44, 515–539. [Google Scholar] [CrossRef] [Green Version]
- Poisson, S.D. Sur le calcul numérique des Intégrales définies. Mémoires l’Institut 1823, 6, 571–602. [Google Scholar]
- Kač, V.; Cheung, P. Quantum Calculus; Universitext; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Berndt, B.C. Character analogues of the Poisson and Euler-Maclaurin summation formulas with applications. J. Num. Theory 1975, 7, 413–445. [Google Scholar] [CrossRef] [Green Version]
- Borwein, J.M.; Calkin, N.J.; Manna, D. Euler-Boole summation revisited. Am. Math. Mon. 2009, 116, 387–412. [Google Scholar] [CrossRef]
- Boole, G. A Treatise on the Calculus of Finite Differences, 2nd ed.; Dover: London, UK, 1960. [Google Scholar]
- Strodt, W. Remarks of the Euler-Maclaurin and Boole summation formulas. Am. Math. Mon. 1960, 67, 542–554. [Google Scholar] [CrossRef]
- Berndt, B.C.; Schoenfeld, L. Periodic analogues of the Euler-Maclaurin and Poisson summation formulas with applications to number theory. Acta Arith. 1975, 28, 23–68. [Google Scholar] [CrossRef] [Green Version]
- Rane, V.V. Analogues of Euler and Poisson summation formulae. Proc. Indian Acad. Sci. Math. Sci. 2003, 113, 213–221. [Google Scholar] [CrossRef] [Green Version]
- Tao, T. The Euler-Maclaurin Formula, Bernoulli Numbers, the Zeta Function and Real Variable Analytic Continuation. 2010. Available online: https://terrytao.wordpress.com/2010/04/10/ (accessed on 15 April 2021).
- Kowalski, E. Un cours de Théorie Analytique des Nombres; Cours Spécialisés; Société Mathématique de France: Paris, France, 2004. [Google Scholar]
- Grigis, A.; Sjostrand, J. Microlocal Analysis for Differential Operators; London Mathematical Society Lecture Note Series; Cambridge University Press: Cambridge, UK, 1994; Volume 196. [Google Scholar]
- Kowalski, E. Smoothing Sums Wiki Page. 2009. Available online: https://blogs.ethz.ch/kowalski/smoothing-sums-wiki-page/ (accessed on 15 April 2021).
- Bachmann, P. Analytische Zahlentheorie; B. G. Teubner: Leipzig, Germany, 1894. [Google Scholar]
- Landau, E. Handbuch der Lehre von der Verteilung der Primzahlen; B. G. Teubner: Leipzig, Germany, 1909. [Google Scholar]
- Knuth, D.E. The Art of Computer Programming, 3rd ed.; Fundamental Algoritms; Addison-Wesley: Reading, MA, USA, 1997; Volume 1. [Google Scholar]
- Hardy, G.H. Orders of Infinity—The ’Infinitarcalcul’ of Paul du Bois-Reymond; Cambridge Tracts in Mathematics and Mathematical Physics; Cambridge University Press: Cambridge, UK, 1910; Volume 12. [Google Scholar]
- Tou, E.R. Math Origins: Orders of Growth. 2018. Available online: https://www.maa.org/press/periodicals/convergence/math-origins (accessed on 15 April 2021).
- Flajolet, P.; Gourdon, X.; Dumas, P. Mellin transforms and asymptotics: Harmonic sums. Theor. Comput. Sci. 1995, 144, 5–38. [Google Scholar] [CrossRef] [Green Version]
- Titchmarsh, E.C. The Theory of the Riemann Zeta-Function, 2nd ed.; Clarendon Press: Oxford, UK, 1986. [Google Scholar]
- Edwards, A.W.F. Sums of Powers of Integers: A little of the History. Math. Gazzete 1982, 66, 22–28. [Google Scholar] [CrossRef]
- Jacobi, C.G. De usu legitimoformulae summatoriae Maclaurinaianae. Crelles J. 1874, 12, 263–272. [Google Scholar]
- Knuth, D.E. Johann Faulhaber and sums of powers. Math. Comput. 1993, 61, 277–294. [Google Scholar] [CrossRef]
- Orosi, G. A simple derivation of Faulhauber’s Formula. Appl. Math. E-Notes 2018, 18, 124–126. [Google Scholar]
- Kovalenko, V. Euler and Divergent Series. Eur. J. Pure Appl. Math. 2011, 4, 370–423. [Google Scholar]
- Polchinski, J. String Theory; Cambridge University Press: Cambridge, UK, 1998; Volume I. [Google Scholar]
- Helson, H. Foundations of the theory of Dirichlet series. Acta Mathe. 1967, 118, 61–77. [Google Scholar] [CrossRef]
- Weil, A. Prehistory of the Zeta-Function. In Number Theory, Trace Formulas and Discrete Groups; Aubert, K.E., Bombieri, E., Goldfeld, D., Eds.; Academic Press: San Diego, CA, USA, 1989; Chapter 1; pp. 1–9. [Google Scholar]
- Patterson, S.J. An Introduction to the Theory of the Riemann Zeta-Function; Cambridge Studies in Advanced Mathematics; Cambridge University Press: Cambridge, UK, 1988; Volume 14. [Google Scholar]
- Ivić, A. The Riemann Zeta-Function: Theory and Applications; Dover Publications: New York, NY, USA, 2003. [Google Scholar]
- Wreszinski, W.F. Perturbative versus non-perturbative Quantum Field theory: Tao’s method, the Casimir effect, and interacting Wightman theories. Universe 2021, 7, 229. [Google Scholar] [CrossRef]
- Amaku, M.; Coutinho, F.A.B.; Éboli, O.J.P.; Wreszinski, W.F. Asymptotic series, divergent series, and Tao’s method: The Casimir effect. arXiv 2021, arXiv:2101.03930. [Google Scholar]
- Casimir, H.B.G. On the attraction between two perfectly conducting plates. Indag. Math. 1948, 10, 261–263. [Google Scholar]
- Milton, K. The Casimir Effect: Physical Manifestations of Zero-Point Energy; World Scientific: River Edge, NJ, USA, 2001. [Google Scholar]
- Kawakami, N.A.; Nemes, M.C.; Wreszinski, W.F. The Casimir effect for parallel plates revisited. J. Math. Phys. 2007, 48, 101302. [Google Scholar] [CrossRef] [Green Version]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Monstepanenko, V.M. Advances in the Casimir Effect; International Series of Monographs on Physics; Oxford Science Publications: New York, NY, USA, 2009; Volume 145. [Google Scholar]
- Zeidler, E. Quantum Field Theory I: Basics in Mathematics and Physics; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Belinfante, F.J. Computing Dirac’s atomic hydrogen wave functions of the continuum, using summation of mathematically divergent series. Comput. Phys. 1991, 5, 319–322. [Google Scholar] [CrossRef] [Green Version]
- Kunihiro, T. Renormalization-group resummation of a divergent series of the perturbative wave functions of quantum systems. Prog. Theor. Phys. Suppl. 1998, 131, 459–470. [Google Scholar] [CrossRef] [Green Version]
- Contino, R.; Gambassi, A. On dimensional regularization of sums. J. Math. Phys. 2003, 44, 570–587. [Google Scholar] [CrossRef] [Green Version]
- Mur, V.D.; Pozdnyakov, S.G.; Popruzhenko, S.V.; Popov, V.S. Summation of Divergent Series and Zeldovich’s Regularization Method. Phys. Atom. Nuclei 2005, 68, 677–685. [Google Scholar] [CrossRef]
- Fermi, D.; Pizzocchero, L. Local Zeta Regularization and the Casimir Effect. Prog. Theor. Phys. 2011, 126, 419–434. [Google Scholar] [CrossRef] [Green Version]
- Berndt, B.C. Ramanujan’s Notebooks Part I; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebooks. Math. Mag. 1978, 51, 147–164. [Google Scholar] [CrossRef]
- Andrews, G.E. An introduction to Ramanujan’s “lost” notebook. Am. Math. Mon. 1979, 86, 89–108. [Google Scholar]
- Rao, K.S. Srinivasa Ramanujan: Life and Work of a Natural Mathematical Genius, Swayambhu; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebooks Part II; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebooks Part III; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebooks Part IV; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebooks Part V; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Andrews, G.E.; Berndt, B.C. Ramanujan’s Lost Notebook Part I; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Andrews, G.E.; Berndt, B.C. Ramanujan’s Lost Notebook Part II; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Andrews, G.E.; Berndt, B.C. Ramanujan’s Lost Notebook Part III; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Andrews, G.E.; Berndt, B.C. Ramanujan’s Lost Notebook Part IV; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Andrews, G.E.; Berndt, B.C. Ramanujan’s Lost Notebook Part V; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Apostol, T.M. Introduction to Analytic Number Theory; Undergraduate Texts in Mathematics; Springer: Berlin/Heidelberg, Germany, 1976; p. 329. [Google Scholar]
- Nathanson, M.B. Additive Number Theory: The Classical Bases; Graduate Texts in Mathematics; Springer: Berlin/Heidelberg, Germany, 1996; Volume 164. [Google Scholar]
- Candelpergher, B. Développements de Taylor et sommation des series. Exp. Math. 1995, 13, 163–222. [Google Scholar]
- Candelpergher, B.; Coppo, M.A.; Delabaere, E. La sommation de Ramanujan. L’Enseignement Math. 1997, 43, 93–132. [Google Scholar]
- Delabaere, E. Ramanujan’s Summation. In Algorithm Seminar 2001–2002; Chyzak, F., Ed.; INRIA: Rocquencourt, France, 2003; pp. 83–88. [Google Scholar]
- Euler, L. Dilucitationes in capita postrema calculi mei differentialis de funcionibus inexplicabilibus. Mémoires de l’Académie des Sciences de St.-Petersbourg 1813, 4, 88–119. [Google Scholar]
- Galvão, A.T.; Chagas, J.Q. Uma introdução a somatórios fracionários: Aprendendo a somar uma quantidade não inteira de parcelas. Revista Matemática Universitária 2021, 1, 15–33. [Google Scholar] [CrossRef]
- Gosper, R.W.; Ismail, M.E.H.; Zhang, R. On some strange summation formulas. Ill. J. Math. 1993, 37, 240–277. [Google Scholar] [CrossRef]
- Bender, C.M.; Brody, D.C.; Müller, M.P. Hamiltonian for the zeros of the Riemann zeta function. Phys. Rev. Lett. 2017, 118, 130201. [Google Scholar] [CrossRef]
- Müller, M. A note on “Hamiltonian for the zeros of the Riemann zeta function”. arXiv 2017, arXiv:1704.04705. [Google Scholar]
- Tenreiro Machado, J. Entropy analysis of systems exhibiting negative probabilities. Commun. Nonlinear Sci. Numer. Simul. 2016, 36, 58–64. [Google Scholar] [CrossRef]
- Uzun, B.U. On the Fractional Sums of Some Special Functions. Results Math. 2019, 74, 50. [Google Scholar] [CrossRef]
- Hurwitz, A. Einige Eigenschaften der Dirichlet’schen Funktionen F(s)=∑, die bei der Bestimmung der Klassenanzahlen binärer quadratischer Formen auftreten. Zeitschrift für Mathematik und Physik 1882, 27, 86–101. [Google Scholar]
- Fine, N.J. Note on the Hurwitz Zeta-Function. Proc. Am. Math. Soc. 1951, 2, 361–364. [Google Scholar] [CrossRef]
- Nakamura, T. Some formulas related to Hurwitz-Lerch zeta functions. Ramanujan J. 2010, 21, 285–302. [Google Scholar] [CrossRef]
- Oppenheim, A.V.; Schafer, R.W.; Buck, J.R. Discrete-Time Signal Processing; Prentice Hall: Upper Saddle River, NJ, USA, 1998. [Google Scholar]
- Lee, E.A.; Varaiya, P. Structure and Interpretation of Signals and Systems; Addison-Wesley: Boston, MA, USA, 2003. [Google Scholar]
- Dutta, H.; Rhoades, B. (Eds.) Current Topics in Summability Theory and Applications; Springer: Singapore, 2016. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chagas, J.Q.; Machado, J.A.T.; Lopes, A.M. Overview in Summabilities: Summation Methods for Divergent Series, Ramanujan Summation and Fractional Finite Sums. Mathematics 2021, 9, 2963. https://doi.org/10.3390/math9222963
Chagas JQ, Machado JAT, Lopes AM. Overview in Summabilities: Summation Methods for Divergent Series, Ramanujan Summation and Fractional Finite Sums. Mathematics. 2021; 9(22):2963. https://doi.org/10.3390/math9222963
Chicago/Turabian StyleChagas, Jocemar Q., José A. Tenreiro Machado, and António M. Lopes. 2021. "Overview in Summabilities: Summation Methods for Divergent Series, Ramanujan Summation and Fractional Finite Sums" Mathematics 9, no. 22: 2963. https://doi.org/10.3390/math9222963
APA StyleChagas, J. Q., Machado, J. A. T., & Lopes, A. M. (2021). Overview in Summabilities: Summation Methods for Divergent Series, Ramanujan Summation and Fractional Finite Sums. Mathematics, 9(22), 2963. https://doi.org/10.3390/math9222963







