Forced Convection of Non-Newtonian Nanofluid Flow over a Backward Facing Step with Simultaneous Effects of Using Double Rotating Cylinders and Inclined Magnetic Field
Abstract
:1. Introduction
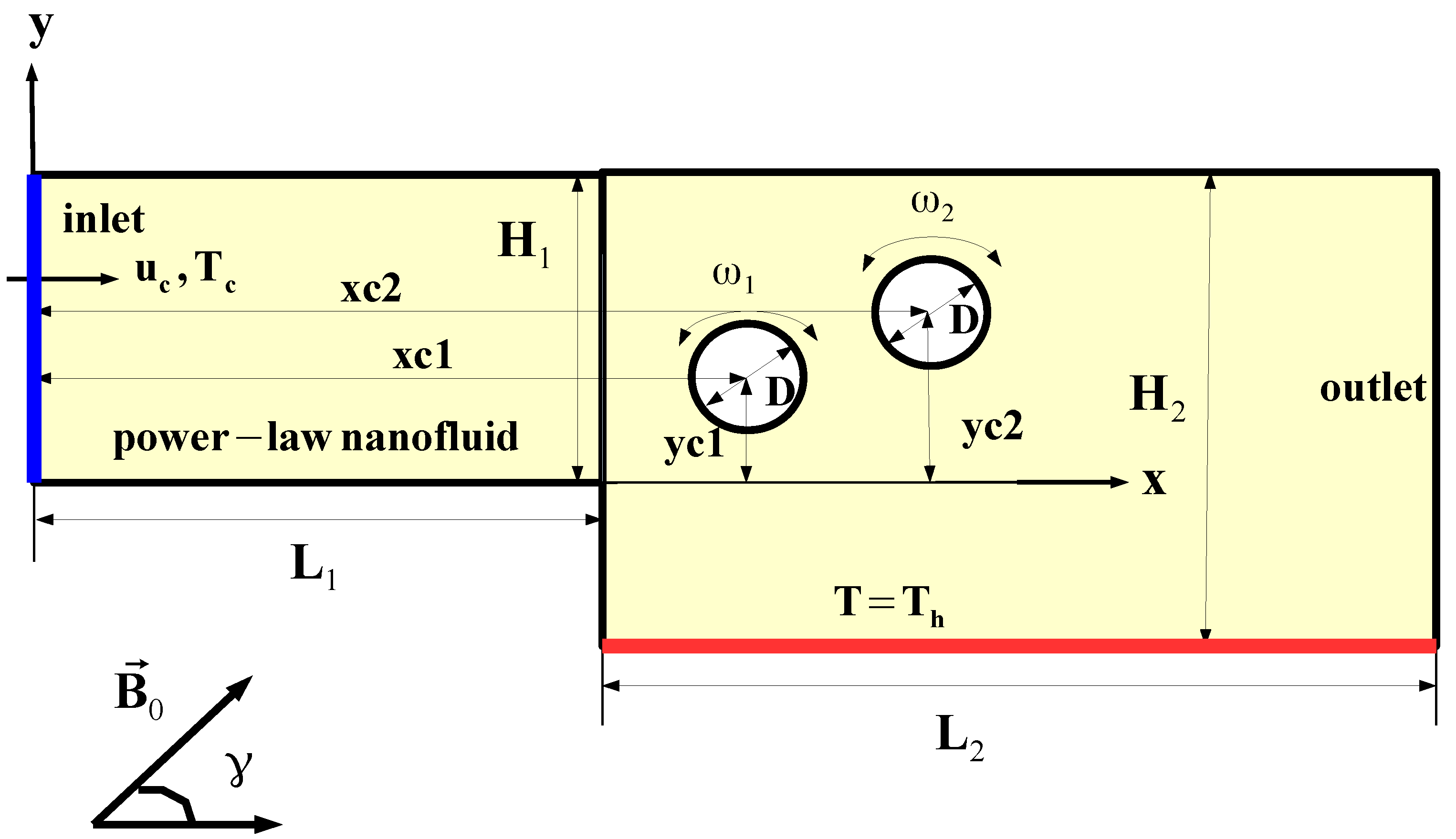
2. Mathematical Formulation
2.1. Nanofluid Relations
2.2. Boundary Conditions and Solver Method
- At the channel inlet, ;
- At the channel exit,
- Bottom wall from step to exit, ;
- Other channel walls,
- For the first cylinder,
- For the second cylinder,
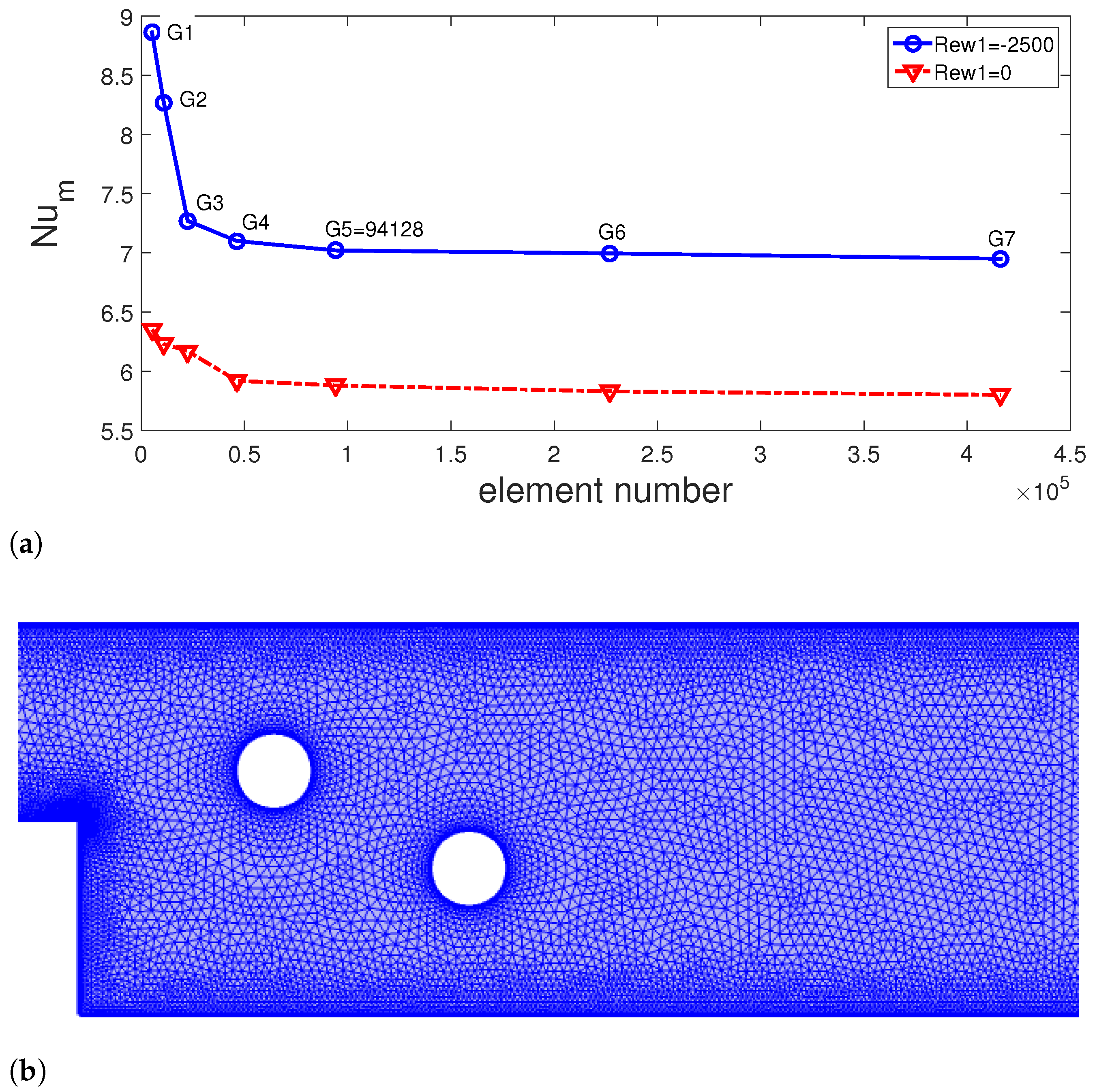
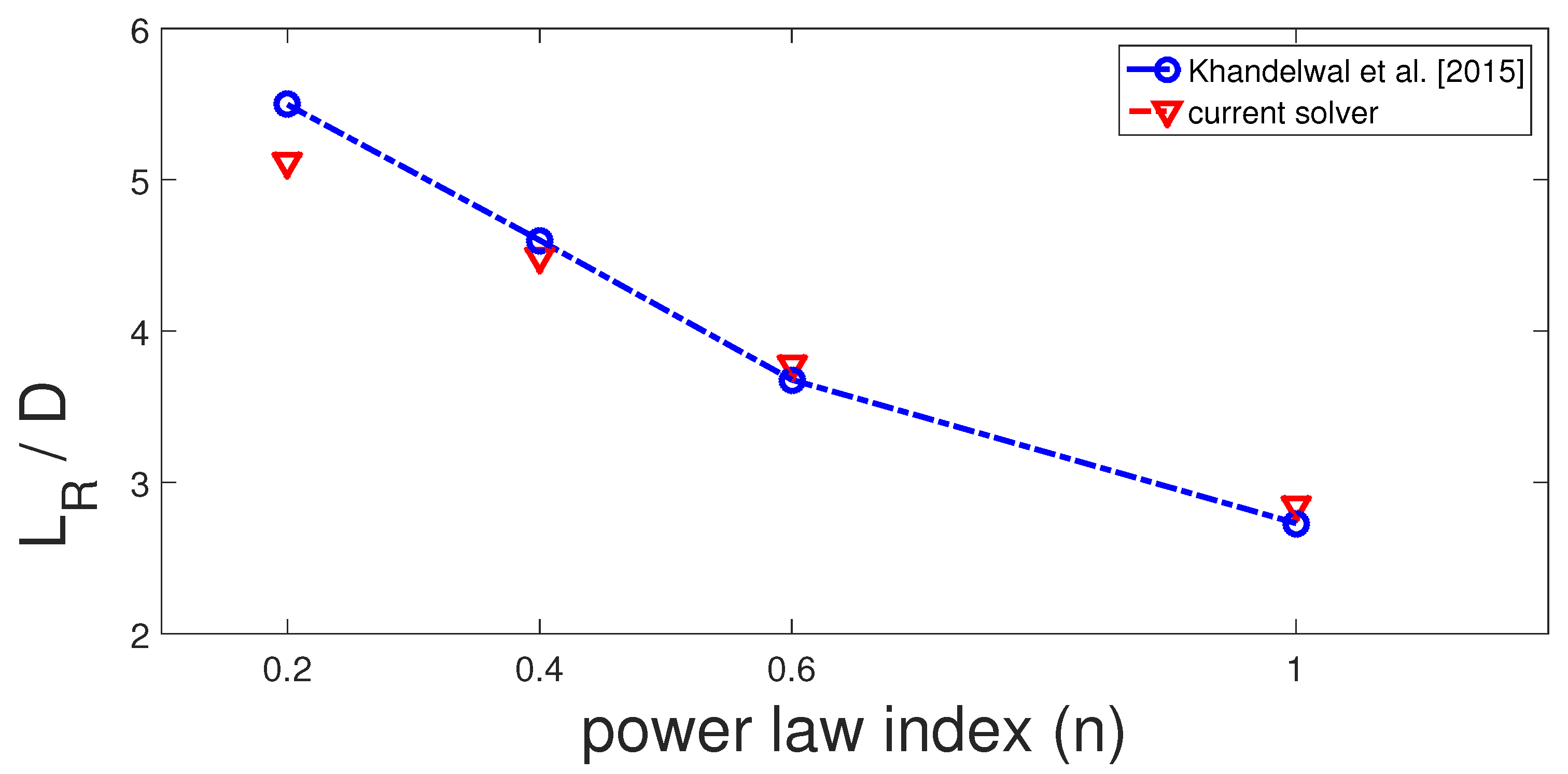
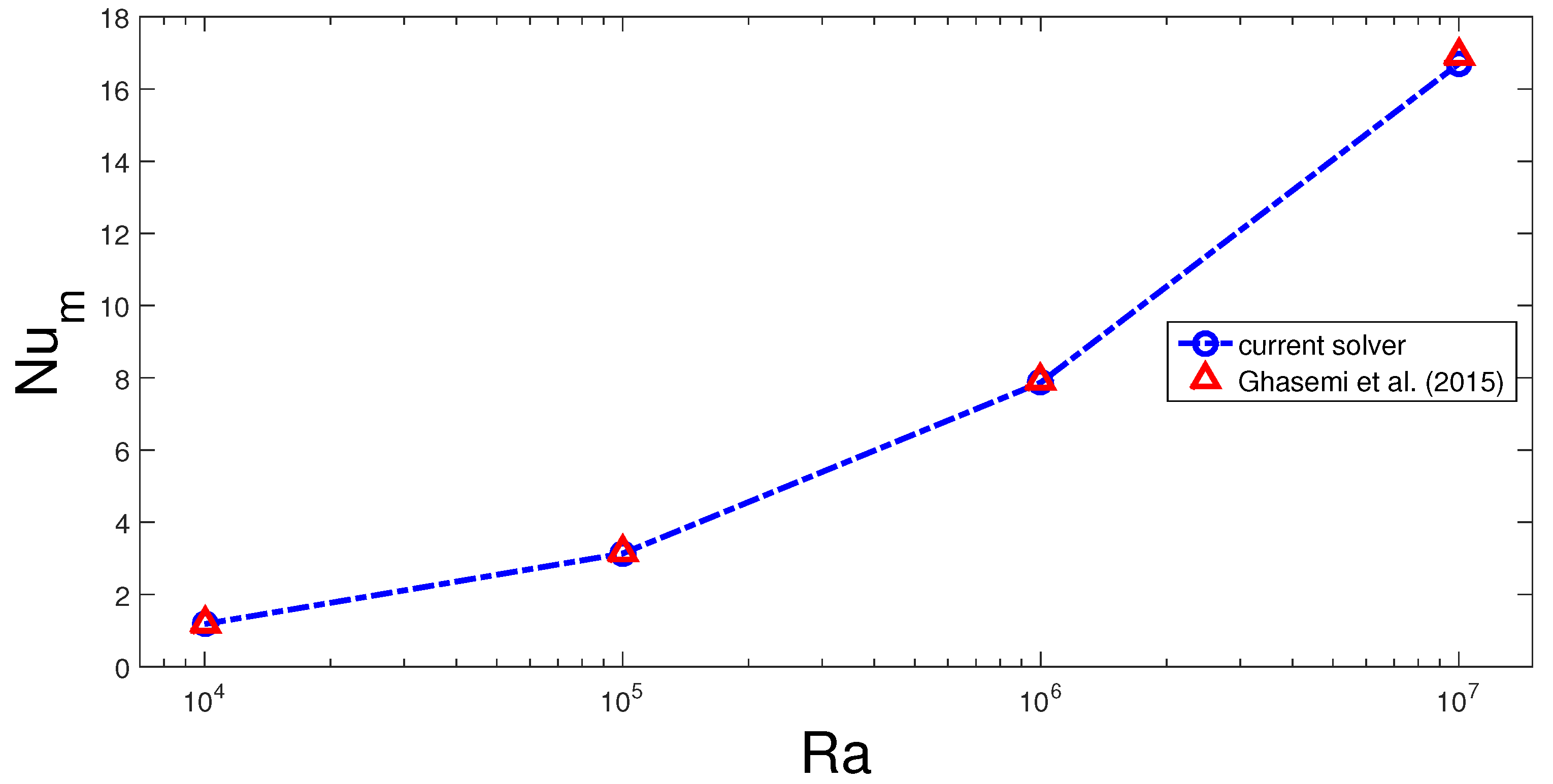
2.3. Grid Independence and Validation
3. Results and Discussion
4. Conclusions
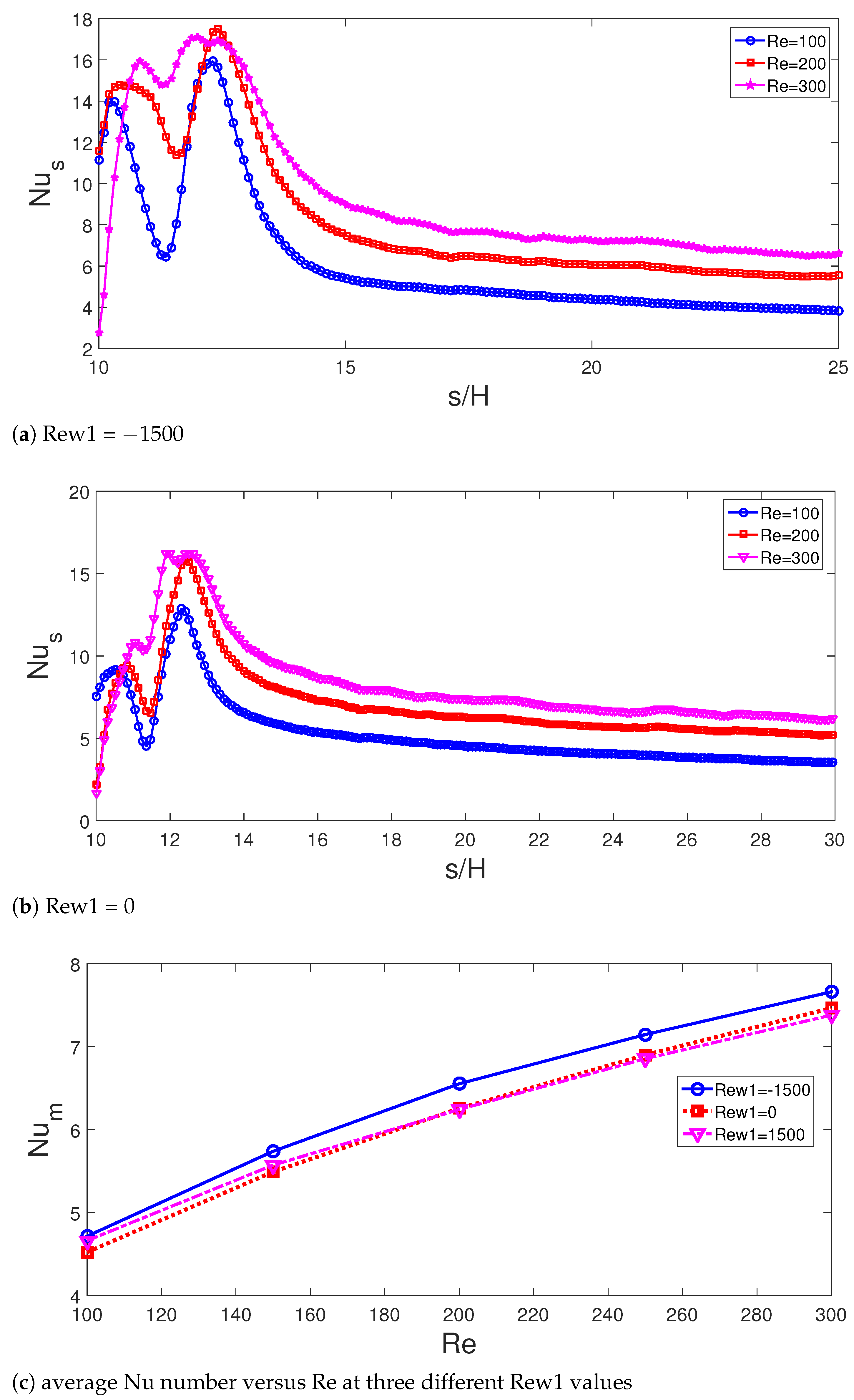
- The presence of double RCs made the flow field complex, especially near the step location. The location and value of the local Nu peak values varied with higher Re numbers and they depend upon the rotational speed and direction of the cylinders. The average Nu number became a higher Re number and the values were higher with CW rotation of the first cylinder.
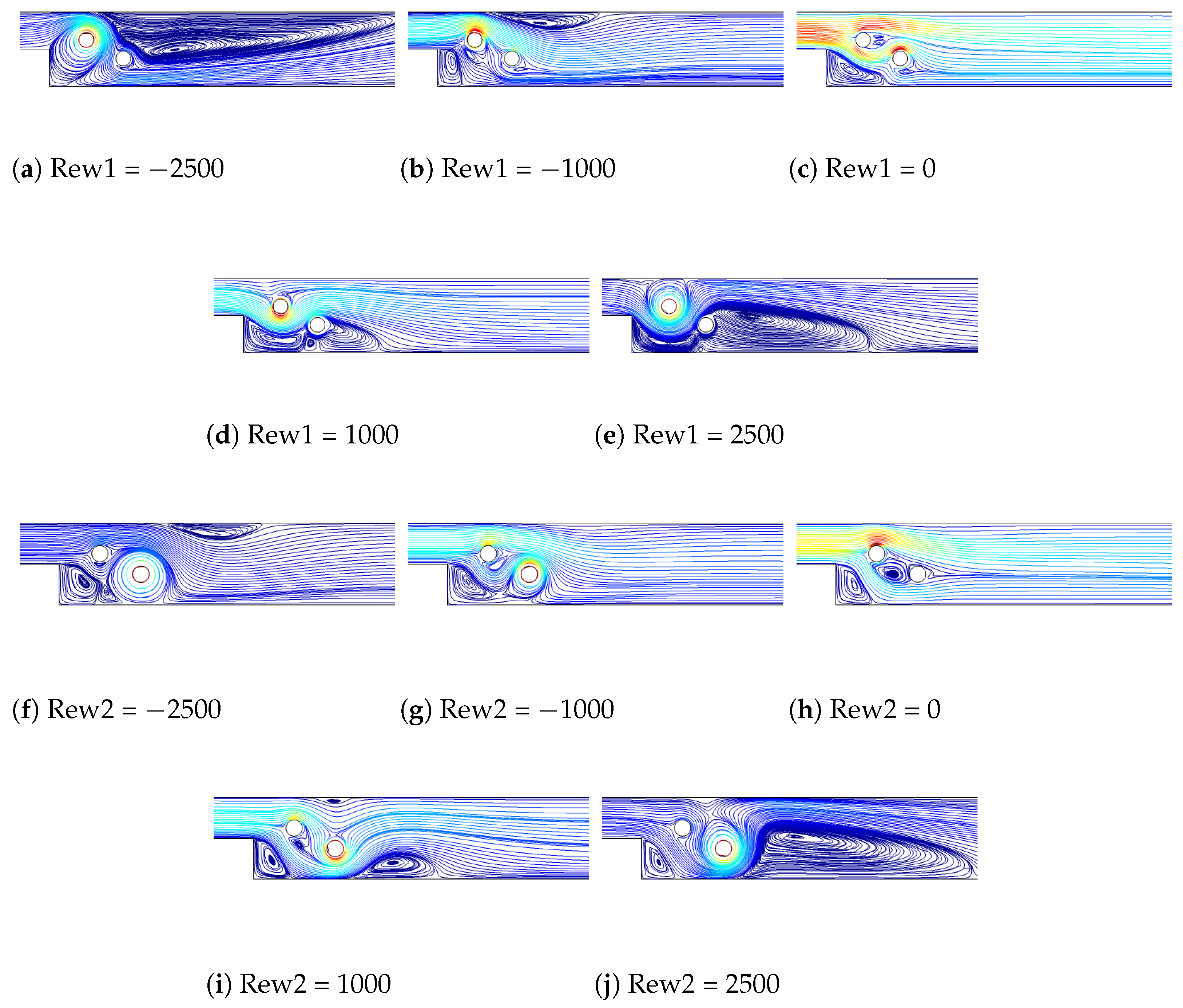
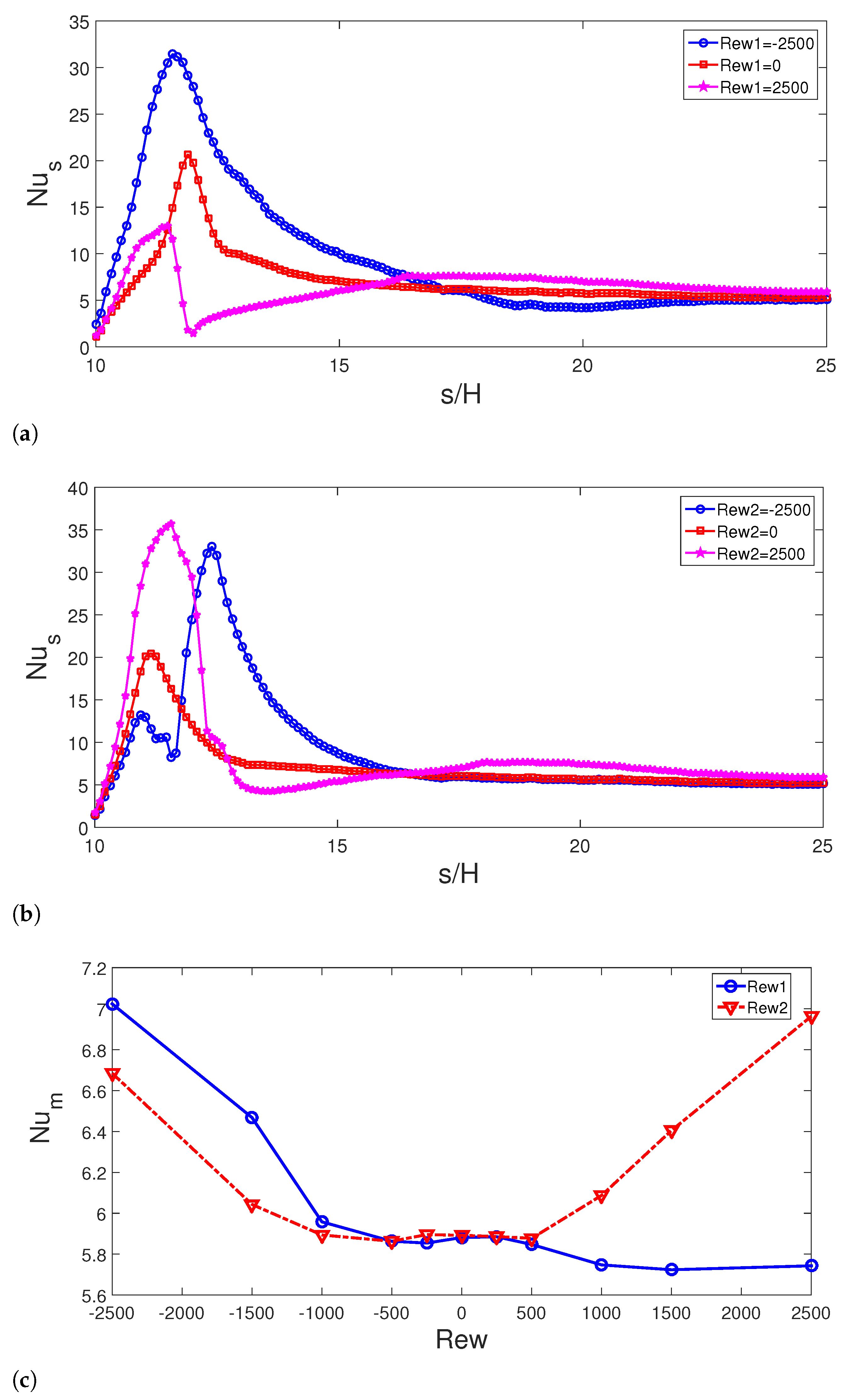
- Vortex formation features and local Nu values were significantly affected by the changing rotational Re numbers of the cylinders. When the first cylinder was considered, the local and average Nu behavior became the opposite for the CW and CCW rotational directions as compared to the motionless case. The CW rotation of the first cylinder had positive impacts of the C-HT features. There were 52% and 37% variations in the local peak Nu value for cases Rew1 = −2500 and Rew1 = 2500 as compared to the configuration for Rew1 = 0 while these amounts become 20% and 2% when average Nu values are compared.
- When the second cylinder is considered, both CW and CCW rotations resulted in positive impacts on the C-HT features. The local peak Nu values increase by approximately 65% and 75% when the rotation of the second cylinder was activated at speeds for Rew2 = −2500 and Rew2 = 2500, and the average Nu value increments were obtained as 14% and 18%.
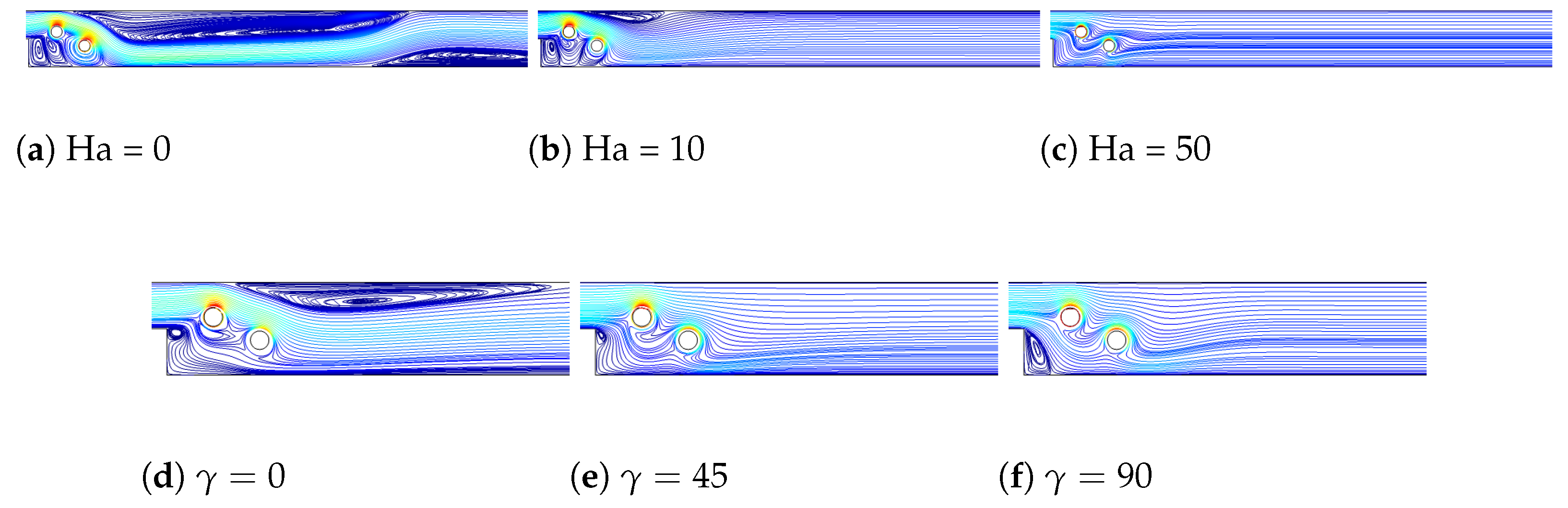
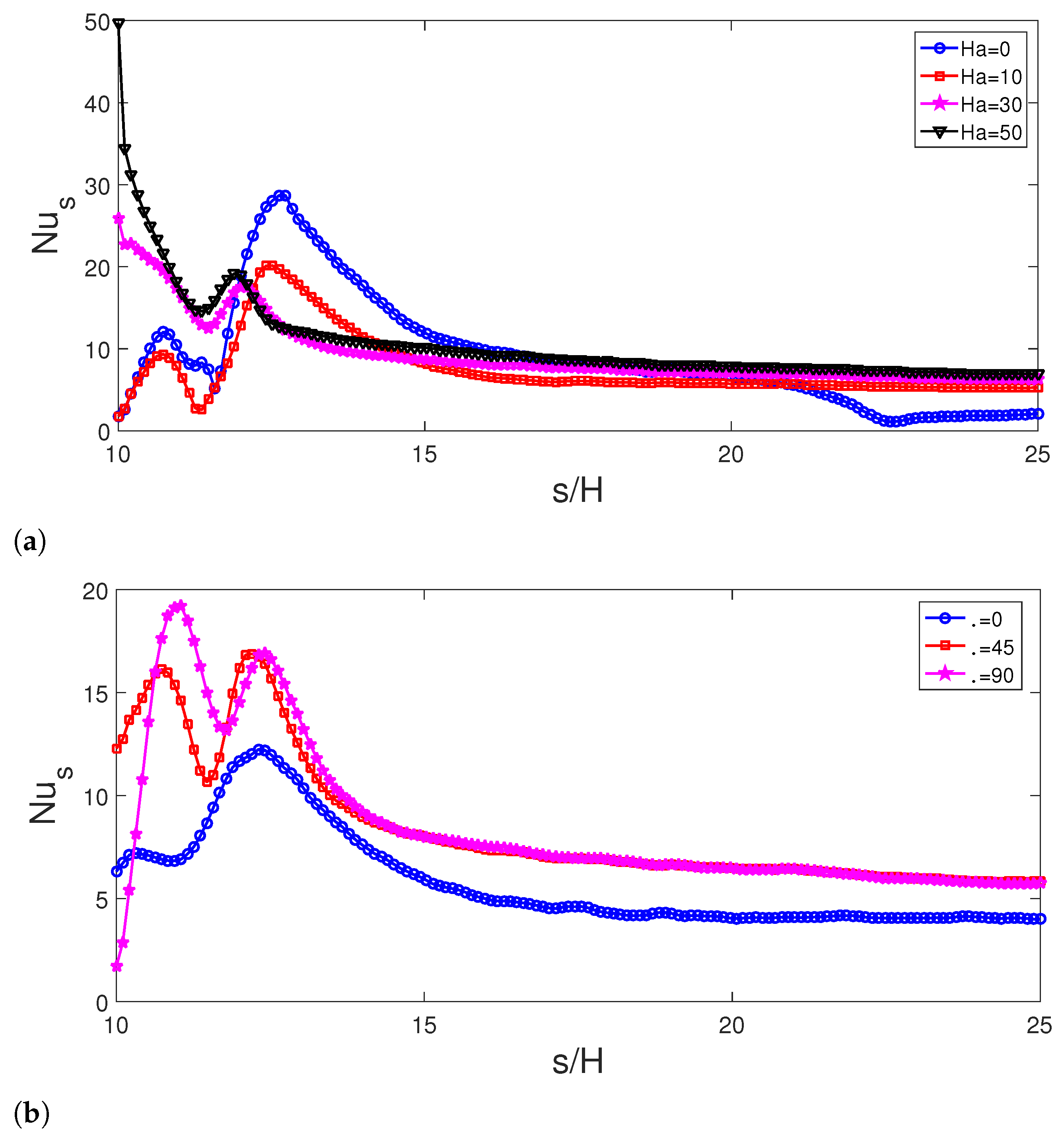
- The suppression of the large vortices near the upper and bottom walls of the channel for the BF-S flow system was observed with higher values of MF strength and inclinations while the number of vortices was reduced. Therefore, the value and location of the local Nu number peaks change with the varying strength and inclination of MF.
- The increment amount in the average Nu number becomes 30% at the highest MF strength while it becomes 44% for the vertical inclination of MF as compared to horizontal alignment.
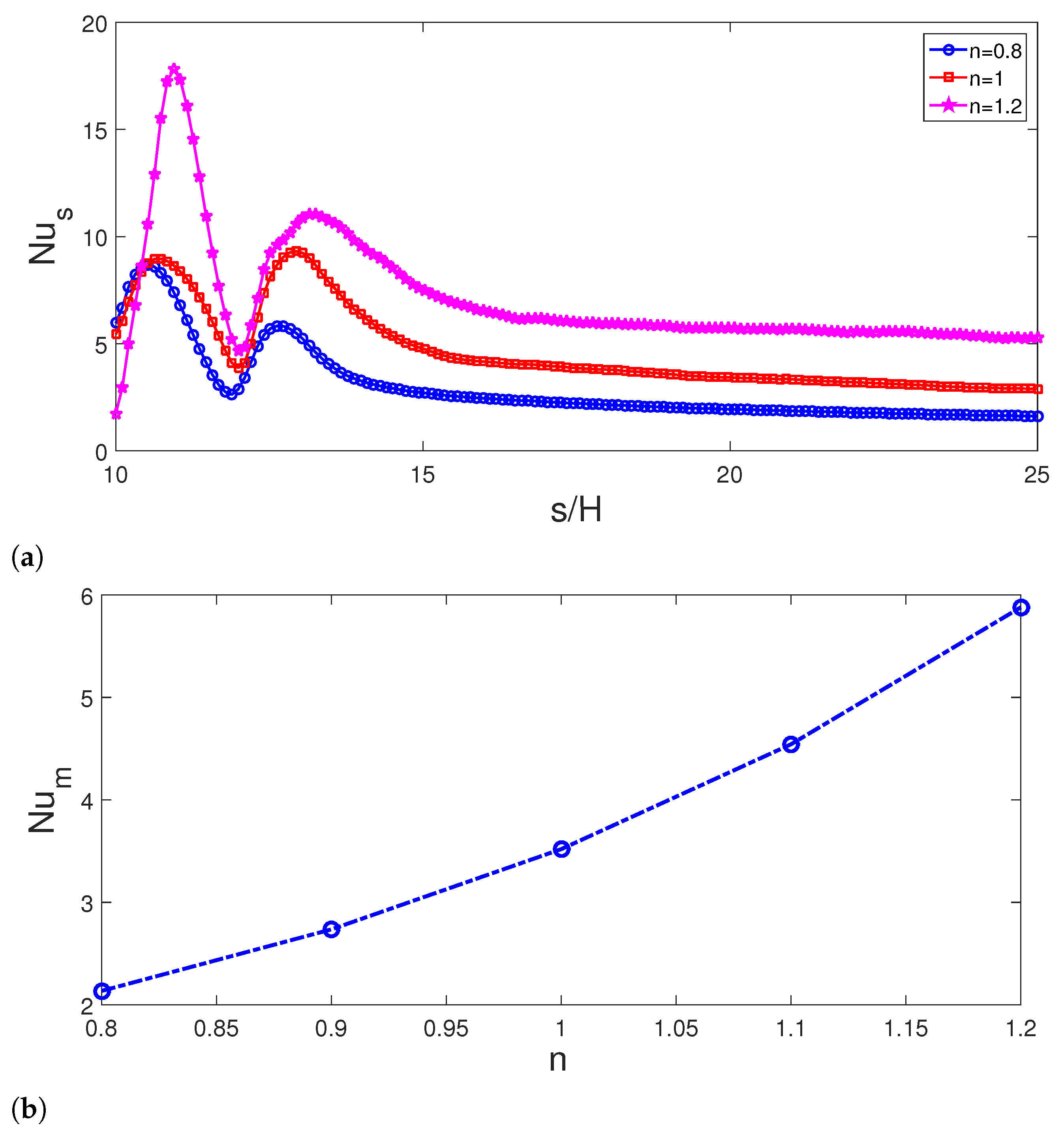
- The vortex formation features near the step are affected by using shear thickening or thinning fluid as compared to using a Newtonian fluid in the BF-S flow system. The value of the first peak in the local Nu number significantly increased from 8.96 and 17.8 when the thermo-fluid system with shear thickening nanofluid was compared with the Newtonian nanofluid. As cases with the power law index of n = 0.8 and n = 1.2 were compared, and the average Nu became 2.75 times higher for the BF-S flow system with double RCs.
- Using alumina particles in the fluid improves the C-HT while the increment amount depends upon the value of the power law index. The enhancement amounts in the average Nu number are 34%, 36% and 36.6% when the values of the indices are n = 0.8, n = 1 and n = 1.2 for the SP-VM of 4%.
- Fourth-order polynomial correlations in terms of the rotational Re number of the RCs are proposed for fluids with different power law indices.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| D | cylinder diameter |
| dp | particle diameter |
| h | heat transfer coefficient |
| k | thermal conductivity |
| k | Boltzmann’s constant |
| H | channel height |
| Ha | Hartmann number |
| M | molecular weight |
| n | power law index |
| NA | Avogadro number |
| Nu | Nusselt number |
| p | pressure |
| Pr | Prandtl number |
| Re | Reynolds number |
| Rew | rotational Reynolds number |
| T | temperature |
| u, v | x–y velocity components |
| x, y | Cartesian coordinates |
| xc1, yc1 | first cylinder center location |
| xc2, yc2 | second cylinder center location |
| Greek Characters | |
| thermal diffusivity | |
| solid volume fraction | |
| kinematic viscosity | |
| density | |
| angle of inclination | |
| shear stress | |
| rotational speed | |
| Subscripts | |
| c | cold |
| h | hot |
| m | average |
| nf | nanofluid |
| p | solid particle |
References
- Armaly, B.F.; Durst, F.; Pereier, J.C.F.; Schonung, B. Experimental and theoretical investigation of backward-facing step flow. J. Fluid Mech. 1983, 127, 473–496. [Google Scholar] [CrossRef]
- Sherry, M.; Lo Jacono, D.; Sheridan, J. An experimental investigation of the recirculation zone formed downstream of a forward facing step. J. Wind Eng. Ind. Aerodyn. 2010, 98, 888–894. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.; Asai, K.; Nonomura, T.; Xi, G.; Liu, T. A review of backward-facing step (BFS) flow mechanisms, heat transfer and control. Therm. Sci. Eng. Prog. 2018, 6, 194–216. [Google Scholar] [CrossRef]
- Kherbeet, A.S.; Safaei, M.R.; Mohammed, H.; Salman, B.; Ahmed, H.E.; Alawi, O.A.; Al-Asadi, M. Heat transfer and fluid flow over microscale backward and forward facing step: A review. Int. Commun. Heat Mass Transf. 2016, 76, 237–244. [Google Scholar] [CrossRef]
- Kumar, S.; Vengadesan, S. The effect of fin oscillation in heat transfer enhancement in separated flow over a backward facing step. Int. J. Heat Mass Transf. 2019, 128, 954–963. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Numerical analysis of laminar pulsating flow at a backward facing step with an upper wall mounted adiabatic thin fin. Comput. Fluids 2013, 88, 93–107. [Google Scholar] [CrossRef]
- Kumar, A.; Dhiman, A.K. Effect of a circular cylinder on separated forced convection at a backward-facing step. Int. J. Therm. Sci. 2012, 52, 176–185. [Google Scholar] [CrossRef]
- Hussain, S.; Ahmed, S.E. Unsteady MHD forced convection over a backward facing step including a rotating cylinder utilizing Fe3O4-water ferrofluid. J. Magn. Magn. Mater. 2019, 484, 356–366. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Polifke, W. Nonlinear, proper-orthogonal-decomposition-based model of forced convection heat transfer in pulsating flow. AIAA J. 2014, 52, 131–145. [Google Scholar] [CrossRef]
- Alsabery, A.; Selimefendigil, F.; Hashim, I.; Chamkha, A.; Ghalambaz, M. Fluid-structure interaction analysis of entropy generation and mixed convection inside a cavity with flexible right wall and heated rotating cylinder. Int. J. Heat Mass Transf. 2019, 140, 331–345. [Google Scholar] [CrossRef]
- Khanafer, K.; Aithal, S. Mixed convection heat transfer in a lid-driven cavity with a rotating circular cylinder. Int. Commun. Heat Mass Transf. 2017, 86, 131–142. [Google Scholar] [CrossRef]
- Kareem, A.K.; Gao, S. Mixed convection heat transfer of turbulent flow in a three-dimensional lid-driven cavity with a rotating cylinder. Int. J. Heat Mass Transf. 2017, 112, 185–200. [Google Scholar] [CrossRef] [Green Version]
- Alsabery, A.I.; Ismael, M.A.; Chamkha, A.J.; Hashim, I. Impact of finite wavy wall thickness on entropy generation and natural convection of nanofluid in cavity partially filled with non-Darcy porous layer. Neural Comput. Appl. 2020, 32, 1–21. [Google Scholar] [CrossRef]
- Costa, V.; Raimundo, A. Steady mixed convection in a differentially heated square enclosure with an active rotating circular cylinder. Int. J. Heat Mass Transf. 2010, 53, 1208–1219. [Google Scholar] [CrossRef]
- Shirani, N.; Toghraie, D.; Zarringhalam, M.; Afrand, M. Numerical simulation of transient mixed convection of water–Cu nanofluid in a square cavity with multiple rotating cylinders having harmonic motion. J. Therm. Anal. Calorim. 2020, 143, 4229–4248. [Google Scholar] [CrossRef]
- Khanafer, K.; Aithal, S.; Vafai, K. Mixed convection heat transfer in a differentially heated cavity with two rotating cylinders. Int. J. Therm. Sci. 2019, 135, 117–132. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Three dimensional unsteady heat and mass transport from six porous moist objects in a channel under laminar forced convection. Appl. Therm. Eng. 2020, 183, 116100. [Google Scholar] [CrossRef]
- Mahmoudi, A.H.; Pop, I.; Shahi, M.; Talebi, F. MHD natural convection and entropy generation in a trapezoidal enclosure using Cu–water nanofluid. Comput. Fluids 2013, 72, 46–62. [Google Scholar] [CrossRef]
- Kahveci, K.; Öztuna, S. MHD natural convection flow and heat transfer in a laterally heated partitioned enclosure. Eur. J. Mech.-B/Fluids 2009, 28, 744–752. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Oztop, H.; Pop, I.; Al-Salem, K. MHD free convection in a wavy open porous tall cavity filled with nanofluids under an effect of corner heater. Int. J. Heat Mass Transf. 2016, 103, 955–964. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. MHD Pulsating forced convection of nanofluid over parallel plates with blocks in a channel. Int. J. Mech. Sci. 2019, 157, 726–740. [Google Scholar] [CrossRef]
- Benzema, M.; Benkahla, Y.K.; Labsi, N.; Ouyahia, S.E.; El Ganaoui, M. Second law analysis of MHD mixed convection heat transfer in a vented irregular cavity filled with Ag–MgO/water hybrid nanofluid. J. Therm. Anal. Calorim. 2019, 137, 1113–1132. [Google Scholar] [CrossRef]
- Abbassi, H.; Nassrallah, S.B. MHD flow and heat transfer in a backward-facing step. Int. Commun. Heat Mass Transf. 2007, 34, 231–237. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Influence of inclination angle of magnetic field on mixed convection of nanofluid flow over a backward facing step and entropy generation. Adv. Powder Technol. 2015, 26, 1663–1675. [Google Scholar] [CrossRef]
- Verma, S.K.; Tiwari, A.K. Progress of nanofluid application in solar collectors: A review. Energy Convers. Manag. 2015, 100, 324–346. [Google Scholar] [CrossRef]
- Mahian, O.; Kianifar, A.; Kalogirou, S.A.; Pop, I.; Wongwises, S. A review of the applications of nanofluids in solar energy. Int. J. Heat Mass Transf. 2013, 57, 582–594. [Google Scholar] [CrossRef]
- Bhattad, A.; Sarkar, J.; Ghosh, P. Improving the performance of refrigeration systems by using nanofluids: A comprehensive review. Renew. Sustain. Energy Rev. 2018, 82, 3656–3669. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Kean, T.H.; Chow, H.K.; Rajaandra, A.; Rahman, S.; Kaur, J. Performance enhancement of cold thermal energy storage system using nanofluid phase change materials: A review. Int. Commun. Heat Mass Transf. 2018, 94, 85–95. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Shehzad, S.; Li, Z. Water based nanofluid free convection heat transfer in a three dimensional porous cavity with hot sphere obstacle in existence of Lorenz forces. Int. J. Heat Mass Transf. 2018, 125, 375–386. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Siavashi, M.; Taylor, R.A.; Niazmand, H.; et al. Recent advances in modeling and simulation of nanofluid flows-Part I: Fundamentals and theory. Phys. Rep. 2019, 790, 1–48. [Google Scholar] [CrossRef]
- Kasaeian, A.; Daneshazarian, R.; Mahian, O.; Kolsi, L.; Chamkha, A.J.; Wongwises, S.; Pop, I. Nanofluid flow and heat transfer in porous media: A review of the latest developments. Int. J. Heat Mass Transf. 2017, 107, 778–791. [Google Scholar] [CrossRef]
- Bahiraei, M.; Heshmatian, S. Graphene family nanofluids: A critical review and future research directions. Energy Convers. Manag. 2019, 196, 1222–1256. [Google Scholar] [CrossRef]
- Sahin, A.Z.; Uddin, M.A.; Yilbas, B.S.; Al-Sharafi, A. Performance enhancement of solar energy systems using nanofluids: An updated review. Renew. Energy 2020, 145, 1126–1148. [Google Scholar] [CrossRef]
- Arshad, W.; Ali, H.M. Graphene nanoplatelets nanofluids thermal and hydrodynamic performance on integral fin heat sink. Int. J. Heat Mass Transf. 2017, 107, 995–1001. [Google Scholar] [CrossRef]
- Ali, H.M.; Ali, H.; Liaquat, H.; Maqsood, H.T.B.; Nadir, M.A. Experimental investigation of convective heat transfer augmentation for car radiator using ZnO-water nanofluids. Energy 2015, 84, 317–324. [Google Scholar] [CrossRef]
- Murshed, S.S.; Estellé, P. A state of the art review on viscosity of nanofluids. Renew. Sustain. Energy Rev. 2017, 76, 1134–1152. [Google Scholar] [CrossRef]
- Pordanjani, A.H.; Aghakhani, S.; Alnaqi, A.A.; Afrand, M. Effect of alumina nano-powder on the convection and the entropy generation of water inside an inclined square cavity subjected to a magnetic field: Uniform and non-uniform temperature boundary conditions. Int. J. Mech. Sci. 2019, 152, 99–117. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Rokni, H.B. Magnetohydrodynamic CuO–water nanofluid in a porous complex-shaped enclosure. J. Therm. Sci. Eng. Appl. 2017, 9, 041007. [Google Scholar] [CrossRef]
- Molana, M.; Dogonchi, A.; Armaghani, T.; Chamkha, A.J.; Ganji, D.; Tlili, I. Investigation of hydrothermal behavior of Fe3O4-H2O nanofluid natural convection in a novel shape of porous cavity subjected to magnetic field dependent (MFD) viscosity. J. Energy Storage 2020, 30, 101395. [Google Scholar] [CrossRef]
- Dogonchi, A.; Seyyedi, S.M.; Hashemi-Tilehnoee, M.; Chamkha, A.J.; Ganji, D. Investigation of natural convection of magnetic nanofluid in an enclosure with a porous medium considering Brownian motion. Case Stud. Therm. Eng. 2019, 14, 100502. [Google Scholar] [CrossRef]
- Ellahi, R. The effects of MHD and temperature dependent viscosity on the flow of non-Newtonian nanofluid in a pipe: Analytical solutions. Appl. Math. Model. 2013, 37, 1451–1467. [Google Scholar] [CrossRef]
- Kefayati, G. Mixed convection of non-Newtonian nanofluid in an enclosure using Buongiornos mathematical model. Int. J. Heat Mass Transf. 2017, 108, 1481–1500. [Google Scholar] [CrossRef]
- Barnoon, P.; Toghraie, D. Numerical investigation of laminar flow and heat transfer of non-Newtonian nanofluid within a porous medium. Powder Technol. 2018, 325, 78–91. [Google Scholar] [CrossRef]
- Ellahi, R.; Aziz, S.; Zeeshan, A. Non-Newtonian nanofluid flow through a porous medium between two coaxial cylinders with heat transfer and variable viscosity. J. Porous Media 2013, 16, 205–2016. [Google Scholar] [CrossRef]
- Sajadifar, S.A.; Karimipour, A.; Toghraie, D. Fluid flow and heat transfer of non-Newtonian nanofluid in a microtube considering slip velocity and temperature jump boundary conditions. Eur. J. Mech.-B/Fluids 2017, 61, 25–32. [Google Scholar] [CrossRef]
- Kefayati, G.R. Simulation of heat transfer and entropy generation of MHD natural convection of non-Newtonian nanofluid in an enclosure. Int. J. Heat Mass Transf. 2016, 92, 1066–1089. [Google Scholar] [CrossRef]
- Siavashi, M.; Karimi, K.; Xiong, Q.; Doranehgard, M.H. Numerical analysis of mixed convection of two-phase non-Newtonian nanofluid flow inside a partially porous square enclosure with a rotating cylinder. J. Therm. Anal. Calorim. 2019, 137, 267–287. [Google Scholar] [CrossRef]
- Ajeeb, W.; Oliveira, M.S.; Martins, N.; Murshed, S.S. Forced convection heat transfer of non-Newtonian MWCNTs nanofluids in microchannels under laminar flow. Int. Commun. Heat Mass Transf. 2021, 127, 105495. [Google Scholar] [CrossRef]
- Al-Rashed, A.A.; Alnaqi, A.A.; Alsarraf, J. Numerical investigation and neural network modeling of the performance of a dual-fluid parabolic trough solar collector containing non-Newtonian water-CMC/Al2O3 nanofluid. Sustain. Energy Technol. Assess. 2021, 48, 101555. [Google Scholar] [CrossRef]
- Alaidrous, A.A.; Eid, M.R. 3-D electromagnetic radiative non-Newtonian nanofluid flow with Joule heating and higher-order reactions in porous materials. Sci. Rep. 2020, 10, 1–19. [Google Scholar] [CrossRef]
- Ali, F.H.; Hamzah, H.K.; Egab, K.; Arıcı, M.; Shahsavar, A. Non-Newtonian nanofluid natural convection in a U-shaped cavity under magnetic field. Int. J. Mech. Sci. 2020, 186, 105887. [Google Scholar] [CrossRef]
- Ghanbari, S.; Javaherdeh, K. Thermal performance enhancement in perforated baffled annuli by nanoporous graphene non-Newtonian nanofluid. Appl. Therm. Eng. 2020, 167, 114719. [Google Scholar] [CrossRef]
- Ibrahim, M.; Algehyne, E.A.; Saeed, T.; Berrouk, A.S.; Chu, Y.M.; Cheraghian, G. Assessment of economic, thermal and hydraulic performances a corrugated helical heat exchanger filled with non-Newtonian nanofluid. Sci. Rep. 2021, 11, 1–16. [Google Scholar]
- Javaherdeh, K.; Karimi, H.; Khojasteh, A. Numerical study of heat transfer enhancement of non-Newtonian nanofluid in porous blocks in a channel partially. Powder Technol. 2021, 383, 270–279. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F.; Kolsi, L.; Omri, M. Performance analysis of thermoelectric generator mounted chaotic channel by using non-Newtonian nanofluid and modeling with efficient computational methods. Alex. Eng. J. 2021. [Google Scholar] [CrossRef]
- Chai, Z.; Shi, B.; Guo, Z.; Rong, F. Multiple-relaxation-time lattice Boltzmann model for generalized Newtonian fluid flows. J. Non-Newton. Fluid Mech. 2011, 166, 332–342. [Google Scholar] [CrossRef]
- Wang, L.; Huang, C.; Yang, X.; Chai, Z.; Shi, B. Effects of temperature-dependent properties on natural convection of power-law nanofluids in rectangular cavities with sinusoidal temperature distribution. Int. J. Heat Mass Transf. 2019, 128, 688–699. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Hayat, T.; Alsaedi, A. MHD free convection of Al2O3–water nanofluid considering thermal radiation: A numerical study. Int. J. Heat Mass Transf. 2016, 96, 513–524. [Google Scholar] [CrossRef]
- Gorla, R.S.R.; Siddiqa, S.; Mansour, M.; Rashad, A.; Salah, T. Heat source/sink effects on a hybrid nanofluid-filled porous cavity. J. Thermophys. Heat Transf. 2017, 31, 847–857. [Google Scholar] [CrossRef]
- Corcione, M. Empirical correlating equations for predicting the effective thermal conductivity and dynamic viscosity of nanofluids. Energy Convers. Manag. 2011, 52, 789–793. [Google Scholar] [CrossRef]
- Maxwell, J.C. A Treatise of Electricity and Magnetism; Oxford University Press: London, UK, 1892; Volume 1. [Google Scholar]
- Minea, A.A. A review on electrical conductivity of nanoparticle-enhanced fluids. Nanomaterials 2019, 9, 1592. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- COMSOL, A.B. COMSOL Multiphysics-CFD Module, Users Guide; COMSOL AB: Stockholm, Sweden, 2017. [Google Scholar]
- Khandelwal, V.; Dhiman, A.; Baranyi, L. Laminar flow of non-Newtonian shear-thinning fluids in a T-channel. Comput. Fluids 2015, 108, 79–91. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S.; Raisi, A. Magnetic field effect on natural convection in a nanofluid-filled square enclosure. Int. J. Therm. Sci. 2011, 50, 1748–1756. [Google Scholar] [CrossRef]





| Coefficients | Case for n = 0.8 | Case for n = 1 | Case for n = 1.28 |
|---|---|---|---|
| p00 | 2.312 (2.306, 2.317) | 3.799 (3.78, 3.819) | 6.441 (6.379, 6.503) |
| p10 | −0.03865 (−0.04448, −0.03283) | −0.1074 (−0.1265, −0.08836) | −0.1756 (−0.2365, −0.1147) |
| p01 | 0.00697 (0.001144, 0.0128) | 0.08156 (0.06251, 0.1006) | 0.05062 (−0.01032, 0.1116) |
| p20 | 0.03983 (0.02966, 0.05) | 0.1509 (0.1177, 0.1842) | 0.08124 (−0.02512, 0.1876) |
| p11 | −0.01954 (−0.02687, −0.0122) | −0.08022 (−0.1042, −0.05624) | −0.03371 (−0.1104, 0.04298) |
| p02 | 0.05901 (0.04884, 0.06917) | 0.2216 (0.1884, 0.2549) | 0.3335 (0.2271, 0.4399) |
| p30 | 0.003582 (0.00127, 0.005894) | −0.009793 (−0.01736, −0.00223) | −0.08369 (−0.1079, −0.05951) |
| p21 | 0.002074 (−0.0001243, 0.004272) | −0.0006278 (−0.007818, 0.006562) | −0.03521 (−0.05821, −0.01222) |
| p12 | 0.01141 (0.009208, 0.0136) | 0.02774 (0.02055, 0.03493) | 0.083 (0.06001, 0.106) |
| p03 | 0.008392 (0.00608, 0.0107) | 0.004152 (−0.003411, 0.01172) | 0.01237 (−0.01182, 0.03655) |
| p40 | −0.006649 (−0.009882, −0.003415) | −0.01813 (−0.02871, −0.007556) | 0.03969 (0.005873, 0.07352) |
| p31 | 0.002751 (0.0004249, 0.005078) | 0.009088 (0.001478, 0.0167) | 0.00398 (−0.02036, 0.02832) |
| p22 | 0.0008172 (−0.001255, 0.002889) | −0.002792 (−0.009571, 0.003987) | −0.03297 (−0.05465, −0.01129) |
| p13 | −0.002167 (−0.004493, 0.0001598) | 0.00895 (0.00134, 0.01656) | 0.002445 (−0.02189, 0.02678) |
| p04 | −0.00168 (−0.004913, 0.001553) | −0.02621 (−0.03678, −0.01563) | 0.004023 (−0.0298, 0.03785) |
| Case for n = 0.8 | Case for n = 1 | Case for n = 1.2 | |
|---|---|---|---|
| R | 0.9843 | 0.9826 | 0.9604 |
| RMSE | 0.0103 | 0.03395 | 0.1086 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolsi, L.; Selimefendigil, F.; Said, L.B.; Mesloub, A.; Alresheedi, F. Forced Convection of Non-Newtonian Nanofluid Flow over a Backward Facing Step with Simultaneous Effects of Using Double Rotating Cylinders and Inclined Magnetic Field. Mathematics 2021, 9, 3002. https://doi.org/10.3390/math9233002
Kolsi L, Selimefendigil F, Said LB, Mesloub A, Alresheedi F. Forced Convection of Non-Newtonian Nanofluid Flow over a Backward Facing Step with Simultaneous Effects of Using Double Rotating Cylinders and Inclined Magnetic Field. Mathematics. 2021; 9(23):3002. https://doi.org/10.3390/math9233002
Chicago/Turabian StyleKolsi, Lioua, Fatih Selimefendigil, Lotfi Ben Said, Abdelhakim Mesloub, and Faisal Alresheedi. 2021. "Forced Convection of Non-Newtonian Nanofluid Flow over a Backward Facing Step with Simultaneous Effects of Using Double Rotating Cylinders and Inclined Magnetic Field" Mathematics 9, no. 23: 3002. https://doi.org/10.3390/math9233002
APA StyleKolsi, L., Selimefendigil, F., Said, L. B., Mesloub, A., & Alresheedi, F. (2021). Forced Convection of Non-Newtonian Nanofluid Flow over a Backward Facing Step with Simultaneous Effects of Using Double Rotating Cylinders and Inclined Magnetic Field. Mathematics, 9(23), 3002. https://doi.org/10.3390/math9233002









