Stability Analysis of Discrete-Time Stochastic Delay Systems with Impulses
Abstract
:1. Introduction
2. Preliminaries
3. Main Results
3.1. Asymptotically Stable
3.2. Almost Sure Exponential Stability
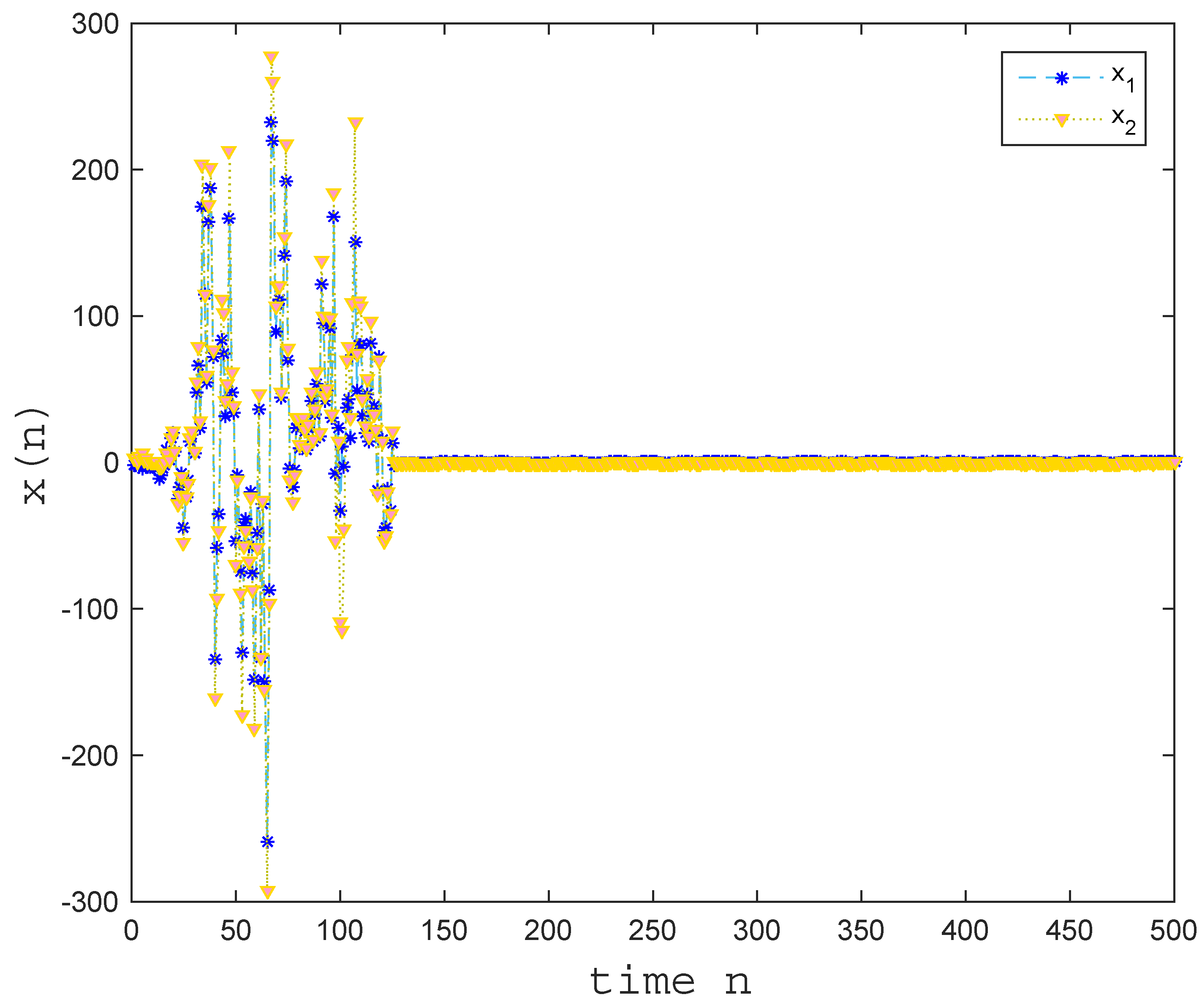
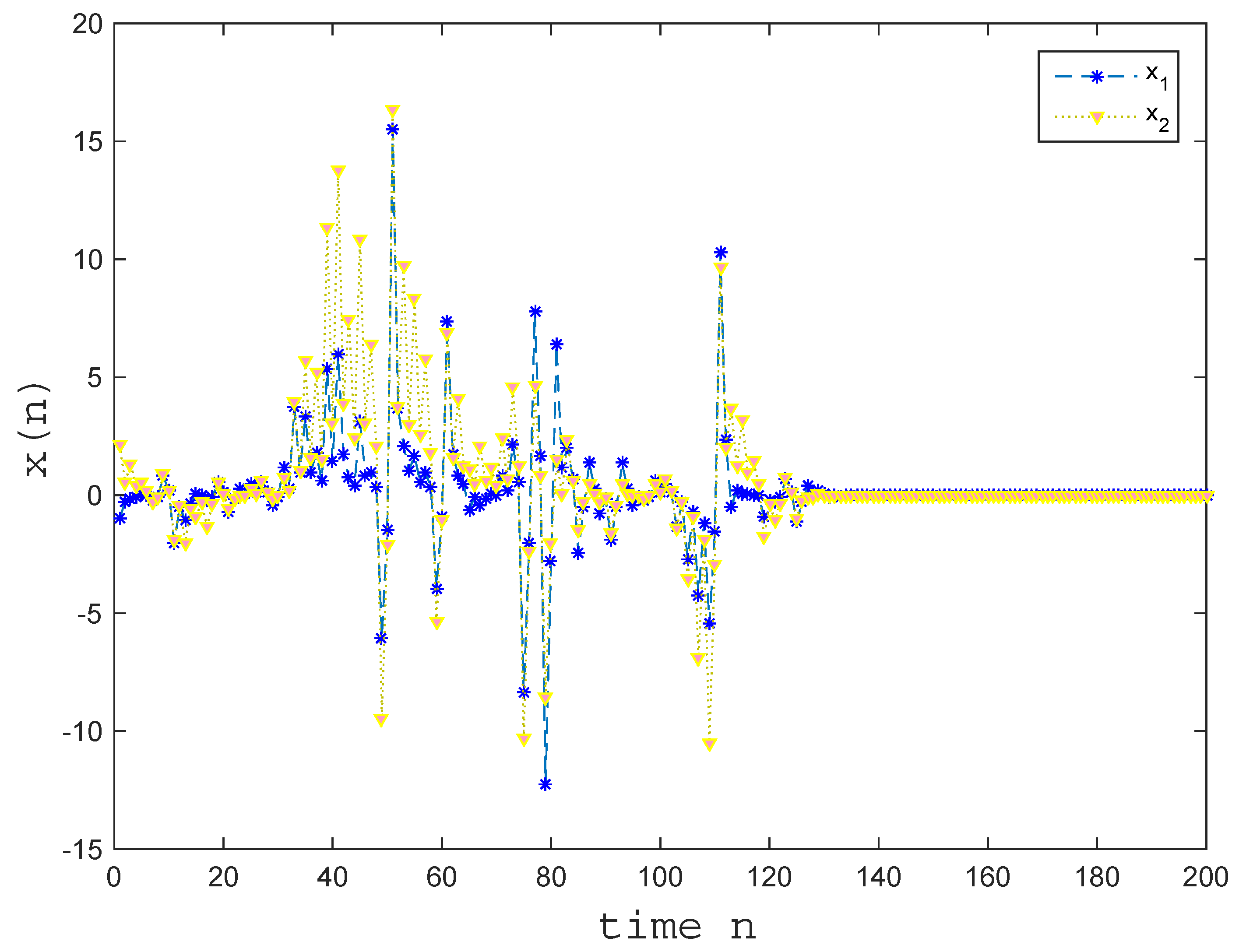
4. Examples
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bainov, D.; Simeonov, P. Systems with Impulse Effect: Stability, Theory, and Applications; Ellis Horwood: Chichester, UK, 1989. [Google Scholar]
- Lakshmikantham, V.; Bainov, D.; Simeonov, P. Theory of Impulsive Differential Equations; World Scientific: Singapore, 1989. [Google Scholar]
- Haddad, W.M.; Chellaboina, V.; Nersesov, S.G. Impulsive and Hybrid Dynamical Systems: Stability, Dissipativity, and Control, Princeton Series in Applied Mathematics; Princeton University Press: Princeton, NJ, USA, 2006. [Google Scholar]
- Mao, X.R. Stochastic Differential Equations and Applications; Horwood Publishing Limited: Oxford, UK, 1997. [Google Scholar]
- Li, X.D.; Zhu, Q.X.; Donal, O. pth Moment exponential stability of impulsive stochastic functional differential equations and application to control problems of NNs. J. Frankl. Inst. 2014, 351, 4435–4456. [Google Scholar] [CrossRef]
- Peng, S.G.; Jia, B.G. Some criteria on pth moment stability of impulsive stochastic functional differential equations. Stat. Probab. Lett. 2010, 80, 1085–1092. [Google Scholar] [CrossRef]
- Cheng, P.; Deng, F.Q.; Yao, F.Q. Exponential stability analysis of impulsive stochastic functional differential systems with delayed impulses. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 2104–2114. [Google Scholar] [CrossRef]
- Li, X.D.; Song, S.J. Stabilization of delay systems: Delay-dependent impulsive control. IEEE Trans. Autom. Control. 2017, 62, 406–411. [Google Scholar] [CrossRef]
- Li, B. Stability of stochastic functional differential equations with impulses by an average approach. Nonlinear Anal. Hybrid Syst. 2018, 29, 221–233. [Google Scholar] [CrossRef]
- Liu, J.; Liu, X.Z.; Xie, W.C. Impulsive stabilization of stochastic functional differential equations. Appl. Math. Lett. 2011, 24, 264–269. [Google Scholar] [CrossRef] [Green Version]
- Fu, X.Z.; Zhu, Q.X.; Guo, Y.X. Stabilization of stochastic functional differential systems with delayed impulses. Appl. Math. Comput. 2019, 346, 776–789. [Google Scholar] [CrossRef]
- Cao, W.P.; Zhu, Q.X. Razumikhin-type theorem for pth exponential stability of impulsive stochastic functional differential equations based on vector Lyapunov function. Nonlinear Anal. Hybrid Syst. 2021. [Google Scholar] [CrossRef]
- Liu, B. Stability of solutions for stochastic impulsive systems via comparison approach. IEEE Trans. Automat. Control. 2008, 53, 2128–2133. [Google Scholar] [CrossRef]
- Cheng, P.; Deng, F.Q. Global exponential stability of impulsive stochastic functional differential systems. Stat. Probab. Lett. 2010, 80, 1854–1862. [Google Scholar] [CrossRef]
- Wang, Y.H.; Li, X.D. Impulsive observer and impulsive control for time-delay systems. J. Frankl. Inst. 2020, 357, 8529–8542. [Google Scholar] [CrossRef]
- Pan, L.J.; Cao, J.D. Exponential stability of impulsive stochastic functional differential equations. J. Math. Anal. Appl. 2011, 382, 672–685. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.L.; Gaans, O.V.; Lunel, S.V. Existence and exponential stability of a class of impulsive neutral stochastic partial differential equations with delays and Poisson jumps. Stat. Probab. Lett. 2018, 141, 7–18. [Google Scholar] [CrossRef]
- Zhou, B.; Zhao, T.R. On asymptotic stability of discrete-time linear time-varying systems. IEEE Trans. Autom. Control. 2017, 62, 4274–4281. [Google Scholar] [CrossRef]
- Zhou, B. On asymptotic stability of linear time-varying systems. Automatica 2016, 68, 266–276. [Google Scholar] [CrossRef]
- Zhang, Y. Global exponential stability of delay difference equations with delayed impulses. Math. Comput. Simul. 2017, 132, 183–194. [Google Scholar] [CrossRef]
- Zong, X.F.; Lei, D.C.; Wu, F.X. Discrete Razumikhin-type stability theorems for stochastic discrete-time delay systems. J. Frankl. Inst. 2018, 355, 8245–8265. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, J.T.; Gang, F. Impulsive control of discrete systems with time delay. IEEE Trans. Autom. Control. 2009, 54, 830–834. [Google Scholar] [CrossRef]
- Dai, Z.L.; Xu, L.G.; Shu, Z.; Sam, G. Attracting sets of discrete-time Markovian jump delay systems with stochastic disturbances via impulsive control. J. Frankl. Inst. 2020, 357, 9781–9810. [Google Scholar] [CrossRef]
- Liu, B.; Hill, D.J.; Sun, Z.J. Input-to-state exponents and related ISS for delayed discrete-time systems with application to impulsive effects. Int. J. Robust Nonlinear Control. 2018, 28, 640–660. [Google Scholar] [CrossRef]
- Pan, S.T.; Sun, J.T.; Zhao, S.W. Stabilization of discrete-time Markovian jump linear systems via time-delayed and impulsive controllers. Automatica 2008, 44, 2954–2958. [Google Scholar] [CrossRef]
- Cai, T.; Cheng, P. Exponential stability theorems for discrete-time impulsive stochastic systems with delayed impulses. J. Frankl. Inst. 2020, 357, 1253–1279. [Google Scholar] [CrossRef]
- Wang, P.F.; Wang, W.Y.; Su, H.; Feng, J.Q. Stability of stochastic discrete-time piecewise homogeneous Markov jump systems with time delay and impulsive effects. Nonlinear Anal. Hybrid Syst. 2020. [Google Scholar] [CrossRef]
- Ning, Z.P.; Zhang, L.X.; Ali, M.P.C. Stability analysis and stabilization of discrete-time non-homogeneous semi-Markov jump linear systems: A polytopic approach. Automatia 2020. [Google Scholar] [CrossRef]
- Dong, Y.L.; Wang, H.M. Robust output feedback stabilization for uncertain discrete-time stochastic neural networks with time-varying delay. Neural Process. Lett. 2020, 51, 83–103. [Google Scholar] [CrossRef]
- Niu, B.; Karimi, H.R.; Wang, H.; Liu, Y. Adaptive output-feedback controller dasign for switched nonlinear stochastic systems with a modified average dwell-time method. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 1371–1382. [Google Scholar] [CrossRef]
- Peng, S.P.; Deng, F.Q.; Zhang, Y. A unified Razumikhin-type criterion on input-to-state stability of time-varying impulsive delayed systems. Syst. Control Lett. 2018, 116, 20–26. [Google Scholar] [CrossRef]
- Hespanha, J.P.; Liberzon, D.; Teel, A.R. Lypunov conditions for input-to-state stability of impulsive systems. Automatica 2008, 44, 857–869. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Ho, D.W.C.; Cao, J. A unified synchronization criterion for impulsive dynamical networks. Automatica 2010, 46, 1215–1221. [Google Scholar] [CrossRef]
- Li, X.D.; Li, P. Stability of time-delay systems with impulsive control involving stabilizing delays. Automatica 2021. [Google Scholar] [CrossRef]
- Zhang, Y. Exponential stability of impulsive discrete systems with time delays. Appl. Math. Lett. 2012, 25, 2290–2297. [Google Scholar] [CrossRef] [Green Version]
- Wu, F.K.; Mao, X.R.; Kloeden, P.E. Discrete Razumikhin-type technique and stability of the Euler-Maruyama method to stochastic functional differential equations. Discret. Contin. Dyn. Syst. 2013, 33, 885–903. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, T.; Cheng, P. Stability Analysis of Discrete-Time Stochastic Delay Systems with Impulses. Mathematics 2021, 9, 418. https://doi.org/10.3390/math9040418
Cai T, Cheng P. Stability Analysis of Discrete-Time Stochastic Delay Systems with Impulses. Mathematics. 2021; 9(4):418. https://doi.org/10.3390/math9040418
Chicago/Turabian StyleCai, Ting, and Pei Cheng. 2021. "Stability Analysis of Discrete-Time Stochastic Delay Systems with Impulses" Mathematics 9, no. 4: 418. https://doi.org/10.3390/math9040418





