Third Zadeh’s Intuitionistic Fuzzy Implication ^†

Abstract

George Klir and Bo Yuan named after Lotfi Zadeh the implication $p\to q=max(1-p,min(p,q))$ (also Early Zadeh implication). In a series of papers, the author introduced two intuitionistic fuzzy forms of Zadeh’s implication and studied their basic properties. In the present paper, a new (third) intuitionistic fuzzy form of Zadeh’s implication is proposed and some of its properties are studied.

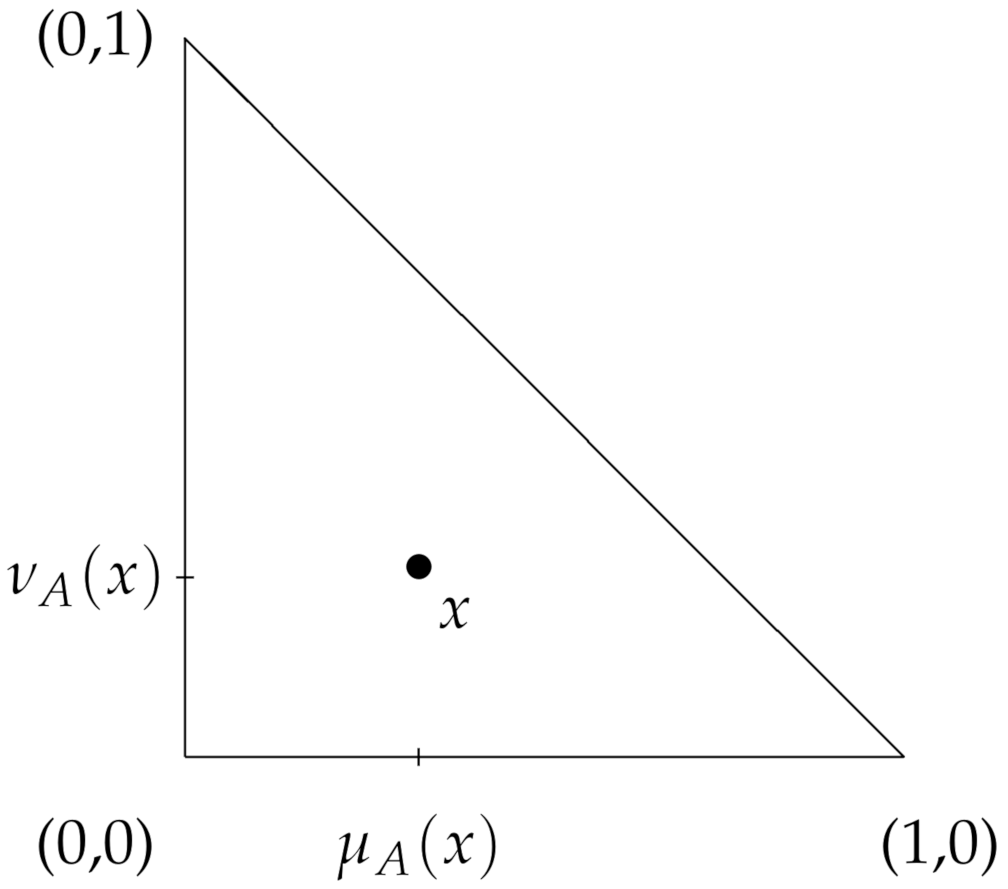
1. Introduction

In the present article, a new operation “implication” over intuitionistic fuzzy sets is introduced. It is based on the definition of the fuzzy implication, proposed by George Klir and Bo Yuan [1] and named after Lotfi Zadeh (also Early Zadeh implication), which has the form

$$p\to q=max(1-p,min(p,q))$$

see also [2], as well as on the two previous intuitionistic fuzzy implications introduced by the author [3,4] also inspired by the Zadeh implication.

In the beginning, the necessary concepts from intuitionistic fuzzy set theory will be given.

Let a set E be fixed. The intuitionistic fuzzy set (IFS; see [5,6]) A in E is defined by:

$$A=\{\langle x,{\mu }_A(x),{\nu }_A(x)\rangle |x\in E\},$$

where functions ${\mu }_A:E\to [0,1]$ and ${\nu }_A:E\to [0,1]$ define the degree of membership and the degree of non-membership of the element $x\in E$, respectively, and for every $x\in E$:

$$0\le {\mu }_A(x)+{\nu }_A(x)\le 1.$$

The two Zadeh’s intuitionistic fuzzy implications have the forms:

$$\begin{array}{cc}\hfill A{\to }_{Z,1}B=& \{\langle x,max({\nu }_A(x),min({\mu }_A(x),{\mu }_B(x))),\hfill \\ & min({\mu }_A(x),{\nu }_B(x))\rangle |x\in E\}.\hfill \end{array}$$

see [3] and

$$\begin{array}{cc}\hfill A{\to }_{Z,2}B=& \{\langle x,max({\nu }_A(x),min({\mu }_A(x),{\mu }_B(x))),\hfill \\ & min({\mu }_A(x),max({\nu }_A(x),{\nu }_B(x)))\rangle |x\in E\}\hfill \end{array}$$

see [4,7]. In [6,7,8] the first Zadeh’s intuitionistic fuzzy implication is assigned with number 1 and in [7,8] the second Zadeh’s intuitionistic fuzzy implication is labelled with number 166. As of 2012 [6], there are definitions of 138 intuitionistic fuzzy implications, in 2017 [7], they already amount to 185, and in 2019 [8], the list is further extended to 190. Among the new 52 implications, published after 2012, 22 of them were introduced by L. Atanassova in [9,10,11,12,13,14] and 3 were proposed by P. Dworniczak in [15,16,17]. All other intuitionistic fuzzy implications are introduced by the author, in some cases in collaboration with B. Riečan, E. Szmidt, J. Kacprzyk, N. Angelova and V. Atanassova. The 190 intuitionistic fuzzy implications from [8] generate 55 different intuitionistic fuzzy negations. An up-to-date list of the existing intuitionistic fuzzy implications is available online at [18].

Below we will call them, respectively, “First” and “Second Zadeh’s intuitionistic fuzzy implication”.

Let for every $x\in E$:

$${\pi }_A(x)=1-{\mu }_A(x)-{\nu }_A(x).$$

Therefore, function $\pi$ determines the degree of uncertainty.

Let us define the empty IFS, the totally uncertain IFS, and the unit IFS (see [5,6]), respectively, by:

$$\begin{array}{cc}\hfill O^{\ast }& =\{\langle x,0,1\rangle |x\in E\},\hfill \\ \hfill U^{\ast }& =\{\langle x,0,0\rangle |x\in E\},\hfill \\ \hfill E^{\ast }& =\{\langle x,1,0\rangle |x\in E\}.\hfill \end{array}$$

The geometrical interpretation of an element $x\in E$ with degrees ${\mu }_A(x)$ and ${\nu }_A(x)$ is shown in Figure 1 (see [5,6]).

An IFS A is called intuitionistic fuzzy tautological set (IFTS) if and only if (iff) for every $x\in E$

$${\mu }_A(x)\ge {\nu }_A(x)$$

and it is called tautological set iff for every $x\in E$: ${\mu }_A(X)=1,{\nu }_A(x)=0.$

For two IFSs A and B:

$$A\subseteq B \mathrm{i}\mathrm{f}\mathrm{f} (\forall x\in E)({\mu }_A(x)\le {\mu }_B(x)\&{\nu }_A(x)\ge {\nu }_B(x)).$$

Therefore, for every two IFSs A and B:

$$A{\to }_{Z,2}B\subseteq A{\to }_{Z,1}B.$$

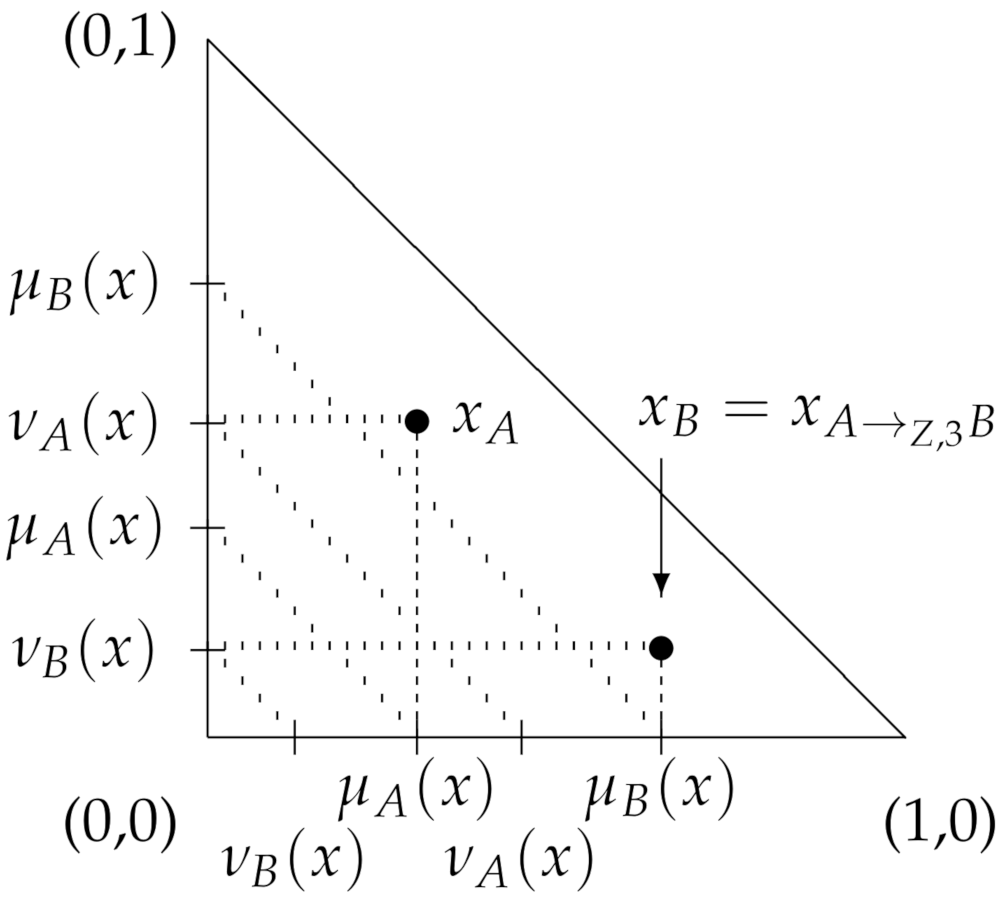
2. Main Results

Let us have two IFSs

$$A=\{\langle x,{\mu }_A(x),{\nu }_A(x)\rangle |x\in E\},$$

and

$$B=\{\langle x,{\mu }_B(x),{\nu }_B(x)\rangle |x\in E\}.$$

Now, we introduce the new (third) Zadeh’s intuitionistic fuzzy implication with the form:

$$\begin{array}{cc}\hfill A{\to }_{Z,3}B=& \{\langle x,max({\mu }_B(x),min({\nu }_A(x),{\nu }_B(x))),\hfill \\ & min({\nu }_B(x),max({\mu }_A(x),{\mu }_B(x)))\rangle |x\in E\}.\hfill \end{array}$$

First, we check that the definition is correct.

Obviously, the membership part

$$0\le max({\mu }_B(x),min({\nu }_A(x),{\nu }_B(x)))\le 1$$

and the non-membership part

$$0\le min({\nu }_B(x),max({\mu }_A(x),{\mu }_B(x)))\le 1.$$

Let

$$X\equiv max({\mu }_B(x),min({\nu }_A(x),{\nu }_B(x)))+min({\nu }_B(x),max({\mu }_A(x),{\mu }_B(x))).$$

We must check the following cases.

Case 1.

Let ${\mu }_A(x)\le {\mu }_B(x)$. Then

$$X=max({\mu }_B(x),min({\nu }_A(x),{\nu }_B(x)))+min({\nu }_B(x),{\mu }_B(x)).$$

1.1.

If ${\nu }_A(x)\le {\nu }_B(x)$, then

$$X=max({\mu }_B(x),{\nu }_A(x))+min({\nu }_B(x),{\mu }_B(x)).$$

1.1.1.

If ${\nu }_A(x)\le {\mu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\mu }_B(x)+min({\nu }_B(x),{\mu }_B(x))\hfill \\ \hfill & \le {\mu }_B(x)+{\nu }_B(x)\le 1.\hfill \end{array}$$

1.1.2.

If ${\nu }_A(x)>{\mu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\nu }_A(x)+min({\nu }_B(x),{\mu }_B(x))\le {\nu }_A(x)+{\mu }_B(x)\hfill \\ \hfill & \le {\nu }_B(x)+{\mu }_B(x)\le 1\hfill \end{array}$$

(by the assumption in 1.1).

1.2.

If ${\nu }_A(x)>{\nu }_B(x)$, then

$$\begin{array}{cc}\hfill X& =max({\mu }_B(x),{\nu }_B(x))+min({\nu }_B(x),{\mu }_B(x))\hfill \\ \hfill & ={\mu }_B(x)+{\nu }_B(x)\le 1.\hfill \end{array}$$

Case 2.

Let ${\mu }_A(x)>{\mu }_B(x)$. Then

$$X=max({\mu }_B(x),min({\nu }_A(x),{\nu }_B(x)))+min({\nu }_B(x),{\mu }_A(x)).$$

2.1.

If ${\nu }_A(x)\le {\nu }_B(x)$, then

$$X=max({\mu }_B(x),{\nu }_A(x))+min({\nu }_B(x),{\mu }_A(x)).$$

2.1.1.

If ${\nu }_A(x)\le {\mu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\mu }_B(x)+min({\nu }_B(x),{\mu }_A(x))\hfill \\ \hfill & \le {\mu }_B(x)+{\nu }_B(x)\le 1.\hfill \end{array}$$

2.1.2.

If ${\nu }_A(x)>{\mu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\nu }_A(x)+min({\nu }_B(x),{\mu }_A(x))\hfill \\ \hfill & \le {\nu }_A(x)+{\mu }_A(x)\le 1.\hfill \end{array}$$

2.2.

If ${\nu }_A(x)>{\nu }_B(x)$, then

$$X=max({\mu }_B(x),{\nu }_B(x))+min({\nu }_B(x),{\mu }_A(x)).$$

2.2.1.

If ${\mu }_B(x)\le {\nu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\nu }_B(x)+min({\nu }_B(x),{\mu }_A(x))\hfill \\ \hfill & <{\nu }_A(x)+{\mu }_A(x)\le 1\hfill \end{array}$$

(by the assumption in 2.2).

2.2.2.

If ${\mu }_B(x)>{\nu }_B(x)$, then

$$\begin{array}{cc}\hfill X& ={\mu }_B(x)+min({\nu }_B(x),{\mu }_A(x))\hfill \\ \hfill & \le {\mu }_B(x)+{\nu }_B(x)\le 1.\hfill \end{array}$$

Therefore, the operation is defined correctly.

Now, we can see that there is not a relation between the third Zadeh’s implication and each one of the first two Zadeh’s implications. Really, if the universe is $E=\{x\}$ and $A=\{\langle x,0,\frac{1}{4}\rangle \},$ $B=\{\langle x,\frac{1}{2},\frac{1}{2}\rangle \}$, then

$$A{\to }_{Z,1}B=\{\langle x,max(\frac{1}{4},min(0,\frac{1}{2})),min(0,\frac{1}{2})\rangle \}=\{\langle x,\frac{1}{4},0\rangle \}$$

while

$$A{\to }_{Z,3}B=\{\langle x,max(\frac{1}{2},min(\frac{1}{4},\frac{1}{2})),min(\frac{1}{2},max(0,\frac{1}{2}))\rangle \}=\{\langle x,\frac{1}{2},\frac{1}{2}\rangle \},$$

i.e., both sets are not comparable.

The new implication generates the classical negation of IFS A, because

$$\neg A=A{\to }_{Z,3}{O}^{\ast }=\{\langle x,{\nu }_A(x),{\mu }_A(x)\rangle |x\in E\}$$

(cf. [5,6]).

We can check directly that

$$\begin{array}{cc}\hfill A{\to }_{Z,3}{U}^{\ast }& =\{\langle x,max(0,min({\nu }_A(x),0)),min(0,max({\mu }_A(x),0))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,0,0\rangle |x\in E\}=U^{\ast },\hfill \\ \hfill A{\to }_{Z,3}{E}^{\ast }& =\{\langle x,max(1,min({\nu }_A(x),0)),min(0,max({\mu }_A(x),1))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,1,0\rangle |x\in E\}=E^{\ast },\hfill \\ \hfill O^{\ast }{\to }_{Z,3}B& =\{\langle x,max({\mu }_B(x),min(1,{\nu }_B(x))),min({\nu }_B(x),max(0,{\mu }_B(x)))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,max({\mu }_B(x),{\nu }_B(x)),min({\mu }_B(x),{\nu }_B(x)))\rangle |x\in E\},\hfill \end{array}$$

$$\begin{array}{cc}\hfill E^{\ast }{\to }_{Z,3}B& =\{\langle x,max({\mu }_B(x),min(0,{\nu }_B(x))),min({\nu }_B(x),max(1,{\mu }_B(x)))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,{\mu }_B(x),{\nu }_B(x)\rangle |x\in E\}=B,\hfill \\ \hfill U^{\ast }{\to }_{Z,3}B& =\{\langle x,max({\mu }_B(x),min(0,{\nu }_B(x))),min({\nu }_B(x),max(0,{\mu }_B(x)))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,{\mu }_B(x),min({\mu }_B(x),{\nu }_B(x))\rangle |x\in E\}.\hfill \end{array}$$

In the particular case, we have

$$O^{\ast }{\to }_{Z,3}{O}^{\ast }=E^{\ast },$$

$$O^{\ast }{\to }_{Z,3}{U}^{\ast }=U^{\ast },$$

$$O^{\ast }{\to }_{Z,3}{E}^{\ast }=E^{\ast },$$

$$U^{\ast }{\to }_{Z,3}{O}^{\ast }=U^{\ast },$$

$$U^{\ast }{\to }_{Z,3}{U}^{\ast }=U^{\ast },$$

$$U^{\ast }{\to }_{Z,3}{E}^{\ast }=E^{\ast },$$

$$E^{\ast }{\to }_{Z,3}{O}^{\ast }=O^{\ast },$$

$$E^{\ast }{\to }_{Z,3}{U}^{\ast }=U^{\ast },$$

$$E^{\ast }{\to }_{Z,3}{E}^{\ast }=E^{\ast }.$$

Four different geometrical interpretations of the element $x\in E$ in IFSs A and B, i.e., with degrees ${\mu }_A(x)$ and ${\nu }_A(x)$, and ${\mu }_B(x)$ and ${\nu }_B(x)$; and the element x from IFS $A{\to }_{Z,3}B$, are shown in Figure 2, Figure 3, Figure 4 and Figure 5. These four scenarios are related to the locations of the element x in A and B. Let us denote the element x in A by $x_A$, in B—by $x_B$, and in $A{\to }_{Z,3}B$—by $x_{A{\to }_{Z,3}B}$.

Nine axioms for implications are introduced in [1]. They are the following:

Axiom 1.$(\forall x,y)(x\le y\to (\forall z)(I(x,z)\ge I(y,z)))$.

Axiom 2.$(\forall x,y)(x\le y\to (\forall z)(I(z,x)\le I(z,y)))$.

Axiom 3.$(\forall y)(I(0,y)=1)$.

Axiom 4.$(\forall y)(I(1,y)=y)$.

Axiom 5.$(\forall x)(I(x,x)=1)$.

Axiom 6.$(\forall x,y,z)(I(x,I(y,z))=I(y,I(x,z)))$.

Axiom 7.$(\forall x,y)(I(x,y)=1$ iff $x\le y)$.

Axiom 8.$(\forall x,y)(I(x,y)=I(N(y),N(x)))$, where N is an operation for a negation.

Axiom 9.I is a continuous function.

Following [6], we will mention that if the axiom is valid as an intuitionistic fuzzy tautology (IFT), that axiom is marked with an asterisk (${}^{\ast }$). Such are the axioms:

Axiom 3*.$(\forall y)(I(0,y)$ is an IFT).

Axiom 5*.$(\forall x)(I(x,x)$ is an IFT).

Axiom 7*.$(\forall x,y)(I(x,y)$ is an IFT iff $x\le y)$.

Theorem 1.

Implication ${\to }_{Z,3}$ satisfies Axioms 1 and 4.

Proof. 

Let $A,B$ and C be three IFSs. In [5,6], the relation ⊆ is defined by

$$A\subseteq B \mathrm{i}\mathrm{f}\mathrm{f} (\forall x\in E)({\mu }_A(x)\le {\mu }_B(x)\&{\nu }_A(x)\ge {\nu }_B(x)).$$

Let $A\subseteq B$. First, we determine

$$\begin{array}{cc}\hfill A{\to }_{Z,3}C=& \{\langle x,max({\mu }_C(x),min({\nu }_A(x),{\nu }_C(x))),\hfill \\ \hfill & min({\nu }_C(x),max({\mu }_A(x),{\mu }_C(x)))\rangle |x\in E\}\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill B{\to }_{Z,3}C=& \{\langle x,max({\mu }_C(x),min({\nu }_B(x),{\nu }_C(x))),\hfill \\ & min({\nu }_C(x),max({\mu }_B(x),{\mu }_C(x)))\rangle |x\in E\}.\hfill \end{array}$$

Now, we see that for each $x\in E$:

$$max({\mu }_C(x),min({\nu }_A(x),{\nu }_C(x)))-max({\mu }_C(x),min({\nu }_B(x),{\nu }_C(x)))$$

$$\ge max({\mu }_C(x),min({\nu }_B(x),{\nu }_C(x)))-max({\mu }_C(x),min({\nu }_B(x),{\nu }_C(x)))=0,$$

(from ${\nu }_A(x)\ge {\nu }_B(x)$)

and

$$min({\nu }_C(x),max({\mu }_B(x),{\mu }_C(x)))-min({\nu }_C(x),max({\mu }_A(x),{\mu }_C(x)))$$

$$\ge min({\nu }_C(x),max({\mu }_A(x),{\mu }_C(x)))-min({\nu }_C(x),max({\mu }_A(x),{\mu }_C(x)))=0.$$

(from ${\mu }_A(x)\le {\mu }_B(x)$).

Therefore, Axiom 1 is valid.

From $E^{\ast }{\to }_{Z,3}A=A$ that we checked above, it follows that Axiom 4 is valid, too.

Since functions max and min are continuous, Axiom 9 is valid. For the rest axioms we can construct counterexamples. For example, for the universe $E=\{x\}$ and the IFSs $A=\{\langle x,0,1\rangle \},$ $B=\{\langle x,0,\frac{1}{2}\rangle \},C=\{\langle x,0,1\rangle \}$ it follows that $A\subset B$ (strong inclusion), but

$$C{\to }_{Z,3}A=\{\langle x,1,0\rangle |x\in E\}\neg \subseteq \{\langle x,\frac{1}{2},0\rangle |x\in E\}=C{\to }_{Z,3}B.$$

Therefore, Axiom 2 is not valid. In the proofs of Theorem 2, we will construct two other counterexamples. □

Theorem 2.

Implication ${\to }_{Z,3}$ satisfies Axioms 3* and 5*, but it does not satisfy Axioms 3 and 5, i.e., in the forms $O^{\ast }{\to }_{Z,3}A$ and $A{\to }_{Z,3}A$ are IFTSs, but $O^{\ast }{\to }_{Z,3}A=E^{\ast }$ and $A{\to }_{Z,3}A=E^{\ast }$ are not tautological sets.

Proof. 

Let A be an IFS. Then for Axiom 3*, as we checked above,

$$O^{\ast }{\to }_{Z,3}A=\{\langle x,max({\mu }_A(x),{\nu }_A(x)),min({\nu }_A(x),{\mu }_A(x))\rangle |x\in E\}$$

that is an IFTS, but, e.g., for ${\mu }_A(x)={\nu }_A(x)=0.5$,

$$A{\to }_{Z,3}A=\{\langle x,0.5,0.5\rangle |x\in E\}$$

is not a tautological set, i.e., Axiom 3 is not valid.

Analogously, for Axiom 5* we have

$$\begin{array}{cc}\hfill A{\to }_{Z,3}A& =\{\langle x,max({\mu }_A(x),min({\nu }_A(x),{\nu }_A(x))),\hfill \\ \hfill & min({\nu }_A(x),max({\mu }_A(x),{\mu }_A(x)))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,max({\mu }_A(x),min({\nu }_A(x),{\nu }_A(x))),\hfill \\ \hfill & min({\nu }_A(x),max({\mu }_A(x),{\mu }_A(x)))\rangle |x\in E\}\hfill \\ \hfill & =\{\langle x,max({\mu }_A(x),{\nu }_A(x)),min({\nu }_A(x),{\mu }_A(x))\rangle |x\in E\},\hfill \end{array}$$

that is an IFTS, but, e.g., for ${\mu }_A(x)={\nu }_A(x)=0.5$,

$$A{\to }_{Z,3}A=\{\langle x,0.5,0.5\rangle |x\in E\}$$

is not a tautological set, i.e., Axiom 5 is not valid. □

To the proofs of both theorems, we can add also that Axiom 7* is not valid (and hence, Axiom 7, too), because the counterexample with the universe $E=\{x\}$ and the IFSs $A=\{\langle x,\frac{1}{2},\frac{1}{2}\rangle \},$ $B=\{\langle x,0,1\rangle \}$. In this case $B\subset A$ (strong inclusion), while

$$A{\to }_{Z,3}B=\{\langle x,\frac{1}{2},\frac{1}{2}\rangle \}$$

is and IFTS.

Now, we will discuss the axioms of the intuitionistic logic (see e.g., [19]).

Theorem 3.

For every three IFSs A, B and C the IFSs

(a) $A\to A,$

(b) $A\to (B\to A),$

(c) $A\to (B\to (A\cap B)),$

(d) $(A\to (B\to C))\to (B\to (A\to C)),$

(e) $(A\to (B\to C))\to ((A\to B)\to (A\to C)),$

(f) $A\to \neg \neg A,$

(g) $\neg (A\cap \neg A),$

(h) $(\neg A\cup B)\to (A\to B),$

(i) $\neg (A\cup B)\to (\neg A\cap \neg B),$

(j) $(\neg A\cap \neg B)\to \neg (A\cup B),$

(k) $(\neg A\cup \neg B)\to \neg (A\cap B),$

(l) $(A\to B)\to (\neg B\to \neg A),$

(m) $(A\to \neg B)\to (B\to \neg A),$

(n) $\neg \neg \neg A\to \neg A,$

(o) $\neg A\to \neg \neg \neg A,$

(p) $\neg \neg (A\to B)\to (A\to \neg \neg B),$

(q) $(C\to A)\to ((C\to (A\to B))\to (C\to B))$

are IFTSs, but none of these is a tautological set.

The proof of this theorem is similar to the above one.

3. Conclusions

Starting with [3], in a series of papers a lot of intuitionistic fuzzy implications were introduced and some of their basic properties were studied. All 190 implications are given in [8]. In future, these properties will be checked for the new implication, too. In [20,21,22], for each intuitionistic fuzzy implication, one or three intuitionistic fuzzy disjunctions and conjunctions are introduced. For example, for disjunction, the following formulas are used:

$$p{\vee }_{1}q=\neg p\to q,$$

$$p{\vee }_{2}q=\neg p\to \neg \neg q,$$

where the operation intuitionistic fuzzy negation (¬) is generated by the respective intuitionistic fuzzy implication (→);

$$p{\vee }_{3}q=\neg p\to q,$$

where the negation ¬ is the classical one. Therefore, in the present case, the three disjunctions coincide, because the negation in the three formulas is only one.

In a similar way, the three different conjunctions are defined. In further research, new conjunctions and disjunctions will be introduced using the above formulas and based on the third Zadeh’s intuitionistic fuzzy implication. Applications of this new implication can be sought in various decision making procedures involving uncertainty such as intuitionistic fuzzy based expert systems.