Performance Evaluation of Hospitals under Data Uncertainty: An Uncertain Common-Weights Data Envelopment Analysis
Abstract
1. Introduction
2. Literature Review
3. Uncertainty Theory
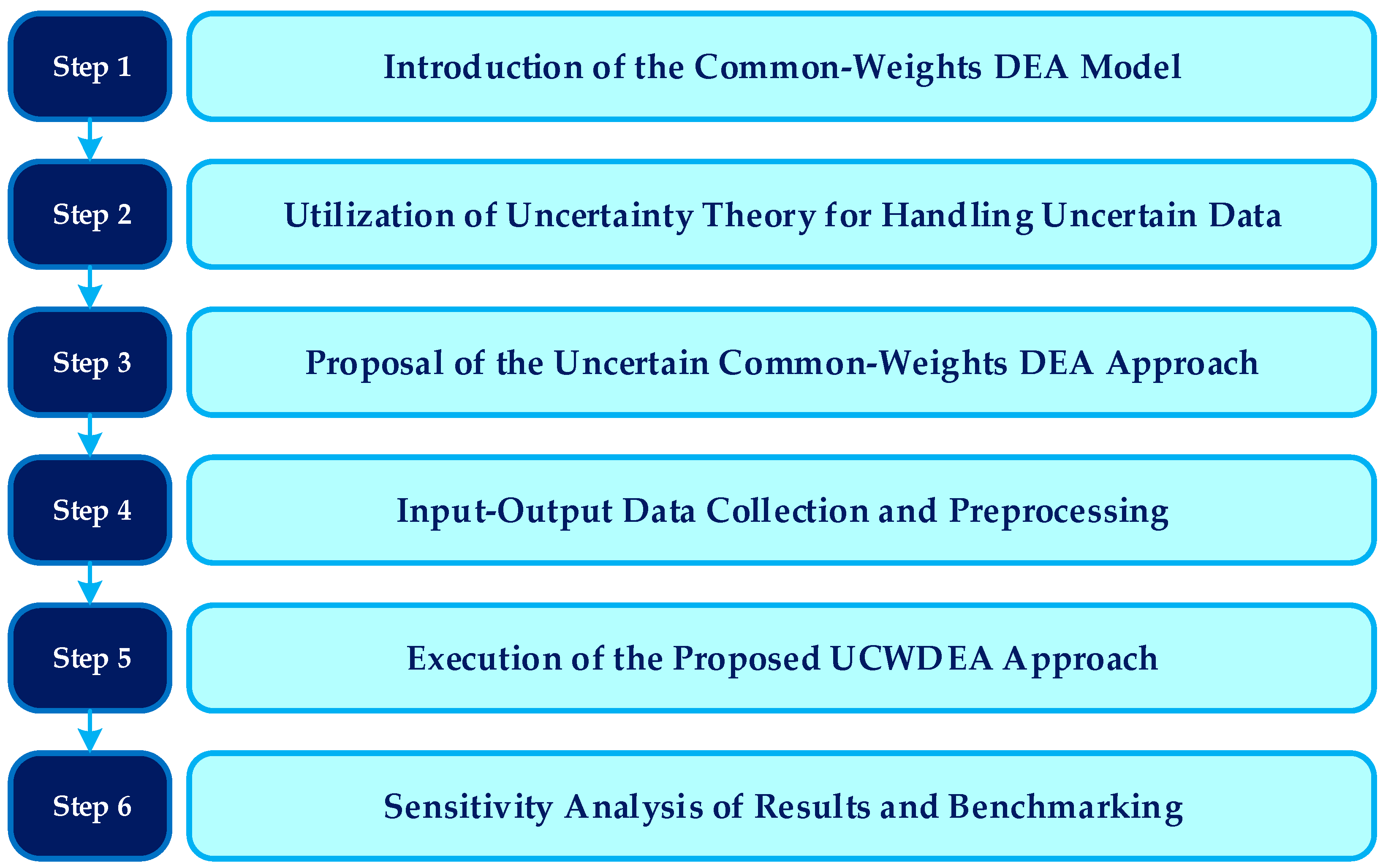
4. The Proposed UCWDEA Approach
5. Case Study and Experimental Results
6. Conclusions and Future Research Directions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Grosskopf, S.; Valdmanis, V. Measuring hospital performance: A non-parametric approach. J. Health Econ. 1987, 6, 89–107. [Google Scholar] [CrossRef] [PubMed]
- Kirigia, J.M.; Emrouznejad, A.; Cassoma, B.; Asbu, E.Z.; Barry, S. A performance assessment method for hospitals: The case of municipal hospitals in Angola. J. Med. Syst. 2008, 32, 509–519. [Google Scholar] [CrossRef] [PubMed]
- Moshiri, H.; Aljunid, S.M.; Amin, R.M. Hospital efficiency: Concept, measurement techniques and review of hospital efficiency studies. Malays. J. Public Health Med. 2010, 10, 35–43. [Google Scholar]
- Davis, P.; Milne, B.; Parker, K.; Hider, P.; Lay-Yee, R.; Cumming, J.; Graham, P. Efficiency, effectiveness, equity (E3). Evaluating hospital performance in three dimensions. Health Policy 2013, 112, 19–27. [Google Scholar] [CrossRef]
- Rahimi, H.; Khammar-Nia, M.; Kavosi, Z.; Eslahi, M. Indicators of hospital performance evaluation: A systematic review. Int. J. Hosp. Res. 2014, 3, 199–208. [Google Scholar]
- Nepomuceno, T.C.C.; Piubello Orsini, L.; de Carvalho, V.D.H.; Poleto, T.; Leardini, C. The core of healthcare efficiency: A comprehensive bibliometric review on frontier analysis of hospitals. Healthcare 2022, 10, 1316. [Google Scholar] [CrossRef]
- Wawo, A.B.; Dharmawati, T.; Anto, L.O.; Kalsum, U. Performance analysis of a public hospital in the COVID-19 pandemic using the balanced scorecard. Int. J. Innov. Creat. Chang. 2020, 14, 486–507. [Google Scholar]
- Gul, M.; Yucesan, M. Hospital preparedness assessment against COVID-19 pandemic: A case study in Turkish tertiary healthcare services. Math. Probl. Eng. 2021, 2021, 2931219. [Google Scholar] [CrossRef]
- Mirmozaffari, M.; Yazdani, R.; Shadkam, E.; Khalili, S.M.; Tavassoli, L.S.; Boskabadi, A. A novel hybrid parametric and non-parametric optimisation model for average technical efficiency assessment in public hospitals during and post-COVID-19 pandemic. Bioengineering 2021, 9, 7. [Google Scholar] [CrossRef]
- Mehdizadeh-Somarin, Z.; Salimi, B.; Tavakkoli-Moghaddam, R.; Hamid, M.; Zahertar, A. Performance assessment and improvement of a care unit for COVID-19 patients with resilience engineering and motivational factors: An artificial neural network method. Comput. Biol. Med. 2022, 149, 106025. [Google Scholar] [CrossRef]
- Rana, W.; Mukhtar, S.; Mukhtar, S. Job satisfaction, performance appraisal, reinforcement and job tasks in medical healthcare professionals during the COVID-19 pandemic outbreak. Int. J. Health Plan. Manag. 2022, 37, 2345–2353. [Google Scholar] [CrossRef]
- Adler, P.S.; Riley, P.; Kwon, S.W.; Signer, J.; Lee, B.; Satrasala, R. Performance improvement capability: Keys to accelerating performance improvement in hospitals. Calif. Manag. Rev. 2003, 45, 12–33. [Google Scholar] [CrossRef]
- Veillard, J.; Champagne, F.; Klazinga, N.; Kazandjian, V.; Arah, O.A.; Guisset, A.L. A performance assessment framework for hospitals: The WHO regional office for Europe PATH project. Int. J. Qual. Health Care 2005, 17, 487–496. [Google Scholar] [CrossRef] [PubMed]
- Chang, W.C.; Tung, Y.C.; Huang, C.H.; Yang, M.C. Performance improvement after implementing the Balanced Scorecard: A large hospital’s experience in Taiwan. Total Qual. Manag. 2008, 19, 1143–1154. [Google Scholar] [CrossRef]
- Groene, O.; Klazinga, N.; Kazandjian, V.; Lombrail, P.; Bartels, P. The World Health Organization Performance Assessment Tool for Quality Improvement in Hospitals (PATH): An analysis of the pilot implementation in 37 hospitals. Int. J. Qual. Health Care 2008, 20, 155–161. [Google Scholar] [CrossRef]
- Carter, R.E.; Lonial, S.C.; Raju, P.S. Impact of quality management on hospital performance: An empirical examination. Qual. Manag. J. 2010, 17, 8–24. [Google Scholar] [CrossRef]
- Buttigieg, S.C.; Pace, A.; Rathert, C. Hospital performance dashboards: A literature review. J. Health Organ. Manag. 2017, 31, 385–406. [Google Scholar] [CrossRef]
- Cantor, V.J.M.; Poh, K.L. Integrated analysis of healthcare efficiency: A systematic review. J. Med. Syst. 2018, 42, 8. [Google Scholar] [CrossRef]
- Lin, C.S.; Chiu, C.M.; Huang, Y.C.; Lang, H.C.; Chen, M.S. Evaluating the operational efficiency and quality of tertiary hospitals in Taiwan: The application of the EBITDA indicator to the DEA method and TOBIT regression. Healthcare 2021, 10, 58. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.S. Healthcare service efficiency: An empirical study on healthcare capacity in various counties and cities in Taiwan. Healthcare 2023, 11, 1656. [Google Scholar] [CrossRef]
- Nayar, P.; Ozcan, Y.A. Data envelopment analysis comparison of hospital efficiency and quality. J. Med. Syst. 2008, 32, 193–199. [Google Scholar] [CrossRef]
- O’Neill, L.; Rauner, M.; Heidenberger, K.; Kraus, M. A cross-national comparison and taxonomy of DEA-based hospital efficiency studies. Socio-Econ. Plan. Sci. 2008, 42, 158–189. [Google Scholar] [CrossRef]
- Kohl, S.; Schoenfelder, J.; Fügener, A.; Brunner, J.O. The use of data envelopment analysis (DEA) in healthcare with a focus on hospitals. Health Care Manag. Sci. 2019, 22, 245–286. [Google Scholar] [CrossRef] [PubMed]
- Zakowska, I.; Godycki-Cwirko, M. Data envelopment analysis applications in primary health care: A systematic review. Fam. Pract. 2020, 37, 147–153. [Google Scholar] [CrossRef]
- Mirmozaffari, M.; Kamal, N. The application of data envelopment analysis to emergency departments and management of emergency conditions: A narrative review. Healthcare 2023, 11, 2541. [Google Scholar] [CrossRef]
- Vörösmarty, G.; Dobos, I. Management applications and methodology developments in DEA-an overview of literature reviews. Int. J. Manag. Decis. Mak. 2023, 22, 472–491. [Google Scholar] [CrossRef]
- Charnes, A.; Cooper, W.W.; Rhodes, E. Measuring the Efficiency of Decision Making Units. Eur. J. Oper. Res. 1978, 2, 429–444. [Google Scholar] [CrossRef]
- Banker, R.D.; Charnes, A.; Cooper, W.W. Some Models for Estimating Technical and Scale Inefficiencies in Data Envelopment Analysis. Manag. Sci. 1984, 30, 1078–1092. [Google Scholar] [CrossRef]
- Liu, J.S.; Lu, L.Y.; Lu, W.M.; Lin, B.J. A survey of DEA applications. Omega 2013, 41, 893–902. [Google Scholar] [CrossRef]
- Emrouznejad, A.; Yang, G.L. A survey and analysis of the first 40 years of scholarly literature in DEA: 1978–2016. Soc.-Econ. Plan. Sci. 2018, 61, 4–8. [Google Scholar] [CrossRef]
- Peykani, P.; Mohammadi, E.; Farzipoor Saen, R.; Sadjadi, S.J.; Rostamy-Malkhalifeh, M. Data envelopment analysis and robust optimization: A review. Expert Syst. 2020, 37, e12534. [Google Scholar] [CrossRef]
- Peykani, P.; Farzipoor Saen, R.; Seyed Esmaeili, F.S.; Gheidar-Kheljani, J. Window data envelopment analysis approach: A review and bibliometric analysis. Expert Syst. 2021, 38, e12721. [Google Scholar] [CrossRef]
- Dyckhoff, H.; Souren, R. Integrating multiple criteria decision analysis and production theory for performance evaluation: Framework and review. Eur. J. Oper. Res. 2022, 297, 795–816. [Google Scholar] [CrossRef]
- Peykani, P.; Hosseinzadeh Lotfi, F.; Sadjadi, S.J.; Ebrahimnejad, A.; Mohammadi, E. Fuzzy chance-constrained data envelopment analysis: A structured literature review, current trends, and future directions. Fuzzy Optim. Decis. Mak. 2022, 21, 197–261. [Google Scholar] [CrossRef]
- Sinuany-Stern, Z. Foundations of Operations Research: From Linear Programming to Data Envelopment Analysis. Eur. J. Oper. Res. 2023, 306, 1069–1080. [Google Scholar] [CrossRef]
- Morita, H.; Seiford, L.M. Characteristics on stochastic DEA efficiency: Reliability and probability being efficient. J. Oper. Res. Soc. Jpn. 1999, 42, 389–404. [Google Scholar]
- Ebrahimnejad, A. Cost efficiency measures with trapezoidal fuzzy numbers in data envelopment analysis based on ranking functions: Application in insurance organization and hospital. Int. J. Fuzzy Syst. Appl. 2012, 2, 51–68. [Google Scholar] [CrossRef]
- Hatami-Marbini, A.; Tavana, M.; Emrouznejad, A. Productivity growth and efficiency measurements in fuzzy environments with an application to health care. Int. J. Fuzzy Syst. Appl. 2012, 2, 1–35. [Google Scholar] [CrossRef]
- Khaki, A.R.; Sadjadi, S.J.; Gharakhani, M.; Rashidi, S. Data envelopment analysis under uncertainty: A case study from public healthcare. Afr. J. Bus. Manag. 2012, 6, 7096–7105. [Google Scholar]
- Costantino, N.; Dotoli, M.; Epicoco, N.; Falagario, M.; Sciancalepore, F. Using cross-efficiency fuzzy Data Envelopment Analysis for healthcare facilities performance evaluation under uncertainty. In Proceedings of the IEEE International Conference on Systems, Man, and Cybernetics, Manchester, UK, 13–16 October 2013; pp. 912–917. [Google Scholar]
- De Nicola, A.; Gitto, S.; Mancuso, P. Evaluating Italian public hospital efficiency using bootstrap DEA and CART. Int. J. Appl. Decis. Sci. 2013, 6, 281–292. [Google Scholar] [CrossRef]
- Khodaparasti, S.; Maleki, H.R. A new combined dynamic location model for emergency medical services in fuzzy environment. In Proceedings of the 13th Iranian Conference on Fuzzy Systems, Qazvin, Iran, 27–29 August 2013; pp. 1–6. [Google Scholar]
- Kalantary, Z.; Azar, A. A robust data envelopment analysis model for ranking: A case of hospitals of Tehran. In Proceedings of the Data Envelopment Analysis and Performance Measurement: Proceedings of the 11th International Conference of DEA, Samsun, Turkey, 20–21 June 2014; pp. 21–28. [Google Scholar]
- Karadayi, M.A.; Karsak, E.E. Imprecise DEA framework for evaluating the efficiency of state hospitals in Istanbul. In Proceedings of the World Congress on Engineering, London, UK, 2–4 July 2014. [Google Scholar]
- Dotoli, M.; Epicoco, N.; Falagario, M.; Sciancalepore, F. A cross-efficiency fuzzy data envelopment analysis technique for performance evaluation of decision making units under uncertainty. Comput. Ind. Eng. 2015, 79, 103–114. [Google Scholar] [CrossRef]
- Haji-Sami, E.; Ebrahimi Khameneh, M.; Rezaeisaray, M.; Esmaeili, S. Robust DEA under discrete uncertain data: An application for Iranian hospital emergency. In Proceedings of the 8th International Conference of Iranian Operations Research Society, Mashhad, Iran, 21–25 May 2015. [Google Scholar]
- Kheirollahi, H.; Matin, B.K.; Mahboubi, M.; Alavijeh, M.M. Chance constrained input relaxation to congestion in stochastic DEA. An application to Iranian hospitals. Glob. J. Health Sci. 2015, 7, 151. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Mitropoulos, P.; Talias, Μ.A.; Mitropoulos, I. Combining stochastic DEA with Bayesian analysis to obtain statistical properties of the efficiency scores: An application to Greek public hospitals. Eur. J. Oper. Res. 2015, 243, 302–311. [Google Scholar] [CrossRef]
- Rabbani, M.; Heidari, N.; Farrokhi-Asl, H. A bootstrap interval robust data envelopment analysis for estimate efficiency and ranking hospitals. J. Ind. Eng. Manag. Stud. 2016, 3, 107–122. [Google Scholar]
- Shwartz, M.; Burgess Jr, J.F.; Zhu, J. A DEA based composite measure of quality and its associated data uncertainty interval for health care provider profiling and pay-for-performance. Eur. J. Oper. Res. 2016, 253, 489–502. [Google Scholar] [CrossRef]
- Arya, A.; Yadav, S.P. A fuzzy dual SBM model with fuzzy weights: An application to the health sector. In Proceedings of the 6th International Conference on Soft Computing for Problem Solving: SocProS, Patiala, India, 23 December 2017; Springer: Singapore, 2017; pp. 230–238. [Google Scholar]
- Karsak, E.E.; Karadayi, M.A. Imprecise DEA framework for evaluating health-care performance of districts. Kybernetes 2017, 46, 706–727. [Google Scholar] [CrossRef]
- Kheirollahi, H.; Hessari, P.; Charles, V.; Chawshini, R. An input relaxation model for evaluating congestion in fuzzy DEA. Croat. Oper. Res. Rev. 2017, 8, 391–408. [Google Scholar] [CrossRef]
- Otay, İ.; Oztaysi, B.; Onar, S.C.; Kahraman, C. Multi-expert performance evaluation of healthcare institutions using an integrated intuitionistic fuzzy AHP&DEA methodology. Knowl. Syst. 2017, 133, 90–106. [Google Scholar]
- Ahmadvand, S.; Pishvaee, M.S. An efficient method for kidney allocation problem: A credibility-based fuzzy common weights data envelopment analysis approach. Health Care Manag. Sci. 2018, 21, 587–603. [Google Scholar] [CrossRef]
- Peykani, P.; Seyed Esmaeili, F.S.; Rostamy-Malkhalifeh, M.; Hosseinzadeh Lotfi, F. Measuring productivity changes of hospitals in Tehran: The fuzzy Malmquist productivity index. Int. J. Hosp. Res. 2018, 7, 1–17. [Google Scholar]
- Wu, D.; Wu, D.D. Risk-based robust evaluation of hospital efficiency. IEEE Syst. J. 2018, 13, 1906–1914. [Google Scholar] [CrossRef]
- Hatefi, S.M.; Haeri, A. Evaluating hospital performance using an integrated balanced scorecard and fuzzy data envelopment analysis. Health Manag. Inf. Sci. 2019, 6, 66–76. [Google Scholar]
- Ji, A.B.; Qiao, Y.; Liu, C. Fuzzy DEA-based classifier and its applications in healthcare management. Health Care Manag. Sci. 2019, 22, 560–568. [Google Scholar] [CrossRef] [PubMed]
- Peykani, P.; Mohammadi, E.; Emrouznejad, A.; Pishvaee, M.S.; Rostamy-Malkhalifeh, M. Fuzzy data envelopment analysis: An adjustable approach. Expert Syst. Appl. 2019, 136, 439–452. [Google Scholar] [CrossRef]
- Arya, A.; Yadav, S.P. Performance efficiency of public health sector using intuitionistic fuzzy DEA. Int. J. Uncertain. Fuzziness Knowl. Syst. 2020, 28, 289–315. [Google Scholar] [CrossRef]
- Ghafari Someh, N.; Pishvaee, M.S.; Sadjadi, S.J.; Soltani, R. Sustainable efficiency assessment of private diagnostic laboratories under uncertainty: An interval data envelopment analysis approach. J. Model. Manag. 2020, 15, 1069–1103. [Google Scholar] [CrossRef]
- Yang, W.; Cai, L.; Edalatpanah, S.A.; Smarandache, F. Triangular single valued neutrosophic data envelopment analysis: Application to hospital performance measurement. Symmetry 2020, 12, 588. [Google Scholar] [CrossRef]
- Abdelfattah, W. Neutrosophic data envelopment analysis: An application to regional hospitals in Tunisia. Neutrosophic Sets Syst. 2021, 41, 89–105. [Google Scholar]
- Gómez-Gallego, J.C.; Gómez-Gallego, M.; García-García, J.F.; Faura-Martinez, U. Evaluation of the efficiency of European health systems using fuzzy data envelopment analysis. Healthcare 2021, 9, 1270. [Google Scholar] [CrossRef] [PubMed]
- Izadikhah, M.; Roostaee, R.; Emrouznejad, A. Fuzzy data envelopment analysis with ordinal and interval data. Int. J. Uncertain. Fuzziness Knowl. Syst. 2021, 29, 385–410. [Google Scholar] [CrossRef]
- Jahani, M.; Kordrostami, S. Sustainability assessment using a fuzzy DEA aggregation approach: A healthcare application. Soft Comput. 2021, 25, 10829–10849. [Google Scholar] [CrossRef]
- Tavana, M.; Nazari-Shirkouhi, S.; Farzaneh Kholghabad, H. An integrated quality and resilience engineering framework in healthcare with Z-number data envelopment analysis. Health Care Manag. Sci. 2021, 24, 768–785. [Google Scholar] [CrossRef] [PubMed]
- Izadikhah, M. A fuzzy stochastic slacks-based data envelopment analysis model with application to healthcare efficiency. Healthc. Anal. 2022, 2, 100038. [Google Scholar] [CrossRef]
- Omrani, H.; Alizadeh, A.; Emrouznejad, A.; Teplova, T. A robust credibility DEA model with fuzzy perturbation degree: An application to hospitals performance. Expert Syst. Appl. 2022, 189, 116021. [Google Scholar] [CrossRef]
- Peykani, P.; Memar-Masjed, E.; Arabjazi, N.; Mirmozaffari, M. Dynamic performance assessment of hospitals by applying credibility-based fuzzy window data envelopment analysis. Healthcare 2022, 10, 876. [Google Scholar] [CrossRef]
- Cinaroglu, S. Fuzzy Efficiency Estimates of the Turkish Health System: A Comparison of Interval, Bias-Corrected, and Fuzzy Data Envelopment Analysis. Int. J. Fuzzy Syst. 2023, 25, 2356–2379. [Google Scholar] [CrossRef]
- Ferreira, D.C.; Figueira, J.R.; Greco, S.; Marques, R.C. Data envelopment analysis models with imperfect knowledge of input and output values: An application to Portuguese public hospitals. Expert Syst. Appl. 2023, 231, 120543. [Google Scholar] [CrossRef]
- Jahani, M.; Kordrostami, S. Estimating sustainability dimensions using fuzzy inverse directional distance model with flexible measures: A health sector application. Soft Comput. 2023, 27, 17025–17041. [Google Scholar] [CrossRef]
- Mohanta, K.K.; Sharanappa, D.S.; Aggarwal, A. Value and ambiguity Index-based ranking approach for solving neutrosophic data envelopment analysis. Neutrosophic Sets Syst. 2023, 57, 25. [Google Scholar]
- Han, P.K.; Klein, W.M.; Arora, N.K. Varieties of uncertainty in health care: A conceptual taxonomy. Med. Decis. Mak. 2011, 31, 828–838. [Google Scholar] [CrossRef]
- Broekhuizen, H.; Groothuis-Oudshoorn, C.G.; van Til, J.A.; Hummel, J.M.; IJzerman, M.J. A review and classification of approaches for dealing with uncertainty in multi-criteria decision analysis for healthcare decisions. Pharmacoeconomics 2015, 33, 445–455. [Google Scholar] [CrossRef] [PubMed]
- Diwekar, U.M.; Diwekar, U.M. Optimization under uncertainty. In Introduction to Applied Optimization; Springer: Berlin/Heidelberg, Germany, 2020; pp. 151–215. [Google Scholar]
- Acar, E.; Bayrak, G.; Jung, Y.; Lee, I.; Ramu, P.; Ravichandran, S.S. Modeling, analysis, and optimization under uncertainties: A review. Struct. Multidiscip. Optim. 2021, 64, 2909–2945. [Google Scholar] [CrossRef]
- Keith, A.J.; Ahner, D.K. A survey of decision making and optimization under uncertainty. Ann. Oper. Res. 2021, 300, 319–353. [Google Scholar] [CrossRef]
- Wen, M.; Guo, L.; Kang, R.; Yang, Y. Data envelopment analysis with uncertain inputs and outputs. J. Appl. Math. 2014, 2014, 307108. [Google Scholar] [CrossRef]
- Lio, W.; Liu, B. Uncertain data envelopment analysis with imprecisely observed inputs and outputs. Fuzzy Optim. Decis. Mak. 2018, 17, 357–373. [Google Scholar] [CrossRef]
- Jiang, B.; Yang, C.; Li, J. The uncertain network DEA model for two-stage system with additive relationship. Symmetry 2021, 13, 1893. [Google Scholar] [CrossRef]
- Chi, E.; Jiang, B.; Peng, L.; Zhong, Y. Uncertain network DEA models with imprecise data for sustainable efficiency evaluation of decentralized marine supply chain. Energies 2022, 15, 5313. [Google Scholar] [CrossRef]
- Jiang, B.; Chi, E.; Li, J. Uncertain Data Envelopment Analysis for Cross Efficiency Evaluation with Imprecise Data. Mathematics 2022, 10, 2161. [Google Scholar] [CrossRef]
- Wu, J.; Sheng, Y. Uncertain DEA-Malmquist productivity index model and its application. J. Intell. Fuzzy Syst. 2023, 44, 5295–5308. [Google Scholar] [CrossRef]
- Mahmoodirad, A.; Dehghan, R.; Niroomand, S. Modelling linear fractional transportation problem in belief degree—Based uncertain environment. J. Exp. Theor. Artif. Intell. 2019, 31, 393–408. [Google Scholar] [CrossRef]
- Mahmoodirad, A.; Niroomand, S. A belief degree-based uncertain scheme for a bi-objective two-stage green supply chain network design problem with direct shipment. Soft Comput. 2020, 24, 18499–18519. [Google Scholar] [CrossRef]
- Mahmoodirad, A.; Niroomand, S. Uncertain location–allocation decisions for a bi-objective two-stage supply chain network design problem with environmental impacts. Expert Syst. 2020, 37, e12558. [Google Scholar] [CrossRef]
- Liu, B. Uncertainty Theory: An Introduction to Its Axiomatic Foundation; Physica-Verlag: Heidelberg, Germany, 2004. [Google Scholar]
- Liu, B. Some research problems in uncertainty theory. J. Uncertain Syst. 2009, 3, 3–10. [Google Scholar]
- Liu, B.; Liu, B. Uncertainty Theory: A Branch of Mathematics for Modeling Human Uncertainty; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Roll, Y.; Golany, B. Alternate methods of treating factor weights in DEA. Omega 1993, 21, 99–109. [Google Scholar] [CrossRef]
- Liu, F.H.F.; Peng, H.H. Ranking of units on the DEA frontier with common weights. Comput. Oper. Res. 2008, 35, 1624–1637. [Google Scholar] [CrossRef]
- Davoodi, A.; Rezai, H.Z. Common set of weights in data envelopment analysis: A linear programming problem. Cent. Eur. J. Oper. Res. 2012, 20, 355–365. [Google Scholar] [CrossRef]
- Hosseinzadeh Lotfi, F.; Hatami-Marbini, A.; Agrell, P.J.; Aghayi, N.; Gholami, K. Allocating fixed resources and setting targets using a common-weights DEA approach. Comput. Ind. Eng. 2013, 64, 631–640. [Google Scholar] [CrossRef]
- Hatami-Marbini, A.; Tavana, M.; Agrell, P.J.; Hosseinzadeh Lotfi, F.; Beigi, Z.G. A common-weights DEA model for centralized resource reduction and target setting. Comput. Ind. Eng. 2015, 79, 195–203. [Google Scholar] [CrossRef]
- Seyed Esmaeili, F.S.; Rostamy-Malkhalifeh, M.; Hosseinzadeh Lotfi, F. A hybrid approach using data envelopment analysis, interval programming and robust optimisation for performance assessment of hotels under uncertainty. Int. J. Manag. Decis. Mak. 2021, 20, 308–322. [Google Scholar]
- Dehnokhalaji, A.; Khezri, S.; Emrouznejad, A. A box-uncertainty in DEA: A robust performance measurement framework. Expert Syst. Appl. 2022, 187, 115855. [Google Scholar] [CrossRef]
- Peykani, P.; Emrouznejad, A.; Mohammadi, E.; Gheidar-Kheljani, J. A novel robust network data envelopment analysis approach for performance assessment of mutual funds under uncertainty. In Annals of Operations Research; Springer: Berlin/Heidelberg, Germany, 2022; pp. 1–27. [Google Scholar]
- Toloo, M.; Mensah, E.K.; Salahi, M. Robust optimization and its duality in data envelopment analysis. Omega 2022, 108, 102583. [Google Scholar] [CrossRef]
- Hafezalkotob, A.; Haji-Sami, E.; Omrani, H. Robust DEA under discrete uncertain data: A case study of Iranian electricity distribution companies. J. Ind. Eng. Int. 2015, 11, 199–208. [Google Scholar] [CrossRef]
- Zahedi-Seresht, M.; Jahanshahloo, G.R.; Jablonsky, J. A robust data envelopment analysis model with different scenarios. Appl. Math. Model. 2017, 52, 306–319. [Google Scholar] [CrossRef]
- Zahedi-Seresht, M.; Khosravi, S.; Jablonsky, J.; Zykova, P. A data envelopment analysis model for performance evaluation and ranking of DMUs with alternative scenarios. Comput. Ind. Eng. 2021, 152, 107002. [Google Scholar] [CrossRef]
- Shakouri, R.; Salahi, M. Stochastic p-robust approach to a centralized two-stage DEA system with resource waste. Adv. Oper. Res. 2022, 2022, 1133882. [Google Scholar] [CrossRef]
- Omrani, H.; Shamsi, M.; Emrouznejad, A.; Teplova, T. A robust DEA model under discrete scenarios for assessing bank branches. Expert Syst. Appl. 2023, 219, 119694. [Google Scholar] [CrossRef]
- Mousazadeh, M.; Torabi, S.A.; Zahiri, B. A robust possibilistic programming approach for pharmaceutical supply chain network design. Comput. Chem. Eng. 2015, 82, 115–128. [Google Scholar] [CrossRef]
- Zahiri, B.; Torabi, S.; Mousazadeh, M.; Mansouri, S. Blood collection management: A robust possibilistic programming approach. Appl. Math. Model. 2015, 39, 7680–7696. [Google Scholar] [CrossRef]
- Rabbani, M.; Zhalechian, M.; Farshbaf-Geranmayeh, A. A robust possibilistic programming approach to multiperiod hospital evacuation planning problem under uncertainty. Int. Trans. Oper. Res. 2018, 25, 157–189. [Google Scholar] [CrossRef]
- Pouraliakbari-Mamaghani, M.; Ghodratnama, A.; Pasandideh, S.H.R.; Saif, A. A robust possibilistic programming approach for blood supply chain network design in disaster relief considering congestion. Oper. Res. 2022, 22, 1987–2032. [Google Scholar] [CrossRef]
- Peykani, P.; Mohammadi, E.; Pishvaee, M.S.; Rostamy-Malkhalifeh, M.; Jabbarzadeh, A. A novel fuzzy data envelopment analysis based on robust possibilistic programming: Possibility, necessity and credibility-based approaches. RAIRO-Oper. Res. 2018, 52, 1445–1463. [Google Scholar] [CrossRef]
- Izadikhah, M.; Azadi, M.; Toloo, M.; Hussain, F.K. Sustainably resilient supply chains evaluation in public transport: A fuzzy chance-constrained two-stage DEA approach. Appl. Soft Comput. 2021, 113, 107879. [Google Scholar] [CrossRef]
- Peykani, P.; Mohammadi, E.; Emrouznejad, A. An adjustable fuzzy chance-constrained network DEA approach with application to ranking investment firms. Expert Syst. Appl. 2021, 166, 113938. [Google Scholar] [CrossRef]
- Arabjazi, N.; Rostamy-Malkhalifeh, M.; Hosseinzadeh Lotfi, F.; Behzadi, M.H. Stability analysis with general fuzzy measure: An application to social security organizations. PLoS ONE 2022, 17, e0275594. [Google Scholar] [CrossRef] [PubMed]
- Pourbabagol, H.; Amiri, M.; Taghavifard, M.T.; Hanafizadeh, P. A new fuzzy DEA network based on possibility and necessity measures for agile supply chain performance evaluation: A case study. Expert Syst. Appl. 2023, 220, 119552. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, Y. Possibilistic chance-constrained data envelopment analysis framework for failure modes and effects analysis. Appl. Soft Comput. 2023, 147, 110830. [Google Scholar] [CrossRef]
| Year | Research | DEA Model | Uncertain Programming Approach | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SO | FO | RO | BS | IP | NT | ZT | UT | |||
| 1999 | Morita and Seiford [36] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2012 | Ebrahimnejad [37] | Cost Efficiency | ✓ | |||||||
| 2012 | Hatami-Marbini et al. [38] | Malmquist Productivity Index | ✓ | |||||||
| 2012 | Khaki et al. [39] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2013 | Costantino et al. [40] | Cross Efficiency | ✓ | |||||||
| 2013 | De Nicola et al. [41] | Banker–Charnes–Cooper | ✓ | |||||||
| 2013 | Khodaparasti and Maleki [42] | Simultaneous Dynamic | ✓ | |||||||
| 2014 | Kalantary and Azar [43] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2014 | Karadayi and Karsak [44] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2015 | Dotoli et al. [45] | Cross Efficiency | ✓ | |||||||
| 2015 | Haji-Sami et al. [46] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2015 | Kheirollahi et al. [47] | Congestion | ✓ | |||||||
| 2015 | Mitropoulos et al. [48] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2016 | Rabbani et al. [49] | Charnes–Cooper–Rhodes | ✓ | ✓ | ✓ | |||||
| 2016 | Shwartz et al. [50] | Banker–Charnes–Cooper | ✓ | |||||||
| 2017 | Arya and Yadav [51] | Slacks-Based Measure | ✓ | |||||||
| 2017 | Karsak and Karadayi [52] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2017 | Kheirollahi et al. [53] | Congestion | ✓ | |||||||
| 2017 | Otay et al. [54] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2018 | Ahmadvand and Pishvaee [55] | Common Set of Weights | ✓ | |||||||
| 2018 | Peykani et al. [56] | Malmquist Productivity Index | ✓ | |||||||
| 2018 | Wu and Wu [57] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2019 | Hatefi and Haeri [58] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2019 | Ji et al. [59] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2019 | Peykani et al. [60] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2020 | Arya and Yadav [61] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2020 | Ghafari Someh et al. [62] | Three-Stage Network | ✓ | |||||||
| 2020 | Yang et al. [63] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2021 | Abdelfattah [64] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2021 | Gómez-Gallego et al. [65] | Banker–Charnes–Cooper | ✓ | |||||||
| 2021 | Izadikhah et al. [66] | Charnes–Cooper–Rhodes | ✓ | ✓ | ||||||
| 2021 | Jahani and Kordrostami [67] | Slacks-Based Measure | ✓ | |||||||
| 2021 | Tavana et al. [68] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2022 | Izadikhah [69] | Slacks-Based Measure | ✓ | ✓ | ||||||
| 2022 | Omrani et al. [70] | Banker–Charnes–Cooper | ✓ | ✓ | ||||||
| 2022 | Peykani et al. [71] | Window Analysis | ✓ | |||||||
| 2023 | Cinaroglu [72] | Charnes–Cooper–Rhodes | ✓ | |||||||
| 2023 | Ferreira et al. [73] | Directional Distance | ✓ | ✓ | ✓ | |||||
| 2023 | Jahani and Kordrostami [74] | Inverse Directional Distance | ✓ | |||||||
| 2023 | Mohanta et al. [75] | Charnes–Cooper–Rhodes | ✓ | |||||||
| The Current Research | Common Set of Weights | ✓ | ||||||||
| Hospitals | NOBE | NOST | SOEF | SOLI |
|---|---|---|---|---|
| Hospital 01 | (114, 126) | (338, 374) | (53, 59) | (68, 76) |
| Hospital 02 | (429, 475) | (834, 922) | (94, 100) | (90, 100) |
| Hospital 03 | (134, 148) | (316, 350) | (57, 63) | (63, 69) |
| Hospital 04 | (271, 299) | (379, 419) | (71, 79) | (65, 71) |
| Hospital 05 | (116, 128) | (344, 380) | (55, 61) | (59, 65) |
| Hospital 06 | (72, 80) | (177, 195) | (49, 55) | (43, 47) |
| Hospital 07 | (119, 131) | (299, 331) | (45, 49) | (56, 62) |
| Hospital 08 | (89, 99) | (166, 184) | (50, 56) | (33, 37) |
| Hospital 09 | (85, 93) | (212, 234) | (45, 49) | (63, 69) |
| Hospital 10 | (19, 21) | (72, 80) | (23, 25) | (33, 37) |
| Hospital 11 | (23, 25) | (95, 105) | (32, 36) | (43, 47) |
| Hospital 12 | (74, 82) | (201, 223) | (37, 41) | (44, 48) |
| Hospital 13 | (29, 32) | (90, 100) | (33, 37) | (31, 35) |
| Hospital 14 | (166, 184) | (304, 336) | (74, 82) | (55, 61) |
| Hospital 15 | (69, 77) | (102, 112) | (35, 39) | (41, 45) |
| Hospital 16 | (71, 79) | (166, 184) | (88, 98) | (84, 92) |
| Hospital 17 | (111, 123) | (359, 397) | (91, 99) | (84, 92) |
| Hospital 18 | (88, 98) | (200, 221) | (70, 78) | (80, 88) |
| Hospital 19 | (86, 95) | (246, 272) | (89, 99) | (88, 98) |
| Hospital 20 | (42, 46) | (93, 103) | (37, 41) | (60, 66) |
| Hospitals | NOOU | NOIN | NOSU |
|---|---|---|---|
| Hospital 01 | (49684, 54913) | (6114, 6757) | (1774, 1960) |
| Hospital 02 | (360182, 398096) | (39230, 43360) | (18355, 20287) |
| Hospital 03 | (64230, 70991) | (8434, 9322) | (3057, 3379) |
| Hospital 04 | (121546, 134341) | (17598, 19451) | (2926, 3234) |
| Hospital 05 | (118536, 131013) | (16699, 18456) | (527, 583) |
| Hospital 06 | (41049, 45370) | (5363, 5927) | (1710, 1890) |
| Hospital 07 | (68156, 75330) | (6209, 6862) | (4012, 4434) |
| Hospital 08 | (85592, 94602) | (7443, 8227) | (1097, 1213) |
| Hospital 09 | (94913, 104904) | (7882, 8711) | (1664, 1840) |
| Hospital 10 | (54048, 59737) | (3549, 3922) | (808, 893) |
| Hospital 11 | (65790, 72716) | (9216, 10186) | (410, 454) |
| Hospital 12 | (83392, 92170) | (8237, 9104) | (2076, 2294) |
| Hospital 13 | (40613, 44888) | (3273, 3618) | (599, 662) |
| Hospital 14 | (118915, 131432) | (10838, 11979) | (5545, 6129) |
| Hospital 15 | (74349, 82175) | (6533, 7221) | (1959, 2165) |
| Hospital 16 | (32568, 35996) | (4308, 4761) | (3092, 3418) |
| Hospital 17 | (41162, 45495) | (5743, 6348) | (2775, 3067) |
| Hospital 18 | (37216, 41134) | (2704, 2988) | (1869, 2065) |
| Hospital 19 | (34032, 37615) | (3921, 4334) | (1704, 1884) |
| Hospital 20 | (8846, 9778) | (1482, 1638) | (689, 761) |
| Hospitals | Belief Degree | ||||
|---|---|---|---|---|---|
| 0% | 25% | 50% | 75% | 100% | |
| Hospital 01 | 13.43488 | 13.75816 | 14.10025 | 14.48392 | 14.87131 |
| Hospital 02 | 0.00000 | 0.00000 | 0.00000 | 0.00000 | 0.00000 |
| Hospital 03 | 10.61700 | 10.89819 | 11.19201 | 11.51168 | 11.83541 |
| Hospital 04 | 8.22185 | 8.45835 | 8.67954 | 8.86653 | 9.04879 |
| Hospital 05 | 4.83058 | 4.95892 | 5.10777 | 5.29900 | 5.49741 |
| Hospital 06 | 6.05503 | 6.22125 | 6.39708 | 6.59224 | 6.79144 |
| Hospital 07 | 8.94735 | 9.17307 | 9.41139 | 9.67641 | 9.94443 |
| Hospital 08 | 0.51054 | 0.50404 | 0.49700 | 0.49061 | 0.48225 |
| Hospital 09 | 3.67848 | 3.78079 | 3.89674 | 4.04001 | 4.18773 |
| Hospital 10 | 0.03883 | 0.01603 | 0.00000 | 0.00000 | 0.00000 |
| Hospital 11 | 0.00000 | 0.00000 | 0.01237 | 0.05015 | 0.09178 |
| Hospital 12 | 2.40902 | 2.47299 | 2.54859 | 2.64826 | 2.75178 |
| Hospital 13 | 1.97477 | 1.99968 | 2.03026 | 2.07488 | 2.11884 |
| Hospital 14 | 4.04720 | 4.14449 | 4.24171 | 4.33951 | 4.43630 |
| Hospital 15 | 0.00000 | 0.00000 | 0.00000 | 0.00000 | 0.00000 |
| Hospital 16 | 10.57316 | 10.83996 | 11.11992 | 11.42801 | 11.73908 |
| Hospital 17 | 16.27186 | 16.70051 | 17.15668 | 17.66985 | 18.19250 |
| Hospital 18 | 11.34755 | 11.63266 | 11.92967 | 12.25247 | 12.57793 |
| Hospital 19 | 13.86641 | 14.19261 | 14.53618 | 14.91889 | 15.30372 |
| Hospital 20 | 8.37461 | 8.58316 | 8.80013 | 9.03519 | 9.27134 |
| Objective | 125.19910 | 128.33490 | 131.65730 | 135.37760 | 139.14200 |
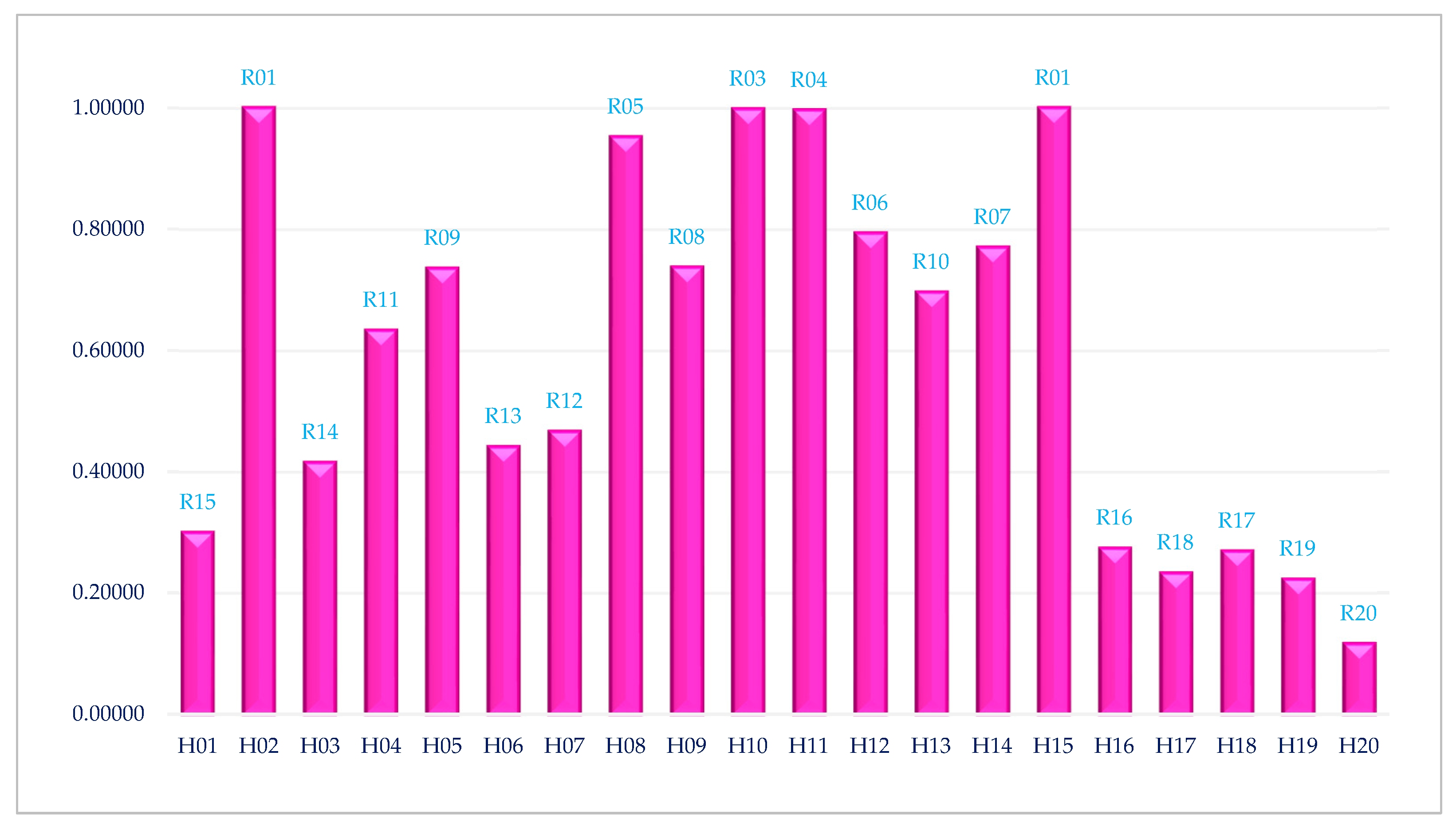
| Hospitals | Belief Degree | ||||
|---|---|---|---|---|---|
| 0% | 25% | 50% | 75% | 100% | |
| Hospital 01 | 0.29998 | 0.30044 | 0.30059 | 0.30014 | 0.29966 |
| Hospital 02 | 1.00000 | 1.00000 | 1.00000 | 1.00000 | 1.00000 |
| Hospital 03 | 0.41630 | 0.41626 | 0.41595 | 0.41510 | 0.41422 |
| Hospital 04 | 0.63343 | 0.63287 | 0.63276 | 0.63354 | 0.63441 |
| Hospital 05 | 0.73757 | 0.73752 | 0.73669 | 0.73435 | 0.73187 |
| Hospital 06 | 0.44282 | 0.44254 | 0.44191 | 0.44059 | 0.43919 |
| Hospital 07 | 0.46695 | 0.46721 | 0.46713 | 0.46636 | 0.46556 |
| Hospital 08 | 0.94855 | 0.95041 | 0.95224 | 0.95392 | 0.95570 |
| Hospital 09 | 0.73956 | 0.73928 | 0.73834 | 0.73613 | 0.73383 |
| Hospital 10 | 0.99340 | 0.99733 | 1.00000 | 1.00000 | 1.00000 |
| Hospital 11 | 1.00000 | 1.00000 | 0.99844 | 0.99387 | 0.98911 |
| Hospital 12 | 0.79549 | 0.79545 | 0.79467 | 0.79243 | 0.79008 |
| Hospital 13 | 0.69256 | 0.69541 | 0.69756 | 0.69818 | 0.69884 |
| Hospital 14 | 0.76974 | 0.77014 | 0.77052 | 0.77085 | 0.77121 |
| Hospital 15 | 1.00000 | 1.00000 | 1.00000 | 1.00000 | 1.00000 |
| Hospital 16 | 0.27432 | 0.27453 | 0.27449 | 0.27397 | 0.27342 |
| Hospital 17 | 0.23390 | 0.23390 | 0.23360 | 0.23275 | 0.23185 |
| Hospital 18 | 0.26915 | 0.26937 | 0.26939 | 0.26900 | 0.26858 |
| Hospital 19 | 0.22239 | 0.22286 | 0.22311 | 0.22289 | 0.22265 |
| Hospital 20 | 0.11626 | 0.11640 | 0.11644 | 0.11627 | 0.11609 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peykani, P.; Pishvaee, M.S. Performance Evaluation of Hospitals under Data Uncertainty: An Uncertain Common-Weights Data Envelopment Analysis. Healthcare 2024, 12, 611. https://doi.org/10.3390/healthcare12060611
Peykani P, Pishvaee MS. Performance Evaluation of Hospitals under Data Uncertainty: An Uncertain Common-Weights Data Envelopment Analysis. Healthcare. 2024; 12(6):611. https://doi.org/10.3390/healthcare12060611
Chicago/Turabian StylePeykani, Pejman, and Mir Saman Pishvaee. 2024. "Performance Evaluation of Hospitals under Data Uncertainty: An Uncertain Common-Weights Data Envelopment Analysis" Healthcare 12, no. 6: 611. https://doi.org/10.3390/healthcare12060611
APA StylePeykani, P., & Pishvaee, M. S. (2024). Performance Evaluation of Hospitals under Data Uncertainty: An Uncertain Common-Weights Data Envelopment Analysis. Healthcare, 12(6), 611. https://doi.org/10.3390/healthcare12060611







