Differential Evolution with Adaptive Grid-Based Mutation Strategy for Multi-Objective Optimization
Abstract
:1. Introduction
2. Background
2.1. Multi-Objective Optimization Problem (MOP)
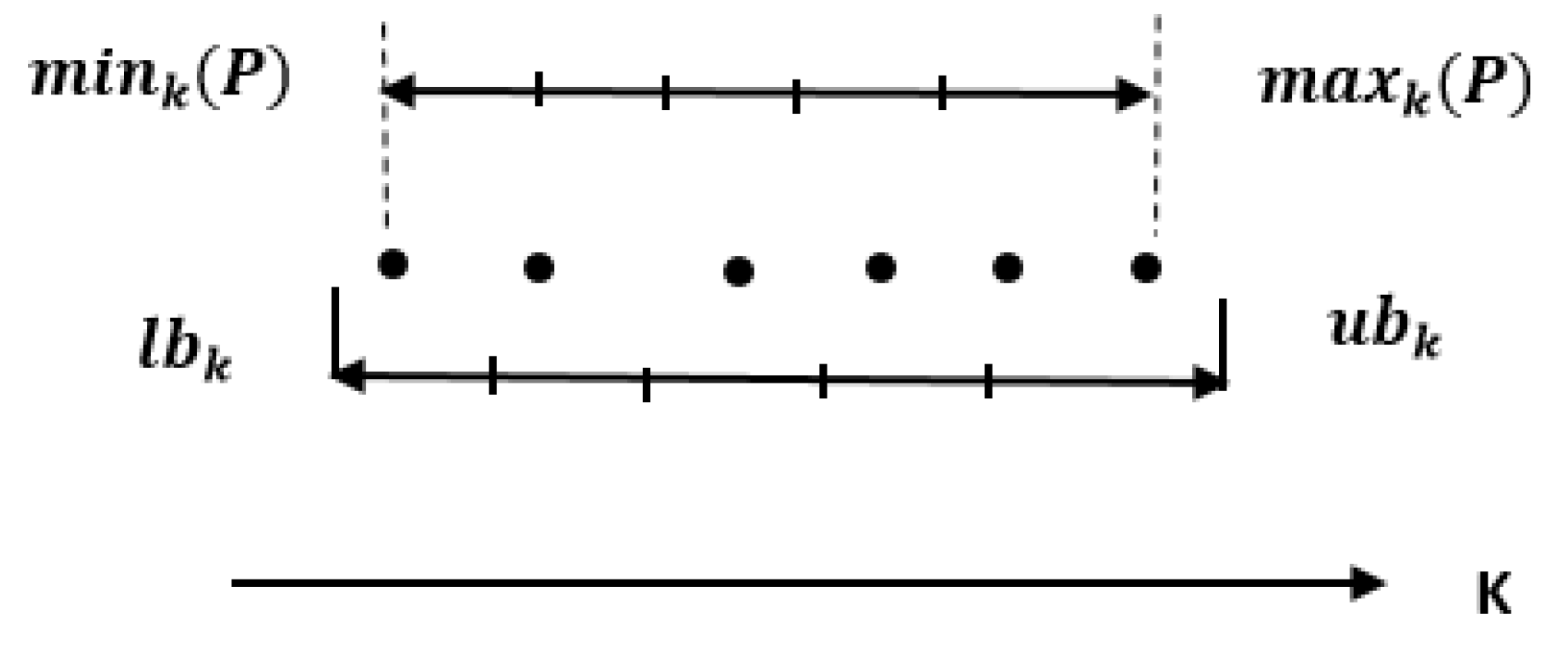
2.2. Definition of a Grid Structure
2.3. Related Work
3. Proposed Method
3.1. General Framework of ad-GrMODE
| Algorithm 1: General framework of ad-GrMODE |
 |
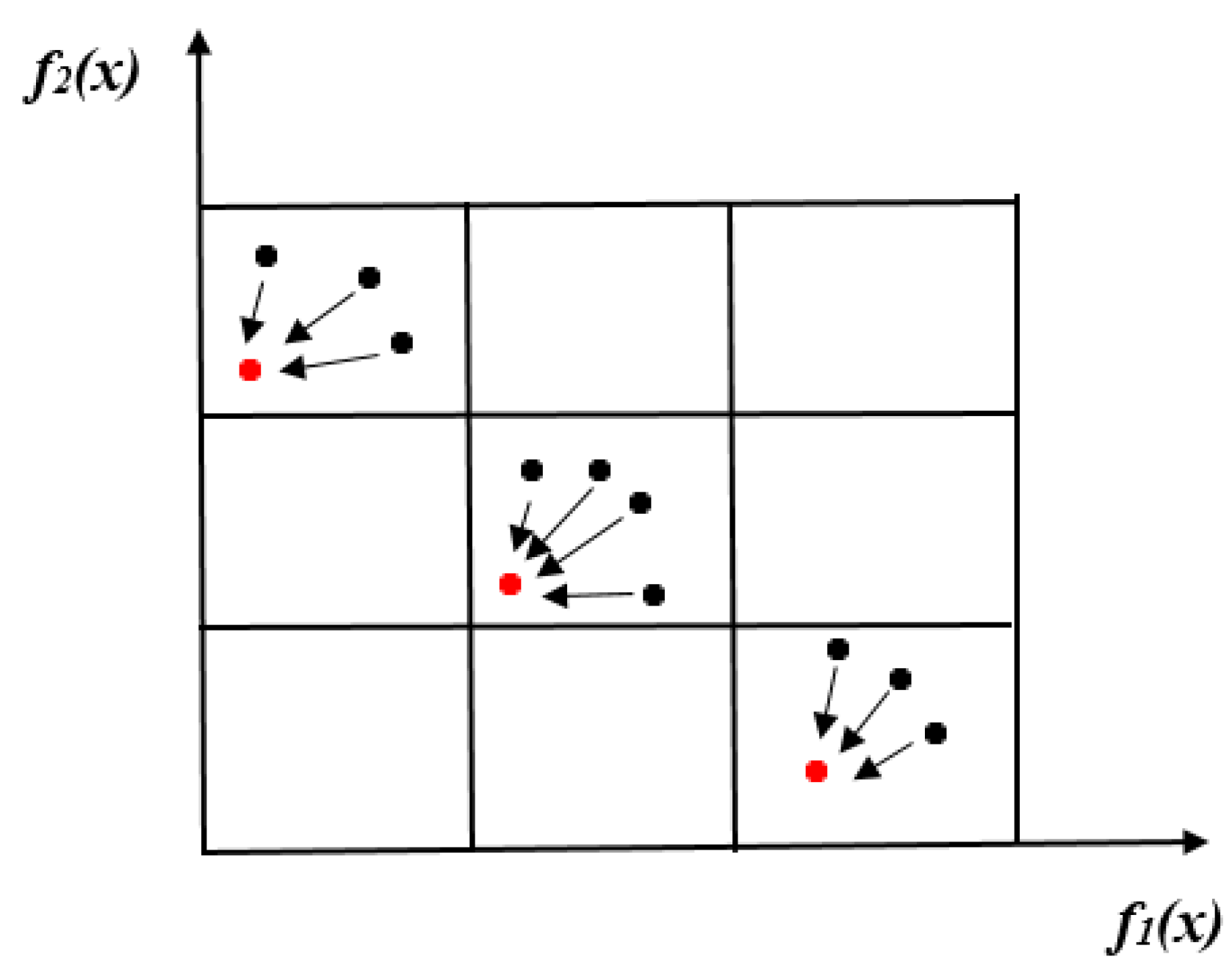
3.2. Grid-Based Mutation Strategy
| Algorithm 2: Grid-based mutation |
 |
3.3. Crossover
3.4. Environmental Selection
| Algorithm 3: Environmental selection |
 |
4. Experimental Setup
- (a)
- An analysis of the performance of the proposed algorithm with different grid settings;
- (b)
- A comparison of the performance of the proposed algorithm with state-of-art algorithms.
4.1. Description of Benchmark Problems
4.2. Performance Metric
5. Experimental Results
- (a)
- First, the performance of the proposed algorithm is analyzed with different grid settings. The important parameter in the grid is “div”, which controls the search space. Hence, we evaluate our algorithm with different values of “div”. Our approach proposes an adaptive grid setting.
- (b)
- Second, the performance of the proposed method with the adaptive grid settings is compared with state-of-the-art algorithms.
5.1. Analysis of Grid Setting in the Proposed Method
- (a)
- The number of grids into which the objective space can be partitioned is evaluated as divM, where M is the number of objectives. For instance, if “div = 20” and “M = 10” then the objective space is divided into 2010 grids. As arbitrarily large population size cannot be used for the evolutionary process, dividing the controlled population size into 2010 grids results in an inadequate number of solutions in each grid. In other words, each grid is associated with hardly one or two solutions, and the proposed grid-based mutation does not work with insufficient solutions within each grid because selection pressure is lost. Thus, the grid setting with minimum “div”, value performs better than and the performance degrades as the “div” value increases.
- (b)
- Second, fixed grid settings do not work for all types of problems. Instead, different grid settings are helpful in exploring the search space at every stage of evolution. The adaptive approach provides different grid settings at each evolution stage and helps achieve better convergence and diversity.
- (c)
- The main principle behind our grid-based mutation is that a few grids are used initially to achieve convergence. Gradually, the number of grids increases, which improves the diversity by providing a proper distribution of solutions.
5.2. Comparing the Proposed Method and State-of-the-Art Algorithms Using the DTLZ Problems Based on the HV Metric
5.3. Comparing the Proposed Method and State-of-the-Art Algorithms Using the WFG Problems Based on the HV Metric
5.4. Comparing the Proposed Method and State-of-the-Art Algorithms Using the DTLZ Problems Based on the IGD Metric
5.5. Comparing the Proposed Method and State-of-the-Art Algorithms Using the WFG Problems Based on the IGD Metric
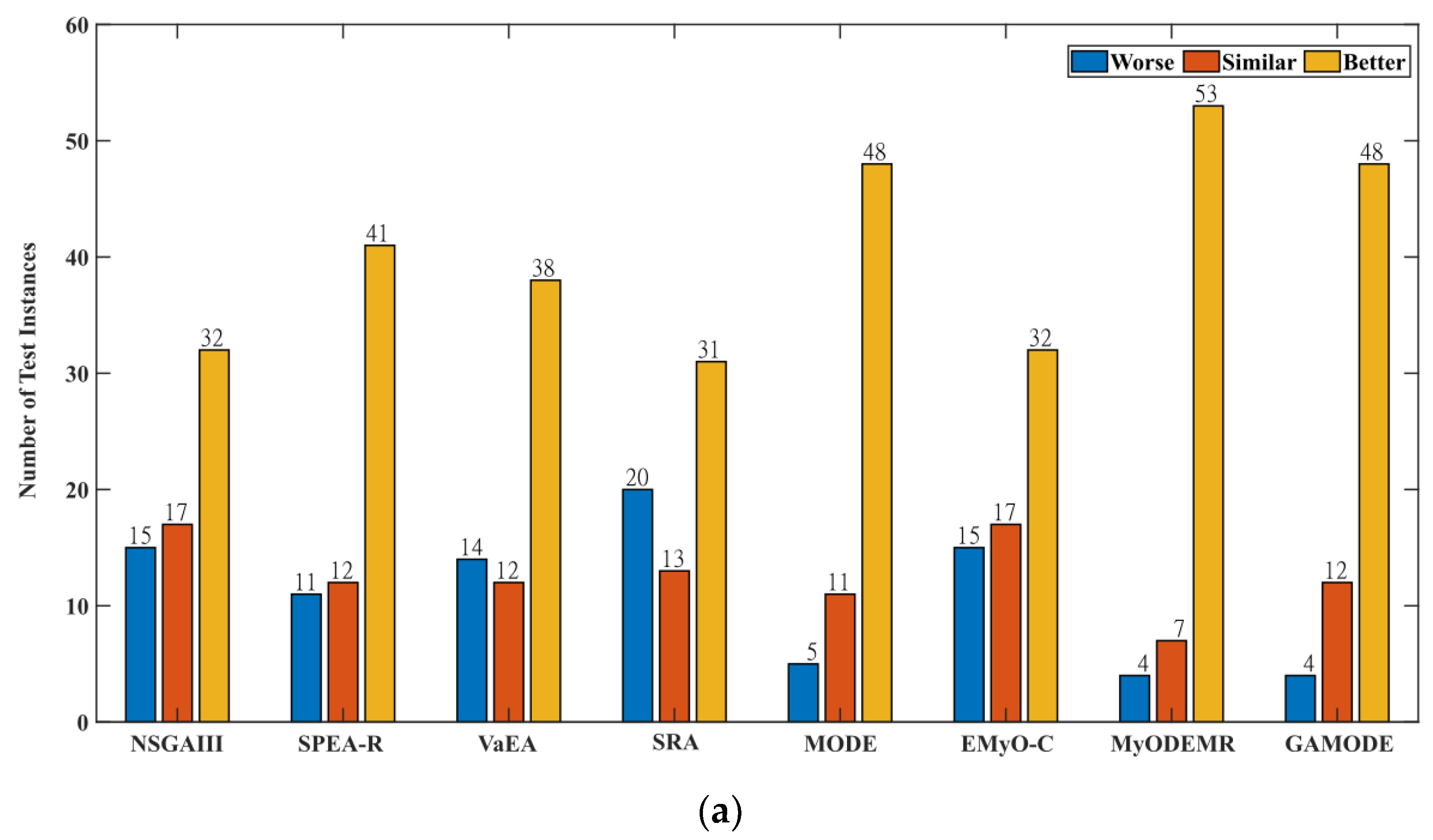
5.6. Overall Performance Comparison of ad-GrMODE with State-of-the-Art Algorithms for the DTLZ and WFG Problems
5.7. Runtime Evaluation of ad-GrMODE Compared with State-of-the-Art Algorithms
5.8. Overall Performance Comparison of ad-GrMODE with State-of-the-Art Algorithms on Real-World Problem
6. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DE | Differential Evolution |
| ad-GrMODE | adaptive Grid-based multi-objective differential evolution |
| MOP | Multi-objective Optimization Problem |
| PS | Pareto optimal Set |
| PF | Pareto Front |
| PSO | Particle Swarm Optimization |
| EA | Evolutionary Algorithm |
| SOP | Single-objective Optimization Problem |
| MODE | Multi-Objective Differential Evolution |
| GrEA | Grid-based evolutionary algorithm |
| PESA-II | Pareto envelope-based selection algorithm II |
| Grid-IGD | Grid-based Inverted Generational Distance |
| GDE3 | Generalized Differential Evolution |
| PDE | Pareto Differential Evolution |
| NSGA-II | Non-dominated Sorting Genetic Algorithm II |
| PDEA | Pareto Differential Evolution Algorithm |
| DEMO | Differential Evolution for Multi-objective Optimization |
| MOED | Multi-objective Energy Disaggregation |
| MOSaDE | Multi-objective Optimization based on Self-adaptive Differential Evolution |
| GrBLS | Grid-based Bidirectional Local Search |
| MOEA | Multi objective Evolutionary Algorithm |
| CCDG-K | Multi objective evolutionary algorithm base on Constrained Decomposition with Grids |
| GSMPSO-MM | Grid Search-based Multi-population Particle Swarm Optimization |
| MOWOA | opposition-based Multi-Objective Whale Optimization Algorithm with global grid ranking |
| NSGA-III | Non-dominated Sorting Genetic Algorithm III |
| SPEA-R | Strength Pareto Evolutionary Algorithm based on Reference direction |
| VaEA | Vector angle-based Evolutionary Algorithm |
| SRA | Stochastic Ranking Algorithm |
| EMyO-C | Clustering-based selection for Evolutionary Many-objective Optimization |
| MyODEMR | Many-Objective Differential Evolution with Mutation Restriction |
| GAMODE | Grid-based Adaptive Multi-Objective Differential Evolution |
| HV | Hypervolume |
| IGD | Inverted Generational Distance |
| RCBD | Reinforced Concrete Beam Design |
| PVD | Pressure Vessel Design |
| GTD | Gear Train Design |
Appendix A
| # | M | ad-GrMODE1 | ad-GrMODE2 | ad-GrMODE3 | ad-GrMODE4 | ad-GrMODE2 | ad-GrMODE1* | ad-GrMODE2* | ad-GrMODE3* | ad-GrMODE4* | ad-GrMODE5* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| DTLZ1 | 3 | 0.8397 | 0.8365 | 0.8128 | 0.7322 | 0.6750 | 0.8392 | 0.8392 | 0.8385 | 0.8374 | 0.8364 |
| 5 | 0.9677 | 0.9515 | 0.1453 | 0.0043 | 0.0023 | 0.9692 | 0.9674 | 0.9671 | 0.9651 | 0.9641 | |
| 8 | 0.9879 | 0.6445 | 0.0302 | 0.0000 | 0.0000 | 0.9927 | 0.9930 | 0.9913 | 0.9894 | 0.9904 | |
| 10 | 0.9974 | 0.9604 | 0.1336 | 0.0063 | 0.0000 | 0.9989 | 0.9987 | 0.9987 | 0.9981 | 0.9785 | |
| DTLZ2 | 3 | 0.5653 | 0.5639 | 0.5631 | 0.5619 | 0.5612 | 0.5659 | 0.5651 | 0.5653 | 0.5644 | 0.5648 |
| 5 | 0.7899 | 0.7688 | 0.7503 | 0.7454 | 0.7461 | 0.7976 | 0.7964 | 0.7941 | 0.7920 | 0.7905 | |
| 8 | 0.8469 | 0.7730 | 0.7492 | 0.7473 | 0.7418 | 0.8889 | 0.8762 | 0.8645 | 0.8563 | 0.8560 | |
| 10 | 0.8980 | 0.8182 | 0.7929 | 0.7833 | 0.7788 | 0.9273 | 0.9215 | 0.9110 | 0.9044 | 0.9007 | |
| DTLZ3 | 3 | 0.5115 | 0.2798 | 0.0609 | 0.0000 | 0.0014 | 0.5546 | 0.5248 | 0.4865 | 0.3856 | 0.3112 |
| 5 | 0.6572 | 0.0417 | 0.0000 | 0.0000 | 0.0000 | 0.7606 | 0.6724 | 0.4271 | 0.2507 | 0.1664 | |
| 8 | 0.5135 | 0.0400 | 0.0000 | 0.0000 | 0.0000 | 0.7730 | 0.7109 | 0.6526 | 0.3768 | 0.2751 | |
| 10 | 0.8629 | 0.2162 | 0.0000 | 0.0000 | 0.0000 | 0.9334 | 0.8773 | 0.8474 | 0.7967 | 0.7024 | |
| DTLZ4 | 3 | 0.5308 | 0.5184 | 0.5224 | 0.5052 | 0.5122 | 0.5442 | 0.5364 | 0.5581 | 0.5454 | 0.5467 |
| 5 | 0.7656 | 0.7438 | 0.7244 | 0.7223 | 0.7251 | 0.7857 | 0.7760 | 0.7815 | 0.7724 | 0.7673 | |
| 8 | 0.8998 | 0.8875 | 0.8832 | 0.8788 | 0.8708 | 0.9144 | 0.9109 | 0.9049 | 0.9056 | 0.9019 | |
| 10 | 0.9606 | 0.9559 | 0.9511 | 0.9442 | 0.9454 | 0.9609 | 0.9668 | 0.9655 | 0.9643 | 0.9654 | |
| DTLZ5 | 3 | 0.1996 | 0.1998 | 0.1996 | 0.1997 | 0.1995 | 0.1998 | 0.1998 | 0.1998 | 0.1997 | 0.1997 |
| 5 | 0.1244 | 0.1247 | 0.1239 | 0.1227 | 0.1234 | 0.1246 | 0.1246 | 0.1245 | 0.1246 | 0.1241 | |
| 8 | 0.1012 | 0.1007 | 0.0984 | 0.0972 | 0.0966 | 0.1003 | 0.1003 | 0.0994 | 0.1004 | 0.1003 | |
| 10 | 0.0955 | 0.0963 | 0.0939 | 0.0920 | 0.0904 | 0.0952 | 0.0952 | 0.0955 | 0.0948 | 0.0944 | |
| DTLZ6 | 3 | 0.1996 | 0.1437 | 0.0000 | 0.0000 | 0.0000 | 0.1999 | 0.1999 | 0.2001 | 0.1907 | 0.1930 |
| 5 | 0.0375 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.1165 | 0.1152 | 0.0688 | 0.0381 | 0.0226 | |
| 8 | 0.0010 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0507 | 0.0361 | 0.0222 | 0.0097 | 0.0009 | |
| 10 | 0.0090 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0759 | 0.0543 | 0.0537 | 0.0171 | 0.0185 | |
| DTLZ7 | 3 | 0.3894 | 0.3896 | 0.3908 | 0.3983 | 0.3810 | 0.3844 | 0.3882 | 0.3972 | 0.3717 | 0.4000 |
| 5 | 0.3225 | 0.2932 | 0.2128 | 0.2077 | 0.2074 | 0.3239 | 0.3251 | 0.3283 | 0.3205 | 0.3208 | |
| 8 | 0.1358 | 0.0096 | 0.0102 | 0.0099 | 0.0102 | 0.2227 | 0.2110 | 0.2208 | 0.1950 | 0.1748 | |
| 10 | 0.0301 | 0.0009 | 0.0007 | 0.0006 | 0.0009 | 0.1862 | 0.1736 | 0.1233 | 0.0843 | 0.0901 |
| # | M | ad-GrMODE1 | ad-GrMODE2 | ad-GrMODE3 | ad-GrMODE4 | ad-GrMODE2 | ad-GrMODE1* | ad-GrMODE2* | ad-GrMODE3* | ad-GrMODE4* | ad-GrMODE5* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| WFG1 | 3 | 0.2852 | 0.2811 | 0.2805 | 0.2793 | 0.2793 | 0.2855 | 0.2827 | 0.2817 | 0.2811 | 0.2810 |
| 5 | 0.2800 | 0.2794 | 0.2789 | 0.2752 | 0.2494 | 0.2801 | 0.2797 | 0.2798 | 0.2795 | 0.2795 | |
| 8 | 0.2294 | 0.2295 | 0.2295 | 0.2268 | 0.2060 | 0.2294 | 0.2294 | 0.2293 | 0.2294 | 0.2294 | |
| 10 | 0.2071 | 0.2070 | 0.2073 | 0.2073 | 0.2054 | 0.2071 | 0.2071 | 0.2072 | 0.2072 | 0.2073 | |
| WFG2 | 3 | 0.2352 | 0.2351 | 0.2347 | 0.2345 | 0.2342 | 0.2352 | 0.2352 | 0.2351 | 0.2352 | 0.2351 |
| 5 | 0.2115 | 0.2113 | 0.2106 | 0.2101 | 0.2100 | 0.2115 | 0.2115 | 0.2114 | 0.2116 | 0.2116 | |
| 8 | 0.1854 | 0.1851 | 0.1840 | 0.1838 | 0.1836 | 0.1855 | 0.1855 | 0.1854 | 0.1853 | 0.1854 | |
| 10 | 0.1729 | 0.1729 | 0.1723 | 0.1720 | 0.1716 | 0.1730 | 0.1730 | 0.1729 | 0.1730 | 0.1730 | |
| WFG3 | 3 | 0.0923 | 0.0919 | 0.0908 | 0.0905 | 0.0902 | 0.0925 | 0.0920 | 0.0922 | 0.0923 | 0.0920 |
| 5 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | |
| 8 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | |
| 10 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 | |
| WFG4 | 3 | 0.1951 | 0.1926 | 0.1834 | 0.1756 | 0.1731 | 0.1964 | 0.1964 | 0.1948 | 0.1938 | 0.1932 |
| 5 | 0.2159 | 0.1969 | 0.1928 | 0.1918 | 0.1932 | 0.2257 | 0.2237 | 0.2203 | 0.2174 | 0.2133 | |
| 8 | 0.1639 | 0.1620 | 0.1605 | 0.1636 | 0.1617 | 0.1685 | 0.1692 | 0.1674 | 0.1704 | 0.1695 | |
| 10 | 0.1599 | 0.1616 | 0.1596 | 0.1615 | 0.1618 | 0.1598 | 0.1624 | 0.1640 | 0.1657 | 0.1638 | |
| WFG5 | 3 | 0.4585 | 0.4562 | 0.4495 | 0.4432 | 0.4414 | 0.4584 | 0.4584 | 0.4584 | 0.4583 | 0.4573 |
| 5 | 0.4622 | 0.4478 | 0.4385 | 0.4366 | 0.4361 | 0.4671 | 0.4652 | 0.4640 | 0.4622 | 0.4611 | |
| 8 | 0.4587 | 0.4431 | 0.4368 | 0.4391 | 0.4380 | 0.4656 | 0.4640 | 0.4611 | 0.4582 | 0.4588 | |
| 10 | 0.4727 | 0.4586 | 0.4533 | 0.4534 | 0.4518 | 0.4770 | 0.4763 | 0.4735 | 0.4724 | 0.4702 | |
| WFG6 | 3 | 0.1914 | 0.1910 | 0.1906 | 0.1905 | 0.1899 | 0.1915 | 0.1915 | 0.1916 | 0.1914 | 0.1913 |
| 5 | 0.1919 | 0.1915 | 0.1903 | 0.1901 | 0.1898 | 0.1920 | 0.1920 | 0.1920 | 0.1919 | 0.1919 | |
| 8 | 0.1893 | 0.1889 | 0.1874 | 0.1869 | 0.1871 | 0.1893 | 0.1893 | 0.1892 | 0.1890 | 0.1890 | |
| 10 | 0.1880 | 0.1878 | 0.1868 | 0.1862 | 0.1863 | 0.1881 | 0.1881 | 0.1881 | 0.1881 | 0.1881 | |
| WFG7 | 3 | 0.0924 | 0.0886 | 0.0823 | 0.0801 | 0.0764 | 0.0931 | 0.0925 | 0.0921 | 0.0920 | 0.0909 |
| 5 | 0.1380 | 0.1260 | 0.0955 | 0.0768 | 0.0725 | 0.1448 | 0.1448 | 0.1411 | 0.1386 | 0.1373 | |
| 8 | 0.1456 | 0.1286 | 0.0639 | 0.0525 | 0.0505 | 0.1540 | 0.1512 | 0.1494 | 0.1467 | 0.1442 | |
| 10 | 0.1515 | 0.1360 | 0.0602 | 0.0489 | 0.0490 | 0.1544 | 0.1544 | 0.1512 | 0.1500 | 0.1488 | |
| WFG8 | 3 | 0.2312 | 0.2313 | 0.2303 | 0.2299 | 0.2292 | 0.2314 | 0.2314 | 0.2316 | 0.2315 | 0.2317 |
| 5 | 0.2379 | 0.2354 | 0.2299 | 0.2278 | 0.2271 | 0.2386 | 0.2382 | 0.2380 | 0.2371 | 0.2374 | |
| 8 | 0.2397 | 0.2355 | 0.2246 | 0.2225 | 0.2228 | 0.2408 | 0.2403 | 0.2397 | 0.2389 | 0.2387 | |
| 10 | 0.2420 | 0.2394 | 0.2268 | 0.2228 | 0.2216 | 0.2426 | 0.2424 | 0.2419 | 0.2412 | 0.2409 | |
| WFG9 | 3 | 0.3753 | 0.3716 | 0.3614 | 0.3565 | 0.3536 | 0.3763 | 0.3757 | 0.3761 | 0.3744 | 0.3759 |
| 5 | 0.4675 | 0.4310 | 0.4143 | 0.4024 | 0.4059 | 0.4995 | 0.4932 | 0.4770 | 0.4733 | 0.4733 | |
| 8 | 0.4339 | 0.3800 | 0.3712 | 0.3704 | 0.3739 | 0.4675 | 0.4597 | 0.4485 | 0.4423 | 0.4322 | |
| 10 | 0.4521 | 0.4103 | 0.3979 | 0.3939 | 0.3989 | 0.4957 | 0.4848 | 0.4697 | 0.4659 | 0.4626 |
| # | M | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | adGrMOEA1* | adGrMOEA2* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| DTLZ1 | 3 | 8.435 × 10−1 (7.81 × 10−4)-(↓) | 8.392 × 10−1 (1.04 × 10−2)=(≡) | 8.144 × 10−1 (4.84 × 10−2)+(†) | 8.332 × 10−1 (2.73 × 10−3)+(†) | 8.136 × 10−1 (4.66 × 10−3)+(†) | 8.390 × 10−1 (1.41 × 10−3)+(†) | 7.012 × 10−1 (1.89 × 10−1)+(†) | 0.7128 × 10−1 (2.03 × 10−1)+(†) | 8.392 × 10−1 (1.04 × 10−3) | 8.392 × 10−1 (1.14 × 10−3) |
| 5 | 9.743 × 10−1 (8.87 × 10−4)-(↓) | 9.282 × 10−1 (6.09 × 10−2)+(†) | 8.785 × 10−1 (4.52 × 10−2)+(†) | 9.692 × 10−1 (1.83 × 10−3)=(↓) | 9.0144. × 10−1 (1.18 × 10−2)+(†) | 9.667 × 10−1 (1.92 × 10−3)+(†) | 8.241 × 10−1 (6.74 × 10−2)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.692 × 10−1 (1.21 × 10−3) | 9.674 × 10−1 (1.57 × 10−3) | |
| 8 | 9.799 × 10−1 (6.89 × 10−2)+(†) | 7.815 × 10−1 (3.00 × 10−1)+(†) | 8.119 × 10−1 (2.28 × 10−1)+(†) | 9.949 × 10−1 (9.25 × 10−4)-(↓) | 9.161 × 10−1 (1.24 × 10−2)+(†) | 9.927 × 10−1 (7.03 × 10−4)=(†) | 4.673 × 10−1 (1.80 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.927 × 10−1 (1.67 × 10−3) | 9.930 × 10−1 (1.39 × 10−3) | |
| 10 | 9.151 × 10−1 (1.63 × 10−1)+(†) | 7.083 × 10−1 (2.67 × 10−1)+(†) | 8.870 × 10−1 (1.78 × 10−1)+(†) | 9.989 × 10−1 (1.21 × 10−4)=(≡) | 9.313 × 10−1 (8.05 × 10−3)+(†) | 9.989 × 10−1 (2.04 × 10−4)=(≡) | 5.374 × 10−1 (1.85 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.989 × 10−1 (2.43 × 10−4) | 9.989 × 10−1 (3.79 × 10−4) | |
| DTLZ2 | 3 | 5.621 × 10−1 (5.01 × 10−4)+(†) | 5.593 × 10−1 (1.66 × 10−3)+(†) | 5.651 × 10−1 (1.11 × 10−3)+(≡) | 5.578 × 10−1 (2.22 × 10−3)+(†) | 5.386 × 10−1 (6.89 × 10−3)+(†) | 5.582 × 10−1 (1.35 × 10−3)+(†) | 5.435 × 10−1 (4.04 × 10−3)+(†) | 5.2890 × 10−1 (4.80 × 10−3)+(†) | 5.659 × 10−1 (1.21 × 10−3) | 5.651 × 10−1 (1.08 × 10−3) |
| 5 | 7.921 × 10−1 (6.97 × 10−4)+(†) | 7.878 × 10−1 (2.39 × 10−3)+(†) | 7.717 × 10−1 (3.04 × 10−3)+(†) | 7.807 × 10−1 (3.33 × 10−3)+(†) | 6.582 × 10−1 (1.96 × 10−2)+(†) | 7.831 × 10−1 (2.91 × 10−3)+(†) | 7.508 × 10−1 (8.13 × 10−3)+(†) | 0.2011 × 10−1 (2.25 × 10−2)+(†) | 7.976 × 10−1 (1.65 × 10−3) | 7.964 × 10−1 (2.12 × 10−3) | |
| 8 | 9.065 × 10−1 (3.91 × 10−2)-(↓) | 9.059 × 10−1 (3.16 × 10−3)-(↓) | 8.889 × 10−1 (5.62 × 10−3)=(↓) | 8.960 × 10−1 (4.84 × 10−3)-(↓) | 6.764 × 10−1 (2.05 × 10−2)+(†) | 9.162 × 10−1 (2.49 × 10−3)-(↓) | 8.889 × 10−1 (8.71 × 10−3)=(↓) | 0.000 × 100 (0.00 × 100)+(†) | 8.889 × 10−1 (1.05 × 10−2) | 8.762 × 10−1 (1.52 × 10−2) | |
| 10 | 9.612 × 10−1 (2.06 × 10−2)-(↓) | 9.500 × 10−1 (3.37 × 10−3)-(↓) | 9.106 × 10−1 (1.49 × 10−2)+(†) | 9.493 × 10−1 (1.67 × 10−3)-(↓) | 6.979 × 10−1 (1.53 × 10−2)+(†) | 9.646 × 10−1 (1.09 × 10−3)-(↓) | 9.502 × 10−1 (4.24 × 10−3)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 9.273 × 10−1 (8.23 × 10−3) | 9.215 × 10−1 (1.14 × 10−2) | |
| DTLZ3 | 3 | 5.546 × 10−1 (5.19 × 10−3)=(↓) | 4.060 × 10−1 (7.83 × 10−2)+(†) | 5.471 × 10−1 (9.45 × 10−3)+(↓) | 5.437 × 10−1 (9.50 × 10−3)+(↓) | 5.036 × 10−1 (1.08 × 10−2)+(†) | 5.148 × 10−1 (1.01 × 10−1)+(†) | 4.663 × 10−1 (1.11 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 5.546 × 10−1 (2.61 × 10−2) | 5.248 × 10−1 (8.89 × 10−3) |
| 5 | 7.766 × 10−1 (3.23 × 10−2)-(↓) | 1.274 × 10−1 (1.61 × 10−1)+(†) | 4.929 × 10−1 (2.10 × 10−1)+(†) | 7.606 × 10−1 (1.10 × 10−2)=(↓) | 1.221 × 10−3 (6.57 × 10−3)+(†) | 7.756 × 10−1 (7.05 × 10−3)-(↓) | 3.779 × 10−1 (1.95 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 7.606 × 10−1 (6.16 × 10−2) | 6.724 × 10−1 (2.07 × 10−1) | |
| 8 | 6.727 × 10−1 (2.16 × 10−1)+(†) | 0.000 × 100 (0.00 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 8.890 × 10−1 (9.47 × 10−3)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 9.015 × 10−1 (8.05 × 10−3)-(↓) | 1.670 × 10−1 (9.99 × 10−2)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 7.730 × 10−1 (2.07 × 10−1) | 7.109 × 10−1 (2.26 × 10−1) | |
| 10 | 8.311 × 10−1 (1.48 × 10−1)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.484 × 10−1 (2.84 × 10−3)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 9.566 × 10−1 (6.92 × 10−3)-(↓) | 1.392 × 10−1 (6.86 × 10−2)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.334 × 10−1 (7.34 × 10−3) | 8.773 × 10−1 (1.17 × 10−1) | |
| DTLZ4 | 3 | 3.615 × 10−1 (1.53 × 10−1)+(†) | 5.583 × 10−1 (1.55 × 10−3)-(↓) | 5.570 × 10−1 (1.57 × 10−3)-(↓) | 5.298 × 10−1 (7.38 × 10−2)+(†) | 2.688 × 10−1 (6.04 × 10−2)+(†) | 5.571 × 10−1 (1.65 × 10−3)-(↓) | 5.364 × 10−1 (5.65 × 10−3)+(≡) | 4.8446 × 10−1 (6.01 × 10−2)+(†) | 5.442 × 10−1 (6.69 × 10−2) | 5.364 × 10−1 (7.57 × 10−2) |
| 5 | 7.059 × 10−1 (8.25 × 10−2)+(†) | 7.857 × 10−1 (2.54 × 10−3)=(↓) | 7.700 × 10−1 (3.25 × 10−3)+(†) | 7.857 × 10−1 (1.55 × 10−2)=(↓) | 3.766 × 10−1 (5.43 × 10−2)+(†) | 7.857 × 10−1 (2.81 × 10−3)=(↓) | 7.603 × 10−1 (3.85 × 10−3)+(†) | 2.9786 × 10−1 (5.66 × 10−2)+(†) | 7.857 × 10−1 (3.27 × 10−2) | 7.760 × 10−1 (4.59 × 10−2) | |
| 8 | 8.837 × 10−1 (5.07 × 10−2)+(†) | 9.006 × 10−1 (5.49 × 10−3)+(†) | 8.772 × 10−1 (9.07 × 10−3)+(†) | 9.109 × 10−1 (3.59 × 10−3)+(≡) | 4.998 × 10−1 (5.74 × 10−2)+(†) | 9.243 × 10−1 (2.37 × 10−3)-(↓) | 9.040 × 10−1 (4.47 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.144 × 10−1 (1.25× 10−2) | 9.109 × 10−1 (1.36 × 10−2) | |
| 10 | 9.429 × 10−1 (3.52 × 10−2)+(†) | 9.380 × 10−1 (6.35 × 10−3)+(†) | 8.996 × 10−1 (1.46 × 10−2)+(†) | 9.609 × 10−1 (1.52 × 10−3)=(†) | 6.318 × 10−1 (3.65 × 10−2)+(†) | 9.702 × 10−1 (1.08 × 10−3)-(↓) | 9.583 × 10−1 (2.20 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 9.609 × 10−1 (3.56 × 10−3) | 9.668 × 10−1 (3.66 × 10−3) | |
| DTLZ5 | 3 | 1.938 × 10−1 (1.93 × 10−3)+(†) | 1.860 × 10−1 (1.76 × 10−3)+(†) | 1.998 × 10−1 (3.01 × 10−4)=(≡) | 1.982 × 10−1 (6.43 × 10−4)+(†) | 1.998 × 10−1 (1.99 × 10−3)=(≡) | 1.998 × 10−1 (4.45 × 10−4)=(≡) | 1.928 × 10−1 (4.11 × 10−3)+(†) | 1.998 × 10−1 (6.01 × 10−4)=(≡) | 1.998 × 10−1 (4.50 × 10−4) | 1.998 × 10−1 (5.60 × 10−4) |
| 5 | 1.246 × 10−1 (1.58 × 10−3)=(≡) | 2.536 × 10−2 (2.30 × 10−2)+(†) | 9.292 × 10−2 (5.92 × 10−3)+(†) | 1.064 × 10−1 (4.15 × 10−3)+(†) | 1.246 × 10−1 (2.91 × 10−3)=(≡) | 1.145 × 10−1 (2.65 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 0.8839 × 10−1 (8.31 × 10−3)+(†) | 1.246 × 10−1 (1.40 × 10−3) | 1.246 × 10−1 (1.14 × 10−3) | |
| 8 | 9.847 × 10−2 (1.90 × 10−3)+(†) | 3.295 × 10−3 (1.14 × 10−2)+(†) | 8.150 × 10−2 (5.84 × 10−3)+(†) | 4.805 × 10−2 (1.56 × 10−2)+(†) | 7.354 × 10−2 (9.58 × 10−3)+(†) | 8.947 × 10−2 (5.40 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 0.4154 × 10−1 (2.01 × 10−2)+(†) | 1.003 × 10−1 (2.23 × 10−3) | 1.003 × 10−1 (3.97 × 10−3) | |
| 10 | 9.526 × 10−2 (1.17 × 10−3)=(≡) | 1.470 × 10−3 (7.63 × 10−3)+(†) | 7.226 × 10−2 (9.91 × 10−3)+(†) | 3.393 × 10−2 (1.99 × 10−2)+(†) | 5.544 × 10−2 (1.23 × 10−2)+(†) | 8.530 × 10−2 (6.39 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 4.0901 × 10−2 (1.63 × 10−2)+(†) | 9.526 × 10−2 (2.17 × 10−3) | 9.526 × 10−2 (2.81 × 10−3) | |
| DTLZ6 | 3 | 1.879 × 10−1 (5.33 × 10−3)+(†) | 1.837 × 10−1 (2.18 × 10−3)+(†) | 1.999 × 10−1 (4.60 × 10−4)=(≡) | 1.989 × 10−1 (1.67 × 10−3)+(†) | 1.961 × 10−1 (1.35 × 10−3)+(†) | 1.999 × 10−1 (4.54 × 10−4)=(≡) | 1.879 × 10−1 (6.77 × 10−3)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 1.999 × 10−1 (4.83 × 10−4) | 1.999 × 10−1 (9.30 × 10−4) |
| 5 | 1.047 × 10−1 (9.41 × 10−3)+(†) | 3.482 × 10−3 (8.66 × 10−3)+(†) | 3.271 × 10−2 (4.08 × 10−2)+(†) | 1.095 × 10−1 (4.70 × 10−3)+(†) | 1.242 × 10−1 (2.69 × 10−3)-(↓) | 1.150 × 10−1 (3.68 × 10−3)+(†) | 4.538 × 10−2 (4.62 × 10−2)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 1.165 × 10−1 (3.42 × 10−3) | 1.152 × 10−1 (2.29 × 10−2) | |
| 8 | 7.919 × 10−2 (3.16 × 10−2)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 7.075 × 10−2 (2.14 × 10−2)-(↓) | 9.317 × 10−2 (9.28 × 10−3)-(↓) | 9.434 × 10−2 (7.31 × 10−3)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 5.073 × 10−2 (4.24 × 10−2) | 3.618 × 10−2 (3.55 × 10−2) | |
| 10 | 8.399 × 10−2 (1.57 × 10−2)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 5.632 × 10−2 (2.91 × 10−2)+(↓) | 7.596 × 10−2 (1.42 × 10−2)=(↓) | 8.682 × 10−2 (1.18 × 10−2)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 7.596 × 10−2 (2.79 × 10−2) | 5.437 × 10−2 (3.99 × 10−2) | |
| DTLZ7 | 3 | 4.160 × 10−1 (1.60 × 10−2)-(↓) | 4.119 × 10−1 (2.41 × 10−3)-(↓) | 4.178 × 10−1 (1.63 × 10−2)-(↓) | 4.118 × 10−1 (2.40 × 10−2)-(↓) | 3.995 × 10−1 (6.24 × 10−3)-(↓) | 4.195 × 10−1 (1.59 × 10−2)-(↓) | 3.844 × 10−1 (2.42 × 10−2)=(†) | 3.9261 × 10−1 (2.83 × 10−2)-(↓) | 3.844 × 10−1 (3.70 × 10−2) | 3.882 × 10−1 (3.93 × 10−2) |
| 5 | 3.113 × 10−1 (4.83 × 10−3)+(†) | 3.008 × 10−1 (3.31 × 10−3)+(†) | 3.036 × 10−1 (5.11 × 10−3)+(†) | 3.251 × 10−1 (3.57 × 10−3)-(≡) | 3.171 × 10−1 (5.86 × 10−3)+(†) | 3.010 × 10−1 (1.18 × 10−2)+(†) | 2.583 × 10−1 (2.13 × 10−2)+(†) | 1.1457 × 10−1 (2.52 × 10−2)+(†) | 3.239 × 10−1 (1.80 × 10−2) | 3.251 × 10−1 (1.45 × 10−2) | |
| 8 | 2.067 × 10−1 (7.55 × 10−3)+(†) | 1.801 × 10−1 (1.84 × 10−2)+(†) | 1.877 × 10−1 (8.11 × 10−3)+(†) | 9.480 × 10−2 (1.37 × 10−2)+(†) | 2.550 × 10−1 (4.17 × 10−3)-(↓) | 5.193 × 10−2 (1.96 × 10−2)+(†) | 1.508 × 10−1 (1.36 × 10−2)+(†) | 3.33 × 10−8 (0.00 × 100)+(†) | 2.227 × 10−1 (3.73 × 10−2) | 2.110 × 10−1 (3.09 × 10−2) | |
| 10 | 1.909 × 10−1 (7.00 × 10−3)-(↓) | 1.590 × 10−1 (1.25 × 10−2)+(†) | 1.394 × 10−1 (1.30 × 10−2)+(†) | 1.054 × 10−1 (2.60 × 10−2)+(†) | 2.403 × 10−1 (2.93 × 10−3)-(↓) | 8.381 × 10−3 (3.76 × 10−3)+(†) | 1.321 × 10−1 (1.16 × 10−2)+(†) | 0.000 × 100 (0.00 × 100)+(†) | 0.1862 (3.79 × 10−2) | 1.736 × 10−1 (4.27 × 10−2) | |
| Ad-GrMODE1* (+/=/-) | 16/3/9 | 22/2/4 | 23/3/2 | 15/5/8 | 20/3/5 | 12/5/11 | 25/2/1 | 26/1/1 | |||
| Ad-GrMODE2* (†/≡/↓) | 16/2/10 | 22/1/5 | 21/3/4 | 13/3/12 | 20/2/6 | 13/3/12 | 25/1/2 | 26/1/1 | |||
| # | M | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | adGrMOEA1* | adGrMOEA2* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| WFG1 | 3 | 2.800 × 10−1 (3.73 × 10−2)+(†) | 3.087 × 10−1 (3.56 × 10−4)-(↓) | 3.049 × 10−1 (1.17 × 10−3)-(↓) | 3.021 × 10−1 (1.92 × 10−3)-(↓) | 0.000 × 100 (0.00 × 100)+(†) | 1.414 × 10−1 (1.48 × 10−2)+(†) | 1.625 × 10−1 (2.68 × 10−2)+(†) | 1.71 × 10−1 (2.15 × 10−2)+(†) | 2.855 × 10−1 (2.77 × 10−3) | 2.827 × 10−1 (3.13 × 10−3) |
| 5 | 2.597 × 10−1 (4.04 × 10−2)+(†) | 2.431 × 10−1 (4.29 × 10−2)+(†) | 2.843 × 10−1 (4.47 × 10−4)-(↓) | 2.657 × 10−1 (8.09 × 10−4)+(†) | 8.122 × 10−3 (1.17 × 10−2)+(†) | 2.660 × 10−1 (2.57 × 10−3)+(†) | 2.306 × 10−1 (1.44 × 10−2)+(†) | 1.78 × 10−1 (1.54 × 10−2)+(†) | 2.801 × 10−1 (9.09 × 10−4) | 2.797 × 10−1 (9.22 × 10−4) | |
| 8 | 2.294 × 10−1 (2.84 × 10−3)=(≡) | 2.138 × 10−1 (1.70 × 10−2)+(†) | 2.311 × 10−1 (4.71 × 10−4)-(↓) | 2.198 × 10−1 (6.64 × 10−4)+(†) | 7.802 × 10−2 (9.71 × 10−3)+(†) | 2.294 × 10−1 (3.72 × 10−4)=(≡) | 1.966 × 10−1 (1.38 × 10−2)+(†) | 1.87 × 10−1 (1.26 × 10−2)+(†) | 2.294 × 10−1 (6.10 × 10−4) | 2.294 × 10−1 (5.30 × 10−4) | |
| 10 | 2.071 × 10−1 (3.94 × 10−4)=(≡) | 2.071 × 10−1 (3.38 × 10−4)=(≡) | 2.031 × 10−1 (4.76 × 10−4)+(†) | 2.071 × 10−1 (4.07 × 10−4)=(≡) | 1.065 × 10−1 (6.96 × 10−3)+(†) | 2.071 × 10−1 (3.83 × 10−4)=(≡) | 1.816 × 10−1 (9.85 × 10−3)+(†) | 1.93 × 10−1 (7.18 × 10−3)+(†) | 2.071 × 10−1 (5.19 × 10−4) | 2.071 × 10−1 (4.70 × 10−4) | |
| WFG2 | 3 | 2.352 × 10−1 (4.84 × 10−4)=(≡) | 2.352 × 10−1 (4.56 × 10−4)=(≡) | 2.352 × 10−1 (5.03 × 10−4)=(≡) | 2.352 × 10−1 (5.54 × 10−4)=(≡) | 2.352 × 10−1 (5.49 × 10−4)=(≡) | 2.352 × 10−1 (5.14 × 10−4)=(≡) | 2.267 × 10−1 (3.50 × 10−3)+(†) | 2.35 × 10−1 (2.83 × 10−4)=(≡) | 2.352 × 10−1 (5.35 × 10−4) | 2.352 × 10−1 (4.91 × 10−4) |
| 5 | 2.115 × 10−1 (4.90 × 10−4)=(≡) | 2.115 × 10−1 (4.13 × 10−4)=(≡) | 2.115 × 10−1 (5.11 × 10−4)=(≡) | 2.115 × 10−1 (4.79 × 10−4)=(≡) | 2.115 × 10−1 (6.47 × 10−4)=(≡) | 2.115 × 10−1 (4.10 × 10−4)=(≡) | 1.416 × 10−1 (4.46 × 10−2)+(†) | 2.11 × 10−1 (4.19 × 10−4)=(≡) | 2.115 × 10−1 (4.77 × 10−4) | 2.115 × 10−1 (3.26 × 10−4) | |
| 8 | 1.855 × 10−1 (3.02 × 10−4)=(≡) | 1.855 × 10−1 (4.29 × 10−4)=(≡) | 1.855 × 10−1 (4.40 × 10−4)=(≡) | 1.855 × 10−1 (4.73 × 10−4)=(≡) | 1.855 × 10−1 (3.75 × 10−4)=(≡) | 1.855 × 10−1 (4.01 × 10−4)=(≡) | 9.438 × 10−2 (1.78 × 10−2)+(†) | 1.85 × 10−1 (3.58 × 10−4)=(≡) | 1.855 × 10−1 (4.80 × 10−4) | 1.855 × 10−1 (3.67 × 10−4) | |
| 10 | 1.730 × 10−1 (3.92× 10−4)=(≡) | 1.730 × 10−1 (3.65 × 10−4)=(≡) | 1.730 × 10−1 (3.25 × 10−4)=(≡) | 1.730 × 10−1 (3.92 × 10−4)=(≡) | 1.730 × 10−1 (3.66 × 10−4)=(≡) | 1.730 × 10−1 (3.12 × 10−4)=(≡) | 9.701 × 10−2 (2.36 × 10−2)+(†) | 1.73 × 10−1 (3.10 × 10−4)=(≡) | 1.730 × 10−1 (3.11 × 10−4) | 1.730 × 10−1 (3.56 × 10−4) | |
| WFG3 | 3 | 8.360 × 10−2 (5.18 × 10−4)+(†) | 8.033 × 10−2 (5.34 × 10−4)+(†) | 8.533 × 10−2 (3.69 × 10−4)+(†) | 8.448 × 10−2 (5.13 × 10−4)+(†) | 8.458 × 10−2 (3.41 × 10−4)+(†) | 8.362 × 10−2 (4.84 × 10−4)+(†) | 3.364 × 10−2 (1.20 × 10−2)+(†) | 9.10 × 10−2 (3.81 × 10−4)+(†) | 9.250 × 10−2 (6.91 × 10−4) | 9.209 × 10−2 (4.94 × 10−4) |
| 5 | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.00 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100) | 0.000 × 100 (0.00 × 100) | |
| 8 | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.00 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100) | 0.000 × 100 (0.00 × 100) | |
| 10 | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100)=(≡) | 0.00 × 100 (0.00 × 100)=(≡) | 0.000 × 100 (0.00 × 100) | 0.000 × 100 (0.00 × 100) | |
| WFG4 | 3 | 1.964 × 10−1 (1.12 × 10−3)=(≡) | 1.956 × 10−1 (6.19 × 10−4)+(†) | 1.933 × 10−1 (1.73 × 10−3)+(†) | 1.946 × 10−1 (1.67 × 10−3)+(†) | 1.596 × 10−1 (6.36 × 10−3)+(†) | 1.728 × 10−1 (3.79 × 10−3)+(†) | 1.836 × 10−1 (4.02 × 10−3)+(†) | 1.70 × 10−1 (7.22 × 10−3)+(†) | 1.964 × 10−1 (1.70 × 10−3) | 1.964 × 10−1 (1.66 × 10−3) |
| 5 | 2.535 × 10−1 (2.46 × 10−3)-(↓) | 2.475 × 10−1 (1.71 × 10−3)-(↓) | 2.429 × 10−1 (2.44 × 10−3)-(↓) | 2.355 × 10−1 (4.35 × 10−3)-(↓) | 1.603 × 10−1 (7.29 × 10−3)+(†) | 1.199 × 10−1 (1.70 × 10−2)+(†) | 2.341 × 10−1 (3.47 × 10−3)-(↓) | 2.09 × 10−1 (1.13 × 10−2)+(†) | 2.257 × 10−1 (5.15 × 10−3) | 2.237 × 10−1 (6.17 × 10−3) | |
| 8 | 2.127 × 10−1 (1.63 × 10−2)-(↓) | 2.227 × 10−1 (4.46 × 10−3)-(↓) | 2.241 × 10−1 (2.62 × 10−3)-(↓) | 1.949 × 10−1 (9.35 × 10−3)-(↓) | 1.331 × 10−1 (4.34 × 10−3)+(†) | 1.674 × 10−2 (1.14 × 10−2)+(†) | 2.053 × 10−1 (5.61 × 10−3)-(↓) | 1.89 × 10−1 (4.74 × 10−3)-(↓) | 1.685 × 10−1 (1.10 × 10−2) | 1.692 × 10−1 (9.86 × 10−3) | |
| 10 | 2.150 × 10−1 (9.07 × 10−3)-(↓) | 2.178 × 10−1 (2.47 × 10−3)-(↓) | 2.110 × 10−1 (3.63 × 10−3)-(↓) | 2.011 × 10−1 (6.87 × 10−3)-(↓) | 1.281 × 10−1 (3.51 × 10−3)+(†) | 6.537 × 10−3 (5.84 × 10−3)+(†) | 1.950 × 10−1 (3.18 × 10−3)-(↓) | 1.83 × 10−1 (3.66 × 10−3)-(↓) | 1.598 × 10−1 (1.05 × 10−2) | 1.624 × 10−1 (6.79 × 10−3) | |
| WFG5 | 3 | 4.584 × 10−1 (1.49 × 10−3)=(≡) | 4.584 × 10−1 (1.47 × 10−3)=(≡) | 4.608 × 10−1 (8.70 × 10−4)-(↓) | 4.525 × 10−1 (1.83 × 10−3)+(†) | 4.301 × 10−1 (3.83 × 10−3)+(†) | 4.486 × 10−1 (2.76 × 10−3)+(†) | 4.346 × 10−1 (5.11 × 10−3)+(†) | 4.56 × 10−1 (1.19 × 10−3)+(†) | 4.584 × 10−1 (1.55 × 10−3) | 4.584 × 10−1 (1.75 × 10−3) |
| 5 | 4.652 × 10−1 (2.70 × 10−3)+(≡) | 4.458 × 10−1 (2.88 × 10−3)+(†) | 4.708 × 10−1 (2.17 × 10−3)-(↓) | 4.597 × 10−1 (5.14 × 10−3)+(†) | 4.305 × 10−1 (5.20 × 10−3)+(†) | 4.606 × 10−1 (5.10 × 10−3)+(†) | 4.459 × 10−1 (5.07 × 10−3)+(†) | 4.68 × 10−1 (3.08 × 10−3)-(↓) | 4.671 × 10−1 (3.59 × 10−3) | 4.652 × 10−1 (3.65 × 10−3) | |
| 8 | 4.612 × 10−1 (3.15 × 10−3)+(†) | 4.368 × 10−1 (4.12 × 10−3)+(†) | 4.536 × 10−1 (2.81 × 10−3)+(†) | 4.533 × 10−1 (3.81 × 10−3)+(†) | 4.347 × 10−1 (4.10 × 10−3)+(†) | 4.557 × 10−1 (4.70 × 10−3)+(†) | 4.357 × 10−1 (7.30 × 10−3)+(†) | 4.65 × 10−1 (1.36 × 10−3)=(↓) | 4.656 × 10−1 (3.69 × 10−3) | 4.640 × 10−1 (3.76 × 10−3) | |
| 10 | 4.682 × 10−1 (2.88 × 10−3)+(†) | 4.426 × 10−1 (3.73 × 10−3)+(†) | 4.636 × 10−1 (1.93 × 10−3)+(†) | 4.608 × 10−1 (2.38 × 10−3)+(†) | 4.499 × 10−1 (2.63 × 10−3)+(†) | 4.886 × 10−1 (3.26 × 10−3)-(↓) | 4.356 × 10−1 (6.70 × 10−2)+(†) | 4.70 × 10−1 (1.31 × 10−3)+(†) | 4.770 × 10−1 (3.02 × 10−3) | 4.763 × 10−1 (2.78 × 10−3) | |
| WFG6 | 3 | 1.878 × 10−1 (2.09 × 10−3)+(†) | 1.887 × 10−1 (6.67 × 10−4)+(†) | 1.894 × 10−1 (6.80 × 10−4)+(†) | 1.897 × 10−1 (7.73 × 10−4)+(†) | 1.858 × 10−1 (1.35 × 10−3)+(†) | 1.915 × 10−1 (5.98 × 10−4)=(≡) | 1.864 × 10−1 (3.84 × 10−3)+(†) | 1.89 × 10−1 (9.51 × 10−4)+(†) | 1.915 × 10−1 (5.18 × 10−4) | 1.915 × 10−1 (4.54 × 10−4) |
| 5 | 1.908 × 10−1 (9.12 × 10−4)+(†) | 1.887 × 10−1 (1.34 × 10−3)+(†) | 1.908 × 10−1 (8.89 × 10−4)+(†) | 1.905 × 10−1 (8.90 × 10−4)+(†) | 1.879 × 10−1 (1.33 × 10−3)+(†) | 1.920 × 10−1 (9.28 × 10−4)=(≡) | 1.843 × 10−1 (4.19 × 10−3)+(†) | 1.92 × 10−1 (6.58 × 10−4)=(≡) | 1.920 × 10−1 (4.44 × 10−4) | 1.920 × 10−1 (4.38 × 10−4) | |
| 8 | 1.893 × 10−1 (1.10 × 10−3)=(≡) | 1.838 × 10−1 (2.80 × 10−3)+(†) | 1.893 × 10−1 (7.27 × 10−4)=(≡) | 1.874 × 10−1 (1.34 × 10−3)+(†) | 1.862 × 10−1 (7.79 × 10−4)+(†) | 1.882 × 10−1 (6.69 × 10−4)+(†) | 1.810 × 10−1 (4.44 × 10−3)+(†) | 1.89 × 10−1 (5.84 × 10−4)=(≡) | 1.893 × 10−1 (5.00 × 10−4) | 1.893 × 10−1 (6.27 × 10−4) | |
| 10 | 1.812 × 10−1 (9.56 × 10−4)+(†) | 1.723 × 10−1 (4.14 × 10−3)+(†) | 1.780 × 10−1 (5.21 × 10−4)+(†) | 1.867 × 10−1 (6.13 × 10−4)+(†) | 1.855 × 10−1 (9.59 × 10−4)+(†) | 1.881 × 10−1 (3.36 × 10−4)=(≡) | 1.815 × 10−1 (2.95 × 10−3)+(†) | 1.88 × 10−1 (5.15 × 10−4)=(≡) | 1.881 × 10−1 (3.57 × 10−4) | 1.881 × 10−1 (3.56 × 10−4) | |
| WFG7 | 3 | 8.092 × 10−2 (1.54 × 10−2)+(†) | 8.430 × 10−2 (2.24 × 10−3)+(†) | 9.021 × 10−2 (1.06 × 10−3)+(†) | 9.506 × 10−2 (9.43 × 10−4)-(↓) | 4.362 × 10−3 (4.36 × 10−3)+(†) | 5.366 × 10−2 (1.02 × 10−2)+(†) | 8.373 × 10−2 (2.03 × 10−3)+(†) | 7.65 × 10−2 (2.14 × 10−3)+(†) | 9.312 × 10−2 (1.39 × 10−3) | 9.251 × 10−2 (1.58 × 10−3) |
| 5 | 7.877 × 10−2 (5.86 × 10−2)+(†) | 1.421 × 10−1 (1.65 × 10−3)+(†) | 1.490 × 10−1 (1.21 × 10−3)-(↓) | 1.521 × 10−1 (1.58 × 10−3)-(↓) | 8.289 × 10- (5.32 × 10−3)+(†) | 8.907 × 10−2 (1.24 × 10−2)+(†) | 1.448 × 10−1 (3.76 × 10−3)=(≡) | 1.18 × 10−1 (9.11 × 10−3)+(†) | 1.448 × 10−1 (4.29 × 10−3) | 1.448 × 10−1 (3.25 × 10−3) | |
| 8 | 1.175 × 10−1 (4.62 × 10−2)+(†) | 1.502 × 10−1 (7.26 × 10−3)+(†) | 1.490 × 10−1 (1.40 × 10−3)+(†) | 1.641 × 10−1 (2.13 × 10−3)-(↓) | 1.058 × 10−2 (2.54 × 10−3)+(†) | 1.274 × 10−1 (7.73 × 10−3)+(†) | 1.371 × 10−1 (4.09 × 10−3)+(†) | 9.86 × 10−2 (1.37 × 10−2)+(†) | 1.540 × 10−1 (2.68 × 10−3) | 1.512 × 10−1 (3.85 × 10−3) | |
| 10 | 1.544 × 10−1 (5.14 × 10−3)=(≡) | 1.544 × 10−1 (3.41 × 10−3)=(≡) | 1.544 × 10−1 (1.41 × 10−3)=(≡) | 1.618 × 10−1 (2.06 × 10−3)-(↓) | 1.201 × 10−2 (1.77 × 10−3)+(†) | 1.430 × 10−1 (4.65 × 10−3)+(†) | 1.409 × 10−1 (3.19 × 10−3)+(†) | 8.59 × 10−2 (7.42 × 10−3)+(†) | 1.544 × 10−1 (1.94 × 10−3) | 1.544 × 10−1 (2.23 × 10−3) | |
| WFG8 | 3 | 2.257 × 10−1 (1.14 × 10−3)+(†) | 2.281 × 10−1 (1.00 × 10−3)+(†) | 2.236 × 10−1 (1.24 × 10−3)+(†) | 2.277 × 10−1 (1.08 × 10−3)+(†) | 2.314 × 10−1 (2.40 × 10−3)=(≡) | 2.277 × 10−1 (7.22 × 10−4)+(†) | 2.243 × 10−1 (2.88 × 10−3)+(†) | 2.24 × 10−1 (9.67 × 10−4)+(†) | 2.314 × 10−1 (7.68 × 10−4) | 2.314 × 10−1 (8.87 × 10−4) |
| 5 | 2.325 × 10−1 (1.21 × 10−3)+(†) | 2.334 × 10−1 (1.65 × 10−3)+(†) | 2.317 × 10−1 (8.80 × 10−4)+(†) | 2.311 × 10−1 (1.28 × 10−3)+(†) | 2.293 × 10−1 (1.94 × 10−3)+(†) | 2.333 × 10−1 (6.80 × 10−4)+(†) | 1.674 × 10−1 (2.49 × 10−2)+(†) | 2.32 × 10−1 (7.55 × 10−4)+(†) | 2.386 × 10−1 (6.68 × 10−4) | 2.382 × 10−1 (6.27 × 10−4) | |
| 8 | 2.299 × 10−1 (1.58 × 10−3)+(†) | 2.180 × 10−1 (6.92 × 10−3)+(†) | 2.355 × 10−1 (7.91 × 10−4)+(†) | 2.334 × 10−1 (1.32 × 10−3)+(†) | 2.325 × 10−1 (1.64 × 10−3)+(†) | 2.345 × 10−1 (9.49 × 10−4)+(†) | 1.428 × 10−1 (1.38 × 10−2)+(†) | 2.35 × 10−1 (8.82 × 10−4)+(†) | 2.408 × 10−1 (7.60 × 10−4) | 2.403 × 10−1 (6.83 × 10−4) | |
| 10 | 2.334 × 10−1 (2.07 × 10−3)+(†) | 2.217 × 10−1 (4.81 × 10−3)+(†) | 2.385 × 10−1 (5.64 × 10−4)+(†) | 2.374 × 10−1 (6.99 × 10−4) +(†) | 2.342 × 10−1 (9.34 × 10−4)+(†) | 2.360 × 10−1 (9.87 × 10−4)+(†) | 1.451 × 10−1 (6.89 × 10−3)+(†) | 2.37 × 10−1 (6.63 × 10−4)+(†) | 2.426 × 10−1 (5.27 × 10−4) | 2.424 × 10−1 (5.30 × 10−4) | |
| WFG9 | 3 | 3.763 × 10−1 (5.81 × 10−3)=(↓) | 3.720 × 10−1 (4.90 × 10−3)+(†) | 3.682 × 10−1 (6.89 × 10−3)+(†) | 3.828 × 10−1 (5.06 × 10−3)-(↓) | 2.053 × 10−1 (1.20 × 10−2)+(†) | 3.658 × 10−1 (3.88 × 10−3)+(†) | 3.600 × 10−1 (5.93 × 10−3)+(†) | 3.40 × 10−1 (6.05 × 10−3)+(†) | 3.763 × 10−1 (4.30 × 10−3) | 3.757 × 10−1 (3.68 × 10−3) |
| 5 | 5.388 × 10−1 (1.43 × 10−2)-(↓) | 5.447 × 10−1 (9.97 × 10−3)-(↓) | 5.258 × 10−1 (9.08 × 10−3)-(↓) | 5.209 × 10−1 (6.09 × 10−3)-(↓) | 1.816 × 10−1 (1.16 × 10−2)+(†) | 5.046 × 10−1 (7.77 × 10−3)-(↓) | 4.995 × 10−1 (1.03 × 10−2)=(↓) | 4.40 × 10−1 (2.19 × 10−2)+(†) | 4.995 × 10−1 (1.20 × 10−2) | 4.932 × 10−1 (1.57 × 10−2) | |
| 8 | 5.861 × 10−1 (2.19 × 10−2)-(↓) | 6.050 × 10−1 (1.54 × 10−2)-(↓) | 5.903 × 10−1 (1.29 × 10−2)-(↓) | 5.657 × 10−1 (1.81 × 10−2)-(↓) | 1.684 × 10−1 (1.07 × 10−2)+(†) | 5.131 × 10−1 (1.29 × 10−2)-(↓) | 3.683 × 10−1 (8.25 × 10−2)+(†) | 3.82 × 10−1 (1.31 × 10−2)+(†) | 4.675 × 10−1 (1.89 × 10−2) | 4.597 × 10−1 (1.99 × 10−2) | |
| 10 | 6.254 × 10−1 (1.56 × 10−2)-(↓) | 6.290 × 10−1 (1.34 × 10−2)-(↓) | 5.938 × 10−1 (9.68 × 10−3)-(↓) | 5.875 × 10−1 (1.31 × 10−2)-(↓) | 1.706 × 10−1 (9.90 × 10−3)+(†) | 5.407 × 10−1 (9.80 × 10−3)-(↓) | 4.069 × 10−1 (6.41 × 10−2)+(†) | 3.98 × 10−1 (1.17 × 10−2)+(†) | 4.957 × 10−1 (1.30 × 10−2) | 4.848 × 10−1 (1.30 × 10−2) | |
| Ad-GrMODE1* (+/=/-) | 16/14/6 | 19/10/7 | 15/9/12 | 16/8/12 | 28/8/0 | 20/12/4 | 28/5/3 | 22/11/3 | |||
| Ad-GrMODE2* (†/≡/↓) | 15/14/7 | 19/10/7 | 15/9/12 | 16/8/12 | 28/8/0 | 20/12/4 | 28/4/4 | 22/10/4 | |||
| # | M | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | adGrMOEA1* | adGrMOEA2* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| DTLZ1 | 3 | 1.79 × 10−2 (9.67 × 10−2)-(↓) | 1.98 × 10−2 (2.68 × 10−3)=(↓) | 3.46 × 10−2 (2.53 × 10−2)+(†) | 2.19 × 10−2 (1.94 × 10−3)+(†) | 3.04 × 10−2 (2.50 × 10−3)+(†) | 2.05 × 10−2 (4.32 × 10−4)+(†) | 8.95 × 10−2 (1.10 × 10−1)+(†) | 1.36 × 100 (1.07 × 100)+(†) | 1.98 × 10−2 (7.92 × 10−4) | 2.01 × 10−2 (7.91 × 10−4) |
| 5 | 6.26 × 10−2 (1.45 × 10−3)+(†) | 9.67 × 10−2 (2.66 × 10−2)+(†) | 1.21 × 10−1 (2.73 × 10−2)+(†) | 6.46 × 10−2 (2.10 × 10−3)+(†) | 9.14 × 10−2 (6.98 × 10−3)+(†) | 6.15 × 10−2 (6.74 × 10−4)+(†) | 1.18 × 10−1 (3.45 × 10−2)+(†) | 1.15 × 10+1 (6.69 × 100)+(†) | 5.48 × 10−2 (1.19 × 10−3) | 5.61 × 10−2 (8.45 × 10−4) | |
| 8 | 1.17 × 10−1 (2.88 × 10−2)+(†) | 2.00 × 10−1 (1.09 × 10−1)+(†) | 2.31 × 10−1 (6.87 × 10−2)+(†) | 1.02 × 10−1 (2.24 × 10−3)=(≡) | 1.71 × 10−1 (1.06 × 10−2)+(†) | 9.95 × 10−2 (6.62 × 10−4)-(↓) | 3.26 × 10−1 (7.21 × 10−2)+(†) | 4.20 × 10−1 (5.49 × 10−1)+(†) | 1.02 × 10−1 (1.72 × 10−3) | 1.02 × 10−1 (1.96 × 10−3) | |
| 10 | 1.57 × 10−1 (8.06 × 10−2)+(†) | 2.46 × 10−1 (9.31 × 10−2)+(†) | 2.27 × 10−1 (7.67 × 10−2)+(†) | 1.07 × 10−1 (1.99 × 10−3)+(≡) | 1.86 × 10−1 (9.50 × 10−3)+(†) | 1.02 × 10−1 (7.09 × 10−4)-(↓) | 3.05 × 10−1 (7.48 × 10−2)+(†) | 1.49 × 10+2 (1.97 × 10+1)+(†) | 1.05 × 10−1 (1.07 × 10−3) | 1.07 × 10−1 (2.44 × 10−3) | |
| DTLZ2 | 3 | 5.50 × 10−2 (1.94 × 10−4)-(↓) | 5.78 × 10−2 (1.31 × 10−3)-(↓) | 5.33 × 10−2 (7.52 × 10−4)-(↓) | 7.75 × 10−2 (6.61 × 10−3)+(↓) | 7.49 × 10−2 (3.89 × 10−3)-(↓) | 5.73 × 10−2 (1.52 × 10−3)-(↓) | 6.84 × 10−2 (2.77 × 10−3)-(↓) | 7.09 × 10−2 (2.62 × 10−3)-(↓) | 7.58 × 10−2 (5.70 × 10−3) | 7.85 × 10−2 (3.76 × 10−3) |
| 5 | 1.85 × 10−1 (5.10 × 10−4)-(↓) | 1.90 × 10−1 (2.87 × 10−3)-(↓) | 1.93 × 10−1 (1.18 × 10−3)-(↓) | 2.07 × 10−1 (2.94 × 10−3)-(↓) | 2.62 × 10−1 (1.34 × 10−2)+(†) | 1.92 × 10−1 (1.65 × 10−3)-(↓) | 2.08 × 10−1 (3.83 × 10−3)-(↓) | 6.72 × 10−1 (4.28 × 10−2)+(†) | 2.20 × 10−1 (3.66 × 10−3) | 2.19 × 10−1 (3.77 × 10−3) | |
| 8 | 3.77 × 10−1 (6.88 × 10−2)+(†) | 3.43 × 10−1 (2.19 × 10−3)+(†) | 3.72 × 10−1 (2.59 × 10−3)+(†) | 3.59 × 10−1 (2.61 × 10−3)+(†) | 5.18 × 10−1 (1.77 × 10−2)+(†) | 3.55 × 10−1 (1.87 × 10−3)+(†) | 3.74 × 10−1 (4.65 × 10−3)+(†) | 1.81 × 100 (3.05 × 10−1)+(†) | 2.76 × 10−1 (1.22 × 10−2) | 2.85 × 10−1 (2.44 × 10−2) | |
| 10 | 4.56 × 10−1 (3.87 × 10−2)+(†) | 4.32 × 10−1 (3.72 × 10−3)=(↓) | 4.39 × 10−1 (8.31 × 10−3)+(†) | 4.09 × 10−1 (1.97 × 10−3)-(↓) | 6.03 × 10−1 (1.88 × 10−2)+(†) | 4.08 × 10−1 (1.49 × 10−3)-(↓) | 4.22 × 10−1 (5.87 × 10−3)-(↓) | 1.45 × 100 (6.20 × 10−2)+(†) | 4.32 × 10−1 (1.58 × 10−2) | 4.37 × 10−1 (1.94 × 10−2) | |
| DTLZ3 | 3 | 5.64 × 10−2 (2.61 × 10−3)-(↓) | 2.3 × 10−1 (1.10 × 10−1)+(†) | 5.57 × 10−2 (4.74 × 10−3)-(↓) | 7.96 × 10−2 (5.58 × 10−3)+(↓) | 1.72 × 10−1 (1.95 × 10−2)+(†) | 8.96 × 10−2 (1.73 × 10−3)+(†) | 1.59 × 10−1 (1.68 × 10−1)+(†) | 6.89 × 10−1 (1.63 × 10−1)+(†) | 7.70 × 10−2 (1.04 × 10−2) | 8.06 × 10−2 (7.97 × 10−2) |
| 5 | 1.93 × 10−1 (2.54 × 10−2)+(†) | 1.28 × 100 (9.29 × 10−1)+(†) | 4.85 × 10−1 (3.92 × 10−1)+(†) | 2.12 × 10−1 (5.38 × 10−3)+(†) | 1.41 × 100 (4.20 × 10−1)+(†) | 1.89 × 10−1 (2.32 × 10−3)+(†) | 6.32 × 10−1 (2.48 × 10−1)+(†) | 1.91 × 10+2 (5.27 × 10+1)+(†) | 1.79 × 10−1 (3.76 × 10−2) | 1.77 × 10−1 (1.73 × 10−1) | |
| 8 | 5.44 × 10−1 (1.61 × 10−1)+(†) | 1.17 × 10−1 (5.50 × 100)+(†) | 5.32 × 100 (2.97 × 100)+(†) | 3.64 × 10−1 (3.54 × 10−3)+(†) | 9.79 × 100 (1.26 × 100)+(†) | 3.49 × 10−1 (2.86 × 10−3)+(†) | 1.15 × 100 (1.75 × 10−1)+(†) | 7.79 × 10+2 (2.13 × 10+2)+(†) | 3.22 × 10−1 (1.24 × 10−1) | 3.41 × 10−1 (2.60 × 10−1) | |
| 10 | 5.55 × 10−1 (1.11 × 10−1)+(†) | 2.57 × 10+1 (1.52 × 10+1)+(†) | 1.09 × 10+1 (4.06 × 100)+(†) | 4.10 × 10−1 (2.45 × 10−3)+(†) | 1.74 × 10+1 (2.76 × 100)+(†) | 4.02 × 10−1 (4.57 × 10−3)+(†) | 1.19 × 100 (4.81 × 10−2)+(†) | 9.29 × 10+2 (1.05 × 10+2)+(†) | 3.40 × 10−1 (1.20 × 10−2) | 3.75 × 10−1 (6.40 × 10−2) | |
| DTLZ4 | 3 | 4.65 × 10−1 (2.93 × 10−1)+(†) | 5.83 × 10−2 (1.67 × 10−3)-(↓) | 5.33 × 10−2 (1.01 × 10−3)-(↓) | 1.40 × 10−1 (1.68 × 10−1)+(≡) | 4.88 × 10−1 (1.18 × 10−1)+(†) | 5.76 × 10−2 (1.41 × 10−3)-(↓) | 7.25 × 10−2 (3.01 × 10−3)-(↓) | 2.09 × 10−1 (1.66 × 10−1)+(†) | 1.23 × 10−1 (1.44 × 10−1) | 1.40 × 10−1 (1.70 × 10−1) |
| 5 | 3.42 × 10−1 (1.46 × 10−1)+(†) | 1.90 × 10−1 (2.68 × 10−3)+(†) | 1.95 × 10−1 (1.45 × 10−3)+(†) | 2.17 × 10−1 (4.08 × 10−2)+(†) | 6.60 × 10−1 (7.93 × 10−2)+(†) | 1.98 × 10−1 (2.46 × 10−3)+(†) | 2.25 × 10−1 (5.47 × 10−3)+(†) | 4.91 × 10−1 (3.07 × 10−2)+(†) | 1.67 × 10−1 (7.58 × 10−2) | 1.80 × 10−1 (8.60 × 10−2) | |
| 8 | 4.29 × 10−1 (8.58 × 10−2)+(†) | 3.75 × 10−1 (4.82 × 10−3)+(†) | 3.75 × 10−1 (4.43 × 10−3)+(†) | 3.65 × 10−1 (2.48 × 10−3)+(†) | 7.29 × 10−1 (4.92 × 10−2)+(†) | 3.71 × 10−1 (2.47 × 10−3)+(†) | 4.10 × 10−1 (6.69 × 10−3)+(†) | 1.59 × 100 (1.91 × 10−1)+(†) | 3.29 × 10−1 (2.59 × 10−2) | 3.38 × 10−1 (3.22 × 10−2) | |
| 10 | 5.07 × 10−1 (6.52 × 10−2)+(†) | 4.83 × 10−1 (6.29 × 10−3)+(†) | 4.51 × 10−1 (1.02 × 10−2)+(†) | 4.11 × 10−1 (2.88 × 10−3)-(↓) | 7.45 × 10−1 (2.54 × 10−2)+(†) | 4.38 × 10−1 (1.78 × 10−3)=(†) | 4.79 × 10−1 (5.64 × 10−3)+(†) | 1.56 × 100 (1.94 × 10−1)+(†) | 4.38 × 10−1 (1.37 × 10−2) | 4.12 × 10−1 (1.21 × 10−2) | |
| DTLZ5 | 3 | 1.58 × 10−2 (4.30 × 10−3)+(†) | 2.96 × 10−2 (3.53 × 10−3)+(†) | 4.45 × 10−3 (1.76 × 10−4)+(†) | 5.18 × 10−3 (7.31 × 10−4)+(†) | 7.71 × 10−3 (1.20 × 10−3)+(†) | 4.78 × 10−3 (2.44 × 10−4)+(†) | 1.04 × 10−2 (1.76 × 10−3)+(†) | 4.18 × 10−3 (1.41 × 10−4)=(†) | 4.18 × 10−3 (4.05 × 10−4) | 4.09 × 10−3 (3.50 × 10−4) |
| 5 | 6.27 × 10−2 (1.51 × 10−2)+(†) | 2.41 × 10−1 (6.50 × 10−2)+(†) | 1.55 × 10−1 (4.47 × 10−2)+(†) | 4.11 × 10−2 (7.24 × 10−3)+(†) | 1.22 × 10−2 (2.57 × 10−3)-(↓) | 4.08 × 10−2 (5.06 × 10−3)+(†) | 7.34 × 10−1 (5.05 × 10−2)+(†) | 1.22 × 10−1 (2.56 × 10−2)+(†) | 1.91 × 10−2 (4.04 × 10−3) | 1.65 × 10−2 (3.16 × 10−3) | |
| 8 | 9.24 × 10−2 (2.28 × 10−2)+(†) | 3.71 × 10−1 (8.17 × 10−2)+(†) | 3.45 × 10−1 (6.47 × 10−2)+(†) | 1.01 × 10−1 (1.83 × 10−2)+(†) | 4.17 × 10−2 (6.11 × 10−3)+(†) | 6.75 × 10−2 (1.25 × 10−2)+(†) | 1.35 × 100 (9.23 × 10−2)+(†) | 2.17 × 10−1 (3.27 × 10−2)+(†) | 2.27 × 10−2 (5.41 × 10−3) | 2.11 × 10−2 (5.47 × 10−3) | |
| 10 | 8.20 × 10−2 (2.15 × 10−2)+(†) | 4.72 × 10−1 (1.61 × 10−1)+(†) | 4.05 × 10−1 (8.55 × 10−2)+(†) | 1.17 × 10−1 (1.69 × 10−2)+(†) | 5.59 × 10−2 (8.06 × 10−3)+(†) | 6.50 × 10−2 (1.47 × 10−2)+(†) | 1.37 × 100 (1.43 × 10−1)+(†) | 1.77 × 10−1 (3.73 × 10−2)+(†) | 2.27 × 10−2 (6.19 × 10−3) | 1.95 × 10−2 (5.94 × 10−3) | |
| DTLZ6 | 3 | 3.71 × 10−2 (2.01 × 10−2)+(†) | 3.44 × 10−2 (4.42 × 10−3)+(†) | 4.31 × 10−3 (2.63 × 10−4)+(≡) | 5.59 × 10−3 (2.93 × 10−3)+(†) | 8.70 × 10−3 (1.30 × 10−3)+(†) | 4.91 × 10−3 (1.87 × 10−4)+(†) | 1.83 × 10−2 (2.13 × 10−2)+(†) | 4.43 × 100 (2.22 × 10−1)+(†) | 4.24 × 10−3 (4.25 × 10−4) | 4.31 × 10−3 (7.13 × 10−4) |
| 5 | 1.41 × 10−1 (6.70 × 10−2)+(†) | 7.20 × 10−1 (3.76 × 10−1)+(†) | 4.33 × 10−1 (2.03 × 10−1)+(†) | 6.46 × 10−2 (1.59 × 10−2)+(†) | 1.05 × 10−2 (1.52 × 10−3)-(↓) | 5.41 × 10−2 (1.40 × 10−2)+(†) | 7.02 × 10−1 (1.11 × 10−1)+(†) | 9.44 × 100 (6.58 × 10−1)+(†) | 4.34 × 10−2 (8.72 × 10−3) | 4.94 × 10−2 (3.11 × 10−2) | |
| 8 | 2.56 × 10−1 (2.57 × 10−1)+(↓) | 2.11 × 100 (7.06 × 10−1)+(†) | 3.11 × 100 (9.10 × 10−1)+(†) | 1.68 × 10−1 (3.13 × 10−2)-(↓) | 2.29 × 10−2 (5.43 × 10−3)-(↓) | 8.22 × 10−2 (2.23 × 10−2)-(↓) | 1.04 × 100 (1.15 × 10−1)+(†) | 9.90 × 100 (4.57 × 10−2)+(†) | 2.27 × 10−1 (3.55 × 10−1) | 3.26 × 10−1 (4. × 10 × 10−1) | |
| 10 | 2.32 × 10−1 (5.65 × 10−2)+(†) | 3.43 × 100 (7.72 × 10−1)+(†) | 3.07 × 100 (6.55 × 10−1)+(†) | 2.03 × 10−1 (5.65 × 10−2)+(†) | 4.47 × 10−2 (2.30 × 10−2)-(↓) | 9.96 × 10−2 (3.81 × 10−2)+(†) | 1.00 × 100 (1.02 × 10−1)+(†) | 9.82 × 100 (2.17 × 10−1)+(†) | 8.81 × 10−2 (1.36 × 10−1) | 8.98 × 10−2 (3.31 × 10−1) | |
| DTLZ7 | 3 | 9.03 × 10−2 (9.14 × 10−2)+(†) | 8.71 × 10−2 (2.94 × 10−3)+(†) | 8.54 × 10−2 (9.16 × 10−2)+(†) | 1.28 × 10−1 (1.39 × 10−1)+(†) | 1.71 × 10−1 (6.14 × 10−2)+(†) | 8.21 × 10−2 (9.40 × 10−2)+(†) | 3.88 × 10−1 (1.06 × 10−1)+(†) | 1.50 × 10−1 (2.04 × 10−1)+(†) | 3.61 × 10−2 (2.09 × 10−1) | 3.12 × 10−2 (2.42 × 10−1) |
| 5 | 3.09 × 10−1 (1.01 × 10−2)+(†) | 3.65 × 10−1 (7.01 × 10−3)+(†) | 3.20 × 10−1 (9.75 × 10−3)+(†) | 2.95 × 10−1 (2.96 × 10−2)+(†) | 5.03 × 10−1 (5.79 × 10−2)+(†) | 2.91 × 10−1 (1.01 × 10−2)+(≡) | 8.14 × 10−1 (1.11 × 10−1)+(†) | 5.18 × 10−1 (3.60 × 10−2)+(†) | 2.13 × 10−1 (2.39 × 10−1) | 2.91 × 10−1 (2.15 × 10−1) | |
| 8 | 6.99 × 10−1 (2.30 × 10−2)+(†) | 9.27 × 10−1 (3.42 × 10−2)+(†) | 6.74 × 10−1 (1.93 × 10−2)=(†) | 7.76 × 10−1 (2.32 × 10−2)+(†) | 1.16 × 100 (1.15 × 10−1)+(†) | 8.03 × 10−1 (4.76 × 10−2)+(†) | 3.30 × 100 (4.94 × 10−1)+(†) | 2.86 × 100 (8.59 × 10−1)+(†) | 6.74 × 10−1 (1.66 × 10−1) | 6.42 × 10−1 (1.20 × 10−1) | |
| 10 | 7.48 × 10−1 (3.52 × 10−2)=(≡) | 1.60 × 100 (4.42 × 10−2)+(†) | 9.40 × 10−1 (2.95 × 10−2)+(†) | 8.34 × 10−1 (1.01 × 10−2)+(†) | 1.45 × 100 (1.63 × 10−1)+(†) | 1.04 × 100 (5.04 × 10−2)+(†) | 4.55 × 100 (5.21 × 10−1)+(†) | 3.96 × 100 (1.34 × 100)+(†) | 7.48 × 10−1 (1.75 × 10−1) | 7.48 × 10−1 (6.33 × 10−2) | |
| Ad-GrMODE1* (+/=/-) | 23/1/4 | 23/2/3 | 23/1/4 | 23/1/4 | 23/0/5 | 20/1/7 | 24/0/4 | 26/1/1 | |||
| Ad-GrMODE2* (†/≡/↓) | 22/1/5 | 23/0/5 | 23/1/4 | 19/3/6 | 23/0/5 | 20/1/7 | 24/0/4 | 27/0/1 | |||
| # | M | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | adGrMOEA1* | adGrMOEA2* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| WFG1 | 3 | 1.58 × 100 (9.52 × 10−2)+(†) | 1.53 × 100 (7.83 × 10−4)+(†) | 1.53 × 100 (2.09 × 10−3)+(†) | 1.53 × 100 (3.97 × 10−3)+(†) | 2.38 × 100 (5.74 × 10−2)+(†) | 1.83 × 100 (2.05 × 10−2)+(†) | 1.89 × 100 (6.79 × 10−2)+(†) | 1.68 × 100 (5.81 × 10−2)+(†) | 1.46 × 100 (4.75 × 10−3) | 1.46 × 100 (6.31 × 10−3) |
| 5 | 2.14 × 100 (1.33 × 10−1)+(†) | 2.39 × 100 (3.06 × 10−1)+(†) | 2.21 × 100 (5.80 × 10−3)+(†) | 1.99 × 100 (6.43 × 10−3)-(↓) | 2.58 × 100 (3.63 × 10−2)+(†) | 2.10 × 100 (1.11 × 10−2)+(†) | 2.66 × 100 (4.07 × 10−1)+(†) | 2.08 × 100 (3.08 × 10−2)+(†) | 2.04 × 100 (1.61 × 10−2) | 2.05 × 100 (1.70 × 10−2) | |
| 8 | 2.72 × 100 (7.44 × 10−2)+(†) | 2.78 × 100 (1.91 × 10−1)+(†) | 2.66 × 100 (1.53 × 10−2)-(↓) | 2.63 × 100 (2.25 × 10−2)-(↓) | 2.96 × 100 (2.20 × 10−2)+(†) | 2.70 × 100 (8.69 × 10−3)=(≡) | 3.89 × 100 (6.35 × 10−1)+(†) | 2.65 × 100 (2.35 × 10−2)-(↓) | 2.70 × 100 (1.75 × 10−2) | 2.70 × 100 (1.94 × 10−2) | |
| 10 | 3.01 × 100 (5.28 × 10−2)-(↓) | 3.02 × 100 (3.70 × 10−2)-(↓) | 3.02 × 100 (1.90 × 10−2)-(↓) | 3.19 × 100 (2.06 × 10−2)+(†) | 3.19 × 100 (9.09 × 10−3)+(†) | 3.03 × 100 (9.74 × 10−3)-(↓) | 4.59 × 100 (8.47 × 10−1)+(†) | 2.99 × 100 (1.57 × 10−2)-(↓) | 3.05 × 100 (2.25 × 10−2) | 3.06 × 100 (2.51 × 10−2) | |
| WFG2 | 3 | 3.04 × 100 (3.90 × 10−3)-(↓) | 3.04 × 100 (2.37 × 10−3)-(↓) | 3.04 × 100 (2.27 × 10−3)-(↓) | 3.05 × 100 (2.96 × 10−3)=(≡) | 3.05 × 100 (5.13 × 10−3)=(≡) | 3.04 × 100 (4.62 × 10−3)-(↓) | 3.12 × 100 (2.94 × 10−2)+(†) | 3.04 × 100 (1.11 × 10−3)-(↓) | 3.05 × 100 (3.13 × 10−3) | 3.05 × 100 (4.04 × 10−3) |
| 5 | 5.69 × 100 (4.83 × 10−3)+(†) | 5.69 × 100 (2.95 × 10−3)+(†) | 5.69 × 100 (2.55 × 10−3)+(†) | 5.69 × 100 (1.20 × 10−3)+(†) | 5.71 × 100 (1.18 × 10−2)+(†) | 5.69 × 100 (3.04 × 10−3)+(†) | 7.12 × 100 (9.66 × 10−1)+(†) | 5.69 × 100 (1.26 × 10−3)+(†) | 4.66 × 100 (2.01 × 10−3) | 4.67 × 100 (1.56 × 10−3) | |
| 8 | 9.41 × 100 (1.26 × 10−2)=(≡) | 9.41 × 100 (9.11 × 10−4)=(≡) | 9.41 × 100 (1.44 × 10−3)=(≡) | 9.41 × 100 (1.11 × 10−3)=(≡) | 9.45 × 100 (2.70 × 10−2)+(†) | 9.41 × 100 (3.40 × 10−3)=(≡) | 1.29 × 10+1 (7.06 × 10−1)+(†) | 9.41 × 100 (1.42 × 10−3)=(≡) | 9.41 × 100 (1.51 × 10−3) | 9.41 × 100 (1.40 × 10−3) | |
| 10 | 1.20 × 10+1 (4.55 × 10−3)=(≡) | 1.20 × 10+1 (1.41 × 10−3)=(≡) | 1.20 × 10+1 (1.54 × 10−3)=(≡) | 1.20 × 10+1 (1.11 × 10−3)=(≡) | 1.20 × 10+1 (2.44 × 10−2)=(≡) | 1.20 × 10+1 (4.57 × 10−3)=(≡) | 1.60 × 10+1 (1.27 × 100)+(†) | 1.20 × 10+1 (1.39 × 10−3)=(≡) | 1.20 × 10+1 (1.03 × 10−3) | 1.20 × 10+1 (8.86 × 10−4) | |
| WFG3 | 3 | 1.39 × 100 (4.46 × 10−3)+(†) | 1.39 × 100 (1.02 × 10−3)+(†) | 1.38 × 100 (1.82 × 10−3)+(†) | 1.38 × 100 (7.19 × 10−4)+(†) | 1.39 × 100 (6.16 × 10−3)+(†) | 1.38 × 100 (5.49 × 10−4)+(†) | 2.74 × 100 (2.31 × 10−1)+(†) | 1.44 × 100 (2.08 × 10−3)+(†) | 1.23 × 100 (4.63 × 10−4) | 1.13 × 100 (2.39 × 10−4) |
| 5 | 2.33 × 100 (8.13 × 10−2)+(†) | 2.28 × 100 (5.09 × 10−3)+(†) | 2.25 × 100 (1.68 × 10−3)+(†) | 2.25 × 100 (1.52 × 10−3)+(†) | 2.27 × 100 (1.41 × 10−2)+(†) | 2.24 × 100 (1.77 × 10−3)+(†) | 5.84 × 100 (3.18 × 10−2)+(†) | 2.32 × 100 (3.04 × 10−3)+(†) | 1.61 × 100 (1.14 × 10−3) | 1.31 × 100 (2.06 × 10−3) | |
| 8 | 3.72 × 100 (4.15 × 10−2)=(†) | 6.28 × 100 (8.42 × 10−1)+(†) | 3.67 × 100 (3.84 × 10−3)-(↓) | 3.67 × 100 (5.33 × 10−3)-(↓) | 3.71 × 100 (1.95 × 10−2)-(≡) | 3.66 × 100 (3.58 × 10−3)-(↓) | 1.04 × 10+1 (9.96 × 10−2)+(†) | 3.74 × 100 (4.92 × 10−3)+(†) | 3.72 × 100 (5.00 × 10−3) | 3.71 × 100 (5.44 × 10−3) | |
| 10 | 3.47 × 100 (1.70 × 10−2)+(†) | 4.88 × 100 (8.23 × 10−1)+(†) | 3.41 × 100 (2.19 × 10−3)+(†) | 3.41 × 100 (4.35 × 10−3)+(†) | 3.44 × 100 (2.11 × 10−2)+(†) | 3.40 × 100 (2.74 × 10−3)+(†) | 9.94 × 100 (1.05 × 10−1)+(†) | 3.53 × 100 (2.55 × 10−3)+(†) | 3.21 × 100 (1.74 × 10−3) | 3.12 × 100 (2.33 × 10−3) | |
| WFG4 | 3 | 7.95 × 100 (2.20 × 10−3)+(†) | 7.93 × 10−1 (1.22 × 10−3)+(†) | 8.06 × 10−1 (4.13 × 10−3)+(†) | 8.47 × 10−1 (9.26 × 10−3)+(†) | 1.06 × 100 (6.75 × 10−2)+(†) | 8.24 × 10−1 (7.07 × 10−3)+(†) | 8.20 × 10−1 (6.89 × 10−3)+(†) | 8.61 × 10−1 (2.14 × 10−2)+(†) | 6.48 × 10−1 (1.01 × 10−2) | 6.48 × 10−1 (7.88 × 10−3) |
| 5 | 1.62 × 100 (8.33 × 10−3)+(†) | 1.65 × 100 (3.48 × 10−3)+(†) | 1.64 × 100 (9.27 × 10−3)+(†) | 1.78 × 100 (2.31 × 10−2)+(†) | 2.95 × 100 (2.61 × 10−1)+(†) | 2.10 × 100 (1.13 × 10−1)+(†) | 1.71 × 100 (2.18 × 10−2)+(†) | 1.75 × 100 (3.61 × 10−2)+(†) | 1.42 × 100 (3.74 × 10−2) | 1.49 × 100 (4.77 × 10−2) | |
| 8 | 3.71 × 100 (1.55 × 10−1)-(↓) | 3.57 × 100 (2.96 × 10−2)-(↓) | 3.36 × 100 (1.85 × 10−2)-(↓) | 3.94 × 100 (1.68 × 10−1)-(↓) | 6.73 × 100 (4.36 × 10−1)+(†) | 4.93 × 100 (1.84 × 10−1)+(†) | 3.73 × 100 (8.90 × 10−2)-(↓) | 3.76 × 100 (5.93 × 10−2)-(↓) | 4.23 × 100 (2.10 × 10−1) | 4.22 × 100 (2.00 × 10−1) | |
| 10 | 4.82 × 100 (1.59 × 10−1)-(↓) | 4.87 × 100 (1.72 × 10−2)-(↓) | 4.32 × 100 (2.24 × 10−2)-(↓) | 5.67 × 100 (3.55 × 10−1)-(↓) | 8.68 × 100 (5.27 × 10−1)+(†) | 6.54 × 100 (2.26 × 10−1)+(†) | 4.93 × 100 (9.02 × 10−2)-(↓) | 4.98 × 100 (9.08 × 10−2)-(↓) | 5.86 × 100 (3.09 × 10−1) | 5.77 × 100 (4.54 × 10−1) | |
| WFG5 | 3 | 3.39 × 10−1 (7.17 × 10−3)+(↓) | 3.49 × 10−1 (1.01 × 10−3)+(↓) | 3.17 × 10−1 (3.06 × 10−3)-(↓) | 3.72 × 10−1 (1.17 × 10−2)+(†) | 3.83 × 10−1 (9.54 × 10−3)+(†) | 3.23 × 10−1 (3.63 × 10−3)+(↓) | 3.68 × 10−1 (1.02 × 10−2)+(≡) | 3.29 × 10−1 (2.52 × 10−3)+(↓) | 3.20 × 10−1 (1.33 × 10−2) | 3.68 × 10−1 (1.09 × 10−2) |
| 5 | 2.31 × 100 (2.43 × 10−2)-(≡) | 2.50 × 100 (2.41 × 10−2) +(†) | 2.23 × 100 (7.90 × 10−3)-(↓) | 2.29 × 100 (1.71 × 10−2)-(↓) | 2.51 × 100 (5.59 × 10−2)+(†) | 2.20 × 100 (9.98 × 10−3)-(↓) | 2.34 × 100 (4.35 × 10−2)=(†) | 2.25 × 100 (1.06 × 10−2)-(↓) | 2.34 × 100 (2.07 × 10−2) | 2.31 × 100 (1.89 × 10−2) | |
| 8 | 6.76 × 100 (2.51 × 10−2)+(†) | 6.83 × 100 (2.64 × 10−2)+(†) | 6.68 × 100 (1.16 × 10−2)+(†) | 6.80 × 100 (2.60 × 10−2)+(†) | 7.23E × 100 (8.51 × 10−2)+(†) | 6.64 × 100 (8.54 × 10−3)+(†) | 7.21 × 100 (1.27 × 10−1)+(†) | 6.74 × 100 (1.84 × 10−2)+(†) | 6.02 × 100 (8.29 × 10−2) | 6.00 × 100 (6.95 × 10−2) | |
| 10 | 9.82 × 100 (2.31 × 10−2)-(↓) | 9.92 × 100 (2.27 × 10−2)-(↓) | 9.76 × 100 (1.29 × 10−2)-(↓) | 9.94 × 100 (3.74 × 10−2)-(↓) | 1.04 × 10+1 (7.00 × 10−2)+(†) | 9.72 × 100 (1.07 × 10−2)-(↓) | 1.07 × 10+1 (1.50 × 100)+(†) | 9.85 × 100 (1.25 × 10−2)-(↓) | 1.01 × 10+1 (9.71 × 10−2) | 1.01 × 10+1 (8.99 × 10−2) | |
| WFG6 | 3 | 2.20 × 100 (3.96 × 10−2)+(†) | 2.18 × 100 (3.01 × 10−3)+(†) | 2.17 × 100 (4.27 × 10−3)+(†) | 2.17 × 100 (3.79 × 10−3)+(†) | 2.22 × 100 (1.57 × 10−2)+(†) | 2.17 × 100 (6.04 × 10−3)+(†) | 2.25 × 100 (3.90 × 10−2)+(†) | 2.18 × 100 (3.00 × 10−3)+(†) | 2.13 × 100 (2.53 × 10−3) | 2.11 × 100 (2.46 × 10−3) |
| 5 | 5.78 × 100 (6.65 × 10−3)=(↓) | 5.78 × 100 (7.77 × 10−3)=(↓) | 5.78 × 100 (5.21 × 10−3)=(↓) | 5.81 × 100 (8.17 × 10−3)+(↓) | 5.87 × 100 (3.39 × 10−2)+(†) | 5.78 × 100 (1.16 × 10−2)=(↓) | 6.08 × 100 (7.74 × 10−2)+(†) | 5.78 × 100 (3.60 × 10−3)=(↓) | 5.78 × 100 (6.74 × 10−3) | 5.82 × 100 (9.97 × 10−3) | |
| 8 | 1.17 × 10+1 (1.30 × 10−2)+(†) | 1.17 × 10+1 (2.65 × 10−2)+(†) | 1.17 × 10+1 (3.99 × 10−3)+(†) | 1.17 × 10+1 (1.92 × 10−2)+(†) | 1.18 × 10+1 (3.86 × 10−2)+(†) | 1.17 × 10+1 (1.62 × 10−2)+(†) | 1.23 × 10+1 (7.11 × 10−2)+(†) | 1.17 × 10+1 (4.49 × 10−3)+(†) | 1.07 × 10+1 (1.33 × 10−2) | 1.07 × 10+1 (1.08 × 10−2) | |
| 10 | 1.56 × 10+1 (4.64 × 10−2)=(≡) | 1.56 × 10+1 (3.33 × 10−2)=(≡) | 1.56 × 10+1 (2.20 × 10−3)=(≡) | 1.56 × 10+1 (3.95 × 10−2)=(≡) | 1.56 × 10+1 (3.34 × 10−2)=(≡) | 1.55 × 10+1 (1.55 × 10−2)-(↓) | 1.63 × 10+1 (5.04 × 10−2)+(†) | 1.54 × 10+1 (3.90 × 10−3)-(↓) | 1.56 × 10+1 (1.03 × 10−2) | 1.56 × 10+1 (1.00 × 10−2) | |
| WFG7 | 3 | 1.34 × 100 (2.46 × 10−1)+(†) | 1.30 × 100 (7.80 × 10−3)+(†) | 1.29 × 100 (4.36 × 10−3)+(†) | 1.30 × 100 (7.11 × 10−3)+(†) | 1.68 × 100 (6.05 × 10−2)+(†) | 1.32 × 100 (1.00 × 10−2)+(†) | 1.34 × 100 (1.44 × 10−2)+(†) | 1.33 × 100 (9.56 × 10−3)+(†) | 1.21 × 100 (6.55 × 10−3) | 1.11 × 100 (8.02 × 10−3) |
| 5 | 2.78 × 100 (7.06 × 10−1)+(†) | 2.21 × 100 (6.47 × 10−3)+(†) | 2.30 × 100 (1.04 × 10−2)+(†) | 2.39 × 100 (2.74 × 10−2)+(†) | 3.16 × 100 (6.71 × 10−2)+(†) | 2.37 × 100 (3.92 × 10−2)+(†) | 2.47 × 100 (4.19 × 10−2)+(†) | 2.42 × 100 (2.69 × 10−2)+(†) | 2.11 × 100 (3.14 × 10−2) | 2.15 × 100 (3.29 × 10−2) | |
| 8 | 4.59 × 100 (7.77 × 10−1)+(†) | 4.29 × 100 (8.99 × 10−2)+(†) | 4.51 × 100 (3.46 × 10−2)+(†) | 4.56 × 100 (5.53 × 10−2)+(†) | 5.76 × 100 (8.00 × 10−2)+(†) | 4.45 × 100 (5.71 × 10−2)+(†) | 4.68 × 100 (6.66 × 10−2)+(†) | 4.58 × 100 (3.98 × 10−2)+(†) | 3.63 × 100 (6.23 × 10−2) | 3.64 × 100 (4.62 × 10−2) | |
| 10 | 5.33 × 100 (5.34 × 10−2)+(†) | 5.51 × 100 (3.60 × 10−2)+(†) | 5.65 × 100 (3.19 × 10−2)+(†) | 5.61 × 100 (5.15 × 10−2)+(†) | 6.99 × 100 (6.45 × 10−2)+(†) | 5.47 × 100 (4.98 × 10−2)+(†) | 5.83 × 100 (4.02 × 10−2)+(†) | 5.80 × 100 (3.95 × 10−2)+(†) | 4.76 × 100 (8.51 × 10−2) | 4.77 × 100 (7.68 × 10−2) | |
| WFG8 | 3 | 2.17 × 100 (2.83 × 10−2)+(†) | 2.09 × 100 (2.54 × 10−3)+(†) | 2.11 × 100 (2.34 × 10−2)+(†) | 2.15 × 100 (2.72 × 10−2)+(†) | 2.14 × 100 (1.71 × 10−2)+(†) | 2.08 × 100 (3.12 × 10−3)+(†) | 2.17 × 100 (3.74 × 10−2)+(†) | 2.10 × 100 (2.43 × 10−3)+(†) | 2.03 × 100 (3.20 × 10−2) | 2.04 × 100 (2.25 × 10−2) |
| 5 | 5.77 × 100 (1.57 × 10−2)+(†) | 5.71 × 100 (3.38 × 10−3)+(†) | 5.72 × 100 (6.52 × 10−3)+(†) | 5.74 × 100 (1.55 × 10−2)+(†) | 5.80 × 100 (2.80 × 10−2)+(†) | 5.70 × 100 (1.07 × 10−2)+(†) | 6.34 × 100 (1.36 × 10−1)+(†) | 5.70 × 100 (4.83 × 10−3)+(†) | 5.59 × 100 (9.66 × 10−3) | 5.52 × 100 (1.12 × 10−2) | |
| 8 | 1.17 × 10+1 (2.68 × 10−2)+(†) | 1.17 × 10+1 (2.64 × 10−2)+(†) | 1.16 × 10+1 (1.04 × 10−2)+(†) | 1.17 × 10+1 (1.58 × 10−2)+(†) | 1.17 × 10+1 (5.16 × 10−2)+(†) | 1.16 × 10+1 (1.29 × 10−2)+(†) | 1.28 × 10+1 (2.00 × 10−1)+(†) | 1.16 × 10+1 (4.33 × 10−3)+(†) | 1.13 × 10+1 (1.23 × 10−2) | 1.12 × 10+1 (1.85 × 10−2) | |
| 10 | 1.55 × 10+1 (2.09 × 10−2)=(≡) | 1.55 × 10+1 (2.22 × 10−2)=(≡) | 1.55 × 10+1 (7.43 × 10−3)=(≡) | 1.55 × 10+1 (1.17 × 10−2)=(≡) | 1.55 × 10+1 (4.88 × 10−2)=(≡) | 1.55 × 10+1 (1.71 × 10−2)=(≡) | 1.67 × 10+1 (2.53 × 10−1)+(†) | 1.55 × 10+1 (4.99 × 10−3)=(≡) | 1.55 × 10+1 (2.09 × 10−2) | 1.55 × 10+1 (3.08 × 10−2) | |
| WFG9 | 3 | 4.00 × 10−1 (9.52 × 10−3)-(↓) | 4.14 × 10−1 (7.36 × 10−3)+(†) | 4.15 × 10−1 (1.17 × 10−2)+(†) | 4.53 × 10−1 (1.60 × 10−2)+(†) | 1.27 × 100 (1.57 × 10−1)+(†) | 4.07 × 10−1 (6.94 × 10−3)+(†) | 4.35 × 10−1 (8.79 × 10−3)+(†) | 4.80 × 10−1 (8.67 × 10−3)+(†) | 4.05 × 10−1 (1.35 × 10−2) | 4.01 × 10−1 (1.35 × 10−2) |
| 5 | 1.13 × 100 (4.00 × 10−2)+(↓) | 1.11 × 100 (8.65 × 10−3)=(↓) | 1.18 × 100 (1.16 × 10−2)+(†) | 1.28 × 100 (1.89 × 10−2)+(†) | 4.57 × 100 (4.04 × 10−1)+(†) | 1.27 × 100 (3.00 × 10−2)+(†) | 1.35 × 100 (4.03 × 10−2)+(†) | 1.51 × 100 (1.62 × 10−1)+(†) | 1.11 × 100 (2.87 × 10−2) | 1.15 × 100 (6.58 × 10−2) | |
| 8 | 3.45 × 100 (1.69 × 10−1)+(†) | 3.22 × 100 (1.16 × 10−1)+(†) | 3.09 × 100 (3.57 × 10−2)+(†) | 3.29 × 100 (1.38 × 10−1)+(†) | 1.01 × 10+1 (4.22 × 10−1)+(†) | 3.80 × 100 (1.94 × 10−1)+(†) | 6.64 × 100 (1.91 × 100)+(†) | 5.59 × 100 (3.66 × 10−1)+(†) | 2.46 × 100 (3.40 × 10−1) | 2.47 × 100 (3.96 × 10−1) | |
| 10 | 4.65 × 100 (2.94 × 10−1)+(†) | 4.66 × 100 (9.98 × 10−2)+(†) | 4.25 × 100 (8.17 × 10−2)-(↓) | 4.54 × 100 (2.39 × 10−1)+(†) | 1.34 × 10+1 (4.62 × 10−1)+(†) | 5.70 × 100 (2.17 × 10−1)+(†) | 8.90 × 100 (1.65 × 100)+(†) | 8.16 × 100 (4.16 × 10−1)+(†) | 4.51 × 100 (4.74 × 10−1) | 4.52 × 100 (3.85 × 10−1) | |
| Ad-GrMODE1* (+/=/-) | 23/6/7 | 25/5/6 | 21/5/10 | 24/5/7 | 31/4/1 | 25/5/6 | 33/1/2 | 24/4/8 | |||
| Ad-GrMODE2* (†/≡/↓) | 22/5/9 | 24/8/4 | 21/4/11 | 23/5/8 | 31/5/0 | 24/4/8 | 33/1/2 | 23/3/10 | |||
References
- Zhou, A.; Qu, B.-Y.; Li, H.; Zhao, S.-Z.; Suganthan, P.N.; Zhang, Q. Multiobjective evolutionary algorithms: A survey of the state of the art. Swarm Evol. Comput. 2011, 1, 32–49. [Google Scholar] [CrossRef]
- Li, B.; Li, J.; Tang, K.; Yao, X. Many-objective evolutionary algorithms: A survey. ACM Comput. Surv. CSUR 2015, 48, 1–35. [Google Scholar] [CrossRef] [Green Version]
- Palakonda, V.; Ghorbanpour, S.; Mallipeddi, R. Pareto dominance-based MOEA with multiple ranking methods for many-objective optimization. In Proceedings of the 2018 IEEE Symposium Series on Computational Intelligence (SSCI), Bangalore, India, 18–21 November 2018. [Google Scholar]
- Chen, J.; Ding, J.; Tan, K.C.; Chen, Q. A decomposition-based evolutionary algorithm for scalable multi/many-objective optimization. Memetic Comput. 2021, 13, 413–432. [Google Scholar] [CrossRef]
- Li, L.; Wang, X. An adaptive multiobjective evolutionary algorithm based on grid subspaces. Memetic Comput. 2021, 13, 249–269. [Google Scholar] [CrossRef]
- Rashno, A.; Shafipour, M.; Fadaei, S. Particle ranking: An Efficient Method for Multi-Objective Particle Swarm Optimization Feature Selection. Knowl.-Based Syst. 2022, 245, 108640. [Google Scholar] [CrossRef]
- Pan, J.-S.; Liu, N.; Chu, S.-C. A competitive mechanism based multi-objective differential evolution algorithm and its application in feature selection. Knowl.-Based Syst. 2022, 245, 108582. [Google Scholar] [CrossRef]
- Ghorbanpour, S.; Pamulapati, T.; Mallipeddi, R.; Lee, M. Energy disaggregation considering least square error and temporal sparsity: A multi-objective evolutionary approach. Swarm Evol. Comput. 2021, 64, 100909. [Google Scholar] [CrossRef]
- Brest, J.; Greiner, S.; Boskovic, B.; Mernik, M.; Zumer, V. Self-adapting control parameters in differential evolution: A comparative study on numerical benchmark problems. IEEE Trans. Evol. Comput. 2006, 10, 646–657. [Google Scholar] [CrossRef]
- Cheng, J.; Zhang, G. Multi-objective differential evolution: A recent survey. Soft. Comput. Appl. 2013, 1, 1–9. [Google Scholar]
- Abbass, H.A.; Sarker, R.; Newton, C. PDE: A Pareto-frontier differential evolution approach for multi-objective optimization problems. In Proceedings of the 2001 congress on evolutionary computation (IEEE Cat. No. 01TH8546), Seoul, Korea, 27–30 May 2001. [Google Scholar]
- Neri, F.; Tirronen, V. Recent advances in differential evolution: A survey and experimental analysis. Artif. Intell. Rev. 2010, 33, 61–106. [Google Scholar] [CrossRef]
- Ghosh, S.; Das, S.; Vasilakos, A.V.; Suresh, K. On convergence of differential evolution over a class of continuous functions with unique global optimum. IEEE Trans. Syst. Man Cybern. Part B 2011, 42, 107–124. [Google Scholar] [CrossRef]
- Tang, L.; Dong, Y.; Liu, J. Differential evolution with an individual-dependent mechanism. IEEE Trans. Evol. Comput. 2014, 19, 560–574. [Google Scholar] [CrossRef] [Green Version]
- Das, S.; Abraham, A.; Chakraborty, U.K.; Konar, A. Differential evolution using a neighborhood-based mutation operator. IEEE Trans. Evol. Comput. 2009, 13, 526–553. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Sanderson, A.C. JADE: Adaptive differential evolution with optional external archive. IEEE Trans. Evol. Comput. 2009, 13, 945–958. [Google Scholar] [CrossRef]
- Islam, S.M.; Das, S.; Ghosh, S.; Roy, S.; Suganthan, P.N. An adaptive differential evolution algorithm with novel mutation and crossover strategies for global numerical optimization. IEEE Trans. Syst. Man Cybern. Part B 2011, 42, 482–500. [Google Scholar] [CrossRef]
- Wang, Y.; Cai, Z.; Zhang, Q. Enhancing the search ability of differential evolution through orthogonal crossover. Inf. Sci. 2012, 185, 153–177. [Google Scholar] [CrossRef]
- Wu, G.; Mallipeddi, R.; Suganthan, P.; Wang, R.; Chen, H. Differential evolution with multi-population based ensemble of mutation strategies. Inf. Sci. 2016, 329, 329–345. [Google Scholar] [CrossRef]
- Huang, V.L.; Qin, A.K.; Suganthan, P.N.; Tasgetiren, M.F. Multi-objective optimization based on self-adaptive differential evolution algorithm. In Proceedings of the 2007 IEEE Congress on Evolutionary Computation, Singapore, 25–28 September 2007. [Google Scholar]
- Huang, V.L.; Zhao, S.Z.; Mallipeddi, R.; Suganthan, P.N. Multi-objective optimization using self-adaptive differential evolution algorithm. In Proceedings of the 2009 IEEE Congress on Evolutionary Computation, Trondheim, Norway, 18–21 May 2009. [Google Scholar]
- Wang, X.; Dong, Z.; Tang, L. Multiobjective differential evolution with personal archive and biased self-adaptive mutation selection. IEEE Trans. Syst. Man Cybern. Syst. 2018, 50, 5338–5350. [Google Scholar] [CrossRef]
- Tang, L.; Wang, X.; Dong, Z. Adaptive multiobjective differential evolution with reference axis vicinity mechanism. IEEE Trans. Cybern. 2018, 49, 3571–3585. [Google Scholar] [CrossRef]
- Cheng, J.; Yen, G.G.; Zhang, G. A grid-based adaptive multi-objective differential evolution algorithm. Inf. Sci. 2016, 367, 890–908. [Google Scholar] [CrossRef]
- Yang, S.; Li, M.; Liu, X.; Zheng, J. A grid-based evolutionary algorithm for many-objective optimization. IEEE Trans. Evol. Comput. 2013, 17, 721–736. [Google Scholar] [CrossRef]
- Corne, D.W.; Jerram, N.R.; Knowles, J.D.; Oates, M.J. PESA-II: Region-based selection in evolutionary multiobjective optimization. In Proceedings of the 3rd Annual Conference on Genetic and Evolutionary Computation, San Francisco, CA, USA, 7–11 July 2001. [Google Scholar]
- Cai, X.; Xiao, Y.; Li, M.; Hu, H.; Ishibuchi, H.; Li, X. A grid-based inverted generational distance for multi/many-objective optimization. IEEE Trans. Evol. Comput. 2020, 25, 21–34. [Google Scholar] [CrossRef]
- Kukkonen, S.; Lampinen, J. GDE3: The third evolution step of generalized differential evolution. In Proceedings of the 2005 IEEE Congress on Evolutionary Computation, Edinburgh, UK, 2–5 September 2005. [Google Scholar]
- Abbass, H.A. The self-adaptive pareto differential evolution algorithm. In Proceedings of the 2002 Congress on Evolutionary Computation, CEC’02 (Cat. No. 02TH8600), Honolulu, HI, USA, 12–17 May 2002. [Google Scholar]
- Madavan, N.K. Multiobjective optimization using a Pareto differential evolution approach. In Proceedings of the 2002 Congress on Evolutionary Computation, CEC’02 (Cat. No. 02TH8600), Honolulu, HI, USA, 12–17 May 2002. [Google Scholar]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A fast and elitist multiobjective genetic algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef] [Green Version]
- Iorio, A.W.; Li, X. Solving rotated multi-objective optimization problems using differential evolution. In Australasian Joint Conference on Artificial Intelligence; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Parsopoulos, K.E.; Tasoulis, D.K.; Pavlidis, N.G.; Plagianakos, V.P.; Vrahatis, M.N. Vector evaluated differential evolution for multiobjective optimization. In Proceedings of the 2004 Congress on Evolutionary Computation (IEEE Cat. No. 04TH8753), Portland, OR, USA, 19–23 June 2004. [Google Scholar]
- Xue, F.; Sanderson, A.C.; Graves, R.J. Pareto-based multi-objective differential evolution. In Proceedings of the 2003 Congress on Evolutionary Computation, 2003. CEC’03, Canberra, ACT, Australia, 8–12 December 2003. [Google Scholar]
- Babu, B.; Jehan, M.M.L. Differential evolution for multi-objective optimization. In Proceedings of the 2003 Congress on Evolutionary Computation, CEC’03, Canberra, ACT, Australia, 8–12 December 2003. [Google Scholar]
- Tušar, T.; Filipič, B. DEMO (Differential Evolution for Multiobjective Optimization); Institut “Jožef Stefan”: Ljubljana, Slovenia, 2009. [Google Scholar]
- Yu, W.-J.; Zhang, J. Multi-population differential evolution with adaptive parameter control for global optimization. In Proceedings of the 13th Annual Conference on Genetic and Evolutionary Computation, Dublin, Ireland, 12–16 July 2011. [Google Scholar]
- Wang, X.; Tang, L. Multi-objective optimization using a hybrid differential evolution algorithm. In Proceedings of the 2012 IEEE Congress on Evolutionary Computation, Brisbane, QLD, Australia, 10–15 June 2012. [Google Scholar]
- Santana-Quintero, L.V.; Coello, C.A.C. An algorithm based on differential evolution for multi-objective problems. Int. J. Comput. Intell. Res. 2005, 1, 151–169. [Google Scholar] [CrossRef]
- Deng, W.; Xu, J.; Song, Y.; Zhao, H. Differential evolution algorithm with wavelet basis function and optimal mutation strategy for complex optimization problem. Appl. Soft Comput. 2021, 100, 106724. [Google Scholar] [CrossRef]
- Zhang, J.; Sanderson, A.C. Self-adaptive multi-objective differential evolution with direction information provided by archived inferior solutions. In Proceedings of the 2008 IEEE Congress on Evolutionary Computation (IEEE World Congress on Computational Intelligence), Hong Kong, China, 1–6 June 2008. [Google Scholar]
- Wang, Y.-N.; Wu, L.-H.; Yuan, X.-F. Multi-objective self-adaptive differential evolution with elitist archive and crowding entropy-based diversity measure. Soft Comput. 2010, 14, 193–209. [Google Scholar] [CrossRef]
- Peng, H.; Han, Y.; Deng, C.; Wang, J.; Wu, Z. Multi-strategy co-evolutionary differential evolution for mixed-variable optimization. Knowl.-Based Syst. 2021, 229, 107366. [Google Scholar] [CrossRef]
- Liang, J.J.; Zheng, B.; Xu, F.Y.; Qu, B.Y.; Song, H. Multi-objective differential evolution algorithm based on fast sorting and a novel constraints handling technique. In Proceedings of the 2014 IEEE Congress on Evolutionary Computation (CEC), Beijing, China, 6–11 July 2014. [Google Scholar]
- Jin, Q.B.; Li, Y.T.; Cai, W. A novel grid-based bidirectional local search algorithm for many-objective optimization. In Proceedings of the 2018 IEEE 3rd Advanced Information Technology, Electronic and Automation Control Conference (IAEAC), Chongqing, China, 12–14 October 2018. [Google Scholar]
- Wang, L.; Cui, G.; Zhou, Q.; Li, K. A multi-clustering method based on evolutionary multiobjective optimization with grid decomposition. Swarm Evol. Comput. 2020, 55, 100691. [Google Scholar] [CrossRef]
- Cai, X.; Sun, H.; Zhang, Q.; Huang, Y. A grid weighted sum Pareto local search for combinatorial multi and many-objective optimization. IEEE Trans. Cybern. 2018, 49, 3586–3598. [Google Scholar] [CrossRef]
- Li, G.; Wang, W.; Zhang, W.; Wang, Z.; Tu, H.; You, W. Grid search based multi-population particle swarm optimization algorithm for multimodal multi-objective optimization. Swarm Evol. Comput. 2021, 62, 100843. [Google Scholar] [CrossRef]
- Wang, W.L.; Li, W.K.; Wang, Z.; Li, L. Opposition-based multi-objective whale optimization algorithm with global grid ranking. Neurocomputing 2019, 341, 41–59. [Google Scholar] [CrossRef]
- Duan, M.; Huang, Q.; Xu, R.; Wang, C.; Xu, J. Optimization of Shearer Drum Based on Multi-Objective Bat Algorithm with Grid (MOBA/G). Machines 2022, 10, 733. [Google Scholar] [CrossRef]
- Zhang, H.; Lei, X.; Wang, C.; Yue, N.; Xie, X. Adaptive grid based multi-objective Cauchy differential evolution for stochastic dynamic economic emission dispatch with wind power uncertainty. PLoS ONE 2017, 12, e0185454. [Google Scholar] [CrossRef] [Green Version]
- Zitzler, E.; Künzli, S. Indicator-based selection in multiobjective search. In International Conference on Parallel Problem Solving from Nature; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Li, M.; Yang, S.; Liu, X. Shift-based density estimation for Pareto-based algorithms in many-objective optimization. IEEE Trans. Evol. Comput. 2013, 18, 348–365. [Google Scholar] [CrossRef] [Green Version]
- Leon, M.; Xiong, N. Differential evolution enhanced with eager random search for solving real-parameter optimization problems. Int. J. Adv. Res. Artif. Intell. 2015 IJARAI-15 2015, 4, 49–57. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.; Qing, A.; Feng, Q. A comparative study of crossover in differential evolution. J. Heuristics 2011, 17, 675–703. [Google Scholar] [CrossRef]
- Deb, K.; Goyal, M. A combined genetic adaptive search (GeneAS) for engineering design. Comput. Sci. Inform. 1996, 26, 30–45. [Google Scholar]
- Li, B.; Tang, K.; Li, J.; Yao, X. Stochastic ranking algorithm for many-objective optimization based on multiple indicators. IEEE Trans. Evol. Comput. 2016, 20, 924–938. [Google Scholar] [CrossRef]
- Deb, K.; Jain, H. An evolutionary many-objective optimization algorithm using reference-point-based nondominated sorting approach, part I: Solving problems with box constraints. IEEE Trans. Evol. Comput. 2013, 18, 577–601. [Google Scholar] [CrossRef]
- Jiang, S.; Yang, S. A strength Pareto evolutionary algorithm based on reference direction for multiobjective and many-objective optimization. IEEE Trans. Evol. Comput. 2017, 21, 329–346. [Google Scholar] [CrossRef] [Green Version]
- Xiang, Y.; Zhou, Y.; Li, M.; Chen, Z. A vector angle-based evolutionary algorithm for unconstrained many-objective optimization. IEEE Trans. Evol. Comput. 2016, 21, 131–152. [Google Scholar] [CrossRef]
- Denysiuk, R.; Costa, L.; Santo, I.E. Clustering-based selection for evolutionary many-objective optimization. In International Conference on Parallel Problem Solving from Nature; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Denysiuk, R.; Costa, L.; Santo, I.E. Many-objective optimization using differential evolution with variable-wise mutation restriction. In Proceedings of the 15th Annual Conference on Genetic and Evolutionary Computation, New York, NY, USA, 6–10 June 2013. [Google Scholar]
- Deb, K.; Thiele, L.; Laumanns, M.; Zitzler, E. Scalable test problems for evolutionary multiobjective optimization. In Evolutionary Multiobjective Optimization; Springer: Berlin/Heidelberg, Germany, 2005; pp. 105–145. [Google Scholar]
- Huband, S.; Hingston, P.; Barone, L.; While, L. A review of multiobjective test problems and a scalable test problem toolkit. IEEE Trans. Evol. Comput. 2006, 10, 477–506. [Google Scholar] [CrossRef]
- Deb, K.; Agrawal, R.B. Simulated binary crossover for continuous search space. Complex. Syst. 1995, 9, 115–148. [Google Scholar]
- While, L.; Hingston, P.; Barone, L.; Huband, S. A faster algorithm for calculating hypervolume. IEEE Trans. Evol. Comput. 2006, 10, 29–38. [Google Scholar] [CrossRef] [Green Version]
- Zitzler, E.; Thiele, L.; Laumanns, M.; Fonseca, C.; da Fonseca, V. Performance assessment of multiobjective optimizers: An analysis and review. IEEE Trans. Evol. Comput. 2003, 7, 117–132. [Google Scholar] [CrossRef] [Green Version]
- Friedman, M. A comparison of alternative tests of significance for the problem of m rankings. Ann. Math. Stat. 1940, 11, 86–92. [Google Scholar] [CrossRef]
- Tanabe, R.; Ishibuchi, H. An easy-to-use real-world multi-objective optimization problem suite. Appl. Soft Comput. 2020, 89, 106078. [Google Scholar] [CrossRef]



| The Parameters and Settings of the DTLZ | |||||
| Problem | M | Parameter | Number of Variables (D) | Generations | |
| K | |||||
| DTLZ1 | 3, 5, 8, 10 | 5 | M + k − 1 | 500 | |
| DTLZ2 | 3, 5, 8, 10 | 10 | 250 | ||
| DTLZ3 | 3, 5, 8, 10 | 10 | 600 | ||
| DTLZ4 | 3, 5, 8, 10 | 10 | 250 | ||
| DTLZ5 | 3, 5, 8, 10 | 10 | 250 | ||
| DTLZ6 | 3, 5, 8, 10 | 10 | 250 | ||
| DTLZ7 | 3, 5, 8, 10 | 20 | 250 | ||
| The Parameters and Settings of the WFG | |||||
| Problem | M | Parameter | Number of Variables (D) | Generations | |
| K | L | ||||
| WFG1 | 3, 5, 8, 10 | M − 1 | 10 | l + k | 500 |
| WFG2 | 3, 5, 8, 10 | 10 | 500 | ||
| WFG3 | 3, 5, 8, 10 | 10 | 600 | ||
| WFG4 | 3, 5, 8, 10 | 10 | 250 | ||
| WFG5 | 3, 5, 8, 10 | 10 | 250 | ||
| WFG6 | 3, 5, 8, 10 | 10 | 250 | ||
| WFG7 | 3, 5, 8, 10 | 10 | 250 | ||
| WFG8 | 3, 5, 8, 10 | 10 | 250 | ||
| WFG9 | 3, 5, 8, 10 | 10 | 250 | ||
| Algorithm | Div | Algorithm | Div |
|---|---|---|---|
| ad-GrMODE1 | Constantly maintains div as “3” | ad-GrMODE1* | Linearly increasing div from 2 to 3 |
| ad-GrMODE2 | Constantly maintains div as “5” | ad-GrMODE2* | Linearly increasing div from 2 to 5 |
| ad-GrMODE3 | Constantly maintains div as “10” | ad-GrMODE3* | Linearly increasing div from 2 to 10 |
| ad-GrMODE4 | Constantly maintains div as “15” | ad-GrMODE4* | Linearly increasing div from 2 to 15 |
| ad-GrMODE5 | Constantly maintains div as “20” | ad-GrMODE5* | Linearly increasing div from 2 to 20 |
| Algorithm | Friedman Test | |
|---|---|---|
| DTLZ | WFG | |
| ad-GrMODE1 | 4.73 | 4.38 |
| ad-GrMODE2 | 6.44 | 6.62 |
| ad-GrMODE3 | 8.08 | 7.72 |
| ad-GrMODE4 | 8.85 | 8.58 |
| ad-GrMODE5 | 9.21 | 9.11 |
| ad-GrMODE1* | 2.07 | 2.58 |
| ad-GrMODE2* | 2.64 | 3.12 |
| ad-GrMODE3* | 3.07 | 3.73 |
| ad-GrMODE4* | 4.76 | 4.30 |
| ad-GrMODE5* | 5.10 | 4.81 |
| Compared with | Problem Suite | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE |
|---|---|---|---|---|---|---|---|---|---|
| Comparison of overall performance based on the HV | |||||||||
| Ad-GrMODE1* (+/=/-) | DTLZ | 16/3/9 | 22/2/4 | 23/3/2 | 15/5/8 | 20/3/5 | 12/5/11 | 25/2/1 | 26/1/1 |
| WFG | 16/14/6 | 19/10/7 | 15/9/12 | 16/8/12 | 28/8/0 | 20/12/4 | 28/5/3 | 22/11/3 | |
| Overall | 32/17/15 | 41/12/11 | 38/12/14 | 31/13/20 | 48/11/5 | 32/17/15 | 53/7/4 | 48/12/4 | |
| Ad-GrMODE2* (†/≡/↓) | DTLZ | 16/2/10 | 22/1/5 | 21/3/24 | 13/3/12 | 20/2/6 | 13/3/12 | 25/1/2 | 26/1/1 |
| WFG | 15/14/7 | 19/10/7 | 15/9/12 | 16/8/12 | 28/8/0 | 20/12/4 | 28/4/4 | 22/10/4 | |
| Overall | 31/16/17 | 41/11/12 | 36/12/16 | 29/11/24 | 48/10/6 | 33/15/16 | 53/5/6 | 48/11/5 | |
| Comparison of overall performance based on the IGD | |||||||||
| Ad-GrMODE1* (+/=/-) | DTLZ | 23/1/4 | 23/2/3 | 23/1/4 | 23/1/4 | 23/0/5 | 20/1/7 | 24/0/4 | 26/1/1 |
| WFG | 23/6/7 | 25/5/6 | 21/5/10 | 24/5/7 | 31/4/1 | 25/5/6 | 33/1/2 | 24/4/8 | |
| Overall | 46/7/11 | 48/7/9 | 44/6/14 | 47/6/11 | 54//4/6 | 45/6/13 | 57/1/6 | 50/5/9 | |
| Ad-GrMODE2* (†/≡/↓) | DTLZ | 22/1/5 | 23/0/5 | 23/1/4 | 19/3/6 | 23/0/5 | 20/1/7 | 24/0/4 | 27/0/1 |
| WFG | 22/5/9 | 24/8/4 | 21/4/11 | 23/5/8 | 31/5/0 | 24/4/8 | 33/1/2 | 23/3/10 | |
| Overall | 44/6/14 | 47/8/9 | 44/5/15 | 42/8/14 | 54/5/5 | 44/5/15 | 57/1/6 | 50/3/11 | |
| # | M | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | ad-GrMODE1* | ad-GrMODE2* |
|---|---|---|---|---|---|---|---|---|---|---|---|
| DTLZ1 | 3 | 0.7826 (0.0619) | 4.3906 (0.3950) | 1.9692 (0.0841) | 48.3371 (1.3896) | 6.0658 (3.2730) | 153.528 (93.8307) | 15.8150 (8.5747) | 58.7822 (32.4275) | 7.4222 (0.5226) | 6.4673 (0.2709) |
| 5 | 1.1940 (0.0396) | 6.3420 (0.1541) | 3.1808 (0.0900) | 82.4202 (0.8441) | 19.1898 (4.4339) | 607.0595 (161.9189) | 49.7501 (11.4753) | 110.7197 (63.4026) | 14.6811 (0.2029) | 12.3303 (0.2275) | |
| 8 | 1.9808 (0.2472) | 9.1888 (0.1052) | 5.1819 (0.1455) | 144.0251 (4.4180) | 38.2911 (6.6981) | 1299.47 (251.6444) | 99.9088 (17.6567) | 405.0303 (230.25) | 29.0712 (0.5332) | 23.8091 (0.3178) | |
| 10 | 3.7808 (0.2605) | 25.6134 (0.2891) | 23.3892 (0.7395) | 556.5698 (10.9311) | 81.4116 (18.5941) | 2971.669 (677.4956) | 233.7770 (56.8272) | 2.8723 × 10+3 (1.6126 × 10+3) | 129.1142 (1.7147) | 105.7771 (1.2593) |
| Problem | NSGAIII | SPEA-R | VaEA | SRA | MODE | EMyO-C | MyODEMR | GAMODE | adGrMOEA1 | adGrMODE2 |
|---|---|---|---|---|---|---|---|---|---|---|
| RCBD | 9.608 × 10−1 (5.89 × 10−3) | 9.601 × 10−1 (9.351 × 10−4) | 9.600 × 10−1 (5.71 × 10−4) | 9.611 × 10−1 (1.72 × 10−4) | 8.574 × 10−2 (2.64 × 10−4) | 9.402 × 10−1 (3.92 × 10−3) | 2.854 × 10−1 (1.85 × 10−1) | 6.231 × 10−1 (1.25 × 10−2) | 9.612 × 10−1 (1.81 × 10−4) | 9.612 × 10−1 (2.49 × 10−4) |
| PVD | 9.636 × 10−1 (5.27 × 10−3) | 9.648 × 10−1 (3.62 × 10−4) | 9.626 × 10−1 (2.08 × 10−3) | 9.661 × 10−1 (3.83 × 10−4) | 7.881 × 10−1 (1.19 × 10−2) | 9.586 × 10−1 (2.50 × 10−3) | 2.655 × 10−2 (1.45 × 10−1) | 9.602 × 10−1 (6.52 × 10−3) | 9.664 × 10−1 (2.73 × 10−4) | 9.663 × 10−1 (2.14 × 10−4) |
| GTD | 7.086 × 10−1 (1.50 × 10−2) | 6.975 × 10−1 (5.97 × 10−3) | 7.093 × 10−1 (4.62 × 10−4) | 7.080 × 10−1 (1.40 × 10−3) | 8.260 × 10−3 (9.63 × 10−5) | 7.086 × 10−1 (8.01 × 10−4) | 2.339 × 10−1 (7.53 × 10−2) | 7.092 × 10−1 (1.54 × 10−2) | 7.094 × 10−1 (4.02 × 10−4) | 7.092 × 10−1 (4.79 × 10−4) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghorbanpour, S.; Jin, Y.; Han, S. Differential Evolution with Adaptive Grid-Based Mutation Strategy for Multi-Objective Optimization. Processes 2022, 10, 2316. https://doi.org/10.3390/pr10112316
Ghorbanpour S, Jin Y, Han S. Differential Evolution with Adaptive Grid-Based Mutation Strategy for Multi-Objective Optimization. Processes. 2022; 10(11):2316. https://doi.org/10.3390/pr10112316
Chicago/Turabian StyleGhorbanpour, Samira, Yuwei Jin, and Sekyung Han. 2022. "Differential Evolution with Adaptive Grid-Based Mutation Strategy for Multi-Objective Optimization" Processes 10, no. 11: 2316. https://doi.org/10.3390/pr10112316
APA StyleGhorbanpour, S., Jin, Y., & Han, S. (2022). Differential Evolution with Adaptive Grid-Based Mutation Strategy for Multi-Objective Optimization. Processes, 10(11), 2316. https://doi.org/10.3390/pr10112316







