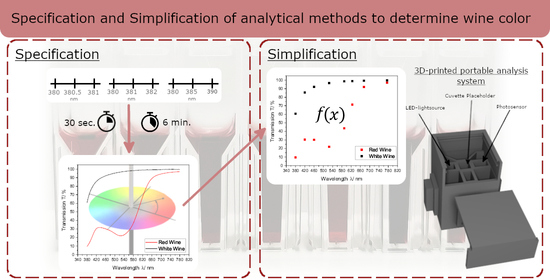
Specification and Simplification of Analytical Methods to Determine Wine Color
Abstract
:1. Introduction
2. Materials and Methods
2.1. Wines
2.2. Specification of Photometer Settings to Obtain CIE L*a*b* Coordinates
2.3. Comparison between CIE L*a*b* Color Space and Glories Color Measurement
2.4. Portable Analysis System
2.4.1. Measurement Chamber
2.4.2. Data Recording
2.4.3. Data Processing of Single Wavelength Measurements to Retrieve CIE L*a*b* Coordinates
Empirical Formulae to Calculate Wine Color
Interpolation Methods
Selection of Supporting Points for Interpolation
2.5. Statistical Analysis
3. Results and Discussion
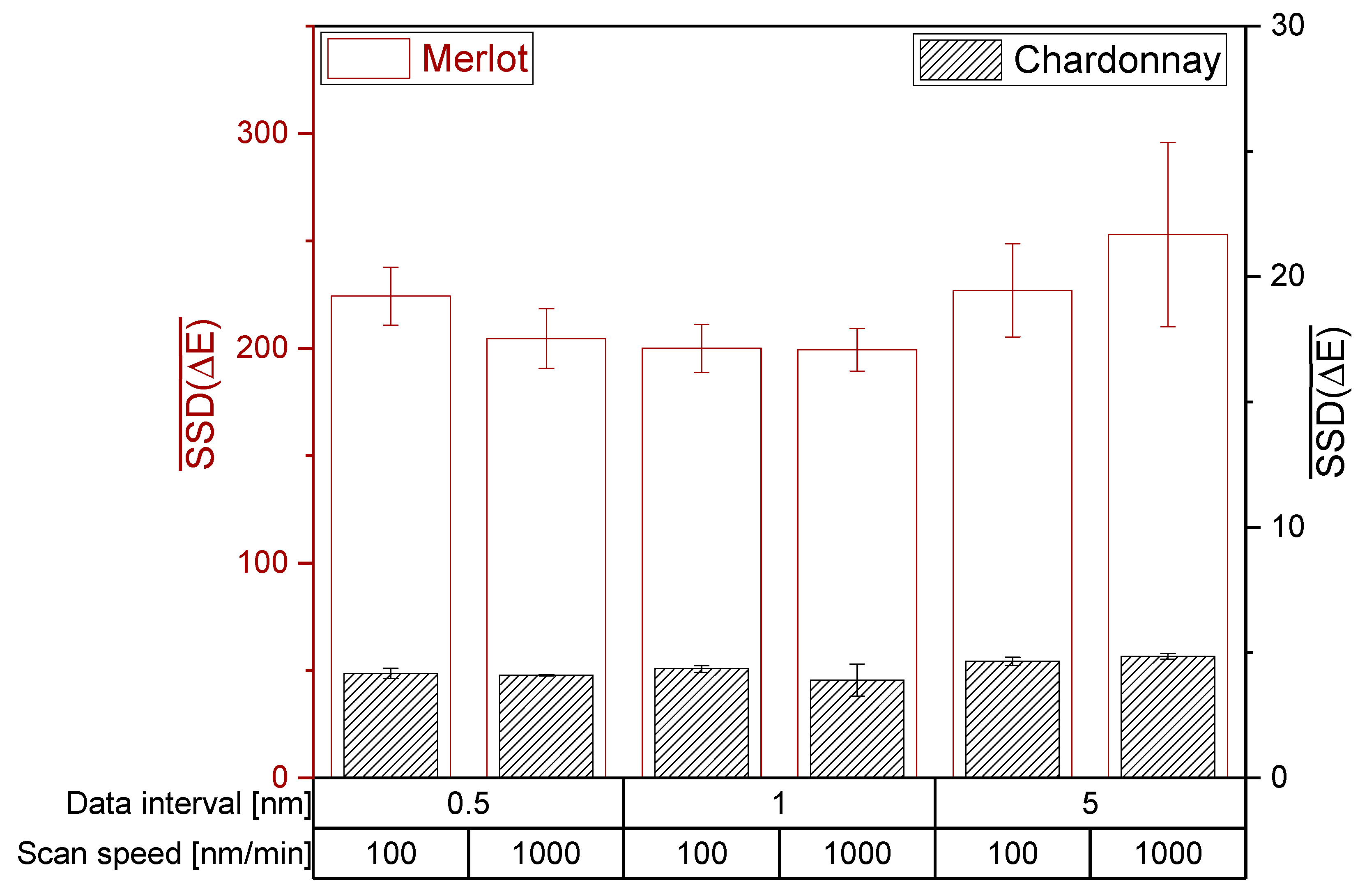
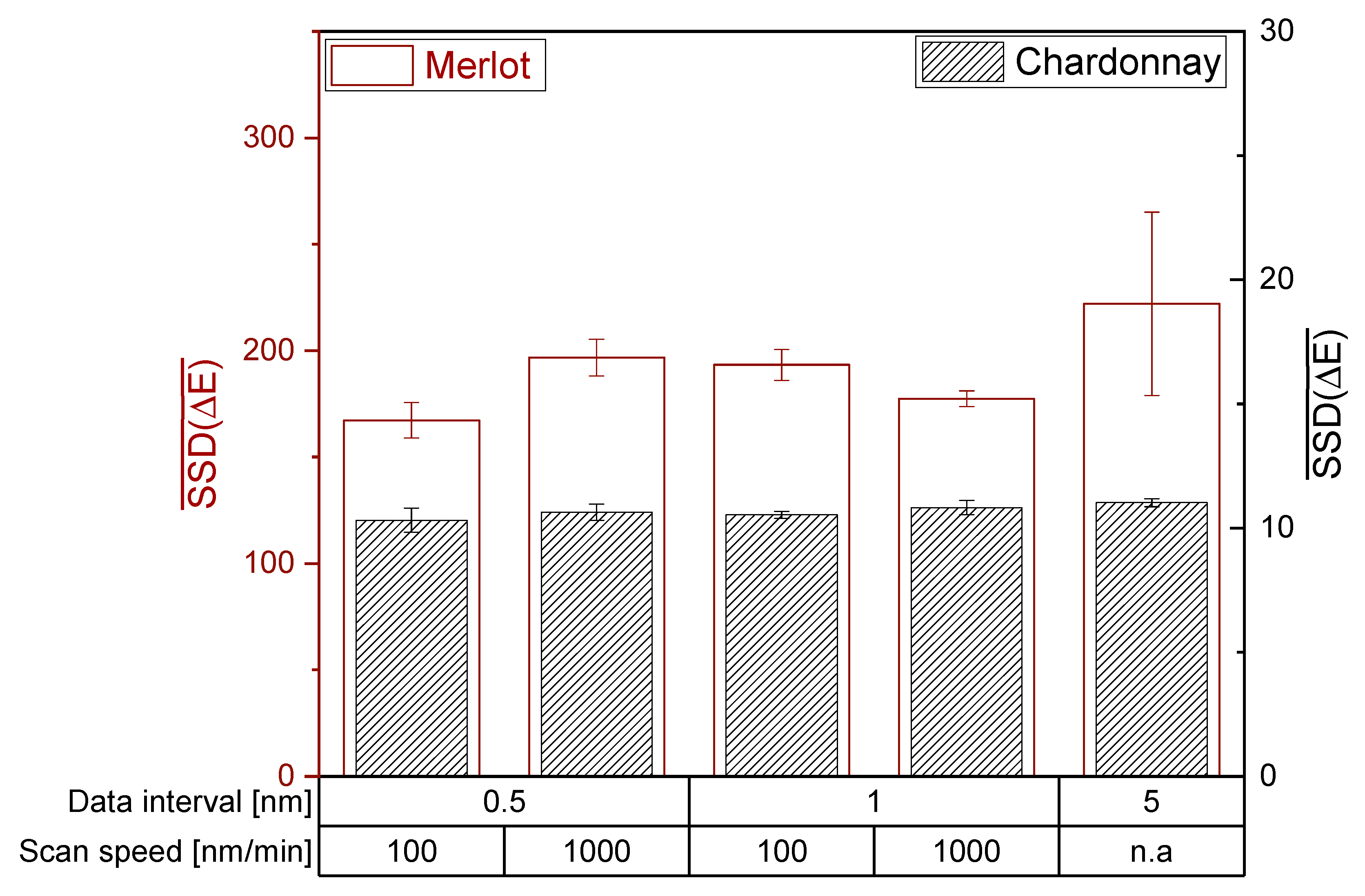
3.1. Influence of Photometer Settings on the Ability to Distinguish Wines and to Obtain Reproducible CIE L*a*b* Coordinates
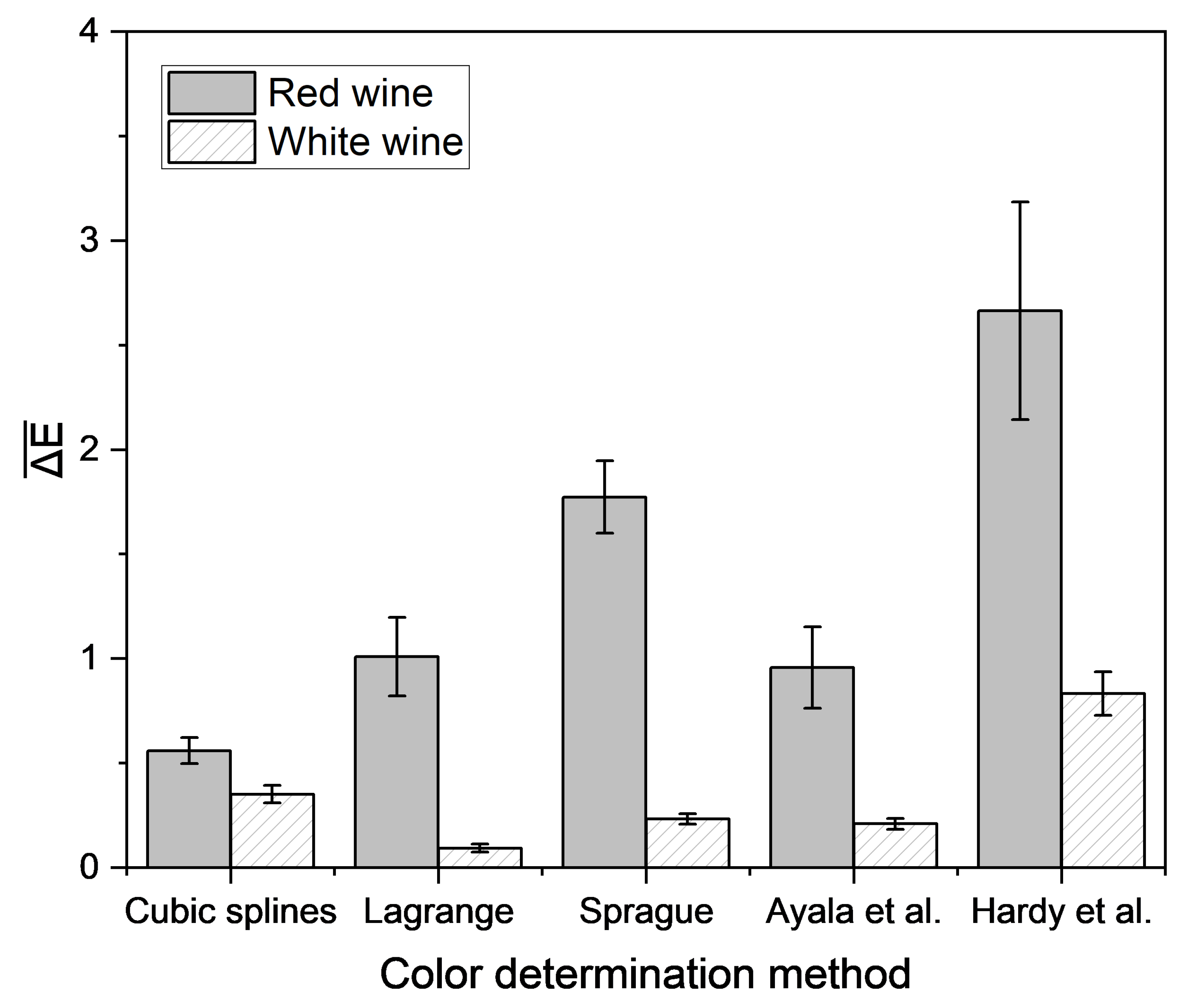
3.2. Correlation between CIE L*a*b* and Glories Method
3.3. Simplification of Wine Color Measurement
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| A | Absorbance |
| ANOVA | Analysis of Variance |
| CIE | Commission Internationale de L’Eclairage |
| ΔE | Euclidean color distance |
| LED | Light Emitting Diodes |
| SMD-LED | Surface-mounted-device LED |
| SSD | Sum of squared deviations |
| OIV | Organisation Internationale de la Vigne et du Vin |
References
- Peynaud, E.; Blouin, J. El Gusto del Vino: El gran Libro de la Degustación, 2a. ed., reimp ed.; Mundi-Prensa: Madrid, Spain, 2002. [Google Scholar]
- Morrot, G.; Brochet, F.; Dubourdieu, D. The color of odors. Brain Lang. 2001, 79, 309–320. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grassmann. XXXVII. On the theory of compound colours. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1854, 7, 254–264. [Google Scholar] [CrossRef]
- Glories, Y. La couleur des vins rouges. lre partie: Les équilibres des anthocyanes et des tanins. OENO ONE 2016, 18, 195. [Google Scholar] [CrossRef] [Green Version]
- Ribéreau-Gayon, P. Handbook of Enology: Volume 2: The Chemistry of Wine Stabilization and Treatments, 2nd ed.; John Wiley: Chichester/West Sussex, England, 2006. [Google Scholar]
- ISO/CIE 11664-1:2019; Colorimetry—Part 1: CIE Standard Colorimetric Observers. DIN e.V.: Berlin, Germany, 2019.
- DIN EN ISO/CIE 11664-2:2019; Colorimetry—Part 2: CIE Standard Illuminants. DIN e.V.: Berlin, Germany, 2011.
- Wright, W.D. A re-determination of the trichromatic coefficients of the spectral colours. Trans. Opt. Soc. 1929, 30, 141–164. [Google Scholar] [CrossRef]
- Wright, W.D. A re-determination of the mixture curves of the spectrum. Trans. Opt. Soc. 1930, 31, 201–218. [Google Scholar] [CrossRef]
- Guild, J. The colorimetric properties of the spectrum. Philos. Trans. R. Soc. Lond. Ser. A Contain. Pap. Math. Phys. Character 1931, 230, 149–187. [Google Scholar] [CrossRef] [Green Version]
- Stiles, W.S.; Burch, J.M. N.P.L. Colour-matching Investigation: Final Report (1958). Opt. Acta Int. J. Opt. 1959, 6, 1–26. [Google Scholar] [CrossRef]
- DIN EN ISO/CIE 11664-4:2019; Colorimetry—Part 4: CIE 1976 L*a*b* Colour Space. DIN e.V.: Berlin, Germany, 2020.
- DIN EN ISO/CIE 11664-6:2019; Colorimetry—Part 6: CIEDE2000 Colour-Difference Formula. DIN e.V.: Berlin, Germany, 2016.
- Compendium of International Analysis of Methods-Method OIV-MA-AS2-11: Determination of Chromatic Characteristics According to CIELab; International Organisation of Wine and Vine: Paris, France, 2006; ISBN 978-2-85038-033-4.
- Compendium of International Analysis of Methods-OIV Chromatic Characteristics-Method OIV-MA-AS2-07B: Determination of Chromatic Characteristics According to CIELab; International Organisation of Wine and Vine: Paris, France, 2006; ISBN 978-2-85038-033-4.
- Pérez-Magariño, S.; González-Sanjosé, M.L. Application of absorbance values used in wineries for estimating CIELAB parameters in red wines. Food Chem. 2003, 81, 301–306. [Google Scholar] [CrossRef]
- García-Marino, M.; Escudero-Gilete, M.L.; Heredia, F.J.; Escribano-Bailón, M.T.; Rivas-Gonzalo, J.C. Color-copigmentation study by tristimulus colorimetry (CIELAB) in red wines obtained from Tempranillo and Graciano varieties. Food Res. Int. 2013, 51, 123–131. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.K.; Lan, Y.B.; Huang, Y.; Zhao, X.; Duan, C.Q. Targeted metabolomics of anthocyanin derivatives during prolonged wine aging: Evolution, color contribution and aging prediction. Food Chem. 2021, 339, 127795. [Google Scholar] [CrossRef]
- Wang, L.J.; Naudé, N.; Chang, Y.C.; Crivaro, A.; Kamoun, M.; Wang, P.; Li, L. An ultra-low-cost smartphone octochannel spectrometer for mobile health diagnostics. J. Biophotonics 2018, 11, e201700382. [Google Scholar] [CrossRef] [PubMed]
- Dutta, S.; Sarma, D.; Patel, A.; Nath, P. Dye-Assisted pH Sensing Using a Smartphone. IEEE Photonics Technol. Lett. 2015, 27, 2363–2366. [Google Scholar] [CrossRef]
- Ju, Y.G. Fabrication of a low-cost and high-resolution papercraft smartphone spectrometer. Phys. Educ. 2020, 55, 035005. [Google Scholar] [CrossRef] [Green Version]
- Kong, W.; Kuang, D.; Wen, Y.; Zhao, M.; Huang, J.; Yang, C. Solution Classification With Portable Smartphone-Based Spectrometer System Under Variant Shooting Conditions by Using Convolutional Neural Network. IEEE Sens. J. 2020, 20, 8789–8796. [Google Scholar] [CrossRef]
- Jian, D.; Wang, B.; Huang, H.; Meng, X.; Liu, C.; Xue, L.; Liu, F.; Wang, S. Sunlight based handheld smartphone spectrometer. Biosens. Bioelectron. 2019, 143, 111632. [Google Scholar] [CrossRef]
- Zhang, C.; Cheng, G.; Edwards, P.; Zhou, M.D.; Zheng, S.; Liu, Z. G-Fresnel smartphone spectrometer. Lab Chip 2016, 16, 246–250. [Google Scholar] [CrossRef]
- Edwards, P.; Zhang, C.; Zhang, B.; Hong, X.; Nagarajan, V.K.; Yu, B.; Liu, Z. Smartphone based optical spectrometer for diffusive reflectance spectroscopic measurement of hemoglobin. Sci. Rep. 2017, 7, 12224. [Google Scholar] [CrossRef] [Green Version]
- Maity, M.; Gantait, K.; Mukherjee, A.; Chatterjee, J. Visible Spectrum-based Classification of Malaria Blood Samples on Handheld Spectrometer. In Proceedings of the 2019 IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Auckland, New Zealand, 20–23 May 2019; IEEE: New York, NY, USA, 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Wilkes, T.C.; Pering, T.D.; McGonigle, A.J.S.; Willmott, J.R.; Bryant, R.; Smalley, A.L.; Mims, F.M.; Parisi, A.V.; England, R.A. The PiSpec: A Low-Cost, 3D-Printed Spectrometer for Measuring Volcanic SO2 Emission Rates. Front. Earth Sci. 2019, 7. [Google Scholar] [CrossRef]
- Wilkes, T.C.; McGonigle, A.J.S.; Willmott, J.R.; Pering, T.D.; Cook, J.M. Low-cost 3D printed 1 nm resolution smartphone sensor-based spectrometer: Instrument design and application in ultraviolet spectroscopy. Opt. Lett. 2017, 42, 4323–4326. [Google Scholar] [CrossRef]
- Albert, D.R.; Todt, M.A.; Davis, H.F. A Low-Cost Quantitative Absorption Spectrophotometer. J. Chem. Educ. 2012, 89, 1432–1435. [Google Scholar] [CrossRef]
- Long, K.D.; Woodburn, E.V.; Le, H.M.; Shah, U.K.; Lumetta, S.S.; Cunningham, B.T. Multimode smartphone biosensing: The transmission, reflection, and intensity spectral (TRI)-analyzer. Lab Chip 2017, 17, 3246–3257. [Google Scholar] [CrossRef] [PubMed]
- Zhao, R.A.; Shen, T.; Lang, T.; Cao, B. Visible smartphone spectrometer based on the transmission grating. In Proceedings of the 2017 16th International Conference on Optical Communications and Networks (ICOCN), Wuzhen, China, 7–10 August 2017; pp. 1–3. [Google Scholar] [CrossRef]
- Plaipichit, S.; Wicharn, S.; Buranasiri, P. Spectroscopy system using digital camera as two dimensional detectors for undergraduate student laboratory. Mater. Today Proc. 2018, 5, 11114–11122. [Google Scholar] [CrossRef]
- Ding, H.; Chen, C.; Qi, S.; Han, C.; Yue, C. Smartphone-based spectrometer with high spectral accuracy for mHealth application. Sens. Actuators A Phys. 2018, 274, 94–100. [Google Scholar] [CrossRef]
- Woodburn, E.V.; Long, K.D.; Cunningham, B.T. Analysis of Paper-Based Colorimetric Assays with a Smartphone Spectrometer. IEEE Sens. J. 2019, 19, 508–514. [Google Scholar] [CrossRef] [PubMed]
- Gallegos, D.; Long, K.D.; Yu, H.; Clark, P.P.; Lin, Y.; George, S.; Nath, P.; Cunningham, B.T. Label-free biodetection using a smartphone. Lab Chip 2013, 13, 2124–2132. [Google Scholar] [CrossRef]
- Liu, L.; Bi, H. Utilising Smartphone Light Sensors to Measure Egg White Ovalbumin Concentration in Eggs Collected from Yinchuan City, China. J. Chem. 2020, 2020, 1–8. [Google Scholar] [CrossRef]
- Cesar Souza Machado, C.; Da Silveira Petruci, J.F.; Silva, S.G. An IoT optical sensor for photometric determination of oxalate in infusions. Microchem. J. 2021, 168, 106466. [Google Scholar] [CrossRef]
- Khoshmaram, L.; Mohammadi, M.; Nazemi Babadi, A. A portable low-cost fluorimeter based on LEDs and a smart phone. Microchem. J. 2021, 171, 106773. [Google Scholar] [CrossRef]
- Valenzuela, I.C.; Cruz, F.R.G. (Eds.) Opto-Electric Characterization of pH Test Strip Based on Optical Absorbance Using Tri-Chromatic LED and Phototransistor; IEEE: New York, NY, USA, 2015. [Google Scholar]
- Negueruela, A.I.; Echávarri, J.F.; Pérez, M.M. A Study of Correlation Between Enological Colorimetric Indexes and CIE Colorimetric Parameters in Red Wines. Am. J. Enol. Vitic. 1995, 353–356. [Google Scholar]
- Ayala, F.; Echávarri, J.F.; Negueruela, A.I. A New Simplified Method for Measuring the Color of Wines. Am. J. Enol. Vitic. 1997, 48, 359–369. [Google Scholar]
- Hardy, A.C. Handbook of Colorimetry, 4. auflage ed.; M.I.T. Press: Cambridge, MA, USA, 1966. [Google Scholar]
- U.S. Bureau of Labor Statistics. CPI Average Price Data, U.S. City Average (AP); U.S. Bureau of Labor Statistics: Washington, DC, USA, 2022.
- Australian Government. Priority 1: Increasing Demand and the Premium Paid for All Australien Wine; Australian Government: Canberra, Australia, 2020.
- OIV. The State of Vitiviniculture; OIV: Paris, France, 2020. [Google Scholar]
- Anderson, K.; Nelgen, S. Database of Regional, National and Global Winegrape Bearing Areas by Variety, 1960 to 2016. Available online: https://economics.adelaide.edu.au/wine-economics/databases#database-of-regional-national-and-global-winegrape-bearing-areas-by-variety-1960-to-2016 (accessed on 2 September 2020).
- Di Nonno, S.; Ulber, R. Portuino-A Novel Portable Low-Cost Arduino-Based Photo- and Fluorimeter. Sensors 2022, 22, 7916. [Google Scholar] [CrossRef] [PubMed]
- Mouser electronics Inc. Phototransistor SFH300. Available online: https://www.mouser.de/ProductDetail/OSRAM-Opto-Semiconductors/SFH-300-FA-3-4/?qs=K5ta8V%252BWhtZU3h4ylF8ddQ==&gclid=EAIaIQobChMI5Zn5r_Hc7gIVleh3Ch2TngduEAAYASAAEgJ1w_D_BwE (accessed on 13 December 2022).
- Dahmen, W.; Reusken, A. Numerik für Ingenieure und Naturwissenschaftler, 2008th ed.; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- de Kerf, J.L. The interpolation method of Sprague-Karup. J. Comput. Appl. Math. 1975, 1, 101–110. [Google Scholar] [CrossRef] [Green Version]
- Colorimetry, 3rd ed.; CIE Technical Report; Commission Internationale de l’Eclairage: Vienna, Austria, 2004; Volume 15.
- Reback, J.; McKinney, W.; Jbrockmendel; den Bossche, J.V.; Augspurger, T.; Cloud, P.; Gfyoung; Sinhrks; Klein, A.; Roeschke, M.; et al. pandas-dev/pandas: Pandas 1.0.3. 2020; Available online: https://zenodo.org/record/3715232#.Y5k4TX1BxPY (accessed on 5 November 2022).
- Harris, C.R.; Millman, K.J.; van der Walt, S.J.; Gommers, R.; Virtanen, P.; Cournapeau, D.; Wieser, E.; Taylor, J.; Berg, S.; Smith, N.J.; et al. Array programming with NumPy. Nature 2020, 585, 357–362. [Google Scholar] [CrossRef] [PubMed]
- Hunter, J.D. Matplotlib: A 2D graphics environment. Comput. Sci. Eng. 2007, 9, 90–95. [Google Scholar] [CrossRef]
- Waskom, M. seaborn: Statistical data visualization. J. Open Source Softw. 2021, 6, 3021. [Google Scholar] [CrossRef]
- Martínez, J.A.; Melgosa, M.; Pérez, M.M.; Hita, E.; Negueruela, A.I. Note. Visual and Instrumental Color Evaluation in Red Wines. Food Sci. Technol. Int. 2001, 7, 439–444. [Google Scholar] [CrossRef]
- HEREDIA, F.J.; GUZMÁN-CHOZAS, M. The color of wine: A historical perspective, II. trichromatic methods. J. Food Qual. 1993, 16, 439–449. [Google Scholar] [CrossRef]






| Number | Region | Wavelength Region | Wavelength of Commercially Available LED |
|---|---|---|---|
| I | Lower bound ultraviolet region | 380 nm | 380 nm |
| II | Local maximum | 400–440 nm | 420 nm |
| III | Gap filler 1 | 440–510 nm | 460 nm |
| IV | Local minimum | 510–560 nm | 525 nm |
| Î V | Gap filler 1 | 560–620 nm | 590 nm |
| VI | Strong increasing slope | 620–650 nm | 625 nm |
| VII | Gap filler 1 | 650–775 nm | 675 nm |
| VIII | Edge infrared region | 780 nm | 775 nm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hensel, M.; Di Nonno, S.; Mayer, Y.; Scheiermann, M.; Fahrer, J.; Durner, D.; Ulber, R. Specification and Simplification of Analytical Methods to Determine Wine Color. Processes 2022, 10, 2707. https://doi.org/10.3390/pr10122707
Hensel M, Di Nonno S, Mayer Y, Scheiermann M, Fahrer J, Durner D, Ulber R. Specification and Simplification of Analytical Methods to Determine Wine Color. Processes. 2022; 10(12):2707. https://doi.org/10.3390/pr10122707
Chicago/Turabian StyleHensel, Marcel, Sarah Di Nonno, Yannick Mayer, Marina Scheiermann, Jörg Fahrer, Dominik Durner, and Roland Ulber. 2022. "Specification and Simplification of Analytical Methods to Determine Wine Color" Processes 10, no. 12: 2707. https://doi.org/10.3390/pr10122707
APA StyleHensel, M., Di Nonno, S., Mayer, Y., Scheiermann, M., Fahrer, J., Durner, D., & Ulber, R. (2022). Specification and Simplification of Analytical Methods to Determine Wine Color. Processes, 10(12), 2707. https://doi.org/10.3390/pr10122707