Self-Circulating Evaporative Cooling System of a Rotor and Its Experimental Verification
Abstract
:1. Introduction
2. Thermal Hydraulics Model of Self-Circulating Evaporative Cooling System of Rotor
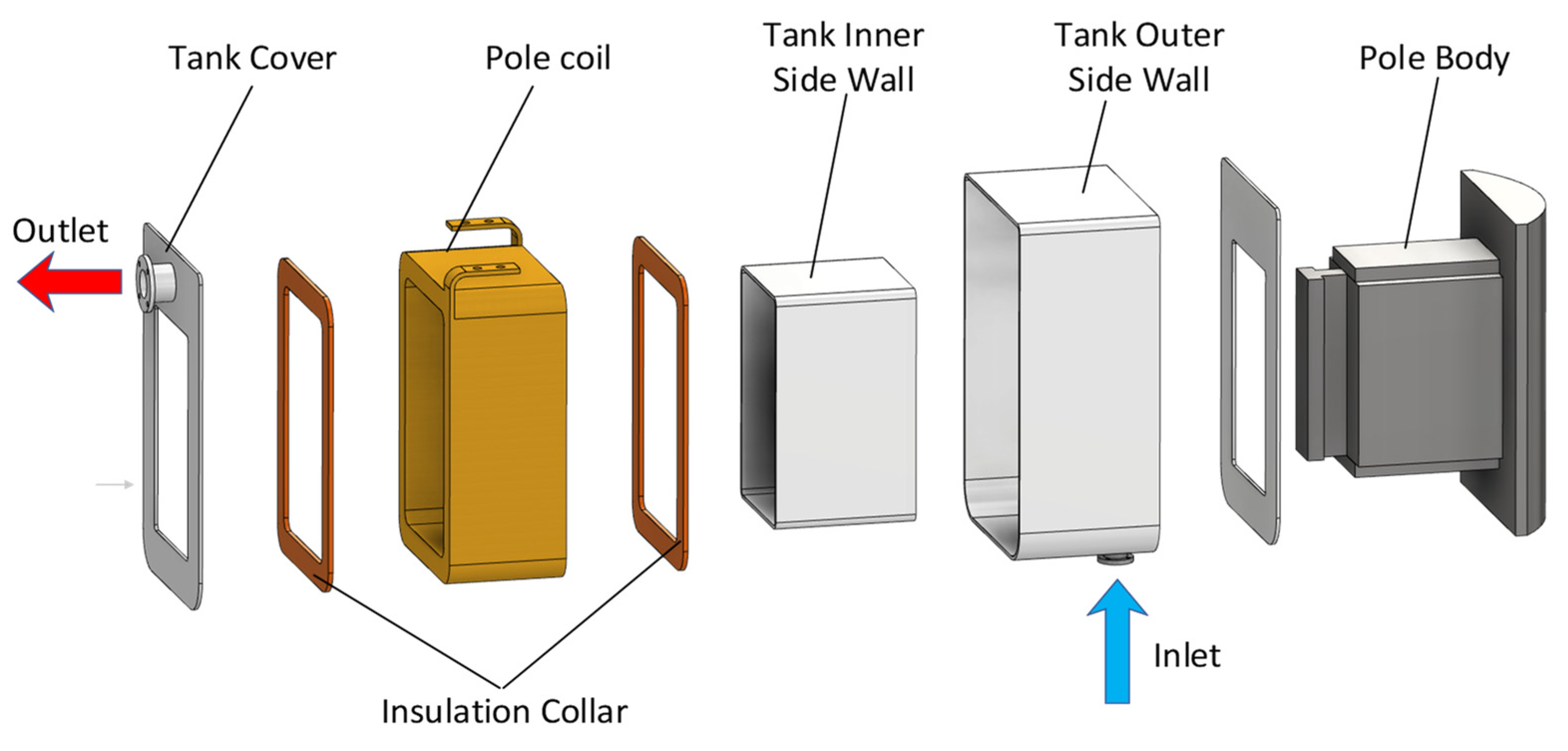
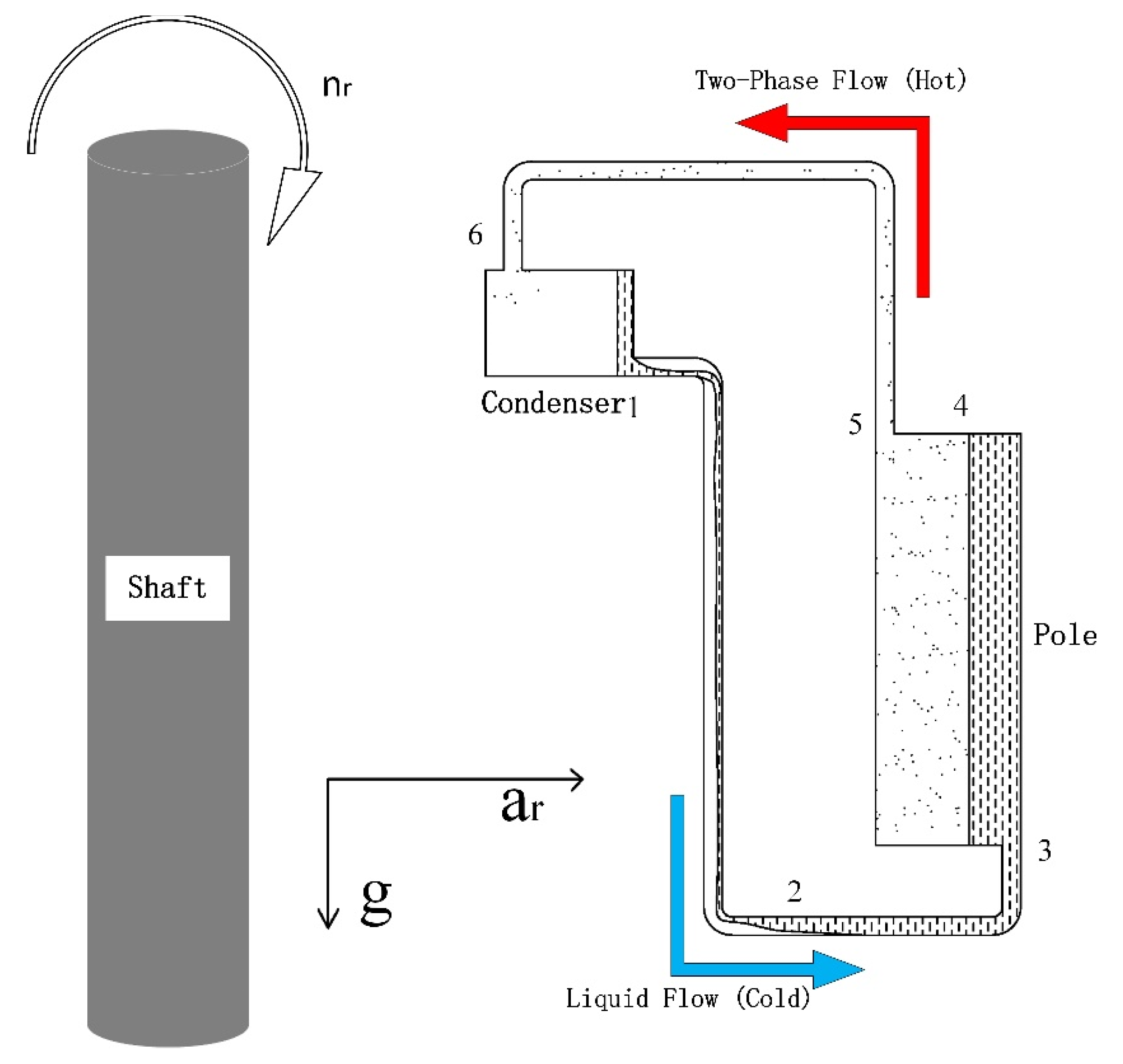
2.1. The Structural Topology
2.2. The Governing Equations
2.3. Evaluation of the Cooling System
3. Materials and Methods
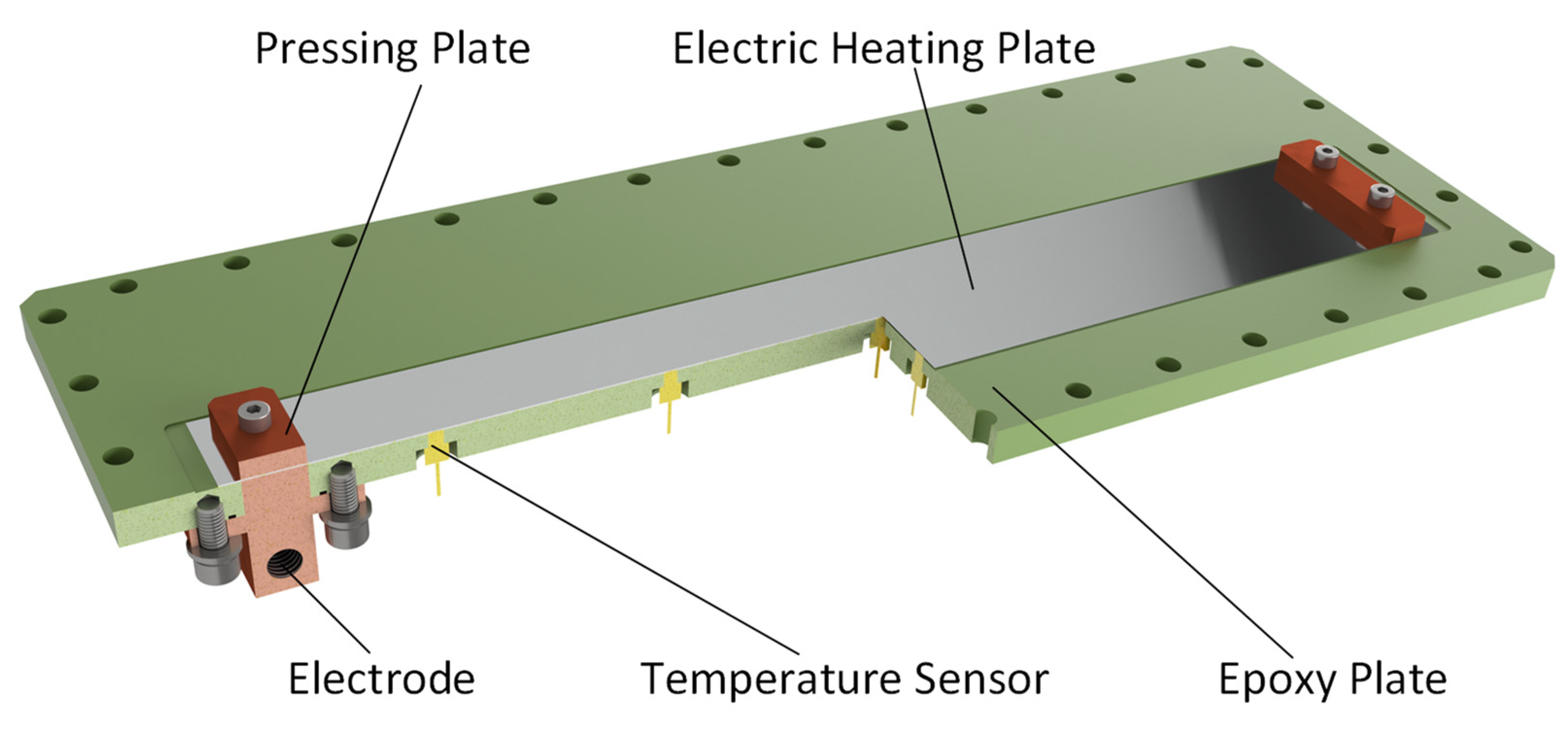
3.1. Experimental Platform Design
3.2. The Experimental Process
4. Results and Discussion
4.1. The Results of Air-Cooled Stage
4.2. The Results of Phase Change Cooling Stage
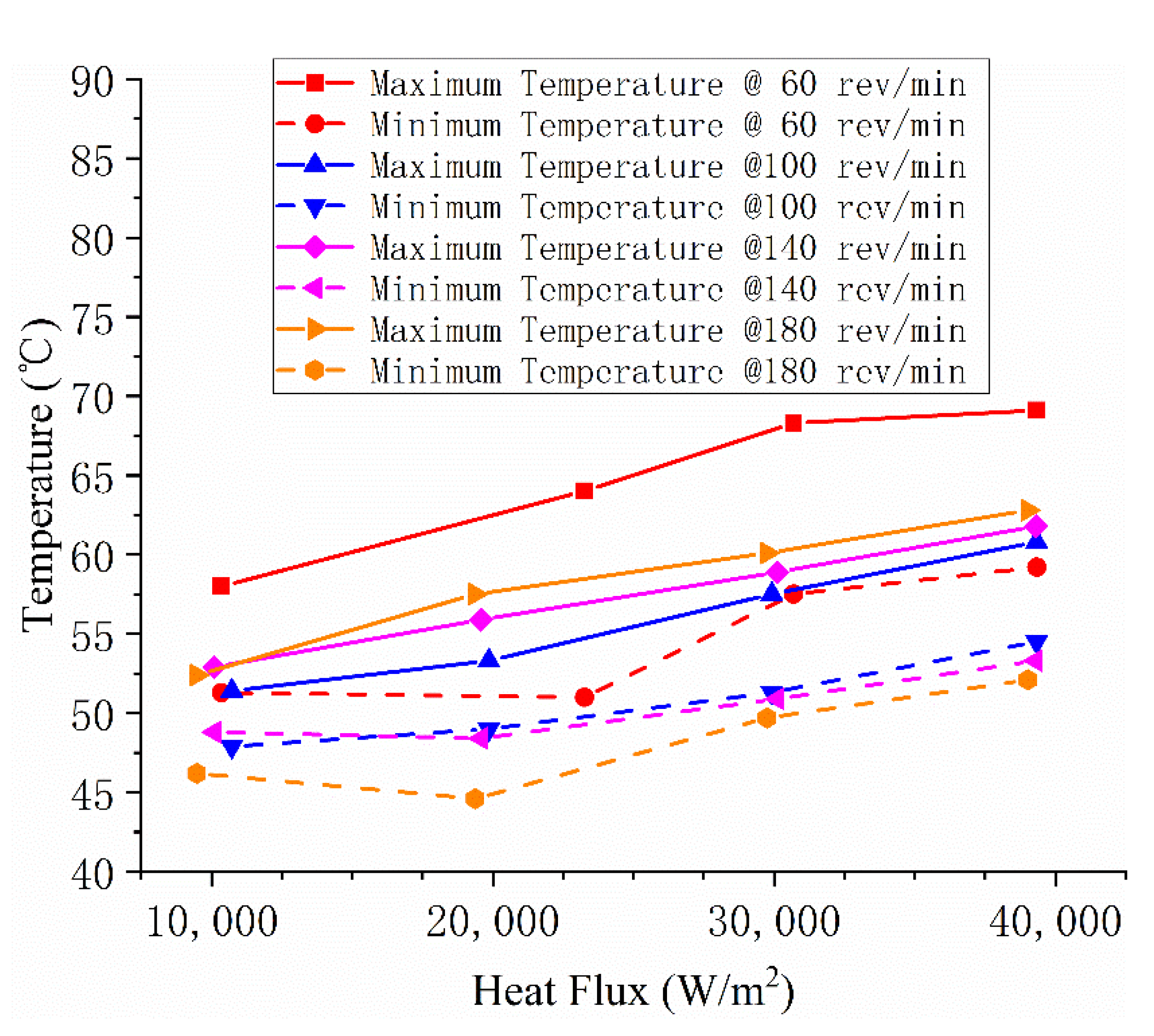
4.2.1. Characteristics of Temperature
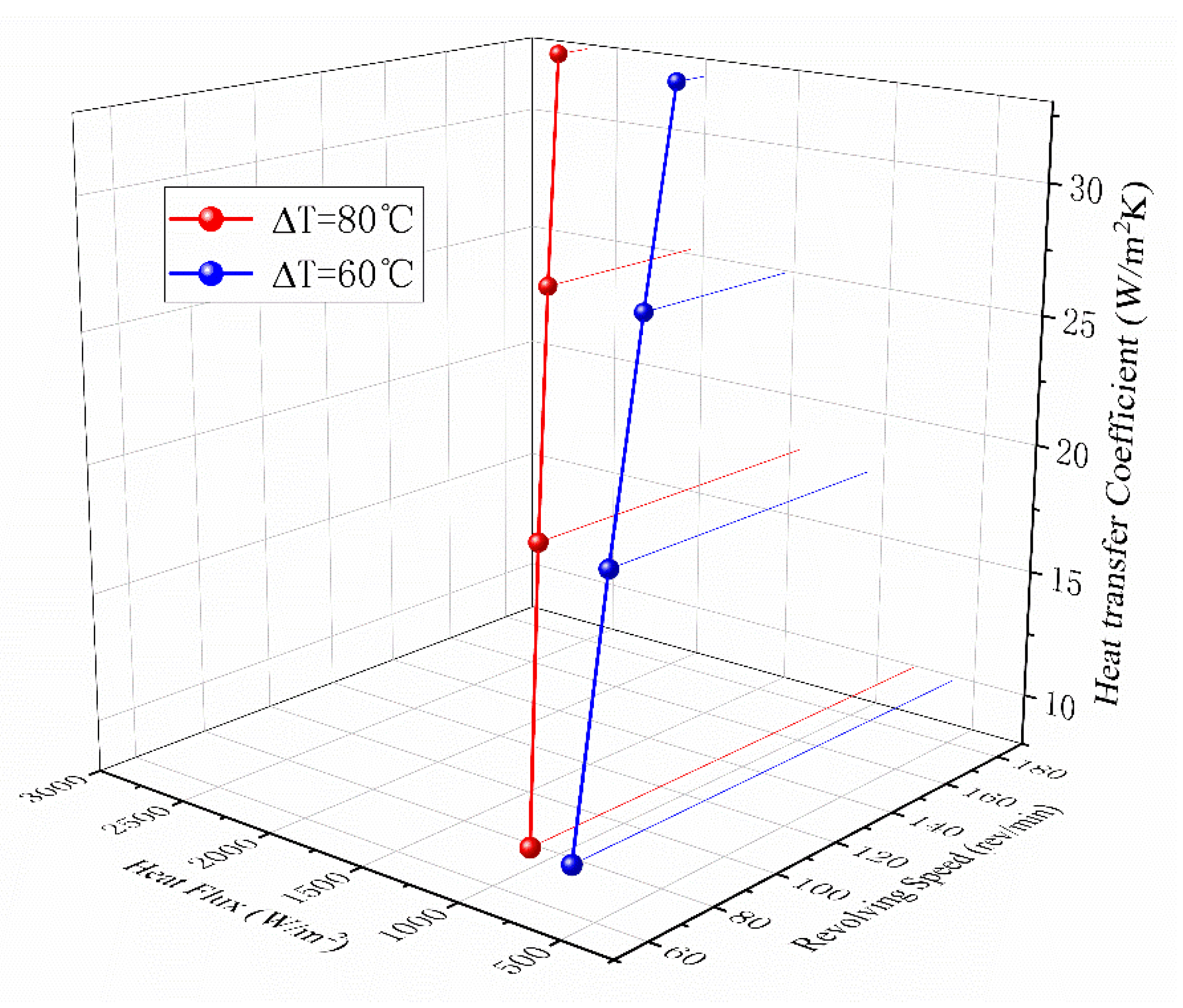
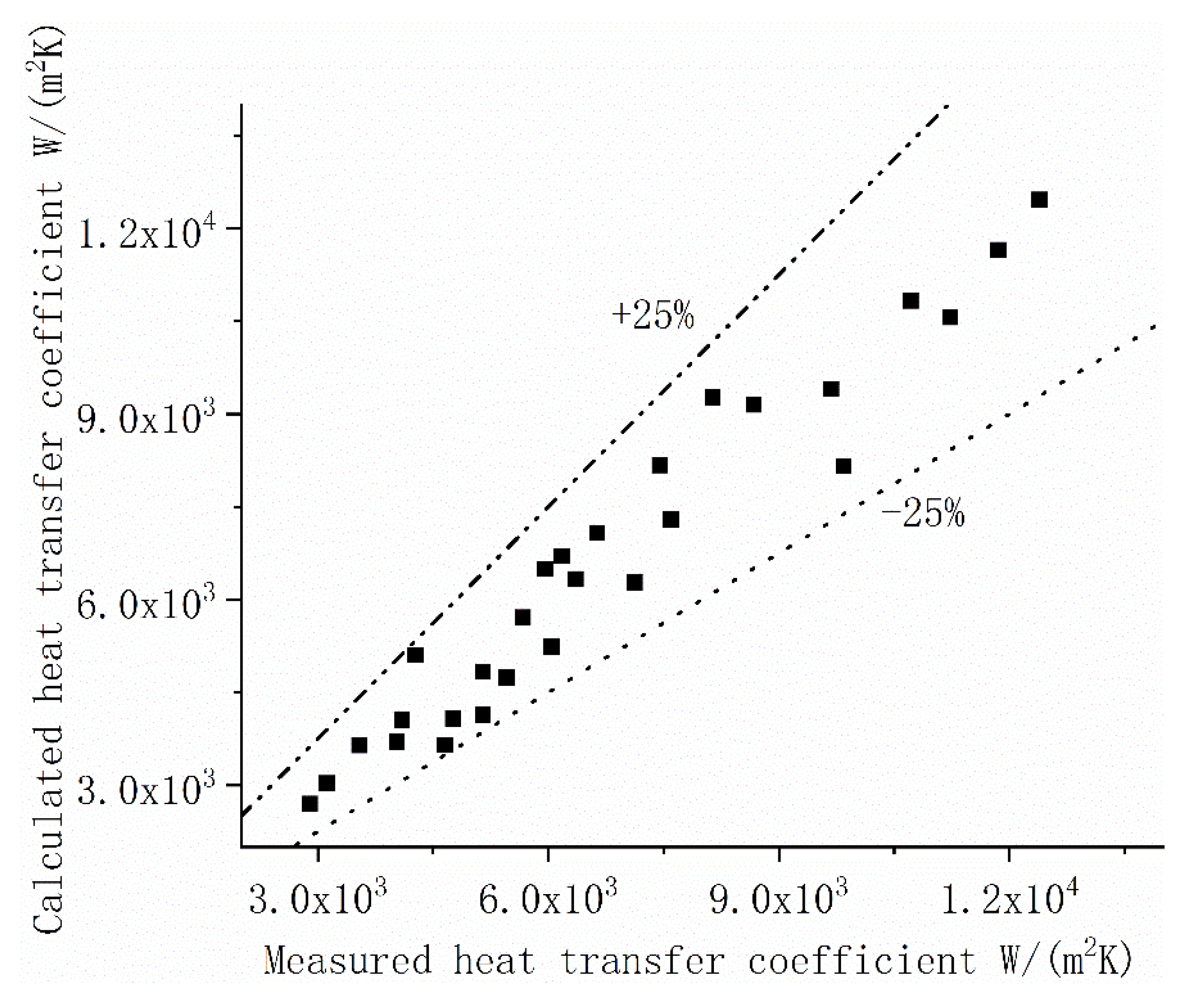
4.2.2. Calculation of Boiling Heat Transfer Coefficient
4.2.3. Characteristics of Pressure
4.3. Advantages of Rotor Evaporative Cooling Systems
4.3.1. Large Heat Transfer Coefficient
4.3.2. Relatively Low Pressure
5. Conclusions
- A new concept for an evaporative cooling system for the rotor of the hydro generator is presented. Based on the analysis of principle, it can be used to maintain the temperature of the pole coil;
- It is proved that the rotor evaporative cooling system can provide a much higher heat dissipation capacity. In the air-cooling test, the temperature rise of the heating plate is up to 80 °C under the heat flux of less than 2700 W/m2. In the phase change cooling test, the temperature rise is only 40–50 °C even if the heat flux increases to 40,000 W/m2;
- A calculation formula of Nusselt number of vertical wall boiling heat transfer under a rotating state is obtained by nonlinear regression analysis. Under experimental conditions, the accuracy of the heat transfer coefficient can reach 25%;
- The heat transfer coefficient of phase change cooling is up to 300 times that of air cooling. This has a good effect on reducing the height of the pole body, improving mechanical strength and reducing magnetic leakage;
- The pressure of the rotor evaporative cooling system does not rise monotonously with the increase of rotational speed. This is very advantageous in terms of craftsmanship.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| ∆T | Temperature difference between the hot wall surface and the coolant, °C |
| Centrifugal acceleration, m/s2 | |
| Width of the pole coil | |
| Bo | Boiling number |
| Velocity, m/s | |
| Fr | Froude number |
| Acceleration of gravity, m/s2 | |
| Gr | Grashof Number |
| Heat transfer coefficient, W/(m2K) | |
| j | Current density, A/mm2 |
| Axial position, m | |
| Revolving speed, rev/min | |
| Nu | Nusselt number |
| Heat flux, W/m2 | |
| Radial position, m | |
| Re | Reynolds number |
| Internal energy, J | |
| specific volume, m3/Kg | |
| Work, J | |
| α, β | Corner markers used to represent the beginning and end of one stage |
| Electrical conductivity of the conductor, S/m | |
| Liquid density, Kg/m3 | |
| Gas density, Kg/m3 |
References
- Torriano, F.; Stella, S.; Jayet, Y.; Ardaillon, T.; Charest-Fournier, J.P.; Hudon, C.; Merkhouf, A.; Guillot, E. Numerical and Experimental Study of the Ventilation in an Operating Hydrogenator. In Proceedings of the International Conference on Numerical Analysis and Applied Mathematics (ICNAAM-2018), Rhodes, Greece, 13–18 September 2018. [Google Scholar]
- Foster, E.N.; Parker, F.J. Hydroelectric Machines. IEE Proc. C Gener. Transm. Distrib. 1986, 133, 126–136. [Google Scholar] [CrossRef]
- Schafer, D. The world’s largest fully water-cooled hydro generators in the Bieudron power plant (Switzerland). In Proceedings of the IEMDC’99—IEEE International Electric Machines and Drives Conference, Seattle, WA, USA, 9–12 May 1999; pp. 827–829. [Google Scholar]
- Traxler-Samek, G.; Zickermann, R.; Schwery, A. Advanced Calculation of Temperature Rises in Large Air-Cooled Hydro-Generators. In Proceedings of the ICEM: 2008 International Conference on Electrical Machines, Vilamoura, Portugal, 6–9 September 2008; pp. 856–861. [Google Scholar]
- Zhang, Q.; Yan, J.; Wang, M.; Chen, Z. Numerical Simulation on Ventilation and Cooling of Hydro-Generator. In Proceedings of the Power and Energy Engineering Conference 2010, Wuhan, China, 11–12 September 2010; p. 277. [Google Scholar]
- Han, J.; Ge, B.; Tao, D.; Zhao, H.; Xiao, F.; Li, W. Calculation and analysis of complex fluid flow and thermal fields in a fully air-cooled hydrogenerator. Int. J. Therm. Sci. 2017, 116, 278–286. [Google Scholar]
- Li, W.; Zhang, Y.; Chen, Y. Calculation and Analysis of Heat Transfer Coefficients and Temperature Fields of Air-Cooled Large Hydro-Generator Rotor Excitation Windings. IEEE Trans. Energy Conv. 2011, 26, 946–952. [Google Scholar]
- Li, D.; Li, W.; Li, J.; Liu, X. Analyzing regularity of interpolar air motion and heat dissipation coefficient distribution of a salient pole synchronous generator considering rotary airflow. Int. Commun. Heat Mass Transf. 2020, 119, 104828. [Google Scholar] [CrossRef]
- Li, W.; Zhou, F.; Hou, Y.; Cheng, S.K. Calculation of rotor temperature field for hydro-generator as well as the analysis on relevant factors. Proc. Chin. Soc. Electr. Eng. 2002, 22, 85–90. [Google Scholar]
- Li, W.; Li, D.; Li, J. Analysis of hydro-generator rotor coupling field on rotor ventilation duct blocked. Electr. Mach. Control 2017, 21, 30–38. [Google Scholar]
- Li, W.; Chen, Y.; Huo, F.; Zhang, Y. Fluid Flow and Temperature Field Analysis between Two Poles of a Large Air-cooled Hydro-Generator Rotor in Rotation. Proc. Chin. Soc. Electr. Eng. 2012, 32, 132–139. [Google Scholar]
- Li, W.; Li, D.; Li, J.; Zhang, X. Influence of Rotor Radial Ventilation Ducts Number on Temperature Distribution of Rotor Excitation Winding and Fluid Flow State between Two Poles of a Fully Air-Cooled Hydro-Generator. IEEE Trans. Ind. Electron. 2017, 64, 3767–3775. [Google Scholar] [CrossRef]
- Cravero, C.; Leutcha, P.J.; Marsano, D. Simulation and Modeling of Ported Shroud Effects on Radial Compressor Stage Stability Limits. Energies 2022, 15, 2571. [Google Scholar] [CrossRef]
- Jamshidi, H.; Nilsson, H.; Chernoray, V. Experimental and numerical investigation of hydro power generator ventilation. In Proceedings of the 27th IAHR Symposium on Hydraulic Machinery and Systems (IAHR 2014), Montreal, QC, Canada, 22–26 September 2014. [Google Scholar]
- Majer, S.; Tukovic, Z. Numerical Study of Windage Power Loss for One Salient Pole Hydro Generator. In Proceedings of the 2020 International Conference on Electrical Machines (ICEM), Gothenburg, Sweden, 23–26 August 2020; Volume 1, pp. 2624–2630. [Google Scholar]
- Gu, G.B.; Ruan, L. A few questions about using the evaporative cooling method as the substitute for the water-cooling method in the three gorges’ hydro-generator. In Proceedings of the ICEMS 2005: Eighth International Conference on Electrical Machines and Systems, Nanjing, China, 27–29 September 2005; Volume 1, pp. 7–11. [Google Scholar]
- Gu, G.; Ruan, L. Applications and Developments of the Evaporative Cooling Technology in the Field of Hydrogenerators. Proc. Chin. Soc. Electr. Eng. 2014, 34, 5112–5119. [Google Scholar]
- Yuan, J.; Gu, G.; Ruan, L.; Tian, X.; Duo, L.; Zhang, T. Applied Engineering Design of Three Gorges’ 840MVA Hydro-generator with Close Loop Self Circulating Evaporative Cooling System. In Proceedings of the ICEMS 2008: 11th International Conference on Electrical Machines and Systems, Wuhan, China, 17–20 October 2008; p. 142. [Google Scholar]
- Ruan, L.; Gu, G.; Tian, X.; Chang, Z. Experimental research of new coolant in evaporative cooling technique of large generators. Adv. Technol. Electr. Eng. Energy 2002, 21, 21–24. [Google Scholar]
- Ruan, L.; Gu, G.; Tian, X. Experiment on Two-Phase Flowing Pressure Drop in the Evaporative Cooling System of Hydro-Generators. J. Tianjin Univ. 2005, 38, 27–30. [Google Scholar]
- Dong, H.; Ruan, L.; Guo, S. Operating Characteristics of Different Inner Evaporative Cooling Systems of Generators. In Proceedings of the 18th International Conference on Electrical Machines and Systems (ICEMS), Pattaya, Thailand, 25–28 October 2015; pp. 1097–1100. [Google Scholar]
- Dong, H.; Ruan, L. Research on Loop Resistance in Self-circulation Evaporative Cooling System of Stator Collector Ring of Hydro-generator. In Proceedings of the 2013 International Conference on Electrical Machines and Systems (ICEMS), Busan, Korea, 26–29 October 2013; pp. 1437–1440. [Google Scholar]
- Xiong, B.; Gu, G.; Ruan, L.; Li, Z.; Fu, D.; Cao, R.; Guo, S.; Gao, J. Improvement of Inner Evaporative Cooling Experiment Model for Large Turbo Generator Stator Bars. In Proceedings of the 2013 International Conference on Electrical Machines and Systems (ICEMS), Busan, Korea, 26–29 October 2013; pp. 101–104. [Google Scholar]
- Dong, H.; Ruan, L. Research on the Temperature Distribution of Stator Collector Ring in Evaporative Cooling Hydro-generator. Electr. Power Energy Syst. 2012, 516–517, 1622–1625. [Google Scholar] [CrossRef]
- Liu, F.; Ruan, L.; Li, Z.; Guo, S. The Calculation on the Coolant Temperature of the Condenser outlet in the Evaporative Cooling System of the Hydro-generator. In Proceedings of the 2013 International Conference on Electrical Machines and Systems (ICEMS), Busan, Korea, 26–29 October 2013; pp. 87–90. [Google Scholar]
- Ruan, L.; Gu, G. Technical feasibility research of application of evaporative cooling technique to large Three Gorges’ hydrogenerators—The selection of material and the size for the hollow conductors in the stator windings. J. Harbin Inst. Technol. 2005, 37, 401–404. [Google Scholar]
- Ruan, L.; Gu, G.; Tian, X.; Chang, Z.; Yuan, J. Analysis of the key techniques in hydro-generator with inner evaporative cooling. In Proceedings of the ICEMS 2003: Sixth International Conference on Electrical Machines and Systems, Beijing, China, 9–11 November 2003; Volume 1, pp. 14–16. [Google Scholar]
- Liu, F.; Ruan, L.; Li, Z. The Calculation on the Coolant Dryness of the Hollow Conductor Outlet in the Evaporative Cooling System of the Hydro-generator. In Proceedings of the 2013 International Conference on Electrical Machines and Systems (ICEMS), Busan, Korea, 26–29 October 2013; pp. 91–94. [Google Scholar]
- Ruan, L.; Chen, J.; Gu, G. Comparison and analysis of stator’s air cooling and evaporative cooling method of 1000MW hydrogenerators. Adv. Technol. Electr. Eng. Energy 2014, 33, 1–6. [Google Scholar]
- Gu, G.; Ruan, L.; Liu, F.; Xiong, B. Developments, Applications and Prospects of Evaporative Cooling Technology. Transa. China Electrotech. Soc. 2015, 30, 1–6. [Google Scholar]
- Zhi, L.; Lin, R. Design for gas-liquid-solid stator insulation system in the evaporative cooling turbogenerator. Electr. Mach. Control 2018, 22, 77–84. [Google Scholar]
- Zhi, L.; Lin, R. Theoretical Analysis and Experimental Verification on Mathematical and Physical Model of Gas-Liquid-Solid Insulation System. Trans. China Electrotech. Soc. 2018, 33, 2097–2104. [Google Scholar]
- Yao, T.; Hou, Z.; Gu, G. Application of evaporative cooling technique to large turbo generator. Trans. China Electrotech. Soc. 2008, 23, 1–6. [Google Scholar]


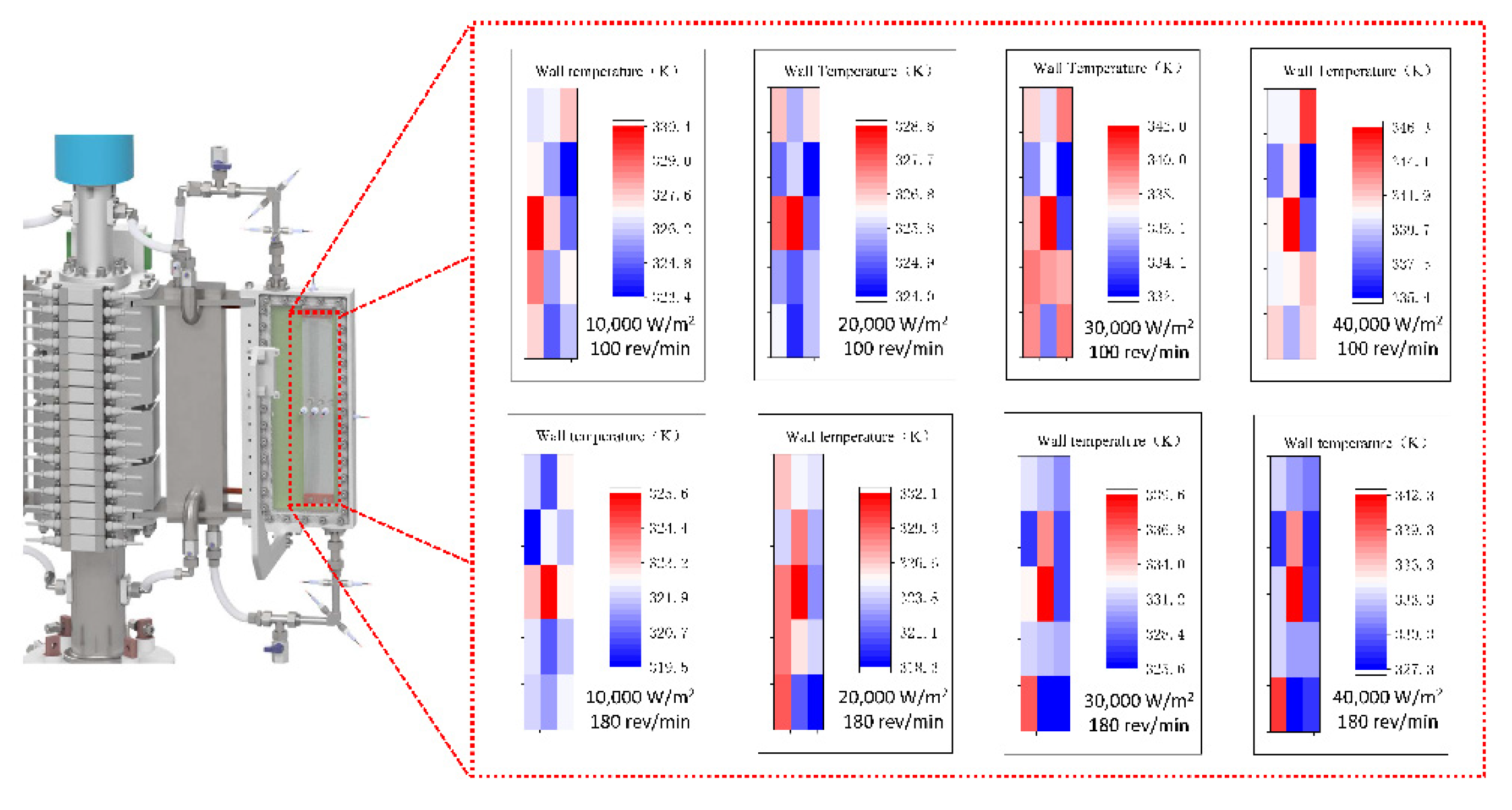


| Device | Range | Accuracy |
|---|---|---|
| Speed measuring instrument | 0~500 rpm | 0.25% |
| AC voltage transmitter | 0~5 V | 0.5% |
| AC current transmitter | 0~200 A | 0.5% |
| RTD | 0~200 °C | A level |
| Micro Pressure Sensor | −50~100 kPa | 0.1% |
| Variable | Unit | Value | ||||
|---|---|---|---|---|---|---|
| Air-Cooling | Evaporate-Cooling | |||||
| Revolving Speed | rev/min | 180 | 60.0 | 100 | 140 | 180 |
| Heat Flux | W/m2 | Adjust the value so that the wall temperature reaches the value of 100 °C | 10,000 | 20,000 | 30,000 | 40,000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Ruan, L. Self-Circulating Evaporative Cooling System of a Rotor and Its Experimental Verification. Processes 2022, 10, 934. https://doi.org/10.3390/pr10050934
Wang Y, Ruan L. Self-Circulating Evaporative Cooling System of a Rotor and Its Experimental Verification. Processes. 2022; 10(5):934. https://doi.org/10.3390/pr10050934
Chicago/Turabian StyleWang, Yu, and Lin Ruan. 2022. "Self-Circulating Evaporative Cooling System of a Rotor and Its Experimental Verification" Processes 10, no. 5: 934. https://doi.org/10.3390/pr10050934
APA StyleWang, Y., & Ruan, L. (2022). Self-Circulating Evaporative Cooling System of a Rotor and Its Experimental Verification. Processes, 10(5), 934. https://doi.org/10.3390/pr10050934






