Simulation Prediction and Experiment of Brittle Damage of Cemented Carbide Microgroove Turning Tools Based on Peridynamics
Abstract
:1. Introduction
2. Materials and Methods
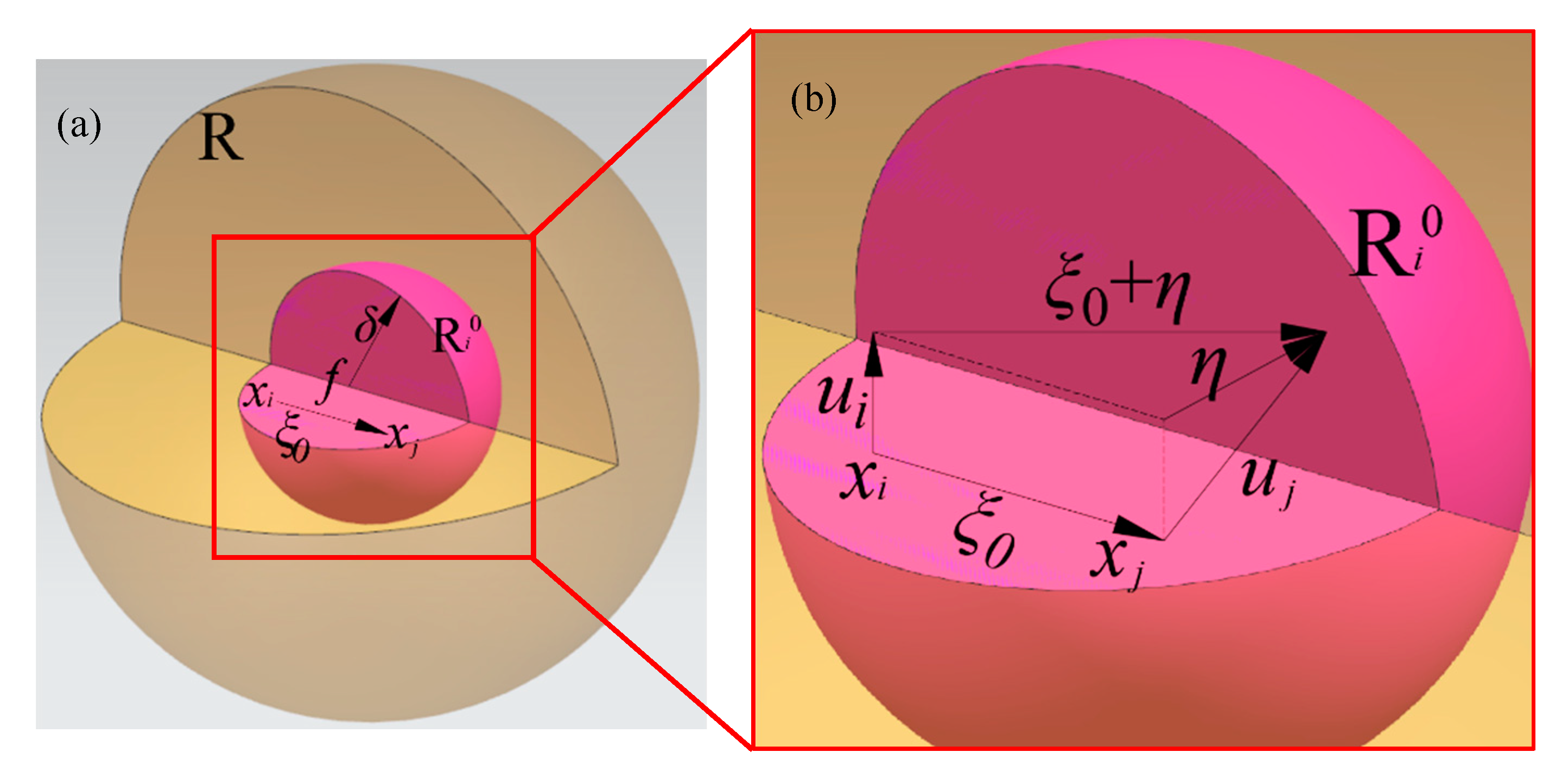
2.1. Establishment of Bond-Based Peridynamic Methods
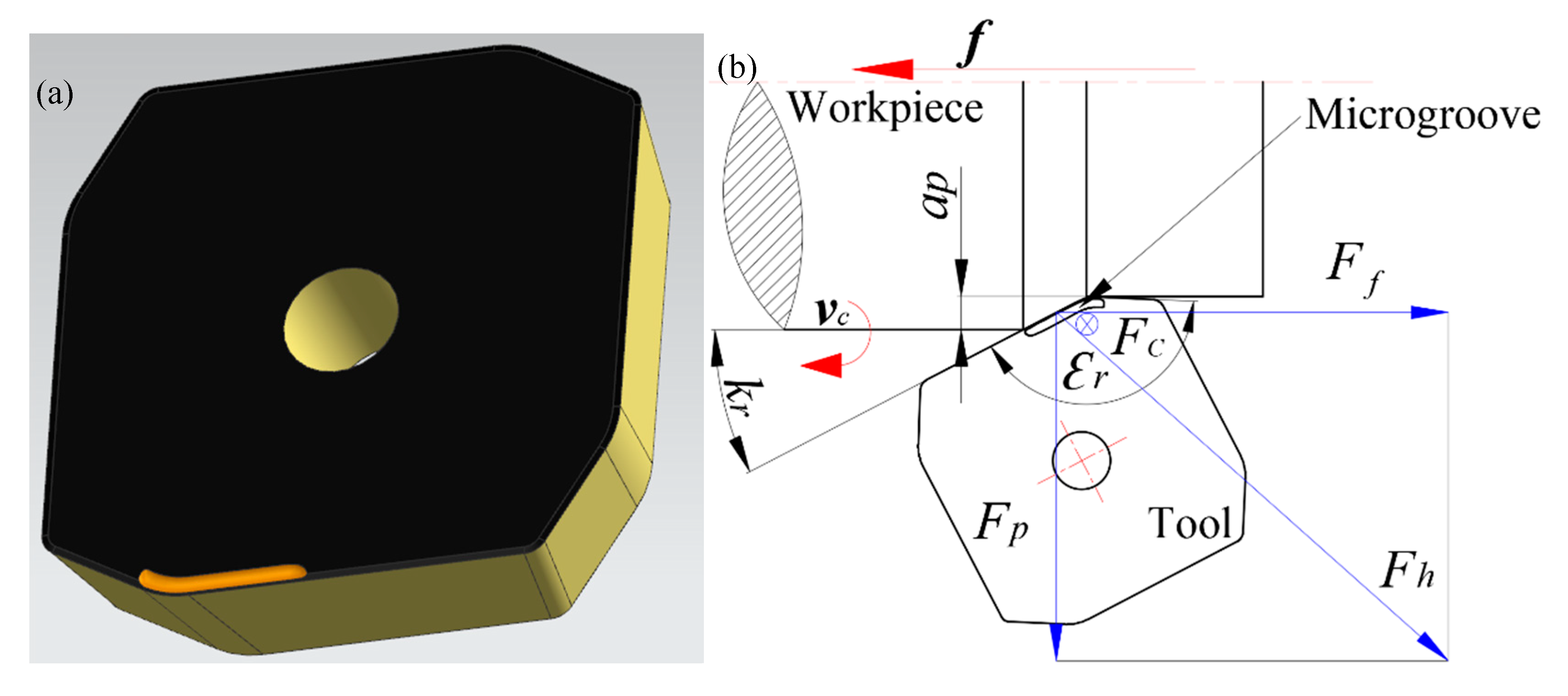
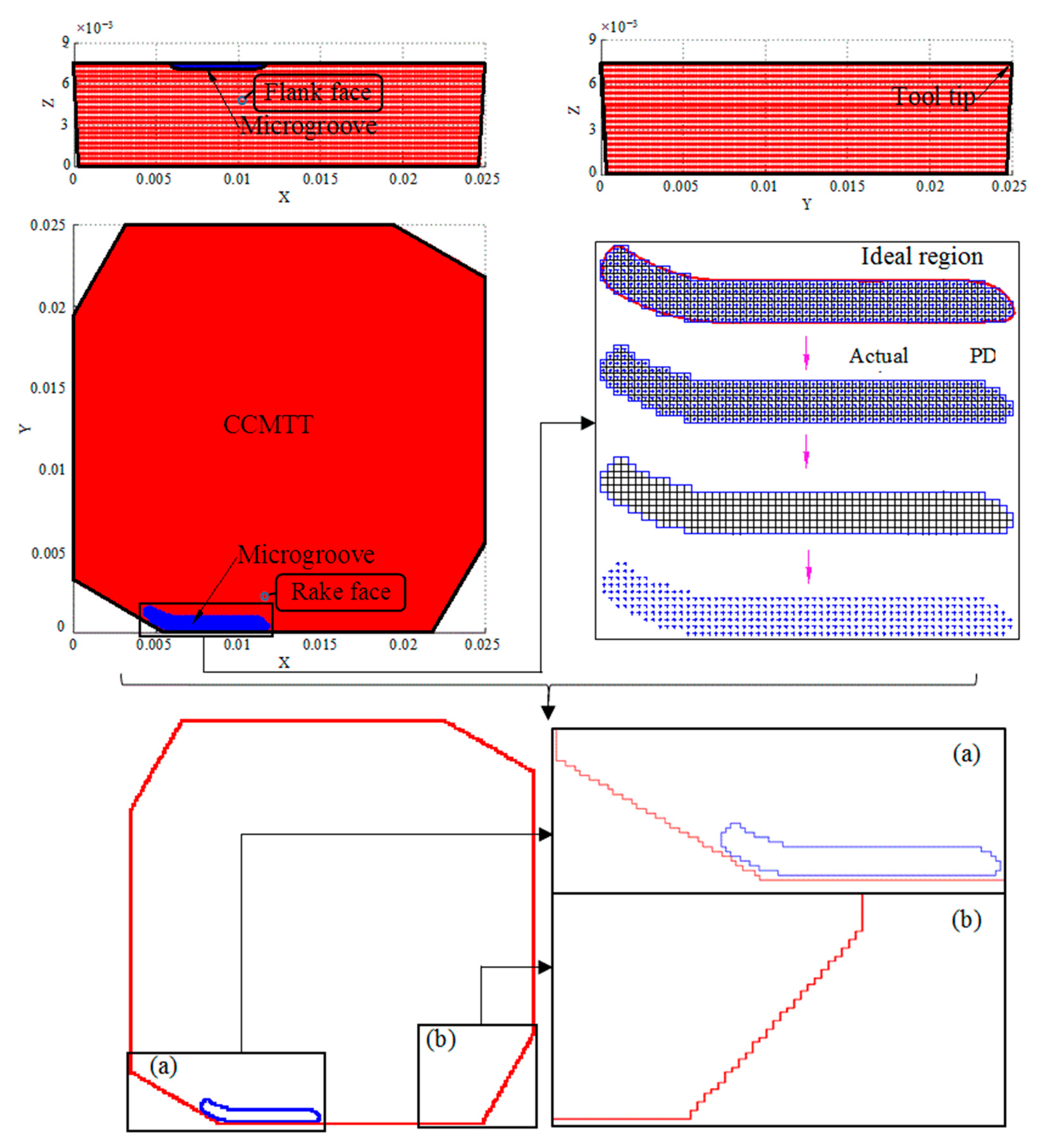
2.2. PD Model of CCMTT
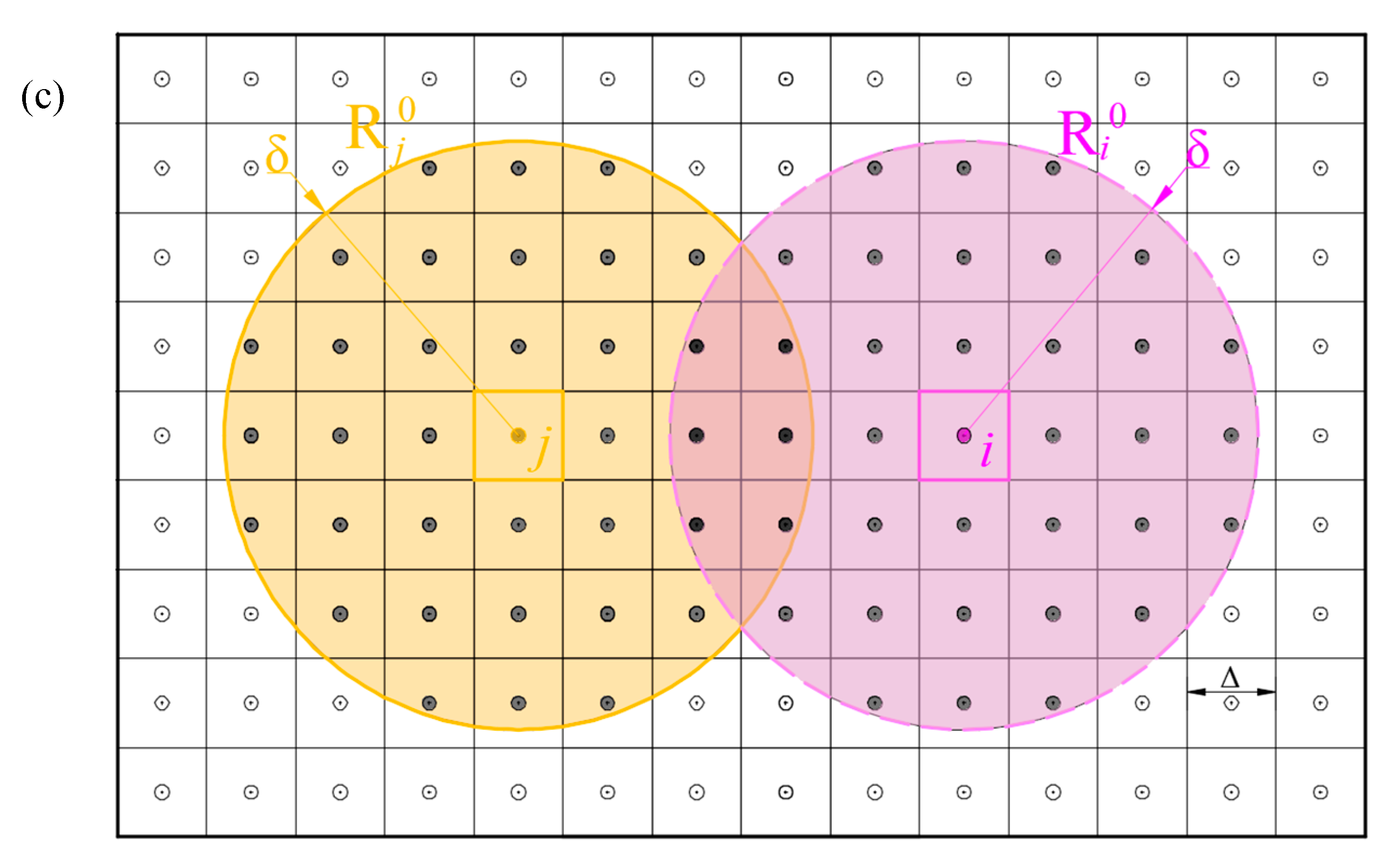
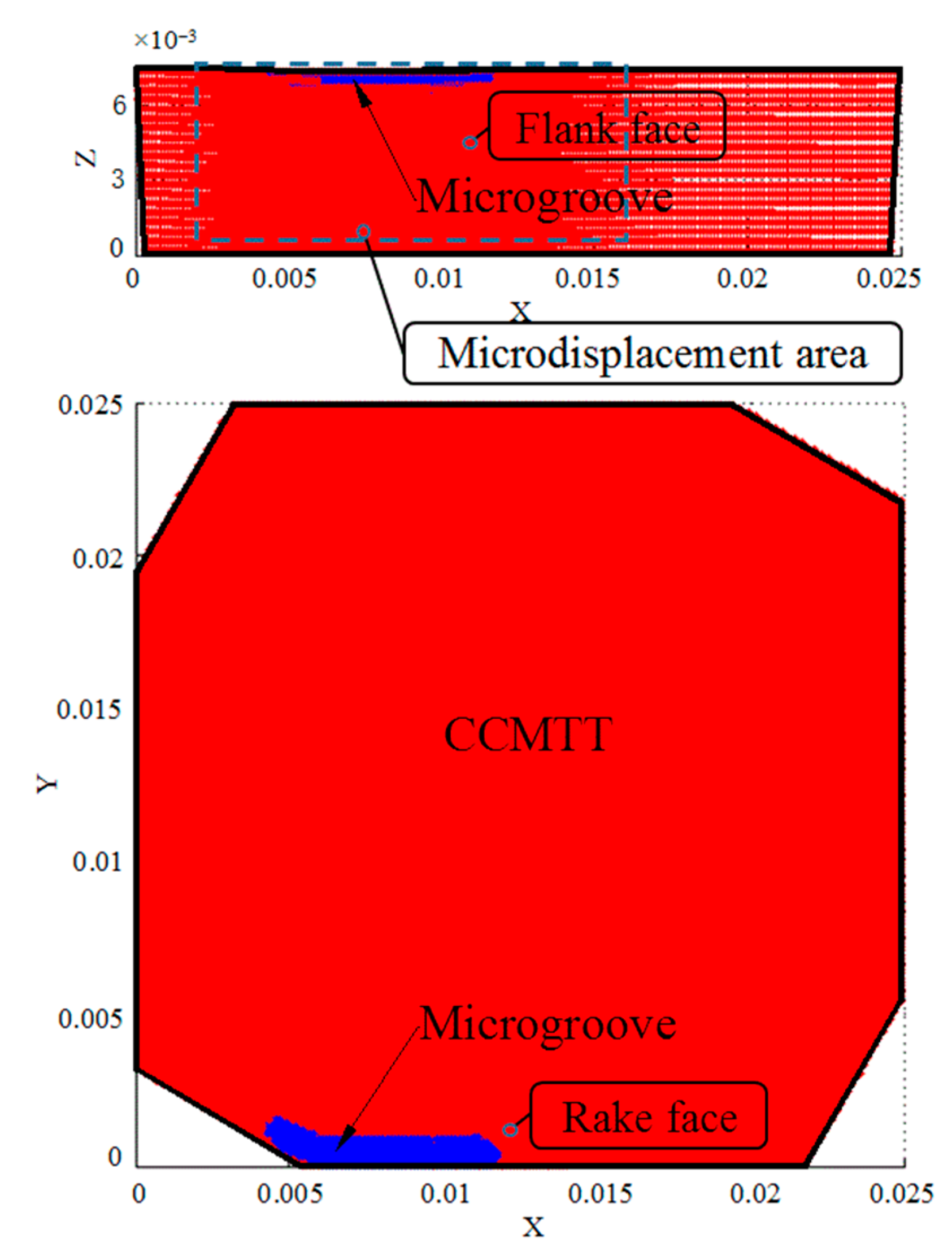
2.3. Determination of Loaded Area
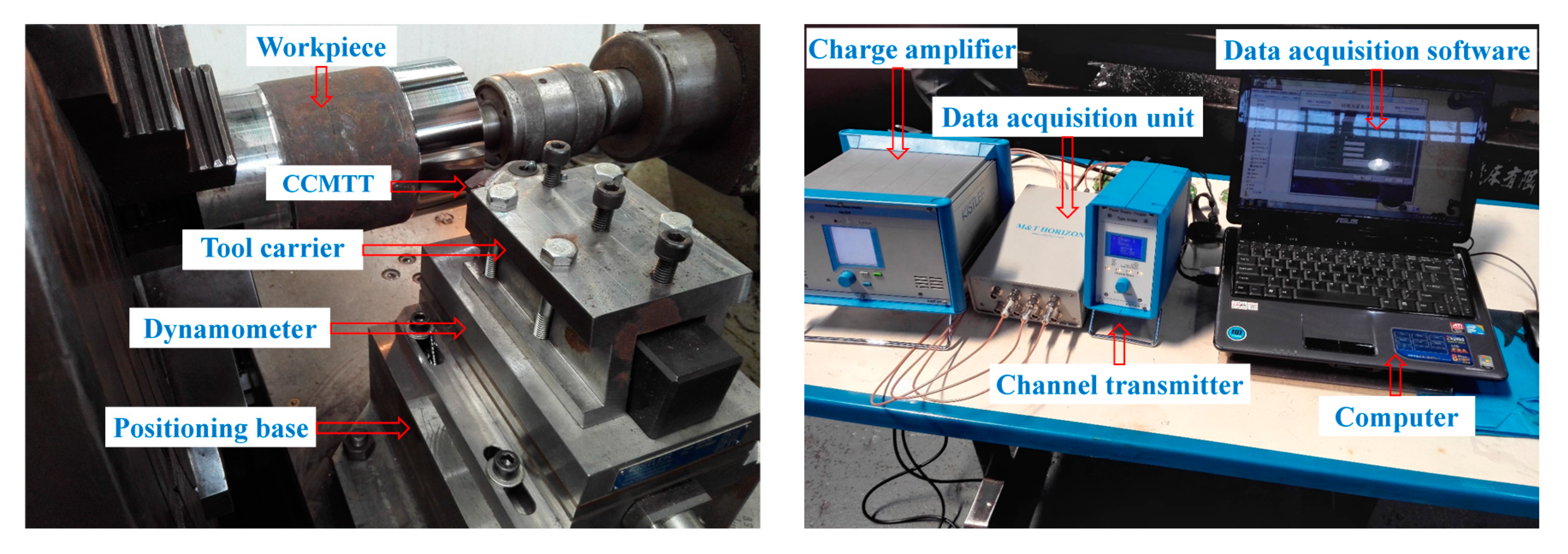
2.4. Experimental Conditions
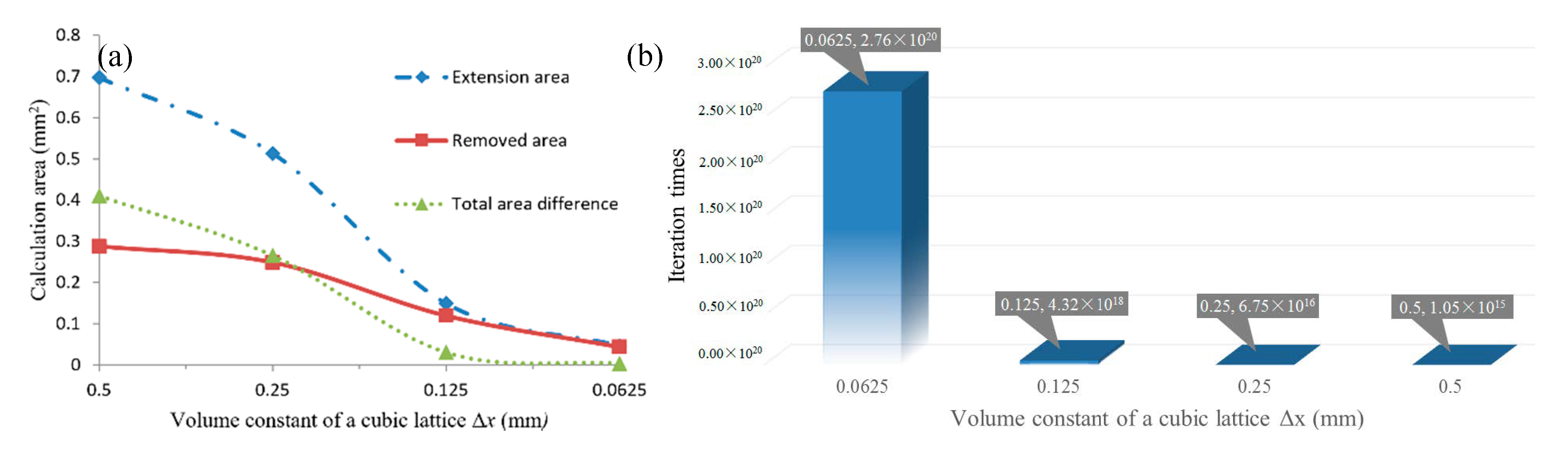
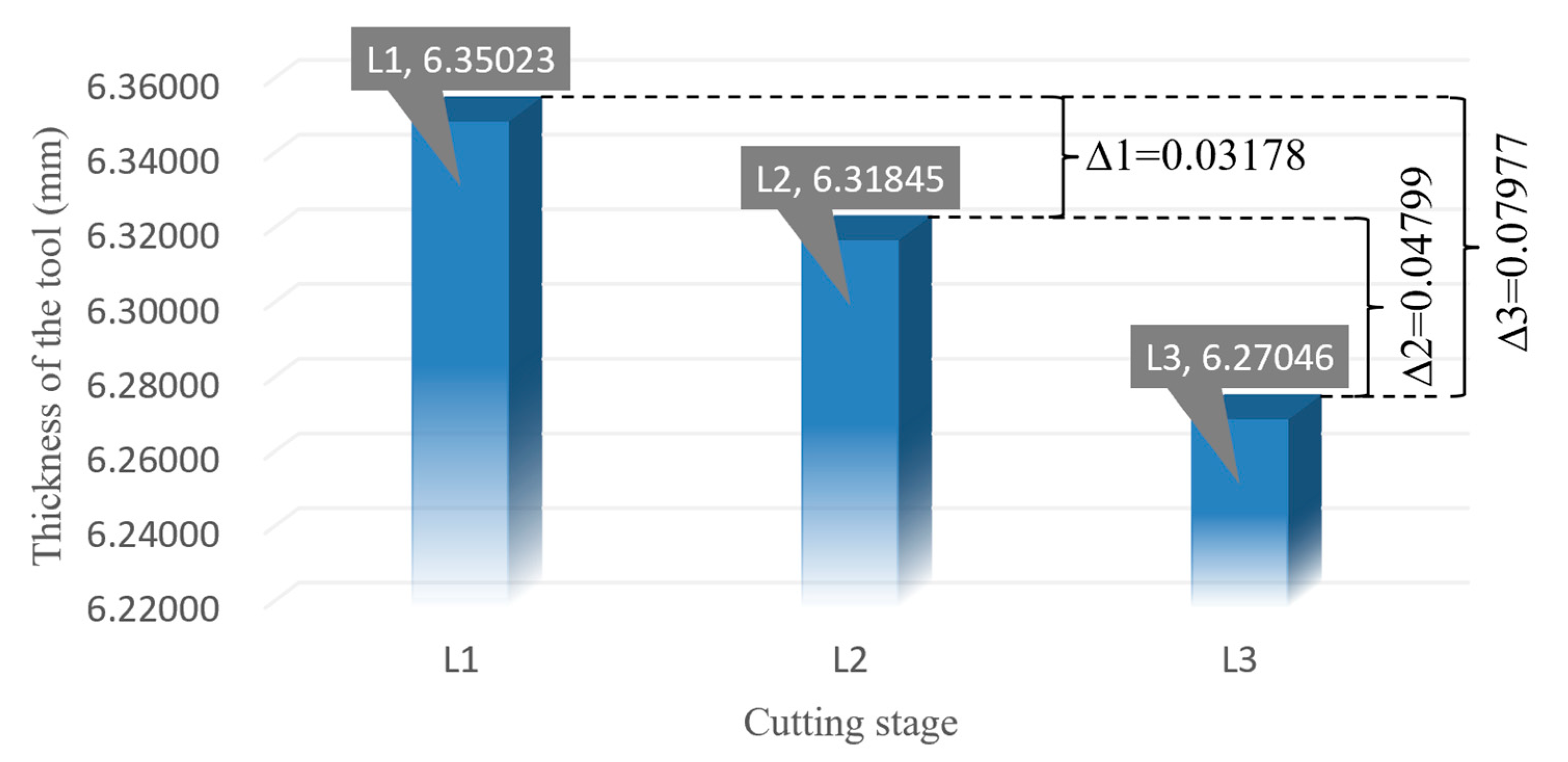
3. Analysis and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Qian, F.; Ma, Y.L.; Wang, D.L.; Dong, H.M.; Gao, Y. Research and system development of tool breakage detection in production line. Univ. Politeh. Buchar. Sci. Bull. D Mech. Eng. 2017, 79, 217–234. [Google Scholar]
- Cho, H.S.; Han, J.-H.; Chi, S.-Y.; Yoo, K.-H. A tool breakage detection system using load signals of spindle motors in CNC machines. In Proceedings of the 2016 Eighth International Conference on Ubiquitous and Future Networks (ICUFN), Vienna, Austria, 5–8 July 2016; pp. 160–163. [Google Scholar] [CrossRef]
- Buchkremer, S.; Klocke, F.; Veselovac, D. 3D FEM simulation of chip breakage in metal cutting. Int. J. Adv. Manuf. Technol. 2015, 82, 645–661. [Google Scholar] [CrossRef]
- Lee, W.K.; Ratnam, M.M.; Ahmad, Z.A. Tool breakage detection from 2D workpiece profile using vision method. Mater. Sci. Eng. 2016, 114, 012132. [Google Scholar] [CrossRef]
- Mohamed, A.S.; Stephen, C.V. Effect of cutting speed on chipping and wear of the sialon ceramic tool in dry finish turning of the precipitation hardenable in 100 aerospace superalloy. J. Tribol. 2019, 141, 021604. [Google Scholar]
- Ma, C.; Wang, Z. Experimental and numerical investigation of the breakage of a cutting tool with ultrasonic vibration. Precis. Eng. 2018, 51, 393–402. [Google Scholar] [CrossRef]
- Chen, J.G.; Zheng, M.L.; Sun, Y.S.; Zhang, W.; Li, P.F. Research on the microscopic mechanism of the bond breakage of cemented carbide tools. J. Mech. Eng. 2018, 64, 726–742. [Google Scholar]
- Cheng, Y.-N.; Nie, W.-Y.; Guan, R.; Jia, W.-K.; Yan, F.-G. Study on damage behavior of carbide tool for milling difficult-to-machine material. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 233, 735–747. [Google Scholar] [CrossRef]
- Kresse, T.; Jürgens, W.; Meinhard, D.; Baumann, W.; Bernthaler, T.; Schneider, G. Wear and Damage Characterization of Coated Carbide Tools by means of FIB-SEM Microscopy. Pr. Met. 2018, 55, 704–715. [Google Scholar] [CrossRef]
- Cui, X.; Guo, J.; Zhao, J.; Yan, Y. Analysis of PCBN tool failure mechanisms in face milling of hardened steel using damage equivalent stress. Int. J. Adv. Manuf. Technol. 2015, 80, 1985–1994. [Google Scholar] [CrossRef]
- Jiang, H.W.; He, L.; Fan, L.; Zhao, X.F.; Zhan, G. Peridynamic theory-based prediction of micro breakage of cemented carbide tool. J. South China Univ. Technol. (Nat. Sci. Ed.) 2016, 44, 8–15. [Google Scholar]
- Jiang, H.; He, L.; Fan, L.; Zhan, G. Numerical analysis method of cemented carbide turning tool’s micro breakage based on peridynamic theory. Int. J. Adv. Manuf. Technol. 2016, 88, 1619–1628. [Google Scholar] [CrossRef]
- Silling, S.A.; Weckner, O.; Askari, E.; Bobaru, F. Crack nucleation in a peridynamic solid. Int. J. Fract. 2010, 162, 219–227. [Google Scholar] [CrossRef]
- Le, Q.V.; Bobaru, F. Surface corrections for peridynamic models in elasticity and fracture. Comput. Mech. 2017, 61, 499–518. [Google Scholar] [CrossRef]
- Liu, W.; Yang, G.; Cai, Y. Modeling of failure mode switching and shear band propagation using the correspondence framework of peridynamics. Comput. Struct. 2018, 209, 150–162. [Google Scholar] [CrossRef]
- Pashazad, H.; Kharazi, M. A peridynamic plastic model based on von Mises criteria with isotropic, kinematic and mixed hardenings under cyclic loading. Int. J. Mech. Sci. 2019, 156, 182–204. [Google Scholar] [CrossRef]
- Zaccariotto, M.; Mudric, T.; Tomasi, D.; Shojaei, A.; Galvanetto, U. Coupling of FEM meshes with Peridynamic grids. Comput. Methods Appl. Mech. Eng. 2018, 330, 471–497. [Google Scholar] [CrossRef]
- Jafari, A.; Ezzati, M.; Atai, A.A. Static and free vibration analysis of Timoshenko beam based on combined peridynamic-classical theory besides FEM formulation. Comput. Struct. 2019, 213, 72–81. [Google Scholar] [CrossRef]
- Wang, Y.; Zhou, X.; Wang, Y.; Shou, Y. A 3-D conjugated bond-pair-based peridynamic formulation for initiation and propagation of cracks in brittle solids. Int. J. Solids Struct. 2018, 134, 89–115. [Google Scholar] [CrossRef]
- Zhang, G.; Gazonas, G.A.; Bobaru, F. Supershear damage propagation and sub-Rayleigh crack growth from edge-on impact: A peridynamic analysis. Int. J. Impact Eng. 2018, 113, 73–87. [Google Scholar] [CrossRef]
- Alali, B.; Gunzburger, M. Peridynamics and Material Interfaces. J. Elast. 2015, 120, 225–248. [Google Scholar] [CrossRef]
- Diana, V.; Casolo, S. A full orthotropic micropolar peridynamic formulation for linearly elastic solids. Int. J. Mech. Sci. 2019, 160, 140–155. [Google Scholar] [CrossRef]
- Shojaei, A.; Mossaiby, F.; Zaccariotto, M.; Galvanetto, U. An adaptive multi-grid peridynamic method for dynamic fracture analysis. Int. J. Mech. Sci. 2018, 144, 600–617. [Google Scholar] [CrossRef]
- Silling, S. Stability of peridynamic correspondence material models and their particle discretizations. Comput. Methods Appl. Mech. Eng. 2017, 322, 42–57. [Google Scholar] [CrossRef]
- Kovalchenko, A.M.; Goel, S.; Zakiev, I.M.; Pashchenko, E.A.; Al-Sayegh, R. Suppressing scratch-induced brittle fracture in silicon by geometric design modification of the abrasive grits. J. Mater. Res. Technol. 2018, 8, 703–712. [Google Scholar] [CrossRef]
- Garcia, J.M.; Brandao, L.P.; Costa, U.O.; Salgado, J.V.; Nunes, L.F.; Paula, A.D.S.; Monteiro, S.N. Experimental creep behavior and life prediction through observation and numerical analysis for AISI 310. J. Mater. Res. Technol. 2019, 9, 222–229. [Google Scholar] [CrossRef]
- Dabees, S.; Tirth, V.; Mohamed, A.; Kamel, B.M. Wear performance and mechanical properties of mwcnt/hdpe nanocomposites for gearing applications. J. Mater. Res. Technol. 2020, 12, 2476–2488. [Google Scholar]
- Zhang, H.; Li, H.; Ye, H.; Zheng, Y. A coupling peridynamic approach for the consolidation and dynamic analysis of saturated porous media. Comput. Mech. 2019, 64, 1097–1113. [Google Scholar] [CrossRef]
- Anbarlooie, B.; Hosseini-Toudeshky, H. Peridynamic micromechanical prediction of nonlocal damage initiation and propagation in DP steels based on real microstructure. Int. J. Mech. Sci. 2019, 153–154, 64–74. [Google Scholar] [CrossRef]
- Madenci, E.; Barut, A. Futch M Peridynamic differential operator and its applications. Comput. Methods Appl. Mech. Eng. 2016, 304, 408–451. [Google Scholar]
- Silling, S.; Littlewood, D.; Seleson, P. Variable horizon in a peridynamic medium. J. Mech. Mater. Struct. 2015, 10, 591–612. [Google Scholar] [CrossRef]
- Shojaei, A.; Mudric, T.; Zaccariotto, M.; Galvanetto, U. A coupled meshless finite point/Peridynamic method for 2D dynamic fracture analysis. Int. J. Mech. Sci. 2016, 119, 419–431. [Google Scholar] [CrossRef]
- Silling, S. Solitary waves in a peridynamic elastic solid. J. Mech. Phys. Solids 2016, 96, 121–132. [Google Scholar] [CrossRef]
- Oterkus, S.; Madenci, E. Peridynamic modeling of fuel pellet cracking. Eng. Fract. Mech. 2017, 176, 23–37. [Google Scholar] [CrossRef]
- Hu, Y.; Madenci, E. Bond-based peridynamic modeling of composite laminates with arbitrary fiber orientation and stacking sequence. Compos. Struct. 2016, 153, 139–175. [Google Scholar] [CrossRef]
- Yaghoobi, A.; Chorzepa, M.G. Fracture analysis of fiber reinforced concrete structures in the micropolar peridynamic analysis framework. Eng. Fract. Mech. 2017, 169, 238–250. [Google Scholar] [CrossRef]
- Du, Q.; Tao, Y.; Tian, X. A Peridynamic Model of Fracture Mechanics with Bond-Breaking. J. Elast. 2017, 132, 197–218. [Google Scholar] [CrossRef]
- John, T.; Xu, X. A generalized, ordinary, finite deformation constitutive correspondence model for peridynamics. Int. J. Solids Struct. 2018, 141, 245–253. [Google Scholar]
- Madenci, E.; Diyaroglu, C.; Anicode, V.; Silling, S. Peridynamic Modeling of Drilling Process in Polymer Matrix Composites. In Proceedings of the AIAA SciTech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]







| Geometric Angle | ||||||
|---|---|---|---|---|---|---|
| Value (°) | 150 | 7 | 27.5 | 2.5 | 0 | 62.5 |
| Material | Tensile Strength (MPa) | Bending Strength (MPa) | Hardness | Poisson’s Ratio | Elastic Modulus (GPa) | |
|---|---|---|---|---|---|---|
| Tool | 13.8 | 784.5 | 1180 | 91.8HRA | 0.23 | 540–600 |
| Workpiece | 7.87 | 1080 | \ | 30HRC | 0.28 | 212 |
| Cutting Force | Main Cutting Force Fc (N) | Axial Thrust Force Ff (N) | Radial Thrust Force Fp (N) | |
|---|---|---|---|---|
| Test Number | ||||
| 1 | 2104.58 | 953.21 | 1103.56 | |
| 2 | 2235.12 | 980.43 | 1086.31 | |
| 3 | 2085.75 | 897.65 | 1018.15 | |
| 4 | 2116.23 | 958.32 | 1132.46 | |
| 5 | 2201.87 | 962.54 | 1107.29 | |
| Average value | 2148.71 | 950.43 | 1089.55 | |
| Uncertainty | −9.0949E-14 | 4.55E-14 | 1.13687E-13 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Z.; Jiang, H.; Zou, Z.; Yuan, S. Simulation Prediction and Experiment of Brittle Damage of Cemented Carbide Microgroove Turning Tools Based on Peridynamics. Processes 2023, 11, 520. https://doi.org/10.3390/pr11020520
Ren Z, Jiang H, Zou Z, Yuan S. Simulation Prediction and Experiment of Brittle Damage of Cemented Carbide Microgroove Turning Tools Based on Peridynamics. Processes. 2023; 11(2):520. https://doi.org/10.3390/pr11020520
Chicago/Turabian StyleRen, Zhongwei, Hongwan Jiang, Zhongfei Zou, and Sen Yuan. 2023. "Simulation Prediction and Experiment of Brittle Damage of Cemented Carbide Microgroove Turning Tools Based on Peridynamics" Processes 11, no. 2: 520. https://doi.org/10.3390/pr11020520
APA StyleRen, Z., Jiang, H., Zou, Z., & Yuan, S. (2023). Simulation Prediction and Experiment of Brittle Damage of Cemented Carbide Microgroove Turning Tools Based on Peridynamics. Processes, 11(2), 520. https://doi.org/10.3390/pr11020520






