Analysis of Sediment and Water Flow and Erosion Characteristics of Large Pelton Turbine Injector
Abstract
:1. Introduction
2. Mathematical Model
2.1. Turbulence Model
2.2. Solid–Liquid Two-Phase Flow Model
2.3. Particle Trajectory Model
2.4. Sediment Erosion Model
3. Geometric Physical Model and Boundary Conditions
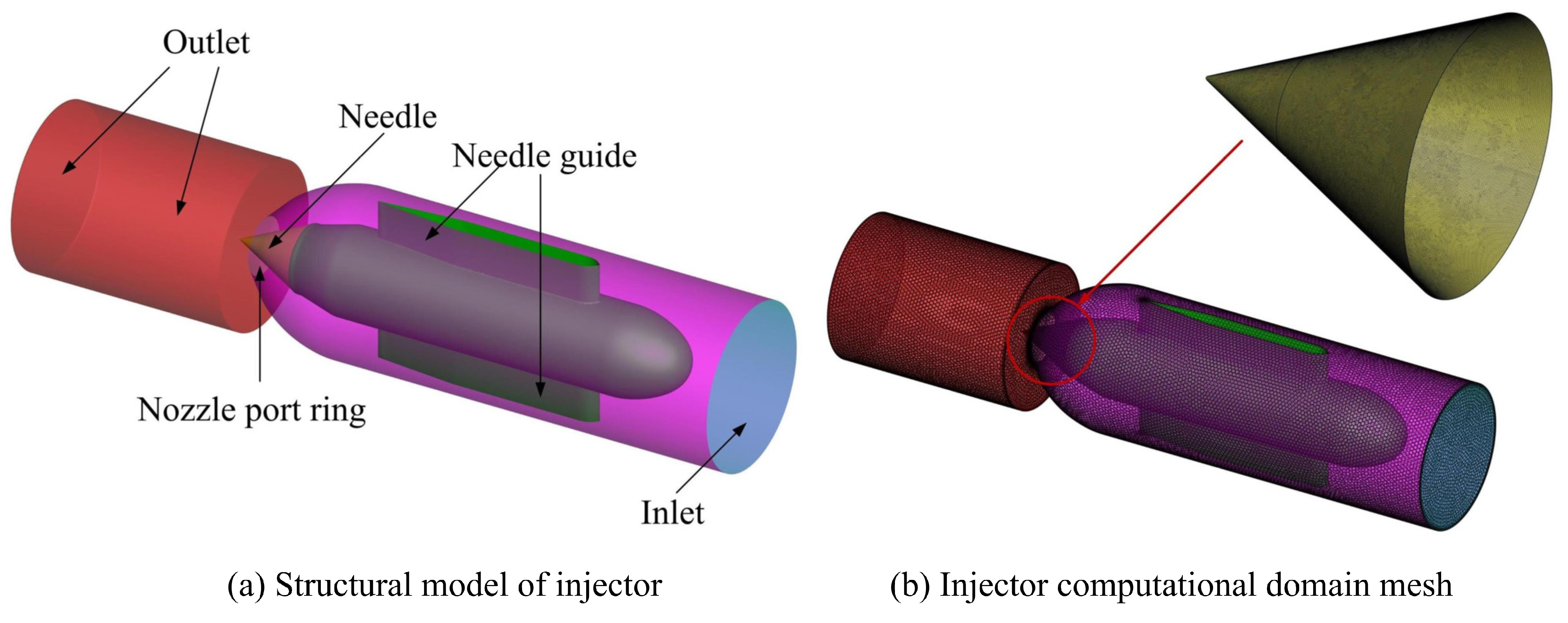
3.1. Geometric Modeling and Meshing
3.2. Boundary Conditions and Calculation Settings
4. Numerical Calculation Results and Analysis
4.1. Analysis of the Flow Field in the Injector
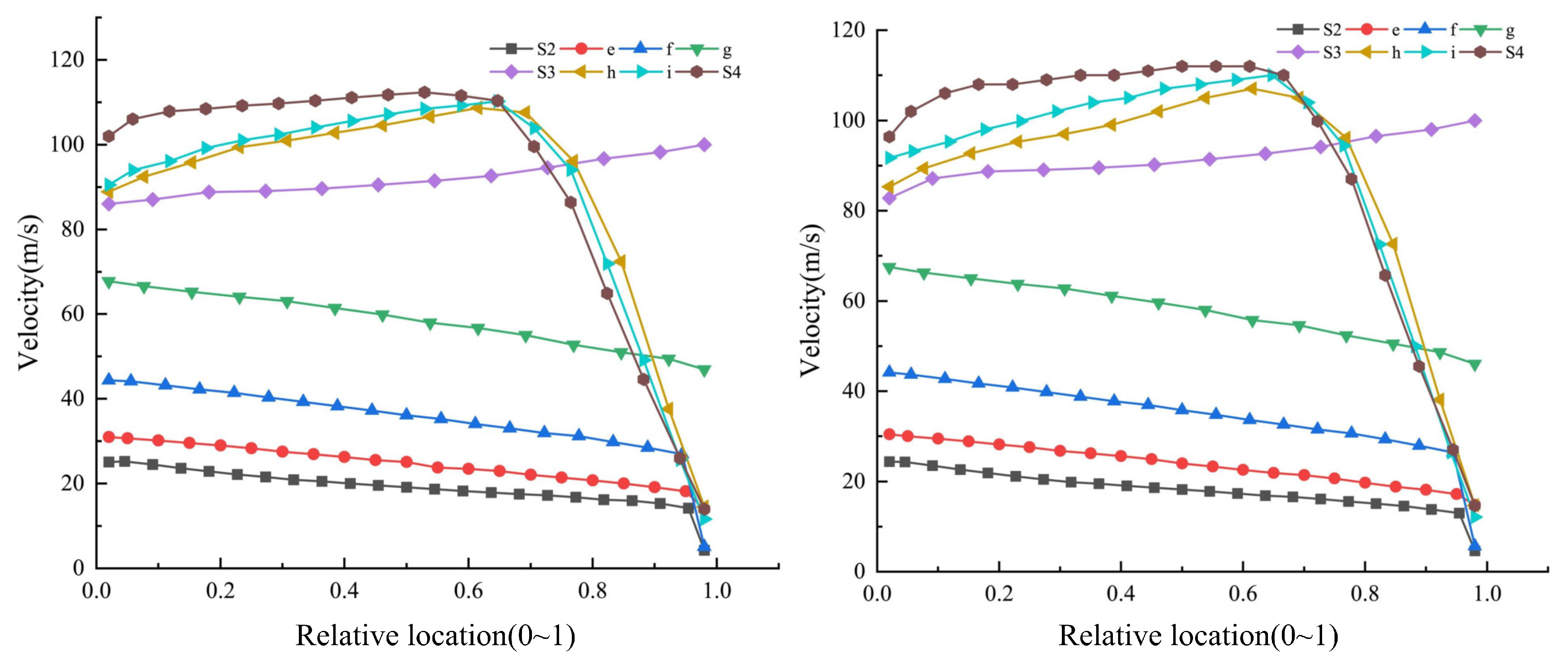
4.2. Analysis of the Velocity Deficit Phenomenon in the Injector
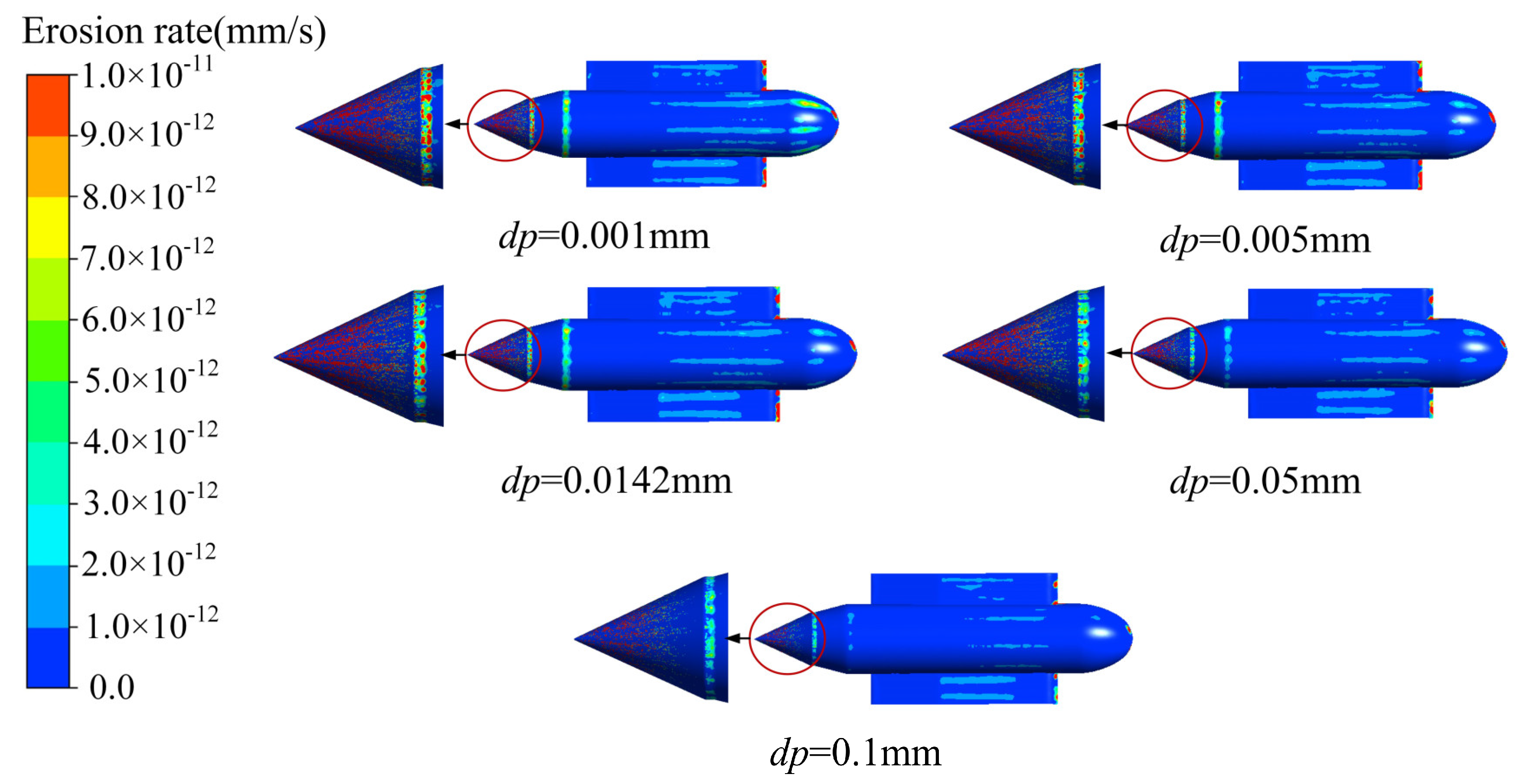
4.3. Effect of Sediment Particle Diameter on the Injector Erosion Analysis
5. Conclusions
- (1)
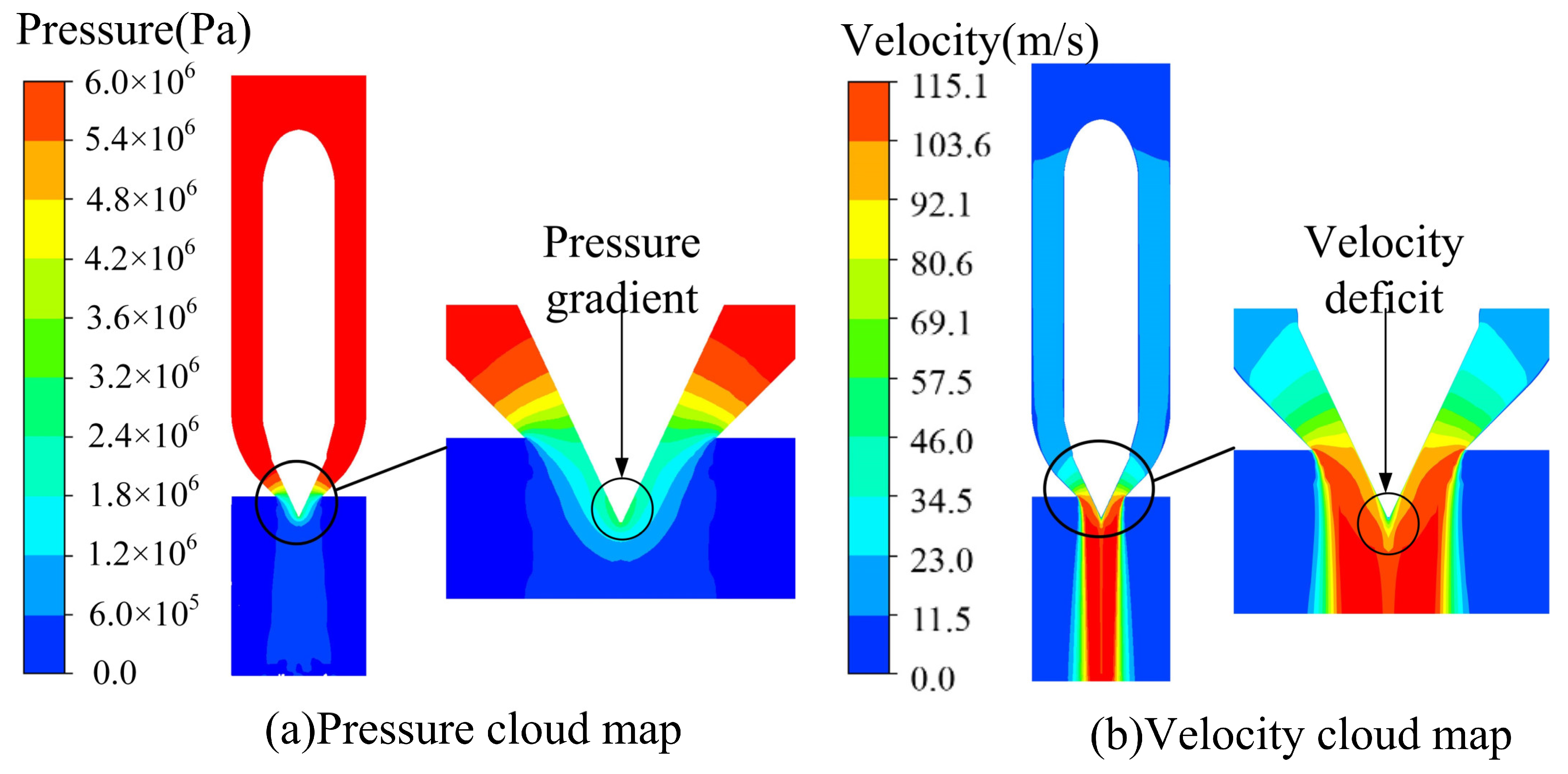
- The pressure at the nozzle outlet is minimum. The direction of the pressure gradient changes, and the velocity is maximum. The boundary layer on the surface of the injector causes a velocity deficit, which affects the velocity distribution of the jet and the quality of the jet. Water shoots out of the nozzle, and the maximum jet velocity continues to increase, before decreasing again.
- (2)
- The sediment particle diameters will affect the erosion of the needle. The smaller the particle size, the more serious the erosion of the needle rod and the head. The erosion of the lower needle guide is more serious than that of the upper needle guide. The erosion of the needle rod and needle guide is groove-shaped. The erosion of the needle is mainly point-like and exhibits asymmetrical distribution.
- (3)
- The particle size has little effect on the erosion location of the nozzle port ring; however, it has an effect on the erosion amount. The erosion of the nozzle port ring exhibits symmetrical distribution. The erosion of the nozzle port ring is greater than that of the needle with the same sediment particle diameter.
- (4)
- The sediment erosion of the power station is very serious, and anti-erosion measures are necessary.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Leguizamón, S.; Alimirzazadeh, S.; Jahanbakhsh, E.; Avellan, F. Multiscale simulation of erosive wear in a prototype-scale Pelton runner. Renew. Energy 2020, 151, 204–215. [Google Scholar] [CrossRef]
- Liu, X. Hydraulic Turbine Sand and Water Flow and Erosion; Water Conservancy and Hydropower Press: Beijing, China, 2020. [Google Scholar]
- Jung, I.H.; Kim, Y.S.; Shin, D.H.; Chung, J.T.; Shin, Y. Influence of spear needle eccentricity on jet quality in micro Pelton turbine for power generation. Energy 2019, 175, 58–65. [Google Scholar] [CrossRef]
- Din, M.Z.U.; Harmain, G.A. Assessment of erosive wear of Pelton turbine injector: Nozzle and spear combination–A study of Chenani hydro-power plant. Eng. Fail. Anal. 2020, 116, 104695. [Google Scholar] [CrossRef]
- Alomar, O.R.; Abd, H.M.; Salih, M.M.M.; Ali, F.A. Performance analysis of Pelton turbine under different operating conditions: An experimental study. Ain Shams Eng. J. 2022, 13, 101684. [Google Scholar] [CrossRef]
- Pang, J.; Liu, H.; Liu, X.; Yang, H.; Peng, Y.; Zeng, Y.; Yu, Z. Study on sediment erosion of high head Francis turbine runner in Minjiang River basin. Renew. Energy 2022, 192, 849–858. [Google Scholar] [CrossRef]
- Zhong, Q.; Lai, X.; You, Q. Study of nozzle flow characteristics of impact turbine and its large fluctuation transition process. Hydroelectr. Energy Sci. 2012, 30, 130–132. [Google Scholar]
- Zeng, C.; Xiao, Y.; Wang, Z.; Zhang, J.; Luo, Y. Numerical analysis of a Pelton bucket free surface sheet flow and dynamic performance affected by operating head. Proc. Inst. Mech. Eng. Part A J. Power Energy 2017, 231, 182–196. [Google Scholar] [CrossRef]
- Zeng, C.; Xiao, Y.; Luo, Y.; Zhang, J.; Wang, Z.; Fan, H.; Ahn, S.-H. Hydraulic performance prediction of a prototype four-nozzle Pelton turbine by entire flow path simulation. Renew. Energy 2018, 125, 270–282. [Google Scholar] [CrossRef]
- Han, L.; Zhang, G.F.; Wang, Y.; Wei, X. Investigation of erosion influence in distribution system and nozzle structure of pelton turbine. Renew. Energy 2021, 178, 1119–1128. [Google Scholar] [CrossRef]
- Han, L.; Duan, X.L.; Gong, R.Z.; Zhang, G.; Wang, H.; Wei, X. Physic of secondary flow phenomenon in distributor and bifurcation pipe of Pelton turbine. Renew. Energy 2019, 131, 159–167. [Google Scholar] [CrossRef]
- Petley, S.; Židonis, A.; Panagiotopoulos, A.; Benzon, D.; Aggidis, G.A.; Anagnostopoulos, J.S.; Papantonis, D.E. Out with the old, in with the new: Pelton hydro turbine performance influence utilizing three different injector geometries. J. Fluids Eng. 2019, 141, e08527. [Google Scholar] [CrossRef]
- Finnie, I. Erosion of surfaces by solid particle. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Grant, G.; Tabakoff, W. Erosion prediction in turbo machinery resulting from environmental solid particles. J. Aircr. 1975, 12, 471–478. [Google Scholar] [CrossRef]
- Oka, Y.I.; Okamura, K.; Yoshida, T. Practical estimation of erosion damage caused by solid particle impact. Wear 2005, 259, 95–101. [Google Scholar] [CrossRef]
- Karimi, S.; Shirazi, S.A.; Mclaury, B.S. Predicting fine particle erosion utilizing computational fluid dynamics. Wear 2017, 376, 1130–1137. [Google Scholar] [CrossRef]
- Rai, A.K.; Kumar, A.; Staubli, T. Analytical modelling and mechanism of hydro-abrasive erosion in pelton buckets. Wear 2019, 436, 203003. [Google Scholar] [CrossRef]
- Rai, A.K.; Kumar, A.; Staubli, T. Effect of concentration and size of sediments on hydro-abrasive erosion of Pelton turbine. Renew. Energy 2020, 145, 893–902. [Google Scholar] [CrossRef]
- Guo, B.; Xiao, Y.; Rai, A.K.; Zhang, J.; Liang, Q. Sediment-laden flow and erosion modeling in a Pelton turbine injector. Renew. Energy 2020, 162, 30–42. [Google Scholar] [CrossRef]
- Guo, B.; Xiao, Y.; Rai, A.K.; Liang, Q.; Liu, J. Analysis of the air-water-sediment flow behavior in Pelton buckets using a Eulerian-Lagrangian approach. Energy 2021, 218, 119522. [Google Scholar] [CrossRef]
- Xiao, Y.; Guo, B.; Rai, A.K.; Liu, J.; Liang, Q.; Zhang, J. Analysis of hydro-abrasive erosion in Pelton buckets using a Eulerian-Lagrangian approach. Renew. Energy 2022, 197, 472–485. [Google Scholar] [CrossRef]
- Ge, X.; Sun, J.; Li, Y.; Zhang, L.; Deng, C.; Wang, J. Erosion Characteristics of Sediment Diameter and Concentration on the Runner of Pelton Turbines. Proc. CSEE 2021, 197, 5025–5033. [Google Scholar]
- Ge, X.; Sun, J.; Zhou, Y.; Cai, J.; Zhang, H.; Zhang, L.; Ding, M.; Deng, C.; Binama, M.; Zheng, Y. Experimental and numerical studies on opening and velocity influence on sediment erosion of Pelton turbine buckets. Renew. Energy 2021, 173, 1040–1056. [Google Scholar] [CrossRef]
- Ge, X.; Sun, J.; Li, Y.; Wu, D.; Zhang, L.; Hua, H. Numerical simulation of sediment wear characteristics of an impact turbine injector. J. Water Resour. 2020, 51, 1486–1494. [Google Scholar]
- Pang, J.; Liu, H.; Liu, X.; Ren, M.; Zhang, P.; Yu, Z. Analysis on the escape phenomenon of oil mist from turbine lower guide bearing based on VOF model. Adv. Mech. Eng. 2021, 13. [Google Scholar] [CrossRef]
- Pang, J.; Zhang, H.; Yang, J.; Chen, Y.; Liu, X.B. Numerical and experimental study on sediment erosion of Francis turbine runner for hydropower stations. Chin. J. Hydrodyn. 2020, 35, 436–443. [Google Scholar]
- Bajracharya, T.R.; Acharya, B.; Joshi, C.B.; Saini, R.; Dahlhaug, O.G. Sand erosion of Pelton turbine nozzles and buckets: A case study of Chilime Hydropower Plant. Wear 2008, 264, 177–184. [Google Scholar] [CrossRef]
- Zhang, H. Pelton Turbine; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Tarodiya, R.; Khullar, S.; Levy, A. Particulate flow and erosion modeling of a Pelton turbine injector using CFD-DEM simulations. Powder Technol. 2022, 399, 117168. [Google Scholar] [CrossRef]
- Tarodiya, R.; Khullar, S.; Levy, A. Assessment of erosive wear performance of Pelton turbine injectors using CFD-DEM simulations. Powder Technol. 2022, 408, 117763. [Google Scholar] [CrossRef]




| Point | Angle | Value |
|---|---|---|
| 1 | 0 | 0 |
| 2 | 20 | 0.8 |
| 3 | 30 | 1 |
| 4 | 45 | 0.5 |
| 5 | 90 | 0.4 |
| Name | Nozzle Inlet Diameter/mm | Nozzle Outlet Diameter/mm | Needle Stroke/mm | Number of Needle Guide/Number |
|---|---|---|---|---|
| Parameter | 1502 | 518 | 316.3 | 2 |
| Option | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
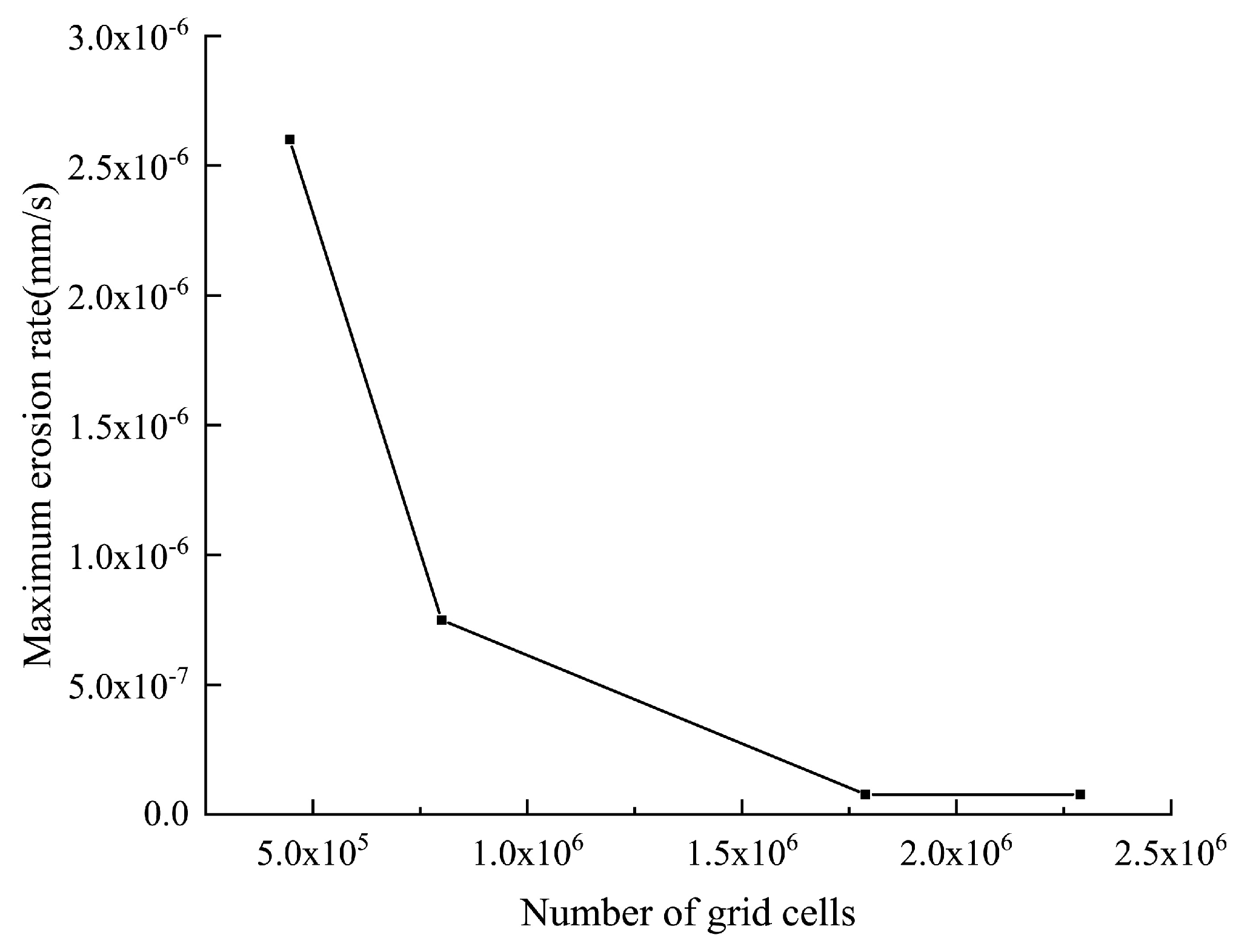
| Numbers of grid cells | 445,814 | 800,121 | 1,788,149 | 2,288,892 |
| Sediment Particle Diameter dp (mm) | Needle Maximum Erosion Rate Ren (mm/s) | Nozzle Maximum Erosion Rate Res (mm/s) |
|---|---|---|
| 0.001 | 3.243 × 10−8 | 7.718 × 10−8 |
| 0.005 | 1.57 × 10−8 | 4.454 × 10−8 |
| 0.0142 | 7.701 × 10−9 | 2.432 × 10−8 |
| 0.05 | 4.761 × 10−9 | 1.064 × 10−8 |
| 0.1 | 2.832 × 10−9 | 9.837 × 10−9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Pang, J.; Liu, X.; Huang, Y.; Deng, H. Analysis of Sediment and Water Flow and Erosion Characteristics of Large Pelton Turbine Injector. Processes 2023, 11, 1011. https://doi.org/10.3390/pr11041011
Liu J, Pang J, Liu X, Huang Y, Deng H. Analysis of Sediment and Water Flow and Erosion Characteristics of Large Pelton Turbine Injector. Processes. 2023; 11(4):1011. https://doi.org/10.3390/pr11041011
Chicago/Turabian StyleLiu, Jitao, Jiayang Pang, Xiaobing Liu, Yu Huang, and Huiming Deng. 2023. "Analysis of Sediment and Water Flow and Erosion Characteristics of Large Pelton Turbine Injector" Processes 11, no. 4: 1011. https://doi.org/10.3390/pr11041011
APA StyleLiu, J., Pang, J., Liu, X., Huang, Y., & Deng, H. (2023). Analysis of Sediment and Water Flow and Erosion Characteristics of Large Pelton Turbine Injector. Processes, 11(4), 1011. https://doi.org/10.3390/pr11041011





