Review of the Research on and Optimization of the Flow Force of Hydraulic Spool Valves
Abstract
1. Introduction
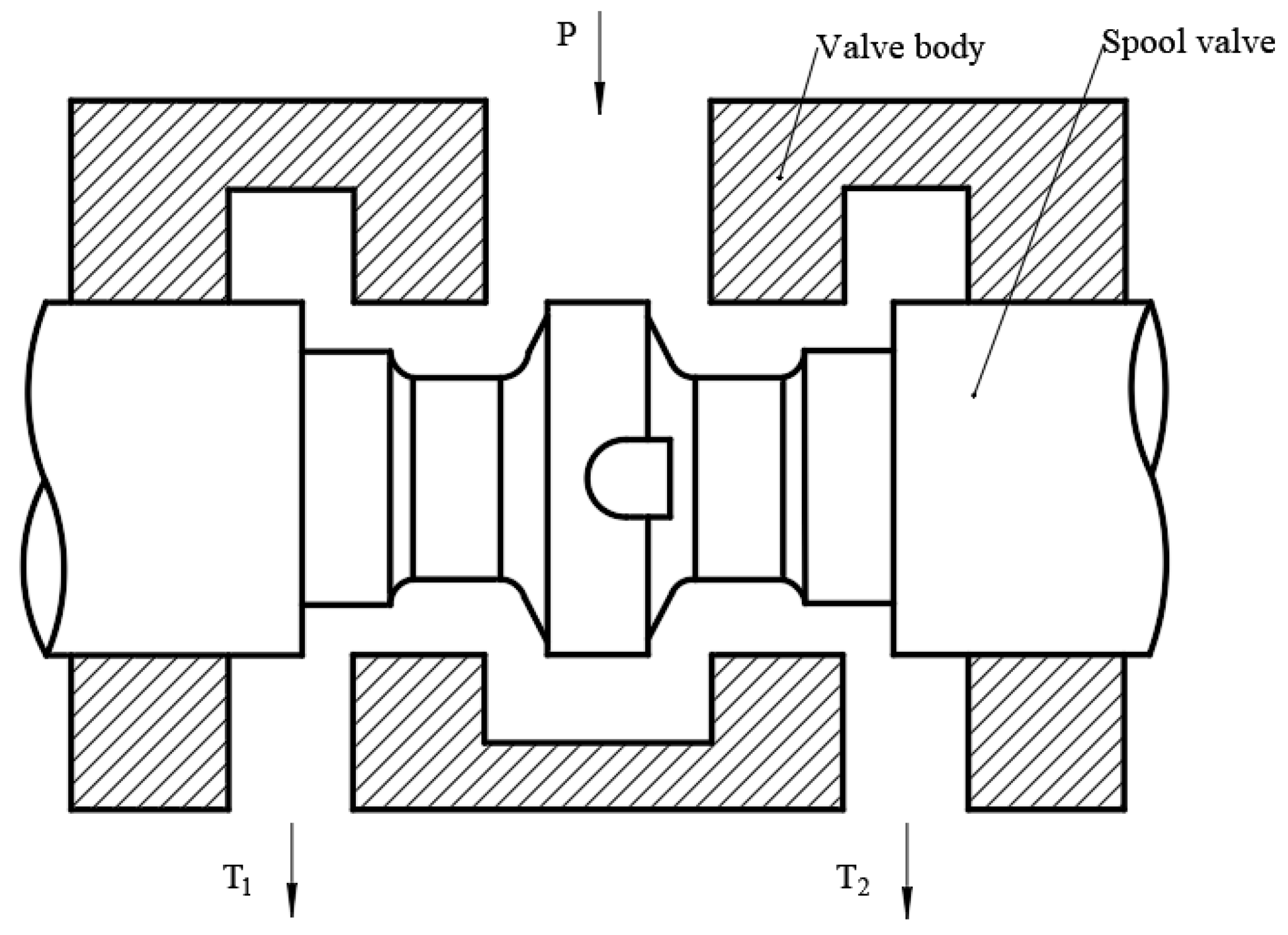
2. Hydrodynamic Analysis
2.1. Steady-State Flow Force
2.1.1. Axial Flow Force
2.1.2. Radial Flow Force
2.2. Transient Flow Force
3. Factors Affecting Flow Force
3.1. Cavitation Phenomenon
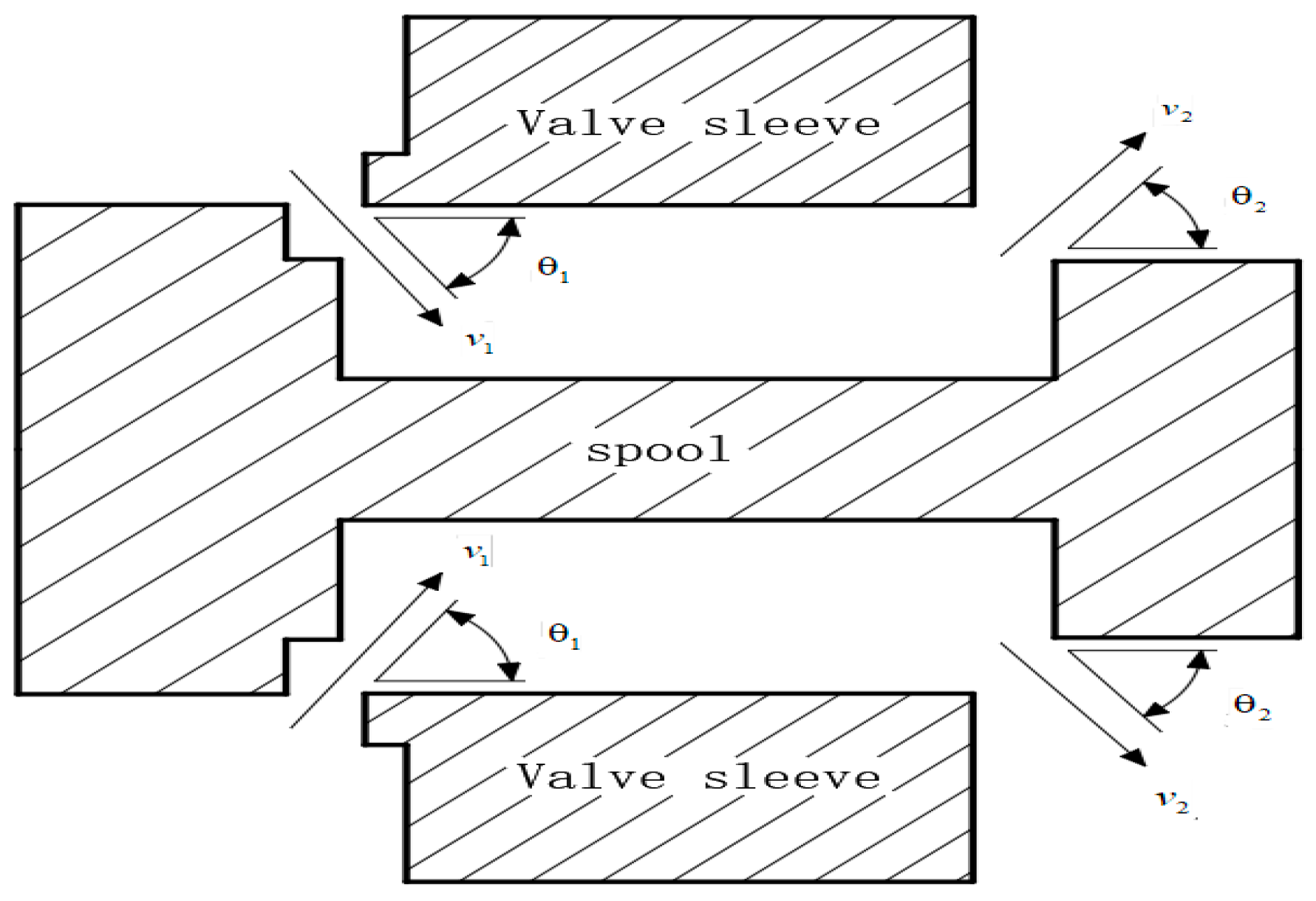
3.2. Jet Angle
3.3. Flow Rate
4. Methods for Reducing Flow Force
4.1. Axial Steady-State Flow Force
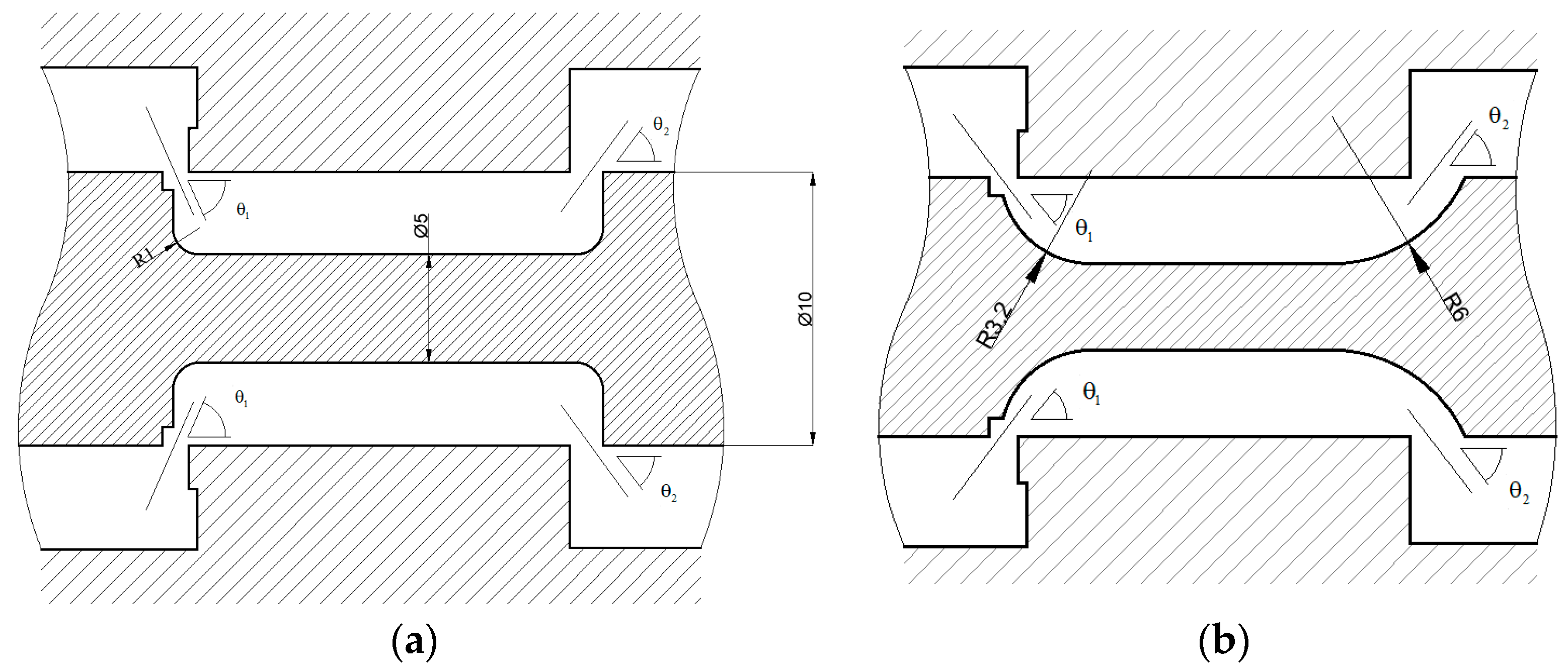
4.1.1. Structural Design of Spool Valves
4.1.2. Structural Design of Valve Sleeves
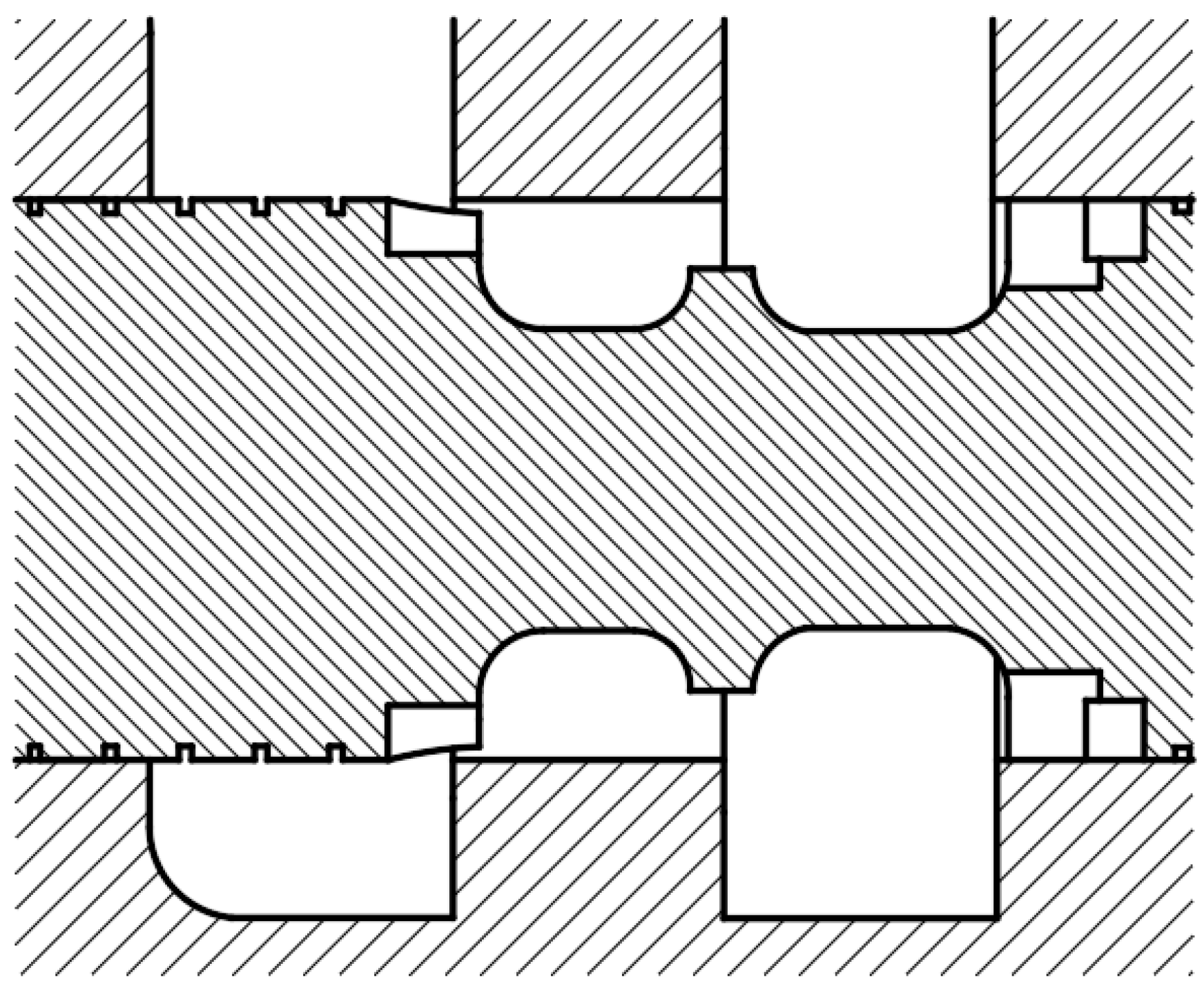
4.1.3. Design of Flow Channels for Valve Bodies
4.2. Radial Flow Force
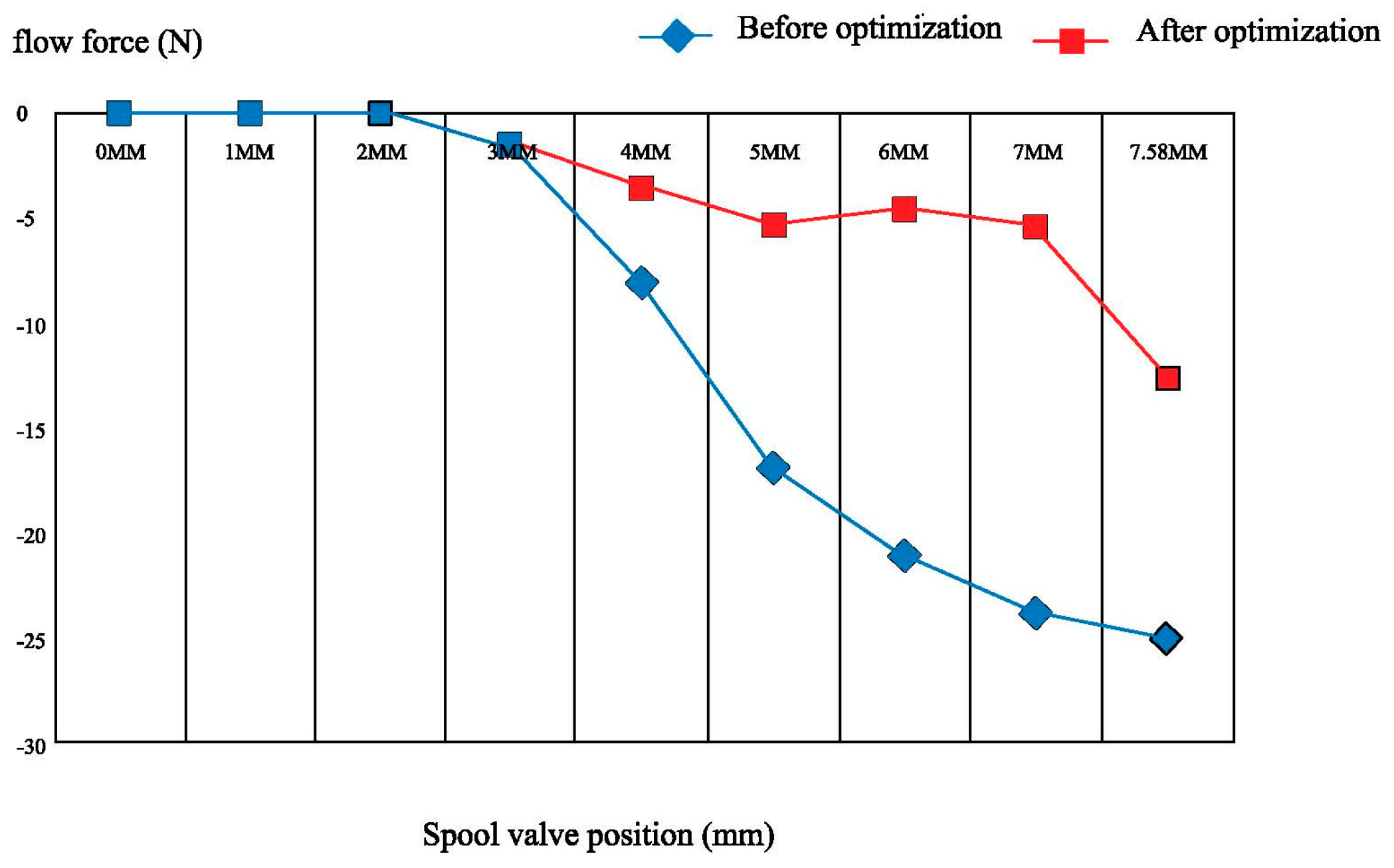
4.3. Transient Flow Force
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Altare, G.; Rundo, M.; Olivetti, M. 3D dynamic simulation of a flow force compensated pressure relief valve. In Proceedings of the ASME 2016 International Mechanical Engineering Congress and Exposition, Phoenix, AZ, USA, 11–17 November 2016. [Google Scholar]
- Wang, B.; Liu, H.; Hao, Y.X.; Quan, L.; Li, Y.W.; Zhao, B. Design and Analysis of a Flow-Control Valve With Controllable Pressure Compensation Capability for Mobile Machinery. IEEE Access 2021, 9, 98361–98368. [Google Scholar] [CrossRef]
- Lü, X.B.; Peng, J.H.; Li, S.J. Dynamic characteristics of the jet force on the flapper of the pilot stage in a flapper nozzle servo valve under the flow-solid interaction. J. Beijing Inst. Technol. 2020, 29, 445–455. [Google Scholar]
- Amirante, R.; Del, V.G.; Lippolis, A. Evaluation of the flow forces on an open centre directional control valve by means of a computational fluid dynamic analysis. Energy Convers. Manag. 2006, 47, 1748–1760. [Google Scholar] [CrossRef]
- Lee, G.S.; Sung, H.J.; Kim, H.C.; Lee, H.W. Flow force analysis of a variable force solenoid valve for automatic transmissions. J. Fluids Eng. 2010, 132, 031103. [Google Scholar] [CrossRef]
- Fan, Y.L.; Lei, Y.; Zhang, J.W.; Wang, J.; Li, Z. Flow Force of Pressure Independent Control Valve with Different Valve Core Structural. J. Phys. Conf. Ser. 2020, 1634, 012149. [Google Scholar] [CrossRef]
- Xue, M.; Lin, H.L.; Zheng, X.L. Influence and Improvement of Hydraulic Power on Spool Valve Reversing. In Proceedings of the 2021 International Conference on Power Grid System and Green Energy (PGSGE 2021), Wuhan, China, 26–28 March 2021; Volume 252. [Google Scholar]
- Finesso, R.; Rundo, M. Numerical and experimental investigation on a conical poppet relief valve with flow force compensation. Int. J. Fluid Power 2017, 18, 111–122. [Google Scholar] [CrossRef]
- Simic, M.; Herakovic, N. Reduction of the flow forces in a small hydraulic seat valve as alternative approach to improve the valve characteristics. Energy Convers. Manag. 2015, 89, 708–718. [Google Scholar] [CrossRef]
- Feng, G.C. Analysis and Calculation of the Orifice Area of Spool with L+U Type Notches. Mach. Tool Hydraul. 2018, 46, 62+63–65. [Google Scholar]
- Rannow, M.B.; Li, P.Y. Soft switching approach to reducing transition losses in an on/off hydraulic valve. In Proceedings of the ASME 2009 Dynamic Systems and Control Conference, Hollywood, CA, USA, 12–14 October 2009; Volume 48920, pp. 613–620. [Google Scholar]
- Tan, L.; Xie, H.; Chen, H.; Yang, H. Structure optimization of conical spool and flow force compensation in a diverged flow cartridge proportional valve. Flow Meas. Instrum. 2019, 66, 170–181. [Google Scholar] [CrossRef]
- Amirante, R.; Andrea, C.L.; Tamburrano, P. The importance of a full 3D fluid dynamic analysis to evaluate the flow forces in a hydraulic directional proportional valve. Eng. Comput. 2014, 31, 898–922. [Google Scholar] [CrossRef]
- Jiang, Y.; Xu, J.M. Simulation research on dynamic performance of cartridge two position four way directional valve. Mach. Tool Hydraul. 2021, 49, 133–135. [Google Scholar]
- He, X.L. Analysis of Internal Energy Consumption Mechanism of Single Section U-Groove Spool Valve. Master’s Thesis, Lanzhou University of Technology, Lanzhou, China, 2017. [Google Scholar]
- Zhang, Y.J. Compensation of Steady State Flow Forces in Slide Valves. Heilongjiang Keji Daxue Xuebao 2018, 18, 51–55. [Google Scholar]
- Lu, J.M.; Zhou, D.J.; Feng, Z.J.; Liu, H. Calculation and Analysis of Flow Area and Hydraulic Diameter for Typical Hydraulic Notches. Hydraul. Pneum. Seals 2016, 36, 44–46. [Google Scholar]
- Zhang, H.P. Correction for Some Wrong Opinions about Flow Forces. Hydraul. Pneum. Seals 2010, 30, 10–15. [Google Scholar]
- Hu, Z.L. Study on Characteristics and Optimization of Hydraulic Solenoid Valve. Master’s Thesis, Shanghai Jiaotong University, Shanghai, China, 2015. [Google Scholar]
- Wang, H.; Jia, W.H.; Zhu, Y.R.; Sun, C.L.; Wang, C. Dynamic Characteristics and Stability Analysis of Poppet Relief Valve. Mach. Tool Hydraul. 2019, 47, 144–147. [Google Scholar]
- Shimizu, F.; Tanaka, K. Generation Mechanism of Flow Force Acting on Spool Valve. JFPS Int. J. Fluid Power Syst. 2022, 15, 71–77. [Google Scholar] [CrossRef]
- Amirante, R.; Moscatelli, P.G.; Catalano, L.A. Evaluation of the flow forces on a direct (single stage) proportional valve by means of a computational fluid dynamic analysis. Energy Convers. Manag. 2007, 48, 942–953. [Google Scholar] [CrossRef]
- Amirante, R.; Catalano, L.A.; Poloni, C.; Tamburrano, P. Fluid-dynamic design optimization of hydraulic proportional directional valves. Eng. Optim. 2014, 46, 1295–1314. [Google Scholar] [CrossRef]
- Amirante, R.; Distaso, E.; Tamburrano, P. Sliding spool design for reducing the actuation forces in direct operated proportional directional valves: Experimental validation. Energy Convers. Manag. 2016, 119, 399–410. [Google Scholar] [CrossRef]
- Li, S.N.; Ge, S.H.; Wang, Y.M.; Shi, W.X. Study and Application of a New Method for the Compensation of Steady State Flow Forces in Slide Valves. J. Xi’an Jiaotong Univ. 1986, 5, 63–70. [Google Scholar]
- Tang, Z.Y.; Cao, B.G.; Shi, W.X. Discussion on Steady State Hydraulic Power Compensation Method for Hydraulic Control Valves—Valve Sleeve Motion Method. Mach. Tool Hydraul. 1995, 2, 91–95. [Google Scholar]
- Li, Z.T. Design and Optimization Study of 6 Diameter Intrinsically Safe Directional Solenoid Valve. Master’s Thesis, Zhejiang University, Zhejiang, China, 2022. [Google Scholar]
- Lisowski, E.; Czyżycki, W.; Rajda, J. Three dimensional CFD analysis and experimental test of flow force acting on the spool of solenoid operated directional control valve. Energy Convers. Manag. 2013, 70, 220–229. [Google Scholar] [CrossRef]
- Huang, H.; Li, X.; Sun, Z.W. Steady hydrodynamic force analysis of non-circular opening hydraulic spool valve. Appl. Mech. Mater. 2012, 220, 1657–1660. [Google Scholar] [CrossRef]
- Liu, J.; Li, R.; Ding, X.; Liu, Q. Flow force research and structure improvement of cartridge valve core based on CFD method. Heliyon 2022, 8, e11700. [Google Scholar] [CrossRef]
- Borghi, M.; Milani, M.; Paoluzzi, R. Stationary axial flow force analysis on compensated spool valves. Int. J. Fluid Power 2000, 1, 17–25. [Google Scholar] [CrossRef]
- Pan, X.D.; Wang, G.L.; Lu, Z.S. Flow field simulation and a flow model of servo-valve spool valve orifice. Energy Convers. Manag. 2011, 52, 3249–3256. [Google Scholar] [CrossRef]
- Shi, W.X.; Li, S.N.; Ge, S.H. A new technique for steady state flow force compensation in spool valves. Proc. Inst. Mech. Eng. Part E 1990, 204, 7–14. [Google Scholar]
- Wang, X.K. Radial Steady—State Flow Force of Hydraulic Directional Valve. Chin. Hydraul. Pneum. 2020, 12, 114–117. [Google Scholar]
- Zheng, C.S.; Fan, J.H.; Du, Q.; You, Y.S.; Liu, Z.Q.; Gao, Z. Study on Characteristics of Flow Field and Steady Flow Force of Hydraulic Spool Valve Based on CFD. Mach. Tool Hydraul. 2017, 45, 145–151. [Google Scholar]
- Lu, Q.Q.; Wan, J.; Li, S. Research on Radial Forces for Hydraulic Slide Valves Caused by Bernoulli Effect. China Mech. Eng. 2017, 28, 2332–2338. [Google Scholar]
- Chen, Q.-P.; Ji, H.; Xing, H.-H.; Zhao, H.-K. Experimental study on thermal deformation and clamping force characteristics of hydraulic spool valve. Eng. Fail. Anal. 2021, 129, 105698. [Google Scholar] [CrossRef]
- Chen, Q.P. Experimental Study on the Micro Mechanism of Thermal-Particle Coupling Clamping of Hydraulic Spool Valve. Doctoral Thesis, Lanzhou University of Technology, Lanzhou, China, 2021. [Google Scholar]
- Merritt, H.E. Hydraulic Control Systems; Wiley: New York, NY, USA, 1967; pp. 76–118. [Google Scholar]
- Nakada, T.; Ikebe, Y. Measurement of the unsteady axial flow force on a spool valve. In Proceedings of the IFAC Symposium, Warsaw, Poland, 20–23 May 1980; pp. 193–198. [Google Scholar]
- Vescovo, D.G.; Lippolis, A. A review analysis of unsteady forces in hydraulic valves. Int. J. Fluid Power 2006, 7, 29–39. [Google Scholar] [CrossRef]
- Manring, N.D.; Zhang, S. Pressure Transient Flow Forces for Hydraulic Spool Valves. J. Dyn. Syst. Meas. Control 2012, 134, 034501. [Google Scholar] [CrossRef]
- Guo, X.E.; Zhao, J.L. The influence of transient hydraulic force on the safe operation of electromagnetic directional valves in hydraulic systems. Min. Saf. Environ. Prot. 2002, 5, 27–29. [Google Scholar]
- Yin, Y.C.; Gao, Y. The influence of transient hydraulic force on the safe operation of electromagnetic directional valve. Coal 2004, 3, 50–52. [Google Scholar]
- Lu, L.; Wang, J.; Ling, Y.Y.; Li, H.X. Numerical Simulation Analysis of Vortex Cavitation in Spool Valve with V-shape Notch. Chin. Hydraul. Pneum. 2022, 46, 110–118. [Google Scholar]
- Han, M.X.; Liu, Y.S.; Wu, D.F.; Tan, H.J. Flow force reduction in large flow cartridge valve by optimizing geometric parameters of orifice. J. Huazhong Univ. Sci. Technol. 2018, 46, 65–68. [Google Scholar]
- Aung, N.Z.; Li, S. A numerical study of cavitation phenomenon in a flapper-nozzle pilot stage of an electrohydraulic servo-valve with an innovative flapper shape. Energy Convers. Manag. 2014, 77, 31–39. [Google Scholar] [CrossRef]
- Chen, Q.; Stoffel, B. CFD simulation of a hydraulic servo valve with turbulent flow and cavitation. In Proceedings of the ASME/JSME 2004 Pressure Vessels and Piping Conference, San Diego, CA, USA, 25–29 July 2004; Volume 46865, pp. 197–203. [Google Scholar]
- Ma, L.; Yan, H.; Ren, Y.; Li, L.; Cai, C. Numerical Investigation of Flow Force and Cavitation Phenomenon in the Pilot Stage of Electrical-Hydraulic Servo Valve under Temperature Shock. Machines 2022, 10, 423. [Google Scholar] [CrossRef]
- Aung, N.Z.; Peng, J.H.; Li, S.J. Reducing the steady flow force acting on the spool by using a simple jet-guiding groove. In Proceedings of the IEEE 2015 International Conference on Fluid Power and Mechatronics (FPM), Harbin, China, 5–7 August 2015; pp. 289–294. [Google Scholar]
- Bai, J.; Zhao, B.; Hao, Y.X.; Wang, B.; Liu, H. Hydrodynamic compensation method of proportional valve based on valve spool structure optimization. J. Mech. Electr. Eng. 2021, 38, 1444–1450. [Google Scholar]
- Zhang, Z.H.; Su, Q.; Li, H.B.; Fang, Z.F.; Chen, D.D.; Wei, Y.H. Optimized Design of Diversion Structure for Low Hydraulic Valve Core of Proportional Servo Valve. Flight Control Detect. 2022, 5, 8–15. [Google Scholar]
- Qu, D.H.; Zhou, Y.S.; Luo, W.; Liu, Y.F.; Fu, B. Influences of Fit Clearances on Steady-state Fluid Forces of Relief Valves. China Mech. Eng. 2018, 29, 893–899. [Google Scholar]
- Shi, J.Y.; Fan, F.H.; Zhang, K.C.; Li, H. Simulation Analysis of Steady Flow Force for Hydraulic Spool Valve Double Triangle Slot Valve Port Based on CFD. Hydraul. Pneum. Seals 2022, 42, 36–38. [Google Scholar]
- Gao, H.; Li, B.; Yang, G. Study on the influence of flow force on a large flowrate directional control valve. IFAC Proc. Vol. 2013, 46, 469–477. [Google Scholar] [CrossRef]
- Zhang, H.; Guan, T.Y.; Zhou, S.C.; Zhang, R.X.; Zhang, R.Z. Steady Flow Force Simulation Analysis of Large Flow Multi-way Valve. Chin. Hydraul. Pneum. 2020, 7, 132–137. [Google Scholar]
- Deng, B.; Qiu, F.C.; Wu, W.H.; Ke, J.; Wang, G.Z. Influence of Steady-state Flow Force on Operating Force of Multi-way Valve Spool. Mach. Tool Hydraul. 2017, 45, 68–71. [Google Scholar]
- Yang, T.; Nie, W.L.; Xia, S.Q. Steady-state Hydrodynamic Force Analysis on Combination Rectangular Notch Orifice of Multiple Directional Valve. Hydraul. Pneum. Seals 2016, 36, 34–37. [Google Scholar]
- Ji, H.; Fu, X.; Yang, H.Y. Study on Steady Flow Force of Non-circular Opeing Spool Valve. J. Mech. Eng. 2003, 6, 13–17. [Google Scholar] [CrossRef]
- Ye, Y.; Yin, C.-B.; Li, X.-D.; Zhou, W.-J.; Yuan, F.-F. Effects of groove shape of notch on the flow characteristics of spool valve. Energy Convers. Manag. 2014, 86, 1091–1101. [Google Scholar] [CrossRef]
- Yao, J.Y. Simulation of Spool Valve with Notches Based on Fluent. Master’s Thesis, Yanshan University, Qinhuangdao, China, 2014. [Google Scholar]
- Frosina, E.; Marinaro, G.; Senatore, A.; Pavanetto, M. Numerical and experimental investigation for the design of a directional spool valve. Energy Procedia 2018, 148, 274–280. [Google Scholar] [CrossRef]
- Qu, D.; Zhou, Y.; Liu, Y.; Luo, W.; Zhang, F. Steady flow force compensation and test research on electrohydraulic proportional relief valve. IEEE Access 2019, 7, 48087–48097. [Google Scholar] [CrossRef]
- Chen, Q.P. Characteristic Study and Modification Method for Steady-State Flow Force of Open Center Multi-Way Valve. Master’s Thesis, Lanzhou University of Technology, Lanzhou, China, 2017. [Google Scholar]
- Zhang, S.F.; Zhang, S.; Wang, Q.; He, X.H.; Xu, L. Transient Properties of Hydraulic Operated Directional Valve Based on Fluid-structure Interaction. J. Ordnance Equip. Eng. 2017, 38, 151–155. [Google Scholar]
- Guo, B.; Xie, N.; Guo, J.J.; Liu, J. Research on Flow Force of the Spool Valve and Structure Analysis. Hydraul. Pneum. Seals 2012, 32, 11–15. [Google Scholar]
- Zhou, S.C.; Zhang, H.; Lu, Y.; Zhan, D.Y.; Zhang, R.X.; Zhang, R.Z. Multi-way Valve Structure Design and Improvement. Chin. Hydraul. Pneum. 2022, 46, 38–44. [Google Scholar]
- Chen, Q.P.; Ji, H.; Zhu, Y.; Yang, X. Proposal for optimization of spool valve flow force based on the MATLAB-AMESim-FLUENT joint simulation method. IEEE Access 2018, 6, 33148–33158. [Google Scholar]
- Gui, S.; Zhang, S.; Fu, B.; Ling, M. Fluid-dynamic analysis and multi-objective design optimization of piezoelectric servo valves. Flow Meas. Instrum. 2022, 85, 102157. [Google Scholar] [CrossRef]
- Gui, S.Y.; Zhang, X.; Zhang, S.S.; Fu, B.; Ling, M.X. Dynamic Characteristic Analysis and Multi-objective Optimization of Hydraulic Servo Valve Spool. J. Xi’an Jiaotong Univ. 2022, 56, 33–45. [Google Scholar]
- Lisowski, E.; Filo, G.; Rajda, J. Analysis of flow forces in the initial phase of throttle gap opening in a proportional control valve. Flow Meas. Instrum. 2018, 59, 157–167. [Google Scholar] [CrossRef]
- Lisowski, E.; Filo, G. Analysis of a proportional control valve flow coefficient with the usage of a CFD method. Flow Meas. Instrum. 2017, 53, 269–278. [Google Scholar] [CrossRef]
- Dong, Y.K.; Fu, L.D. Structural Simulation Analysis of Spool Valve with V-shaped Throttle Groove. J. Wuhan Univ. Sci. Technol. 2021, 44, 119–124. [Google Scholar]
- Guan, T.Y. Optimal Design of Multi-Way Valve Notches Based on Steady-State Flow Force Analysis. Master’s Thesis, Dalian University of Technology, Dalian, China, 2020. [Google Scholar]
- Li, Z.C. The Steady Flow Force Optimization of Truck Cranes Multi-Way Valve Based on CFD. Master’s Thesis, Dalian University of Technology, Dalian, China, 2020. [Google Scholar]
- Zhang, G.; Huang, J.; Qiu, R. Evaluation of the Flow Forces on an Electro-hydraulic Proportional Valve Based on CFD Analysis. J. Mach. Des. 2010, 27, 93–96. [Google Scholar]
- Herakovič, N. Flow-force analysis in a hydraulic sliding-spool valve. Stroj. Časopis Teor. Praksu Stroj. 2009, 51, 555–564. [Google Scholar]
- Song, Z.L.; Zhang, X.J.; Liang, Y.W.; Zhang, J. Flow Field Characteristics and Optimization of Non-full Circumference Open Spool Valve. J. Mech. Electr. Eng. 2022, 39, 317–323. [Google Scholar]
- Duan, S.S.; Yao, P.X.; Zhang, H. Steady-state Fluid Force of Spool Valve and Its Compensation Method. Fluid Power Transm. Control 2010, 3, 27–30. [Google Scholar]
- Wu, D.; Wang, X.; Ma, Y.; Wang, J.; Tang, M.; Liu, Y. Research on the dynamic characteristics of water hydraulic servo valves considering the influence of steady flow force. Flow Meas. Instrum. 2021, 80, 101966. [Google Scholar] [CrossRef]
- Herakovič, N.; Duhovnik, J.; Šimic, M. CFD simulation of flow force reduction in hydraulic valves. Tech. Gaz. 2015, 22, 453–463. [Google Scholar] [CrossRef]
- Wang, L.X.; Zhang, M.C.; Fang, Z.H. The Influence of Valve Inner Passage Arrangement on Hydraulic Power. Mach. Tool Hydraul. 2000, 6, 27–28. [Google Scholar]
- Song, B.S. Research on Valve Port Characteristics and Hydraulic Force of Load Sensitive Multi-Way Valve. Master’s Thesis, Dalian University of Technology, Dalian, China, 2019. [Google Scholar]
- Lu, Q.; Tiainen, J.; Kiani-Oshtorjani, M.; Ruan, J. Radial flow force at the annular orifice of a two-dimensional hydraulic servo valve. IEEE Access 2020, 8, 207938–207946. [Google Scholar] [CrossRef]
- Hong, S.H.; Kim, K.W. A new type groove for hydraulic spool valve. Tribol. Int. 2016, 103, 629–640. [Google Scholar] [CrossRef]
- Lu, Q.Q.; Ruan, J.; Li, S. Study on Steady-state Flow Force of Pilot Helical Orifice of 2D Servo Valve. Fluid Mach. 2019, 47, 25–30+47. [Google Scholar]
- Zeng, Q.L.; Cui, J.; Zhao, W.M. Simulation analysis for hydraulic clamping force of bidirectional hydraulic lock’valve spool based on Fluent. Adv. Mater. Res. 2012, 542, 1091–1095. [Google Scholar] [CrossRef]
- Li, G.Q.; Zhu, Y.Q.; Gao, Y.J.; Guo, B.J. Transient Flow Force Analysis of High-pressure Large-flow Safety Valve for Hydraulic Powered Support. Chin. Hydraul. Pneum. 2014, 7, 7–9+14. [Google Scholar]
- Wang, A.L.; Wu, X.F.; Zhou, C.L.; Ma, B. Multidisciplinary Optimization of a Hydraulic Slide Valve Based on CFD. J. Shanghai Jiaotong Univ. 2010, 44, 1767–1772. [Google Scholar]
- Zhang, S.; He, X.H.; Wang, Q.; Wang, X.W. Transient Properties of the Hydraulic Spool Valve Based on CFD. Equip. Manuf. Technol. 2016, 12, 28–30+40. [Google Scholar]

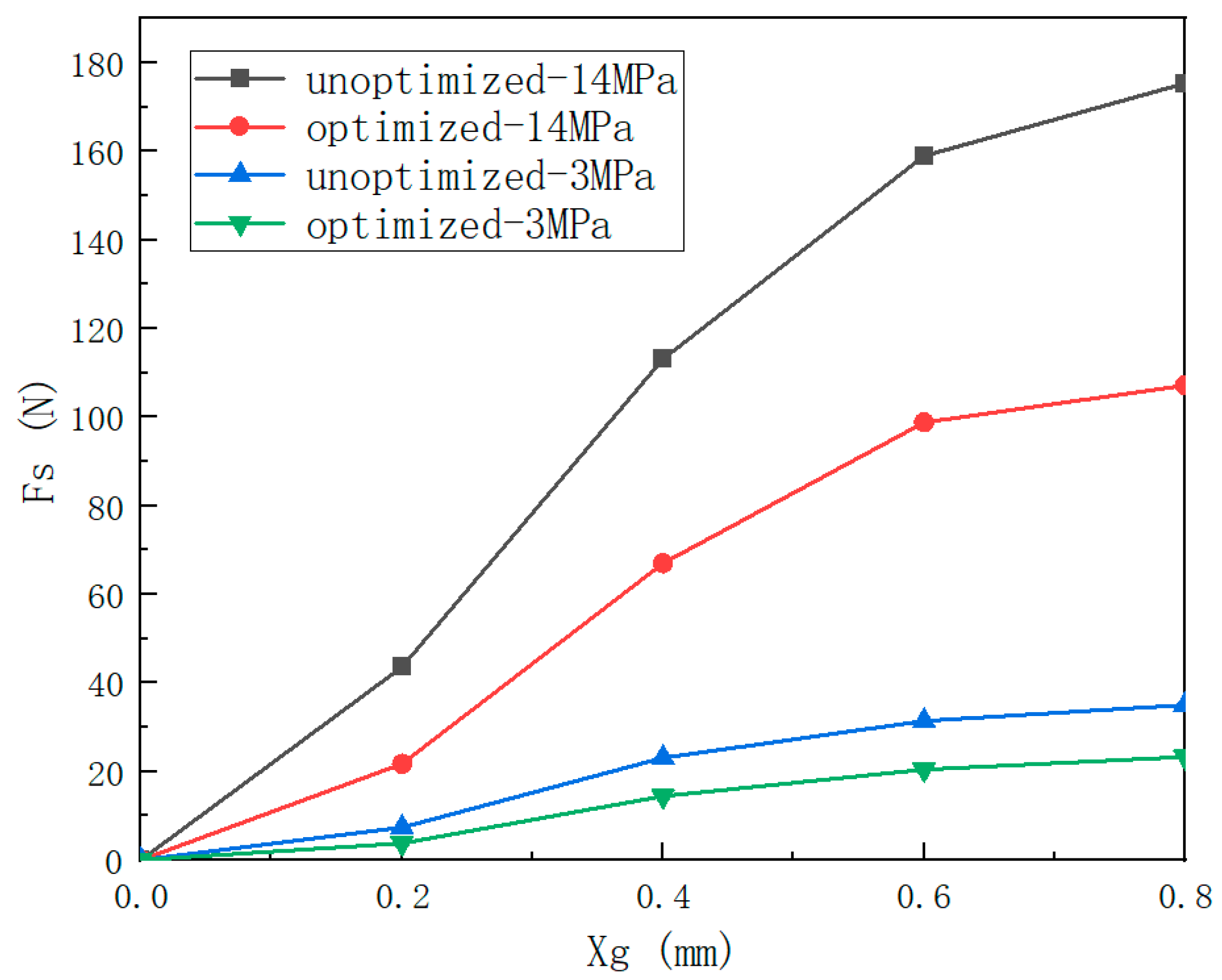
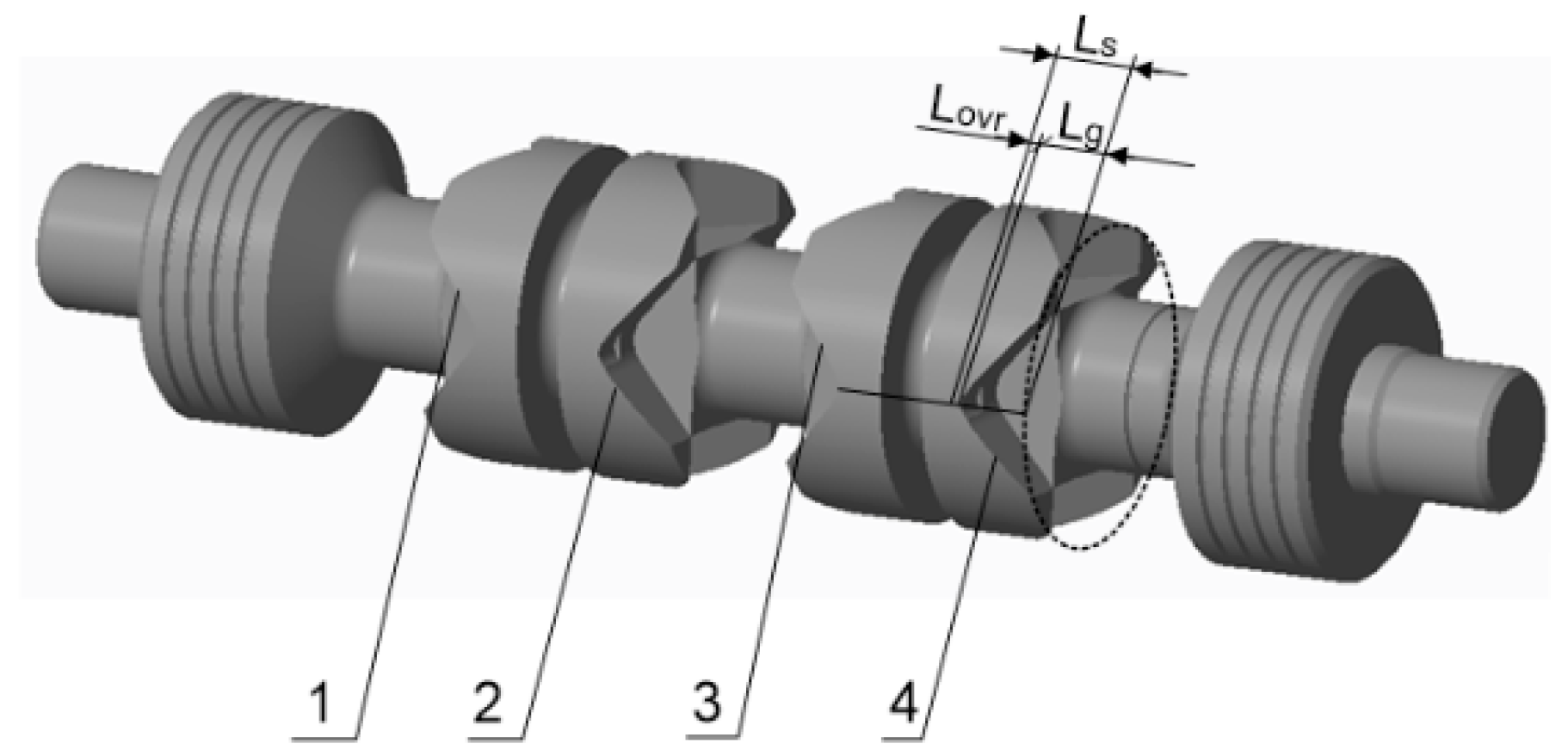

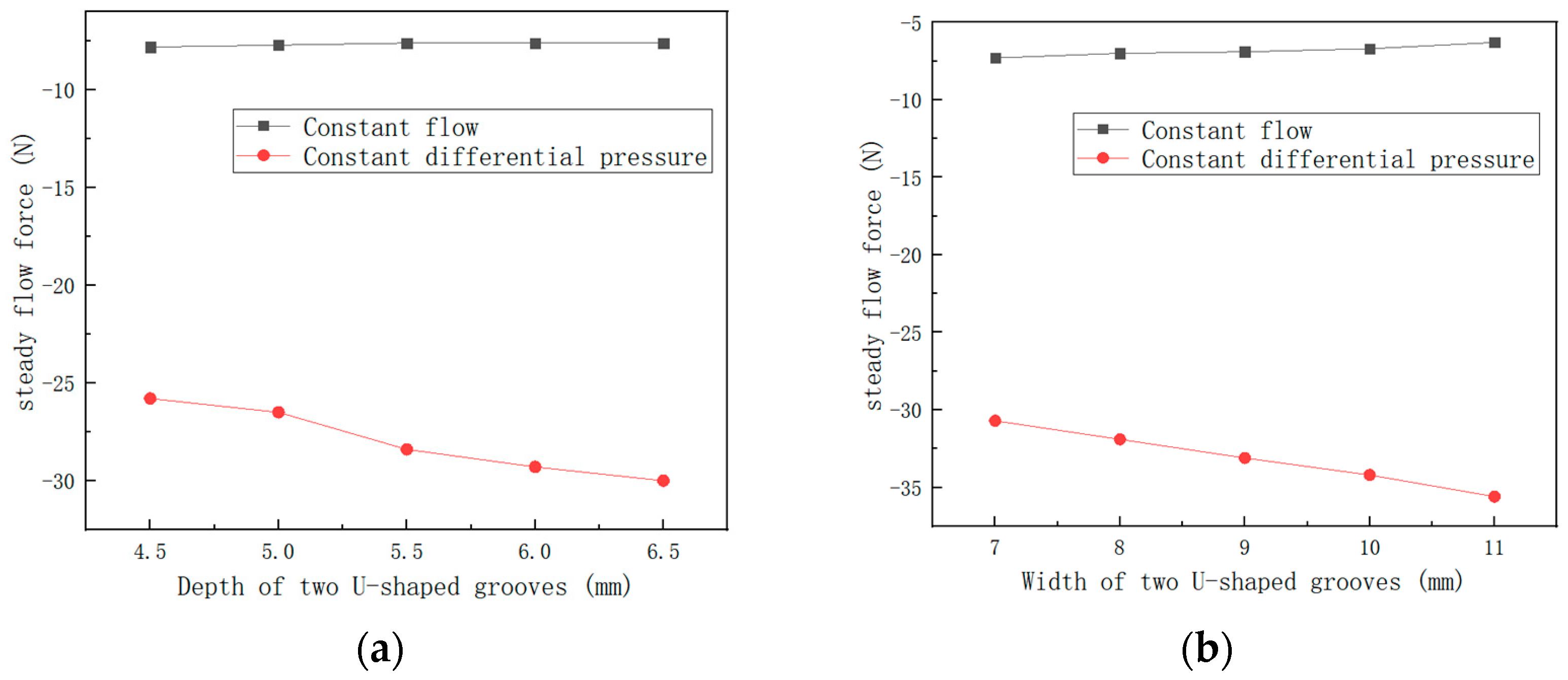
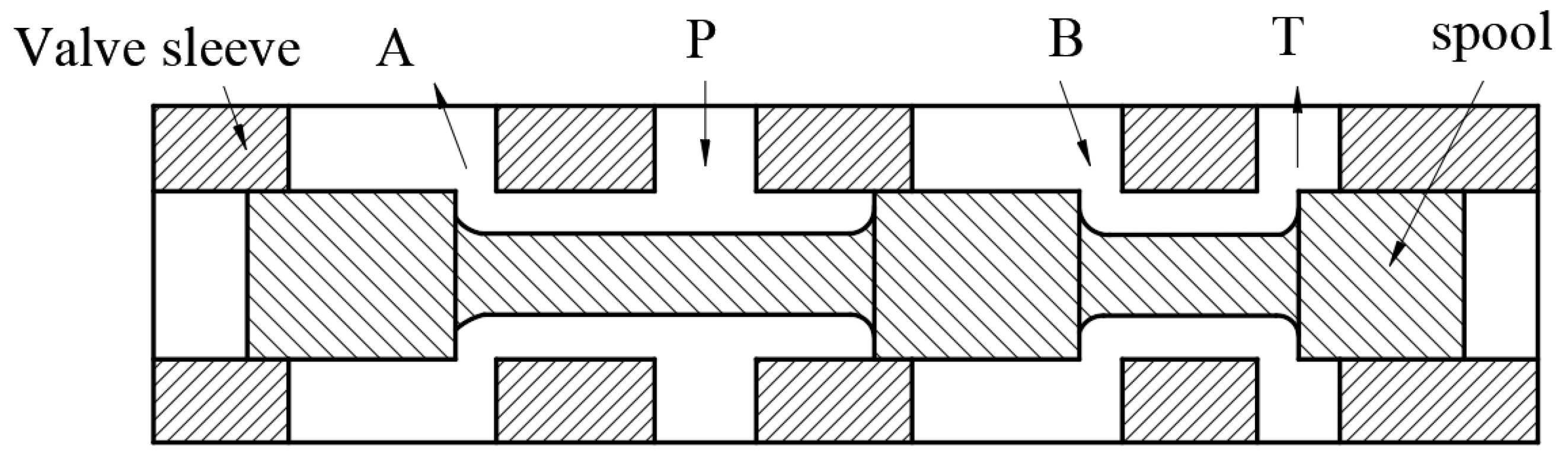
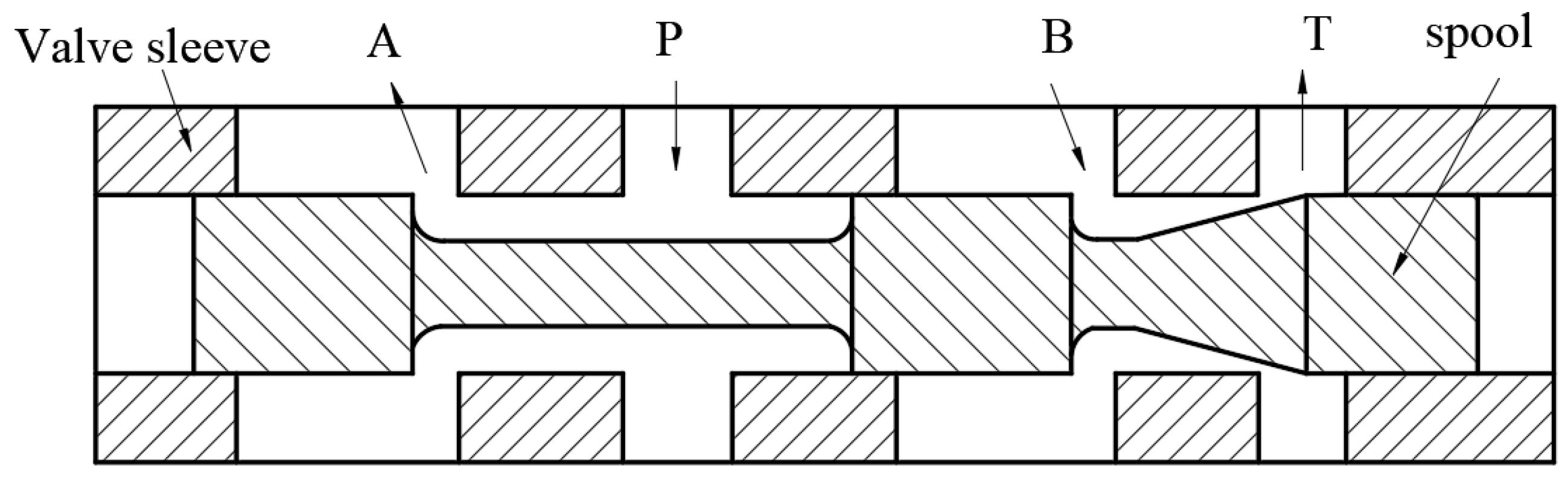

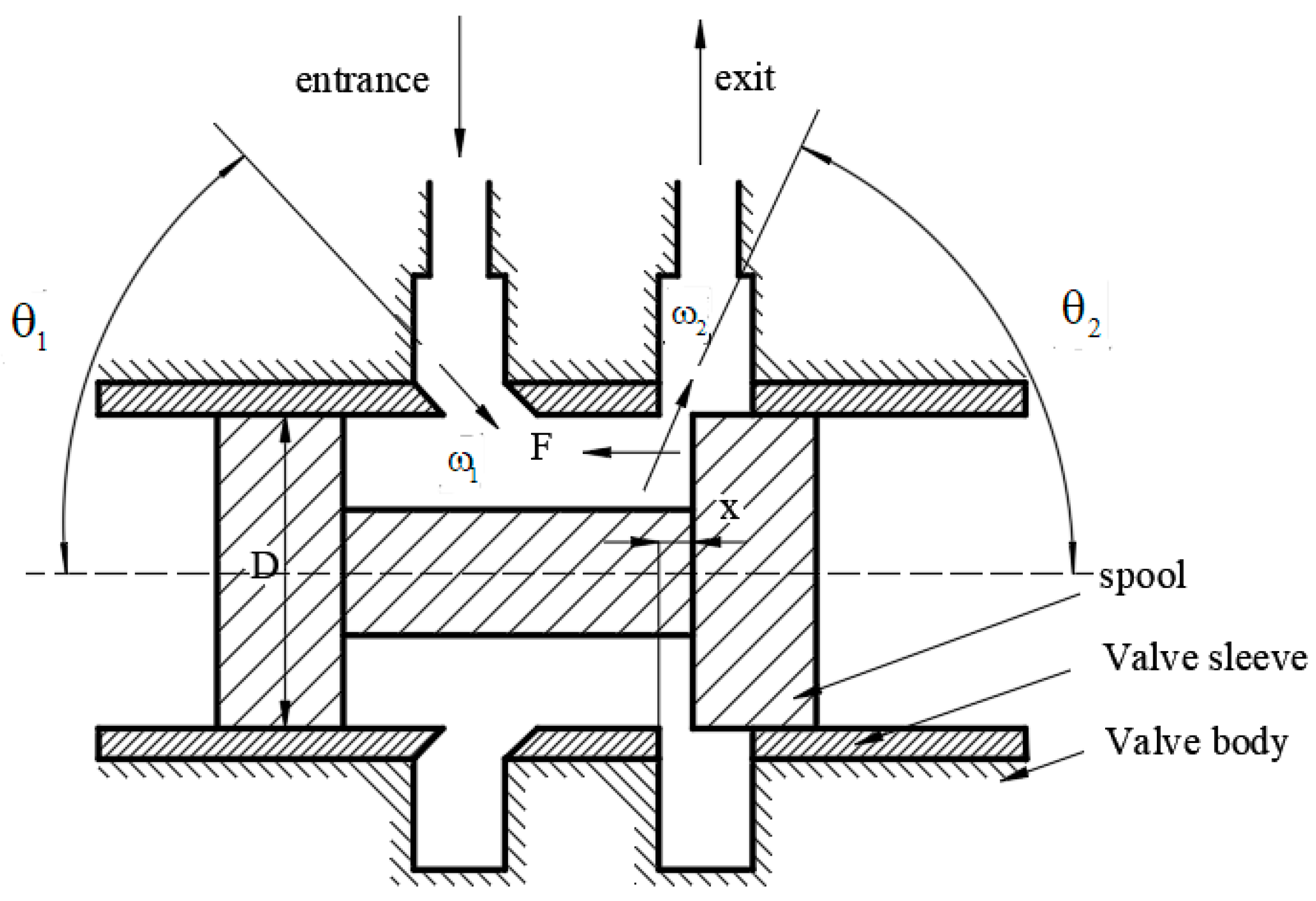

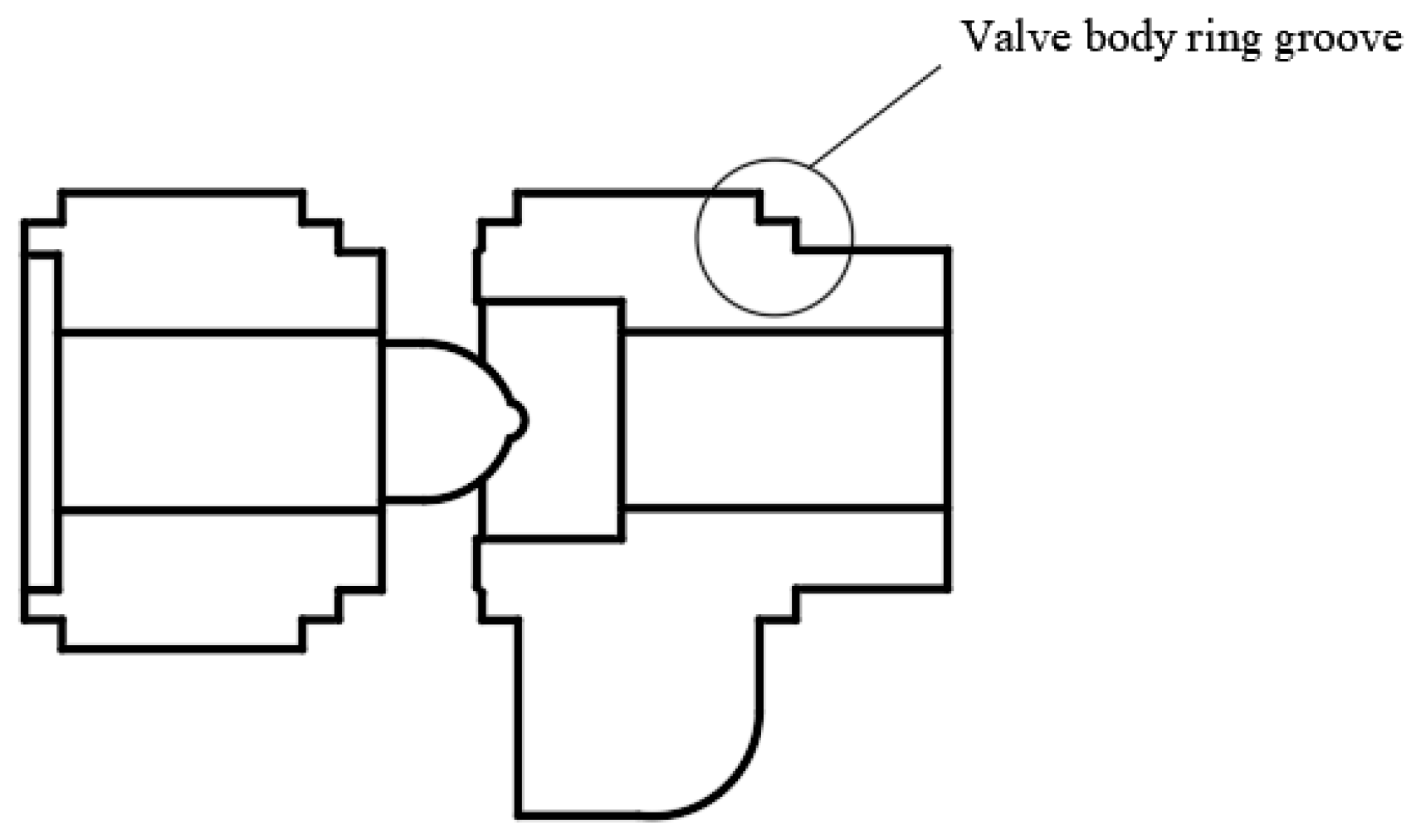


| Ring Boss Diameter/mm | Maximum Flow Loss/% | 2.1 mm Improvement Progress/% | 4 mm Improvement Progress/% |
|---|---|---|---|
| 13 | 0.4 | 24.4 | 7.2 |
| 14 | 5.7 | 40.7 | 27.8 |
| 15 | 10.0 | 44.0 | 32.2 |
| 16 | 15.6 | 45.3 | 44.1 |
| 17 | 22.6 | 38.7 | 55.1 |
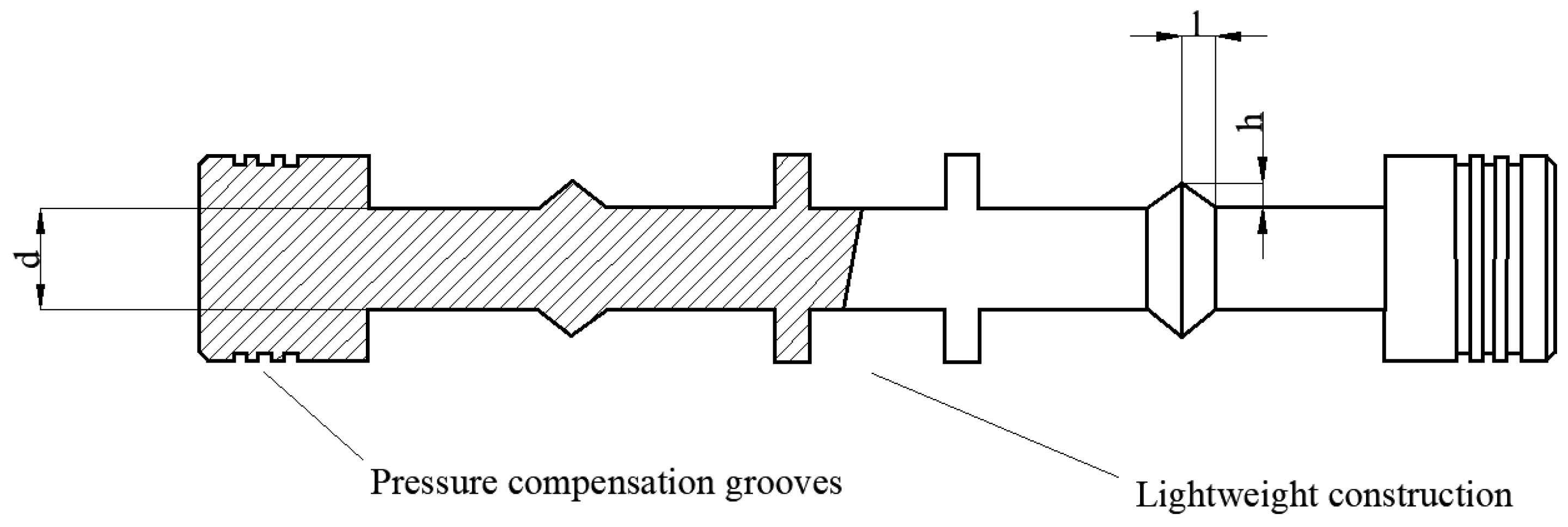
| Minor Diameter of the Spool d/mm | Height of the Compensation Profile h/mm | Half-Length of the Compensation Profile l/mm | Steady-State Flow Force FS/N |
|---|---|---|---|
| 5.4 | 1.12 | 1.17 | 84.83 |
| 5.5 | 1.13 | 1.99 | 57.46 |
| 5.6 | 1.07 | 1.98 | 56.14 |
| 5.7 | 1.05 | 1.98 | 55.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, R.; Sun, Y.; Wu, X.; Zhang, P.; Li, D.; Lin, J.; Xia, Y.; Sun, Q. Review of the Research on and Optimization of the Flow Force of Hydraulic Spool Valves. Processes 2023, 11, 2183. https://doi.org/10.3390/pr11072183
Li R, Sun Y, Wu X, Zhang P, Li D, Lin J, Xia Y, Sun Q. Review of the Research on and Optimization of the Flow Force of Hydraulic Spool Valves. Processes. 2023; 11(7):2183. https://doi.org/10.3390/pr11072183
Chicago/Turabian StyleLi, Ruichuan, Yuhang Sun, Xiaowei Wu, Peng Zhang, Defang Li, Jianghai Lin, Yuhai Xia, and Qiyou Sun. 2023. "Review of the Research on and Optimization of the Flow Force of Hydraulic Spool Valves" Processes 11, no. 7: 2183. https://doi.org/10.3390/pr11072183
APA StyleLi, R., Sun, Y., Wu, X., Zhang, P., Li, D., Lin, J., Xia, Y., & Sun, Q. (2023). Review of the Research on and Optimization of the Flow Force of Hydraulic Spool Valves. Processes, 11(7), 2183. https://doi.org/10.3390/pr11072183










