Optimization Operation Strategy for Comprehensive Energy System Considering Multi-Mode Hydrogen Transportation
Abstract
1. Introduction
2. A Comprehensive Energy System Structure Considering Multi-Mode Hydrogen Transportation
3. Hydrogen Multi-Mode Transportation Model
3.1. A Hydrogen Transport Model for Long Tube Hydrogen Trailers
3.2. A Hydrogen Transportation Model for Hydrogen Pipelines
4. Integrated Energy System Optimization Operation Framework
4.1. Constraint Condition
4.2. Objective Function
5. Case Study
5.1. Parameter Settings
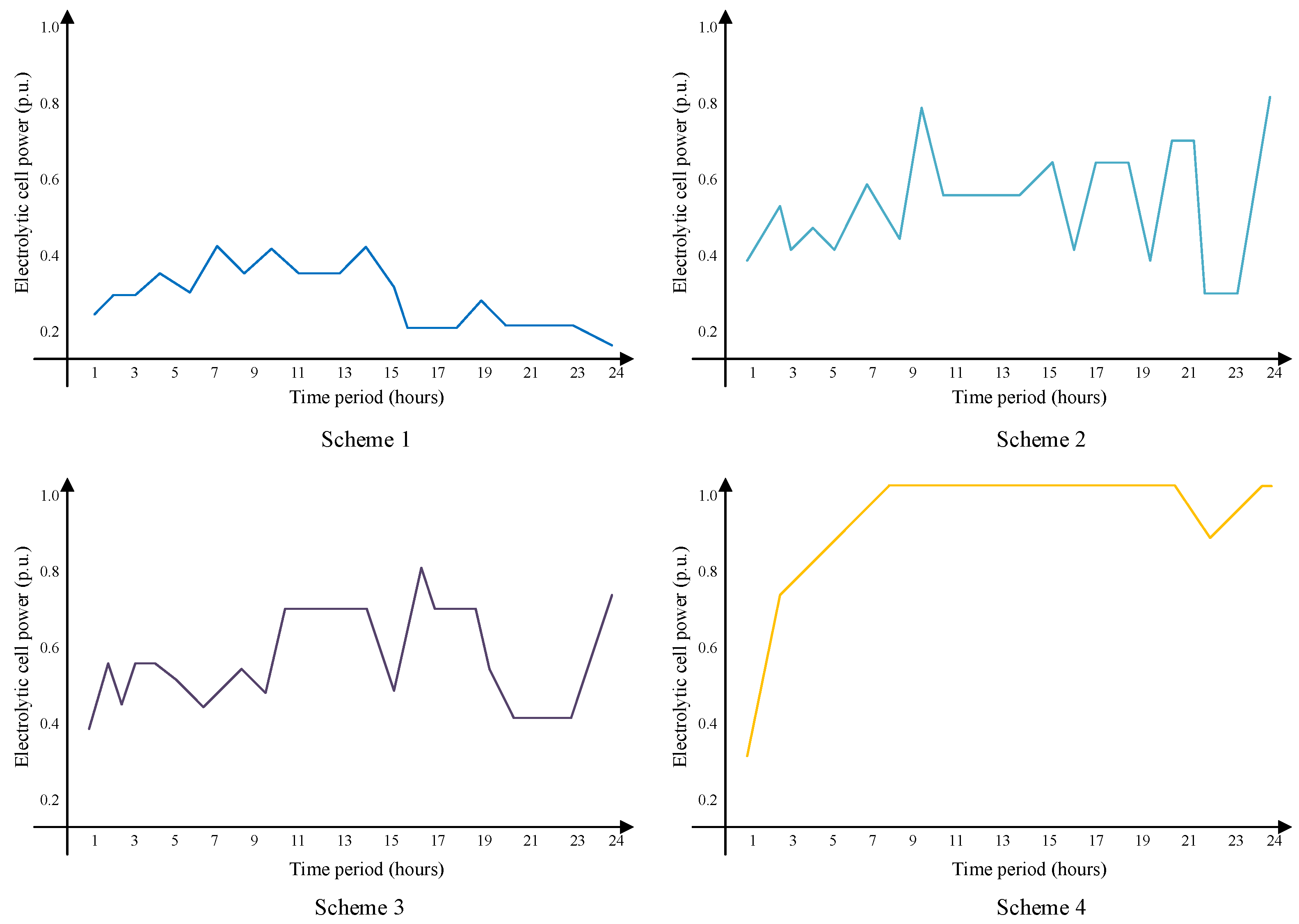
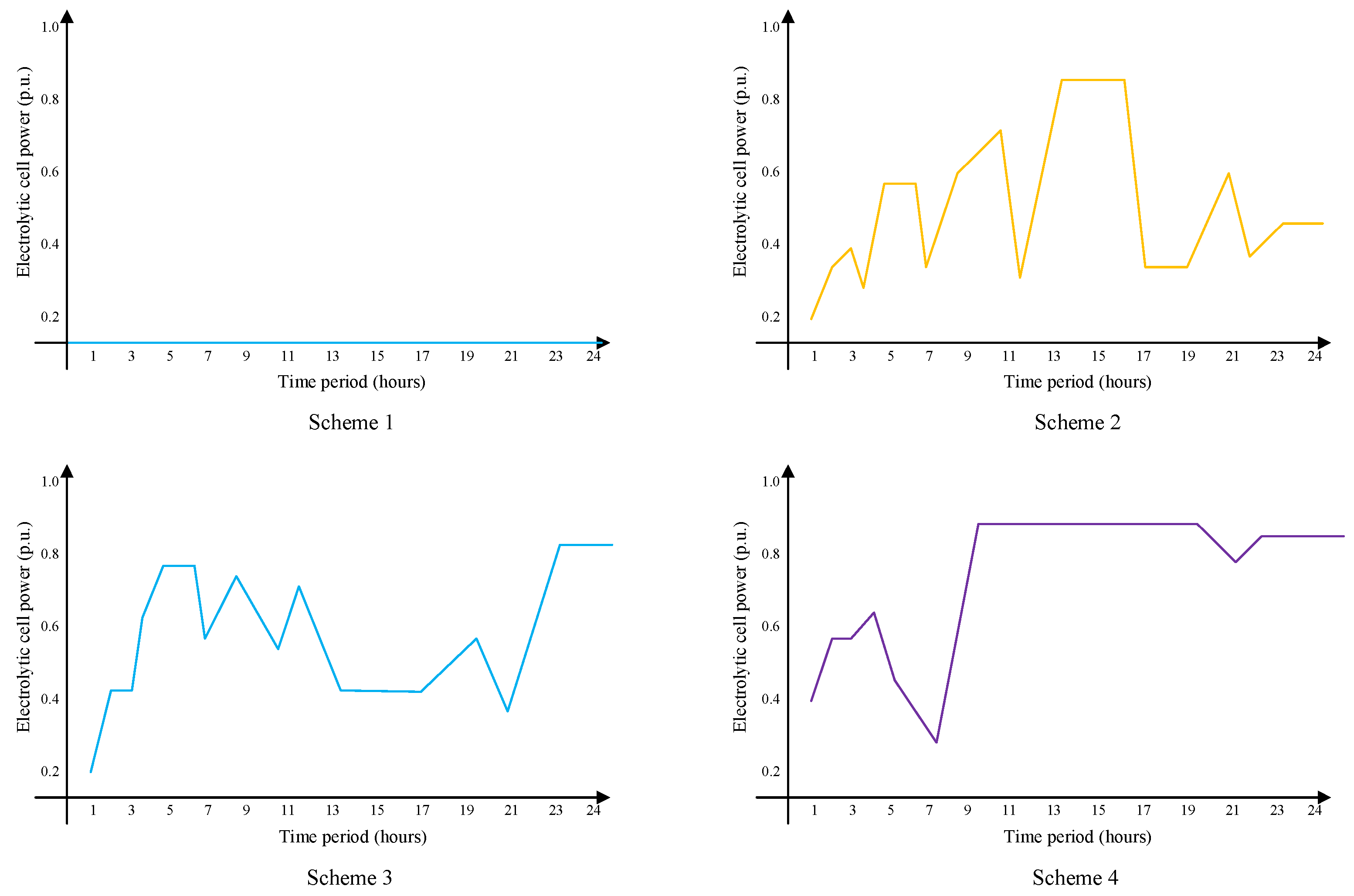
5.2. Comparative Analysis of Plans
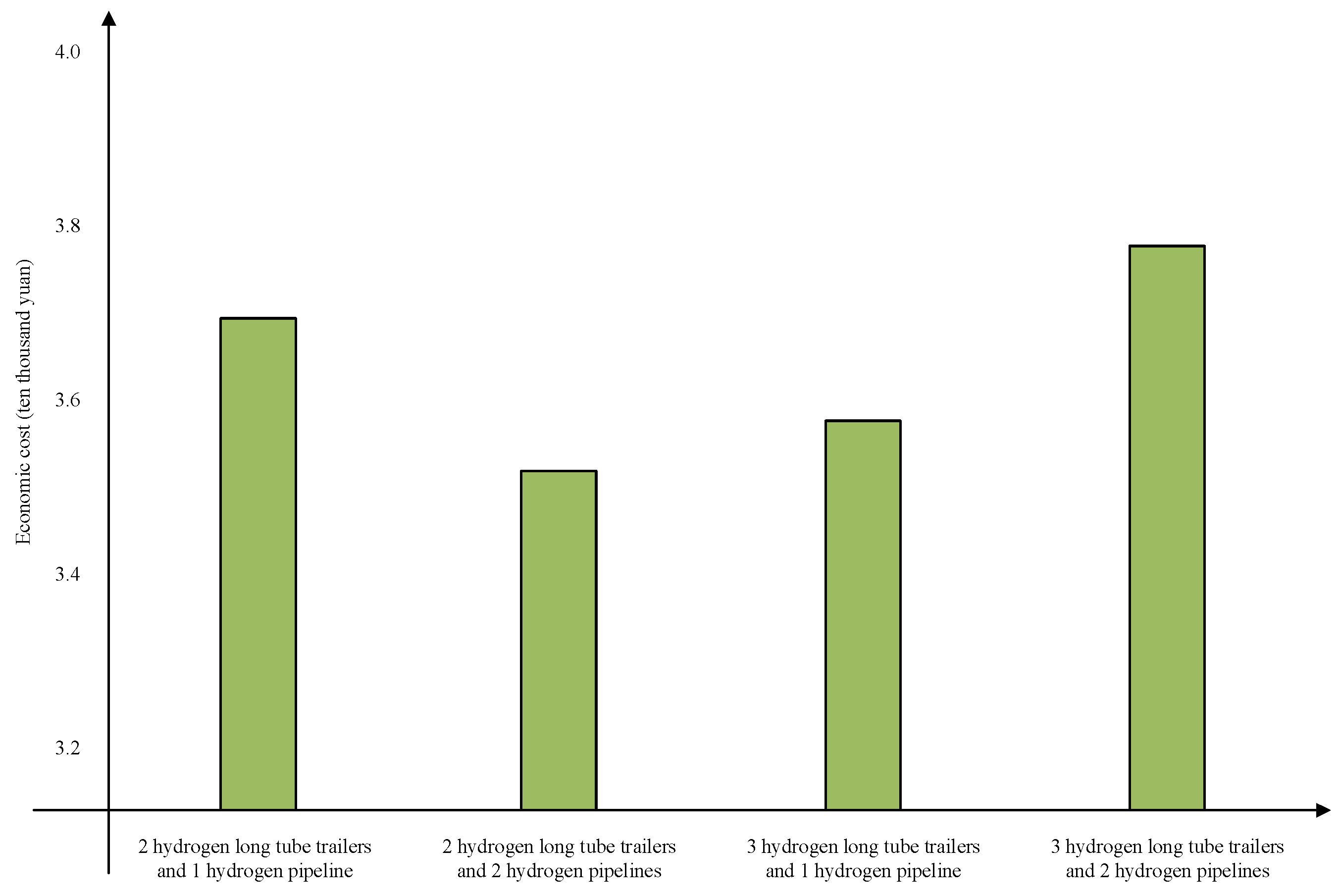
5.3. Analysis of the Impact of Hydrogen Long Tube Trailers and the Number of Hydrogen Pipelines on System Operating Costs
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- McKinsey. Net-Zero Germany: Opportunities and Challenges on the Pathway to Climate Neutrality by 2045; McKinsey: New York, NY, USA, 2021. [Google Scholar]
- U.S. Energy Information Administration. Annual Energy Outlook 2022 AEO2022 Highlights; U.S. Energy Information Administration: Washington, DC, USA, 2022.
- United States Department of State. The Long-Term Strategy of the United States: Pathways to Net-Zero Greenhouse Gas Emissions by 2050; United States Department of State: Washington, DC, USA, 2021.
- Züttel, A.; Remhof, A.; Borgschulte, A.; Friedrichs, O. Hydrogen: The future energy carrier. Phil. Trans. Math. Phys. Eng. Sci. 2010, 368, 3329–3342. [Google Scholar] [CrossRef] [PubMed]
- Cui, J.; Aziz, M. Techno-economic analysis of hydrogen transportation infrastructure using ammonia and methanol. Int. J. Hydrogen Energy 2023, 48, 15737–15747. [Google Scholar] [CrossRef]
- Faye, O.; Szpunar, J.; Eduok, U. A critical review on the current technologies for the generation, storage, and transportation of hydrogen. Int. J. Hydrogen Energy 2022, 47, 13771–13802. [Google Scholar] [CrossRef]
- Noh, H.; Kang, K.; Seo, Y. Environmental and energy efficiency assessments of offshore hydrogen supply chains utilizing compressed gaseous hydrogen, liquefied hydrogen, liquid organic hydrogen carriers and ammonia. Int. J. Hydrogen Energy 2023, 48, 7515–7532. [Google Scholar] [CrossRef]
- Sdanghi, G.; Maranzana, G.; Celzard, A.; Fierro, V. Review of the current technologies and performances of hydrogen compression for stationary and automotive applications. Renew. Sustain. Energy Rev. 2019, 102, 150–170. [Google Scholar] [CrossRef]
- Hassan, I.A.; Ramadan, H.S.; Saleh, M.A.; Hissel, D. Hydrogen storage technologies for stationary and mobile applications: Review, analysis and perspectives. Renew. Sustain. Energy Rev. 2021, 149, 111311. [Google Scholar] [CrossRef]
- Di Lullo, G.; Giwa, T.; Okunlola, A.; Davis, M.; Mehedi, T.; Oni, A.O.; Kumar, A. Large-scale long-distance land-based hydrogen transportation systems: A comparative techno-economic and greenhouse gas emission assessment. Int. J. Hydrogen Energy 2022, 47, 35293–35319. [Google Scholar] [CrossRef]
- Wang, H.; Tong, Z.; Zhou, G.; Zhang, C.; Zhou, H.; Wang, Y.; Zheng, W. Research and demonstration on hydrogen compatibility of pipelines: A review of current status and challenges. Int. J. Hydrogen Energy 2022, 47, 28585–28604. [Google Scholar] [CrossRef]
- Gordon, J.A.; Balta-Ozkan, N.; Nabavi, S.A. Socio-technical barriers to domestic hydrogen futures: Repurposing pipelines, policies, and public perceptions. Appl. Energy 2023, 336, 120850. [Google Scholar] [CrossRef]
- Zhou, J.; Li, S.; Zhou, X.; Li, C.; Xiong, Z.; Zhao, Y.; Liang, G. Operation optimization for gas-electric integrated energy system with hydrogen storage module. Int. J. Hydrogen Energy 2022, 47, 36622–36639. [Google Scholar] [CrossRef]
- Wang, J.; Xue, K.; Guo, Y.; Ma, J.; Zhou, X.; Liu, M.; Yan, J. Multiobjective capacity programming and operation optimization of an integrated energy system considering hydrogen energy storage for collective energy communities. Energy Convers. Manag. 2022, 268, 116057. [Google Scholar] [CrossRef]
- Schrotenboer, A.H.; Veenstra, A.A.T.; uit het Broek, M.A.J.; Ursavas, E. A Green Hydrogen Energy System: Optimal control strategies for integrated hydrogen storage and power generation with wind energy. Renew. Sustain. Energy Rev. 2022, 168, 112744. [Google Scholar] [CrossRef]
- Ruiming, F. Multi-objective optimized operation of integrated energy system with hydrogen storage. Int. J. Hydrogen Energy 2019, 44, 29409e17. [Google Scholar] [CrossRef]
- Song, Y.; Mu, H.; Li, N.; Wang, H. Multi-objective optimization of large-scale grid-connected photovoltaic-hydrogen-natural gas integrated energy power station based on carbon emission priority. Int. J. Hydrogen Energy 2023, 48, 4087–4103. [Google Scholar] [CrossRef]
- Sohani, A.; Delfani, F.; Hosseini, M.; Sayyaadi, H.; Karimi, N.; Li, L.K.B.; Doranehgard, M.H. Dynamic multi-objective optimization applied to a solar-geothermal multi-generation system for hydrogen production, desalination, and energy storage. Int. J. Hydrogen Energy 2022, 47, 31730e41. [Google Scholar] [CrossRef]
- Dehdari, L.; Burgers, I.; Xiao, P.; Li, K.G.; Singh, R.; Webley, P.A. Purification of hydrogen from natural gas/hydrogen pipeline mixtures. Sep. Purif. Technol. 2022, 282, 120094. [Google Scholar] [CrossRef]
- Hansen, O.R. Hydrogen infrastructure—Efficient risk assessment and design optimization approach to ensure safe and practical solutions. Process Saf. Environ. Prot. 2020, 143, 164–176. [Google Scholar] [CrossRef]
- Luo, H.; Sohn, S.S.; Lu, W.; Li, L.; Li, X.; Soundararajan, C.K.; Krieger, W.; Li, Z.; Raabe, D. A strong and ductile medium-entropy alloy resists hydrogen embrittlement and corrosion. Nat. Commun. 2020, 11, 3081. [Google Scholar] [CrossRef]
- Correa-Posada, C.M.; Sánchez-Martín, P. Integrated Power and Natural Gas Model for Energy Adequacy in Short-Term Operation. IEEE Trans. Power Syst. 2015, 30, 3347–3355. [Google Scholar] [CrossRef]
- Menon, E.S. Gas Pipeline Hydraulics; Taylor & Francis: Boca Raton, FL, USA, 2005. [Google Scholar]
- Correa-Posada, C.M.; Sanchez-Martin, P. Gas Network Optimization: A Comparison of Piecewise Linear Models. Unpubl. Prepr. 2014, pp. 1–24. Available online: http://www.optimization-online.org/DB_FILE/2014/10/4580.pdf (accessed on 27 October 2024).
- Zhang, C.; Kuang, Y. Low-Carbon Economy Optimization of Integrated Energy System Considering Electric Vehicles Charging Mode and Multi-Energy Coupling. IEEE Trans. Power Syst. 2023, 39, 3649–3660. [Google Scholar] [CrossRef]
- Tao, Y.; Qiu, J.; Lai, S.; Sun, X. Coordinated Planning of Electricity and Hydrogen Networks with Hydrogen Supply Chain for Fuel Cell Electric Vehicles. IEEE Trans. Sustain. Energy 2023, 14, 1010–1023. [Google Scholar] [CrossRef]
- Fang, S.; Zhang, S.; Zhao, T.; Liao, R. Optimal Power-Hydrogen Networked Flow Scheduling for Residential Carpark With Convex Approximation. IEEE Trans. Ind. Appl. 2022, 58, 2751–2759. [Google Scholar] [CrossRef]

| Equipment | Rated Capacity |
|---|---|
| Hydrogen storage tank | 3000 kg |
| Electrolytic tank | 20 MW |
| Cogeneration unit | 100 km3 |
| Hydrogen fuel cell | 10 MW |
| Wind turbines | 50 MW |
| Photovoltaic unit | 40 MW |
| Electrochemical energy storage | 10 MWh |
| Hydrogen pipeline | 10 km3/h |
| Hydrogen long tube trailer | 200 kg |
| Time | Wind Power | Photovoltaic | Electric Load | Hydrogen Load | Heat Load |
|---|---|---|---|---|---|
| 1 | 0.95 | 0 | 0.21 | 0 | 0.1795 |
| 2 | 0.7 | 0 | 0.05 | 0 | 0.141 |
| 3 | 1 | 0 | 0.1 | 0 | 0.1621 |
| 4 | 0.55 | 0 | 0.14 | 0 | 0.2083 |
| 5 | 0.78 | 0 | 0.23 | 0 | 0.2723 |
| 6 | 0.7 | 0 | 0.38 | 0.1464 | 0.4733 |
| 7 | 0.43 | 0.18 | 0.49 | 0.5323 | 0.5299 |
| 8 | 0.1 | 0.26 | 0.73 | 1 | 0.628 |
| 9 | 0.45 | 0.29 | 0.9 | 0.57 | 0.5821 |
| 10 | 0.23 | 0.47 | 0.95 | 0.2338 | 0.4742 |
| 11 | 0.83 | 0.59 | 0.77 | 0.1509 | 0.3865 |
| 12 | 0.98 | 0.82 | 0.51 | 0.1021 | 0.6943 |
| 13 | 1 | 0.97 | 0.63 | 0.2338 | 0.6615 |
| 14 | 0.95 | 1 | 0.77 | 0.0912 | 0.3856 |
| 15 | 0.2 | 0.94 | 0.87 | 0.5243 | 0.222 |
| 16 | 0.35 | 0.88 | 0.95 | 0.57 | 0.2256 |
| 17 | 0.2 | 0.82 | 1 | 0.8367 | 0.3466 |
| 18 | 0.5 | 0.59 | 0.79 | 0.825 | 0.8025 |
| 19 | 0.22 | 0.29 | 0.73 | 0.2558 | 0.8745 |
| 20 | 0.06 | 0.06 | 0.54 | 0.2272 | 1 |
| 21 | 0.5 | 0 | 0.46 | 0.136 | 0.9776 |
| 22 | 0.75 | 0 | 0.38 | 0.1039 | 0.8377 |
| 23 | 0.2 | 0 | 0.28 | 0 | 0.7038 |
| 24 | 0.75 | 0 | 0.21 | 0 | 0.2468 |
| Scheme | Total Cost | Abandoned Scenery Costs | Electricity Purchase Costs | Gas Purchase Costs | Hydrogen Transportation Costs |
|---|---|---|---|---|---|
| 1 | 12,834.00 | 4250.40 | 3891.60 | 5106.00 | 0 |
| 2 | 1077.80 | 2925.60 | 3891.60 | 2939.40 | 897.00 |
| 3 | 11,178.00 | 2898.00 | 3477.60 | 3726.00 | 1076.40 |
| 4 | 5078.40 | 0 | 1449.00 | 1518.00 | 2111.40 |
| Scheme | Power Generation/MW | Abandoned Power/MW | Abandonment Rate |
|---|---|---|---|
| 1 | 995.4 | 88 | 8.84% |
| 2 | 995.4 | 60.57 | 6.09% |
| 3 | 995.4 | 60 | 6.03% |
| 4 | 995.4 | 0 | 0% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Zhou, Z.; Chen, J.; Zheng, D.; Zou, H. Optimization Operation Strategy for Comprehensive Energy System Considering Multi-Mode Hydrogen Transportation. Processes 2024, 12, 2893. https://doi.org/10.3390/pr12122893
Liu Q, Zhou Z, Chen J, Zheng D, Zou H. Optimization Operation Strategy for Comprehensive Energy System Considering Multi-Mode Hydrogen Transportation. Processes. 2024; 12(12):2893. https://doi.org/10.3390/pr12122893
Chicago/Turabian StyleLiu, Qingming, Zhengkun Zhou, Jingyan Chen, Dan Zheng, and Hongbo Zou. 2024. "Optimization Operation Strategy for Comprehensive Energy System Considering Multi-Mode Hydrogen Transportation" Processes 12, no. 12: 2893. https://doi.org/10.3390/pr12122893
APA StyleLiu, Q., Zhou, Z., Chen, J., Zheng, D., & Zou, H. (2024). Optimization Operation Strategy for Comprehensive Energy System Considering Multi-Mode Hydrogen Transportation. Processes, 12(12), 2893. https://doi.org/10.3390/pr12122893





