Viscoelastic Behavior of an Epoxy Resin Modified with Recycled Waste Particles Analyzed through a Fractional Model

Abstract

It is well-known that the addition of randomly dispersed particles in polymers influences their linear viscoelastic behavior and dynamic mechanical properties. The aim of this study was to describe the viscoelastic behavior of an epoxy resin modified by waste glass and rubber particles using the linear fractional spring-pot model. Unlike complex classical exponential models, fractional models, being only two-parameter dependent, make it easier to characterize the viscoelastic behavior of materials. Isothermal relaxation and single frequency sweep temperature dynamic tests were carried out in a dynamic mechanical analyzer DMA$150$ by varying the content of the particles from 0 to 20% by weight. Overall, the results of this study evidence that using waste materials as additives for polymer compounds is a practical and sustainable possibility when it comes to modifying their viscoelastic properties.

1. Introduction

Nowadays, the use of polymeric materials in building fields is becoming more promising due to their ease of manufacturing, durability properties, lightness, and economy. Furthermore, the possibility to significantly modify their mechanical and viscoelastic behaviors was demonstrated by the addition of fillers of different nature [1]. However, a thorough study aimed at understanding and predicting the long-term viscoelastic behavior of these type of material is compulsory [2]. Engineers seek thorough knowledge on the viscoelastic properties of the material for several industrial applications [3]. Most of the materials exhibit viscoelastic behavior, this implies that, when a constant stress is applied, an increase in strain is observed over time (creep). On the contrary, when a constant strain is imposed, the stress progressively decreases (relaxation).

Up until the first decades of the past century, classical models, such as those proposed by Maxwell and Kelvin-Voigt, were commonly used to describe the viscoelastic behavior of the materials. For example, the Maxwell model is used in papers [4,5,6,7], while the Kelvin-Voigt model is used in papers [4,5,8,9] Other classical models, such as standard linear solid, the Zener models, the Burger models, etc., are obtained by means of more complex and sophisticated arrangements of springs and dashpots (see, e.g., [10]). These are based on exponential equations to define the constitutive law through combinations of integer derivative functions of stress and strain. The shortcomings of these models were centered on the impossibility of defining an univocal constitutive law capable of describing both creep and relaxation conditions. Furthermore, in some cases, a satisfying approximation of the actual experimental viscoelastic behavior could only be obtained by complicated combinations of elements in which many parameters need to be calculated.

Around the 1950s Blair and Caffyn [11], based on previous experimental evidence obtained by Nutting (1921) [12] and Gemant (1936) [13], demonstrated that viscoelastic behavior, in terms of creep and relaxation of most of the materials (e.g., concrete, rubber, polymer), could be modeled by a power law in which only two parameters needed to be defined. In fact, they showed that experimental data of relaxation tests were well fitted by a power law decay:

$$E\left(t\right)=\frac{C_{\beta }}{\Gamma (1-\beta )}{t}^{-\beta }$$

(1)

in which $\beta$ ($0\le \beta \le 1$) and $C_{\beta }$, were coefficients obtained by a best fitting procedure of the experimental results. These two parameters define the constitutive law of most of the materials, both for relaxation and creep conditions [14,15,16,17]. Recent studies have considered the fractional models as the limit of the classical generalized Maxwell model (GMM) and generalized Kelvin model (GKM), when the number of elements in these models tend to infinite [18,19].

The proposed paper evaluates how the viscoelastic and the dynamical mechanical behavior of an epoxy resin can be modified by the addition of disperse waste powders of two different natures: glass (stiff additive) and rubber (soft additive). A two-parameter fractional model was evidenced to well fit the experimental results [20]. This makes possible the prediction of the behavior of this type of material and, hence, the possible application in civil and building fields. Another very important feature is the use of recycled particles, which leads to a lower consumption of new raw materials, by extending the life cycle, and consequently to effective environmental benefits. A vast experimental campaign was performed on the modified polymers, and for each percentage of the two different additives, the power law parameters ($\beta$ and $C_{\beta }$) were obtained by best fitting.

2. Materials and Methods

2.1. Raw Materials

2.1.1. Epoxy Resin (SX 8)

An epoxy resin (SX 8 EVO supplied by Mates Italiana S.r.l. [21]), mixed with its own M-type hardener in a 100 : 30 mix ratio by weight, was under study. This thermosetting polymer, due to its higher mechanical properties and better resistance to environmental degradation compared to the polyester resins, is widely used in several high-performance applications, such as aircraft components and boat building. In particular, its low viscosity enables the production of fiber reinforced composites through different manufacturing processes (e.g., vacuum bagging and resin infusion). Some data-sheet information about the properties of the resin in use are reported in

Table 1. Some datasheet information of the used epoxy resin [21].

SX8 EVO Epoxy Resin System
Density [g/cm${}^3$]	1.2
Viscosity at $25{}^{\circ }$C [mPas]	550
Gel Time at $25{}^{\circ }$C [h]	3
Tensile modulus [GPa]	2.7
Tensile strength [MPa]	60

.

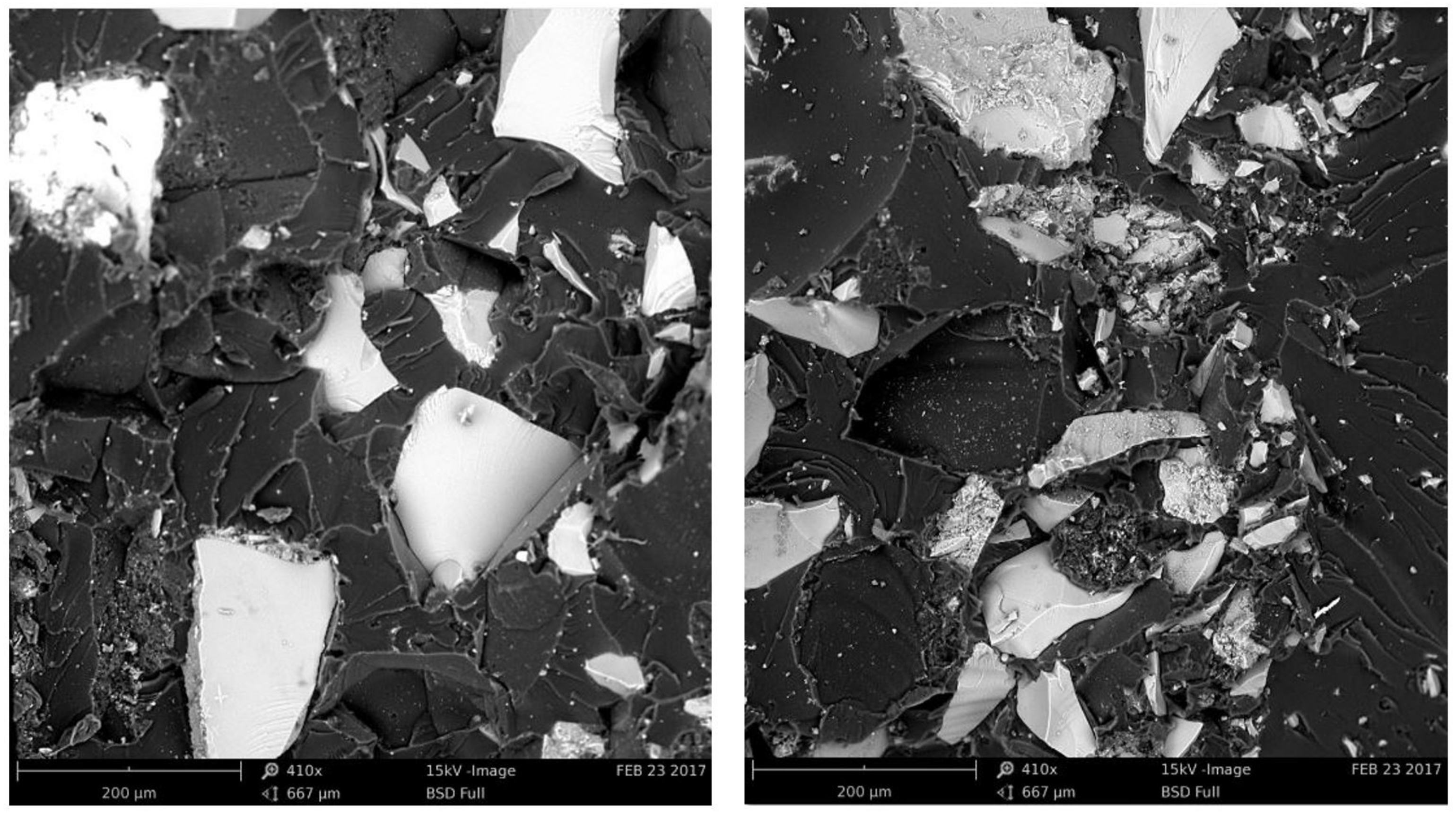
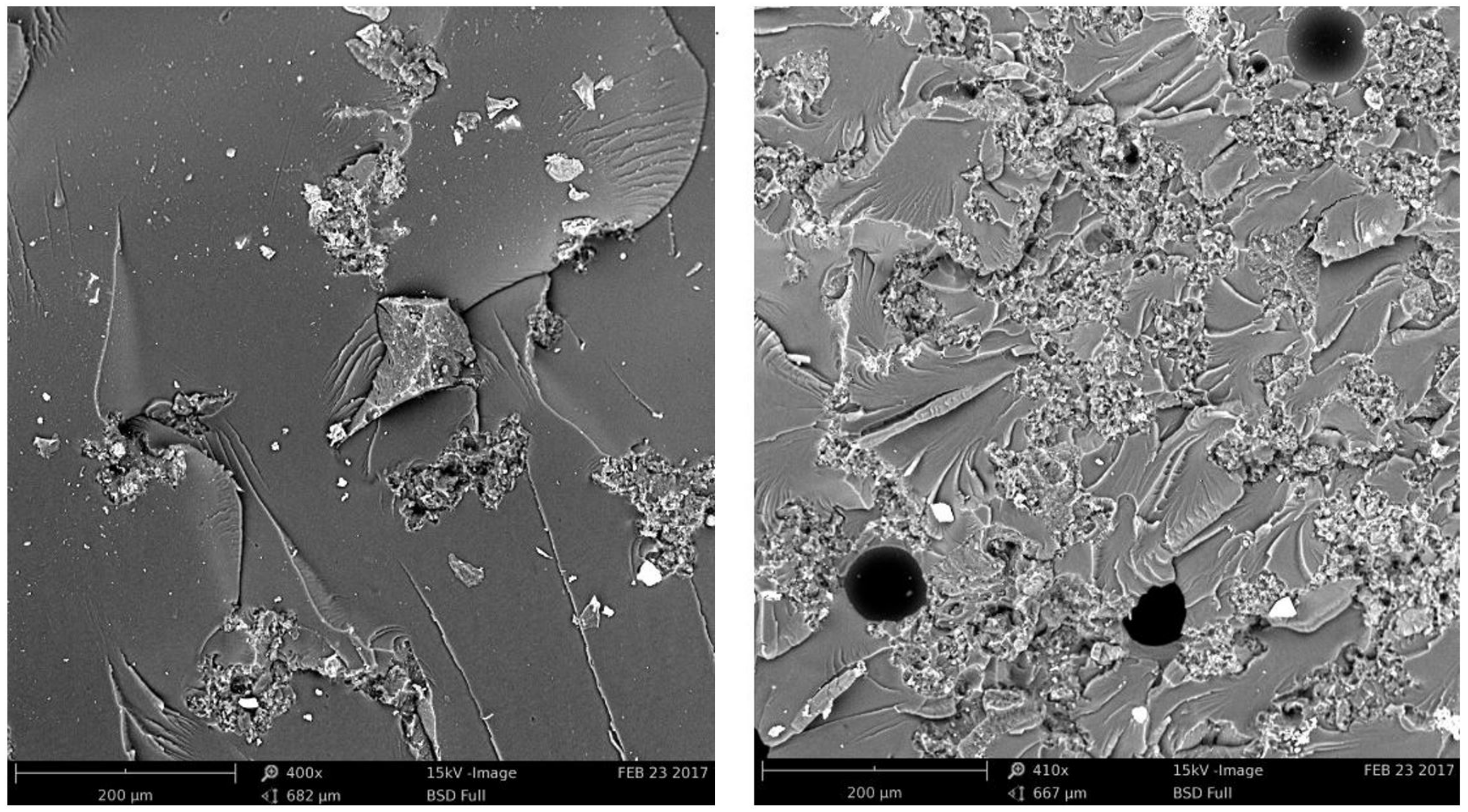
2.1.2. Waste Glass Powder

The recycled glass powder, derived from waste glass residues, was provided by Sarco S.r.l. It was used as additive in particulate composites. The complete chemical characterization of the glass powder was performed in a previous study [22]. Firstly, this powder was dried in an oven at 110${}^{\circ }$C and then sieved to describe the granulometric distribution. Crystalline morphology investigated by XRD analysis, evidenced the prevalence of silica in the amorphous state, absence of organic compounds. The quantitative simultaneous thermogravimetric analysis (STA) revealed the presence of 0.3% wt of CaCO${}_3$. Since the particle shape influences the stress concentration at the matrix–particle interface and the matrix–particle interaction, the morphology of the glass particles was investigated through SEM observations. It was observed that most of the particles were of angular shape rather than spherical shape due to the milling process. Furthermore, the elemental analysis (SEM-EDS) confirmed that the powder used was a common soda lime glass. The waste glass was first mechanically milled using a mortar and then manually sieved with the aid of common sieves in order to obtain particle sizes in the range between 100 and 200 $\mathsf{\mu }$m.

2.1.3. Waste Rubber Powder

The recycled rubber powder was derived from out-of-service pneumatic tire, which are those tires that no longer have the performance to ensure safety and efficiency and, thus, become waste, to be recovered and reused. These elastomers are characterized by high deformability and elasticity. Different products can be obtained from these residues, among which we can remember the rubber chipboard of size 20–50 mm; the granular rubber with dimensions 0.8–20 mm; the rubber dust <8 mm, and finally the textile used as additive in particulate composites. The composition of tires affects the key characteristics of the final product, with the variations observed in the material quantities used depending on the application. According to industry bodies [23], the simplified composition of tires include varying levels of core ingredients:

• Natural and synthetic rubbers;
• Fillers (carbon black, silica, chalk, or carbon);
• Reinforcing materials (metals and textiles);
• Plasticisers (oils and resins);
• Vulcanising agents (sulphur and zinc oxide);
• Additives.

As reported in the literature [24,25], the constituent materials of passenger car tires are the following: rubber/elastomers 47%; carbon black 21.5%; metals 16.5%; textiles 5.5%; zinc oxide 1%; sulfur 1%; and additives 7.5%. The waste rubber was first mechanically shredded using a PROXXON TBH Bench Drill and then manually sieved with the aid of common sieves in order to obtain particle size in the range of 100 to 200 $\mathsf{\mu }$m.

2.2. Composites Manufacturing

Nine different batches of particulate composites were manufacturedm depending on the percentage by weight of glass and rubber powders as additive of the epoxy resin. In particular, neat resin and 2, 5, 10, and 20% by weight of both glass and rubber waste particles were prepared. The powders were previously mixed with the hardener for 5 min with the aid of a magnetic stirrer in order to improve the dispersion of the particles. Then, the resin in the stoichiometric amount was added and mixed for further 5 min until complete homogenization of the mixtures. The blends were then degassed for 5 min before casting in appropriate molds and the curing process was carried out for 2 h at 40${}^{\circ }$C in oven, in order to accelerate the hardening of the samples and reduce the sedimentation phenomenon, followed by 22 h at 30${}^{\circ }$C within molds. The samples were then taken out of the molds and post-cured for 24 h in the oven at 60${}^{\circ }$C. Geometry and specimen dimensions were defined according to ASTM D4065-01 Standard [26] and the DMA$150$ Metravib manual, for three-point bending and the tensile dynamic-mechanical testing mode. For the flexural relaxation test, at least seven specimens, of nominal dimensions: 3 × 8 × 65 mm, for each batch were prepared, while for tensile tests, at least five samples per type, having 3 × 3.5 × 45 mm sizes were made. The actual width and thickness of each specimen was recorded before the tests using a centesimal digital caliber as the average of three evenly-spaced measurements.

2.3. Viscoelastic Characterization

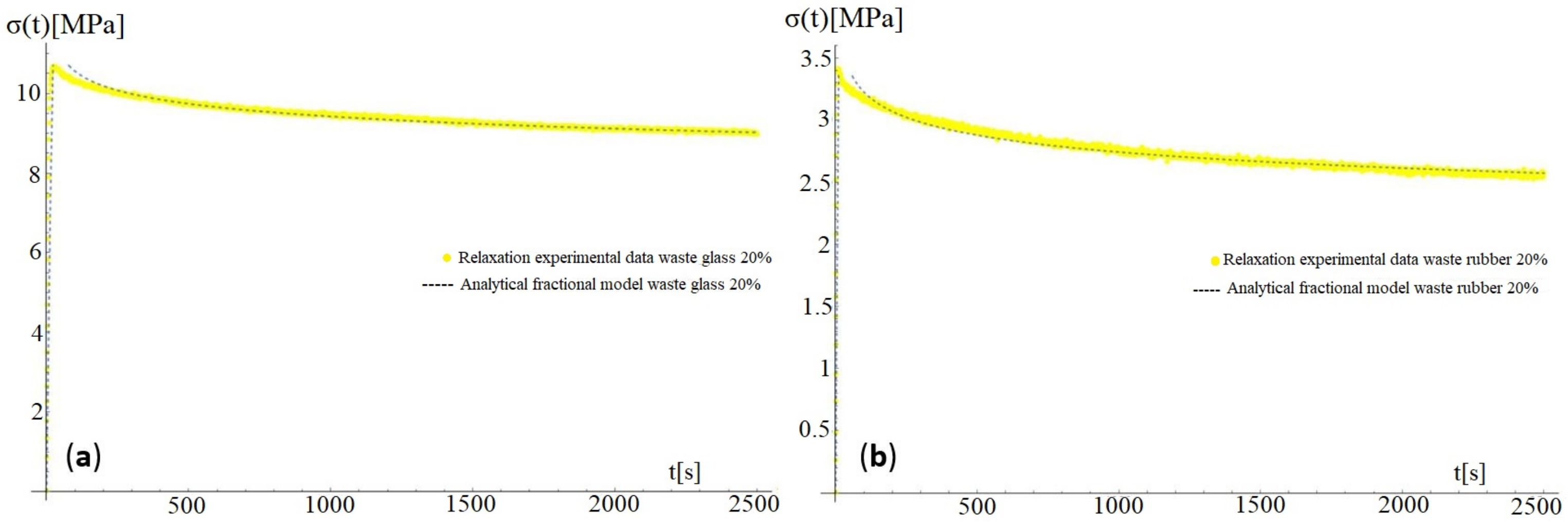
2.3.1. Relaxation Test

Relaxation experimental tests in three-point bending mode (TPB) were performed in a DMA$150$ Metravib on specimens placed on two supports with a span length of 55 mm. The tests were performed in displacement control at 30${}^{\circ }$C. The crosshead speed was 2 mm/min while the imposed displacement was equal to 0.37 mm, which corresponds to a maximum static strain of 0.22%, a value that ensures remaining within the linear viscoelastic behavior of the material. The relaxation time was set to 2500 s. At least five specimens of each type were tested. The best fitting procedure of the experimental relaxation data was performed using the software Wolfram Mathematica.

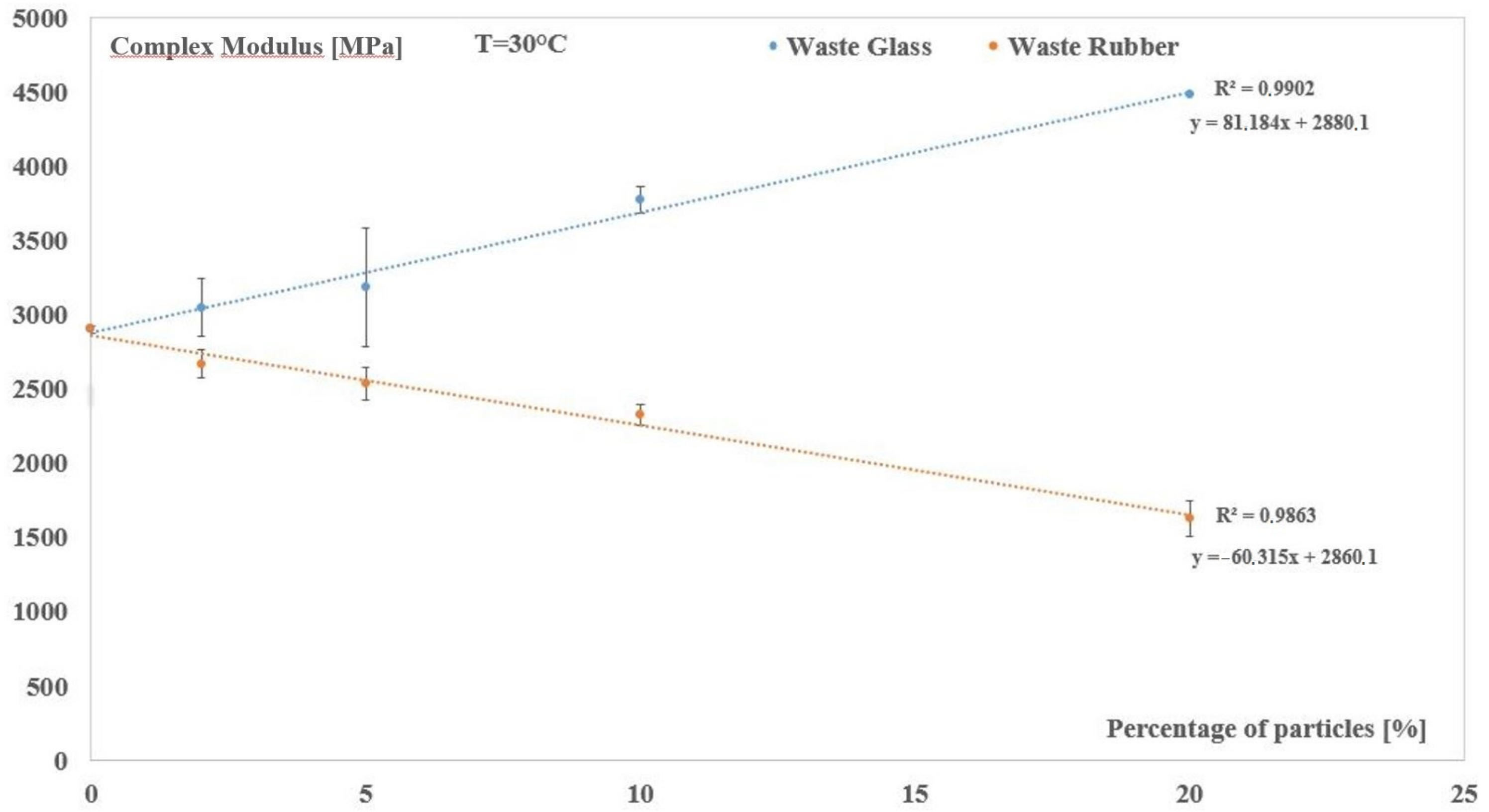
2.3.2. Dynamic Mechanical Thermal Analysis (DMTA)

The dynamic mechanical thermal test on the materials obtained for the different compositions was performed obtaining the Complex Young Modulus $E\left(T\right)$, being $T[{}^{\circ }$C] the temperature of the test. The Storage Modulus $E^{\prime }\left(T\right)=\Re e\left(E\left(T\right)\right)$, the Loss Modulus $E^{\prime \prime }\left(T\right)=\Im m\left(E\left(T\right)\right)$, and the ratio $E^{\prime \prime }\left(T\right)/E^{\prime }\left(T\right)=tan\delta$ [27].

Tan$\delta$ peak is the most common method used to calculate the glass transition temperature (Tg) that is defined as the temperature corresponding to the maximum of the $tan\delta$ curve. It is considered as the midpoint between the glassy and rubbery state. As ascertained in previous studies, for a polymer (thermoplastic or thermosetting) material, $tan\delta$ curve, and transition temperature are characterized by the sliding capacity of the macromolecules, which is deeply influenced by the quality of the filler-matrix interface [28]. A weak filler-matrix adhesion leads to higher values of the $tan\delta$ peak and lower Tg values, while a good filler-matrix adhesion hinders the mobility of the polymer chains delaying the glass transition.

Dynamic mechanical thermal tests were performed in tensile mode using a Metravib DMA$150$. These were carried out at a single frequency of 1 Hz, applying a temperature ramp from 25${}^{\circ }$C to 130${}^{\circ }$C with a gradient of 3${}^{\circ }$C/min. The distance between grips was 30 mm while the imposed displacement was set to 10 $\mathsf{\mu }$m in the tension-compression mode. At least three specimens for each type were tested [26].

3. Fractional Viscoelastic Model

The spring-pot model was used as the analytical model [20]. This model was already demonstrated to well fit with the experimental results obtained by Cataldo et al. for stress relaxation tests performed on giant reed stems in the TPB mode [29]. It assesses that the Young Modulus, which defines the constitutive model, is a time dependent fractional coefficient power law as reported in Equation (2):

$$E\left(t\right)=\frac{C_{\beta }}{\Gamma (1-\beta )}{t}^{-\beta };0\le \beta \le 1$$

(2)

in which $E\left(t\right)$ is the relaxation function, $\Gamma (·)$ is the Euler Gamma function, t is the relaxation time, while $\beta$ and $C_{\beta }$ are the two parameters, obtained from the best fitting of the experimental data, which entirely characterize the viscoelastic behavior of the material. In particular, for $\beta =0$ the behavior of the material is perfectly elastic, while for $\beta =1$, it is perfectly viscous. The parameter $C_{\beta }$ is a function of the imposed maximum strain ${ϵ}_0$, $\beta$, the time t, and the cross section of the sample. The real experimental tests cannot be performed by imposing a unit step function $U\left(t\right)$, but it is an initial ramp that usually is not taken into account during the best fitting. It was demonstrated that, especially for polymers, the initial ramp (usually a linear one) had a strong influence on the response. For this motivation, the best fitting of the experimental results was evaluated using the following Equation (3), which also takes into account the loading ramp:

$$E\left(t\right)=\frac{C_{\beta }}{\Gamma (2-\beta )}\left[\frac{t^{1-\beta }-{(t-t_0)}^{1-\beta }U(t-t_0)}{t_0}\right]$$

(3)

where $t_0$ is the loading time and $U(t-t_0)$ is the unit step function ($U\left(t\right)=1\forall t\ge 0,U\left(t\right)=0\forall t<0$)

As the relaxation was defined by Equation (2), the corresponding creep function, labeled as $J\left(t\right)$, is easily derived as:

$$J\left(t\right)=\frac{1}{C_{\beta }\Gamma (1-\beta )}{t}^{\beta }$$

(4)

by using the Boltzmann superposition principle the stress and strain can be evaluated in the form:

$$\sigma \left(t\right)=C_{\beta }{(}_0^C{D}_t^{\beta }ϵ)\left(t\right);ϵ\left(t\right)=\frac{1}{C_{\beta }}{(}_0{I}_t^{\beta }\sigma )\left(t\right)$$

(5)

where ${(}_0^C{D}_t^{\beta }f)\left(t\right)$ is the so called Caputo’s fractional derivative defined as:

$${(}_0^C{D}_t^{\beta }f)\left(t\right)=\frac{1}{\Gamma (1-\beta )}{\int }_0^t{(t-\tau )}^{-\beta }\dot{f}\left(\tau \right)d\tau$$

(6)

and ${(}_0{I}_t^{\beta }f)\left(t\right)$ is the Riemann–Liouville fractional integral defined as:

$${(}_0{I}_t^{\beta }f)\left(t\right)=\frac{1}{\Gamma \left(\beta \right)}{\int }_0^t{(t-\tau )}^{\beta -1}f\left(\tau \right)d\tau$$

(7)

Equations (5) are the linear fractional viscoelastic constitutive laws.

4. Results and Discussions

The parameters $\beta$ and $C_{\beta }$ were obtained from the best fitting between the experimental relaxation data and the fractional spring-pot model. Figure 1 shows, as an example, the best fitting for 20% glass powder composites and 20% rubber powder composites.

The overall results of $\beta$ and $C_{\beta }$ for the neat resin and the composites with both waste glass (W.G) and waste rubber (W.R.) additives at the different percentages are reported in

Table 2. Stress relaxation results.

Sample	$\mathit{\beta }$	${\mathit{C}}_{\mathit{\beta }}$ [$\mathbf{MPa}*{\mathit{s}}^{-\mathit{\beta }}$]
Neat	0.0607 ± 0.0018	3628 ± 174
W.G. 2%	0.0504 ± 0.0040	3643 ± 40
W.G. 5%	0.0469 ± 0.0027	4030 ± 120
W.G. 10%	0.0416 ± 0.0026	5053 ± 173
W.G. 20%	0.0412 ± 0.0056	5810 ± 280
W.R. 2%	0.0050 ± 0.0016	3346 ± 166
W.R. 5%	0.0525 ± 0.0034	3127 ± 60
W.R. 10%	0.0512 ± 0.0062	2933 ± 159
W.R. 20%	0.0706 ± 0.0016	2012 ± 123

as average and standard deviation.

The $\beta$ values for both types of blends are reported as a function of the powder content in Figure 2.

A different viscoelastic behavior for the two types of materials investigated can be observed. In particular, for epoxy resin modified with glass particles, $\beta$ decreased in function of the weight content until approximately 10%, which can be considered a plateau value. In regard to the polymers with waste rubber additives, there was a slight decrease of the coefficient $\beta$ in function of the particle content until approximately 10% followed by a significant increase for higher percentage loaded composites. Below this value, it is dominated by the polymer properties, while beyond this limit, the rubbery nature of the particles prevails over the matrix and the material exhibits a more viscous behavior. In regard to the parameter $C_{\beta }$, it mostly influences the material elasticity, as can be seen in Figure 3, it experimented a linear trend for both typologies of fillers analyzed. In particular, glass particles led to an upward trend of the coefficient in the function of the percentage, whereas the rubber powders revealed the opposite effect. These considerations can be better highlighted and corroborated considering the results of the dynamic–mechanical thermal test.

Figure 4 shows the typical dynamic–mechanical thermal behavior of neat, waste glass 10 % (W.G. 10 %) and waste rubber 10 % (W.R. 10 %) composites. It is possible to notice that the addition of waste glass particles leads to the increase of both the storage and the loss modulus throughout the temperature ramp compared to the neat resin. Moreover, the shift to the right of the tan $\delta$ curve, as well as its lower peak, compared to the neat resin, confirms the positive effect of the glass powders to increase the glass transition temperature of the neat resin. On the other hand, opposite trends were experimented for the composites with waste rubber particles.

The overall results obtained from the DMTA test are reported in

Table 3. DMTA Experimental results.

Sample	${\mathit{E}}_{{30}^{\circ }\mathbf{C}}$ [MPa]	${\mathit{E}}_{{30}^{\circ }\mathbf{C}}^{\prime }$ [MPa]	${\mathit{E}}_{{30}^{\circ }\mathbf{C}}^{\prime \prime }$ [MPa]	$\mathbf{Tg}$ [${}^{\circ }\mathbf{C}$]	$\mathbf{tan}\mathit{\delta }$
Neat	2905 ± 24.7	2905 ± 21.21	84.8 ± 3.11	81.3 ± 0.035	1.187 ± 0.014
W.G. 2%	3050 ± 191	3060 ± 212.1	93.6 ± 4.95	83.1 ± 0.283	1.206 ± 0.011
W.G. 5%	3190 ± 400	3185 ± 397.1	105.2 ± 14.1	82 ± 0.775	1.180 ± 0.030
W.G. 10%	3770 ± 91.7	3770 ± 84.8	107.4 ± 22.1	83.4 ± 0.707	1.160 ± 0.009
W.G. 20%	4490 ± 4.69	4485 ± 7.07	147.5 ± 4.95	80.3 ± 0.177	1.077 ± 0.012
W.R. 2%	2670 ± 96.6	2670 ± 95.4	71.1 ± 4.12	80.9 ± 0.679	1.229 ± 0.007
W.R. 5%	2535 ± 112	2535 ± 106.9	68.4 ± 7.36	79.0 ± 0.278	1.179 ± 0.021
W.R. 10%	2330 ± 68.8	2325 ± 70.2	61.3 ± 2.45	76.9 ± 0.626	1.176 ± 0.002
W.R. 20%	1630 ± 120	1630 ± 121.0	37.0 ± 5.95	78.8 ± 0.375	1.056 ± 0.012

.

The addition of waste glass powder leads to a rise of the Tg, which is the temperature at which a significant increase in the mobility of the polymeric chains occurs, whereas rubber particles tend to anticipate the mobility of the polymeric chains and, hence, the Tg decreases. The trends of the Tg values of both types of blends are reported in function of the percentage of particles in Figure 5.

The dynamic complex modulus of the composites at the temperature of 30${}^{\circ }$C is reported in function of the waste particles percentage in Figure 6. For the composites with waste glass additives, the linear upward trend is evident, reaching approximately 4.5 GPa for W.G. 20%. By contrast, a downward trend characterized the waste rubber composites where the complex modulus was about 1.60 GPa at the maximum particles content considered.

Referring to the composites filled with waste glass particles, from the $tan\delta$ curves, it can be assessed that the Tg increases with the weight content from 81.3${}^{\circ }$C of the neat, to 83.4${}^{\circ }$C of W.G. 10%. This increase may be justified considering the downward trend of the $tan\delta$ peak in function of the waste particles percentage. Fiore et al. found similar results for an epoxy resin with plasma-treated marble powders. The decrease in $tan\delta$ peak was attributed to the improvement of the interaction between the matrix and treated disperse particles, which led to a higher glass transition temperature [28]. The dynamic behavior of particulate composites with waste rubber is different compared to the composites with glass powder additives previously analyzed. In fact, a significant decrease in the value of Tg, from 81.3${}^{\circ }$C of the Neat to 76.9${}^{\circ }$C of W.R. 10% was reported, despite a good interaction between particles and matrix being evidenced by the lower values of the $tan\delta$ peaks. This can be explainable by the negative glass transition temperature of the rubber particles (−70 $\pm 20{}^{\circ }$C), which consequently leads to lower Tg values of the composites.

The previous considerations can be confirmed by the observation of bending fractured surfaces of the samples at the scanning electron microscope (SEM), shown in Figure 7. These have evidenced a rather better glass particle/matrix interface for the W.G. 5% composites compared to the W.G. 20% ones. The latter, in fact, evidenced more presence of glass particle to particle interface rather than particle to matrix interface.

In regard to the W.R. composites, SEM observations, shown in Figure 8, revealed a rather better rubber particle/matrix interface for the W.R. 5% composites compared to the W.R. 20% ones. The latter, in fact, evidenced more presence of rubber particle to particle interface rather than particle to matrix interface, as well as the presence of voids.

5. Conclusions

This study aimed to characterize the viscoelastic and dynamic behavior of an epoxy resin modified with waste glass and rubber particles. Using an easy analytical fractional model, it is possible to evaluate the viscoelastic behavior of this type of material. Best fitting of the experimental data were performed through the spring-pot model. This fits the experimental stress–relaxation results by means of only two parameters $\beta$ and $C_{\beta }$. A good interaction between matrix and waste particles was evidenced up to 10% by weight. In the experimental campaign, some very interesting effects were detected: (i) the parameters $C_{\beta }$ and $\beta$ strongly depend on the percentage of the disperse waste powders; (ii) the complex modulus has a linear trend with the percentage of the disperse waste; (iii) As expected, as the percentage of the powders increase, two different behaviors may be evidenced. In particular, by adding glass, $\beta$ decrease ($\beta =0$ purely elastic behavior) while, by increasing the rubber powder content, $\beta$ increased ($\beta =1$ purely viscous). Using waste materials as additives for polymer compounds is a practical and sustainable possibility when it comes to modifying their viscoelastic properties. Further research should investigate the influence of the particle size on the viscoelastic properties of this type of material.