An Experimental Investigation of Water Vapor Condensation from Biofuel Flue Gas in a Model of Condenser, (2) Local Heat Transfer in a Calorimetric Tube with Water Injection
Abstract
:1. Introduction
2. Experimental Setup
3. Materials and Methods
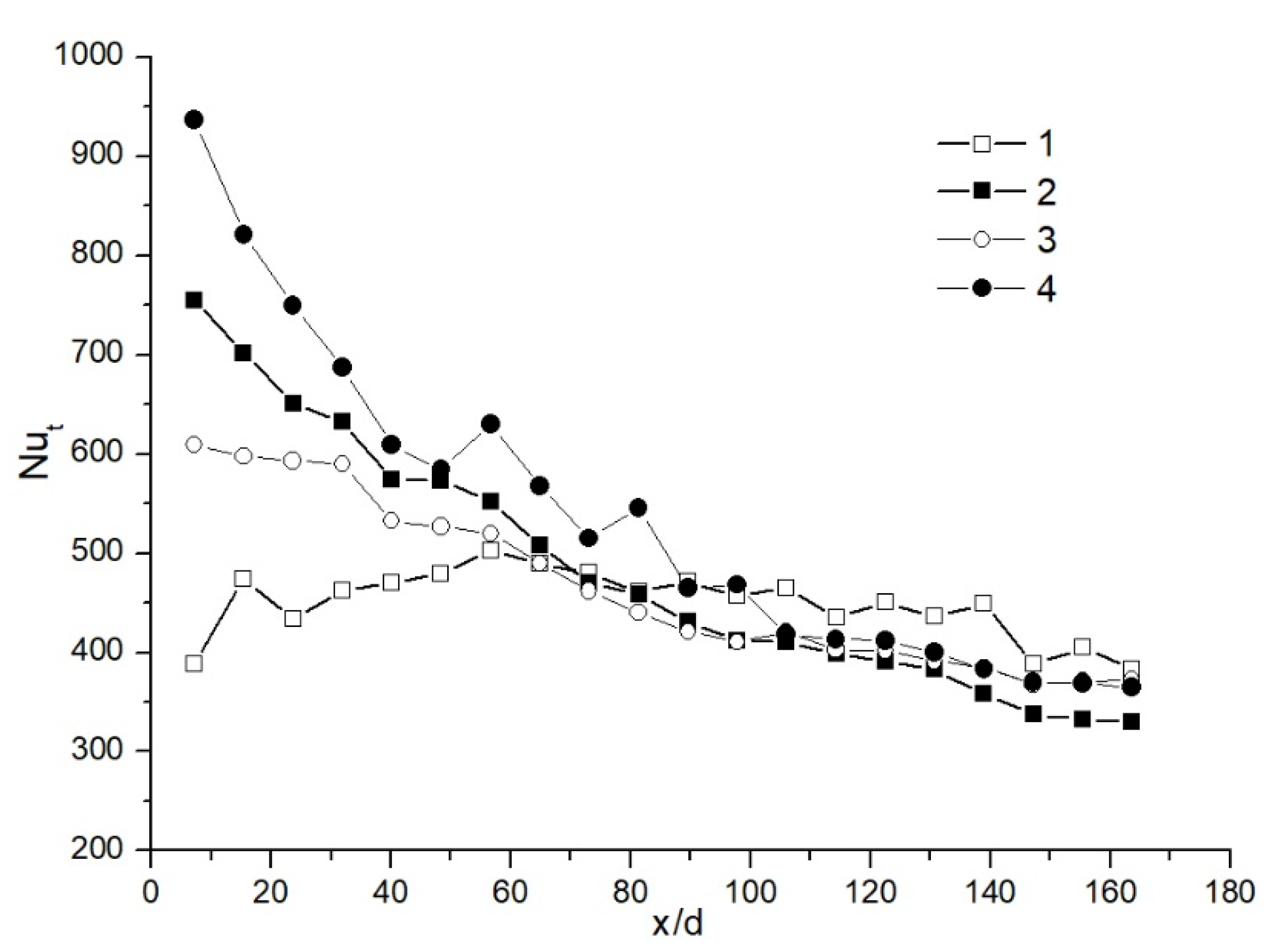
4. Results and Discussions
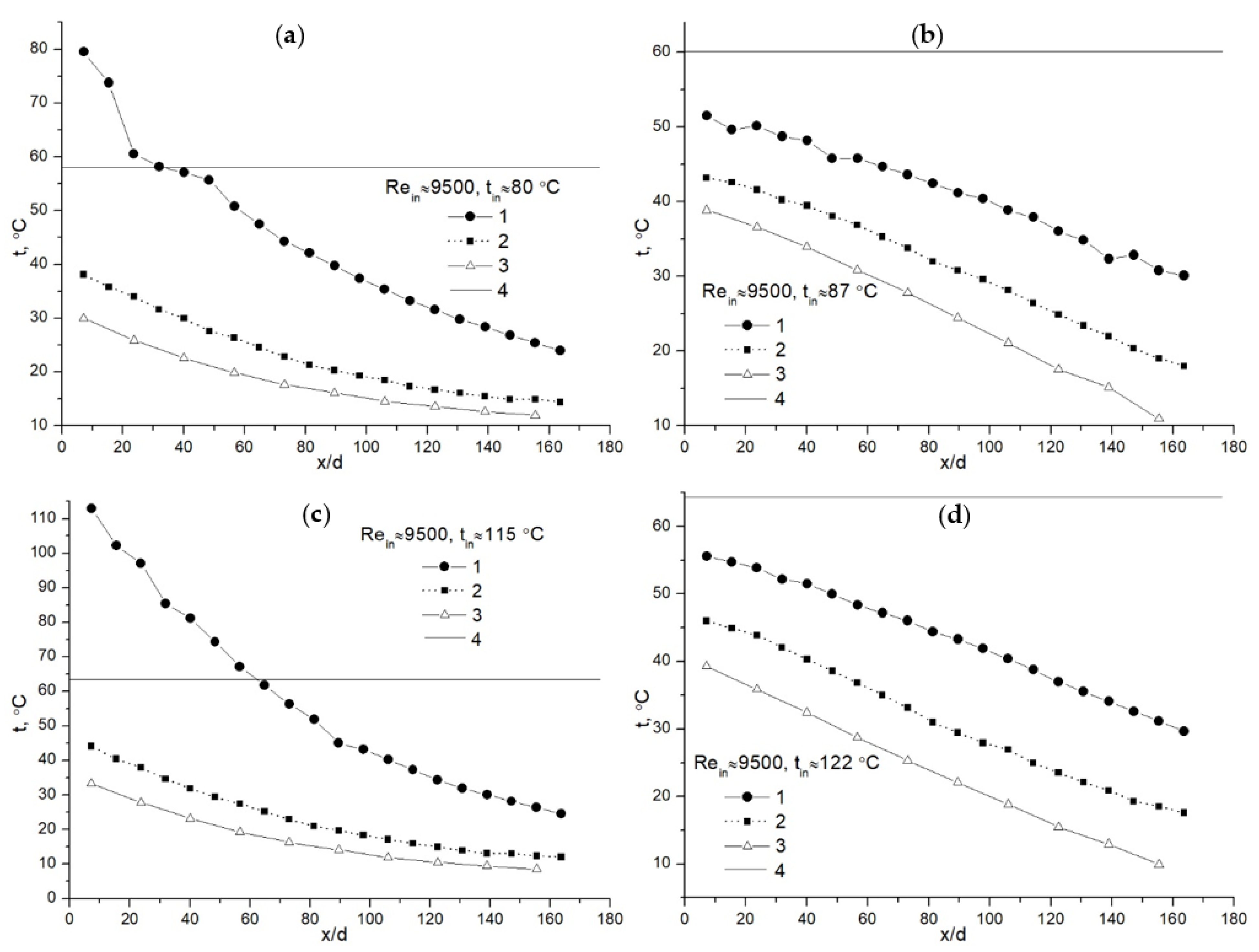
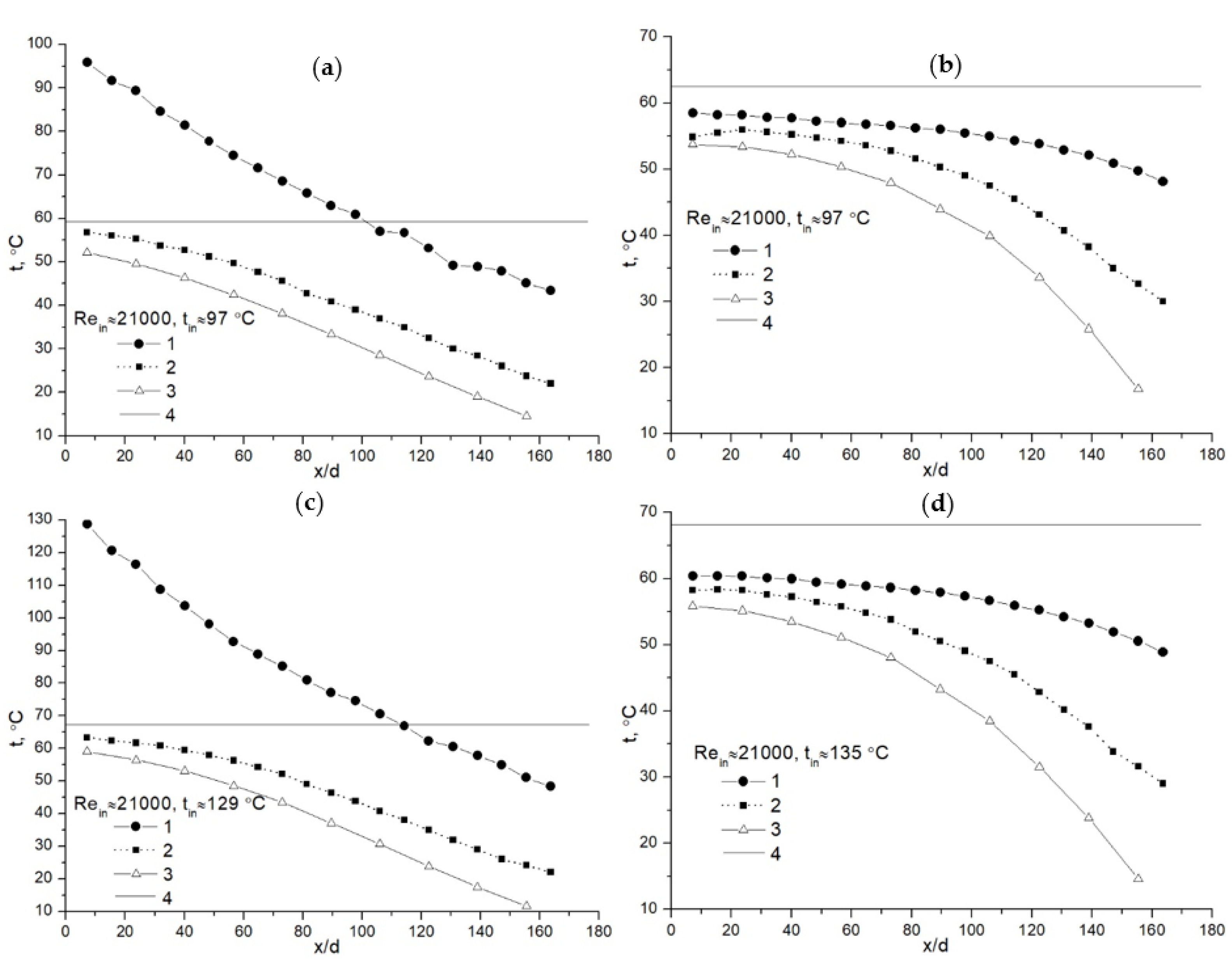
4.1. Water Injection with a Temperature of 25 °C
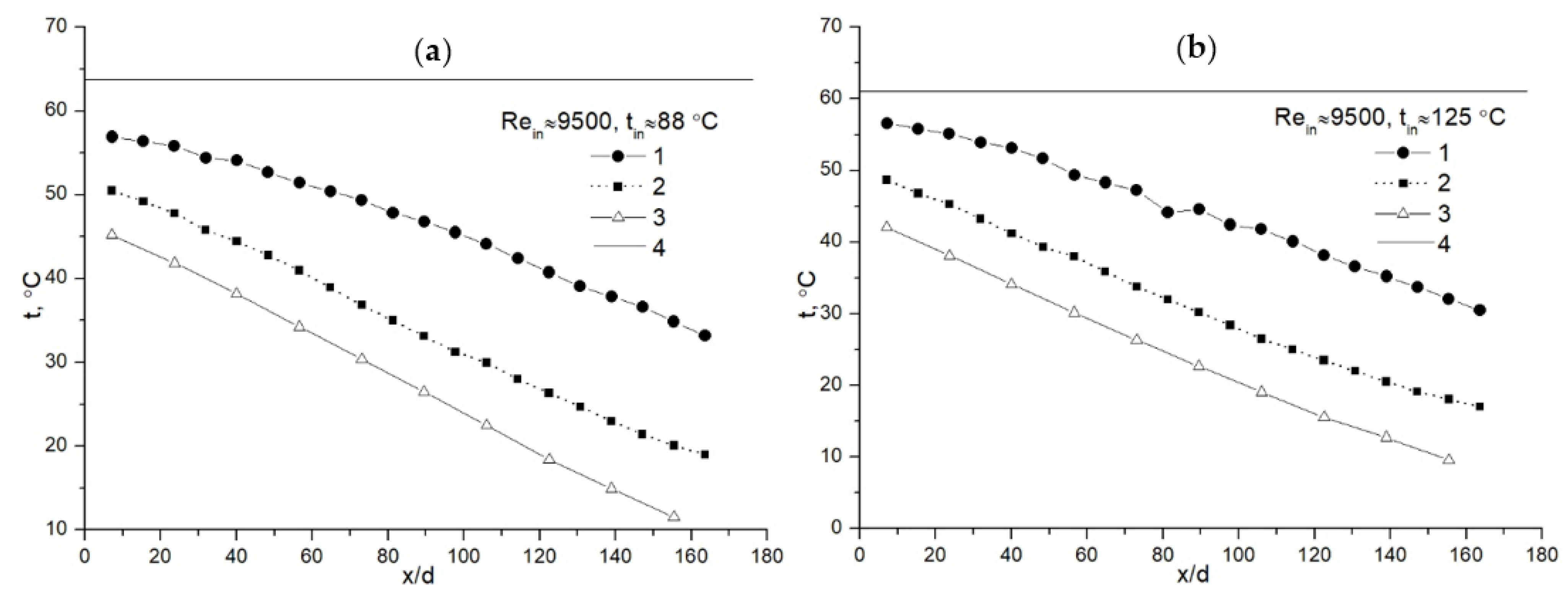
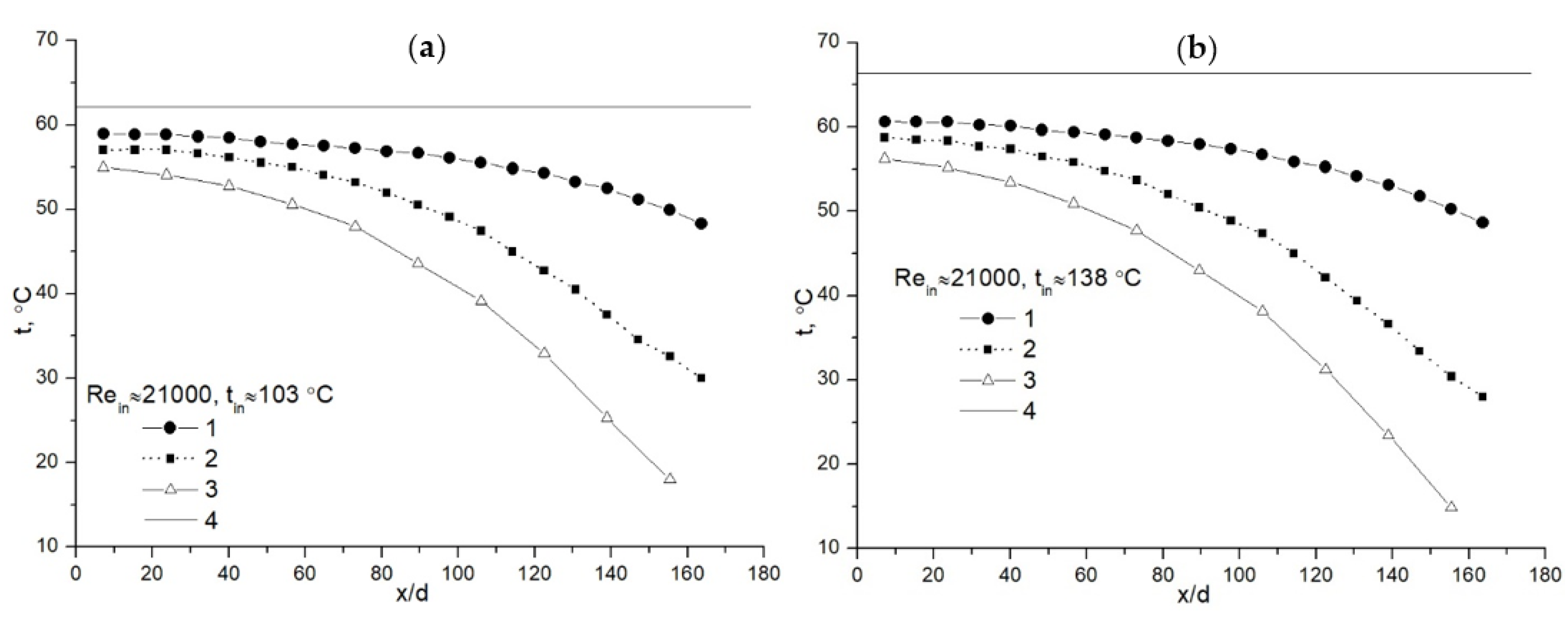
4.2. Water Injection with a Temperature of 40 °C
4.3. Comparison of Heat Transfer Results for Different Injected Water Temperatures
5. Conclusions
- Performed investigations revealed the regularities of the local heat transfer during operation of condensing heat exchangers with water injection.
- Water injection drastically changes the distribution of temperatures and has a significant effect on heat transfer along the calorimetric tube.
- In the case of the water injection with a temperature of 25 °C, at lower Rein numbers, the local total heat transfer along the tube increased by at least four times, and at higher Rein numbers, at least by two times in comparison with the case without water injection.
- In the case of water injection with a temperature of 40 °C, at lower Rein numbers, the local total heat transfer increased by at least 2.3 times and at higher Rein numbers, by at least 1.7 times in comparison with the case without water injection.
- At higher flue gas inlet temperatures, the effect of water injection on heat transfer is also stronger. For lower Rein, the effect is more pronounced in the initial part of the tube (up to x/d ≈ 60), and for higher Rein, it is in the x/d range between 20 and 110.
- Condensation efficiency increases with decreasing Rein number, flue gas temperature and injected water temperature.
- To optimize the operation of condensing heat exchangers with water injection, it is necessary to perform wider investigations on the effect of different flue gases, cooling water and injected water parameters.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Poskas, R.; Sirvydas, A.; Kulkovas, V.; Poskas, P. An Experimental investigation of water vapor condensation from biofuel flue gas in a model of vertical condensing heat exchanger. 1. Base case: Local heat transfer in a calorimetric tube without water injection. Processes 2021, 9, 844. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, F.; Ma, X. Industrial application of a deep purification technology for flue gas involving phase-transition agglomeration and dehumidification. Engineering 2018, 4, 416–420. [Google Scholar] [CrossRef]
- Xia, J.L.; Järvi, J.; Nurminen, E.; Peuraniemi, E.; Gasik, M. Cooling of gas by a water spray in a straight duct—cross flow. In Proceedings of the 10th International Congress on Liquid Atomization and Spray Systems, ICLASS-2006, Kyoto, Japan, 27 August–1 September 2006. [Google Scholar]
- Priedniecea, V.; Kirsanovsa, V.; Dzikēvičs, M.; Vīgants, G.; Veidenbergs, I.; Blumberga, D. Experimental and analytical study of the flue gas condenser—fog unit. Energy Procedia 2019, 158, 822–827. [Google Scholar] [CrossRef]
- Cao, R.; Ruan, R.; Tan, H.; Bai, S.; Du, Y.; Liu, H. Condensational growth activated by cooling method for multi-objective treatment of desulfurized flue gas: A full-scale study. Chem. Eng. J. 2021, 410, 128296. [Google Scholar] [CrossRef]
- Egilegor, B.; Jouhara, H.; Al-Mansour, F.; Plesnik, K.; Montorsi, L.; Manzini, L. ETEKINA: Analysis of the potential for waste heat recovery in three sectors: Aluminium low pressure die casting, steel sector and ceramic tiles manufacturing sector. Int. J. Thermofluids 2020, 1–2, 100002. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, L.; Wua, H.; Yanga, H. Promoting fine particle removal in double-tower cascade wet flue gas desulfurization system by flue gas temperature reduction. Chem. Eng. J. 2020, 373, 581–589. [Google Scholar] [CrossRef]
- Gimbutaite, I.; Venckus, Z. Air pollution burning different kinds of wood in small power boilers. J. Environ. Eng. Landsc. Manag. 2008, 16, 97–103. [Google Scholar] [CrossRef]
- Wang, H.; Li, B.; Liu, S.; Pan, Z.; Yan, G. The effect of water spray upon incineration flue gas clean-up. J. Therm. Sci. 2000, 9, 182–186. [Google Scholar] [CrossRef]
- Poullikkas, A. Review of Design, Operating, and Financial Considerations in Flue Gas Desulfurization Systems. Energy Technol. Policy 2015, 2, 92–103. [Google Scholar] [CrossRef]
- Liua, D.; Jina, J.; Gaoa, M.; Xionga, Z.; Stangerb, R.; Wall, T. A comparative study on the design of direct contact condenser for air and oxy-fuel combustion flue gas based on Callide Oxy-fuel Project. Int. J. Greenhouse Gas Control 2018, 75, 74–84. [Google Scholar] [CrossRef]
- Terhan, M.; Comakli, K. Design and economic analysis of a flue gas condenser to recover latent heat from exhaust flue gas. Appl. Therm. Eng. 2016, 100, 1007–1015. [Google Scholar] [CrossRef]
- Venturelli, M.; Brough, D.; Milani, M.; Montorsi, L.; Jouhara, H. Comprehensive numerical model for the analysis of potential heat recovery solutions in a ceramic industry. Int. J. Thermofluids 2021, 10, 100080. [Google Scholar] [CrossRef]
- Sideman, S.; Moalem-Maron, D. Direct Contact Condensation. Adv. Heat Transf. 1982, 15, 227–281. [Google Scholar] [CrossRef]
- Takahashi, M.; Nayak, A.K.; Kitagawa, S.I.; Murakoso, H. Heat transfer in direct contact condensation of steam to subcooled water spray. J. Heat Transf. 2001, 123, 703–710. [Google Scholar] [CrossRef]
- Lee, S.Y.; Tankin, R.S. Study of liquid spray (water) in a condensable environment (steam). Int. J. Heat Mass Transf. 1984, 27, 363–374. [Google Scholar] [CrossRef]
- Chung, J.N.; Chang, T.H. A mathematical model of condensation heat and mass transfer to a moving droplet in its own vapor. J. Heat Transf. 1984, 6, 417–424. [Google Scholar] [CrossRef]
- Weinberg, S. Heat transfer to low pressure sprays of water steam atmosphere. Environ. Sci. 1953, 167, 240–258. [Google Scholar] [CrossRef]
- Choon, L.H.; Hwan, K.M.; Ki, P.S. The effect of non-condensable gas on direct contact condensation of steam/air mixture. Nucl. Eng. Technol. 2001, 33, 585–595. [Google Scholar]
- Gümrük, S.; Aktaş, M.K. Experimental Study of Direct Contact Condensation of Steam on Water Droplets. In Proceedings of the World Congress on Engineering 2015, London, UK, 1–3 July 2015. [Google Scholar]
- Long, P.; Li, Z.; Li, M. Numerical simulation study of quench tower in flue gas purification system. IOP Conf. Ser. Earth Environ. Sci. 2020, 569, 012028. [Google Scholar] [CrossRef]
- Ye, W.; Zhang, Q.; Xie, Y.; Cai, J.; Zhang, X. Spray cooling for high temperature of exhaust gas using a nozzle array in a confined space: Analytical and empirical predictions on cooling capacity. Appl. Therm. Eng. 2017, 127, 889–900. [Google Scholar] [CrossRef]
- Tissot, J.; Boulet, P.; Labergue, A.; Castanet, G.; Trinquet, F.; Fournaison, L. Experimental study on air cooling by spray in the upstream flow of a heat exchanger. Int. J. Therm. Sci. 2012, 60, 23–31. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.W.; Yang, C.Y.; Hu, Y.T. Heat Transfer Enhancement of Spray Cooling on Flat Aluminum Tube Heat Exchanger. Heat Transf. Eng. 2013, 34, 29–36. [Google Scholar] [CrossRef]
- Thulukkanam, K. Heat Exchanger Design Handbook, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Siddique, M. The Effects of Noncondensable Gases on Steam Condensation under Forced Convection Conditions. Ph.D. Thesis, Department of Nuclear Engineering, Massachusetts Institute of Technology, Cambridge, MA, USA, 1992. [Google Scholar]
- Jeong, K.; Kessen, M.J.; Bilirgen, H.; Levy, E.K. Analytical modelling of water condensation in condensing heat exchanger. Int. J. Heat Mass Transf. 2010, 53, 2361–2368. [Google Scholar] [CrossRef]
- Barford, N.C. Experimental Measurements: Precision, Error and Truth, 2nd ed.; John Willey & Sons: Hoboken, NJ, USA, 1985. [Google Scholar]
- American Meteorological Society. Available online: https://journals.ametsoc.org/doi/pdf/10.1175/BAMS-86-2-225 (accessed on 18 May 2021).






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Poškas, R.; Sirvydas, A.; Kulkovas, V.; Jouhara, H.; Poškas, P.; Miliauskas, G.; Puida, E. An Experimental Investigation of Water Vapor Condensation from Biofuel Flue Gas in a Model of Condenser, (2) Local Heat Transfer in a Calorimetric Tube with Water Injection. Processes 2021, 9, 1310. https://doi.org/10.3390/pr9081310
Poškas R, Sirvydas A, Kulkovas V, Jouhara H, Poškas P, Miliauskas G, Puida E. An Experimental Investigation of Water Vapor Condensation from Biofuel Flue Gas in a Model of Condenser, (2) Local Heat Transfer in a Calorimetric Tube with Water Injection. Processes. 2021; 9(8):1310. https://doi.org/10.3390/pr9081310
Chicago/Turabian StylePoškas, Robertas, Arūnas Sirvydas, Vladislavas Kulkovas, Hussam Jouhara, Povilas Poškas, Gintautas Miliauskas, and Egidijus Puida. 2021. "An Experimental Investigation of Water Vapor Condensation from Biofuel Flue Gas in a Model of Condenser, (2) Local Heat Transfer in a Calorimetric Tube with Water Injection" Processes 9, no. 8: 1310. https://doi.org/10.3390/pr9081310
APA StylePoškas, R., Sirvydas, A., Kulkovas, V., Jouhara, H., Poškas, P., Miliauskas, G., & Puida, E. (2021). An Experimental Investigation of Water Vapor Condensation from Biofuel Flue Gas in a Model of Condenser, (2) Local Heat Transfer in a Calorimetric Tube with Water Injection. Processes, 9(8), 1310. https://doi.org/10.3390/pr9081310






