Effect of Tip Clearance on Helico-Axial Flow Pump Performance at Off-Design Case
Abstract
:1. Introduction
2. Physical Model of a Helico-Axial Flow Pump
3. Helico-Axial Flow Pump Test Rig
4. Numerical Methods and Verification
4.1. Governing Equation
4.2. Mesh Arrangement
4.3. Mesh Independence Verification
4.4. Numerical Method and Setting
4.5. Numerical Validation
5. Results and Discussion
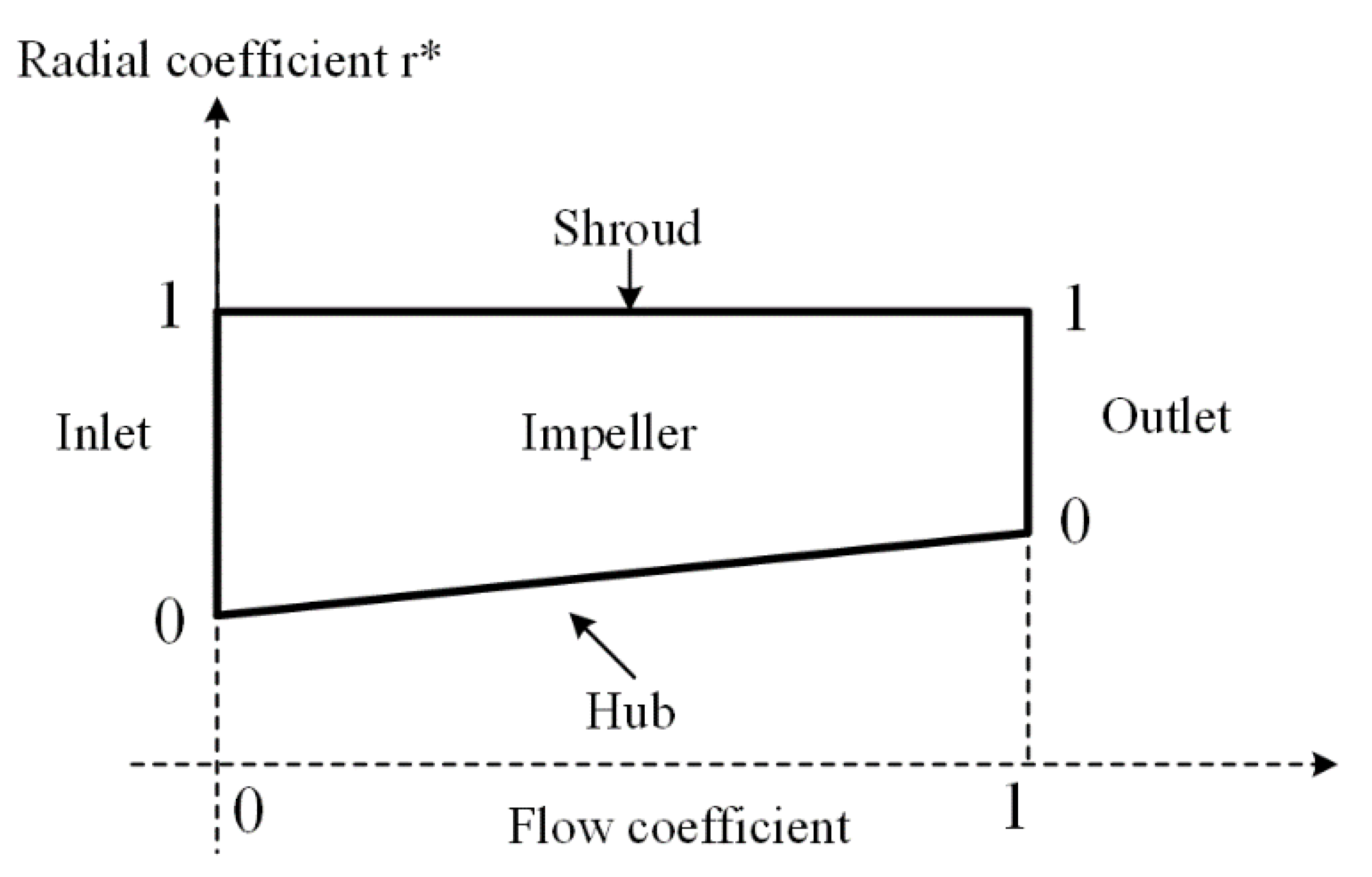
5.1. Parameter Definition
5.2. Helico-Axial Flow Pump Performance
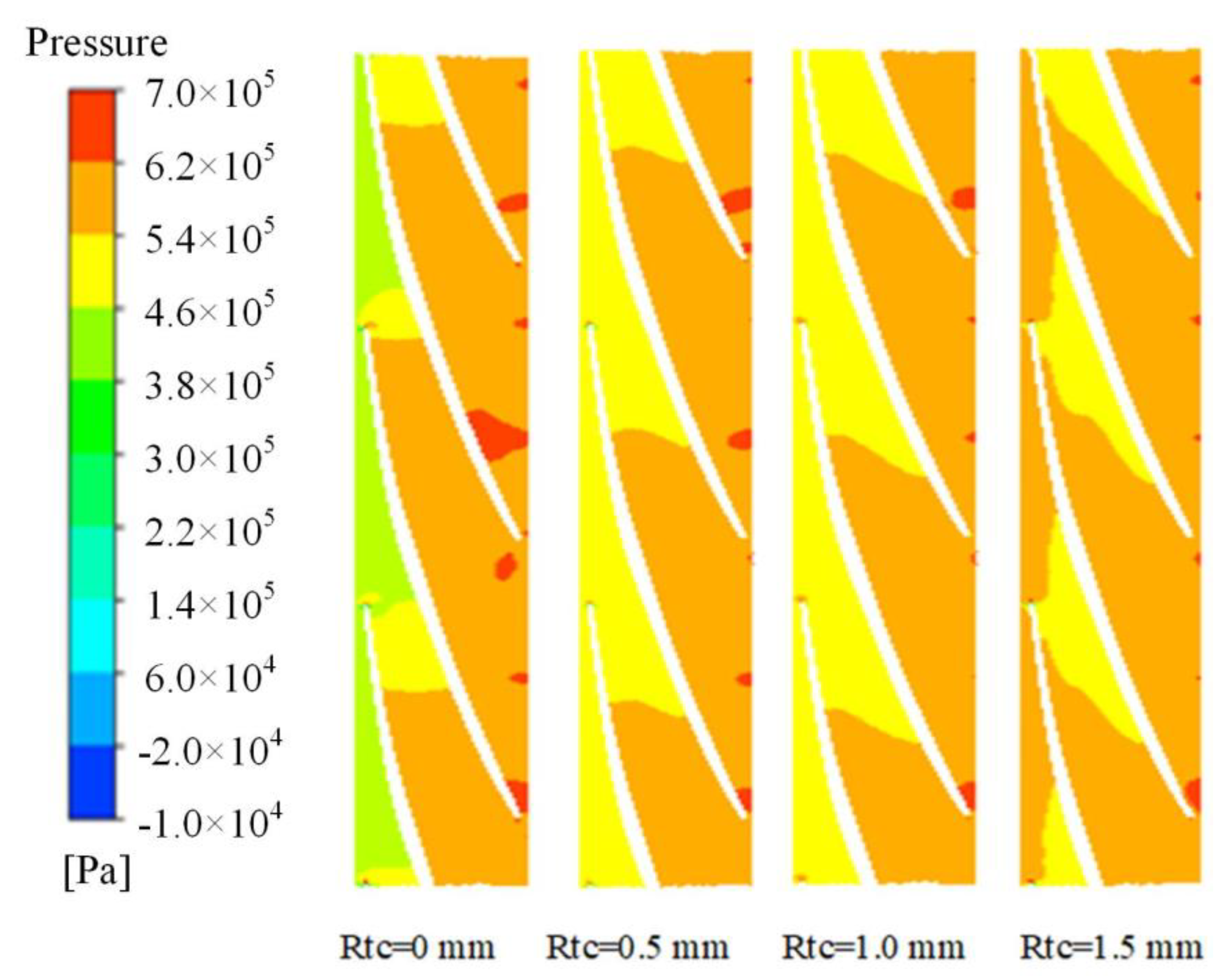
5.3. Pressure on Blade Surface
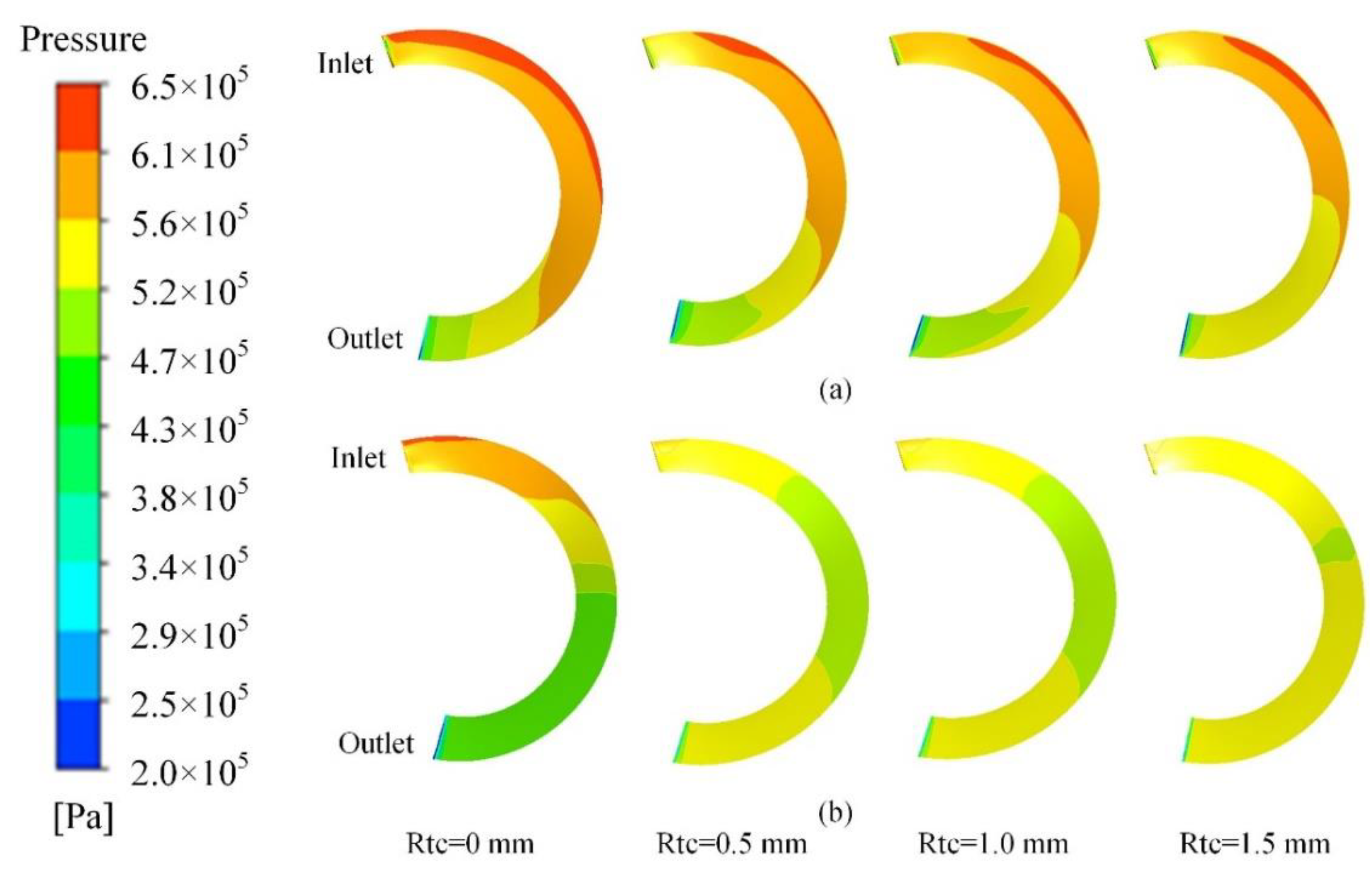
5.4. Impeller Radial Pressurization Performance along Flow Direction
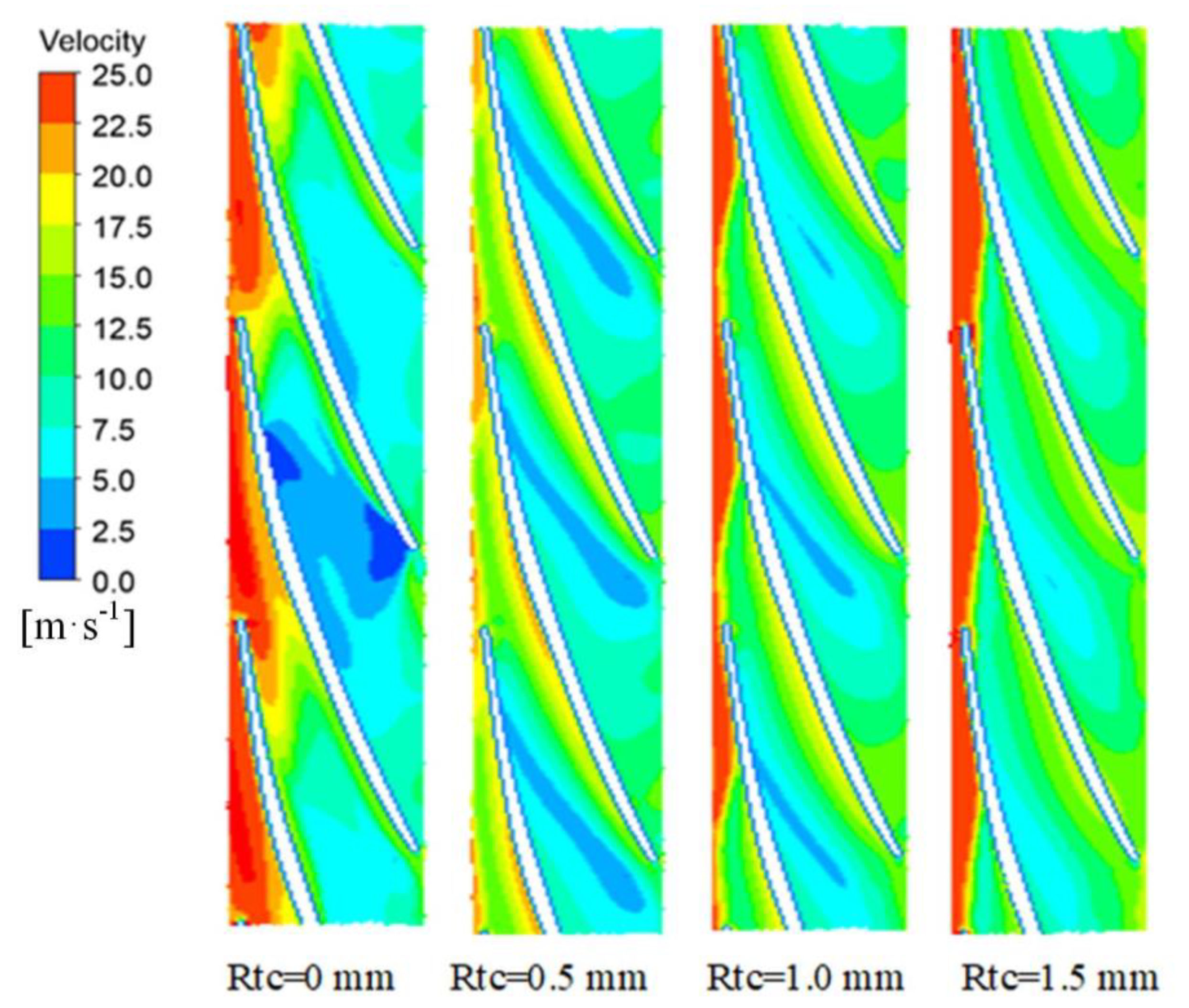
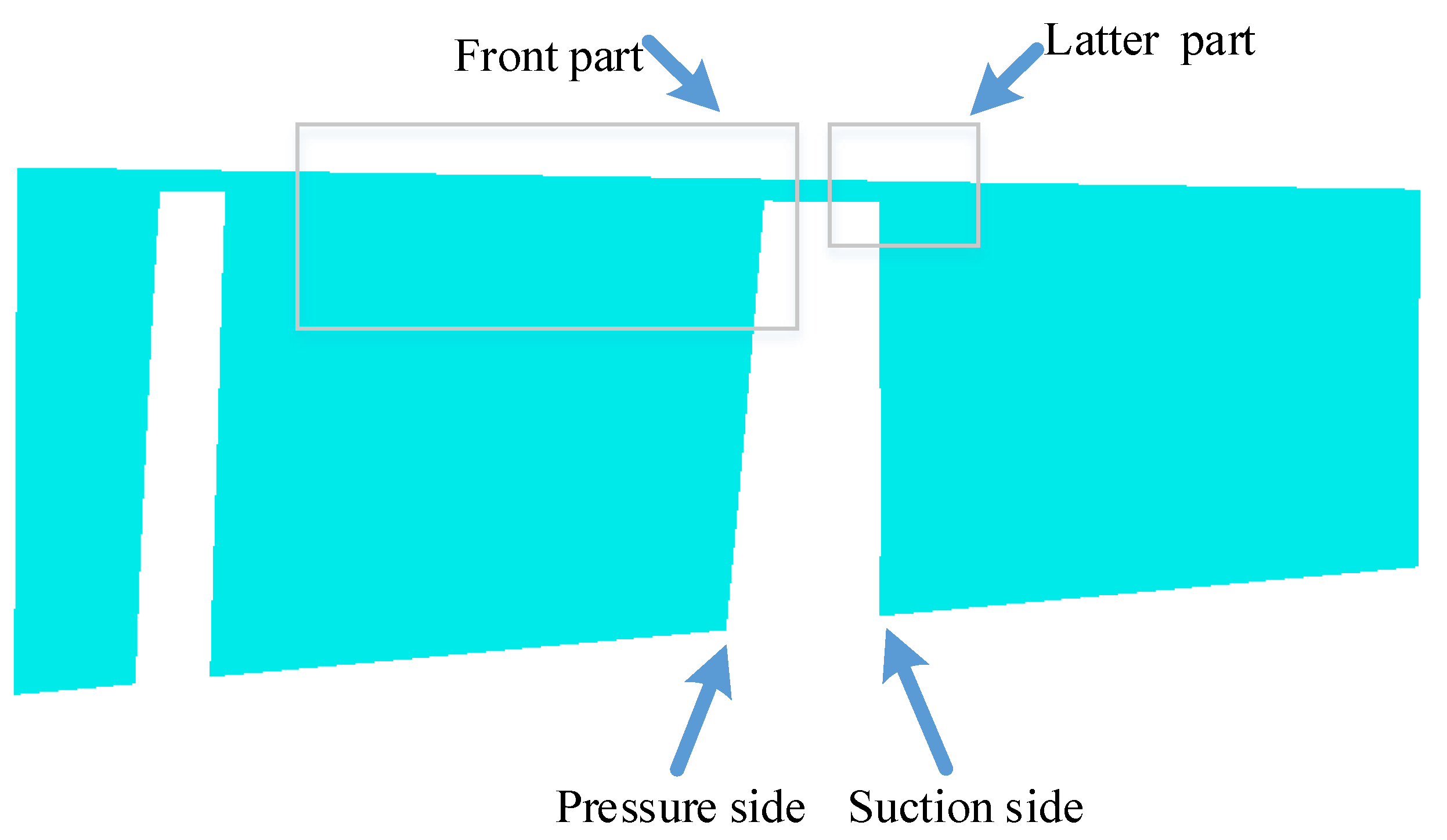
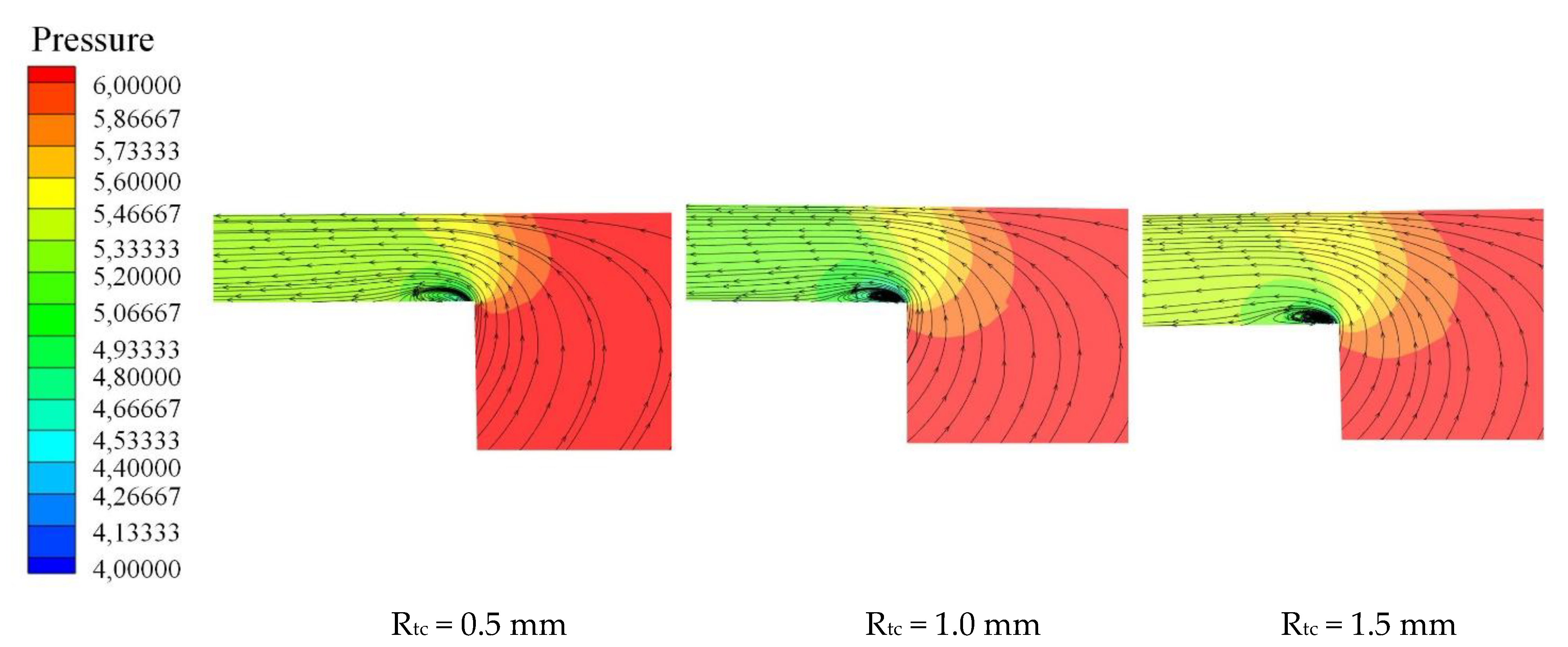
5.5. Pressure, Velocity, and Streamlines near the Blade Tip along the Circumferential Direction
5.6. Turbulent Kinetic Energy in the Impeller and Diffuser
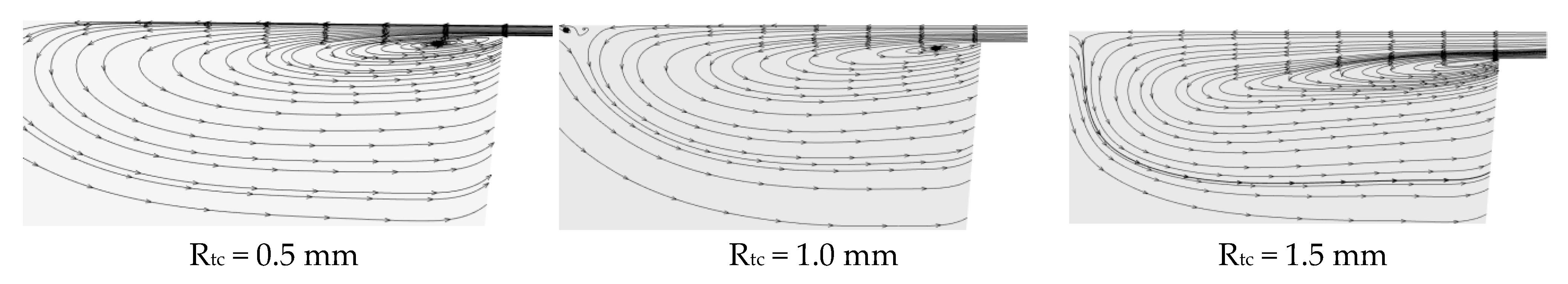
5.7. TLV Characteristics
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| BEP | Best efficiency point |
| CFD | Computational fluid dynamics |
| FVM | Finite volume method |
| IGVFs | Inlet gas void fractions |
| LE | Leading edge |
| PIV | Particle image velocimetry |
| PS | Pressure side |
| RANS | Reynolds-averaged Navier–Stokes equations |
| RMS | Root mean square |
| SS | Suction side |
| TE | Trailing edge |
| TLF | Tip leakage flow |
| TLV | Tip leakage vortex |
| TSV | Tip separation vortex |
| Symbols | |
| r* | Radial coefficient |
References
- Kim, J.; Lee, H.; Yoon, J.; Lee, K.; Lee, Y.; Choi, Y. Multi objective optimization of a multiphase pump for offshore plants. In Proceedings of the ASME 2014 4th Joint Us-European Fluids Engineering Division Summer Meeting, Chicago, IL, USA, 3–7 August 2014; pp. 1–7. [Google Scholar]
- Kim, J.; Lee, H.; Kim, J.; Choi, Y.; Yoon, J.; Yoo, I.; Choi, W. Improvement of hydrodynamic performance of a multiphase pump using design of experiment techniques. J. Fluids Eng. 2015, 137, 1–15. [Google Scholar]
- Zhang, J.; Cai, S.; Zhu, H.; Qiang, R. Experimental study of gas-liquid two-phase flow pattern in a helico-axial multipahse pump by visualization. J. Eng. Thermophys. 2015, 36, 1937–1941. [Google Scholar]
- Zhang, J.; Cai, S.; Zhu, H. Visualization test for flow field of gas-liquid two-phase in the entrance of rotodynamic multiphase pump. J. Mech. Eng. 2015, 51, 184–190. [Google Scholar]
- Shi, G.; Liu, Z.; Xiao, Y.; Li, H.; Liu, X. Effect of the inlet gas void fraction on the tip leakage vortex in a multiphase pump. Renew. Energy 2020, 150, 46–57. [Google Scholar] [CrossRef]
- Wang, L.; Lu, J.; Liao, W.; Zhao, Y.; Wang, W. Numerical simulation of the tip leakage vortex characteristics in a semi-open centrifugal pump. Appl. Sci. 2019, 9, 5244. [Google Scholar] [CrossRef] [Green Version]
- Miorini, R.; Wu, H.; Katz, J. The internal structure of the tip leakage vortex within the rotor of an axial waterjet pump. J. Turbomach. 2012, 134, 1–14. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Chang, H.; Yang, Z. Energy characteristics of mixed-flow pump under different tip clearances based on entropy production analysis. Energy 2020, 199, 117447. [Google Scholar] [CrossRef]
- Liu, Y.; Tan, L. Tip clearance on pressure fluctuation intensity and vortex characteristic of a mixed flow pump as turbine at pump mode. Renew. Energy 2018, 129, 606–615. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, L.; Chen, J.; Pan, Q.; Shi, W. Experimental analysis on characteristic of cavitation in tip region of axial flow pump impeller. J. Zhejiang Univ. (Eng. Sci.) 2016, 50, 1585–1592. [Google Scholar]
- Li, Y.; Hu, P.; Li, R.; Li, P.; Bi, Z. Numerical analysis for effects of different blade tip clearance on performance in mixed-flow pump. Trans. Chin. Soc. Agric. Eng. 2014, 30, 86–93. [Google Scholar]
- Zhang, J.; Fan, H.; Zhang, W.; Xie, Z. Energy performance and flow characteristics of a multiphase pump with different tip clearance sizes. Adv. Mech. Eng. 2019, 11, 1–14. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, W.; Van Esch, B.; Shi, L.; Dubuisson, M. Numerical and experimental investigation of tip leakage vortex trajectory and dynamics in an axial flow pump. Comput. Fluids 2015, 112, 61–71. [Google Scholar] [CrossRef]
- Shi, G.; Liu, Z.; Xiao, Y.; Li, H.; Liu, X. Velocity characteristics in a multiphase pump under different tip clearances. Proc. Inst. Mech. Eng. 2021, 235, 454–475. [Google Scholar] [CrossRef]
- Shi, G.; Liu, Z.; Liu, X.; Xiao, Y.; Tang, X. Phase Distribution in the Tip Clearance of a Multiphase Pump at Multiple Operating Points and Its Effect on the Pressure Fluctuation Intensity. Processes 2021, 9, 556. [Google Scholar] [CrossRef]
- Han, W.; Liu, Y.; Gong, C.; Su, Y.; Guo, P.; Su, M.; Shi, F.; Wei, Z. Effect of tip clearance on performance of contra-rotating axial flow water-jet propulsion pump. Mod. Phys. Lett. B 2020, 34, 1–16. [Google Scholar] [CrossRef]
- Shen, S.; Qian, Z.; Ji, B.; Agarwal, R. Numerical investigation of tip flow dynamics and main flow characteristics with varying tip clearance widths for an axial-flow pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2019, 233, 1–13. [Google Scholar] [CrossRef]
- Xu, B.; Shen, X.; Zhang, D.; Zhang, W. Experimental and numerical investigation on the tip leakage vortex cavitation in an axial flow pump with different tip clearances. Processes 2019, 7, 935. [Google Scholar] [CrossRef] [Green Version]
- Ji, L.; Li, W.; Shi, W.; Agarwal, R. Transient characteristics of internal flow fields of mixed-flow pump with different tip clearances under stall condition. Proc. Inst. Mech. Eng. Part A J. Power Energy 2021, 235, 700–717. [Google Scholar] [CrossRef]
- Zhang, D.; Shen, X.; Dong, Y.; Wang, C.; Liu, A.; Shi, W. Numerical simulation of different blade tip clearances on internal flow characteristics in mixed-flow pump. J. Drain. Irrig. Mach. Eng. 2020, 38, 757–763. [Google Scholar]
- Li, W.; Ji, L.; Shi, W.; Li, E.; Yang, Z. Particle image velocimetry measurement of flow fields in a mixed-flow pump with non-uniform tip clearance. J. Vis. 2020. (prepublish). [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Zhou, L.; Agarwal, R.; Sun, S. Experimental Study of Pressure Pulsation in a Mixed-Flow Pump with Different Tip Clearances Based on Wavelet Analysis. Shock. Vib. 2020. [Google Scholar] [CrossRef]
- Parikh, T.; Mansour, M.; Thévenin, D. Investigations on the effect of tip clearance gap and inducer on the transport of air-water two-phase flow by centrifugal pumps. Chem. Eng. Sci. 2020, 218. [Google Scholar] [CrossRef]
- Zhang, Q.; Yang, J.; Li, H.; Yan, S.; Li, Y. Study on the Flow Characteristics of Variable Tip Clearance for Semi-open lmpeller Centrifugal pump. Hydraul. Pneum. Seals 2021, 41, 1–6. [Google Scholar]
- Cui, B.; Huang, D.; Shi, P.; Jin, Q. Effect of tip clearance on performance of low specific speed centrifugal pump with semi-open impeller. J. Drain. Irrig. Mach. Eng. 2012, 30, 283–288. [Google Scholar]
- Hao, Y.; Tan, L. Symmetrical and unsymmetrical tip clearances on cavitation performance and radial force of a mixed flow pump as turbine at pump mode. Renew. Energy 2018, 127, 368–376. [Google Scholar] [CrossRef]
- Xu, Y.; Tan, L.; Liu, Y.; Cao, S. Pressure fluctuation and flow pattern of a mixed-flow pump with different blade tip clearances under cavitation condition. Adv. Mech. Eng. 2017, 9, 1–12. [Google Scholar] [CrossRef]
- Han, W.; Xu, D.; Guo, W.; Su, M.; Chen, Y.; Han, Y. Effects of Axial Clearance on Hydraulic Characteristics of Counter-Rotating Water-Jet Pump. J. Propuls. Technol. 2019, 40, 2144–2152. [Google Scholar]
- Ngoc Tran, B.; Jeong, H.; Kim, J.; Park, J.; Yang, C. Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine. Energies 2020, 13, 4055. [Google Scholar] [CrossRef]
- Zhu, W.; Wang, S.; Zhang, L.; Ding, J.; Wang, Z. Effect of tip clearance size on the performance of a low-reaction transonic axial compressor rotor. Proc. Inst. Mech. Eng. Part A J. Power Energy 2020, 234, 127–142. [Google Scholar] [CrossRef]
- Geng, M.; Song, Y.; Cheng, A.; Feng, H. Numerical Simulation of the Influence of Tip Clearance on the Performance of Centrifugal Cold Compressor. Int. J. Fluid Mach. Syst. 2020, 13, 214–221. [Google Scholar] [CrossRef]
- Suh, J.; Kim, J.; Choi, Y.; Kim, J.; Joo, W.; Lee, K. Multi-objective optimization of the hydrodynamic performance of the second stage of a multi-phase pump. Energies 2017, 10, 1334. [Google Scholar] [CrossRef] [Green Version]
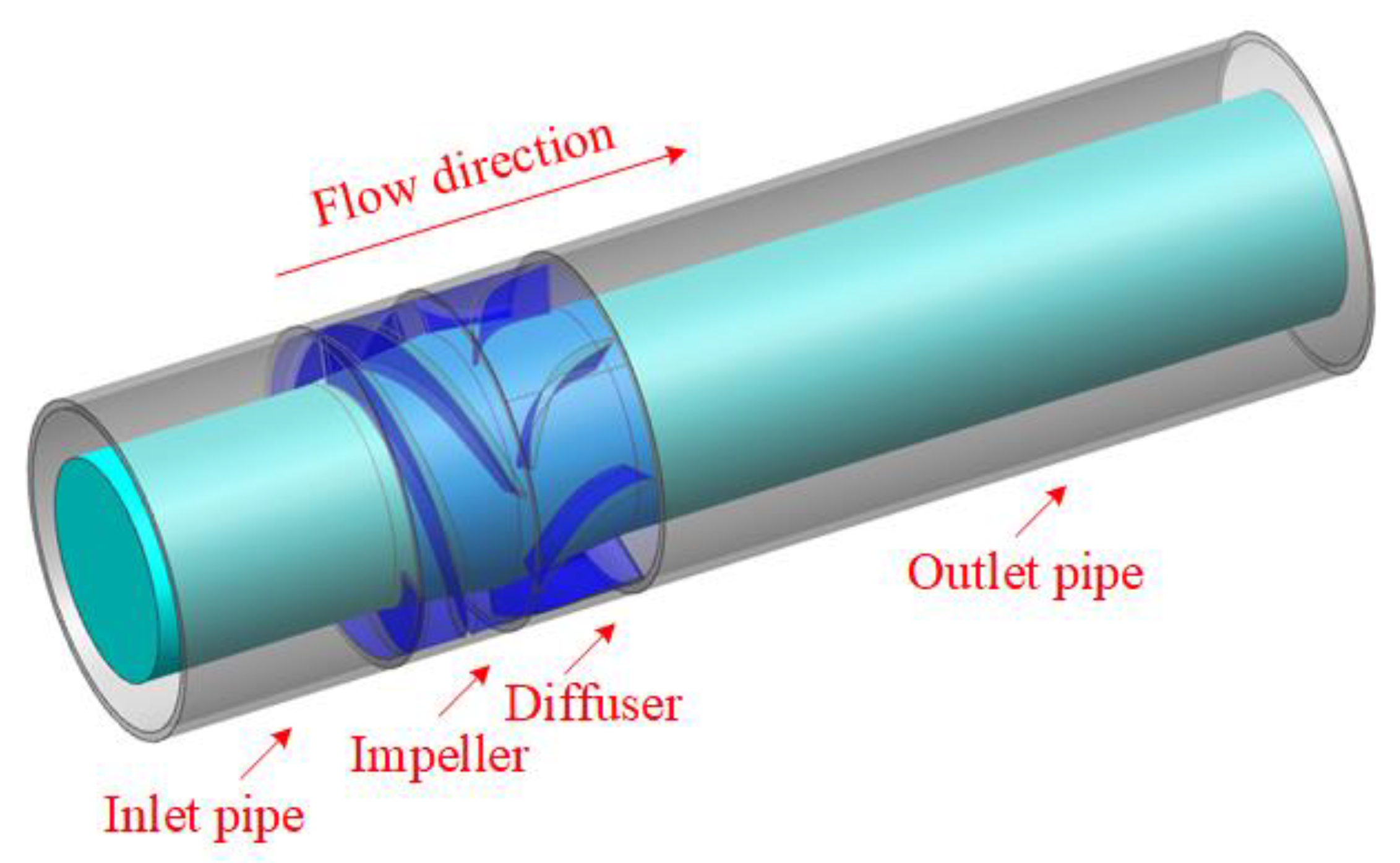
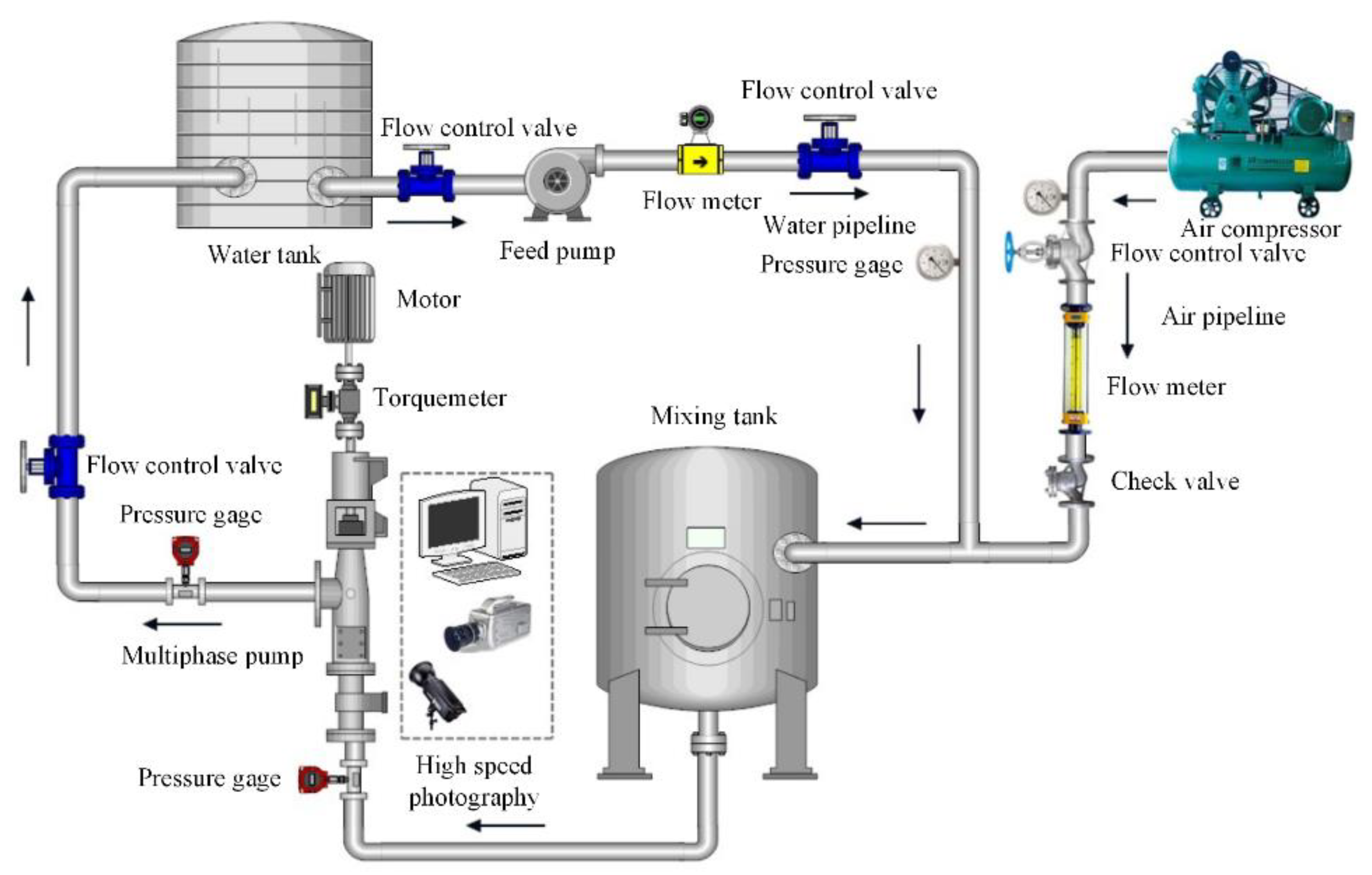
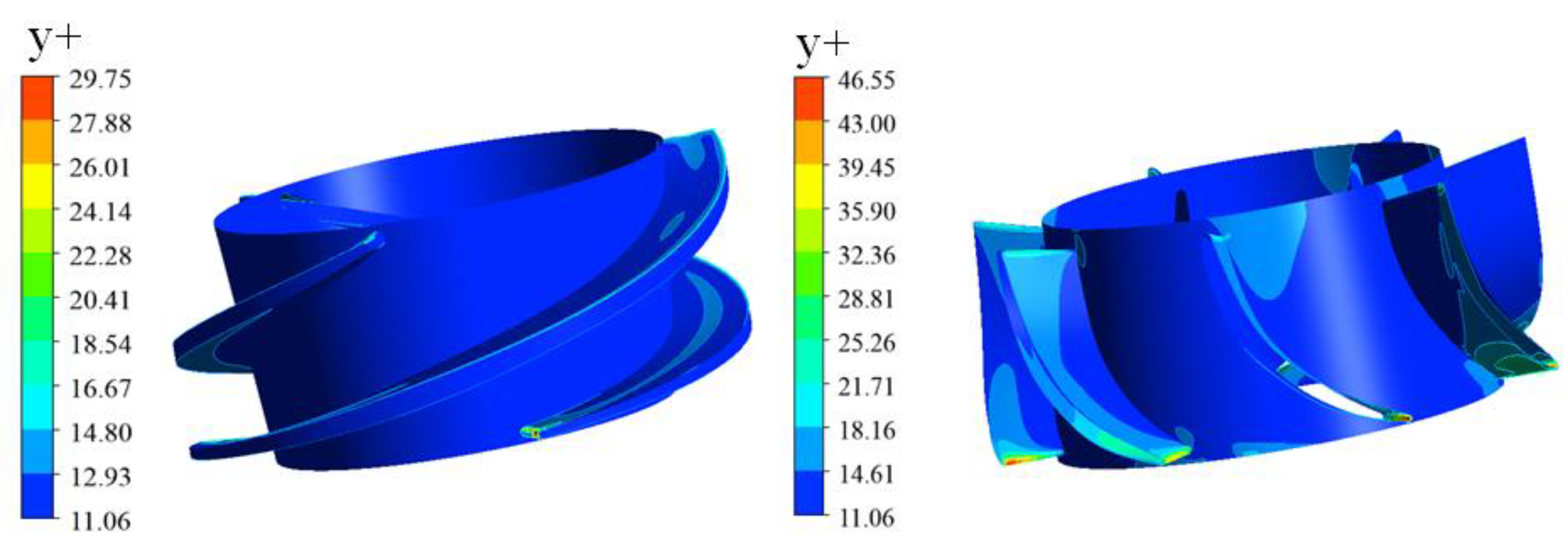


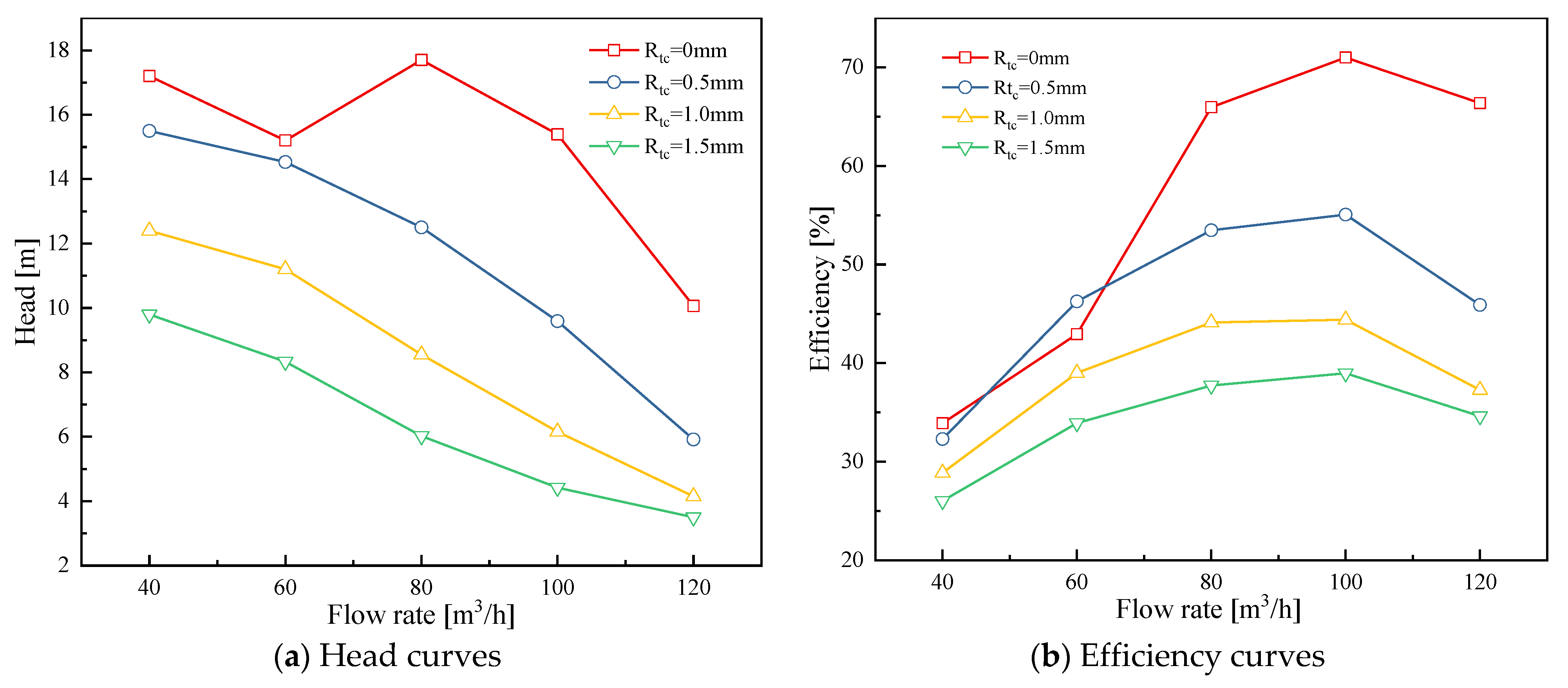



| Parameters | Value |
|---|---|
| Design flow rate Q/m3/h | 100 |
| Design rotational speed n/rpm | 3000 |
| Impeller blades Z1 (-) | 3 |
| Diffuser blades Z2 (-) | 7 |
| Number | Equipment | Value | Precision |
|---|---|---|---|
| 1 | High-speed photography (RDT16-4G) | 500 fps, 1280 × 1024 pixels | (-) |
| 2 | Pressure gauge | 0–5 Mpa | 0.25% |
| 3 | Flow meter | 14–200 m3/h | 0.5% |
| 4 | Air compressor | 200 m3/h | (-) |
| 5 | Flow control valve | DN = 100 mm | (-) |
| Parameters | Inlet Pipe | Impeller | Diffuser | Outlet Pipe | Total |
|---|---|---|---|---|---|
| Mesh 1 | 182,476 | 1,001,925 | 443,534 | 642,488 | 2,270,423 |
| Mesh 2 | 182,476 | 1,736,925 | 750,596 | 642,488 | 3,312,485 |
| Mesh 3 | 182,476 | 2,686,650 | 1,146,663 | 642,488 | 4,658,277 |
| Mesh 4 | 182,476 | 3,234,534 | 1,361,612 | 642,488 | 5,421,110 |
| Mesh 5 | 182,476 | 4,618,575 | 1,689,240 | 642,488 | 7,132,779 |
| Parameters | Mesh 1 | Mesh 2 | Mesh 3 | Mesh 4 | Mesh 5 |
|---|---|---|---|---|---|
| Head | 8.2623 | 8.2620 | 8.3326 | 8.3242 | 8.3991 |
| Efficiency | 42.40% | 42.59% | 42.83% | 42.94% | 42.97% |
| Head/Head1 | 1 | 0.9999 | 1.0085 | 1.0074 | 1.0165 |
| Efficiency/Efficiency1 | 1 | 1.0043 | 1.0100 | 1.0127 | 1.0134 |
| Properties | Density | Dynamic Viscosity | Molar Mass |
|---|---|---|---|
| Water | 997 kg/m3 | 9.028 × 10−4 Pa·s | 18.02 kg/mol |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kan, N.; Liu, Z.; Shi, G.; Liu, X. Effect of Tip Clearance on Helico-Axial Flow Pump Performance at Off-Design Case. Processes 2021, 9, 1653. https://doi.org/10.3390/pr9091653
Kan N, Liu Z, Shi G, Liu X. Effect of Tip Clearance on Helico-Axial Flow Pump Performance at Off-Design Case. Processes. 2021; 9(9):1653. https://doi.org/10.3390/pr9091653
Chicago/Turabian StyleKan, Nengqi, Zongku Liu, Guangtai Shi, and Xiaobing Liu. 2021. "Effect of Tip Clearance on Helico-Axial Flow Pump Performance at Off-Design Case" Processes 9, no. 9: 1653. https://doi.org/10.3390/pr9091653
APA StyleKan, N., Liu, Z., Shi, G., & Liu, X. (2021). Effect of Tip Clearance on Helico-Axial Flow Pump Performance at Off-Design Case. Processes, 9(9), 1653. https://doi.org/10.3390/pr9091653






