Beam Tailoring Devices Using Transmission-Type Subwavelength Grating
Abstract
1. Introduction
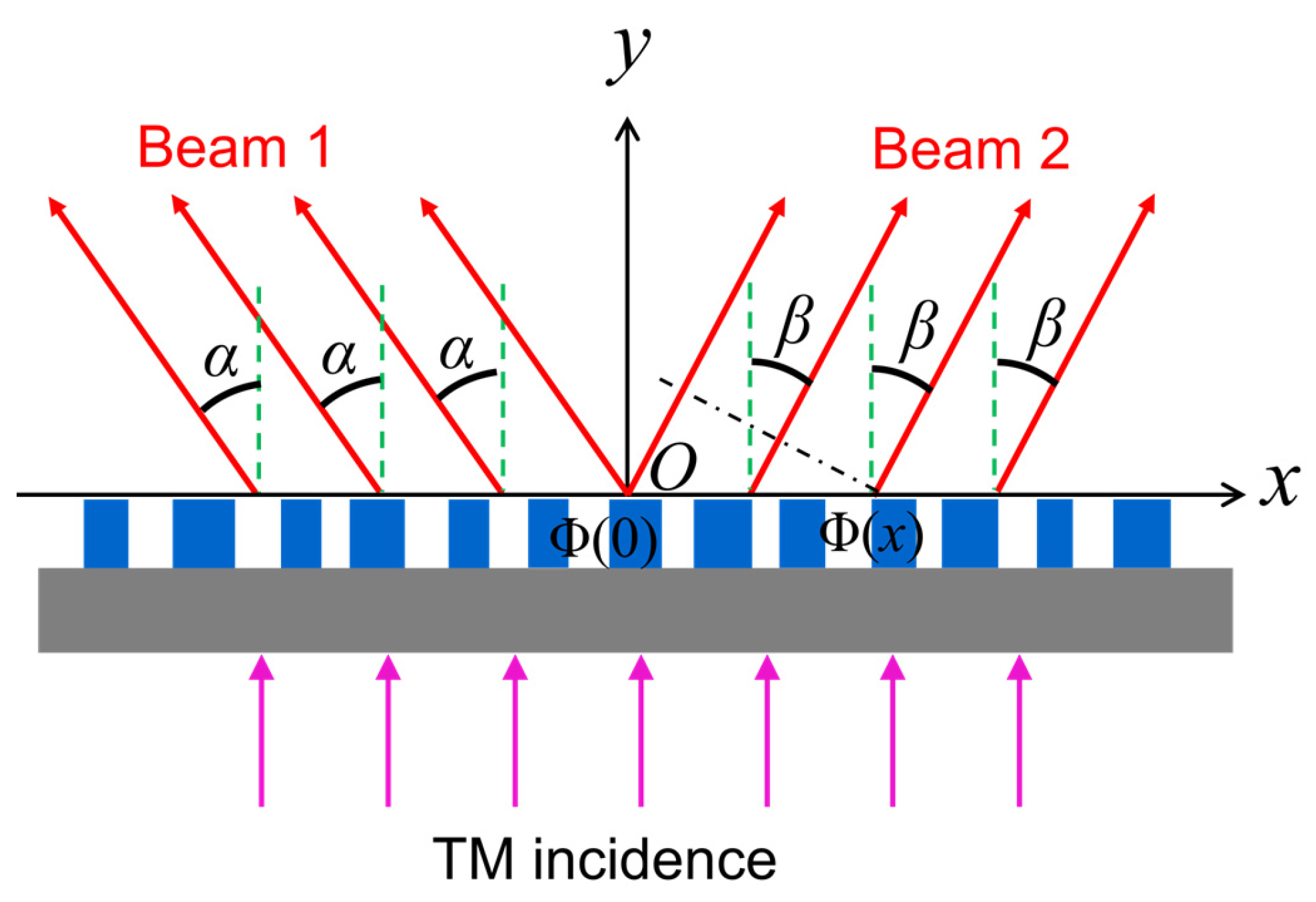
2. Theoretical Background
3. Design Process and Numerical Simulation
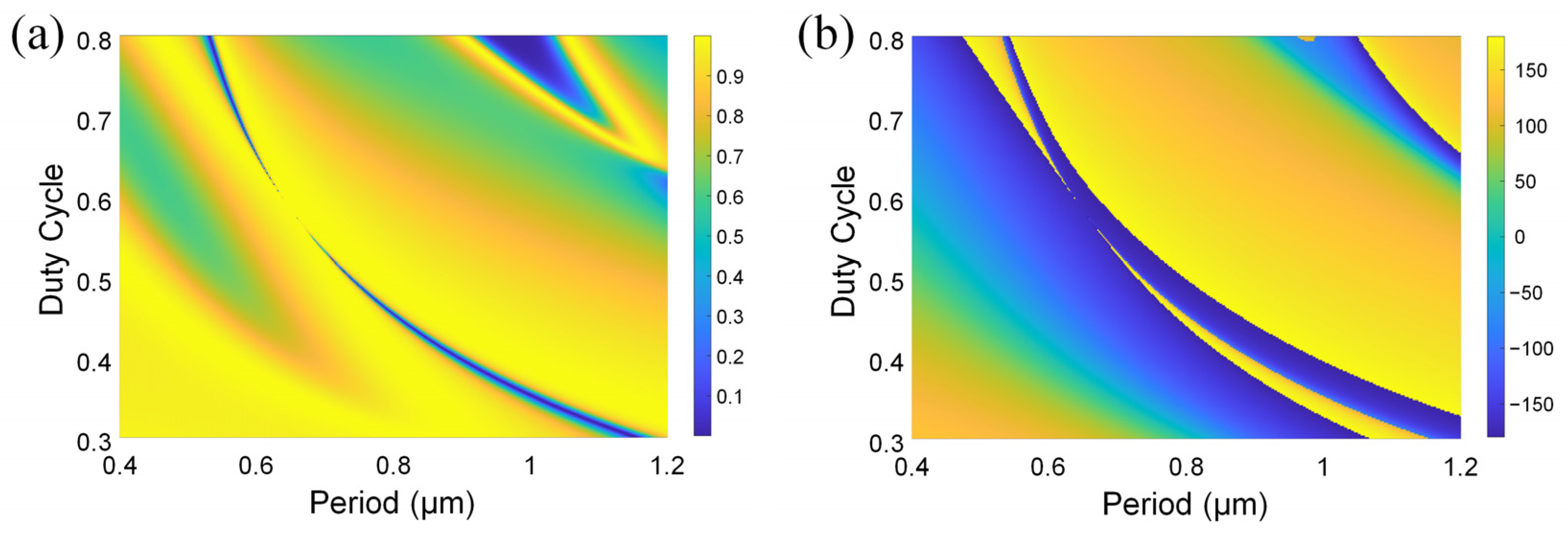
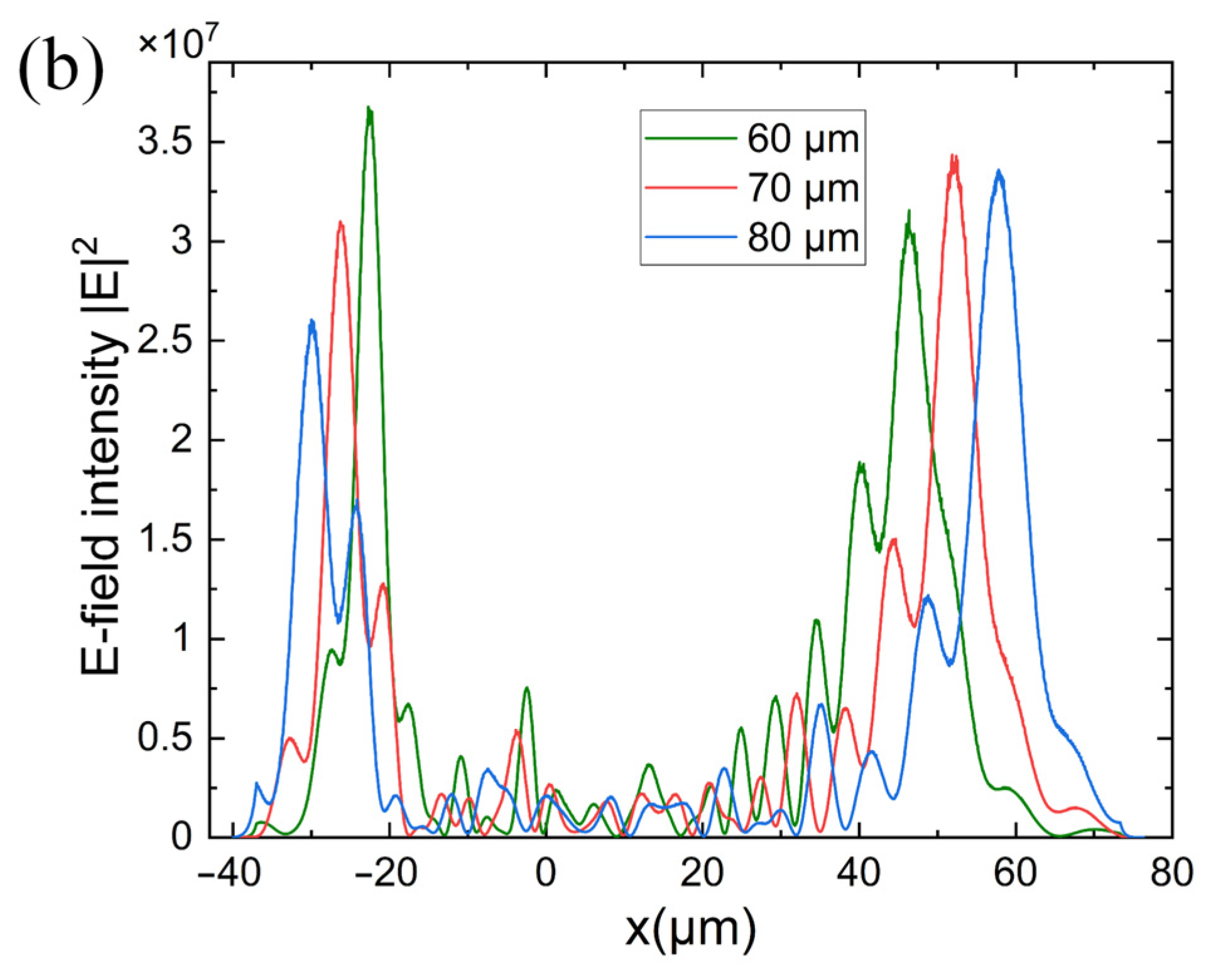
3.1. SWG Bifocal Lens
3.2. SWG Beam Splitter
3.3. SWG Beam Combiner
4. Conclusions
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Choi, M.; Leem, J.W.; Yu, J.S. Optical performance improvement of semitransparent metal film electrodes with biomimetic SWGs for high-performance optoelectronic device applications. RSC Adv. 2015, 5, 84865–84871. [Google Scholar] [CrossRef]
- Parandin, F.; Heidari, F.; Rahimi, Z.; Olyaee, S. Two-dimensional photonic crystal biosensors: A review. Opt. Laser Technol. 2021, 144, 107397. [Google Scholar] [CrossRef]
- Maleki, M.J.; Mir, A.; Soroosh, M. Design and analysis of a new compact all-optical full-adder based on photonic crystals. Optik 2021, 227, 166107. [Google Scholar] [CrossRef]
- Benmerkhi, A.; Bounouioua, A.; Bouchemat, M. Two-dimensional photonic crystal resonator for high pressure sensing. In Proceedings of the Applied Nanotechnology and Nanoscience International Conference, Paris, France, 24 March 2021. [Google Scholar]
- Vlasov, Y.A.; Moll, N.; McNab, S.J. Intrinsic diffraction losses in 2D SOI photonic crystal waveguides. In Proceedings of the International Quantum Electronics Conference, San Francisco, CA, USA, 21 May 2004; pp. 823–824. [Google Scholar]
- Wang, J.; Glesk, I.; Chen, L.R. Subwavelength grating devices in silicon photonics. Sci. Bull. 2016, 61, 879–888. [Google Scholar] [CrossRef]
- Chang-Hasnain, C.J. High-contrast gratings as a new platform for integrated optoelectronics. Semicond. Sci. Technol. 2011, 26, 014043. [Google Scholar] [CrossRef]
- Zohar, M.; Auslender, M.; Hava, S. Ultrathin high efficiency photodetectors based on SWG and near-field enhanced absorption. Nanoscale 2015, 7, 5476–5479. [Google Scholar] [CrossRef]
- Xie, H.; Zheng, J.J.; Xu, P.P.; Yao, J.T.; Whitehead, J.; Majumdar, A. Ultra-compact subwavelength-grating-assisted polarization-independent directional coupler. IEEE Photonics Technol. Lett. 2019, 31, 1538–1541. [Google Scholar] [CrossRef]
- Sumi, R.; Gupta, N.D.; Das, B.K. Integrated optical linear edge filters using apodized sub-wavelength grating waveguides in SOI. IEEE Photonics Technol. Lett. 2019, 31, 1449–1452. [Google Scholar] [CrossRef]
- Ma, M.; Park, A.H.K.; Wang, Y.; Shoman, H.; Zhang, F.; Jaeger, N.; Chrostowski, L. Sub-wavelength grating-assisted polarization splitter-rotators for silicon-on-insulator platforms. Opt. Express 2019, 27, 17581–17591. [Google Scholar] [CrossRef]
- Tahmasebpour, M.; Bahrami, M.; Asgari, A. Design of a high figure of merit subwavelength grating based plasmonic sensor for detection of DNA hybridization. Optik 2015, 126, 2747–2751. [Google Scholar] [CrossRef]
- Cencillo-Abad, P.; Plum, E.; Rogers, E.T.; Zheludev, N. Spatial optical phase-modulating metadevice with subwavelength pixelation. Opt. Express 2016, 24, 18790–18798. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, M.X.; Gu, P.; Weng, Z.Y.; Yan, Z.D.; Gao, F.; Tang, C.J.; Yan, B.; Zhu, M.W. Silver mirror for enhancing the magnetic plasmon resonance and sensing performance in plasmonic metasurface. Appl. Phys. Express 2022, 15, 102003. [Google Scholar] [CrossRef]
- Yuan, Y.; Sun, M.Y.; Bi, Y.; Gao, W.N.; Zhang, S.; Zhang, W.P. Controlling the light wavefront through a scattering medium based on direct digital frequency synthesis technology. Chin. Phys. B 2021, 30, 014209. [Google Scholar] [CrossRef]
- Liang, X.B.; Li, Y.Q.; Liu, K. Wide dynamic range wavefront sensor using sub-wavelength grating array. Opt. Commun. 2015, 347, 68–74. [Google Scholar] [CrossRef]
- Fattal, D.; Li, J.; Peng, Z.; Fiorentino, M.; Beausoleil, R.G. Flat silicon grating reflectors with high focusing power. Opt. Photonics News 2010, 21, 42. [Google Scholar] [CrossRef]
- Arbabi, A.; Horie, Y.; Ball, A.J.; Bagheri, M.; Faraon, A. Subwavelength-thick lenses with high numerical apertures and large efficiency based on high contrast transmitarrays. Nat. Commun. 2015, 6, 7069. [Google Scholar] [CrossRef]
- Kaur, H.; Kumar, M. Two-way reflector based on two-dimensional sub-wavelength high-index contrast grating on SOI. Opt. Commun. 2016, 366, 266–270. [Google Scholar] [CrossRef]
- Halir, R.; Cheben, P.; Schmid, J.H.; Ma, R.; Bedard, D.; Janz, S.; Xu, D.X.; Densmore, A.; Lapointe, J.; Molina-Fernandez, I. Continuously apodized fiber-to-chip surface grating coupler with refractive index engineered subwavelength structure. Opt. Lett. 2010, 35, 3243–3245. [Google Scholar] [CrossRef]
- Stowasser, A.; Rapaport, A.; Layne, J.E.; Morgan, R.C.; Buschbeck, E.K. Biological Bifocal Lenses with Image Separation. Curr. Biol. 2010, 20, 1482–1486. [Google Scholar] [CrossRef]
- Moharam, M.G.; Grann, E.B.; Pommet, D.A.; Gaylord, T.K. Formulation for stable and efficient implementation of the rigorous coupled-wave analysis of binary gratings. J. Opt. Soc. Am. A 1995, 12, 1068–1076. [Google Scholar] [CrossRef]
- Ma, C.L.; Huang, Y.Q.; Duan, X.F.; Ren, X.M. High-transmissivity non-periodic sub-wavelength high-contrast grating with large-angle beam-steering ability. Chin. Opt. Lett. 2014, 12, 120501. [Google Scholar] [CrossRef]
- Gal, J.; Horvath, G.; Clarkson, E.N.K.; Haiman, O. Image formation by bifocal lenses in a trilobite eye? Vision Res. 2000, 40, 843–853. [Google Scholar] [CrossRef] [PubMed]
- Kroger, R.H.H.; Campbell, M.C.W.; Fernald, R.D.; Wagner, H.J. Multifocal lenses compensate for chromatic defocus in vertebrate eyes. J. Comp. Physiol. A 1999, 184, 361–369. [Google Scholar] [CrossRef]
- Ma, C.L.; Huang, Y.Q.; Ren, X.M. High-numerical-aperture high-reflectivity focusing reflectors using concentric circular high-contrast gratings. Appl. Opt. 2015, 54, 973–978. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Zhang, C.C.; Yang, Y.Y.; Ke, L.; Fang, B.; Li, C.X.; Hong, Z.; Jing, X.F. Polarization sensitive terahertz all dielectric continuous trapezoid metasurface beam splitter. Infrared Phys. Technol. 2023, 130, 104604. [Google Scholar] [CrossRef]
- Torcal-Milla, F.J.; Sanchez-Brea, L.M. Design and numerical analysis of metallic Ronchi diffraction gratings acting as reflective beam-splitter. Optik 2022, 271, 170119. [Google Scholar] [CrossRef]
- Yang, J.B.; Xin, H.; Han, Y.X.; Chen, D.B.; Zhang, J.J.; Huang, J.; Zhang, Z.J. Ultra-compact beam splitter and filter based on a graphene plasmon waveguide. Appl. Opt. 2017, 56, 9814–9821. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, Y.; Lv, P.; Sun, Q. Simulation method of non-ideal light source in laser system. Infrared Laser Eng. 2014, 11, 3527–3530. (In Chinese) [Google Scholar]
- Wu, G.; Huang, Y.Q.; Duan, X.F.; Liu, K.; Niu, H.J.; Ren, X.M. Light control elements as convex cylindrical lens and concave cylindrical mirror based on 2D high-index contrast gratings. Jpn. J. Appl. Phys. 2020, 59, 062003. [Google Scholar] [CrossRef]
- Takashima, Y.; Haraguchi, M.; Naoi, Y. High-sensitivity refractive index sensor with normal incident geometry using a subwavelength grating operating near the ultraviolet wavelength. Sens. Actuators B 2018, 255, 1711–1715. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, G.; Hou, S.; Lei, J.; Yan, Z. Beam Tailoring Devices Using Transmission-Type Subwavelength Grating. Photonics 2023, 10, 607. https://doi.org/10.3390/photonics10060607
Wu G, Hou S, Lei J, Yan Z. Beam Tailoring Devices Using Transmission-Type Subwavelength Grating. Photonics. 2023; 10(6):607. https://doi.org/10.3390/photonics10060607
Chicago/Turabian StyleWu, Gang, Shanglin Hou, Jingli Lei, and Zuyong Yan. 2023. "Beam Tailoring Devices Using Transmission-Type Subwavelength Grating" Photonics 10, no. 6: 607. https://doi.org/10.3390/photonics10060607
APA StyleWu, G., Hou, S., Lei, J., & Yan, Z. (2023). Beam Tailoring Devices Using Transmission-Type Subwavelength Grating. Photonics, 10(6), 607. https://doi.org/10.3390/photonics10060607




