Fluorescent Microscopy of Hot Spots Induced by Laser Heating of Iron Oxide Nanoparticles
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. IONPs Synthesis
2.3. Temperature Measuring in IONPs Water Colloids
2.4. Temperature Measuring of IONPs “Hot Spots” in Cells In Vitro
2.5. Modeling of Local Field Enhancement and Heating for IONPs Ensembles under the Action of EM Radiation
3. Results
3.1. NPs Characterization
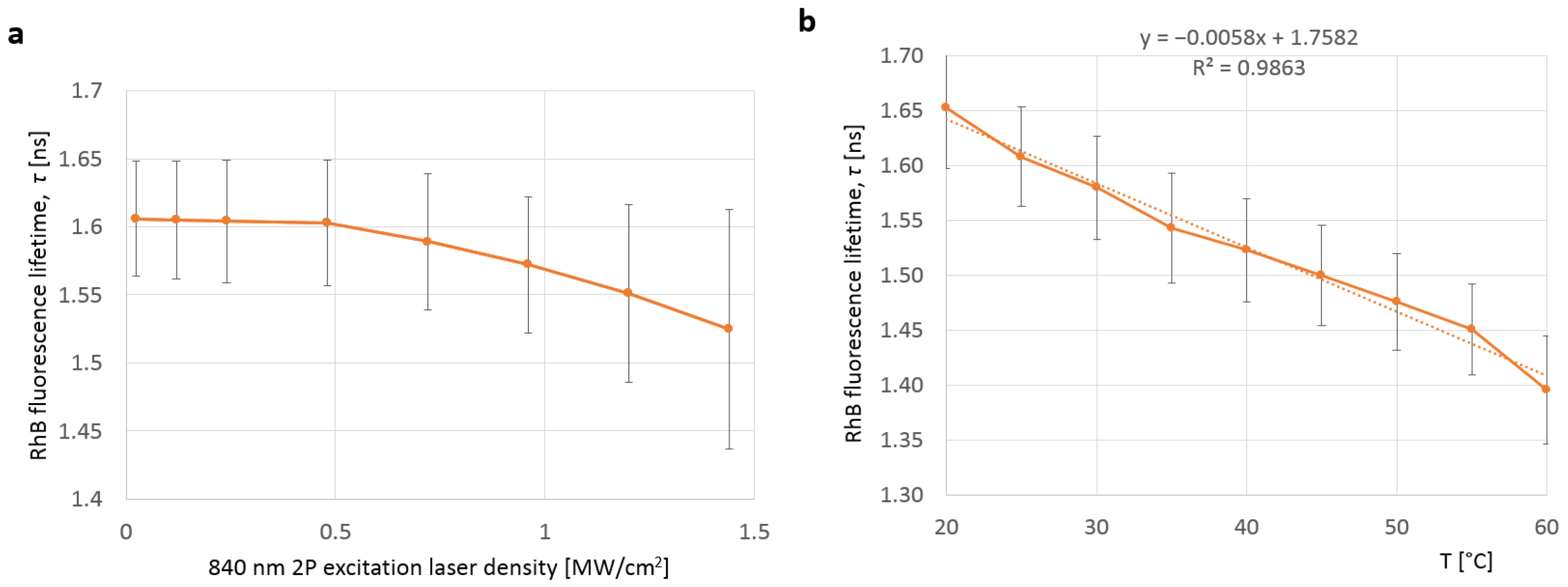
3.2. Measurements in RhB Solutions
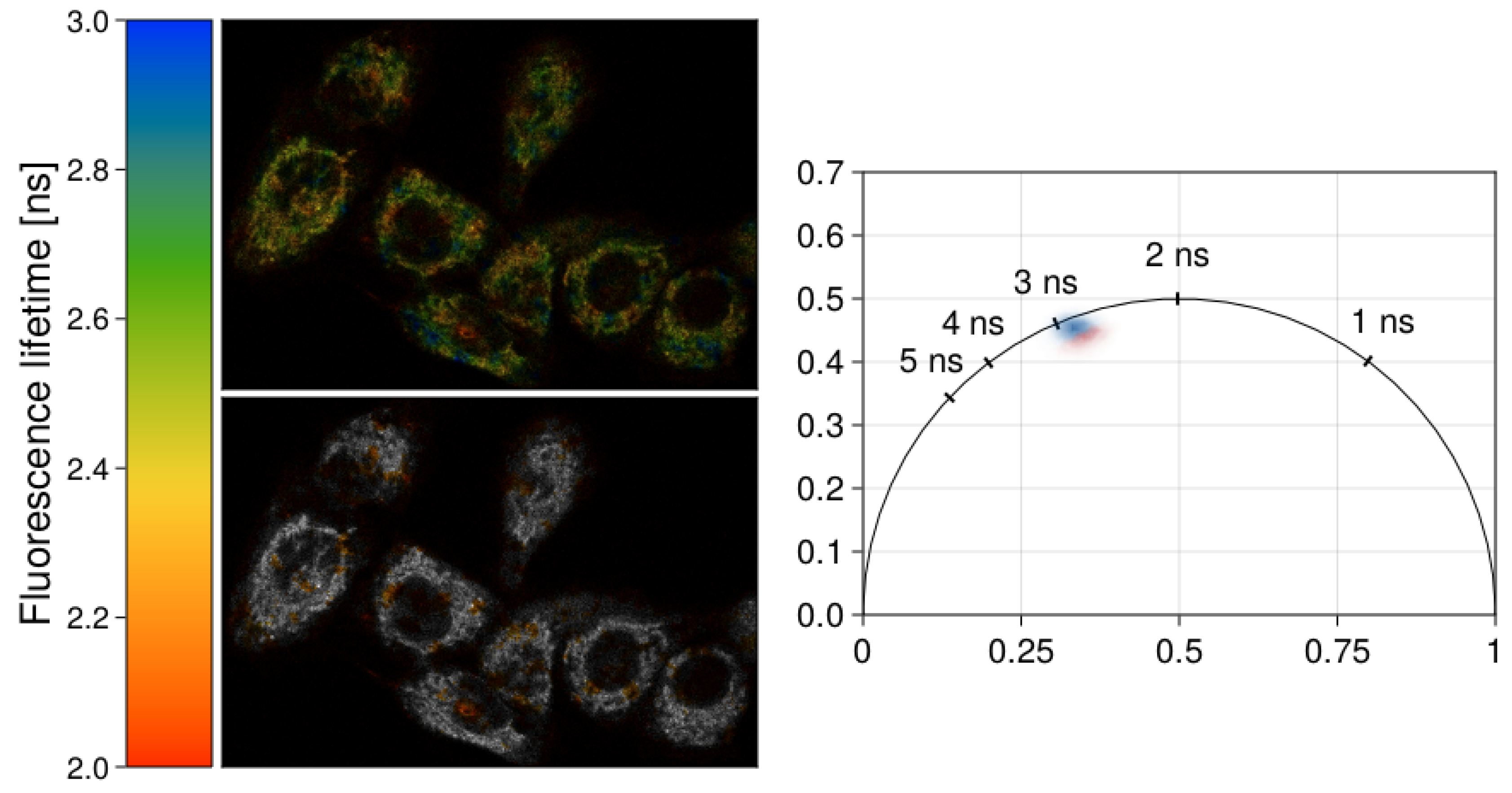
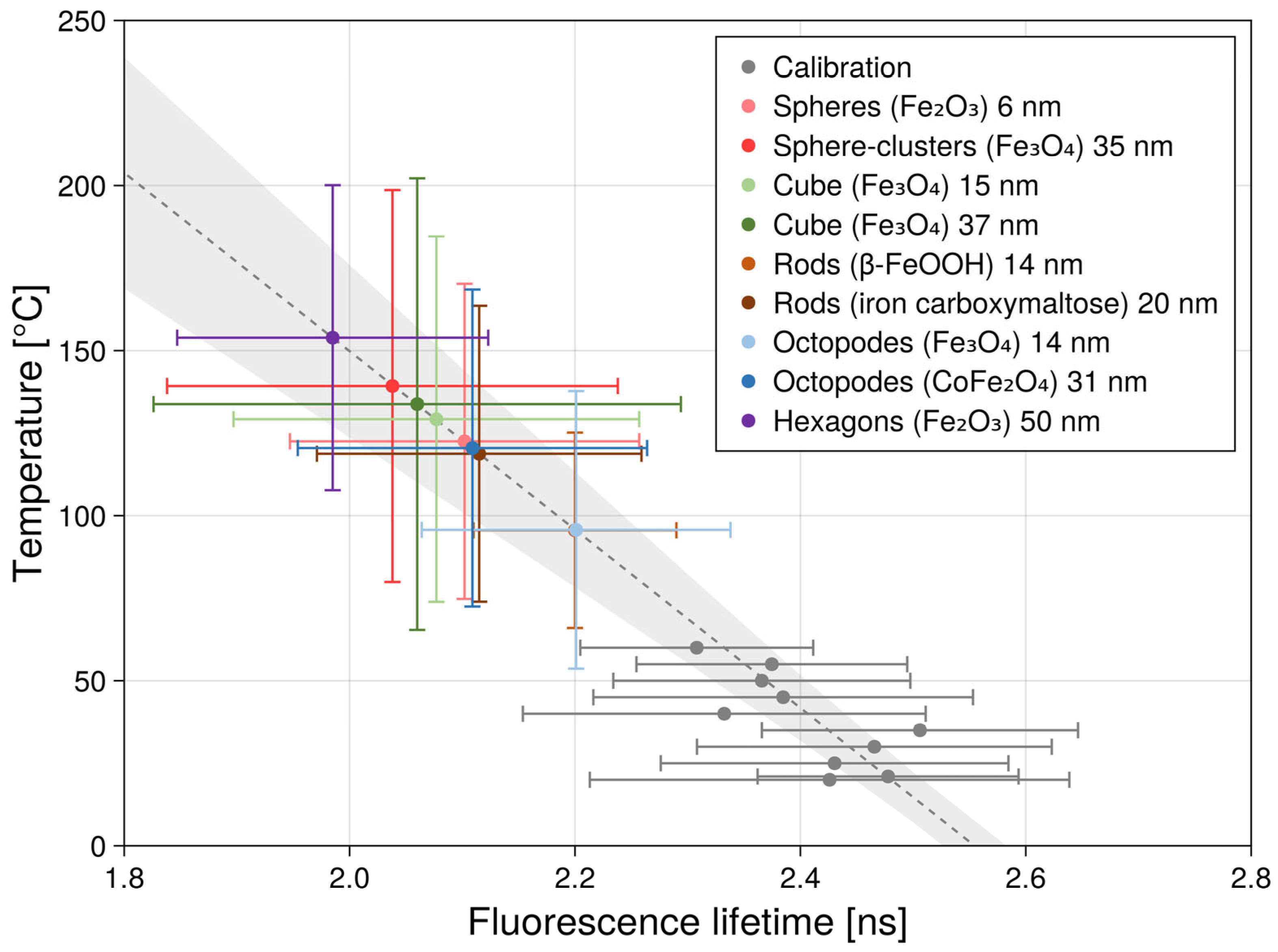
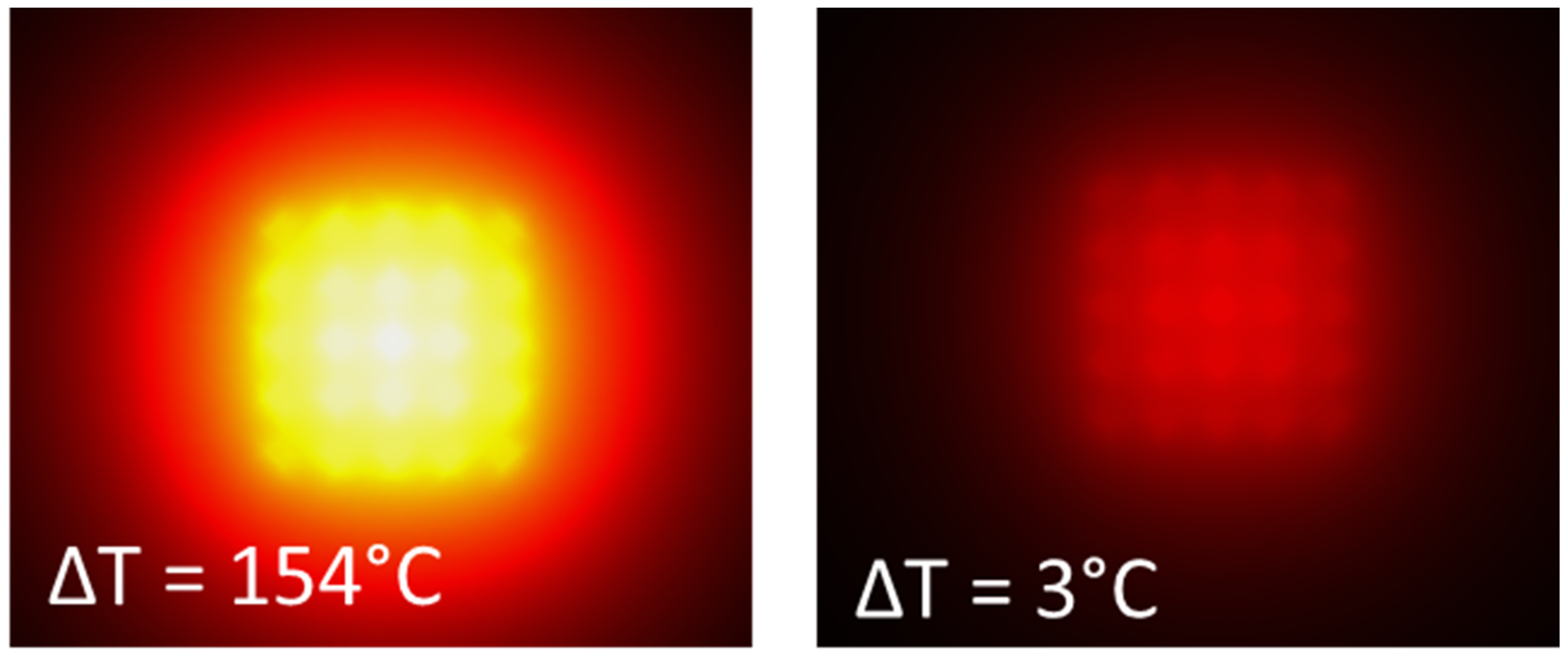
3.3. Assessing the Temperature in Cells
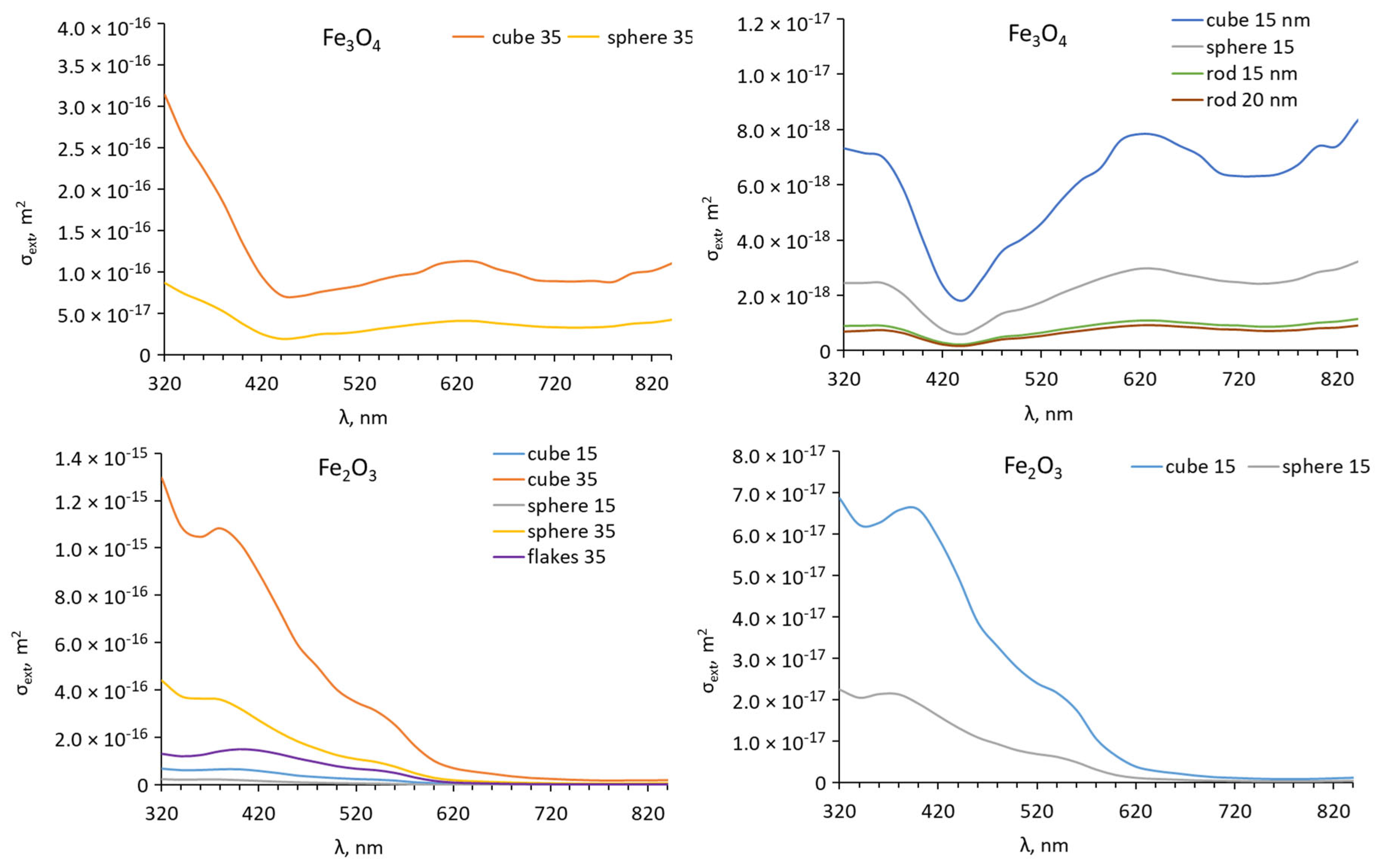
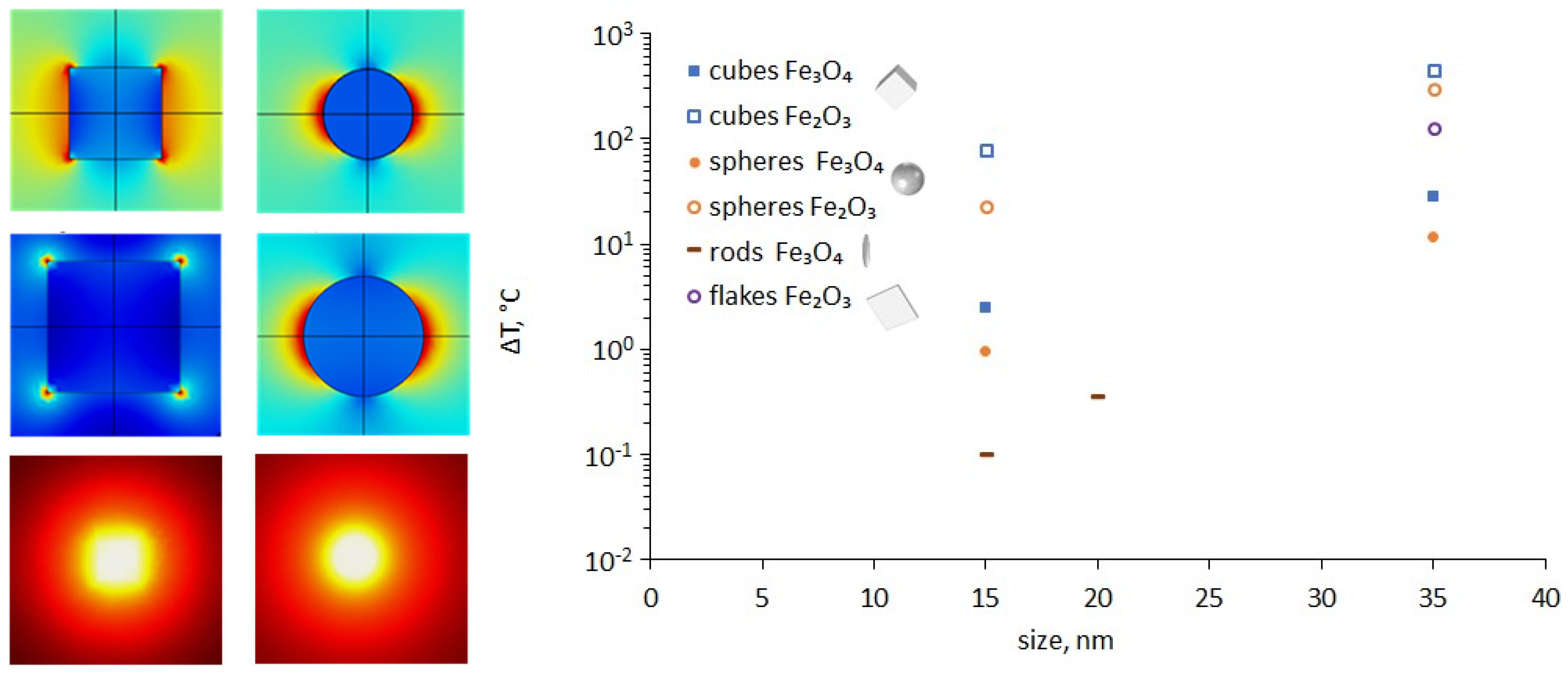
3.4. Modelling
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sharma, S.K.; Shrivastava, N.; Rossi, F.; Tung, L.D.; Thanh, N.T.K. Nanoparticles-Based Magnetic and Photo Induced Hyperthermia for Cancer Treatment. Nano Today 2019, 29, 100795. [Google Scholar] [CrossRef]
- Sun, R.; Chen, H.; Sutrisno, L.; Kawazoe, N.; Chen, G. Nanomaterials and Their Composite Scaffolds for Photothermal Therapy and Tissue Engineering Applications. Sci. Technol. Adv. Mater. 2021, 22, 404–428. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Boltasseva, A.; Pedersen, R.H.; Bakker, R.; Kildishev, A.V.; Drachev, V.P.; Shalaev, V.M. Plasmonic Nanoantenna Arrays for the Visible. Metamaterials 2008, 2, 45–51. [Google Scholar] [CrossRef]
- Hatab, N.A.; Hsueh, C.-H.; Gaddis, A.L.; Retterer, S.T.; Li, J.-H.; Eres, G.; Zhang, Z.; Gu, B. Free-Standing Optical Gold Bowtie Nanoantenna with Variable Gap Size for Enhanced Raman Spectroscopy. Nano Lett. 2010, 10, 4952–4955. [Google Scholar] [CrossRef]
- Nien, L.-W.; Lin, S.-C.; Chao, B.-K.; Chen, M.-J.; Li, J.-H.; Hsueh, C.-H. Giant Electric Field Enhancement and Localized Surface Plasmon Resonance by Optimizing Contour Bowtie Nanoantennas. J. Phys. Chem. C 2013, 117, 25004–25011. [Google Scholar] [CrossRef]
- Giannini, V.; Fernández-Domínguez, A.I.; Heck, S.C.; Maier, S.A. Plasmonic Nanoantennas: Fundamentals and Their Use in Controlling the Radiative Properties of Nanoemitters. Chem. Rev. 2011, 111, 3888–3912. [Google Scholar] [CrossRef]
- Baranov, D.G.; Zuev, D.A.; Lepeshov, S.I.; Kotov, O.V.; Krasnok, A.E.; Evlyukhin, A.B.; Chichkov, B.N. All-Dielectric Nanophotonics: The Quest for Better Materials and Fabrication Techniques. Optica 2017, 4, 814. [Google Scholar] [CrossRef] [Green Version]
- Shi, L.; Fenollosa, R.; Tuzer, T.U.; Meseguer, F. Angle-Dependent Quality Factor of Mie Resonances in Silicon-Colloid-Based Microcavities. ACS Photonics 2014, 1, 408–412. [Google Scholar] [CrossRef]
- Ma, C.; Yan, J.; Wei, Y.; Liu, P.; Yang, G. Enhanced Second Harmonic Generation in Individual Barium Titanate Nanoparticles Driven by Mie Resonances. J. Mater. Chem. C 2017, 5, 4810–4819. [Google Scholar] [CrossRef]
- Lozano-Pedraza, C.; Plaza-Mayoral, E.; Espinosa, A.; Sot, B.; Serrano, A.; Salas, G.; Blanco-Andujar, C.; Cotin, G.; Felder-Flesch, D.; Begin-Colin, S.; et al. Assessing the Parameters Modulating Optical Losses of Iron Oxide Nanoparticles under near Infrared Irradiation. Nanoscale Adv. 2021, 3, 6490–6502. [Google Scholar] [CrossRef]
- Boltasseva, A.; Atwater, H.A. Low-Loss Plasmonic Metamaterials. Science 2011, 331, 290–291. [Google Scholar] [CrossRef]
- Vallejo-Fernandez, G.; Whear, O.; Roca, A.G.; Hussain, S.; Timmis, J.; Patel, V.; O’Grady, K. Mechanisms of Hyperthermia in Magnetic Nanoparticles. J. Phys. D Appl. Phys. 2013, 46, 312001. [Google Scholar] [CrossRef]
- Espinosa, A.; Kolosnjaj-Tabi, J.; Abou-Hassan, A.; Sangnier, A.P.; Curcio, A.; Silva, A.K.A.; Di Corato, R.; Neveu, S.; Pellegrino, T.; Liz-Marzán, L.M.; et al. Magnetic (Hyper) Thermia or Photothermia? Progressive Comparison of Iron Oxide and Gold Nanoparticles Heating in Water, in Cells, and In Vivo. Adv. Funct. Mater. 2018, 28, 1803660. [Google Scholar] [CrossRef]
- Zhao, S.; Yu, X.; Qian, Y.; Chen, W.; Shen, J. Multifunctional Magnetic Iron Oxide Nanoparticles: An Advanced Platform for Cancer Theranostics. Theranostics 2020, 10, 6278–6309. [Google Scholar] [CrossRef]
- Espinosa, A.; Di Corato, R.; Kolosnjaj-Tabi, J.; Flaud, P.; Pellegrino, T.; Wilhelm, C. Duality of Iron Oxide Nanoparticles in Cancer Therapy: Amplification of Heating Efficiency by Magnetic Hyperthermia and Photothermal Bimodal Treatment. ACS Nano 2016, 10, 2436–2446. [Google Scholar] [CrossRef]
- Yan, H.; Shang, W.; Sun, X.; Zhao, L.; Wang, J.; Xiong, Z.; Yuan, J.; Zhang, R.; Huang, Q.; Wang, K.; et al. “All-in-One” Nanoparticles for Trimodality Imaging-Guided Intracellular Photo-magnetic Hyperthermia Therapy under Intravenous Administration. Adv. Funct. Mater. 2018, 28, 1705710. [Google Scholar] [CrossRef]
- Lin, S.-Y.; Huang, R.-Y.; Liao, W.-C.; Chuang, C.-C.; Chang, C.-W. Multifunctional PEGylated Albumin/IR780/Iron Oxide Nanocomplexes for Cancer Photothermal Therapy and MR Imaging. Nanotheranostics 2018, 2, 106–116. [Google Scholar] [CrossRef] [Green Version]
- Cabana, S.; Curcio, A.; Michel, A.; Wilhelm, C.; Abou-Hassan, A. Iron Oxide Mediated Photothermal Therapy in the Second Biological Window: A Comparative Study between Magnetite/Maghemite Nanospheres and Nanoflowers. Nanomaterials 2020, 10, 1548. [Google Scholar] [CrossRef]
- Liang, X.; Chen, M.; Bhattarai, P.; Hameed, S.; Tang, Y.; Dai, Z. Complementing Cancer Photodynamic Therapy with Ferroptosis through Iron Oxide Loaded Porphyrin-Grafted Lipid Nanoparticles. ACS Nano 2021, 15, 20164–20180. [Google Scholar] [CrossRef] [PubMed]
- Penon, O.; Marín, M.J.; Amabilino, D.B.; Russell, D.A.; Pérez-García, L. Iron Oxide Nanoparticles Functionalized with Novel Hydrophobic and Hydrophilic Porphyrins as Potential Agents for Photodynamic Therapy. J. Colloid Interface Sci. 2016, 462, 154–165. [Google Scholar] [CrossRef] [PubMed]
- Pominova, D.V.; Romanishkin, I.D.; Plotnikova, E.A.; Morozova, N.B.; Loschenov, V.B.; Wittig, R.; Linden, M.; Steiner, R.W.; Ryabova, A.V. Photo-Induced Processes of Iron Oxide Nanoparticles to Enhance Laser Therapy. Biomed. Photonics 2022, 10, 44–58. [Google Scholar] [CrossRef]
- Zhou, J.; del Rosal, B.; Jaque, D.; Uchiyama, S.; Jin, D. Advances and Challenges for Fluorescence Nanothermometry. Nat. Methods 2020, 17, 967–980. [Google Scholar] [CrossRef]
- Mercadé-Prieto, R.; Rodriguez-Rivera, L.; Chen, X.D. Fluorescence Lifetime of Rhodamine B in Aqueous Solutions of Polysaccharides and Proteins as a Function of Viscosity and Temperature. Photochem. Photobiol. Sci. 2017, 16, 1727–1734. [Google Scholar] [CrossRef]
- von Steyern, F.V.; Josefsson, J.O.; Tågerud, S. Rhodamine B, a Fluorescent Probe for Acidic Organelles in Denervated Skeletal Muscle. J. Histochem. Cytochem. 1996, 44, 267–274. [Google Scholar] [CrossRef] [PubMed]
- Reungpatthanaphong, P.; Dechsupa, S.; Meesungnoen, J.; Loetchutinat, C.; Mankhetkorn, S. Rhodamine B as a Mitochondrial Probe for Measurement and Monitoring of Mitochondrial Membrane Potential in Drug-Sensitive and -Resistant Cells. J. Biochem. Biophys. Methods 2003, 57, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Moreau, D.; Lefort, C.; Burke, R.; Leveque, P.; O’Connor, R.P. Rhodamine B as an Optical Thermometer in Cells Focally Exposed to Infrared Laser Light or Nanosecond Pulsed Electric Fields. Biomed. Opt. Express 2015, 6, 4105. [Google Scholar] [CrossRef] [Green Version]
- Fan, X.; Teng, C.P.; Yeo, J.C.C.; Li, Z.; Wang, T.; Chen, H.; Jiang, L.; Hou, X.; He, C.; Liu, J. Temperature and PH Responsive Light-Harvesting System Based on AIE-Active Microgel for Cell Imaging. Macromol. Rapid Commun. 2021, 42, 2000716. [Google Scholar] [CrossRef]
- Kumar, A.; Goudar, V.S.; Kaladharan, K.; Santra, T.S.; Tseng, F.-G. Synthesis and Characterization of a Fluorescent Polymeric Nano-Thermometer: Dynamic Monitoring of 3D Temperature Distribution in Co-Cultured Tumor Spheroids. Analyst 2023, 148, 2045–2057. [Google Scholar] [CrossRef] [PubMed]
- Arai, S.; Suzuki, M.; Park, S.-J.; Yoo, J.S.; Wang, L.; Kang, N.-Y.; Ha, H.-H.; Chang, Y.-T. Mitochondria-Targeted Fluorescent Thermometer Monitors Intracellular Temperature Gradient. Chem. Commun. 2015, 51, 8044–8047. [Google Scholar] [CrossRef]
- 1309-37-1-Iron(III) Oxide, Industrial, NanoArc®-45007-Alfa Aesar. Available online: https://www.alfa.com/ru/catalog/045007/ (accessed on 5 May 2023).
- Vifor Pharma. Iron Products. Available online: https://www.viforpharma.com/products/iron-products (accessed on 5 May 2023).
- Ostroverkhov, P.V.; Semkina, A.S.; Naumenko, V.A.; Plotnikova, E.A.; Melnikov, P.A.; Abakumova, T.O.; Yakubovskaya, R.I.; Mironov, A.F.; Vodopyanov, S.S.; Abakumov, A.M.; et al. Synthesis and Characterization of Bacteriochlorin Loaded Magnetic Nanoparticles (MNP) for Personalized MRI Guided Photosensitizers Delivery to Tumor. J. Colloid Interface Sci. 2019, 537, 132–141. [Google Scholar] [CrossRef]
- Naumenko, V.; Nikitin, A.; Kapitanova, K.; Melnikov, P.; Vodopyanov, S.; Garanina, A.; Valikhov, M.; Ilyasov, A.; Vishnevskiy, D.; Markov, A.; et al. Intravital Microscopy Reveals a Novel Mechanism of Nanoparticles Excretion in Kidney. J. Control. Release 2019, 307, 368–378. [Google Scholar] [CrossRef] [PubMed]
- Nikitin, A.A.; Yurenya, A.Y.; Zatsepin, T.S.; Aparin, I.O.; Chekhonin, V.P.; Majouga, A.G.; Farle, M.; Wiedwald, U.; Abakumov, M.A. Magnetic Nanoparticles as a Tool for Remote DNA Manipulations at a Single-Molecule Level. ACS Appl. Mater. Interfaces 2021, 13, 14458–14469. [Google Scholar] [CrossRef]
- Nikitin, A.; Khramtsov, M.; Garanina, A.; Mogilnikov, P.; Sviridenkova, N.; Shchetinin, I.; Savchenko, A.; Abakumov, M.; Majouga, A. Synthesis of Iron Oxide Nanorods for Enhanced Magnetic Hyperthermia. J. Magn. Magn. Mater. 2019, 469, 443–449. [Google Scholar] [CrossRef]
- Shelekhov, E.V.; Sviridova, T.A. Programs for X-ray Analysis of Polycrystals. Met. Sci. Heat Treat. 2000, 42, 309–313. [Google Scholar] [CrossRef]
- Farooq, S.; de Araujo, R.E. Engineering a Localized Surface Plasmon Resonance Platform for Molecular Biosensing. Open J. Appl. Sci. 2018, 08, 126–139. [Google Scholar] [CrossRef] [Green Version]
- Kholodtsova, M.N.; Grachev, P.V.; Blondel, W.C.; Zelenkov, P.V.; Potapov, A.A.; Shcherbakov, I.A.; Loschenov, V.B. Application of Devices for Space-Resolved Spectroscopy on the Example of Two-Layer Phantoms Containing Metallic Nanoparticles. BMP 2018, 7, 4–12. [Google Scholar] [CrossRef] [Green Version]
- Rakić, A.D.; Djurišić, A.B.; Elazar, J.M.; Majewski, M.L. Optical Properties of Metallic Films for Vertical-Cavity Optoelectronic Devices. Appl. Opt. 1998, 37, 5271. [Google Scholar] [CrossRef]
- Querry, M.R. Optical Constants; Technical Reports and Bulletins; Defense Technical Information Center: Kansas City, MO, USA, 1985. [Google Scholar]
- Rashidi, S.; Karimi, N.; Mahian, O.; Abolfazli Esfahani, J. A Concise Review on the Role of Nanoparticles upon the Productivity of Solar Desalination Systems. J. Therm. Anal. Calorim. 2019, 135, 1145–1159. [Google Scholar] [CrossRef] [Green Version]
- Iron Oxide, Hematite (Fe2O3). Available online: https://janaf.nist.gov/tables/Fe-030.html (accessed on 5 May 2023).
- NIST-JANAF Thermochemical Tables, 4th ed.; Chase, M.W.; National Institute of Standards and Technology (USA) (Eds.) American Chemical Society; American Institute of Physics for the National Institute of Standards and Technology: Washington, DC, USA, 1998; ISBN 978-1-56396-831-0. [Google Scholar]
- Lu, K.; Wazawa, T.; Sakamoto, J.; Vu, C.Q.; Nakano, M.; Kamei, Y.; Nagai, T. Intracellular Heat Transfer and Thermal Property Revealed by Kilohertz Temperature Imaging with a Genetically Encoded Nanothermometer. Nano Lett. 2022, 22, 5698–5707. [Google Scholar] [CrossRef]
- Song, P.; Gao, H.; Gao, Z.; Liu, J.; Zhang, R.; Kang, B.; Xu, J.-J.; Chen, H.-Y. Heat Transfer and Thermoregulation within Single Cells Revealed by Transient Plasmonic Imaging. Chem 2021, 7, 1569–1587. [Google Scholar] [CrossRef]
- Hoejholt, K.L.; Mužić, T.; Jensen, S.D.; Dalgaard, L.T.; Bilgin, M.; Nylandsted, J.; Heimburg, T.; Frandsen, S.K.; Gehl, J. Calcium Electroporation and Electrochemotherapy for Cancer Treatment: Importance of Cell Membrane Composition Investigated by Lipidomics, Calorimetry and in Vitro Efficacy. Sci. Rep. 2019, 9, 4758. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baffou, G.; Quidant, R.; Girard, C. Heat Generation in Plasmonic Nanostructures: Influence of Morphology. Appl. Phys. Lett. 2009, 94, 153109. [Google Scholar] [CrossRef] [Green Version]
- Gervits, N.E.; Gippius, A.A.; Tkachev, A.V.; Demikhov, E.I.; Starchikov, S.S.; Lyubutin, I.S.; Vasiliev, A.L.; Chekhonin, V.P.; Abakumov, M.A.; Semkina, A.S.; et al. Magnetic Properties of Biofunctionalized Iron Oxide Nanoparticles as Magnetic Resonance Imaging Contrast Agents. Beilstein J. Nanotechnol. 2019, 10, 1964–1972. [Google Scholar] [CrossRef] [Green Version]
- Nikitin, A.A.; Shchetinin, I.V.; Tabachkova, N.Y.; Soldatov, M.A.; Soldatov, A.V.; Sviridenkova, N.V.; Beloglazkina, E.K.; Savchenko, A.G.; Fedorova, N.D.; Abakumov, M.A.; et al. Synthesis of Iron Oxide Nanoclusters by Thermal Decomposition. Langmuir 2018, 34, 4640–4650. [Google Scholar] [CrossRef]
- Azevedo, R.C.S.; Sousa, R.G.; Macedo, W.A.A.; Sousa, E.M.B. Combining Mesoporous Silica–Magnetite and Thermally-Sensitive Polymers for Applications in Hyperthermia. J. Sol-Gel Sci. Technol. 2014, 72, 208–218. [Google Scholar] [CrossRef]
- Steinmark, I.E.; James, A.L.; Chung, P.-H.; Morton, P.E.; Parsons, M.; Dreiss, C.A.; Lorenz, C.D.; Yahioglu, G.; Suhling, K. Targeted Fluorescence Lifetime Probes Reveal Responsive Organelle Viscosity and Membrane Fluidity. PLoS ONE 2019, 14, e0211165. [Google Scholar] [CrossRef]
- Maeda, H.; Maeda, Y. Spectroscopic Ellipsometry Study on Refractive Index Spectra of Colloidal β-FeOOH Nanorods with Their Self-Assembled Thin Films. Langmuir 2011, 27, 2895–2903. [Google Scholar] [CrossRef]
- Erdem, D.; Bingham, N.S.; Heiligtag, F.J.; Pilet, N.; Warnicke, P.; Heyderman, L.J.; Niederberger, M. CoFe2O4 and CoFe2O4-SiO2 Nanoparticle Thin Films with Perpendicular Magnetic Anisotropy for Magnetic and Magneto-Optical Applications. Adv. Funct. Mater. 2016, 26, 1954–1963. [Google Scholar] [CrossRef]
- Melamed, J.R.; Edelstein, R.S.; Day, E.S. Elucidating the Fundamental Mechanisms of Cell Death Triggered by Photothermal Therapy. ACS Nano 2015, 9, 6–11. [Google Scholar] [CrossRef]
- Estelrich, J.; Busquets, M. Iron Oxide Nanoparticles in Photothermal Therapy. Molecules 2018, 23, 1567. [Google Scholar] [CrossRef] [Green Version]
- Shi, D.; Sadat, M.E.; Dunn, A.W.; Mast, D.B. Photo-Fluorescent and Magnetic Properties of Iron Oxide Nanoparticles for Biomedical Applications. Nanoscale 2015, 7, 8209–8232. [Google Scholar] [CrossRef]
- Shen, S.; Wang, S.; Zheng, R.; Zhu, X.; Jiang, X.; Fu, D.; Yang, W. Magnetic Nanoparticle Clusters for Photothermal Therapy with Near-Infrared Irradiation. Biomaterials 2015, 39, 67–74. [Google Scholar] [CrossRef] [PubMed]
- Nemec, S.; Kralj, S.; Wilhelm, C.; Abou-Hassan, A.; Rols, M.-P.; Kolosnjaj-Tabi, J. Comparison of Iron Oxide Nanoparticles in Photothermia and Magnetic Hyperthermia: Effects of Clustering and Silica Encapsulation on Nanoparticles’ Heating Yield. Appl. Sci. 2020, 10, 7322. [Google Scholar] [CrossRef]
- Chung, T.; Lee, S.-Y.; Song, E.Y.; Chun, H.; Lee, B. Plasmonic Nanostructures for Nano-Scale Bio-Sensing. Sensors 2011, 11, 10907–10929. [Google Scholar] [CrossRef] [Green Version]
- Montaño-Priede, J.L.; Pal, U. Estimating Near Electric Field of Polyhedral Gold Nanoparticles for Plasmon-Enhanced Spectroscopies. J. Phys. Chem. C 2019, 123, 11833–11839. [Google Scholar] [CrossRef]
- Paria, D.; Zhang, C.; Barman, I. Towards Rational Design and Optimization of Near-Field Enhancement and Spectral Tunability of Hybrid Core-Shell Plasmonic Nanoprobes. Sci. Rep. 2019, 9, 16071. [Google Scholar] [CrossRef] [Green Version]
- Agrawal, A.; Kriegel, I.; Milliron, D.J. Shape-Dependent Field Enhancement and Plasmon Resonance of Oxide Nanocrystals. J. Phys. Chem. C 2015, 119, 6227–6238. [Google Scholar] [CrossRef] [Green Version]
- Lio, G.E.; Palermo, G.; De Luca, A.; Caputo, R. Tensile Control of the Thermal Flow in Plasmonic Heaters Realized on Flexible Substrates. J. Chem. Phys. 2019, 151, 244707. [Google Scholar] [CrossRef] [PubMed]
- Ovejero, J.G.; Armenia, I.; Serantes, D.; Veintemillas-Verdaguer, S.; Zeballos, N.; López-Gallego, F.; Grüttner, C.; De La Fuente, J.M.; Puerto Morales, M.D.; Grazu, V. Selective Magnetic Nanoheating: Combining Iron Oxide Nanoparticles for Multi-Hot-Spot Induction and Sequential Regulation. Nano Lett. 2021, 21, 7213–7220. [Google Scholar] [CrossRef] [PubMed]




| k [W/m·K] | ρ [kg/m3] | cp [J/Mol·K] | References | |
|---|---|---|---|---|
| Fe2O3 | 7 | 5240 | 104 | [41,42] |
| Fe3O4 | 5.9 | 5170 | 104 | [43] |
| water | 0.6 | 1028 | 4182 | [44] |
| cells | 0.4 | 1028 | 4182 | [45] |
| cell membrane | 0.2 | 997 | 30 | [44,46] |
| Shape Size of Magnetic Core [nm] Phase Composition | Microphotograph of NPs | Size Distribution | |
|---|---|---|---|
| 1 | Spheres 6 nm 6 ± 2 nm Fe2O3 Hydrodynamic diameter of clusters 37.28 ± 0.11 nm, average number of magnetic cores in a cluster 4 ± 2 | 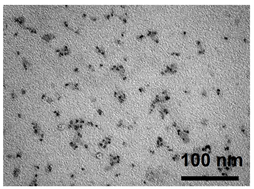 | 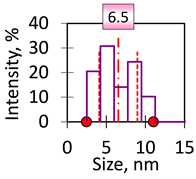 |
| 2 | Sphere-clusters 35 nm 35 ± 7 nm Fe3O4 |  | 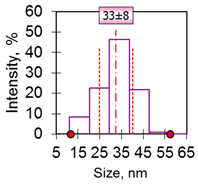 |
| 3 | Cube 15 nm 15 ± 4 nm Fe3O4 |  | 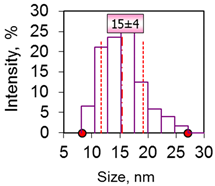 |
| 4 | Cube 37 nm 37 ± 6 nm Fe3O4 |  |  |
| 5 | Rods 14 nm 14 ± 4 nm (length), 3 ± 1 nm (diameter) β-FeOOH |  | 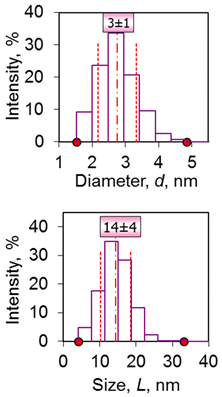 |
| 6 | Rods 20 nm 20 ± 5 nm (length) 6 ± 1 nm (diameter) iron carboxymaltose |  | 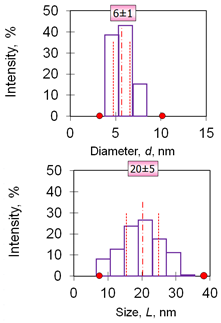 |
| 7 | Octopodes 14 nm 14 ± 2 nm Fe3O4 |  |  |
| 8 | Octopodes 31 nm 31 ± 4 nm CoFe2O4 |  |  |
| 9 | Hexagons 50 nm 50 ± 25 nm γ-Fe2O3 |  | 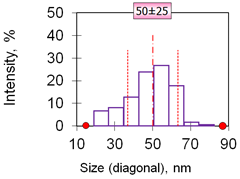 |
| NPs | ΔT, °C | |E/E0| | ΔT, °C | |E/E0| | |
|---|---|---|---|---|---|
| λ = 420 nm | λ = 840 nm | ||||
| Fe₃O₄ | |||||
| cubes 15 nm | single NP | 2.5 | 1.4 | 5.6 | 1.2 |
| array 3 × 3 × 3 | 55.9 | 1.5 | 127.0 | 1.3 | |
| array 5 × 5 × 3 | 154.0 | 1.7 | 355.0 | 1.5 | |
| cubes 35 nm | single NP | 28.8 | 1.8 | 66.7 | 1.2 |
| spheres 15 nm | single NP | 1.0 | 2.2 | 2.3 | 1.8 |
| spheres 35 nm | single NP | 11.8 | 2.0 | 26.7 | 1.8 |
| rods 15 nm | single NP (along x) | 0.0 | 1.6 | 0.1 | 1.5 |
| single NP (along y) | 0.1 | 2.3 | 0.2 | 1.5 | |
| single NP (along z) | 0.0 | 1.6 | 0.1 | 2.2 | |
| rods 20 nm | single NPs (along x) | 0.1 | 1.6 | 0.3 | 1.5 |
| single NP (along y) | 0.4 | 2.5 | 0.8 | 1.7 | |
| single NP (along z) | 0.1 | 1.6 | 0.3 | 1.6 | |
| Fe₂O₃ | |||||
| cubes 15 nm | single NP | 76.8 | 1.6 | 0.8 | 1.4 |
| cubes 35 nm | single NP | 450.0 | 3.1 | 9.5 | 1.7 |
| spheres 15 nm | single NP | 22.6 | 2.9 | 0.3 | 2.1 |
| spheres 35 nm | single NP | 292.0 | 2.6 | 3.3 | 2.1 |
| flakes 35 nm | single NP | 124.0 | 2.3 | 1.2 | 1.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryabova, A.; Pominova, D.; Markova, I.; Nikitin, A.; Ostroverkhov, P.; Lasareva, P.; Semkina, A.; Plotnikova, E.; Morozova, N.; Romanishkin, I.; et al. Fluorescent Microscopy of Hot Spots Induced by Laser Heating of Iron Oxide Nanoparticles. Photonics 2023, 10, 705. https://doi.org/10.3390/photonics10070705
Ryabova A, Pominova D, Markova I, Nikitin A, Ostroverkhov P, Lasareva P, Semkina A, Plotnikova E, Morozova N, Romanishkin I, et al. Fluorescent Microscopy of Hot Spots Induced by Laser Heating of Iron Oxide Nanoparticles. Photonics. 2023; 10(7):705. https://doi.org/10.3390/photonics10070705
Chicago/Turabian StyleRyabova, Anastasia, Daria Pominova, Inessa Markova, Aleksey Nikitin, Petr Ostroverkhov, Polina Lasareva, Alevtina Semkina, Ekaterina Plotnikova, Natalia Morozova, Igor Romanishkin, and et al. 2023. "Fluorescent Microscopy of Hot Spots Induced by Laser Heating of Iron Oxide Nanoparticles" Photonics 10, no. 7: 705. https://doi.org/10.3390/photonics10070705
APA StyleRyabova, A., Pominova, D., Markova, I., Nikitin, A., Ostroverkhov, P., Lasareva, P., Semkina, A., Plotnikova, E., Morozova, N., Romanishkin, I., Linkov, K., Abakumov, M., Pankratov, A., Steiner, R., & Loschenov, V. (2023). Fluorescent Microscopy of Hot Spots Induced by Laser Heating of Iron Oxide Nanoparticles. Photonics, 10(7), 705. https://doi.org/10.3390/photonics10070705








