A Practicable Optoelectronic Oscillator with Ultra-Low Phase Noise
Abstract
1. Introduction
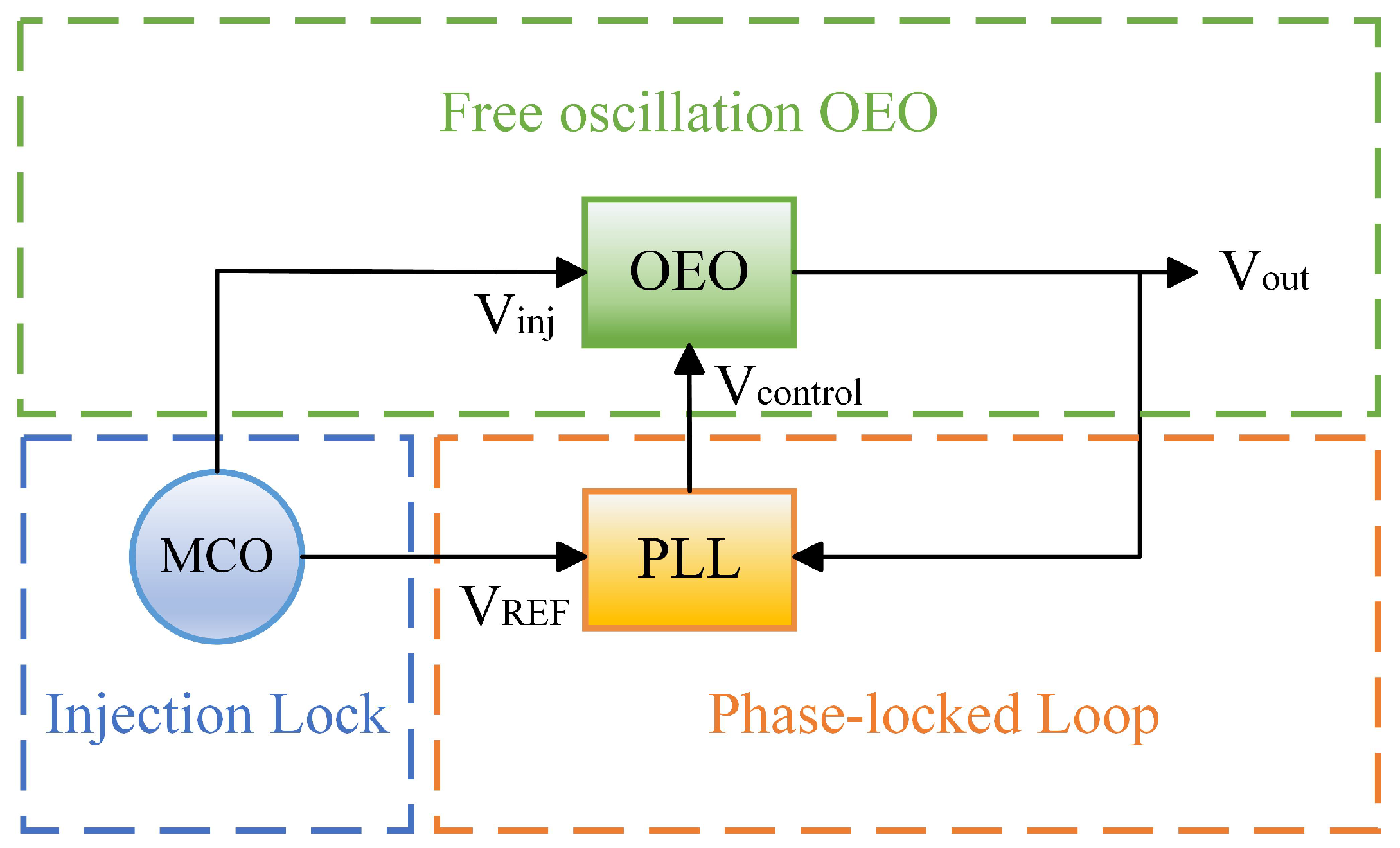
2. Experimental Principle
2.1. Injection-Locked Module
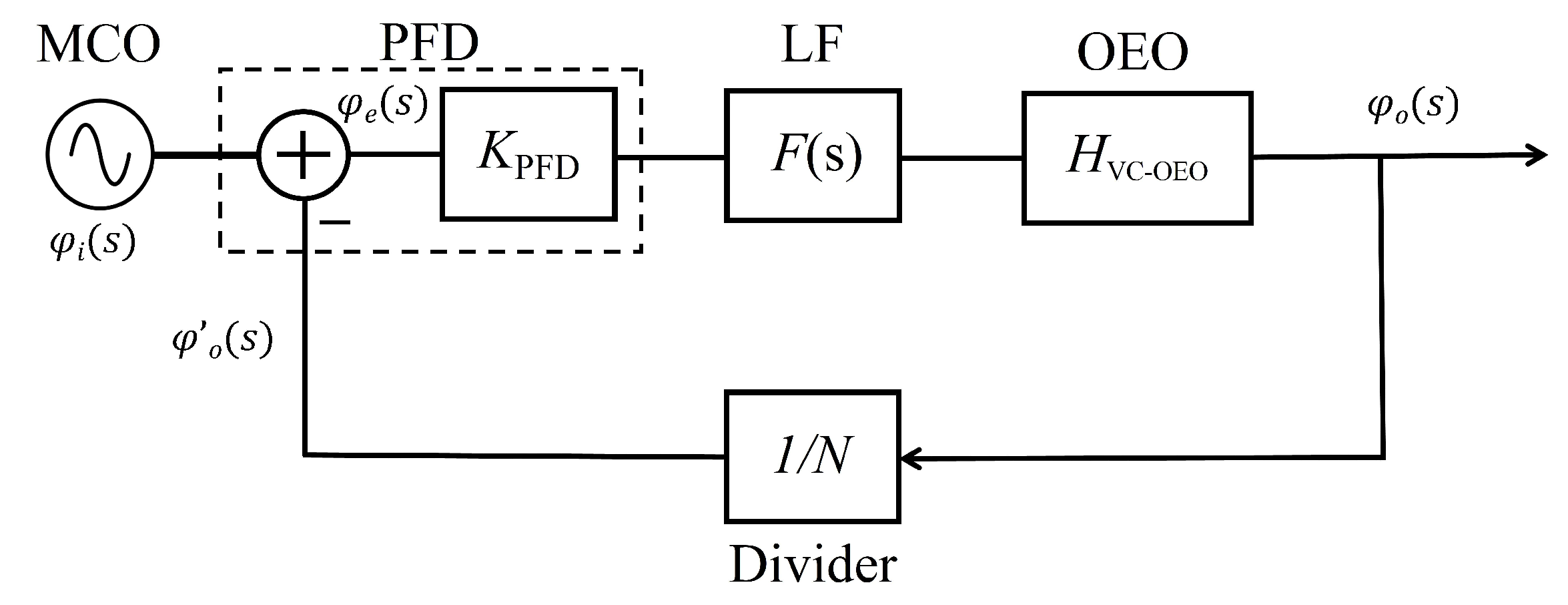
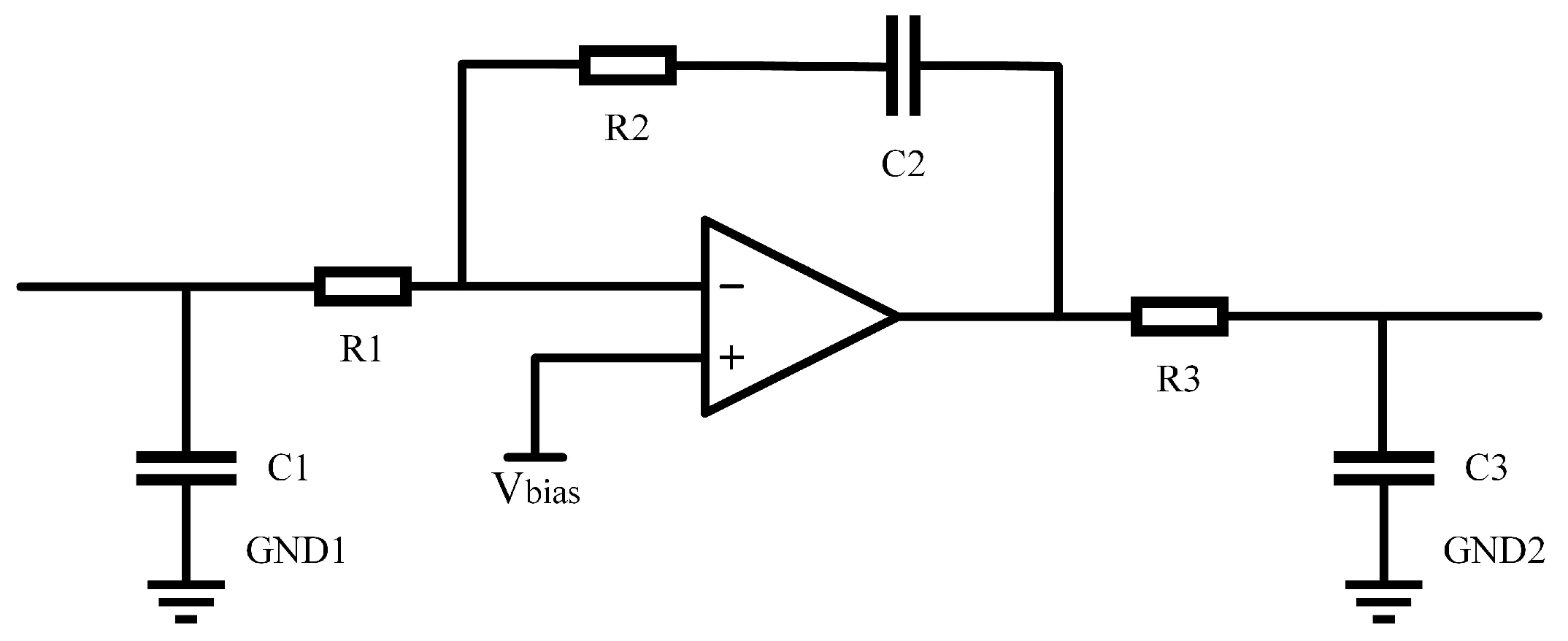
2.2. Phase-Locked Loop Module
3. Experimental Setup
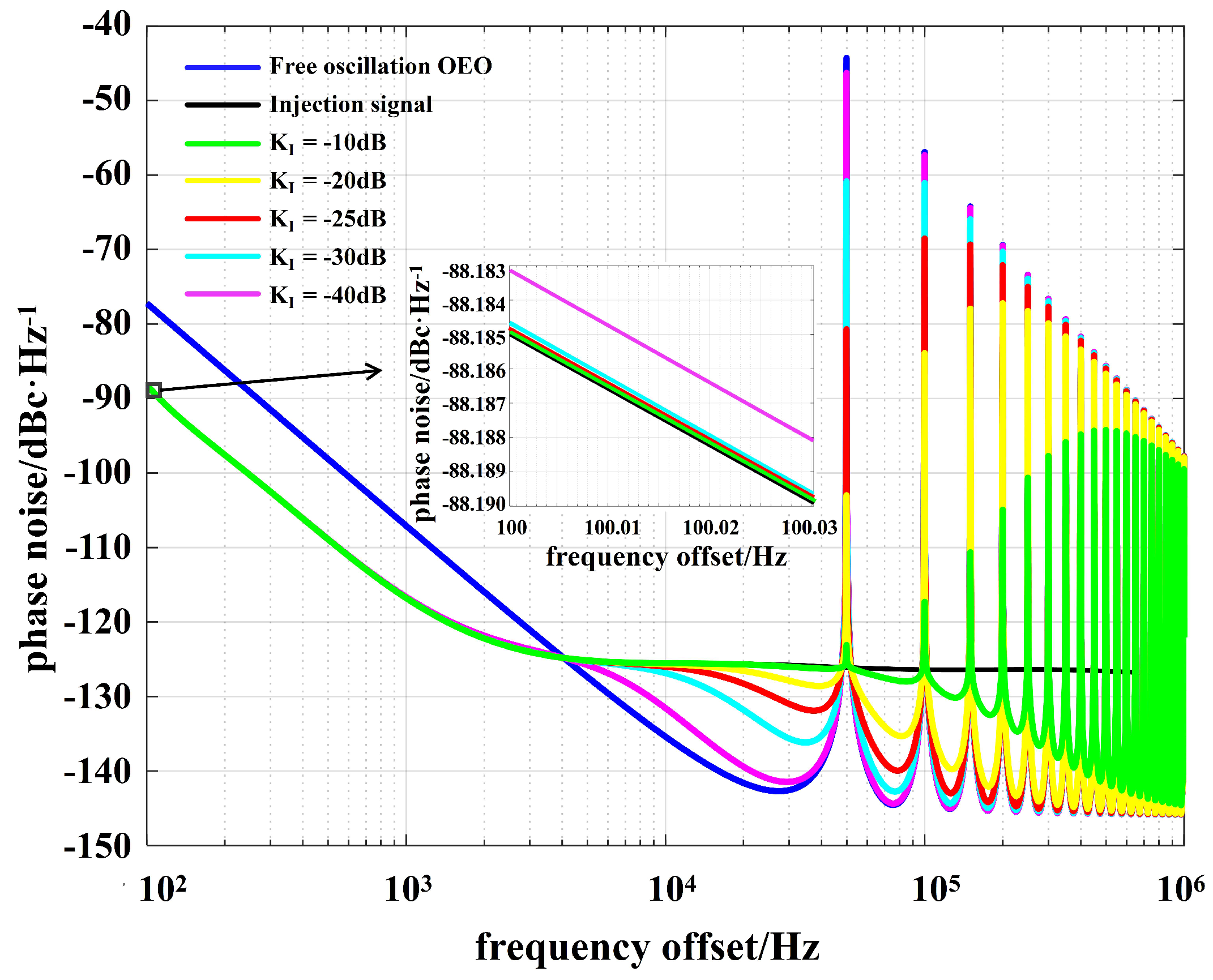
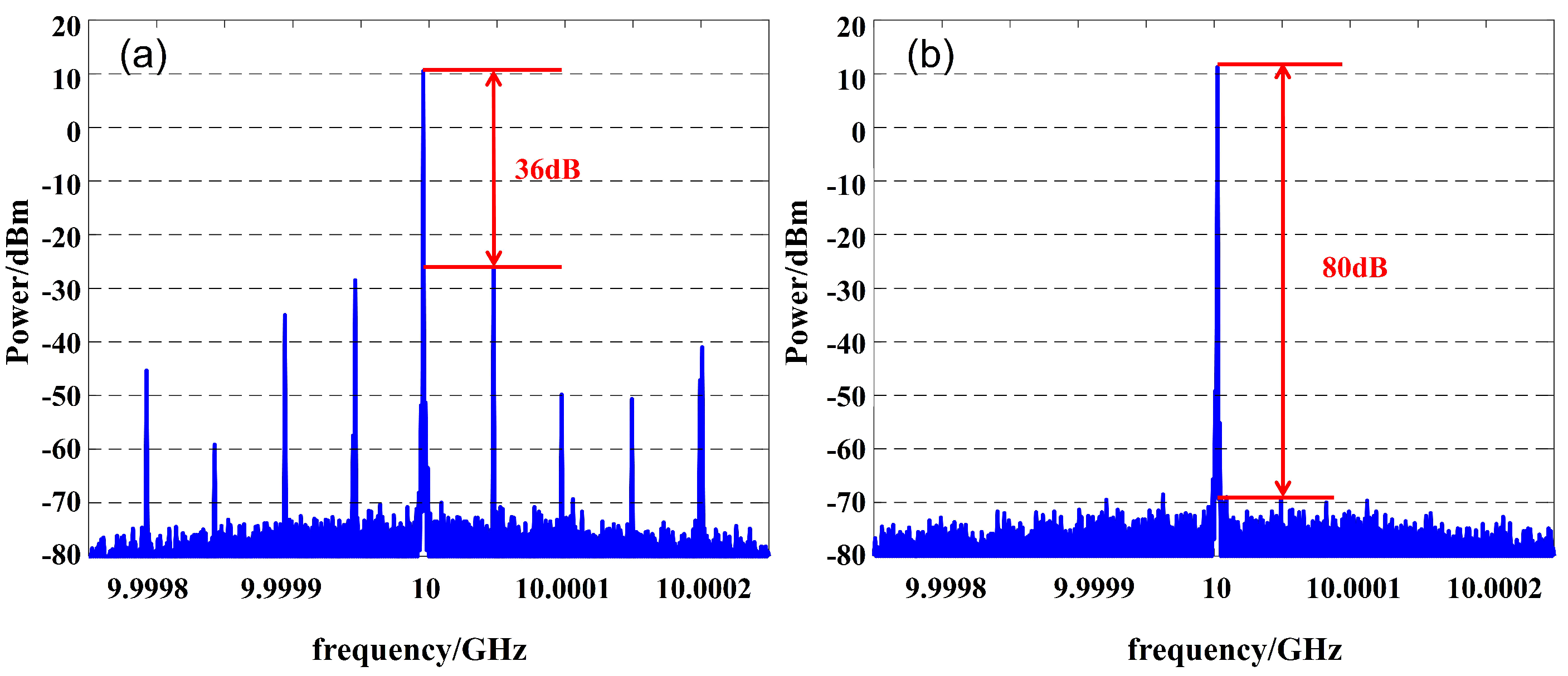
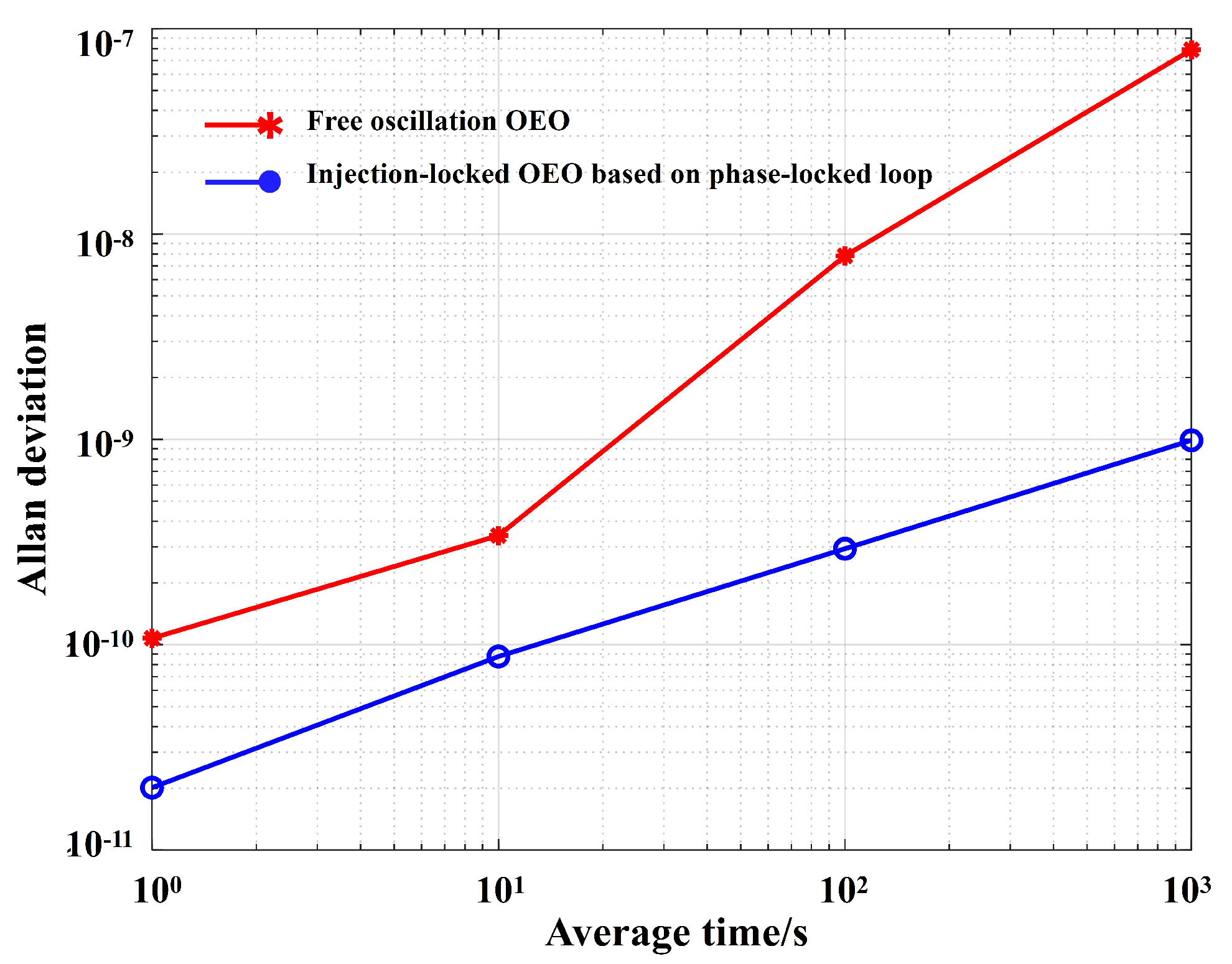
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pozar, D.M. Microwave Engineering, 4th ed.; John Wiley& Sons: Hoboken, NJ, USA, 2011; pp. 1–4. [Google Scholar]
- Prasad, R. Overview of Wireless Personal Communications: Microwave Perspectives. IEEE Commun. Mag. 1997, 35, 104–108. [Google Scholar] [CrossRef]
- Peng, Z.; Li, C. Portable Microwave Radar Systems for Short-Range Localization and Life Tracking: A Review. Sensors 2019, 19, 1136. [Google Scholar] [CrossRef] [PubMed]
- Rong, W.B.; Shan, X.R. Influence of Frequency Source Vibration on Velocity Measurement Accuracy. IEEE J. Spacecr. Tt C Technol. 2015, 34, 230–233. [Google Scholar]
- Yao, X.S.; Maleki, L. Converting light into spectrally pure microwave oscillation. Opt. Lett. 1996, 21, 483–485. [Google Scholar] [CrossRef]
- Yao, X.S.; Maleki, L. Optoelectronic microwave oscillato. J. Opt. Soc. Am. B 1996, 13, 34–35. [Google Scholar]
- Yao, X.S.; Maleki, L. Optoelectronic oscillator for photonic systems. IEEE J. Quantum Electron. 1996, 32, 1141–1149. [Google Scholar] [CrossRef]
- Chembo, Y.K.; Brunner, D.; Jacquot, M.; Larger, L. Optoelectronic oscillators with time-delayed feedback. Rev. Mod. Phys. 2019, 91, 035006. [Google Scholar] [CrossRef]
- Li, M.; Hao, T.; Li, W.; Dai, Y. Tutorial on optoelectronic oscillators. APL Photonics 2021, 6, 061101. [Google Scholar] [CrossRef]
- Yao, X.S.; Maleki, L.; Ji, Y.; Lutes, G.; Tu, M. A Dua loop optoelectronic oscillor. In IEEE International Frequency Control Symposium; IEEE: Piscataway, NJ, USA, 1998; pp. 545–549. [Google Scholar]
- Guo, T.; Han, Y.; Lu, G.; Qie, T. Frequency model of dual-loop optoelectronic oscillators. In Proceedings of the 2021 China Automation Congress, Beijing, China, 22–24 October 2021; pp. 7725–7728. [Google Scholar]
- Kim, J.-Y.; Jo, J.-H.; Choi, W.-Y.; Sung, H.-K. Dual-Loop Dual-Modulation Optoelectronic Oscillators with Highly Suppressed Spurious Tones. IEEE Photonics Technol. Lett. 2012, 24, 706–708. [Google Scholar] [CrossRef]
- Yao, X.S.; Maleki, L. Multiloop optoelectronic oscillator. IEEE J. Quantum Electron. 2000, 36, 79–84. [Google Scholar] [CrossRef]
- Eliyahu, D.; Sariri, K.; Taylor, J.; Lute, M. Opto-electronic Oscillator with Improved Phase Noise and Frequency Stability. Proc. SPIE 2003, 4998, 139–147. [Google Scholar]
- Fleyer, M.; Sherman, A.; Horowitz, M.; Namer, M. Wideband-frequency tunable optoelectronic oscillator based on injection locking to an electronic oscillator. Opt. Lett. 2016, 41, 1993–1996. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, A.; Britto, L.A.D.D.; Pacheco, G.M.A. Theoretical and Experimental Study of Injection-Locking and Injection-Pulling for Optoelectronic Oscillators Under Radio Frequency Signal Injection. J. Light. Technol. 2020, 38, 1210–1220. [Google Scholar] [CrossRef]
- Fan, Z.; Su, J.; Lin, Y.; Jiang, D.; Chen, Y.; Li, X.; Qiu, Q. Injection locking and pulling phenomena in an optoelectronic oscillator. Opt. Express 2024, 29, 4681–4699. [Google Scholar] [CrossRef]
- Lee, K.H.; Kim, J.Y.; Choi, W.Y. Injection-Locked Hybrid Optoelectronic Oscillators for Single-Mode Oscillation. IEEE Photonics Technol. Lett. 2008, 20, 1645–1647. [Google Scholar] [CrossRef]
- Lai, T.-H.; Jin, T.; Zhou, J.-H.; Chi, H.; Tong, G.-C.; Zhu, X.; Li, D.; Zuo, L.-L. Improving of Stability of Optoelectronic Oscillator Basedon Self-phase-locked Technique. Acta Photonica Sin. 2018, 47, 27–31. [Google Scholar]
- Wang, Y.F.; Yan, J.J. Stability improvement of a dual-loop optoelectronic oscillator based on self-phase locking. Appl. Opt. 2022, 61, 8912–8916. [Google Scholar] [CrossRef]
- Peng, H.; Liu, N.; Li, Y.; Xie, X.; Chen, Z. Low Phase Noise and Highly Stable Optoelectronic Oscillator by Using Frequency-Multiplying Phase Locked Loop. In Proceedings of the 2020 Joint Conference of the IEEE International Frequency Control Symposium and International Symposium on Applications of Ferroelectrics, Keystone, CO, USA, 19–23 July 2020; pp. 1–2. [Google Scholar]
- Zhang, Y.; Hou, D.; Zhao, J. Long-Term Frequency Stabilization of an Optoelectronic Oscillator Using Phase-Locked Loop. J. Light. Technol. 2014, 32, 2408–2414. [Google Scholar] [CrossRef]
- Fu, R.R.; Zhu, Y.H.; Jin, X.F. Frequency stabilization of an optoelectronic oscillator based on phase-locked loop. J. Spacecr. TT&C Technol. 2017, 36, 67–71. [Google Scholar]
- Eliyahu, D.; Sariri, K.; Kamran, A.; Tokhmakhian, M. Improving short and long term frequency stability of the opto-electronic oscillator. In Proceedings of the 2002 IEEE International Frequency Control Symposium and PDA Exhibition, New Orleans, LA, USA, 31 May 2002; pp. 580–583. [Google Scholar]
- Wang, X.; Yao, X.S. Phase-Locked Opto-Electronic Oscillator (OEO) of Ultralow Phase Noise With Record-Low Allan Deviation of 3.4 × 10−14 at 1 s. IEEE Clarivate Anal. Web Sci. 2023, 71, 5381–5391. [Google Scholar] [CrossRef]
- Banerjee, A.; de Britto, L.A.D.; Pacheco, G.M. Analysis of Injection Locking and Pulling in Single-Loop Optoelectronic Oscillator. IEEE Trans. Microw. Theory Tech. 2019, 67, 2087–2094. [Google Scholar] [CrossRef]
- Adler, R. A study of locking phenomena in oscillators. Proc. IEEE 1973, 61, 1380–1385. [Google Scholar] [CrossRef]
- Hosseini, S.E.; Banai, A. Theoretical investigation of the capture effect in intensity-modulation direct-detection microwave photonic links. Appl. Opt. 2013, 52, 7011–7021. [Google Scholar] [CrossRef] [PubMed]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, Z.; Yu, J.; Wang, J.; Ma, C.; Luo, H.; Su, X.; Gao, Y. A Practicable Optoelectronic Oscillator with Ultra-Low Phase Noise. Photonics 2024, 11, 614. https://doi.org/10.3390/photonics11070614
Zheng Z, Yu J, Wang J, Ma C, Luo H, Su X, Gao Y. A Practicable Optoelectronic Oscillator with Ultra-Low Phase Noise. Photonics. 2024; 11(7):614. https://doi.org/10.3390/photonics11070614
Chicago/Turabian StyleZheng, Ziyue, Jinlong Yu, Ju Wang, Chuang Ma, Hao Luo, Xuemin Su, and Ye Gao. 2024. "A Practicable Optoelectronic Oscillator with Ultra-Low Phase Noise" Photonics 11, no. 7: 614. https://doi.org/10.3390/photonics11070614
APA StyleZheng, Z., Yu, J., Wang, J., Ma, C., Luo, H., Su, X., & Gao, Y. (2024). A Practicable Optoelectronic Oscillator with Ultra-Low Phase Noise. Photonics, 11(7), 614. https://doi.org/10.3390/photonics11070614





