Dynamical Collective Excitations and Entanglement of Two Strongly Correlated Rydberg Superatoms
Abstract
:1. Introduction
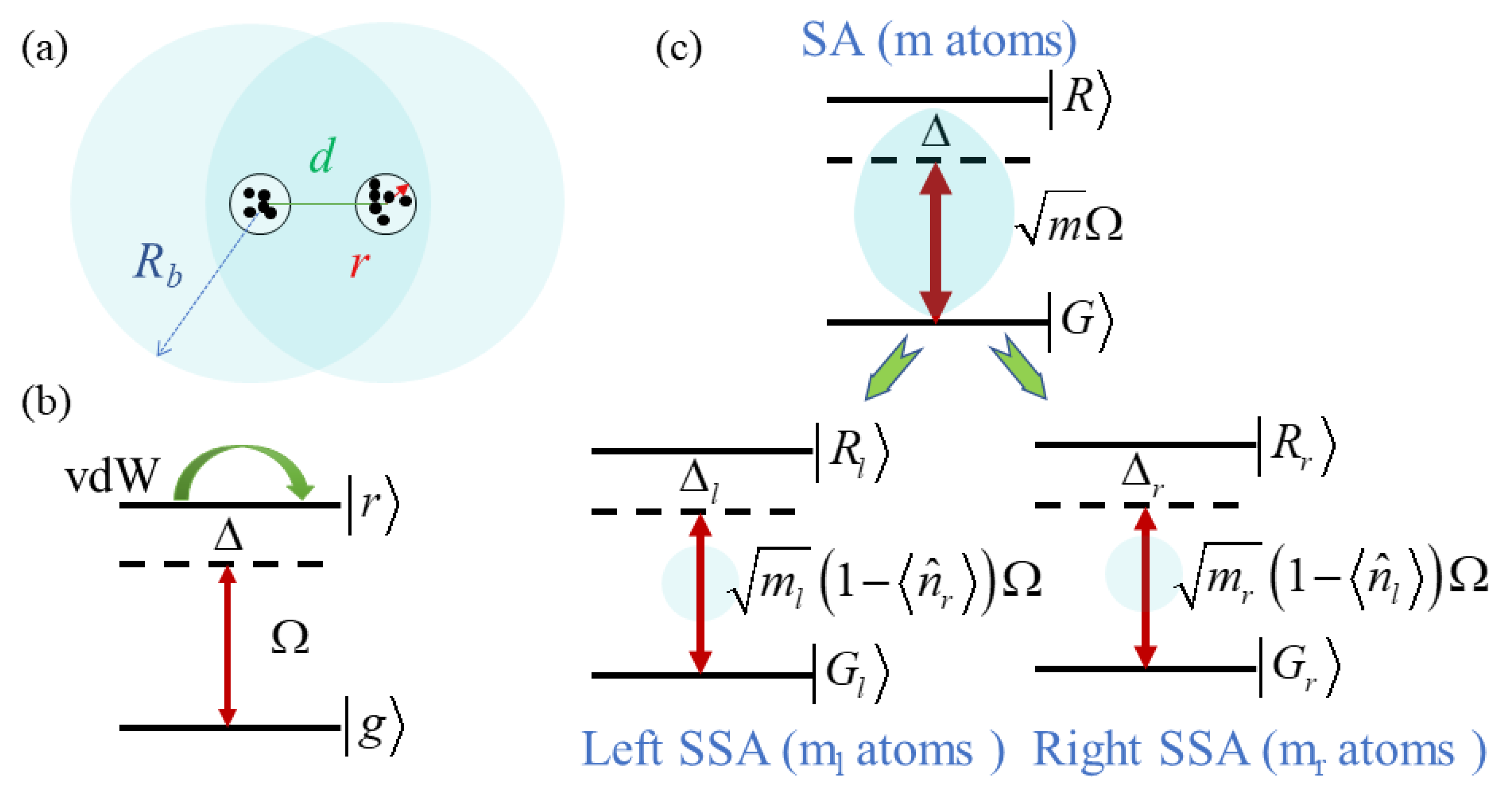
2. Model and Equations
3. Results and Discussions
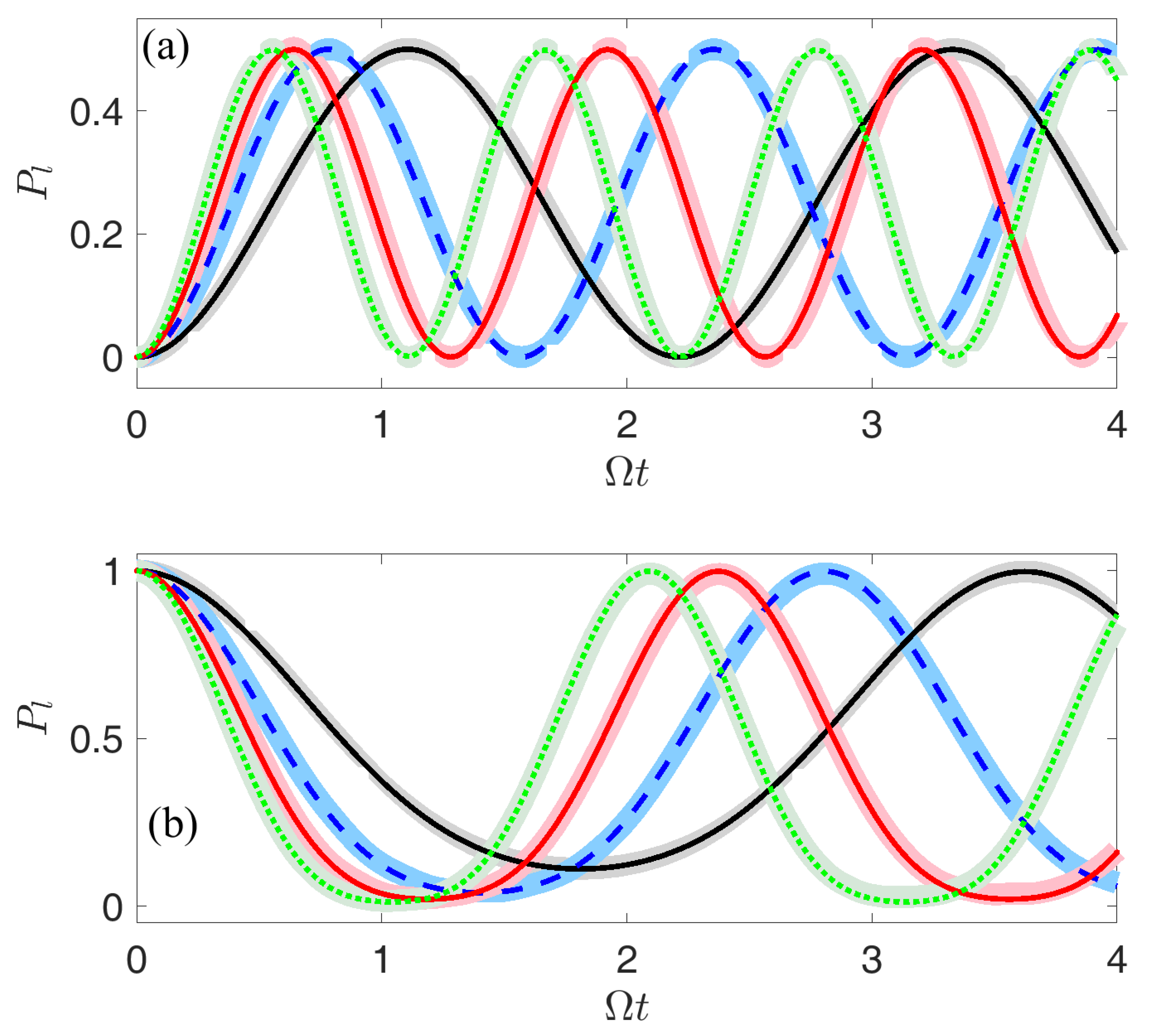
3.1. Time Evolution Starting from the Separable Collective States
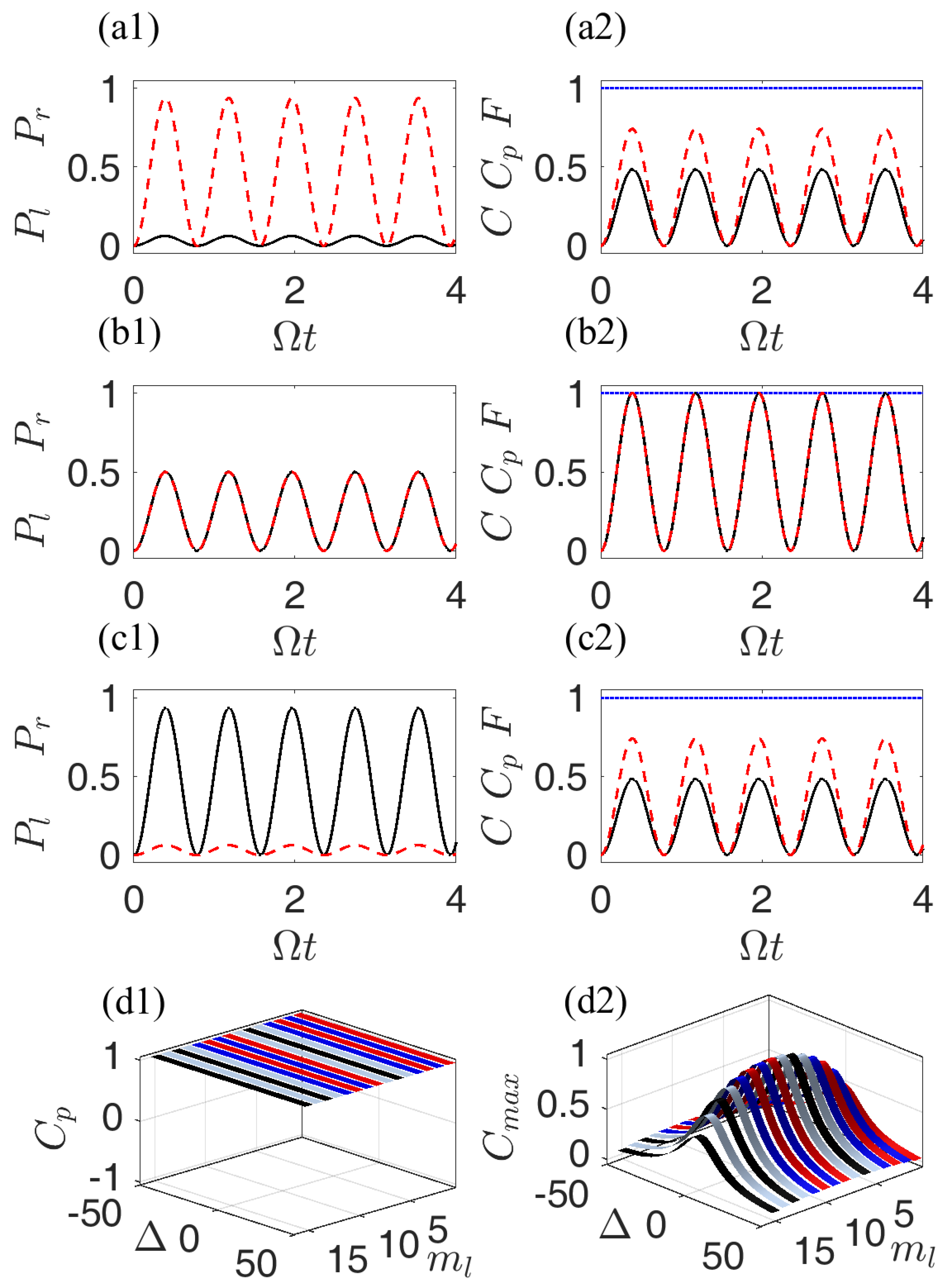
3.2. Time Evolution Starting from the Entangled Collective States
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jaksch, D.; Cirac, J.I.; Zoller, P.; Rolston, S.L.; Côté, R.; Lukin, M.D. Fast Quantum Gates for Neutral Atoms. Phys. Rev. Lett. 2000, 85, 2208–2210. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lukin, M.D.; Fleischhauer, M.; Côté, R.; Duan, L.M.; Jaksch, D.; Cirac, J.I.; Zoller, P. Dipole Blockade and Quantum Information Processing in Mesoscopic Atomic Ensembles. Phys. Rev. Lett. 2001, 87, 37901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Protsenko, I.E.; Reymond, G.; Schlosser, N.; Grangier, P. Operation of a quantum phase gate using neutral atoms in microscopic dipole traps. Phys. Rev. A 2002, 65, 52301. [Google Scholar] [CrossRef] [Green Version]
- Brion, E.; Mølmer, K.; Saffman, M. Quantum Computing with Collective Ensembles of Multilevel Systems. Phys. Rev. Lett. 2007, 99, 260501. [Google Scholar] [CrossRef] [Green Version]
- Wu, H.Z.; Yang, Z.B.; Zheng, S.B. Implementation of a multiqubit quantum phase gate in a neutral atomic ensemble via the asymmetric Rydberg blockade. Phys. Rev. A 2010, 82, 34307. [Google Scholar] [CrossRef]
- Wu, H.; Huang, X.-R.; Hu, C.-S.; Yang, Z.-B.; Zheng, S.-B. Rydberg-interaction gates via adiabatic passage and phase control of driving fields. Phys. Rev. A 2017, 96, 22321. [Google Scholar] [CrossRef] [Green Version]
- Shi, X.-F. Deutsch, Toffoli, and CNOT Gates via Rydberg Blockade of Neutral Atoms. Phys. Rev. Appl. 2018, 9, 51001. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.-L.; Wang, Y.; Han, J.-X.; Su, S.-L.; Xia, Y.; Jiang, Y.Y.; Song, J. Resilient quantum gates on periodically driven Rydberg atoms. Phys. Rev. A 2021, 103, 12601. [Google Scholar] [CrossRef]
- Weimer, H.; Müller, M.; Lesanovsky, I.; Zoller, P.; Büchler, H.P. A Rydberg quantum simulator. Nat. Phys. 2010, 6, 382–388. [Google Scholar] [CrossRef] [Green Version]
- Labuhn, H.; Barredo, D.; Ravets, S.; de Léséleuc, S.; Macrí, T.; Lahaye, T.; Browaeys, A. Tunable two-dimensional arrays of single Rydberg atoms for realizing quantum Ising models. Nature 2016, 534, 667–670. [Google Scholar] [CrossRef] [Green Version]
- Bernien, H.; Schwartz, S.; Keesling, A.; Levine, H.; Omran, A.; Pichler, H.; Choi, S.; Zibrov, A.S.; Endres, M.; Greiner, M.; et al. Probing many-body dynamics on a 51-atom quantum simulator. Nature 2017, 551, 579–584. [Google Scholar] [CrossRef] [PubMed]
- Barredo, D.; Lienhard, V.; de Léséleuc, S.; Lahaye, T.; Browaeys, A. Synthetic three-dimensional atomic structures assembled atom by atom. Nature 2018, 561, 79–82. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, H.; Park, Y.J.; Kim, K.; Sim, H.-S.; Ahn, J. Detailed Balance of Thermalization Dynamics in Rydberg-Atom Quantum Simulators. Phys. Rev. Lett. 2018, 120, 180502. [Google Scholar] [CrossRef] [Green Version]
- Robinson, M.P.; Laburthe-Tolra, B.; Noel, M.W.; Gallagher, T.F.; Pillet, P. Spontaneous Evolution of Rydberg Atoms into an Ultracold Plasma. Phys. Rev. Lett. 2000, 85, 4466. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bannasch, G.; Pohl, T. Rydberg-atom formation in strongly correlated ultracold plasmas. Phys. Rev. A 2011, 84, 52710. [Google Scholar] [CrossRef] [Green Version]
- Bannasch, G.; Killian, T.C.; Pohl, T. Strongly Coupled Plasmas via Rydberg Blockade of Cold Atoms. Phys. Rev. Lett. 2013, 110, 253003. [Google Scholar] [CrossRef] [Green Version]
- Robert-de-Saint-Vincent, M.; Hofmann, C.S.; Schempp, H.; Günter, G.; Whitlock, S.; Weidemüller, M. Spontaneous Avalanche Ionization of a Strongly Blockaded Rydberg Gas. Phys. Rev. Lett. 2013, 110, 45004. [Google Scholar] [CrossRef] [Green Version]
- Firstenberg, O.; Adams, C.S.; Hofferber, S. Nonlinear quantum optics mediated by Rydberg interactions. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 152003. [Google Scholar] [CrossRef]
- Liang, Q.-Y.; Venkatramani, A.V.; Cantu, S.H.; Nicholson, T.L.; Gullans, M.J.; Gorshkov, A.V.; Thompson, J.D.; Chin, C.; Lukin, M.D.; Vuletic, V. Observation of three-photon bound states in a quantum nonlinear medium. Science 2018, 359, 783–786. [Google Scholar] [CrossRef] [Green Version]
- Labuhn, H.; Ravets, S.; Barredo, D.; Béguin, L.; Nogrette, F.; Lahaye, T.; Browaeys, A. Single-atom addressing in microtraps for quantum-state engineering using Rydberg atoms. Phys. Rev. A 2014, 90, 23415. [Google Scholar] [CrossRef] [Green Version]
- Petrosyan, D.; Bhaktavatsala Rao, D.D.; Mølmer, K. Filtering single atoms from Rydberg-blockaded mesoscopic ensembles. Phys. Rev. A 2015, 91, 43402. [Google Scholar] [CrossRef] [Green Version]
- Saffman, M.; Walker, T.G. Creating single-atom and single-photon sources from entangled atomic ensembles. Phys. Rev. A 2002, 66, 65403. [Google Scholar] [CrossRef] [Green Version]
- Walker, T.G. Strongly interacting photons. Nature 2012, 488, 39–40. [Google Scholar] [CrossRef]
- Müller, M.; Kölle, A.; Löw, R.; Pfau, T.; Calarco, T.; Montangero, S. Room-temperature Rydberg single-photon source. Phys. Rev. A 2013, 87, 53412. [Google Scholar] [CrossRef] [Green Version]
- Dudin, Y.O.; Kuzmich, A. Strongly Interacting Rydberg Excitations of a Cold Atomic Gas. Science 2012, 336, 887–889. [Google Scholar] [CrossRef] [PubMed]
- Maxwell, D.; Szwer, D.J.; Paredes-Barato, D.; Busche, H.; Pritchard, J.D.; Gauguet, A.; Weatherill, K.J.; Jones, M.P.A.; Adams, C.S. Storage and Control of Optical Photons Using Rydberg Polaritons. Phys. Rev. Lett. 2013, 110, 103001. [Google Scholar] [CrossRef] [Green Version]
- Peyronel, T.; Firstenberg, O.; Liang, Q.-Y.; Hofferberth, S.; Gorshkov, A.V.; Pohl, T.; Lukin, M.D.; Vuletic, V. Quantum nonlinear optics with single photons enabled by strongly interacting atoms. Nature 2012, 488, 57–60. [Google Scholar] [CrossRef] [Green Version]
- Gorshkov, A.V.; Nath, R.; Pohl, T. Dissipative Many-Body Quantum Optics in Rydberg Media. Phys. Rev. Lett. 2013, 110, 153601. [Google Scholar] [CrossRef] [Green Version]
- Honer, J.; Löw, R.; Weimer, H.; Pfau, T.; Büchler, H.P. Artificial Atoms Can Do More Than Atoms: Deterministic Single Photon Subtraction from Arbitrary Light Fields. Phys. Rev. Lett. 2011, 107, 93601. [Google Scholar] [CrossRef] [Green Version]
- Gorshkov, A.V.; Otterbach, J.; Fleischhauer, M.; Pohl, T.; Lukin, M.D. Photon-Photon Interactions via Rydberg Blockade. Phys. Rev. Lett. 2011, 107, 133602. [Google Scholar] [CrossRef] [Green Version]
- Gorniaczyk, H.; Tresp, C.; Schmidt, J.; Fedder, H.; Hofferberth, S. Single-Photon Transistor Mediated by Interstate Rydberg Interactions. Phys. Rev. Lett. 2014, 113, 53601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Iiarks, D.; Baur, S.; Schneider, K.; Dürr, S.; Rempe, G. Single-Photon Transistor Using a Förster Resonance. Phys. Rev. Lett. 2014, 113, 53602. [Google Scholar]
- Chen, W.; Beck, K.M.; Bücker, R.; Gullans, M.; Lukin, M.D.; Tanji-Suzuki, H.; Vuletić, V. All-Optical Switch and Transistor Gated by One Stored Photon. Science 2013, 341, 768–770. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baur, S.; Tiarks, D.; Rempe, G.; Dürr, S. Single-Photon Switch Based on Rydberg Blockade. Phys. Rev. Lett. 2014, 112, 73901. [Google Scholar] [CrossRef]
- Tresp, C.; Zimmer, C.; Mirgorodskiy, I.; Gorniaczyk, H.A. Single-Photon Absorber Based on Strongly Interacting Rydberg Atoms. Phys. Rev. Lett. 2016, 117, 223001. [Google Scholar] [CrossRef]
- Friedler, I.; Kurizki, G.; Petrosyan, D. Deterministic quantum logic with photons via optically induced photonic band gaps. Phys. Rev. A 2005, 71, 23803. [Google Scholar] [CrossRef] [Green Version]
- Paredes-Barato, D.; Adams, C.S. All-Optical Quantum Information Processing Using Rydberg Gates. Phys. Rev. Lett. 2014, 112, 40501. [Google Scholar] [CrossRef] [Green Version]
- Yu, D. Single-photon emitter based on an ensemble of lattice-trapped interacting atoms. Phys. Rev. A 2014, 89, 63809. [Google Scholar] [CrossRef]
- Saffman, M.; Walker, T.G.; Mølmer, K. Quantum information with Rydberg atoms. Rev. Mod. Phys. 2010, 82, 2313. [Google Scholar] [CrossRef]
- Olmos, B.; Li, W.; Hofferberth, S.; Lesanovsky, I. Amplifying single impurities immersed in a gas of ultracold atoms. Phys. Rev. A 2011, 84, 41607. [Google Scholar] [CrossRef] [Green Version]
- Fan, C.H.; Zhang, H.X.; Wu, J.H. In-phase and antiphase dynamics of Rydberg atoms with distinguishable resonances. Phys. Rev. A 2019, 99, 33813. [Google Scholar] [CrossRef]
- Zhang, H.X.; Fan, C.H.; Wu, J.H. In-phase and anti-phase entanglement dynamics of Rydberg atomic pairs. Opt. Express 2020, 28, 35350–35362. [Google Scholar] [CrossRef] [PubMed]
- Müller, M.; Lesanovsky, I.; Weimer, H.; Buchler, H.P.; Zoller, P. Mesoscopic Rydberg Gate Based on Electromagnetically Induced Transparency. Phys. Rev. Lett. 2009, 102, 170502. [Google Scholar] [CrossRef] [Green Version]
- Carmele, A.; Vogell, B.; Stannigel, K.; Zoller, P. Opto-nanomechanics strongly coupled to a Rydberg superatom: Coherent versus incoherent dynamics. New J.Phys. 2014, 16, 63042. [Google Scholar] [CrossRef] [Green Version]
- Zhao, P.Z.; Wu, X.; Xing, T.H.; Xu, G.F.; Tong, D.M. Nonadiabatic holonomic quantum computation with Rydberg superatoms. Phys. Rev. A 2018, 98, 32313. [Google Scholar] [CrossRef] [Green Version]
- Nie, W.; Peng, Z.H.; Nori, F.; Liu, Y.-X. Topologically Protected Quantum Coherence in a Superatom. Phys. Rev. Lett. 2020, 124, 23603. [Google Scholar] [CrossRef] [Green Version]
- Petrosyan, D.; Otterbach, J.; Fleischhauer, M. Electromagnetically Induced Transparency with Rydberg Atoms. Phys. Rev. Lett. 2011, 107, 213601. [Google Scholar] [CrossRef]
- Stanojevic, J.; Côté, R. Many-body Rabi oscillations of Rydberg excitation in small mesoscopic samples. Phys. Rev. A 2009, 80, 33418. [Google Scholar] [CrossRef] [Green Version]
- Dür, W.; Vidal, G.; Cirac, J.I. Three qubits can be entangled in two inequivalent ways. Phys. Rev. A 2000, 62, 62314. [Google Scholar] [CrossRef] [Green Version]
- Heidemann, R.; Raitzsch, U.; Bendkowsky, V.; Butscher, B.; Löw, R.; Santos, L.; Pfau, T. Evidence for Coherent Collective Rydberg Excitation in the Strong Blockade Regime. Phys. Rev. Lett. 2007, 99, 163601. [Google Scholar] [CrossRef] [Green Version]
- Kumar, S.; Sheng, J.T.; Sedlacek, J.A.; Fan, H.Q.; Shaffer, J.P. Collective state synthesis in an optical cavity using Rydberg atom dipole blockade. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 64014. [Google Scholar] [CrossRef]
- Yan, D.; Liu, Y.-M.; Bao, Q.-Q.; Fu, C.-B.; Wu, J.-H. Electromagnetically induced transparency in an inverted-Y system of interacting cold atoms. Phys. Rev. A 2012, 86, 23828. [Google Scholar] [CrossRef]
- Liu, Y.-M.; Tian, X.-D.; Wang, X.; Yan, D.; Wu, J.-H. Cooperative nonlinear grating sensitive to light intensity and photon correlation. Opt. Lett. 2016, 41, 408–411. [Google Scholar] [CrossRef] [PubMed]
- Asghar, S.; Qamar, Z.S.; Qamar, S. Electromagnetically induced grating with Rydberg atoms. Phys. Rev. A 2016, 94, 33823. [Google Scholar] [CrossRef]
- Carmele, A.; Qamar, Z.S.; Qamar, S. Strong coupling of a Rydberg superatom to a moving membrane. Proc. SPIE 2015, 9357, 93570S. [Google Scholar]
- Bariani, F.; Otterbach, J.; Tan, H.; Meystre, P. Single-atom quantum control of macroscopic mechanical oscillators. Phys. Rev. A 2014, 89, 11801. [Google Scholar] [CrossRef] [Green Version]
- Yan, D.; Wang, Z.-H.; Ren, C.-N.; Gao, H.; Li, Y.; Wu, J.-H. Duality and bistability in an optomechanical cavity coupled to a Rydberg superatom. Phys. Rev. A 2015, 91, 23813. [Google Scholar] [CrossRef]
- Weber, T.M.; Höning, M.; Niederprüm, T.; Manthey, T.; Thomas, O.; Guarrera, V.; Fleischhauer, M.; Barontini, G.; Ott, H. Mesoscopic Rydberg-blockaded ensembles in the superatom regime and beyond. Nat. Phys. 2015, 11, 157–161. [Google Scholar] [CrossRef] [Green Version]
- Su, S.-L.; Gao, Y.; Liang, E.J.; Zhang, S. Fast Rydberg antiblockade regime and its applications in quantum logic gates. Phys. Rev. A 2017, 95, 22319. [Google Scholar] [CrossRef]
- Li, W.B.; Viscor, D.; Hofferberth, S.; Lesanovsky, I. Electromagnetically Induced Transparency in an Entangled Medium. Phys. Rev. Lett. 2014, 112, 243601. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.-M.; Tian, X.-D.; Yan, D.; Zhang, Y.; Cui, C.-L.; Wu, J.-H. Nonlinear modifications of photon correlations via controlled single and double Rydberg blockade. Phys. Rev. A 2015, 91, 43802. [Google Scholar] [CrossRef]
- Huygens, C. Horologium Oscillatorium; F. Muguet: Paris, France, 1673. [Google Scholar]
- Pikovsky, A.S.; Rosenblum, M.; Kurths, J. Synchronization: A Universal Concept in Nonlinear Science; Cambridge University Press: New York, NY, USA, 2001. [Google Scholar]
- Osipov, G.V.; Kurths, J.; Zhou, C. Synchronization in Oscillatory Networks; Springer Series in Synergetics; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Acebŕon, J.A.; Bonilla, L.L.; Ṕerez Vicente, C.J.; Ritort, F.; Spigler, R. The Kuramoto model: A simple paradigm for synchronization phenomena. Rev. Mod. Phys. 2005, 77, 137. [Google Scholar] [CrossRef] [Green Version]
- Lee, T.E.; Sadeghpour, H.R. Quantum Synchronization of Quantum van der Pol Oscillators with Trapped Ions. Phys. Rev. Lett. 2013, 111, 234101. [Google Scholar] [CrossRef] [Green Version]
- Walter, S.; Nunnenkamp, A.; Bruder, C. Quantum synchronization of two Van der Pol oscillators. Ann. Phys. 2015, 527, 131. [Google Scholar] [CrossRef] [Green Version]
- Giorgi, G.L.; Galve, F.; Zambrini, R. Probing the spectral density of a dissipative qubit via quantum synchronization. Phys. Rev. A 2016, 94, 52121. [Google Scholar] [CrossRef] [Green Version]
- Roulet, A.; Bruder, C. Synchronizing the Smallest Possible System. Phys. Rev. Lett. 2018, 121, 53601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hillbrand, J.; Auth, D.; Piccardo, M.; Opačak, N.; Gornik, E.; Strasser, G.; Capasso, F.; Breuer, S.; Schwarz, B. In-Phase and Anti-Phase Synchronization in a Laser Frequency Comb. Phys. Rev. Lett. 2020, 124, 23901. [Google Scholar] [CrossRef] [Green Version]
- Parra-López, Á.; Bergli, J. Synchronization in two-level quantum systems. Phys. Rev. A 2020, 101, 62104. [Google Scholar] [CrossRef]
- Bellomo, B.; Giorgi, G.L.; Palma, G.M.; Zambrini, R. Quantum synchronization as a local signature of super- and subradiance. Phys. Rev. A 2017, 95, 43807. [Google Scholar] [CrossRef] [Green Version]
- Karpat, G.; Yalçınkaya, İ.; Çakmak, B. Quantum synchronization in a collision model. Phys. Rev. A 2019, 100, 12133. [Google Scholar] [CrossRef] [Green Version]
- Karpat, G.; Yalçınkaya, İ.; Çakmak, B. Quantum synchronization of few-body systems under collective dissipation. Phys. Rev. A 2020, 101, 42121. [Google Scholar] [CrossRef]
- Jebarathinam, C.; Home, D.; Sinha, U. Pearson correlation coefficient as a measure for certifying and quantifying high-dimensional entanglement. Phys. Rev. A 2020, 101, 22112. [Google Scholar] [CrossRef] [Green Version]
- Wootters, W.K. Entanglement of Formation of an Arbitrary State of Two Qubits. Phys. Rev. Lett. 1998, 80, 2245. [Google Scholar] [CrossRef] [Green Version]
- Singer, K.; Stanojevic, J.; Weidemuller, M.; Côté, R. Long-range interactions between alkali Rydberg atom pairs correlated to the ns-ns, np-np and nd-nd asymptotes. J. Phys. B At. Mol. Opt. 2005, 38, S295. [Google Scholar] [CrossRef]
- Balewski, J.B.; Krupp, A.T.; Gaj, A.; Hofferberth, S.; Löw, R.; Pfau, T. Rydberg dressing: Understanding of collective many-body effects and implications for experiments. New J. Phys. 2014, 16, 63012. [Google Scholar] [CrossRef] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, D.; Bai, W.; Bai, J.; Chen, L.; Han, H.; Wu, J. Dynamical Collective Excitations and Entanglement of Two Strongly Correlated Rydberg Superatoms. Photonics 2022, 9, 242. https://doi.org/10.3390/photonics9040242
Yan D, Bai W, Bai J, Chen L, Han H, Wu J. Dynamical Collective Excitations and Entanglement of Two Strongly Correlated Rydberg Superatoms. Photonics. 2022; 9(4):242. https://doi.org/10.3390/photonics9040242
Chicago/Turabian StyleYan, Dong, Wenjie Bai, Jiannan Bai, Li Chen, Haiyan Han, and Jinhui Wu. 2022. "Dynamical Collective Excitations and Entanglement of Two Strongly Correlated Rydberg Superatoms" Photonics 9, no. 4: 242. https://doi.org/10.3390/photonics9040242
APA StyleYan, D., Bai, W., Bai, J., Chen, L., Han, H., & Wu, J. (2022). Dynamical Collective Excitations and Entanglement of Two Strongly Correlated Rydberg Superatoms. Photonics, 9(4), 242. https://doi.org/10.3390/photonics9040242




