The Role of Inorganic Fillers in Electrostatic Discharge Composites
Abstract
:1. Introduction
2. Electrostatic Charging Mechanisms and Definitions: A Phenomenological Survey
3. ESD Materials Classification and Protecting Capability
4. The Role of Conductive Fillers in ESD Composite Materials
- In the simplest case, when a single filler (either spherical particles or fibers) is dispersed into a single polymer matrix, the contact between the filler particles/fibers is reached at the percolation threshold and can be maximized by increasing the filler concentration above the percolation threshold (Figure 6, Panel A).
- If dual fillers (both spherical particles and fibers) are dispersed into a single polymer matrix, fibrous fillers connect the islands of granular fillers (short-distance conductivity), thus extending the connectivity (long-distance conductivity) of the network and consequently extending the electrically conductive network (Figure 6, Panel B).
- A single filler (either spherical particles or fibers) dispersed into a blend of two immiscible polymers may interact differently with the two polymeric matrices, causing the formation of a conductive network either within a particular polymer phase or at the interface between the two immiscible polymers. This may result in the formation of a filler-rich polymeric phase. To be electrically conductive, these composites require a rich phase of fillers forming a continuous phase [100] (Figure 6, Panel C).
- Dual fillers (both spherical particles and fibers) are dispersed into a blend of two immiscible polymers. The related systems show relevant bridging effects, low percolation threshold, and high conductivity (Figure 6, Panel D).
5. A Survey on the Chemical Nature of Fillers for ESD Composites from the Inorganic Chemistry Viewpoint
6. Actual Technological Solutions
7. Conclusions and Perspectives
Author Contributions
Funding
Conflicts of Interest
References
- Voldman, S.H. ESD: Physics and Devices; Wiley: Hoboken, NY, USA, 2004; ISBN 978-0-4708-4753-4. [Google Scholar]
- Kaiser, K.L. Electrostatic Discharge; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2006; ISBN 978-0-8493-7188-2. [Google Scholar]
- Jonassen, N. Electrostatics; Springer Science & Business Media: Boston, MA, USA, 1998; ISBN 978-0-4757-1184-4. [Google Scholar]
- Voldman, S.H. A review of electrostatic discharge (ESD) in advanced semiconductor technology. Microelectron. Reliab. 2004, 44, 33–46. [Google Scholar] [CrossRef]
- Semenov, O.; Somov, S. ESD protection design for I/O libraries in advanced CMOS technologies. Solid State Electron. 2008, 52, 1127–1139. [Google Scholar] [CrossRef]
- Smallwood, J. ESD in industry—Present and future. J. Phys. Conf. Ser. 2015, 646, 012018. [Google Scholar] [CrossRef]
- Okoniewska, E.; Stuchly, M.A.; Okoniewski, M. Interactions of electrostatic discharge with the human body. IEEE T Microw. Theory 2004, 52, 2030–2039. [Google Scholar] [CrossRef]
- Wang, A.Z.; Feng, H.G.; Gong, K.; Zhan, R.Y.; Stine, J. On-chip ESD protection design for integrated circuits: An overview for IC designers. Microelectron. J. 2001, 32, 733–747. [Google Scholar] [CrossRef]
- ESDA.org. Available online: https://www.esda.org (accessed on 14 January 2020).
- Incompliancemag.com. Fundamentals of Electrostatic Discharge Part One—An introduction to ESD. Available online: https://incompliancemag.com/article/fundamentals-of-electrostatic-discharge-part-one-an-introduction-to-esd/ (accessed on 29 April 2020).
- Voldman, S.H. Evolution, revolution, and technology scaling—The impact on ESD and EOS reliability. Front. Mater. 2018, 5, 33. [Google Scholar] [CrossRef]
- Hu, Y.; Wang, D.; Liu, J.; Gao, J. A case study of the electrostatic accidents in the process of oil-gas storage and transportation. J. Phys. Conf. Ser. 2013, 418, 012037. [Google Scholar] [CrossRef]
- Choi, K.; Sakasai, H.; Nishimura, L. Experimental study on the ignitability of pure aluminum powders due to electrostatic discharge and nitrogen’s effect. J. Loss Prevent. Proc. 2015, 35, 232–235. [Google Scholar] [CrossRef]
- Wu, Z.; Chen, Y.; Hu, X.; Liu, S. Research on ESD ignition hazards of textiles. J. Electrostat. 2003, 57, 203–207. [Google Scholar] [CrossRef]
- Ge, C.; Cosgrove, K. Preparation of PVOH coatings with graphene nanoplatelets for electrostatics discharge protective packaging. J. Electrostat. 2015, 77, 157–162. [Google Scholar] [CrossRef]
- Koul, S.; Chandra, R.; Dhawan, S.K. Conducting polyaniline composite for ESD and EMI at 101 GHz. Polymer 2000, 41, 9305–9310. [Google Scholar] [CrossRef]
- Tsurumaki, A.; Tajima, S.; Iwata, T.; Scrosati, B.; Ohno, H. Antistatic effects of ionic liquids for polyether-based polyurethanes. Electrochim. Acta 2015, 175, 13–17. [Google Scholar] [CrossRef]
- Narkis, M.; Lidor, G.; Vaxman, A.; Zuri, L. New injectable moldable electrostatic dissipative (ESD) composites based on very low carbon black loadings. J. Electrostat. 1999, 47, 201–214. [Google Scholar] [CrossRef]
- Wang, C.X.; Lv, J.C.; Ren, Y.; Zhi, T.; Chen, J.Y.; Zhou, Q.Q.; Lu, Z.Q.; Gao, D.W.; Jin, L.M. Surface modification of polyester fabric with plasma pretreatment and carbon nanotube coating for antistatic property improvement. Appl. Surf. Sci. 2015, 359, 196–203. [Google Scholar] [CrossRef]
- Li, C.; Liang, T.; Lu, W.; Tang, C.; Hu, X.; Cao, M.; Liang, J. Improving the antistatic ability of polypropylene fibers by inner antistatic agent filled with carbon nanotubes. Compos. Sci. Technol. 2004, 64, 2089–2096. [Google Scholar] [CrossRef]
- Dharmasena, R.D.I.G.; Silva, S.R.P. Towards optimized triboelectric nanogenerators. Nano Energy 2019, 62, 530–549. [Google Scholar] [CrossRef]
- Chen, J.; Qiu, Q.; Han, Y.; Lau, D. Piezoelectric materials for sustainable building structures: Fundamentals and applications. Renew. Sust. Energ. Rev. 2019, 101, 14–25. [Google Scholar] [CrossRef]
- Zabek, D.; Morini, F. Solid state generators and energy harvesters for waste heat recovery and thermal energy harvesting. Therm. Sci. Eng. Prog. 2019, 9, 235–247. [Google Scholar] [CrossRef]
- Yang, X.; Xu, C.; Kunieda, M. Miniaturization of WEDM using electrostatic induction feeding method. Precis. Eng. 2010, 34, 279–285. [Google Scholar] [CrossRef]
- McCarty, L.S.; Whitesides, G.M. Electrostatic charging due to separation of ions at interfaces: Contact electrification of ionic electrets. Angew. Chem. Int. Ed. 2008, 47, 2188–2207. [Google Scholar] [CrossRef]
- Galembeck, F.; Burgo, T.A.L.; Balestrin, L.B.S.; Gouveia, R.F.; Silva, C.A.; Galembeck, A. Friction, tribochemistry, and triboelectricity: Recent progress and perspectives. RSC Adv. 2014, 4, 64280–64298. [Google Scholar] [CrossRef]
- Freeman, G.R.; March, N.H. Triboelectricity and some associated phenomena. Mater. Sci. Technol. 1999, 15, 1454–1458. [Google Scholar] [CrossRef]
- Matsusaka, S.; Maruyama, H.; Matsuyama, T.; Ghadiri, M. Triboelectric charging of powders: A review. Chem. Eng. Sci. 2010, 65, 5781–5807. [Google Scholar] [CrossRef] [Green Version]
- Zou, H.; Zhang, Y.; Guo, L.; Wang, P.; He, X.; Dai, G.; Zheng, H.; Chen, C.; Wang, A.C.; Xu, C.; et al. Quantifying the triboelectric series. Nat. Commun. 2019, 10, 1427. [Google Scholar] [CrossRef] [Green Version]
- Diaz, A.F.; Felix-Navarro, R.M. A semi-quantitative tribo-electric series for polymeric materials: The influence of chemical structure and properties. J. Electrostat. 2004, 62, 277–290. [Google Scholar] [CrossRef]
- Henniker, J. Triboelectricity in polymers. Nature 1962, 196, 474. [Google Scholar] [CrossRef]
- Kim, W.; Bhatia, D.; Hwang, H.J.; Choi, K.; Choi, D. Double impact triboelectric nanogenerators for harvesting broadband vibrations from vehicles. Funct. Compos. Struct. 2019, 1, 035003. [Google Scholar] [CrossRef]
- Xu, M.; Zhao, T.; Wang, C.; Zhang, S.L.; Li, Z.; Pan, X.; Wang, Z.L. High power density tower-like triboelectric nanogenerator for harvesting arbitrary directional water wave energy. ACS Nano 2019, 13, 1932–1939. [Google Scholar] [CrossRef]
- Feng, Y.; Zhang, L.; Zheng, Y.; Wang, D.; Zhou, F.; Liu, W. Leaves based triboelectric nanogenerator (TENG) and TENG tree for wind energy harvesting. Nano Energy 2019, 55, 260–268. [Google Scholar] [CrossRef]
- Xia, K.; Zhu, Z.; Zhang, H.; Du, C.; Fu, J.; Xu, Z. Milk-based triboelectric nanogenerator on paper for harvesting energy from human body motion. Nano Energy 2019, 56, 400–410. [Google Scholar] [CrossRef]
- Zheng, Q.; Shi, B.; Li, Z.; Wang, Z.L. Recent progress on piezoelectric and triboelectric energy harvesters in biomedical systems. Adv. Sci. 2017, 4, 1700029. [Google Scholar] [CrossRef]
- Americanpiezo.com. Piezoelectricity. Available online: https://www.americanpiezo.com/knowledge-center/piezo-theory/piezoelectricity.html (accessed on 29 April 2020).
- Goel, S.; Kumar, B. A review on piezo-/ferro-electric properties of morphologically diverse ZnO nanostructures. J. Alloys Compd. 2010, 816, 152491. [Google Scholar] [CrossRef]
- Song, R.; Zhao, Y.; Li, W.; Yu, Y.; Sheng, J.; Li, Z.; Zhang, Y.; Xia, H.; Fei, W.-D. High temperature stability and mechanically quality factor of donor-acceptor co-doped BaTiO3 piezoelectrics. Acta Mater. 2019, 181, 200–206. [Google Scholar] [CrossRef]
- Hao, J.; Li, W.; Zhai, J.; Chen, H. Progress in high-strain perovskite piezoelectric ceramics. Mater. Sci. Eng. R. 2019, 135, 1–57. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, H.; Chen, W. A brief review of Ba(Ti0.8Zr0.2)O3-(Ba0.7Ca0.3)TiO3 based lead-free piezoelectric ceramics: Past, present and future perspectives. J. Phys. Chem. Solids 2018, 114, 207–219. [Google Scholar] [CrossRef]
- Li, J.; Long, Y.; Yang, F.; Wang, X. Degradable piezoelectric biomaterials for wearable and implantable bioelectronics. Curr. Opin. Solid State Mater. Sci. 2020, 24, 100806. [Google Scholar] [CrossRef]
- Street, R.M.; Huseynova, T.; Xu, X.; Chandrasekaran, P.; Han, L.; Shih, W.Y.; Shih, W.-H.; Schauer, C.L. Variable piezoelectricity of electrospun chitin. Carbohydr. Polym. 2018, 195, 218–224. [Google Scholar] [CrossRef]
- Yang, Y.; Pan, H.; Xie, G.; Jiang, Y.; Chen, C.; Su, Y.; Wang, Y.; Tai, H. Flexible piezoelectric pressure sensor based on polydopamine-modified BaTiO3/PVDF composite film for human motion monitoring. Sens. Actuator A Phys. 2020, 301, 111789. [Google Scholar] [CrossRef]
- Sinar, D.; Knopf, G.K. Disposable piezoelectric vibration sensors with PDMS/ZnO transducers on printed graphene-cellulose electrodes. Sens. Actuator A Phys. 2020, 302, 111800. [Google Scholar] [CrossRef]
- Ejeian, F.; Azadi, S.; Razmjou, A.; Orooji, Y.; Kottapalli, A.; Warkiani, M.E.; Asadnia, M. Design and applications of MEMS flow sensors: A review. Sens. Actuator A Phys. 2019, 295, 483–502. [Google Scholar] [CrossRef]
- Tiller, B.; Reid, A.; Zhu, B.; Guerreiro, J.; Domingo-Roca, R.; Jackson, J.C.; Windmill, J.F.C. Piezoelectric microphone via a digital light processing 3D printing process. Mater. Des. 2019, 165, 107593. [Google Scholar] [CrossRef]
- Ezzhra-Bouharras, F.; Raihane, M.; Ameduri, B. Recent progress on core-shell structured BaTiO3@polymer/fluorinated polymers nanocomposites for high energy storage: Synthesis, dielectric properties and applications. Prog. Mater. Sci. 2020, 113, 100670. [Google Scholar] [CrossRef]
- Ali, F.; Raza, W.; Li, X.; Gul, H.; Kim, K.-H. Piezoelectric energy harvesters for biomedical applications. Nano Energy 2019, 57, 879–902. [Google Scholar] [CrossRef]
- Tandon, B.; Blaker, J.J.; Cartmell, S.H. Piezoelectric materials as stimulatory biomedical materials and scaffolds for bone repair. Acta Biomater. 2018, 73, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Rajabi, A.H.; Jaffe, M.; Arinzeh, T.L. Piezoelectric materials for tissue regeneration: A review. Acta Biomater. 2015, 24, 12–23. [Google Scholar] [CrossRef] [Green Version]
- Lagerwall, S.T. Ferroelectric and antiferroelectric liquid crystals. In Encyclopedia of Materials: Science and Technology, 2nd ed.; Buschow, J., Cahn, R.W., Flemings, M.C., Ilschner, B., Kramer, E.J., Mahajan, S., Veyssiere, P., Eds.; Pergamon Press: Oxford, UK, 2001; pp. 3044–3063. ISBN 978-0-08-043152-9. [Google Scholar] [CrossRef]
- Damjanovic, D. Ferroelectric dielectric and piezoelectric properties of ferroelectric thin films in ceramics. Rep. Prog. Phys. 1998, 61, 1267–1324. [Google Scholar] [CrossRef] [Green Version]
- Kao, K.C. 2—Electric polarization and relaxation. In Dielectric Phenomena in Solids, with Emphasis on Physical Concepts of Electronic Processes; Kao, K.C., Ed.; Academic Press: San Diego, CA, USA, 2004; pp. 41–114. ISBN 0-12-396561-9780123965615. [Google Scholar] [CrossRef]
- Nisticò, R.; Cesano, F.; Garello, F. Magnetic materials and systems: Domain structure visualization and other characterization techniques for the application in the materials science and biomedicine. Inorganics 2020, 8, 6. [Google Scholar] [CrossRef] [Green Version]
- Nisticò, R. Magnetic materials and water treatments for a sustainable future. Res. Chem. Intermed. 2017, 43, 6911–6949. [Google Scholar] [CrossRef]
- Stojanovic, B.D. Introduction to ferroics and multiferroics: Essential background. In Magnetic, Ferroelectric, and Multiferroic Metal Oxides; Stojanovic, B.D., Ed.; Elsevier: Amsterdam, The Netherlands, 2018; pp. xxxi–xlii. ISBN 978-0-12-811180-2. [Google Scholar] [CrossRef]
- Maiwa, H. 12—Thermal energy harvesting of PLZT and BaTiO3 ceramics using pyroelectric effects. In Nanoscale Ferroelectric-Multiferroic Materials for Energy Harvesting Applications; Kimura, H., Cheng, Z., Jia, T., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; pp. 217–229. ISBN 978-0-12-814499-2. [Google Scholar] [CrossRef]
- Li, J.; Li, J.; Cheng, X.; Feng, G. Investigation of induced charge mechanisms on a rod electrode. Electronics 2019, 8, 977. [Google Scholar] [CrossRef] [Green Version]
- Zhao, S.; Castle, G.S.P.; Adamial, K. Comparison of conduction and induction charging in liquid spraying. J. Electrostat. 2005, 63, 871–876. [Google Scholar] [CrossRef]
- Salome, P.; Rochier, C. Electrostatic discharges (ESD), latch-up and pad design constraints. Microelectron. Eng. 1999, 49, 83–94. [Google Scholar] [CrossRef]
- Ye, H.; Wang, Q.; Sun, Q.; Xu, L. High energy density and interfacial polarization on poly(vinylidene fluoride-chlorotrifluoroethylene) nanocomposite incorporated with halloysite nanotube architecture. Colloids Surf. A Physicochem. Eng. Asp. 2020, 606, 125495. [Google Scholar] [CrossRef]
- Heinert, C.; Sankaran, R.M.; Lacks, D.J. Electrostatic charge generation on material surface from the evaporation of liquids. J. Electrostat. 2020, 105, 103450. [Google Scholar] [CrossRef]
- Kok, J.F.; Renno, N.O. Enhancement of the emission of mineral dust aerosols by electric forces. Geophys. Res. Lett. 2006, 33, L19S10. [Google Scholar] [CrossRef] [Green Version]
- Yadav, R.; Tirumali, M.; Wang, X.; Naebe, M.; Kandasubramanian, B. Polymer composite for antistatic application in aerospace. Def. Technol. 2020, 16, 107–118. [Google Scholar] [CrossRef]
- Sugo ESD Plastics. ESD Materials Categories. Available online: http://en.sugoplas.com/news/industry/95.html (accessed on 27 February 2021).
- Markarian, J. New developments in antistatic and conductive additives. Plast. Addit. Compd. 2008, 10, 22–25. [Google Scholar] [CrossRef]
- Zhang, R.; Agar, J.C.; Wong, C.P. Conductive polymer composites. In Encyclopedia of Polymer Science and Technology; John Wiley and Sons: Hoboken, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Amoabeng, D.; Velankar, S.S. A review of conductive polymer composites filled with low melting point metal alloys. Polym. Eng. Sci. 2018, 58, 1010–1019. [Google Scholar] [CrossRef] [Green Version]
- Meenach, S.A.; Burdick, J.; Kunwar, A.; Wang, J. Metal/conducting-polymer composite nanowires. Small 2007, 3, 239–243. [Google Scholar] [CrossRef]
- Wang, M.; Tang, X.-H.; Cai, J.-H.; Wu, H.; Shen, J.-B.; Guo, S.-Y. Construction, mechanism and prospective of conductive polymer composites with multiple interfaces for electromagnetic interface shielding: A review. Carbon 2021, 177, 377–402. [Google Scholar] [CrossRef]
- Kondrashov, S.V.; Soldatov, M.A.; Gunyaeva, A.G.; Shashkeev, K.A.; Komarova, O.K.; Barinov, D.Y.; Yurkov, G.Y.; Shevchenko, V.G.; Muzafarov, A.M. The use of noncovalently modified carbon nanotubes for preparation of hybrid polymeric composite materials with electrically conductive and lightning resistant properties. J. Appl. Polym. Sci. 2018, 135, 46108. [Google Scholar] [CrossRef]
- Clingerman, M.L.; Weber, E.H.; King, J.A.; Schulz, K.H. Synergistic effect of carbon filler in electrically conductive nylon 6,6 and polycarbonate based resins. Polym. Compos. 2002, 23, 911–924. [Google Scholar] [CrossRef]
- Wang, P.; Ding, T. Conductivity and piezoresistivity of conductive carbon black filled polymer composite. J. Appl. Polym. Sci. 2010, 116, 2035–2039. [Google Scholar] [CrossRef]
- Ning, W.; Xingxiang, Z.; Jiugao, Y.; Jianming, F. Partially miscible poly(lactic acid)-blend-poly(propylene carbonate) filled with carbon black as conductive polymer composite. Polym. Int. 2008, 57, 1027–1035. [Google Scholar] [CrossRef]
- Cataldi, P.; Bayer, I.S.; Bonaccorso, F.; Pellegrini, V.; Athanassiou, A.; Cingolani, R. Foldable conductive cellulose fiber networks modified by graphene nanoplatelet bio-based composites. Adv. Electron. Mater. 2015, 1, 1500224. [Google Scholar] [CrossRef]
- Yang, S.; Chalivendra, V.; Benjamin, E.; Kim, Y. Electrical response of novel carbon nanotubes embedded and carbon fiber Z-axis reinforced jute/epoxy laminated composites. Polym. Compos. 2019, 40, E1189–E1198. [Google Scholar] [CrossRef]
- Syurik, Y.V.; Ghislandi, M.G.; Tkalya, E.E.; Paterson, G.; McGrouther, D.; Ageev, O.A.; Loos, J. Graphene network organization in conductive polymer composites. Macromol. Chem. Phys. 2012, 213, 1251–1258. [Google Scholar] [CrossRef]
- Dos Santos, A.M.; Merlini, C.; Ramoa, S.D.A.S.; Barra, G.M.O. Comparative study of electrically conductive polymer composites of polyester-based thermoplastic polyurethane matrix with polypyrrole and montmorillonite/polypyrrole additive. Polym. Compos. 2020, 41, 2003–2012. [Google Scholar] [CrossRef]
- Hansen, T.S.; West, K.; Hassanger, O.; Larsen, N.B. Highly stretchable and conductive polymer material made from poly(3,4-ethylenedioxythiophene) and polyurethane elastomers. Adv. Funct. Mater. 2007, 17, 3069–3073. [Google Scholar] [CrossRef]
- Shankar, U.; Bhandari, S.; Khastgir, D. Carbon black-filled nitrile rubber composite as a flexible electrode for electrochemical synthesis of supercapacitive polyaniline. Polym. Compos. 2019, 40, E1537–E1547. [Google Scholar] [CrossRef]
- Omastova, M.; Pavlinec, J.; Pionteck, J.; Simon, F. Synthesis, electrical properties and stability of polypyrrole-containing conducting polymer composites. Polym. Int. 1997, 43, 109–116. [Google Scholar] [CrossRef]
- Kosinski, S.; Rykowska, I.; Gonsior, M.; Krzyzanowski, P. Ionic liquids as antistatic additives for polymer composites—A review. Polym. Test. 2022, 112, 107649. [Google Scholar] [CrossRef]
- Plastucranger.com. Antistatic vs. Static Dissipative Plastics|Which One to Choose? Available online: https://plasticranger.com/antistatic-vs-dissipative-plastics/ (accessed on 21 June 2022).
- Reeves, G.K.; Leech, P.W.; Harrison, H.B. Understanding the sheet resistance parameter of alloyed ohmic contacts using a transmission line model. Solid State Electron. 1995, 38, 745–751. [Google Scholar] [CrossRef]
- Ausserlechner, U. Van-der-Pauw measurement on devices with four contacts and two orthogonal mirror symmetries. Solid State Electron. 2017, 133, 53–63. [Google Scholar] [CrossRef]
- Pveducation.org. Four Point Probe Resistivity Measurements. Available online: https://www.pveducation.org/pvcdrom/characterisation/four-point-probe-resistivity-measurements (accessed on 29 June 2022).
- Ossila.com. Sheet Resistance: A Guide to Theory. Available online: https://www.ossila.com/pages/sheet-resistance-theory (accessed on 29 June 2022).
- Singh, Y. Electrical Resistivity Measurements: A Review. Int. J. Mod. Phys. C 2013, 22, 745–756. [Google Scholar] [CrossRef]
- Embeddedcomputing.com. Fundamentals of HBM, MM, and CDM Tests by Bonnie Baker. Available online: https://embeddedcomputing.com/technology/analog-and-power/fundamentals-of-hbm-mm-and-cdm-tests#:~:text=The%20human-body%20model%20%28HBM%29%20characterizes%20an%20electronic%20device%E2%80%99s,ESD%29%20charges%20the%20capacitor%20in%20the%20test%20circuit (accessed on 27 June 2022).
- Electronicdesign.com. What’s the Difference between HBM, CDM, and MM Test? Available online: https://www.electronicdesign.com/power-management/article/21799383/whats-the-difference-between-hbm-cdm-and-mm-test (accessed on 27 June 2022).
- Brodbeck, T.; Gaertner, R. Experience in HBM ESD testing of high pin count devices. Microelectron. Reliab. 2007, 47, 1025–1029. [Google Scholar] [CrossRef]
- Meeder, M.G.; Marchut, L.; Antonell, M.J.; Fresina, M.T.; Novak, C.E.; Darche, T.C. Application of machine model ESD tester to high volume capacitor reliability testing. Microelectron. Reliab. 2011, 51, 246–251. [Google Scholar] [CrossRef]
- Greason, W.D. Analysis of charged device model (CDM) ESD in MEMS. J. Electrostat. 2010, 68, 159–167. [Google Scholar] [CrossRef]
- Tamminen, P.; Viheriakoski, T.; Sydanheimo, L.; Ukkonen, L. ESD qualification data used as the basis for building electrostatic discharge protected areas. J. Electrostat. 2015, 77, 174–181. [Google Scholar] [CrossRef]
- Sankaran, S.; Deshmukh, K.; Ahamed, M.B.; Pasha, S.K.K. Recent advances in electromagnetic interference shielding properties of metal and carbon filler reinforced flexible polymer composites: A review. Compos. A Appl. Sci. Manuf. 2018, 114, 49–71. [Google Scholar] [CrossRef]
- Al-Saleh, M.H.; Gelves, G.A.; Sundararaj, U. Copper nanowire/polystyrene nanocomposites: Lower percolation threshold and higher EMI shielding. Compos. A Appl. Sci. Manuf. 2011, 42, 92–97. [Google Scholar] [CrossRef]
- Folorunso, O.; Hamam, Y.; Sadiku, R.; Ray, S.S.; Kumar, N. Investigation and modeling of the electrical conductivity of graphene nanoplatelets-loaded doped pyrrole. Polymers 2021, 13, 1034. [Google Scholar] [CrossRef]
- Li, C.; Thostenson, E.T.; Chou, T.-W. Dominat role of tunnelling resistance in the electrical conductivity of carbon nanotube-based composites. Appl. Phys. Lett. 2007, 91, 223114. [Google Scholar] [CrossRef]
- Lou, C.-W.; Huang, C.-L.; Pan, Y.-J.; Lin, Z.-I.; Song, X.-M.; Lin, J.-H. Crystallization, mechanical, and electromagnetic properties of conductive polypropylene/SEBS composites. J. Polym. Res. 2016, 23, 84. [Google Scholar] [CrossRef]
- Adeniyi, A.G.; Ighalo, J.O. A systematic review of pure metals reinforced plastic composites. Iran. Polym. J. 2021, 30, 751–768. [Google Scholar] [CrossRef]
- Mamunya, Y.P.; Davydenko, V.V.; Pissis, P.; Lebedev, E.V. Electrical and thermal conductivity of polymers filled with metal powders. Eur. Polym. J. 2002, 38, 1887–1897. [Google Scholar] [CrossRef]
- Nassiet, V.; Hassoune-Rhabbour, B.; Tramis, O.; Petit, J.-A. 22—Electrical and Electronics. In Adhesive Bonding, Woodhead Publishing Series in Welding and Other Joining Technologies, 2nd ed.; Adams, R.D., Ed.; Woodhead Publishing: Duxford, UK, 2021; pp. 719–761. ISBN 9780128199541. [Google Scholar] [CrossRef]
- Fernandes, M.; Padrao, J.; Ribeiro, A.I.; Fernandes, R.D.V.; Melro, L.; Nicolau, T.; Mehravani, B.; Alves, C.; Rodrigues, R.; Zille, A. Polysaccharides and metal nanoparticles for functional textiles. A review. Nanomaterials 2022, 12, 1006. [Google Scholar] [CrossRef]
- Zhu, A.; Wang, H.; Sun, S.; Zhang, C. The synthesis and antistatic, anticorrosive properties of polyaniline composite coating. Prog. Org. Coat. 2018, 122, 270–279. [Google Scholar] [CrossRef]
- Thangamani, J.G.; Deshmukh, K.; Sadasivuni, K.K.; Ponnamma, D.; Goutham, S.; Rao, K.V.; Chidambaram, K.; Ahamed, M.B.; Grace, A.N.; Faisal, M.; et al. White graphene reinforced polypyrrole and poly(vinyl alcohol) blend nanocomposites as chemiresistive sensors for room temperature detection of liquid petroleum gases. Microchim. Acta 2017, 184, 3977–3987. [Google Scholar] [CrossRef]
- Aradhana, R.; Mohanty, S.; Nayak, S.K. A review on epoxy-based electrically conductive adhesives. Int. J. Adhes. Adhes. 2020, 99, 102596. [Google Scholar] [CrossRef]
- Chen, C.; Wang, L.; Yu, H.; Jiang, G.; Yang, Q.; Zhou, J.; Xiang, W.; Zhang, J. Study on the growth mechanism of silver nanorods in the nanowire-seeding polyol process. Mater. Chem. Phys. 2008, 107, 13–17. [Google Scholar] [CrossRef]
- Nisticò, R.; Rivolo, P.; Giorgis, F. Tips and tricks for the surface engineering of well-ordered morphologically driven silver-based nanomaterials. ChemistryOpen 2019, 8, 508–519. [Google Scholar] [CrossRef] [PubMed]
- Nisticò, R.; Barrasso, M.; Carrillo Le Roux, G.A.; Seckler, M.M.; Sousa, W.; Malandrino, M.; Magnacca, G. Biopolymers from composted biowaste as stabilizers for the synthesis of spherical and homogeneously sized silver nanoparticles for textile applications on natural fibers. ChemPhysChem 2015, 16, 3902–3909. [Google Scholar] [CrossRef] [PubMed]
- Nisticò, R.; Rivolo, P.; Novara, C.; Giorgis, F. New branched flower-like Ag nanostructures for SERS analysis. Colloids Surf. A Physicochem. Eng. Asp. 2019, 578, 123600. [Google Scholar] [CrossRef]
- Le Ouay, B.; Stellacci, F. Antibacterial activity of silver nanoparticles: A surface science insight. Nano Today 2015, 10, 339–354. [Google Scholar] [CrossRef]
- Nisticò, R.; Rosellini, A.; Rivolo, P.; Faga, M.G.; Lamberti, R.; Martorana, S.; Castellino, M.; Virga, A.; Mandracci, P.; Malandrino, M.; et al. Surface functionalisation of polypropylene hernia-repair meshes by RF-activated plasma polymerisation of acrylic acid and silver nanoparticles. Appl. Surf. Sci. 2015, 328, 287–295. [Google Scholar] [CrossRef]
- Abdulkareem, S.A.; Adeniyi, A.G. Recycling copper and polystyrene from solid waste stream in developing conductive composites. J. Solid Waste Technol. Manag. 2019, 45, 39–44. [Google Scholar] [CrossRef]
- Osman, A.F.; Mariatti, M. Properties of aluminum filled polypropylene composites. Polym. Polym. Compos. 2006, 14, 623–633. [Google Scholar] [CrossRef]
- Lu, S.; Gao, X.; Hu, C.-Y.; Xia, Y. Heavy metal release from irradiated LDPE/nanometal composite films into food stimulants. Food Packag. Shelf Life 2020, 26, 100571. [Google Scholar] [CrossRef]
- Spitalsky, Z.; Tasis, D.; Papagelis, K.; Galiotis, C. Carbon nanotube-polymer composites: Chemistry, processing, mechanical and electrical properties. Prog. Polym. Sci. 2010, 35, 357–401. [Google Scholar] [CrossRef]
- Jariwala, D.; Sangwan, V.K.; Lauhon, L.J.; Marks, T.J.; Hersam, M.C. Carbon nanomaterials for electronics, optoelectronics, photovoltaics, and sensing. Chem. Soc. Rev. 2013, 42, 2824–2860. [Google Scholar] [CrossRef] [Green Version]
- Lavagna, L.; Nisticò, R.; Musso, S.; Pavese, M. Functionalization as a way to enhance dispersion of carbon nanotubes in matrices: A review. Mater. Today Chem. 2021, 20, 100477. [Google Scholar] [CrossRef]
- Lavagna, L.; Marchisio, S.; Merlo, A.; Nisticò, R.; Pavese, M. Polyvinyl butyral-based composites with carbon nanotubes: Efficient dispersion as a key to high mechanical properties. Polym. Compos. 2020, 41, 3627–3637. [Google Scholar] [CrossRef]
- Ardanuy, M.; Rodriguez-Perez, M.A.; Algaba, I. Electrical conductivity and mechanical properties of vapor-grown carbon nanofibers/trifunctional epoxy composites prepared by direct mixing. Compos. B Eng. 2011, 42, 675–681. [Google Scholar] [CrossRef]
- Amoli, B.M.; Trinidad, J.; Rivers, G.; Sy, S.; Russo, P.; Yu, A.; Zhou, N.Y.; Zhao, B. SDS-stabilized graphene nanosheets for highly electrically conductive adhesives. Carbon 2015, 91, 188–199. [Google Scholar] [CrossRef]
- Khanam, P.N.; Ponnamma, D.; Al-Madeed, M.A. Electrical proeprties of graphene polymer nanocomposites. In Graphene-Based Polymer Nanocomposites in Electronics; Springer Series on Polymer and Composite Materials; Sadasivuni, K.K., Ponnamma, D., Kim, J., Thomas, S., Eds.; Springer International Publishing AG Switzerland: Cham, Switzerland, 2015; pp. 25–47. ISBN 978-3-3191-3874-9. [Google Scholar] [CrossRef]
- Boehm, H.P. Some aspects of the surface chemistry of carbon blacks and other carbons. Carbon 1994, 32, 759–769. [Google Scholar] [CrossRef]
- Fan, Y.; Fowler, G.D.; Zhao, M. The past, present and future of carbon black as a rubber reinforcing filler—A review. J. Clean. Prod. 2020, 247, 119115. [Google Scholar] [CrossRef]
- Sanchez-Gonzalez, J.; Marcias-Garcia, A.; Alexandre-Franco, M.F.; Gomez-Serrano, V. Electrical conductivity of carbon blacks under compression. Carbon 2005, 43, 741–747. [Google Scholar] [CrossRef]
- Kumar, S.; Saeed, G.; Zhu, L.; Hui, K.N.; Kim, N.H.; Lee, J.H. 0D to 3D carbon-based networks combined with pseudocapacitative electrode material for high energy density supercapacitor: A review. Chem. Eng. J. 2021, 403, 126352. [Google Scholar] [CrossRef]
- Mora, A.; Verma, P.; Kumar, S. Electrical conductivity of CNT/polymer composites: 3D printing, measurements and modeling. Compos. B Eng. 2020, 183, 107600. [Google Scholar] [CrossRef]
- Poyato, R.; Osuna, J.; Morales-Rodriguez, A.; Gallardo-Lopez, A. Electrical conduction mechanisms in graphene nanoplatelet/yttria tetragonal zirconia composites. Ceram. Int. 2018, 44, 14610–14616. [Google Scholar] [CrossRef]
- McNaught, A.D.; Wilkinson, A.; IUPAC. Compendium of Chemical Terminology, 2nd ed.; (the “Gold Book”); Blackwell Scientific Publications: Oxford, UK, 1997; ISBN 0-9678550-9-8. [Google Scholar] [CrossRef]
- Cui, H.-W.; Li, D.-S.; Fang, Q.; Lai, H.-X. Electrical and mechanical properties of electrically conductive adhesives from epoxy, micro-silver flakes, and nano-hexagonal boron nitride particles after humid and thermal aging. Int. J. Adhes. Adhes. 2013, 44, 232–236. [Google Scholar] [CrossRef]
- Zhao, J.-C.; Hu, J.-D.; Jiao, D.-N.; Tosto, S. Application of face centred cubic TiB powder as conductive filler for electrically conductive adhesives. Trans. Nonferrous Met. Soc. China 2014, 24, 1773–1778. [Google Scholar] [CrossRef]
- Jeong, D.-Y.; Ryu, J.; Lim, Y.-S.; Dong, S.; Park, D.-S. Piezoresistive TiB2/silicone rubber composites for circuit breakers. Sens. Actuator A Phys. 2009, 149, 246–250. [Google Scholar] [CrossRef]
- Paszkiewicz, S.; Taraghi, I.; Szymczyk, A.; Huczko, A.; Kurcz, M.; Przybyszewski, B.; Stanik, R.; Linares, A.; Ezquerra, T.A.; Roslaniec, Z. Electrically and thermally conductive thin elastic polymer foils containing SiC nanofibers. Compos. Sci. Technol. 2017, 146, 20–25. [Google Scholar] [CrossRef]
- Bouzidi, A.; Omri, K.; El Mir, L.; Guermazi, H. Preparation, structural and optical investigations of ITO nanopowder and ITO/epoxy nanocomposites. Mater. Sci. Semicond. Process. 2015, 39, 536–543. [Google Scholar] [CrossRef]
- Esdsafematerials.com. Available online: https://www.esdsafematerials.com/ (accessed on 3 November 2022).
- Uvex-safety.com. Electrostatic Discharge Capability of Clothing. Available online: https://www.uvex-safety.com/blog/electrostatic-discharge-capability-of-clothing-part-two-of-two/ (accessed on 3 November 2022).
- Pcbatools.com. ESD Mat—Antistatic Mat. Available online: https://www.pcbatools.com/esd-mat-antistatic-mat.html (accessed on 3 November 2022).
- Wellplast.com. ESD Protection—For Products Sensitive to Static Discharge. Available online: https://www.wellplast.com/esd-materials/ (accessed on 3 November 2022).
- Ppcflex.com. Pink Poly Bags vs. Shield Bags. Available online: https://ppcflex.com/project/pink-poly-bags-vs-shield-bags/ (accessed on 4 November 2022).
- Formlabs.com. New ESD Safe Material Enables Manufactures to Scale with 3D Printing. Available online: https://formlabs.com/eu/blog/introducing-esd-resin/ (accessed on 4 November 2022).
- Yang, X.; Yu, Z.; Fang, W.; Wan, Z.; Qian, Q.; Li, W.; Jiao, H.; Li, J.; Chang, J.; Li, Q. Improving antistatic and mechanical properties of glass fiber reinforced polypropylene composites through polar adsorption and anchoring effect of organic salt. Compos. Sci. Technol. 2022, 220, 109285. [Google Scholar] [CrossRef]
- Zhiani, M.; Zare, A. Investigation of electrical resistivity and electrostatic discharge sensitivity of Mg/Ba(NO3)2/novolac resin composite. J. Electrostat. 2022, 120, 103755. [Google Scholar] [CrossRef]
- Novak, D.; Plavan, B.V.; Kucherenko, E. Effect of the colloidal graphite filler on the properties of electroconductive polyethylene compositions. Mater. Today Proc. 2022, 50, 514–517. [Google Scholar] [CrossRef]
- Musiol, M.; Rydz, J.; Janeczek, H.; Kordyka, A.; Andrzejewski, J.; Sterzynski, T.; Jurczyk, S.; Cristea, M.; Musiol, K.; Kampik, M.; et al. (Bio)degradable biochar composites—Studies on degradation and electrostatic properties. Mater. Sci. Eng. B 2022, 275, 115515. [Google Scholar] [CrossRef]
- Luo, C.; Li, J.; Chen, Z.; Lin, J.; Chen, L.; He, S. Improving the charge dissipating performance and breakdown strength of epoxy resin by incorporating polydopamine-coated barium titanate. Mater. Today Commun. 2022, 31, 103619. [Google Scholar] [CrossRef]
- Yu, Z.; Wang, C.; Zhang, X.; Phule, A.D.; Zhao, Y.; Wen, S.; Zhang, Z.X. Self-healing performance of lightweight and electrically conductive ethylene-vinyl acetate copolymer/carbon nanotubes composite foam. Compos. Commun. 2022, 29, 101051. [Google Scholar] [CrossRef]
- Hassan, M.M. Enhanced colour, hydrophobicity, UV radiation absorption and antistatic properties of wool fabric multifunctionalised with silver nanoparticles. Colloids Surf. A Physicochem. Eng. Asp. 2019, 581, 123819. [Google Scholar] [CrossRef]
- Mousavi, S.R.; Estaji, S.; Kiaei, H.; Mansourian-Tabaei, M.; Nouranian, S.; Jafari, S.H.; Ruckdaschel, H.; Arjmand, M.; Khonakdar, H.A. A review of electrical and thermal conductivities of epoxy resin systems reinforced with carbon nanotubes and graphene-based nanoparticles. Polym. Test. 2022, 112, 107645. [Google Scholar] [CrossRef]
- Ai, J.; Zeng, B.; Qin, J.; Zheng, Y.; Guo, S. Experiment and theory study on the electrical property of MWCNT filled thermoplastic polyurethane bilayer composites with tunable layer thickness ratios. Polym. Test. 2022, 115, 107749. [Google Scholar] [CrossRef]
- DeArmitt, C.; Rothon, R. Surface modifiers for use with particulate fillers. In Fillers for polymer applications. Polymers and polymeric compoistes: A reference series; Rothon, R., Ed.; Springer: Cham, Switzerland, 2017; pp. 29–49. ISBN 978-3-319-28117-9. [Google Scholar] [CrossRef]
- D’Arienzo, M.; Dirè, S.; Cobani, E.; Orsini, S.; Di Credico, B.; Antonini, C.; Callone, E.; Parrino, F.; Dalle Vacche, S.; Trusiano, G.; et al. SiO2/ladder-like polysilsesquioxanes nanocomposites coatings: Playing with the hybrid interface for tuning thermal properties and wettability. Coatings 2020, 10, 913. [Google Scholar] [CrossRef]
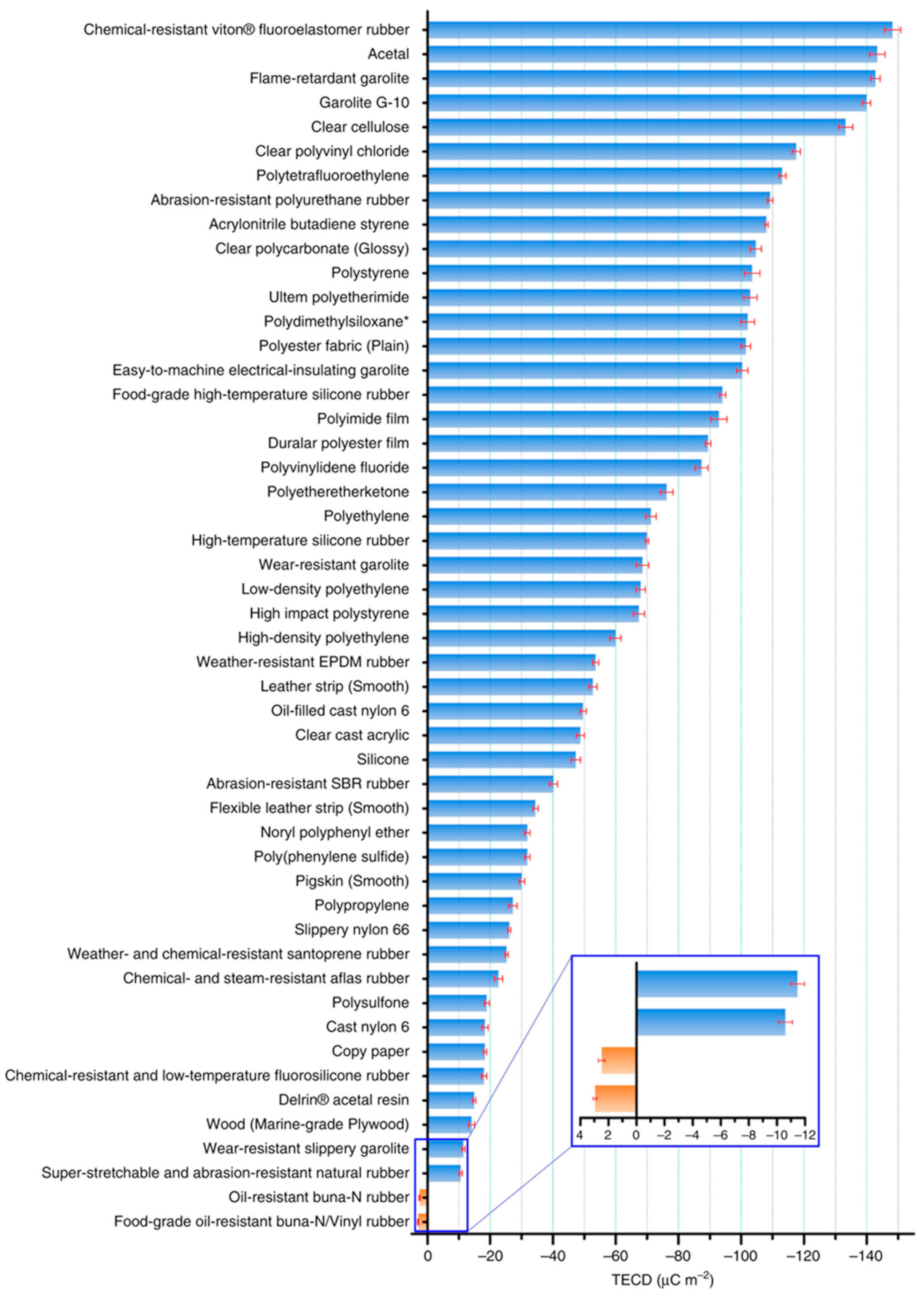


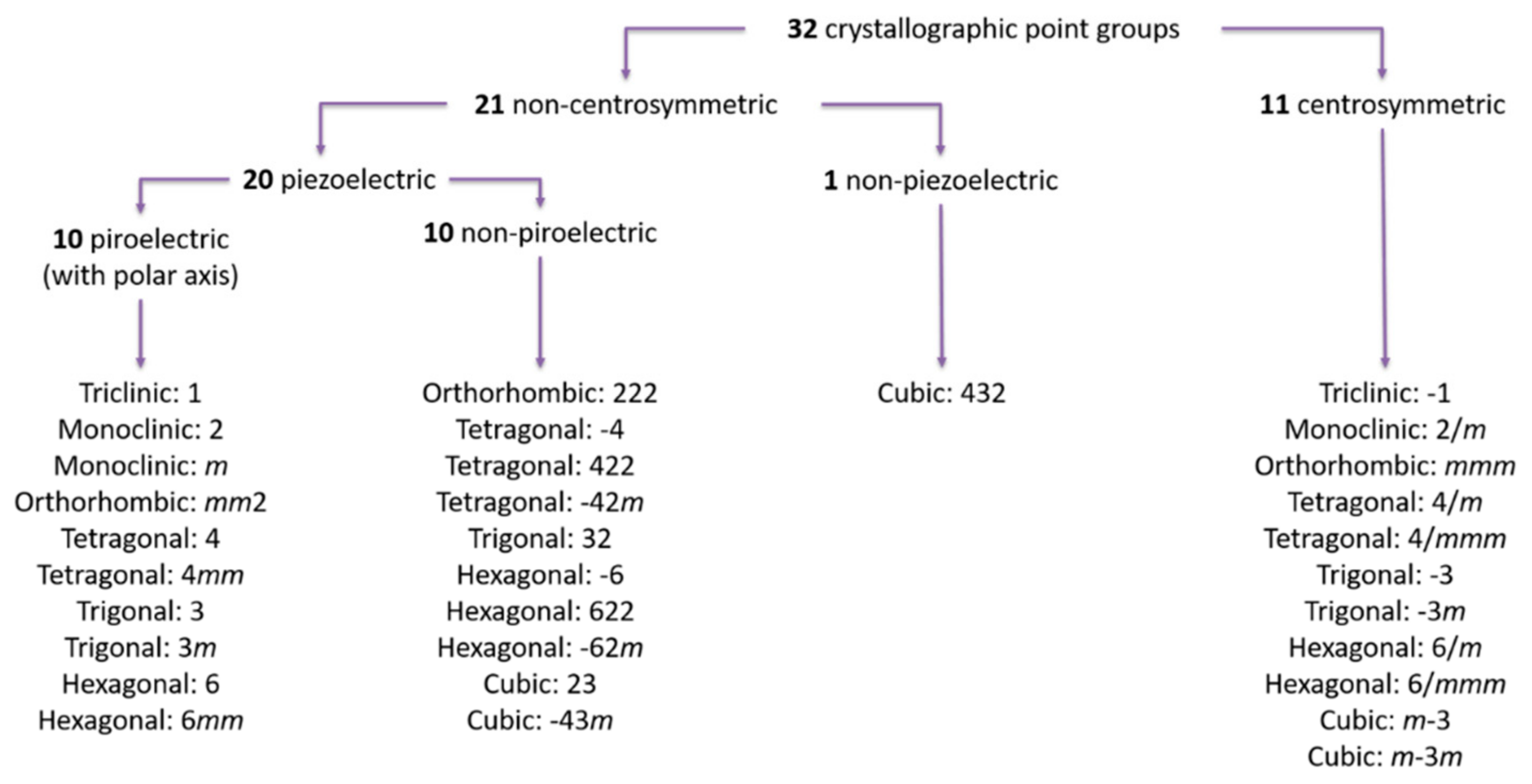
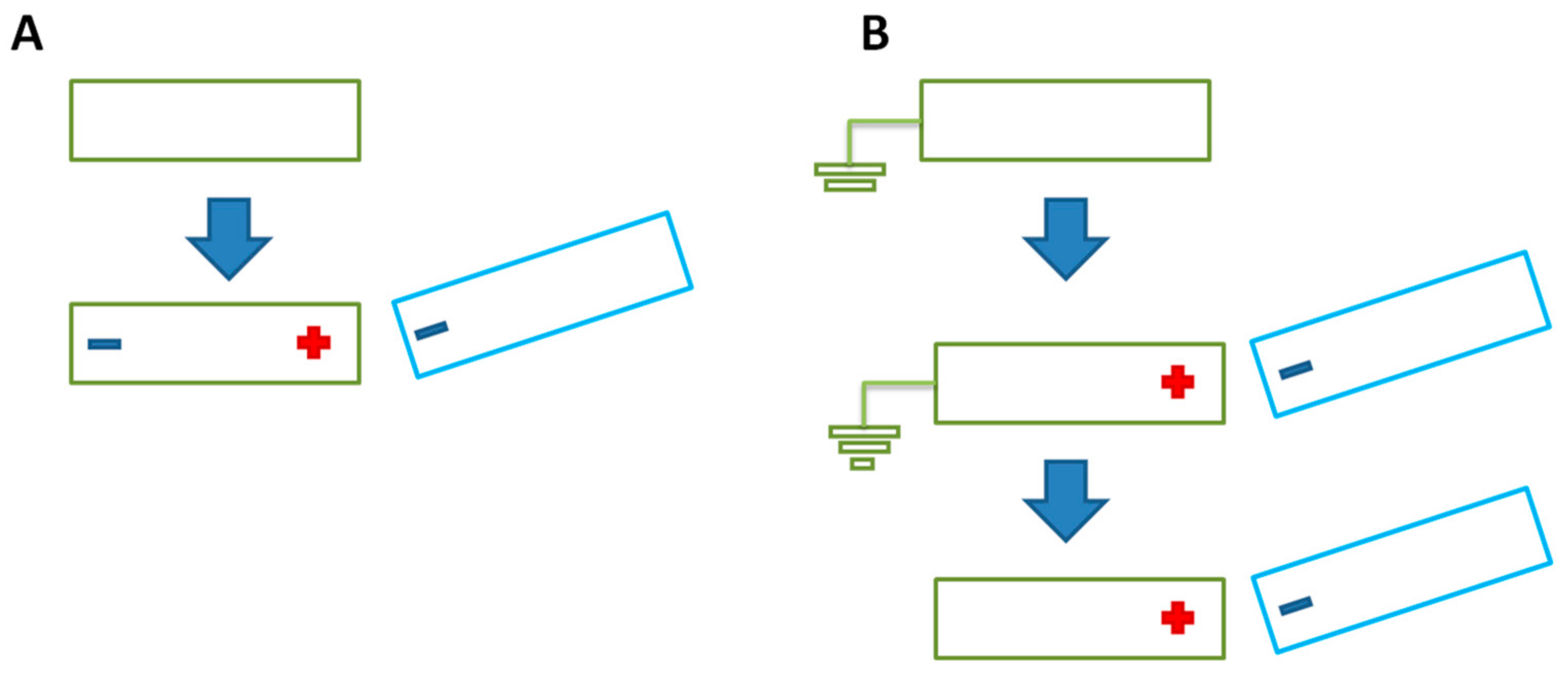

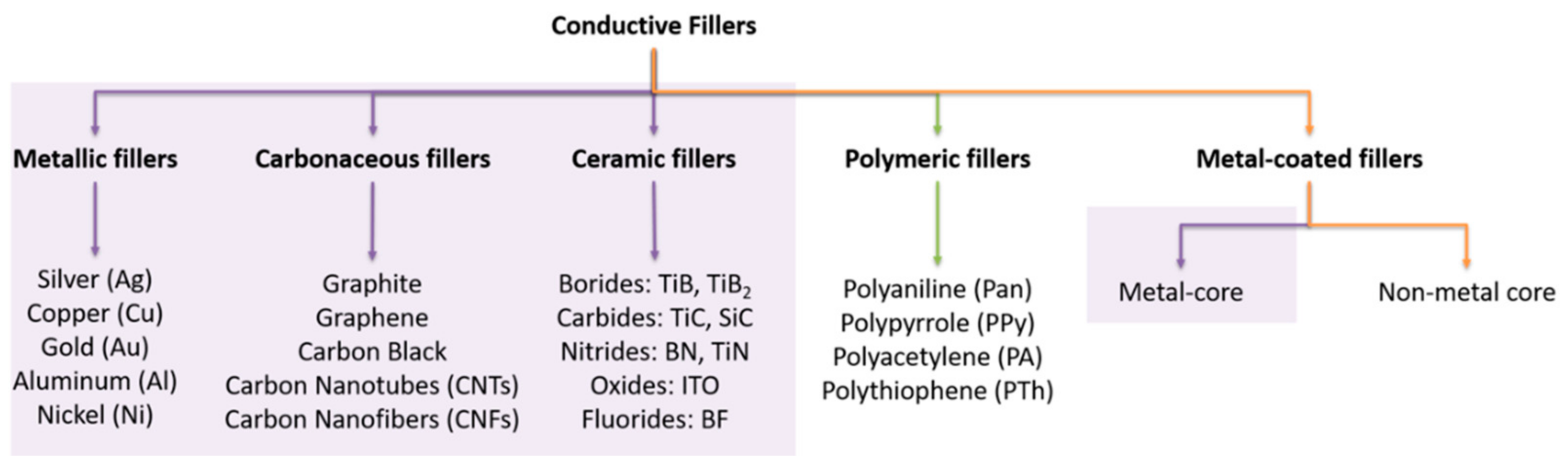
| Materials | Sheet Resistance (Ω/sq) | Bulk Resistance (Ω m) |
|---|---|---|
| Metals | <10−4 | <10−7 |
| Carbons | ca. 10−4–100 | ca. 10−7–10−3 |
| Shielding-conductive composites | ca. 100–105 | ca. 10−3–102 |
| Static-dissipative composites | ca. 105–109 | ca. 102–106 |
| Antistatic composites | ca. 109–1012 | ca. 106–109 |
| Insulant polymers | ca. >1012 | ca. >109 |
| Classification | Voltage Range |
|---|---|
| Class 0 | <250 V (fail for ESD pulse of 250 V) |
| Class 1A | 250 V to <500 V (pass 250 V, fail 500 V) |
| Class 1B | 500 V to <1000 V (pass 500 V, fail 1000 V) |
| Class 1C | 1000 V to <2000 V (pass 1000 V, fail 2000 V) |
| Class 2 | 2000 V to <4000 V (pass 2000 V, fail 4000 V) |
| Class 3A | 4000 V to <8000 V (pass 4000 V fail 8000 V) |
| Class 3B | ≥8000 V (pass 8000 V or above) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nisticò, R.; D’Arienzo, M.; Di Credico, B.; Mostoni, S.; Scotti, R. The Role of Inorganic Fillers in Electrostatic Discharge Composites. Inorganics 2022, 10, 222. https://doi.org/10.3390/inorganics10120222
Nisticò R, D’Arienzo M, Di Credico B, Mostoni S, Scotti R. The Role of Inorganic Fillers in Electrostatic Discharge Composites. Inorganics. 2022; 10(12):222. https://doi.org/10.3390/inorganics10120222
Chicago/Turabian StyleNisticò, Roberto, Massimiliano D’Arienzo, Barbara Di Credico, Silvia Mostoni, and Roberto Scotti. 2022. "The Role of Inorganic Fillers in Electrostatic Discharge Composites" Inorganics 10, no. 12: 222. https://doi.org/10.3390/inorganics10120222
APA StyleNisticò, R., D’Arienzo, M., Di Credico, B., Mostoni, S., & Scotti, R. (2022). The Role of Inorganic Fillers in Electrostatic Discharge Composites. Inorganics, 10(12), 222. https://doi.org/10.3390/inorganics10120222














