Next Article in Journal
Pincer Complexes Derived from Tridentate Schiff Bases for Their Use as Antimicrobial Metallopharmaceuticals
Previous Article in Journal
The Crystal Structure of Carbonic Acid
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Inorganics
Volume 10
Issue 9
10.3390/inorganics10090133
inorganics-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Crystallographic and Theoretical Study of Osme Bonds in Nitrido-Osmium(VI) Complexes

by
Rosa M. Gomila
and
Antonio Frontera
*
Department of Chemistry, Universitat de les Illes Balears, Crta de Valldemossa km 7.5, 07122 Palma de Mallorca, Spain
*
Author to whom correspondence should be addressed.
Inorganics 2022, 10(9), 133; https://doi.org/10.3390/inorganics10090133
Submission received: 17 August 2022 / Revised: 31 August 2022 / Accepted: 2 September 2022 / Published: 5 September 2022
(This article belongs to the Section Inorganic Solid-State Chemistry)
Browse Figures
Review Reports Versions Notes

Abstract

:
Osme bonds have been recently defined as the attractive interaction between an element of group 8 acting as an electrophile and any atom or group of atoms acting as a nucleophile. To date, the known examples of osme bonds in X-ray structures involve mostly the highly reactive OsO4 and amines and amine oxides. In this work, evidence supporting the existence of osme bonds in osmium(VI) derivatives is reported. In particular, nitrido-osmium(VI) complexes that present square-pyramidal geometries are well disposed to participate in osme bonds opposite to the Os≡N bond. By using a combination of experimental and theoretical results, the existence and importance of this new class of σ-hole interactions is demonstrated in the solid state of several nitrido-osmium(VI) derivatives.

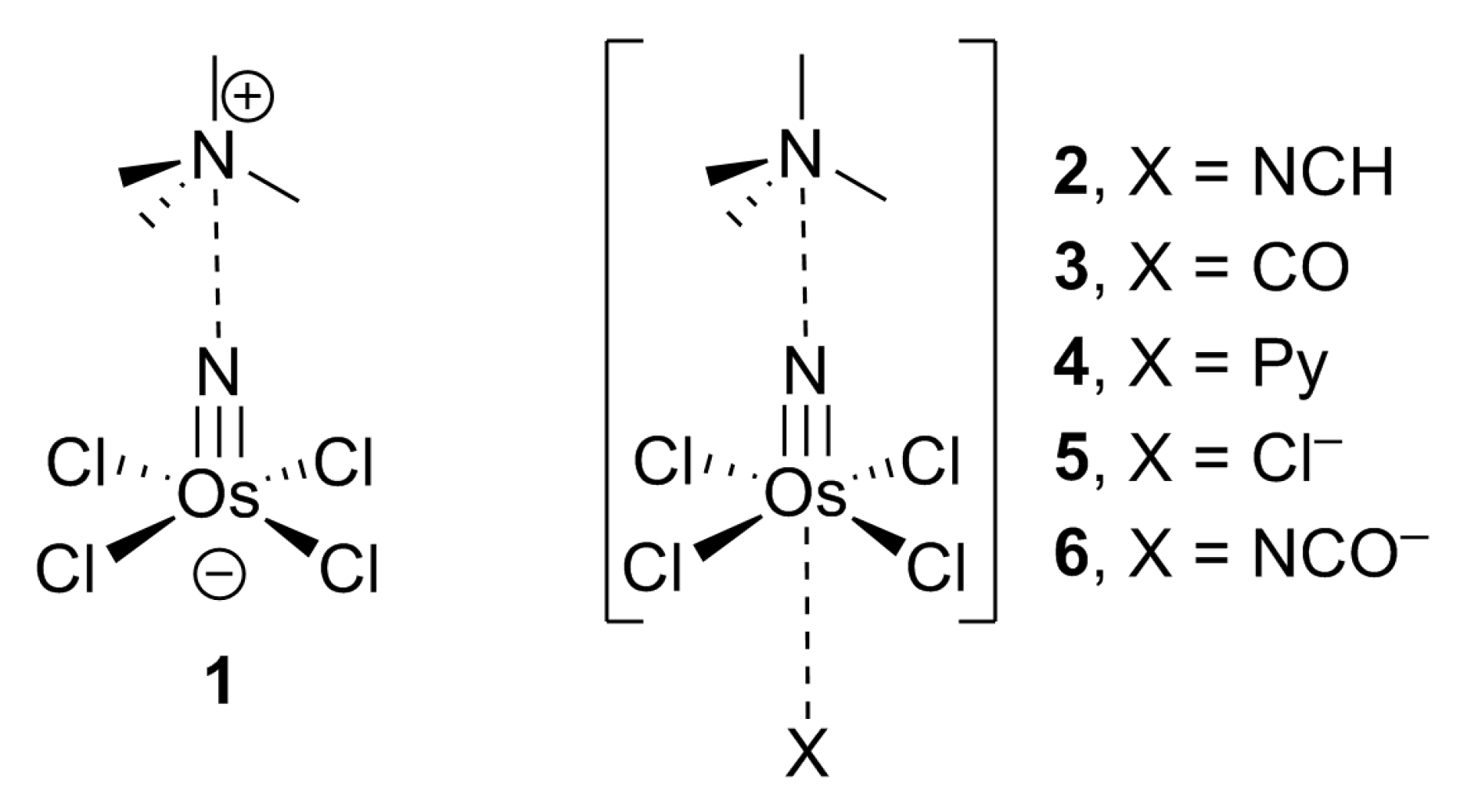
1. Introduction

Noncovalent interactions involving p-block elements from groups 18 to 13 as electron acceptors, namely aerogen, halogen, chalcogen, pnictogen, tetrel and triel bonds, respectively, are, nowadays, commonly used by synthetic chemists (for catalytic purposes), supramolecular chemists and crystal engineers [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21]. These interactions have been rationalized using the σ-hole and π-hole concepts proposed by Politzer and coworkers [22].
More recently, theoretical and crystallographic scientists are expanding the σ-hole concept to the d-block of elements (groups 3–10) and post-transition metals (groups 11 and 12). In fact, several noncovalent interactions were defined by combining theoretical and experimental evidence, which are regium (or coinage) [23,24,25] and spodium bonding [26] interactions (SpB) for post-transition elements of groups 11 and 12, respectively. In these interactions, the metal centers act as Lewis acids, and these names are used to differentiate noncovalent interactions from coordination bonds, which have a more covalent character. Similarly, the terms “wolfium bond” [27] and “matere bond” [28] were used to define the net attractive interaction between elements from groups 6 and 7, respectively, acting as Lewis acids and any electron donor.
Experimental and theoretical evidence of σ-hole interactions in adducts between electron-rich atoms (pyridines and pyridine N-oxide derivatives) and osmium tetraoxide has been recently reported [29]. The term “osme bond” (OmB) has been proposed and used [30] for defining the noncovalent interaction wherein any element belonging to group 8 has the role of the electrophile. To date, the experimental evidence of osme bonds is limited to osmium(VIII) derivatives. Herein, we report experimental and theoretical evidence of the formation of osme bonds in nitrido-osmium(VI) complexes (see Scheme 1), which is unprecedented in the literature. These high-valence nitrido-osmium(VI) complexes have been known for a long time [31], and they exhibit interesting photochemical [32,33,34] and electrochemical [35,36] properties. However, they have not been used to date to analyze their ability to establish osme bonds opposite to the Os≡N triple bond, as far as our knowledge extends. A search in the Cambridge Structural Database (CSD) [37] has been performed, showing a few X-ray structures exhibiting OmBs that are important for determining their crystal packing. Moreover, theoretical density functional theory (DFT) calculations further support the formation of OmB in nitrido-osmium(VI) derivatives and the noncovalent nature of the interaction, which was analyzed using several computational tools, as explained in the following sections.

2. Results and Discussion

2.1. CSD Search

We have explored the entire CSD and found 25 X-ray structures containing the nitrido-Os(VI) fragment, which are listed in Table 1. Only four structures form directional Os···X osme bonds, as represented in Figure 1 and indicated in italics in Table 1. This is likely due to the fact that, in many structures, the [OsNL4] moiety is negatively charged and, consequently, the interaction of this unit with electron-rich species is not favored.
The first selected example (refcode FEJZUP) corresponds to a neutral complex where the organic ligand is trianionic, 1-(4-tert-Butylpyridine-2-carboxamido)-4,5-dichloro-2-(2-hydroxybenzamido)benzene (H3L), thus, forming a complex with the formula OsNL. In solid state, it forms self-assembled dimers, where the phenoxi O atom of one monomer is located opposite to the N≡Os bond of the other monomer, and vice versa, thus, forming two symmetrically equivalent OmBs (Os···O distance: 2.878 Å). This distance is longer than the sum of the covalent radii ΣRcov(Os+O) = 2.00 Å, thus, suggesting a strong noncovalent character. A remarkable example is shown in Figure 2b, where a pyrazine molecule cocrystallizes with two molecules of tetrachloro-nitrido-osmium(VI) forming 1:2 adducts. The Os⋅⋅⋅N interactions involving the pyrazine nitrogen atoms are longer (2.518 Å) than the ΣRcov(Os+N) = 2.15 Å but remarkably shorter than the sum of the van der Waals radii of the involved atoms ΣRvdw(Os+N) = 3.55 Å, thus, evidencing that the self-assembly of the cocrystals is largely driven by both OmBs. The interactions are quite directional (N≡Os···N angles = 177°). It is also worth emphasizing that the [OsNCl4]− units are negatively charged. For clarity, the counterions (tetrabutylammonium) are not shown. The bond distances of these OmBs are slightly longer than those previously reported in similar adducts of OsO4 and bipyridine [29,30]. An intramolecular version of the OmB is observed in the NOGZOY structure, where the Os···N distance opposite to the Os≡N bond is longer than the other two Os–N distances in the equatorial plane (see Figure 1c) but much shorter than the ΣRvdw(Os+N) = 3.55 Å. Therefore, in this particular case, a partial covalent character of the OmB is envisaged. A similar situation is observed for pentacyano-nitrido-osmium(VI), refcode VECMUL, where the apical cyano groups exhibit a longer Os···C distance (2.364 Å) than the other four Os···C equatorial distances that range from 2.066 Å to 2.093 Å, almost identical to the ΣRcov(Os+C) = 2.10 Å. For the last two examples (NOGZOY and VECNUL), the OmB distances are similar to those previously reported for OsO4 [29].

2.2. MEP Surface Anaysis

Inspired by the JOMKAX structure, the tetramethyl ammonium salt of tetrachloro-nitrido-osmium(VI) (1) has been used as a theoretical model to investigate the existence of a σ-hole on the extension of the N≡Os bond. Figure 2 shows the MEP surfaces of compound 1 using two different orientations and MEP scales. The MEP maximum is, as expected, located at the cationic part of the salt (+79.1 kcal/mol), and the minimum MEP is located along the bisector of the Cl–Os–Cl angle (−56.5 kcal/mol). Remarkably, the MEP surface reveals the presence of a σ-hole opposite to the N≡Os bond (+11.0 kcal/mol), which is adequate for interacting with electron-rich atoms. It is interesting to highlight the positive MEP value, taking into consideration the global negative charge of the [OsNCl4]− unit. This analysis is useful to understand the formation of the JOMKAX cocrystal and the strong directionality of the interaction.

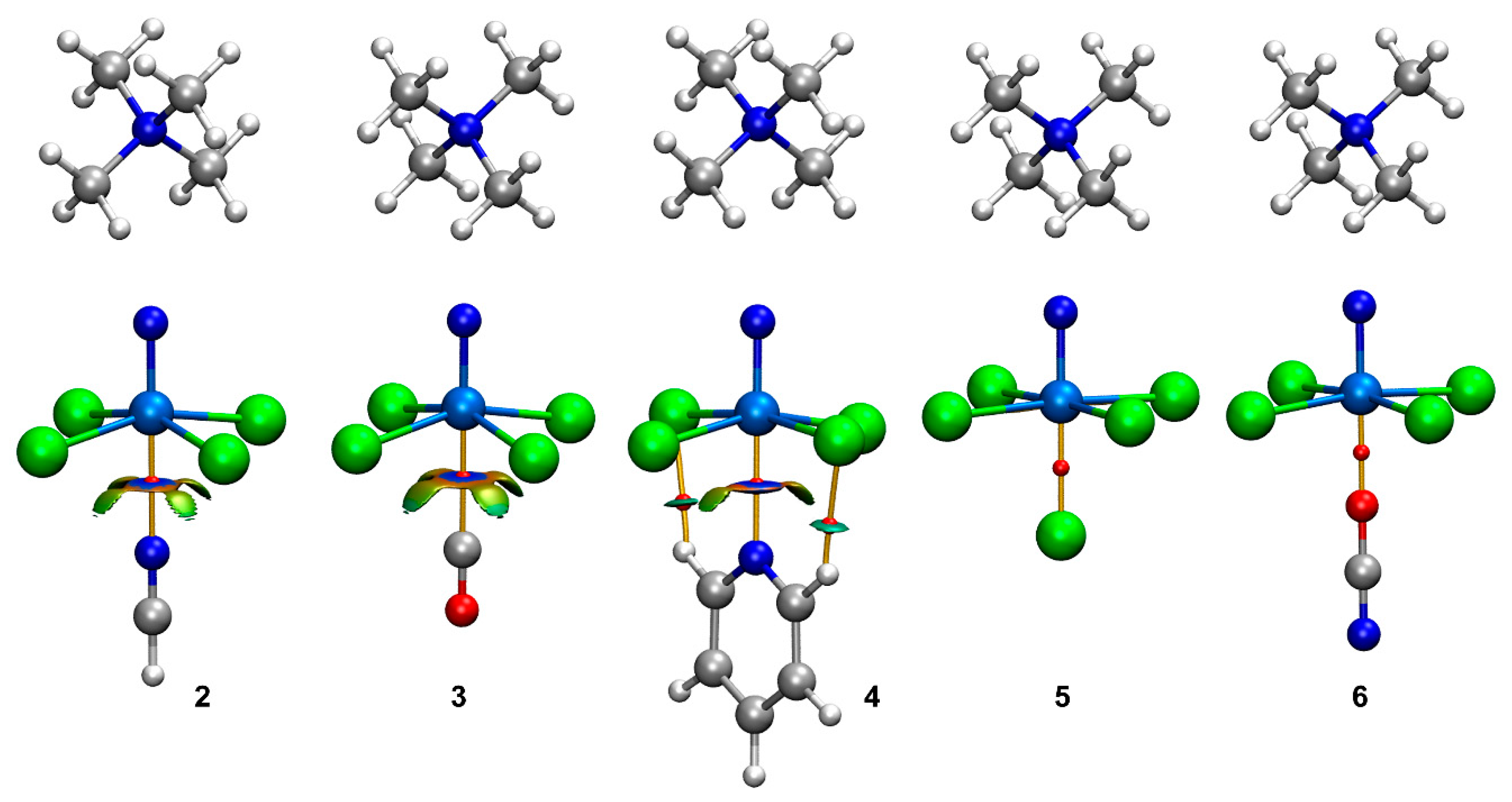
2.3. Energetic and Geometric Analyses

The energetic and geometric features of some model osme-bonded complexes were analyzed in this section. Three neutral Lewis bases and two anionic electron donors (see Scheme 2) were selected to investigate the nature and strength of the OmBs.
Table 2 gathers the interaction energies and equilibrium distances for complexes 2–6. Moreover, the N≡Os–Cl angle values are also summarized in Table 2 as a measure of the deformation of the tetrachloro-nitrido-osmium(VI) unit upon complexation. It can be observed that, in all cases, the interaction energies are moderately strong, varying from −3.33 to −13.82 kcal/mol. The equilibrium distances of the complexes with neutral Lewis bases are clearly longer than the ΣRcov (values in parenthesis in Table 2), thus, disclosing a noncovalent character. This is confirmed by the low deformation of the square-pyramidal geometry of the Os atom since the N≡Os–Cl angles in complexes 2–4 are similar to the angle observed in 1 (104.4°). For the anionic donors, the equilibrium distances are almost identical to the ΣRcov values, thus, suggesting the formation of covalent bonds. Moreover, the geometry of the Os atom changes to an almost perfect square-bipyramid geometry with N≡Os–Cl angles close to 90°.

2.4. Combined QTAIM/NCIplot Analysis

In order to characterize the osme bonds in complexes 2–6, the quantum theory of atoms in molecules (QTAIM) and the noncovalent interaction plot (NCIplot) analyses were combined in the same representation since they are useful to reveal noncovalent interactions in real space. In these representations, blue and green colors are used for strong and weak interactions, respectively, and red and yellow colors are used for strong and weak repulsive interactions, respectively. Figure 3 shows the QTAIM analyses for all compounds, evidencing that, in all cases, the OmB is characterized by a bond critical point (CP, represented as a small red sphere) and a bond path (represented by an orange line) that connects the Os atom to the electron-rich atom of the Lewis base/anion. The density values at the bond CPs are summarized in Table 2, showing larger values for the anionic complexes in line with the shorter distances. In these representations, only the bond CPs and the bond paths connecting the [OsNCl4]− anionic part of the salt and the electron donors are shown.
The NCIplot analysis shows the presence of reduced density gradient (RDG) isosurfaces for the complexes with the neutral electron donors (2–4). For the anionic complexes, no RDG isosurfaces were obtained below the cut-off of 0.05 a.u., used for the electron density in these plots, thus, further confirming the covalent nature of the interaction for the anionic donors. For complexes 2–4, a blue RDG isosurface (meaning attractive interaction) is found coincident with the location of the bond CPs. Additional yellowish isosurfaces are located between the Cl atoms and the lone pair donor atom, thus, suggesting some repulsion with the negative belts of the chlorido ligands. In the case of pyridine, the QTAIM/NCIplot analyses reveal the existence of two ancillary C–H···Cl H-bonding interactions that are characterized by the corresponding bond CPs, bond paths and bluish RDG surfaces interconnecting the H and Cl atoms. The existence of the C–H···Cl interactions explains the larger binding energy obtained for this complex (see Table 2).

2.5. NBO Analysis

A differentiating feature of the σ-hole bonding with respect to coordination bonds is the existence of the typical LP→σ* donor–acceptor interaction. A convenient strategy to analyze such an orbital effect is the natural bond orbital (NBO) method and, in particular, the second-order perturbation analysis (see theoretical methods below). The analysis was been performed on the neutral donors, since they establish non-covalent OmBs. Figure 4 represents the donor and acceptor orbitals and the stabilization energies due to the LP→σ*(Os–N) orbital interaction (E(2) values). In all cases, the LP at the electron-rich atom points to the antibonding σ*(Os–N) orbital that is composed of the dz2 atomic orbital of Os and the sp orbital of N. The E(2) values are large in all cases due to a significant overlap of the donor–acceptor orbitals, in agreement with the short distances. Such large orbital donor–acceptor contributions likely compensate for the electrostatic repulsion between the anionic tetrachloro-nitrido-osmium(VI) moiety and the lone pair. This NBO analysis strongly supports the σ-hole nature of the OmB contacts in complexes 2–4.

2.6. Electron Density (ED) vs. Electrostatic Potential (ESP) Analysis

In order to further analyze the nature of the Os···N contact in compound 4 (also as a model of the X-ray structure JOMKAX), the order of electron density (ED; ρ(r)min) and the electrostatic potential (ESP; φ(r)min) minima have been carried out in their 1D profiles along the Os···N bond path. The QTAIM is based on the concept of atomic basins that are assigned using the zero-flux condition [∇ρ(r)·n(r) = 0] [38] in the electron density. The corresponding QTAIM surfaces are used to establish the interatomic boundaries. Equivalent boundaries can also be determined by using the electrostatic potential instead of the electron density, namely ∇φ(r)·n(r) = 0 [39], which establishes the bonded electroneutral centers. The resulting difference in both boundaries is useful to reveal the donor–acceptor nature of the interaction. In any interaction, the φ(r)min is shifted toward the electron donor atom, while the ρ(r)min is shifted toward the σ-hole donor atom (electron acceptor) [40,41,42].
The 1D profile of the ED and ESP functions along the Os(VI)···N bond path is shown in Figure 5. It can be observed that the Os(VI) atom acts as the electron acceptor since an evident shift of the ED minima toward the Os(VI) electron density basin is appreciated (see small arrow in Figure 5). This evidences that the N atom of pyridine is partially donating electrons to the electrophilic σ–hole of the Os(VI) metal center. This result is in line with the MEP analysis that shows a positive MEP at the Os(VI) atom in spite of the negative charge at the [OsNCl4]− unit.

3. Computational Methods

The geometric optimizations were carried out using the Turbomole 7.2 program [43]. The level of theory used for these optimizations was the PBE0-D3/def2-TZVP [44,45,46]. For osmium, the def2-TZVP basis set used in this work includes effective core potentials (ECP), and relativistic effects are used for the inner electrons [46]. This combination of functional and basis sets has been successfully used before to study osme bonds [29,30]. The MEP surface plots are computed at the same level of theory and at the 0.002 a.u. isosurface. The analysis of the electron charge density was performed using the quantum theory of atoms in molecules (QTAIM) [47] and complemented by the noncovalent interCartesian Coordinates action plot index (NCIplot) [48] by using the reduced density gradient (RDG) isosurfaces. They were plotted using the VMD program [49]. The settings for the RDG plots were as follows: s = 0.5 a.u.; cut-off ρ = 0.05 a.u.; color scale −0.04 a.u. ≤ sign(λ2)ρ ≤ 0.04 a.u. The natural bond orbital (NBO) [50] analysis was performed using the NBO7.0 program [51] at the PBE0-D3/def2-TZVP level of theory (Cartesian Coordinates in Supplementary Material).

4. Conclusions

The combined crystallographic and theoretical results reported in this work consistently prove the attractive interaction between nitrido-osmium(VI) complexes and lone pair donor atoms as an unprecedented σ-hole osme bond interaction. In several crystal structures, it is evidenced that the osme bond has a prominent role in determining the X-ray packing. They are particularly relevant in the formation of self-assembled dimers in FEJZUP and in the cocrystal of pyrazine and tetrachloro-nitrido-osmium(vi), governing the formation of 1:2 adducts (JOMKAX). Finally, the existence of a σ-hole opposite to the N≡Os bond and the participation of the antibonding σ*(N–Os) orbital in the interactions have been also evidenced using the MEP surface and NBO analyses, respectively, thus, strongly supporting the σ-hole nature of the OmBs reported herein.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/inorganics10090133/s1, Cartesian coordinates of the optimized compounds.

Author Contributions

Conceptualization, R.M.G. and A.F.; methodology, R.M.G. and A.F.; formal analysis, R.M.G. and A.F.; investigation, R.M.G. and A.F.; writing—original draft preparation, A.F.; writing—review and editing, R.M.G. and A.F.; visualization, R.M.G. and A.F.; supervision, A.F.; project administration, A.F.; funding acquisition, A.F. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the MICIU/AEI of Spain (project PID2020-115637GB-I00 FEDER funds).

Informed Consent Statement

Not applicable.

Data Availability Statement

Not applicable.

Acknowledgments

We thank the Centre de Tecnologies de la Informació (CTI) at University of the Balearic Islands (UIB) for the computational facilities.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Cavallo, G.; Metrangolo, P.; Pilati, T.; Resnati, G.; Terraneo, G. Naming interactions from the electrophilic site. Cryst. Growth Des. 2014, 14, 2697–2702. [Google Scholar] [CrossRef]
  2. Scheiner, S.; Michalczyk, M.; Zierkiewicz, W. Coordination of anions by noncovalently bonded σ-hole ligands. Coord. Chem. Rev. 2020, 405, 213136. [Google Scholar] [CrossRef]
  3. Bauza, A.; Frontera, A. σ/π-Hole noble gas bonding interactions: Insights from theory and experiment. Coord. Chem. Rev. 2020, 404, 213112. [Google Scholar] [CrossRef]
  4. Lim, J.Y.C.; Beer, P.D. Sigma-hole interactions in anion recognition. Chem 2018, 4, 731–783. [Google Scholar] [CrossRef]
  5. Kolar, M.H.; Hobza, P. Computer modeling of halogen bonds and other σ-hole interactions. Chem. Rev. 2016, 116, 5155–5187. [Google Scholar] [CrossRef] [PubMed]
  6. Daolio, A.; Scilabra, P.; Terraneo, G.; Resnati, G. C(sp3) atoms as tetrel bond donors: A crystallographic survey. Coord. Chem. Rev. 2020, 413, 213265. [Google Scholar] [CrossRef]
  7. Fourmigué, M.; Dhaka, A. Chalcogen bonding in crystalline diselenides and selenocyanates: From molecules of pharmaceutical interest to conducting materials. Coord. Chem. Rev. 2020, 403, 213084. [Google Scholar] [CrossRef]
  8. Biot, N.; Bonifazi, D. Chalcogen-bond driven molecular recognition at work. Coord. Chem. Rev. 2020, 413, 213243. [Google Scholar] [CrossRef]
  9. Scilabra, P.; Terraneo, G.; Resnati, G. The chalcogen bond in crystalline solids: A world parallel to halogen bond. Acc. Chem. Res. 2019, 52, 1313–1324. [Google Scholar] [CrossRef]
  10. Vogel, L.; Wonner, P.; Huber, S.M. Chalcogen bonding: An overview. Angew. Chem. Int. Ed. 2019, 58, 1880–1891. [Google Scholar] [CrossRef]
  11. Strakova, K.; Assies, L.; Goujon, A.; Piazzolla, F.; Humeniuk, H.V.; Matile, S. Dithienothiophenes at work: Access to mechanosensitive fluorescent probes, chalcogen-bonding catalysis, and beyond. Chem. Rev. 2019, 119, 10977–11005. [Google Scholar] [CrossRef] [PubMed]
  12. Politzer, P.; Murray, J.S.; Clark, T. Halogen bonding and other σ-hole interactions: A perspective. Phys. Chem. Chem. Phys. 2013, 15, 11178–11189. [Google Scholar] [CrossRef] [PubMed]
  13. Bauza, A.; Mooibroek, T.J.; Frontera, A. The bright future of unconventional σ/π-hole interactions. ChemPhysChem 2015, 16, 2496–2517. [Google Scholar] [CrossRef] [PubMed]
  14. Pascoe, J.; Ling, K.B.; Cockroft, S.L. The origin of chalcogen-bonding interactions. J. Am. Chem. Soc. 2017, 139, 15160–15167. [Google Scholar] [CrossRef]
  15. Riwar, L.J.; Trapp, N.; Root, K.; Zenobi, R.; Diederich, F. Supramolecular capsules: Strong versus weak chalcogen bonding. Angew. Chem. Int. Ed. 2018, 57, 17259–17264. [Google Scholar] [CrossRef]
  16. Macchione, M.; Goujon, A.; Strakova, K.; Humeniuk, H.V.; Licari, G.; Tajkhorshid, E.; Sakai, N.; Matile, S. A chalcogen-bonding cascade switch for Planarizable push-pull probes. Angew. Chem. Int. Ed. 2019, 58, 15752–15756. [Google Scholar] [CrossRef] [PubMed]
  17. Wonner, P.; Dreger, A.; Vogel, L.; Engelage, E.; Huber, S.M. Chalcogen bonding catalysis of a nitro-Michael reaction. Angew. Chem. Int. Ed. 2019, 58, 16923–16927. [Google Scholar] [CrossRef]
  18. Wang, W.; Zhu, H.; Liu, S.; Zhao, Z.; Zhang, L.; Hao, J.; Wang, Y. Chalcogen-chalcogen bonding catalysis enables assembly of discrete molecules. J. Am. Chem. Soc. 2019, 141, 9175–9179. [Google Scholar] [CrossRef]
  19. Borissov, A.; Marques, I.; Lim, J.Y.C.; Félix, V.; Smith, M.D.; Beer, P.D. Anion recognition in water by charge-neutral halogen and chalcogen bonding foldamer receptors. J. Am. Chem. Soc. 2019, 141, 4119–4129. [Google Scholar] [CrossRef]
  20. Benz, S.; Macchione, M.; Verolet, Q.; Mareda, J.; Sakai, N.; Matile, S. Anion transport with chalcogen bonds. J. Am. Chem. Soc. 2016, 138, 9093–9096. [Google Scholar] [CrossRef] [Green Version]
  21. Heywood, V.L.; Alford, T.P.J.; Roeleveld, J.J.; Lekanne Deprez, S.J.; Verhoofstad, A.; van der Vlugt, J.I.; Domingos, S.R.; Schnell, M.; Davis, A.P.; Mooibroek, T.J. Observations of tetrel bonding between sp3-carbon and THF. Chem. Sci. 2020, 11, 5289–5293. [Google Scholar] [CrossRef] [PubMed]
  22. Politzer, P.; Murray, J.S. σ-holes and π-holes: Similarities and differences. J. Comput. Chem. 2018, 39, 464–471. [Google Scholar] [CrossRef] [PubMed]
  23. Stenlid, J.H.; Johansson, A.J.; Brinck, T. σ-Holes and σ-lumps direct the Lewis basic and acidic interactions of noble metal nanoparticles: Introducing regium bonds. Phys. Chem. Chem. Phys. 2018, 20, 2676–2692. [Google Scholar] [CrossRef] [PubMed]
  24. Stenlid, J.H.; Brinck, T. Extending the σ-hole concept to metals: An electrostatic interpretation of the effects of nanostructure in gold and platinum catalysis. J. Am. Chem. Soc. 2017, 139, 11012–11015. [Google Scholar] [CrossRef]
  25. Legon, A.C.; Walker, N.R. What’s in a name? ‘Coinage-metal’ non-covalent bonds and their definition. Phys. Chem. Chem. Phys. 2018, 20, 19332–19338. [Google Scholar] [CrossRef]
  26. Bauzá, A.; Alkorta, I.; Elguero, J.; Mooibroek, T.J.; Frontera, A. Spodium bonds: Noncovalent interactions involving group 12 elements. Angew. Chem. Int. Ed. 2020, 59, 17482–17487. [Google Scholar] [CrossRef]
  27. Bauzá, A.; Frontera, A. Noncovalent interactions involving group 6 in biological systems: The case of molybdopterin and Tungstopterin cofactors. Chem Eur. J. 2022, 28, e202201660. [Google Scholar] [CrossRef]
  28. Daolio, A.; Pizzi, A.; Terraneo, G.; Frontera, A.; Resnati, G. Anion···anion interactions involving σ-holes of perrhenate, pertechnetate and permanganate anions. ChemPhysChem 2021, 22, 2281–2285. [Google Scholar] [CrossRef]
  29. Daolio, A.; Pizzi, A.; Calabrese, M.; Terraneo, G.; Bordignon, S.; Frontera, A.; Resnati, G. Molecular electrostatic potential and noncovalent interactions in derivatives of group 8 elements. Angew. Chem. Int. Ed. 2021, 60, 20723–20727. [Google Scholar] [CrossRef]
  30. Daolio, A.; Pizzi, A.; Calabrese, M.; Terraneo, G.; Bordignon, S.; Frontera, A.; Resnati, G. Osme bond: Anisotropic distribution of electron density in action. Acta Cryst. 2021, A77, C800. [Google Scholar]
  31. Chan, P.M.; Yu, W.-Y.; Che, C.-M.; Cheung, K.-K. Nitrido-ruthenium(VI) and -osmium(VI) complexes containing chelating multianionic (N, O) ligands. Synthesis, crystal structures and reactions with triphenylphosphine. J. Chem. Soc. Dalton Trans. 1998, 3183–3190. [Google Scholar] [CrossRef]
  32. Che, C.-M.; Lau, T.-C.; Lam, H.-W.; Poon, C.-K. Metal-nitrido photo-oxidants: Synthesis, photophysics, and photochemistry of [OsVI(NH3)4(N)](X)3(X = Cl, CF3SO3). J. Chem. Soc. Chem. Commun. 1989, 114–116. [Google Scholar] [CrossRef]
  33. Lam, H.-W.; Che, C.-M.; Wong, K.-Y. Photoredox properties of [OsN(NH3)4]3+ and mechanism of formation of [{Os(NH3)4(CH3CN)}2N2]5+ through a nitrido-coupling reaction. J. Chem Soc. Dalton Trans. 1992, 1411–1416. [Google Scholar] [CrossRef]
  34. Chin, K.-F.; Cheung, K.-K.; Yip, H.-K.; Mak, T.C.-W.; Che, C.-M. Luminescent nitridometal complexes. Photophysical and photochemical properties of the 3[(dxy)1(dπ*)1] excited state of nitridoosmium(VI) complexes with polypyridine ligands. J. Chem. Soc. Dalton Trans. 1995, 657–663. [Google Scholar] [CrossRef]
  35. Che, C.-M.; Wong, K.-Y.; Lam, H.-W.; Chin, K.-F.; Zhou, Z.-Y.; Mak, T.C.-W. Photophysical properties, electrochemistry and crystal structure of nitridoosmium(VI) complexes of 2,3-diamino-2,3-dimethylbutane. J. Chem. Soc. Dalton Trans. 1993, 857–861. [Google Scholar] [CrossRef]
  36. Pipes, D.W.; Bakir, M.; Vitols, S.E.; Hodgson, D.J.; Meyer, T.J. Reversible interconversion between a nitrido complex of osmium(VI) and an ammine complex of osmium(II). J. Am. Chem. Soc. 1990, 112, 5507–5514. [Google Scholar] [CrossRef]
  37. Groom, C.R.; Bruno, I.J.; Lightfoot, M.P.; Ward, S.C. The Cambridge structural database. Acta Cryst. 2016, B72, 171–179. [Google Scholar] [CrossRef]
  38. Tsirelson, V.G.; Avilov, A.S.; Lepeshov, G.G.; Kulygin, A.K.; Stahn, J.; Pietsch, U.; Spence, J.C.H. Zero-flux surfaces of the electrostatic potential: The border of influence zones of nucleophilic and electrophilic sites in crystalline environment. J. Phys. Chem. B 2001, 105, 5068–5074. [Google Scholar] [CrossRef]
  39. Mata, I.; Molins, E.; Alkorta, I.; Espinosa, E. Topological properties of the electrostatic potential in weak and moderate N···H hydrogen bonds. J. Phys. Chem. A 2007, 111, 6425–6433. [Google Scholar] [CrossRef]
  40. Bartashevich, E.V.; Matveychuk, Y.V.; Troitskaya, E.A.; Tsirelson, V.G. Characterizing the multiple non-covalent interactions in N, S-heterocycles–diiodine complexes with focus on halogen bonding. Comput. Theor. Chem. 2014, 1037, 53–62. [Google Scholar] [CrossRef]
  41. Bartashevich, E.; Yushina, I.; Kropotina, K.; Muhitdinova, S.; Tsirelson, V. Testing the tools for revealing and characterizing the iodine-iodine halogen bond in crystals. Acta Cryst. 2017, B73, 217–226. [Google Scholar] [CrossRef] [PubMed]
  42. Bartashevich, E.; Mukhitdinova, S.; Yushina, I.; Tsirelson, V. Electronic criterion for categorizing the chalcogen and halogen bonds: Sulfur-iodine interactions in crystals. Acta Cryst. 2019, B7, 117–126. [Google Scholar] [CrossRef] [PubMed]
  43. Ahlrichs, R.; Bar, M.; Haser, M.; Horn, H.; Kolmel, C. Electronic structure calculations on workstation computers: The program system turbomole. Chem. Phys. Lett. 1989, 162, 165–169. [Google Scholar] [CrossRef]
  44. Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
  45. Weigend, F.; Ahlrichs, R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef] [PubMed]
  46. Weigend, F. Accurate Coulomb-fitting basis sets for H to Rn. Phys. Chem. Chem. Phys. 2006, 8, 1057–1065. [Google Scholar] [CrossRef] [PubMed]
  47. Bader, R.F.W. A quantum theory of molecular structure and its applications. Chem. Rev. 1991, 91, 893–928. [Google Scholar] [CrossRef]
  48. Contreras-García, J.; Johnson, E.R.; Keinan, S.; Chaudret, R.; Piquemal, J.-P.; Beratan, D.N.; Yang, W. NCIPLOT: A program for plotting non-covalent interaction regions. J. Chem. Theory Comput. 2011, 7, 625–632. [Google Scholar] [CrossRef] [PubMed]
  49. Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual molecular dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]
  50. Glendening, E.D.; Landis, C.R.; Weinhold, F. Natural bond orbital methods. WIREs Comput. Mol. Sci. 2012, 2, 1–42. [Google Scholar] [CrossRef]
  51. Glendening, E.D.; Badenhoop, J.K.; Reed, A.E.; Carpenter, J.E.; Bohmann, J.A.; Morales, C.M.; Karafiloglou, P.; Landis, C.R.; Weinhold, F. NBO 7.0: New Vistas in Localized and Delocalized Chemical Bonding Theory; Theoretical Chemistry Institute, University of Wisconsin-Madison: Madison, WI, USA, 2018. [Google Scholar]
Inorganics 10 00133 sch001 550
Scheme 1. Osme bonds previously described in the literature (left) [29] and the new ones proposed in this work (right) using nitro-osmium(VI) derivatives.
Scheme 1. Osme bonds previously described in the literature (left) [29] and the new ones proposed in this work (right) using nitro-osmium(VI) derivatives.
Inorganics 10 00133 sch001
Inorganics 10 00133 g001 550
Figure 1. (a) Partial view of the X-ray structure with refcode FEJZUP. Distances are in Å. H atoms are omitted for clarity; (b) partial view of the X-ray structure with refcode JOMKAX. Distances are in Å. H atoms and counter-ions are omitted for clarity; (c) partial view of the X-ray structure with refcode NOGZOY. Distances are in Å. H atoms are omitted for clarity; (d) partial view of the X-ray structure with refcode VECMUL. Distances are in Å. H atoms and counter-ions are omitted for clarity.
Figure 1. (a) Partial view of the X-ray structure with refcode FEJZUP. Distances are in Å. H atoms are omitted for clarity; (b) partial view of the X-ray structure with refcode JOMKAX. Distances are in Å. H atoms and counter-ions are omitted for clarity; (c) partial view of the X-ray structure with refcode NOGZOY. Distances are in Å. H atoms are omitted for clarity; (d) partial view of the X-ray structure with refcode VECMUL. Distances are in Å. H atoms and counter-ions are omitted for clarity.
Inorganics 10 00133 g001
Inorganics 10 00133 g002 550
Figure 2. MEP surface of compound 1 using perspective (a) and “on-top” (b) representations. The MEP values at some points on the surfaces are given in kcal/mol. Level of theory: PBE0-D3/def2-TZVP. Isosurface: 0.002 a.u.
Figure 2. MEP surface of compound 1 using perspective (a) and “on-top” (b) representations. The MEP values at some points on the surfaces are given in kcal/mol. Level of theory: PBE0-D3/def2-TZVP. Isosurface: 0.002 a.u.
Inorganics 10 00133 g002
Inorganics 10 00133 sch002 550
Scheme 2. Compound 1 and complexes 2–6 used in this work.
Scheme 2. Compound 1 and complexes 2–6 used in this work.
Inorganics 10 00133 sch002
Inorganics 10 00133 g003 550
Figure 3. QTAIM/NCIPlot analyses of the OmB complexes 2–6 at the PBE0-D3/def2-TZVP. Only intermolecular contacts between the anionic unit and the electron donors are represented by bond CPs and RDG isosurfaces. See computational methods for the NCIplot settings.
Figure 3. QTAIM/NCIPlot analyses of the OmB complexes 2–6 at the PBE0-D3/def2-TZVP. Only intermolecular contacts between the anionic unit and the electron donors are represented by bond CPs and RDG isosurfaces. See computational methods for the NCIplot settings.
Inorganics 10 00133 g003
Inorganics 10 00133 g004 550
Figure 4. Representation of the NBOs corresponding to the LP→σ* donor–acceptor interactions in the OmB complexes 2 (a), 3 (b) and 4 (c). The isosurface used for the MOs is 0.008 a.u.
Figure 4. Representation of the NBOs corresponding to the LP→σ* donor–acceptor interactions in the OmB complexes 2 (a), 3 (b) and 4 (c). The isosurface used for the MOs is 0.008 a.u.
Inorganics 10 00133 g004
Inorganics 10 00133 g005 550
Figure 5. The ED (blue curve) and ESP (orange curve) 1D profile along the Os(VI)···N for complex 4.
Figure 5. The ED (blue curve) and ESP (orange curve) 1D profile along the Os(VI)···N for complex 4.
Inorganics 10 00133 g005
Table
Table 1. CSD reference codes of the nitrido-osmium(VI) derivatives present in the database. Those exhibiting osme bonds are shown in italics.
Table 1. CSD reference codes of the nitrido-osmium(VI) derivatives present in the database. Those exhibiting osme bonds are shown in italics.
Reference Codes
BAGRIKLIFJUFSEMHEA
FEJZUPMIZWATSEMHIE
FEKBECMOQQUEVECMUL
FOCWOKNOGZEOWAKGUK
FUTNAKNOGZISWAKHAR
HUZYUWNOGZOYYACDIP
HUZZADQADJOVYUNYUY
HUZZEHQADJUB
JOMKAXQUFYAU
Table
Table 2. Interaction energies (ΔE, kcal/mol), equilibrium distances (d, Å), N≡Os–Cl angle (α, °) and electron density at the bond critical points (ρ, a.u.) of complexes 2–6.
Table 2. Interaction energies (ΔE, kcal/mol), equilibrium distances (d, Å), N≡Os–Cl angle (α, °) and electron density at the bond critical points (ρ, a.u.) of complexes 2–6.
ComplexΔEd 1α 2ρ
2−3.102.523 (2.15)100.4880.038
3−5.042.604 (2.21)101.5650.037
4−13.822.555 (2.15)100.5030.042
5−9.112.452 (1.46)92.2270.067
6−3.332.095 (2.00)94.2900.082
1 Measure from the osmium to the electron-rich atom. 2 The ΣRcov is indicated in parenthesis. The N≡Os–Cl angle in 1 is 104.4°.
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Gomila, R.M.; Frontera, A. Crystallographic and Theoretical Study of Osme Bonds in Nitrido-Osmium(VI) Complexes. Inorganics 2022, 10, 133. https://doi.org/10.3390/inorganics10090133

AMA Style

Gomila RM, Frontera A. Crystallographic and Theoretical Study of Osme Bonds in Nitrido-Osmium(VI) Complexes. Inorganics. 2022; 10(9):133. https://doi.org/10.3390/inorganics10090133

Chicago/Turabian Style

Gomila, Rosa M., and Antonio Frontera. 2022. "Crystallographic and Theoretical Study of Osme Bonds in Nitrido-Osmium(VI) Complexes" Inorganics 10, no. 9: 133. https://doi.org/10.3390/inorganics10090133

APA Style

Gomila, R. M., & Frontera, A. (2022). Crystallographic and Theoretical Study of Osme Bonds in Nitrido-Osmium(VI) Complexes. Inorganics, 10(9), 133. https://doi.org/10.3390/inorganics10090133

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop