Micromixing and Co-Precipitation in Continuous Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows
Abstract
:1. Introduction
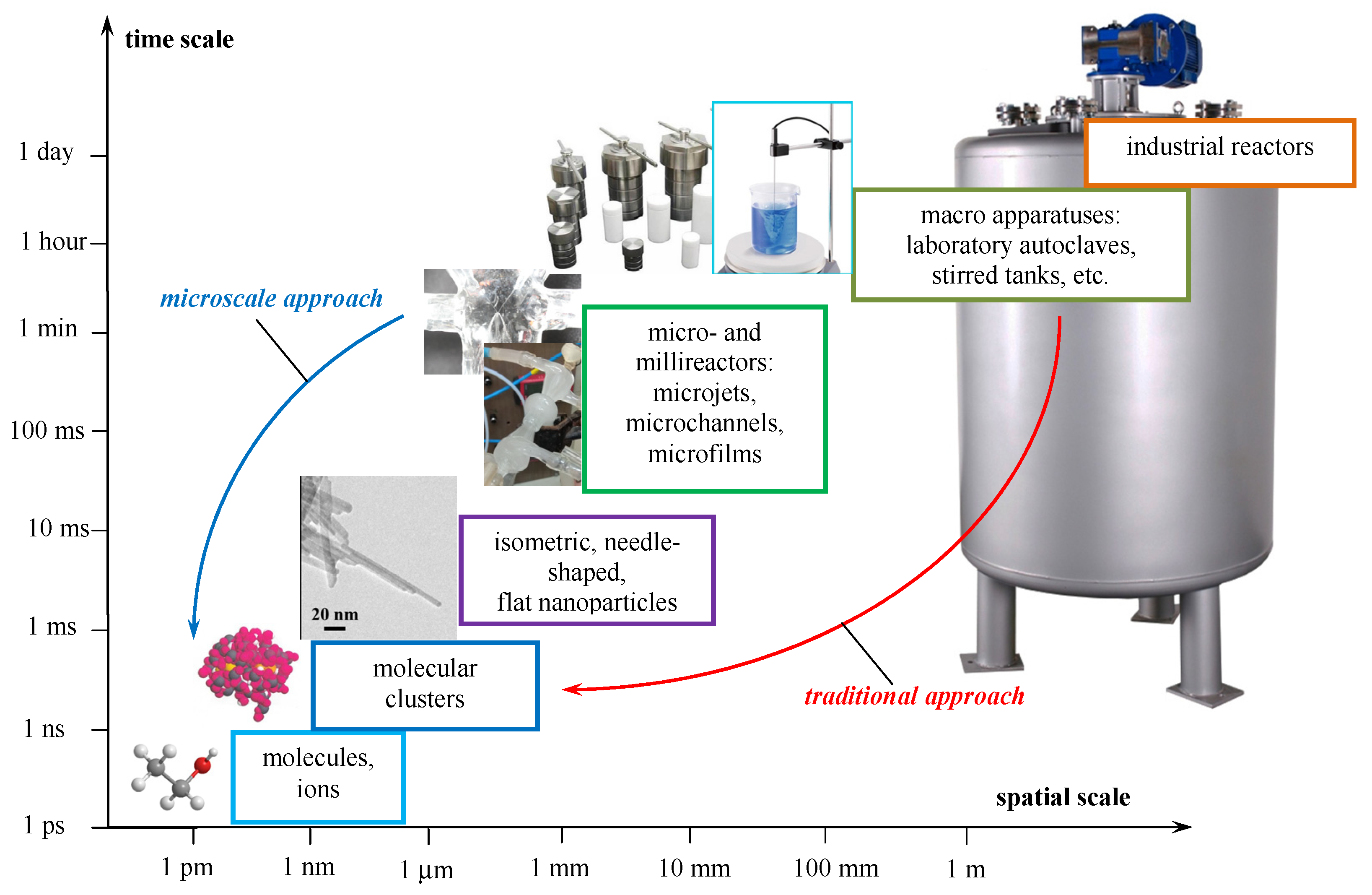
1.1. Macroscaled Reactors vs. Milli or Microscaled Reactors
- (1)
- At the first stage of the process, the substances participating in the formation of particles are distributed fairly uniformly over the volume of the solution at the lowest possible size level, i.e., at the ionic level. As a result of such uniform distribution, the formation of clusters (with a size less than the critical size of the nucleus) with a given chemical composition is ensured.
- (2)
- After the first stage, there is a short period of time (the second stage) during which the formation of nuclei from clusters occurs. The second stage is needed to allow the clusters to aggregate into nuclei.
- (3)
- At the third stage of the process, after the formation of clusters and nuclei, the separation of particles is necessary, which prevents their further spontaneous uncontrolled growth.
- (a)
- To give an opportunity to form nuclei (either amorphous or crystal) with a given chemical composition and structure.
- (b)
- To allow them to reach a critical size (or slightly larger, depending on the main task of synthesis).
- (c)
- To separate particles in space after the formation of nuclei, as far as possible preventing them from forming larger aggregates and agglomerates.
1.2. Macro-, Meso- and Micromixing Scales
- Macro-mixing corresponds to the whole scale of the apparatus (i.e., it has a spatial order of the apparatus diameter, the stirrer diameter). It determines the conditions for the transfer of matter by large-scale convection over the entire volume of the flow in the reactor and is characterized by the average velocity of macrovolumes movement and the residence time distribution. Essentially, the average residence time characterizes the duration of the liquid macrovolumes “live”, from the input into the apparatus to the output from it. This movement is responsible for the transfer of liquid macrovolumes from the “passive” zones to the zones with a high energy dissipation rate (in the immediate vicinity of the mixer). A simple example is the circulating flow in a reactor with a pitched blade or Rushton turbine.
- Meso-mixing takes place at an intermediate level and is responsible for large-scale turbulent transfer between the energy-carrying flow introduced into the apparatus (stirrer, free-convective flows, jets, bubbling gas, etc.) and its environment. A fast chemical reaction usually takes place near the point of entry (zone of “feed”, entry of the “fresh” stream), which forms a “plume” (blob) with a high concentration of product around the injected stream. This plume is an intermediate scale between the micromixing level and the size of the reactor. The spatial evolution of the plume is determined by the turbulent diffusion process. Another aspect of meso-mixing relates to the inertial-convective process of disintegration of large vortices and is characterized by an almost complete absence of the influence of molecular diffusion since the dominant mechanism is convection. On the other hand, inertial-convective mixing influences micro-mixing processes. A complete understanding and precise description of inertial-convective mixing are still lacking. The turbulence kinetic energy k, the scale of the length of turbulent fluctuations L, and their combination, expressed by the coefficient of turbulent diffusion Dt, are used as quantitative characteristics of meso-mixing. At the same time, meso-mixing also depends on specific conditions, such as the geometry of the apparatus, the ratio of the feed rates, and the average flow velocity in the apparatus.
- Micro-mixing is the last stage of mixing in traditional apparatus and consists of viscous-convective deformation of liquid elements, which accelerates the disintegration of liquid aggregates up to the diffusion scale [18]. The selectivity of the reactions ultimately depends on micromixing, i.e., on how the reagents are mixed at the molecular level [16]. This mechanism entails the engulfment and deformation of vortices of the Kolmogorov scale λk and is the limiting process in reducing local concentration gradients. Quantitative characteristics are the micro-mixing time associated with the energy dissipation rate ε [16]: the higher ε, the better the micromixing and the higher the selectivity of fast reactions.
2. Features of Hydrodynamics of Microreactors with Swirled Flows of Different Types
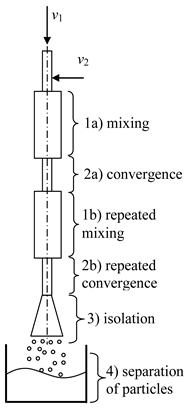
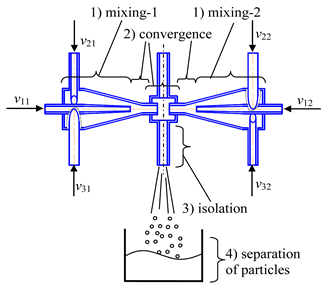
2.1. Descriptions of Four Microreactors with Swirled Flows
- (1)
- Microreactor with swirled flows (Table 1, c) contains a housing, two or more feeding pipes for supplying initial solutions, and an outlet pipe for removing products, the housing has the shape of a cylinder with a lid, which turns into a conical confuser with a neck in a narrow part and subsequent conical expansion in the form of a diffuser.
- (2)
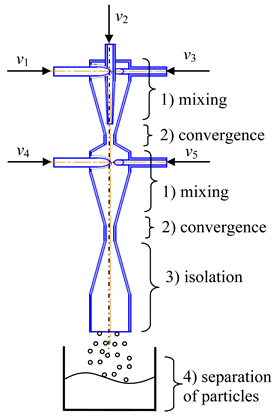
- Two-step (multistage) microreactor with swirled flows (two-step/multistage micro-VJA) (Table 1, e). A multistage (particularly, two-step) swirling flow microreactor contains a body, pipes for supplying solutions of the reacting components, and a pipe for product removal. The body has a multitier shape, each tier corresponds to one mixing stage, the tiers are located coaxially to each other, each tier includes a lid, a cylindrical part that turns into a conical confuser with a neck in a narrow part, and the last tier is equipped with a conical expansion in the form of a diffuser, with an outlet for the removal of the product. At least one feeding pipe is installed on each tier tangentially to the cylindrical part of the body, and a central feeding pipe is installed in the cover of the upper tier coaxially with the body.
- (3)
- Microreactor with impinging swirled flows (counter-current axial velocities) (micro-VJA-ISF-CC) (Table 1, d) contains housing, nozzles for supplying solutions of initial components, feeding pipes for supplying solutions of additional components. The body of a microreactor consists of two swirl chambers, each of which is similar to the case shown in Table 1, c. The throats of each swirl chamber are located coaxially to each other in a mixing chamber equipped with an outlet pipe for products, and there is an axial gap between the throats.
- (4)
- Microreactor with impinging swirled flows (co-directional axial velocities) (micro-VJA-ISF-CD) (Table 1, f) contains a housing, feeding pipes for supplying initial solutions and additional components, and an outlet pipe. The housing of the microreactor is made of two coaxially arranged swirl chambers, each of which contains a cover, a cylindrical part, passing into a conical confuser with a throat in a narrow part.
2.2. Comparison with the Microstructured Cyclone Type Micromixer (KIT)
- (1)
- In new microreactors the flow has a varying cross-section. The confinement in the confuser results in the acceleration of both vorticity ω and circular velocity wt in the area close to the throat of the device. This leads to the additional elongation and stretching of the vortices (see Figure 3); hence, better engulfment and micromixing could be expected.
- (2)
- In new microreactors with impinging swirled flows the impingement of the results of the flow in the transformation of doubled kinetic energies during flow collision. This is expected to bring an additional increase in micromixing.
2.3. Velocity Transformations within Microreactor with Swirled Flows
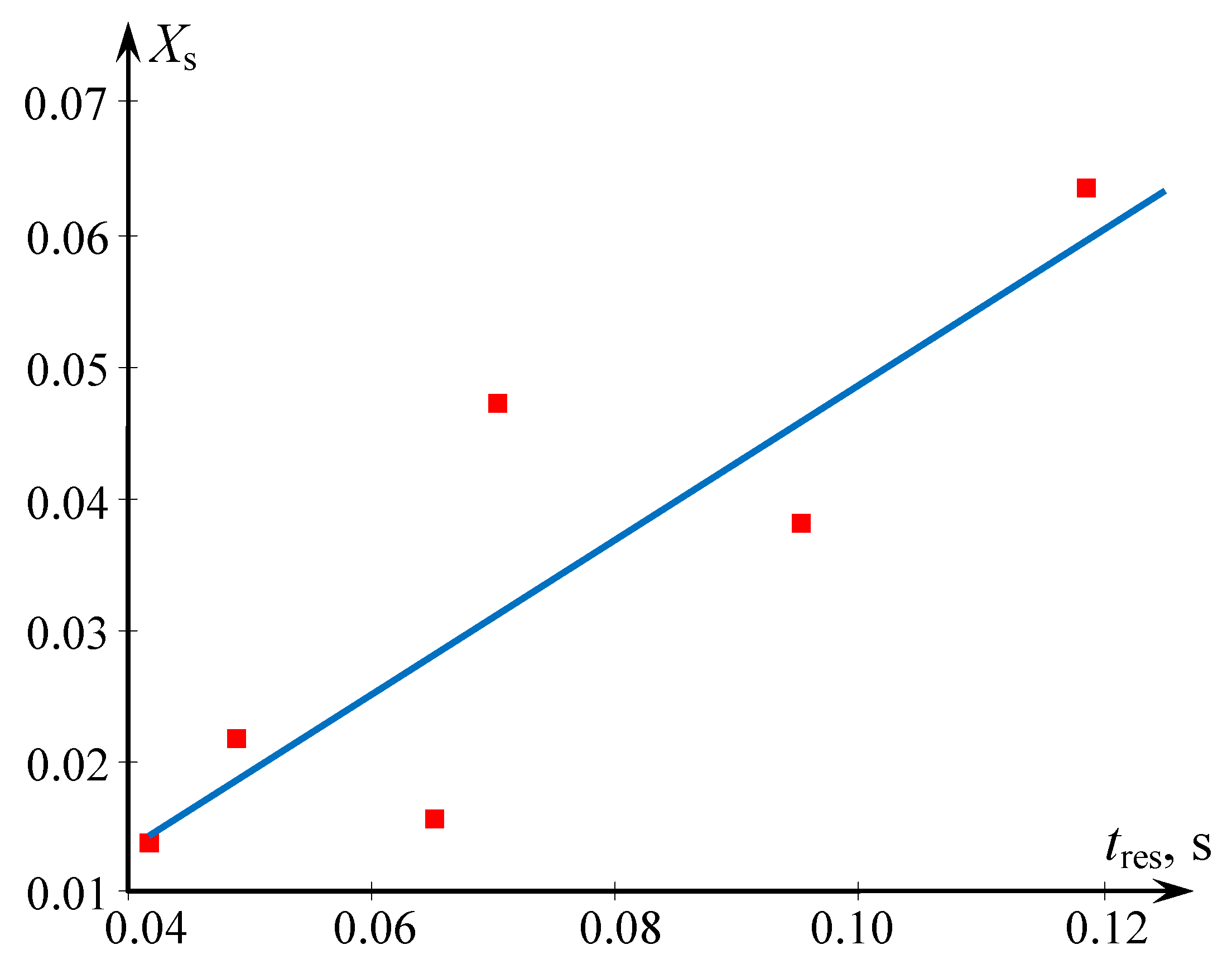
2.4. Experimental Study of Micromixing in Two-Step (Multistage) Microreactor with Swirled Flows
3. Examples of the Nanosized Particles Synthesis in Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows
3.1. Synthesis of Zirconium Dioxide Based Powders in a Microreactor with Impinging Swirling Flows
3.2. Synthesis of Fluorides in Microreactor with Impinging Swirled Flows
4. Conclusions
- (1)
- A detailed experimental study of the influence of various factors on the micromixing in all four types of Microreactors with swirled flows and Microreactors with impinging swirled flows should be performed (at the moment two of four micro-VJA reactors are studied, preparation of papers is in progress);
- (2)
- Extensive CFD studies of the micro-VJA reactor should be done in the nearest future, in order to find the optimal regimes of operation;
- (3)
- Detailed correlations between various input parameters and the “composition-structure-properties” triad should be found.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notation
| D—diffusivity, m2/s |
| Dt—turbulent diffusion, m2/s |
| k—turbulence kinetic energy, m2/s2 |
| K—specific kinetic energy of flow, dynamic pressure (K = ρw2/2), Pa |
| p—hydrostatic pressure, Pa |
| Q—flow rate, m3/s |
| r—radial axis |
| R—radius, m |
| t—time, s |
| tdif—diffusion characteristic time, s |
| tm—micromixing time, s |
| Vμm—volume of the micromixing zone, m3 |
| v—velocity, m/s |
| wa, wt, wr—axial, tangential, and radial components of liquid velocity, m/s |
| w—velocity vector, m/s |
| Xs—segregation index |
| δ—thickness of the layer, m |
| ε—energy dissipation rate, W/kg |
| ρ—liquid density, kg/m3 |
| φ—degree of filling |
| ω—vorticity, 1/s |
| Subscripts: |
| low—lower chamber |
| res—residence |
| up—upper chamber |
| 1, 2, 3, 4, 5, 11,12, 21, 22, 31—number of flow (Table 1) |
References
- Abiev, R.S.; Almjasheva, O.V.; Popkov, V.I.; Proskurina, O.V. Microreactor Synthesis of Nanosized Particles: The Role of Micromixing, Aggregation, and Separation Processes in Heterogeneous Nucleation. Chem. Eng. Res. Des. 2022, 178, 73–94. [Google Scholar] [CrossRef]
- Chatterjee, S.; Houlding, T.K.; Doluda, V.Y.; Molchanov, V.P.; Matveeva, V.G.; Rebrov, E.V. Thermal Behavior of a Catalytic Packed-Bed Milli-reactor Operated under Radio Frequency Heating. Ind. Eng. Chem. Res. 2017, 56, 13273–13280. [Google Scholar] [CrossRef] [Green Version]
- Bai, X.; Tong, H.; Wang, C.; Li, Y.; Tan, Q.; Ye, Z.; Pan, L.; Zhao, Y. Helical Carbon Nanowires for Magnetic-Field-Controlled Swimming. ACS Appl. Nano Mater. 2022, 5, 9981–9989. [Google Scholar] [CrossRef]
- Chesnitskiy, A.V.; Gayduk, A.E.; Seleznev, V.A.; Prinz, V.Y. Bio-Inspired Micro- and Nanorobotics Driven by Magnetic Field. Materials 2022, 15, 7781. [Google Scholar] [CrossRef]
- Shen, F.; Cao, N.; Li, H.; Wu, Z.; Xie, S.; Luo, J. Local Three-Dimensional Characterization of Nonlinear Grain Boundary Length within Bulk ZnO Using Nanorobot in SEM. Crystals 2022, 12, 1558. [Google Scholar] [CrossRef]
- Almjasheva, O.V.; Gusarov, V.V. Metastable clusters and aggregative nucleation mechanism. Nanosyst. Phys. Chem. Math. 2014, 5, 405–416. [Google Scholar]
- Abiev, R.S.; Almjasheva, O.V.; Gusarov, V.V.; Izotova, S.G. Method of Producing Nanopowder of Cobalt Ferrite and Microreactor to This End. Patent RU 2625981, 20 July 2017. [Google Scholar]
- Abiev, R.S. Method of Producing Gas-Fluid Reactions in Sub- and Supercritical Fluid. Patent RU 2342990, 10 January 2009. [Google Scholar]
- Abiev, R.S. Microreactor with Swirled Reagent Solution Streams. Patent RU 2736287, 13 November 2020. [Google Scholar]
- Abiev, R.S. Mixer Microreactor with Counter Swirling Flows. Patent RU 2744173, 3 March 2021. [Google Scholar]
- Abiev, R.S. Microreactor-Multi-Stage Mixer with Swirling Flows. Patent RU 2748486, 26 May 2021. [Google Scholar]
- Abiev, R.S. Microreactor-Mixer in Opposite Swirled Flows. Patent RU 2741735, 28 January 2021. [Google Scholar]
- Teychené, S.; Rodríguez-Ruiz, I.; Ramamoorthy, R.K. Reactive crystallization: From mixing to control of kinetics by additives. Curr. Opin. Colloid Interface Sci. 2020, 46, 1–19. [Google Scholar] [CrossRef]
- Bałdyga, J.; Bourne, J.R. Transport phenomena and hydrodynamics of complex flows. In Encyclopedia of Fluid Mechanics; Cheremisinoff, N.P., Ed.; Gulf Publishing Company: Houston, TX, USA, 1986; Volume 1, p. 145. [Google Scholar]
- Bałdyga, J.; Bourne, J.R. Interactions between mixing on various scales in stirred tank reactors. Chem. Eng. Sci. 1992, 47, 1839–1848. [Google Scholar] [CrossRef]
- Bałdyga, J.; Bourne, J.R. Turbulent Mixing and Chemical Reactions; Wiley: Chichester, UK, 1999. [Google Scholar]
- Ghanem, A.; Lemenand, T.; Valle, D.D.; Peerhossaini, H. Static mixers: Mechanisms, applications, and characterization methods—A review. Chem. Eng. Res. Des. 2014, 92, 205–228. [Google Scholar] [CrossRef]
- Bałdyga, J.; Bourne, J.R. Simplification of micro-mixing calculations. I. Derivation and application of a new model. Chem. Eng. J. 1989, 42, 83–92. [Google Scholar] [CrossRef]
- Kölbl, A.; Kraut, M.; Dittmeyer, R. Kinetic investigation of the Dushman reaction at concentrations relevant to mixing studies in microstructured cyclone type mixers. Chem. Eng. Sci. 2013, 101, 454–460. [Google Scholar] [CrossRef]
- Kölbl, A.; Kraut, M.; Wenka, A. Design parameter studies on cyclone type mixers. Chem. Eng. J. 2011, 167, 444–454. [Google Scholar] [CrossRef]
- Guichardon, P.; Falk, L. Characterisation of micromixing efficiency by the iodide-iodate reaction system. Part I: Experimental procedure. Chem. Eng. Sci. 2000, 55, 4233–4243. [Google Scholar]
- Falk, L.; Commenge, J.-M. Performance comparison of micromixers. Chem. Eng. Sci. 2010, 65, 405–411. [Google Scholar] [CrossRef]
- Fedorenko, N.Y.; Abiev, R.S.; Kudryashova, Y.S.; Ugolkov, V.L.; Khamova, T.V.; Mjakin, S.V.; Zdravkov, A.V.; Kalinina, M.V.; Shilova, O.A. Comparative study of zirconia based powders prepared by co-precipitation and in a microreactor with impinging swirled flows. Ceram. Int. 2022, 48, 13006–13013. [Google Scholar] [CrossRef]
- Anciferova, I.V.; Makarova, E.N. Study of ultrasonic treatment and pre-wetting in ethanol on ZrO2–Y2O3–CeO2–Al2O3 nanopowders size distribution and agglomeration degree. Perspect. Mater. 2015, 1, 41–48. (In Russian) [Google Scholar]
- Fenelonov, V.B. Introduction to the Physical Chemistry of the Formation of the Supramolecular Structure of Adsorbents and Catalysts; SO RAN: Novosibirsk, Russia, 2002; 414p. (In Russian) [Google Scholar]
- Fedorenko, N.Y.; Kudryashova, Y.S.; Myakin, S.V.; Shilova, O.A.; Kalinina, M.V.; Zdravkov, A.V.; Abiev, R.S. Comparative Characteristics of Xerogels Based on Zirconium Dioxide Obtained by the Method of Joint Deposition of Hydroxides in a Volume and a Microreactor with Counter Swirled Flows. Glass Phys. Chem. 2021, 47, 653–656. [Google Scholar] [CrossRef]
- Abiev, R.S.; Zdravkov, A.V.; Kudryashova, Y.S.; Alexandrov, A.A.; Kuznetsov, S.V.; Fedorov, P.P. Synthesis of Calcium Fluoride Nanoparticles in a Microreactor with Intensely Swirling Flows. Russ. J. Inorg. Chem. 2021, 66, 1047–1052. [Google Scholar] [CrossRef]
- Fedorov, P.P.; Osiko, V.V. Relationship between the faceting of crystals and their formation mechanism. Dokl. Phys. 2019, 64, 353–355. [Google Scholar] [CrossRef]
- Kudryashova, Y.S.; Zdravkov, A.V.; Abiev, R.S. Synthesis of Yttrium-Aluminum Garnet Using a Microreactor with Impinging Jets. Glass Phys. Chem. 2021, 47, 260–264. [Google Scholar] [CrossRef]
- Abiev, R.S.; Makusheva, I.V. Energy Dissipation Rate and Micromixing in a Two-Step Micro-Reactor with Intensively Swirled Flows. Micromachines 2022, 13, 1859. [Google Scholar] [CrossRef] [PubMed]
- Monbaliu, J.-C.M.; Legros, J. Will the next generation of chemical plants be in miniaturized flow reactors? Lab A Chip 2023. Advance Article. [Google Scholar] [CrossRef] [PubMed]
- Atobe, M.; Tateno, H.; Matsumura, Y. Applications of Flow Microreactors in Electrosynthetic Processes. Chem. Rev. 2018, 118, 4541–4572. [Google Scholar] [CrossRef] [PubMed]
- Hardwick, T.; Ahmed, N. Advances in electro- and sono-microreactors for chemical synthesis. RSC Adv. 2018, 8, 22233–22249. [Google Scholar] [CrossRef]



| a | b |
 |  |
| c | d |
 |  |
| e | f |
 |  |
| Q1 = Q2 = 0.18 L/min | Q1 = Q2 = 0.5 L/min | Q1 = Q2 = 0.95 L/min | Q1 = Q2 = 1.6 L/min | Q1 = Q2 = 2.3 L/min | Q1 = Q2 = 2.7 L/min |
|---|---|---|---|---|---|
 |  |  |  |  |  |
| The upper chamber is filled by φ = 15% | The upper chamber is filled by φ = 29% | Complete filling of the upper chamber. The presence of bubbles | Complete filling of the upper chamber. The presence of bubbles only at the start of pumping | Complete filling of the upper chamber | Complete filling of the upper chamber |
| The lower chamber is filled by φ = 40% | The lower chamber is filled by φ = 72% | Complete filling of the lower chamber. A lot of bubbles in the lower chamber | Complete filling of the lower chamber. The presence of bubbles only at the start of pumping | Complete filling of the lower chamber | Complete filling of the lower chamber |
 there is a plug on the branch pipe, solutions are not supplied;
there is a plug on the branch pipe, solutions are not supplied;  solution is supplied to the branch pipe at a flow rate Q1;
solution is supplied to the branch pipe at a flow rate Q1;  solution is supplied to the branch pipe at a flow rate Q2.
solution is supplied to the branch pipe at a flow rate Q2.| System | Synthesis Conditions | X-ray Phase Analysis | SEM | Ref. |
|---|---|---|---|---|
| CaF2 | micro-VJA-ISF-CC sample 1 flow rates of both solutions are 2.1 L/min 1st flow [KF] = 0.7692 mole/L 2nd flow [Ca(NO3)2] = 0.3846 mole/L sample 2 flow rates of both solutions are 2.6 L/min 1st flow [KF] = 0.3846 mole/L 2nd flow [Ca(NO3)2] = 0.1923 mole/L sample 3 flow rates of both solutions are 3.2 L/min 1st flow [KF] = 0.1923 mole/L 2nd flow [Ca(NO3)2] = 0.0967 mole/L |  coherent scattering regions: sample 2: 46 nm sample 3: 37 nm | Samples 1–3 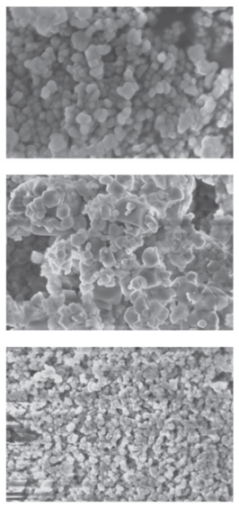 | [27] |
| SrF2 | micro-VJA-ISF-CC Case 1: 1st flow [KF] = 0.16 mole/L 2nd flow [Sr(NO3)2] = 0.08 mole/L Case 2: 1st flow [KF] = 0.3 mole/L 2nd flow [Sr(NO3)2] = 0.15 mole/L Case 3: 1st flow [KF] = 0.16 mole/L 2nd flow [Sr(NO3)2] = 0.08 mole/L (Er + Yb) In all cases, the flow rate of each flow was 2.5 L/min | 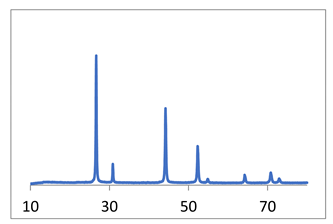 coherent scattering regions: Case 1: 35 nm Case 2: 55 nm Case 3: 17 nm |  | Not published |
| ZrO2 | 1. synthesis by means of coprecipitation 2. synthesis in micro-VJA-ISF-CC | 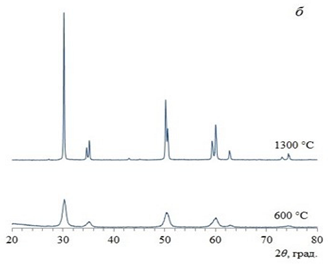 coherent scattering regions: 1. coprecipitation: 13–14 nm 2. micro-VJA-ISF-CC: 11 nm | 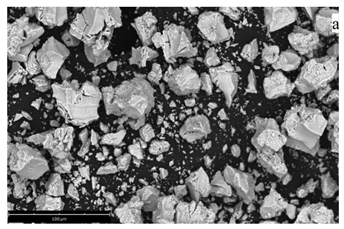 | [23] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abiev, R.S.; Kudryashova, Y.S.; Zdravkov, A.V.; Fedorenko, N.Y. Micromixing and Co-Precipitation in Continuous Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows. Inorganics 2023, 11, 49. https://doi.org/10.3390/inorganics11020049
Abiev RS, Kudryashova YS, Zdravkov AV, Fedorenko NY. Micromixing and Co-Precipitation in Continuous Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows. Inorganics. 2023; 11(2):49. https://doi.org/10.3390/inorganics11020049
Chicago/Turabian StyleAbiev, Rufat Sh., Yulia S. Kudryashova, Andrey V. Zdravkov, and Nadezhda Yu. Fedorenko. 2023. "Micromixing and Co-Precipitation in Continuous Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows" Inorganics 11, no. 2: 49. https://doi.org/10.3390/inorganics11020049
APA StyleAbiev, R. S., Kudryashova, Y. S., Zdravkov, A. V., & Fedorenko, N. Y. (2023). Micromixing and Co-Precipitation in Continuous Microreactors with Swirled Flows and Microreactors with Impinging Swirled Flows. Inorganics, 11(2), 49. https://doi.org/10.3390/inorganics11020049








