Dry Reforming of Methane over a Ruthenium/Carbon Nanotube Catalyst
Abstract
:1. Introduction
2. Materials and Methods
2.1. Carbon Nanotubes
2.2. Catalyst Testing System
3. Results
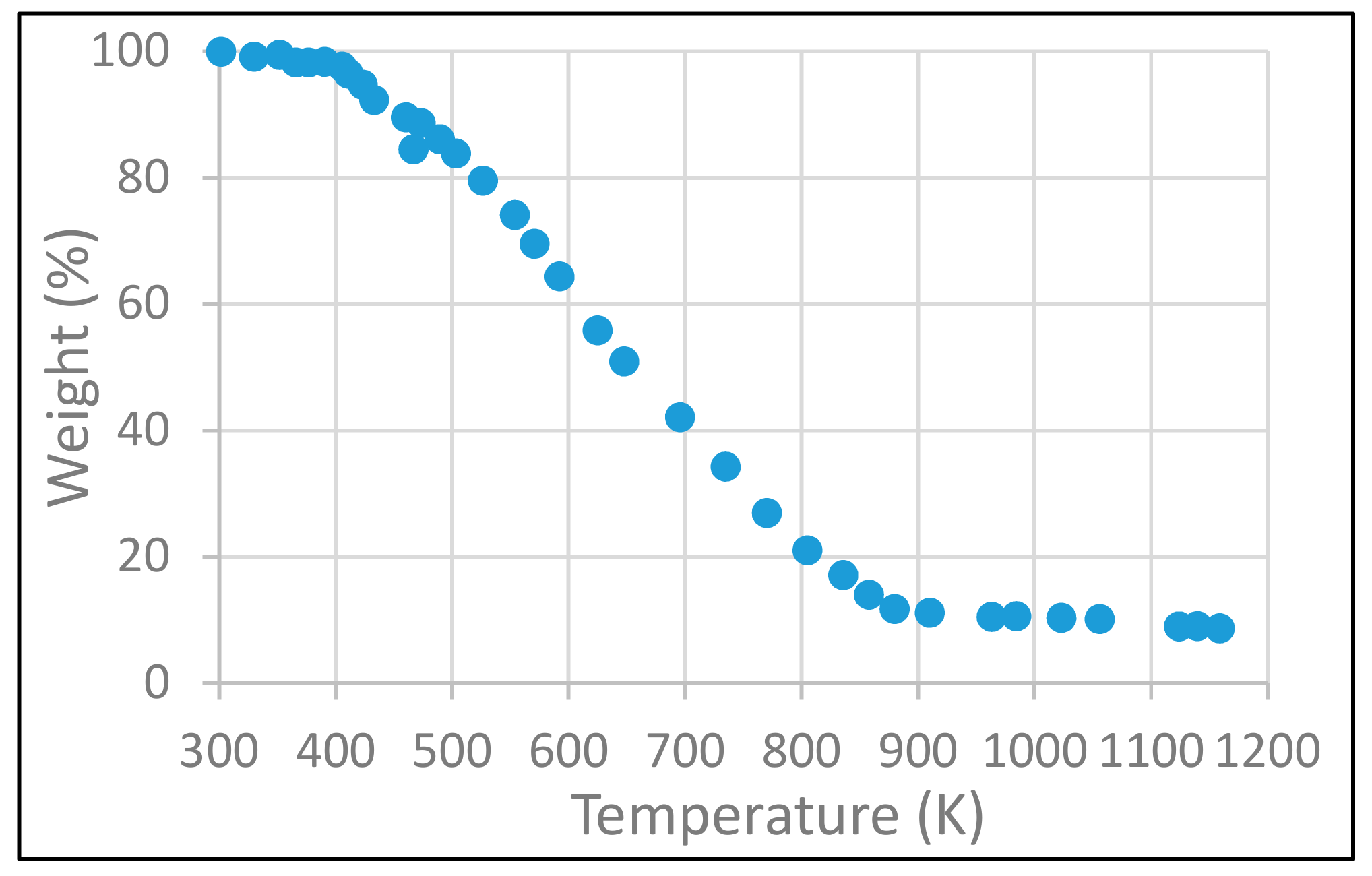
3.1. Catalyst Characterization
3.2. Modeling
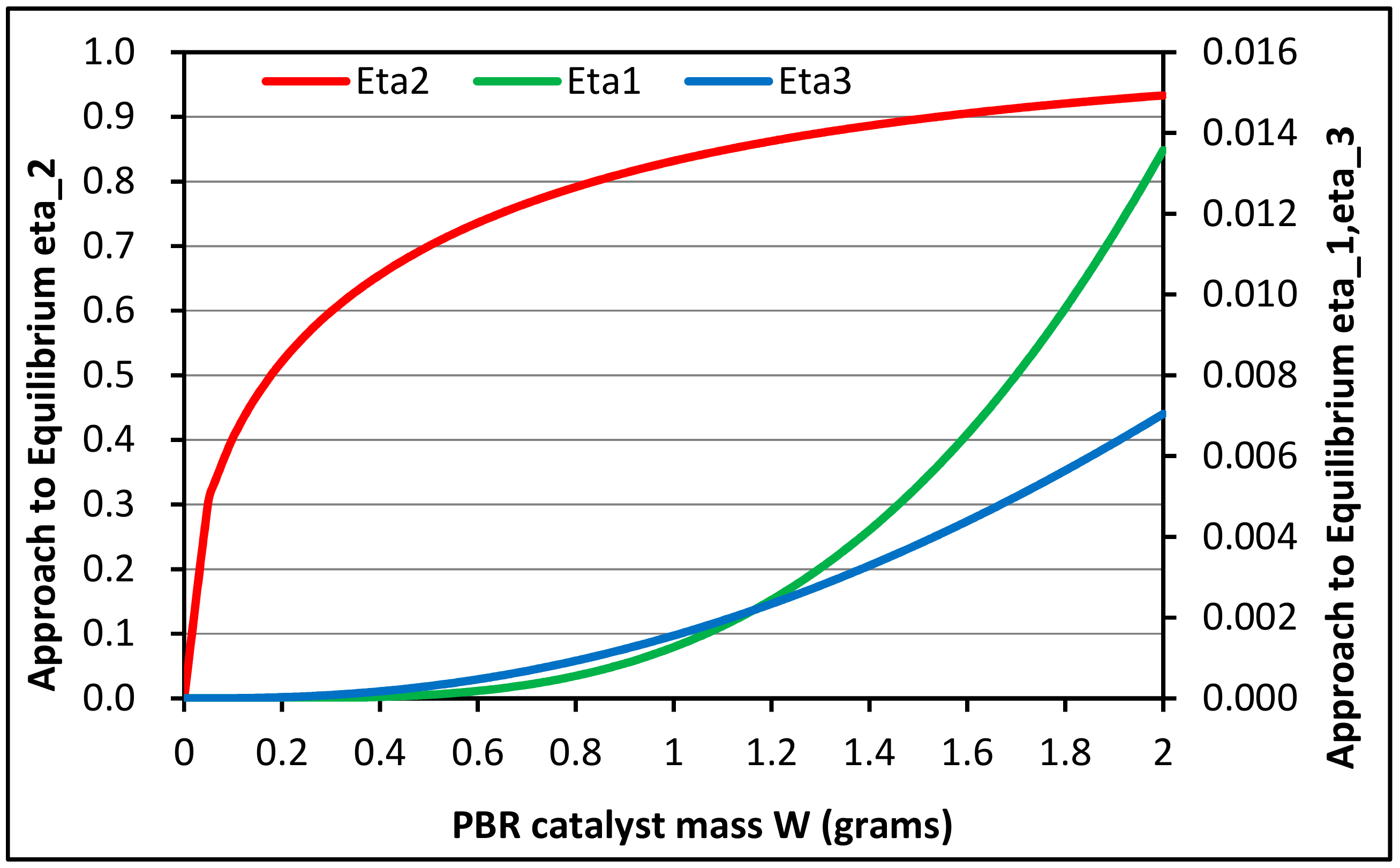
3.2.1. Equilibrium Calculations
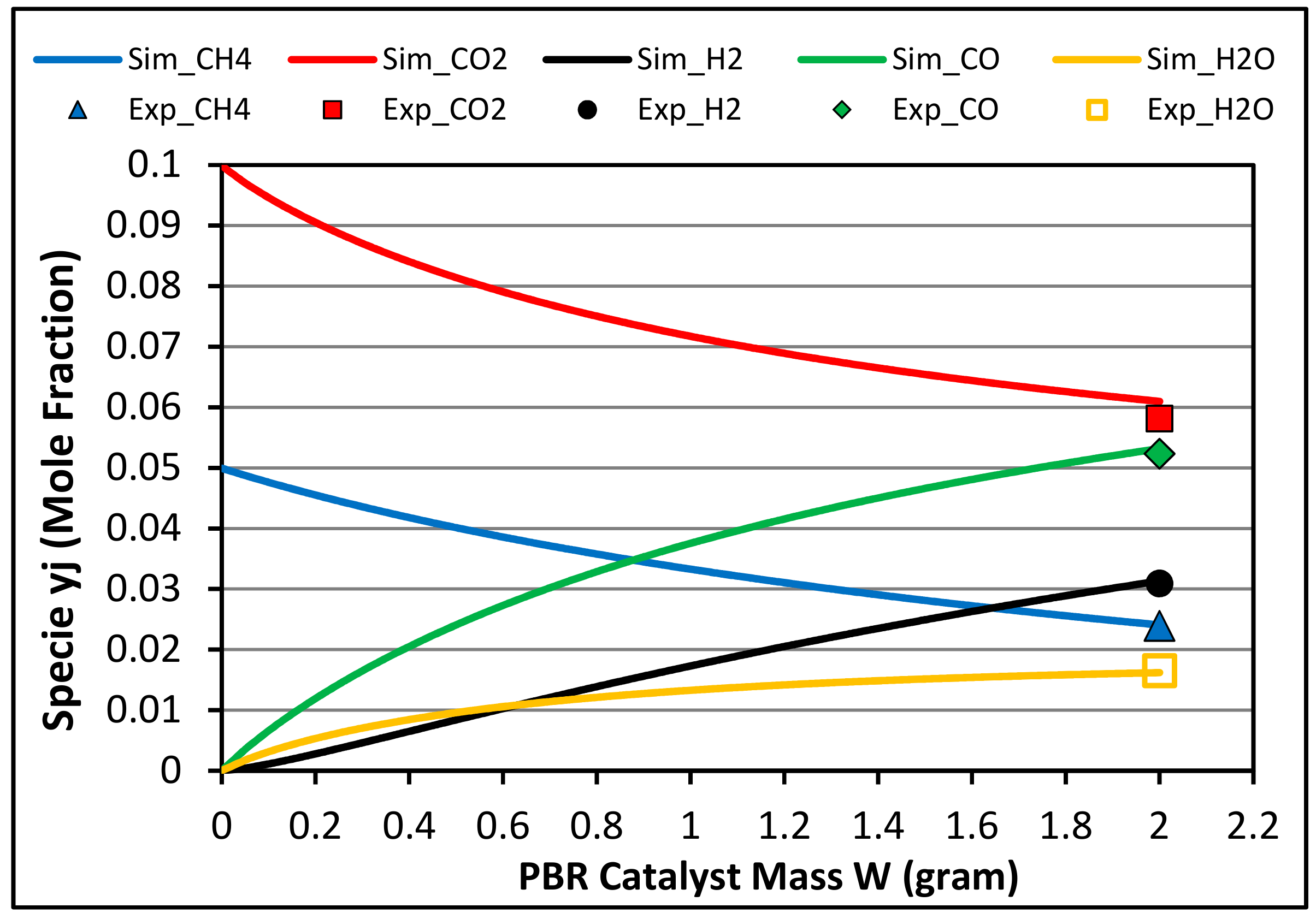
3.2.2. Global Kinetic Model
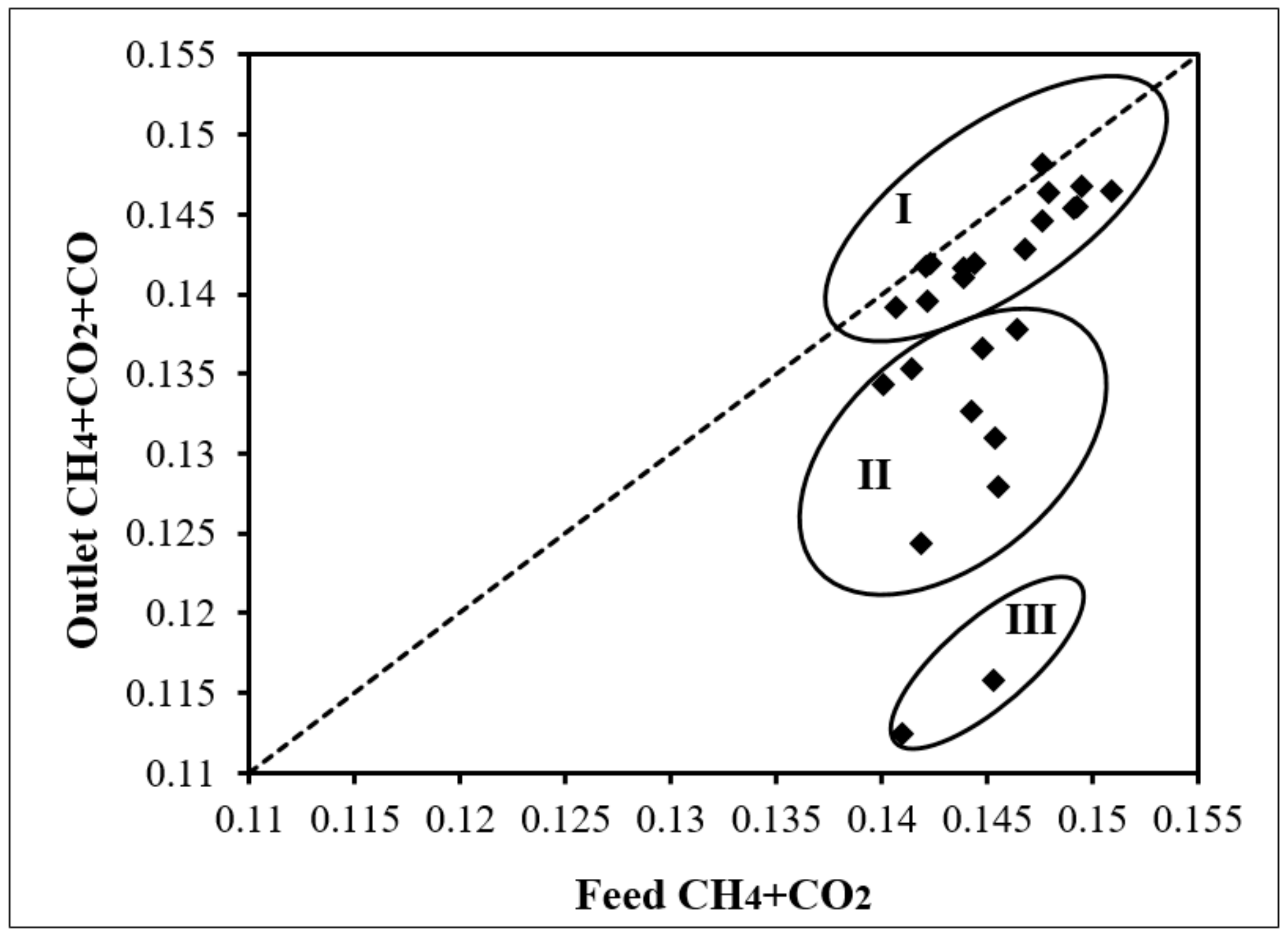
- Circle I shows the runs at 773, 823, and 873 K. These are very close or even at the parity line suggesting little carbon deposition at these lower temperatures.
- Circle II and III present the runs at 923 K and 973 K. Compared to circle I, higher temperature favors coke formation.
- Circle III shows two cases at 973 K. They are even further from the parity line than the other runs at 973K (Circle II, feed CH4/CO2 range 0.51–1.01) due to higher feed CH4/CO2 (1.53, 2.08). The same observation is obtained at the other temperatures—higher CH4/CO2 at comparable temperatures favors coke formation.
- For all runs, the further below the parity line, the higher H2/CO is observed. It is implied that the formation of higher H2 is coincident with coke formation.
4. Discussion
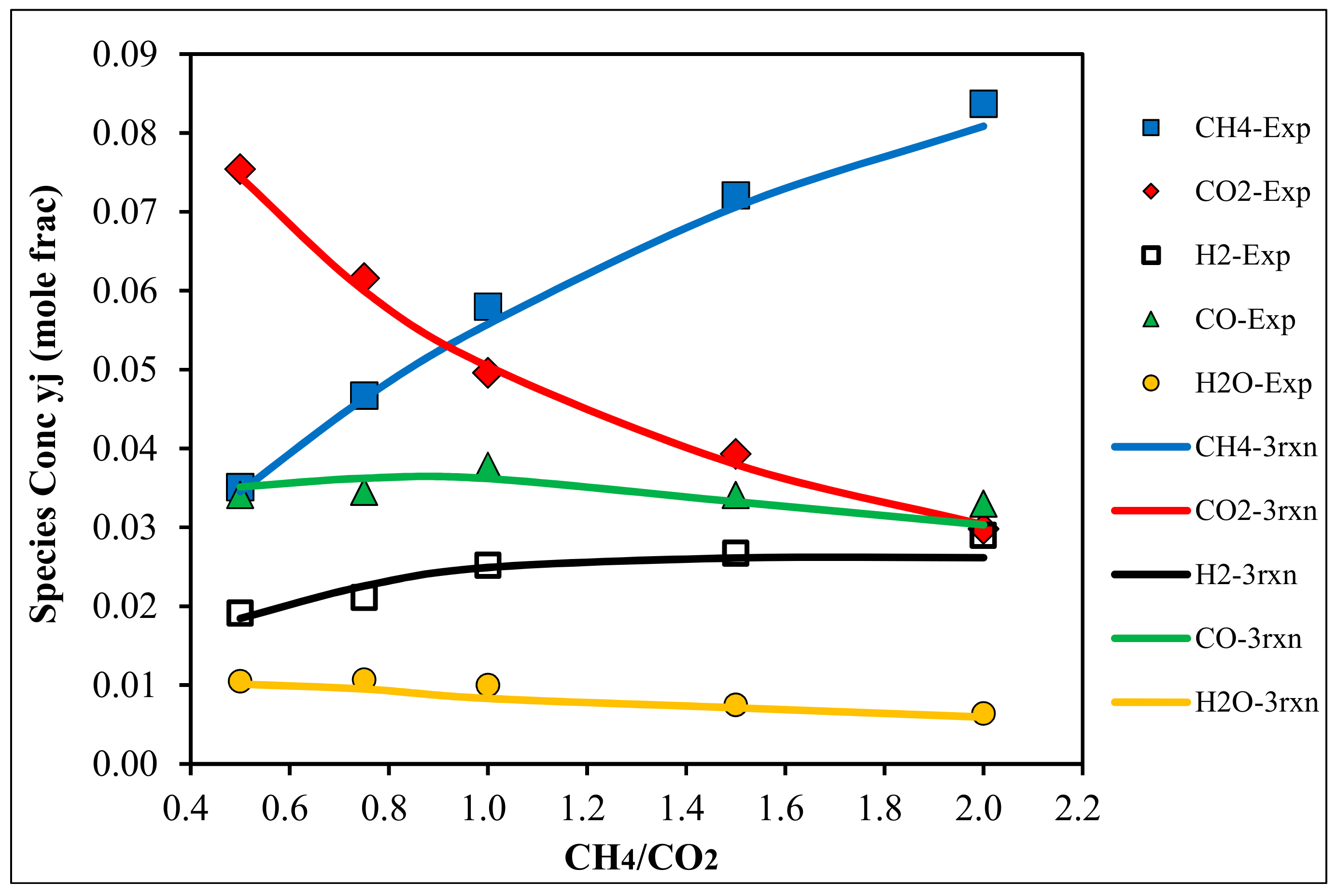
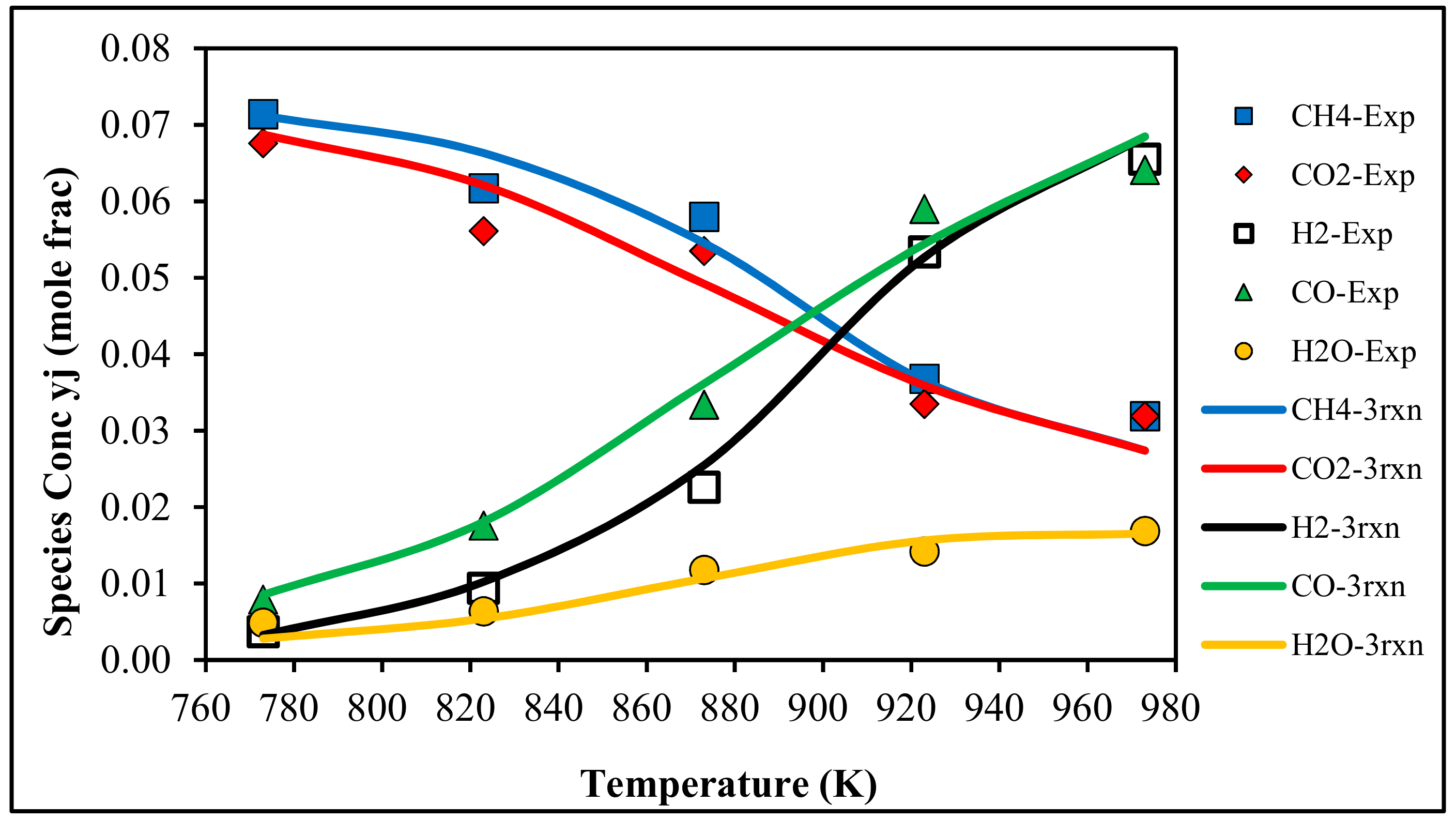
4.1. Species Concentrations at Reactor Outlet
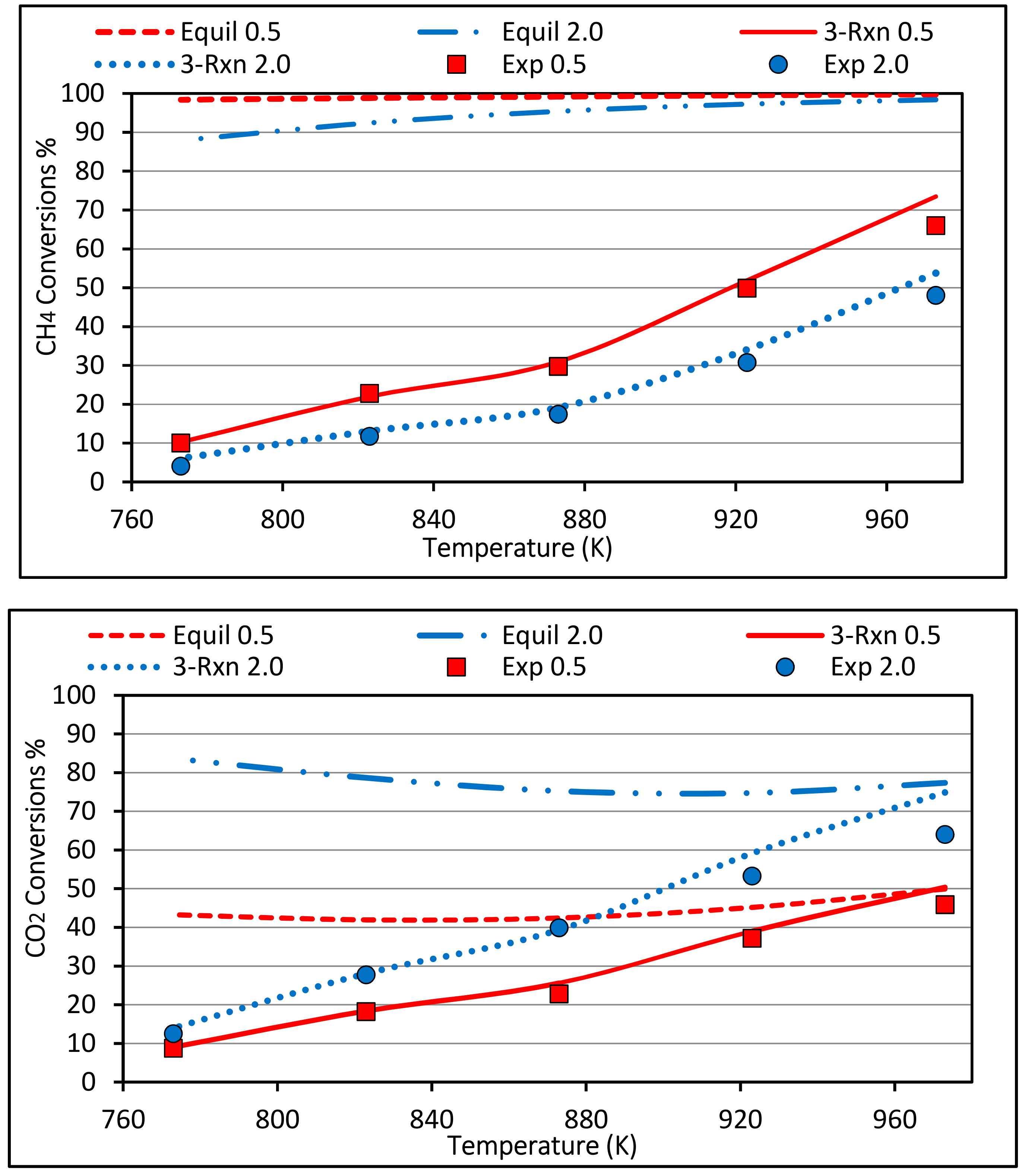
4.2. Methane and Carbon Dioxide Conversions
4.3. Syngas Molar Ratio H2/CO
4.4. Comparison of Ru/CNT to Pt_Pd/CNT
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kanellos, M. The Mind-Boggling Statistics around Wasted Natural Gas. Forbes. Available online: http://www.forbes.com/sites/michaelkanellos/2015/01/29/the-mind-boggling-statistics-around-wasted-natural-gas/#14587d1a7e18 (accessed on 29 January 2015).
- Nunez, C. Oil Drillers’ Burning of Natural Gas Costs U.S. Millions in Revenue. In National Geographic; National Geographic Partners: Washington, DC, USA, 2014. [Google Scholar]
- Yang, S.; Dezember, R. The U.S. is overflowing with Natural Gas. Not Everyone Can Get It. Wall Street Journal, 8 July 2019. [Google Scholar]
- Harder, A. EPA to Propose Rules Cutting Methane Emissions from Oil and Gas Drilling. Wall Street Journal, 17 August 2015. [Google Scholar]
- Elrod, M.J. Greenhouse Warming Potentials from the Infrared Spectroscopy of Atmospheric Gases. J. Chem. Educ. 1999, 76, 1702. [Google Scholar] [CrossRef]
- Usman, M.; Daud, W.W.; Abbas, H.F. Dry reforming of methane: Influence of process parameters—A review. Renew. Sustain. Energy Rev. 2015, 45, 710–744. [Google Scholar] [CrossRef] [Green Version]
- Ma, Q.; Wang, D.; Wu, M.; Zhao, T.; Yoneyama, Y.; Tsubaki, N. Effect of catalytic site position: Nickel nanocatalyst selectively loaded inside or outside carbon nanotubes for methane dry reforming. Fuel 2013, 108, 430–438. [Google Scholar] [CrossRef]
- Qu, Y.; Sutherland, A.M.; Guo, T. Carbon Dioxide Reforming of Methane by Ni/Co Nanoparticle Catalysts Immobilized on Single-Walled Carbon Nanotubes. Energy Fuels 2008, 22, 2183–2187. [Google Scholar] [CrossRef]
- Wu, T.; Zhang, Q.; Cai, W.; Zhang, P.; Song, X.; Sun, Z.; Gao, L. Phyllosilicate evolved hierarchical Ni- and Cu–Ni/SiO2 nanocomposites for methane dry reforming catalysis. Appl. Catal. A Gen. 2015, 503, 94–102. [Google Scholar] [CrossRef]
- Drif, A.; Bion, N.; Brahmi, R.; Ojala, S.; Pirault-Roy, L.; Turpeinen, E.; Seelam, P.K.; Keiski, R.L.; Epron, F. Study of the dry reforming of methane and ethanol using Rh catalysts supported on doped alumina. Appl. Catal. A Gen. 2015, 504, 576–584. [Google Scholar] [CrossRef]
- Hinkley, J.; Agrafiotis, C. Solar Thermal Energy and its Conversion to Solar Fuels via Thermochemical Processes. In Chapter 9, Polygeneration with Polystorage for Chemical and Energy Hubs for Energy and Chemicals; Khalilpour, K.R., Ed.; Academic Press: Cambridge, MA, USA, 2019; pp. 247–286. [Google Scholar]
- Park, J.-H.; Yeo, S.; Chang, T.S. Effect of supports on the performance of Co-based catalysts in methane dry reforming. J. CO2 Util. 2018, 26, 465–475. [Google Scholar] [CrossRef]
- Zhang, J.; Li, F. Coke-resistant Ni@SiO2 catalyst for dry reforming of methane. Appl. Catal. B Environ. 2015, 176, 513–521. [Google Scholar] [CrossRef]
- Yamagishi, T.; Furikado, I.; Ito, S.; Miyao, T.; Naito, S.; Tomishige, K.; Kunimori, K. Catalytic Performance and Characterization of RhVO4/SiO2 for Hydroformylation and CO Hydrogenation. J. Mol. Catal. A Chem. 2006, 244, 201–212. [Google Scholar] [CrossRef]
- Giehr, A.; Maier, L.; Schunk, S.A.; Deutschmann, O. Thermodynamic Considerations on the Oxidation State of Co/γ-Al2 O3 and Ni/γ-Al2 O3 Catalysts under Dry and Steam Reforming Conditions. ChemCatChem 2018, 10, 751–757. [Google Scholar] [CrossRef]
- Song, Y.; Ozdemir, E.; Ramesh, S.; Adishev, A.; Subramanian, S.; Harale, A.; AlBuali, M.; Fadhel, B.A.; Jamal, A.; Moon, D.; et al. Dry reforming of methane by stable Ni–Mo nanocatalysts on single-crystalline MgO. Science 2020, 367, 777–781. [Google Scholar] [CrossRef] [PubMed]
- Tomishige, K.; Asadullah, M.; Kunimori, K. Syngas Production by Biomass Gasification Using Rh/CeO2/SiO2 Catalysts and Fluidized Bed Reactor. Catal. Today 2004, 89, 389–403. [Google Scholar] [CrossRef]
- Iijima, S. Helical microtubules of graphitic carbon. Nature 1991, 354, 56–58. [Google Scholar] [CrossRef]
- Fu, X.; Yu, H.; Peng, F.; Wang, H.; Qian, Y. Facile Preparation of RuO2 /CNT Catalyst by a Homogeneous Oxidation Precipitation Method and Its Catalytic Performance. Appl. Catal. A Gen. 2007, 321, 190–197. [Google Scholar] [CrossRef]
- Donphai, W.; Faungnawakij, K.; Chareonpanich, M.; Limtrakul, J. Effect of Ni-CNTs/mesocellular silica composite catalysts on carbon dioxide reforming of methane. Appl. Catal. A Gen. 2014, 475, 16–26. [Google Scholar] [CrossRef]
- Khavarian, M.; Chai, S.-P.; Mohamed, A.R. The effects of process parameters on carbon dioxide reforming of methane over Co–Mo–MgO/MWCNTs nanocomposite catalysts. Fuel 2015, 158, 129–138. [Google Scholar] [CrossRef]
- Kim, J.-Y.; Kim, K.-H.; Park, S.-H.; Kim, K.-B. Microwave-polyol synthesis of nanocrystalline ruthenium oxide nanoparticles on carbon nanotubes for electrochemical capacitors. Electrochim. Acta 2010, 55, 8056–8061. [Google Scholar] [CrossRef]
- Zhu, Y. CO2 Reduction over Nobel Metal/Carbon Nanotube Catalyst. Ph.D. Thesis, Chemical Engineering Department, New Jersey Institute of Technology, Newark, NJ, USA, 2017. [Google Scholar]
- Zhu, Y.; Chen, K.; Yi, C.; Mitra, S.; Barat, R. Dry reforming of methane over palladium–platinum on carbon nanotube catalyst. Chem. Eng. Commun. 2018, 205, 888–896. [Google Scholar] [CrossRef]
- Chemkin-Pro Version 15131. Reaction Design, San Diego. Available online: http://www.ansys.com/products/fluids/ansys-chemkin-pro (accessed on 1 March 2017).
- Reynolds, W.C. The Element Potential Method for Chemical Equilibrium Analysis: Implementation in the Interactive Program STANJAN; Department of Mechanical Engineering, Stanford University: Stanford, CA, USA, 1986. [Google Scholar]
- Pakhare, D.; Spivey, J.J. A review of dry (CO2) reforming of methane over noble metal catalysts. Chem. Soc. Rev. 2014, 43, 7813–7837. [Google Scholar] [CrossRef] [PubMed]
- Wei, J.; Iglesia, E. Mechanism and Site Requirements for Activation and Chemical Conversion of Methane on Supported Pt Clusters and Turnover Rate Comparisons among Noble Metals. J. Phys. Chem. B 2004, 108, 4094–4103. [Google Scholar] [CrossRef]
- Bale, C.; Belisle, E. Reaction Web. Available online: http://www.crct.polymtl.ca/reacweb.htm (accessed on 1 June 2016).
- Foppa, L.; Silaghi, M.-C.; Larmier, K.; Comas-Vives, A. Intrinsic reactivity of Ni, Pd and Pt surfaces in dry reforming and competitive reactions: Insights from first principles calculations and microkinetic modeling simulations. J. Catal. 2016, 343, 196–207. [Google Scholar] [CrossRef]
- Zhu, Y.; Al-Ebbinni, N.; Henney, R.; Yi, C.; Barat, R. Extension to multiple temperatures of a three-reaction global kinetic model for methane dehydroaromatization. Chem. Eng. Sci. 2018, 177, 132–138. [Google Scholar] [CrossRef]
- Li, L.; Borry, R.W.; Iglesia, E. Design and optimization of catalysts and membrane reactors for the non-oxidative conversion of methane. Chem. Eng. Sci. 2002, 57, 4595–4604. [Google Scholar] [CrossRef]
- Karakaya, C.; Morejudo, S.H.; Zhu, H.; Kee, R.J. Catalytic Chemistry for Methane Dehydroaromatization (MDA) on a Bifunctional Mo/HZSM-5 Catalyst in a Packed Bed. Ind. Eng. Chem. Res. 2016, 55, 9895–9906. [Google Scholar] [CrossRef]
- Bartholomew, C.H.; Farrauto, R.J. Industrial Catalysis Processes; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Quiroga, M.M.B.; Luna, A.E.C. Kinetic Analysis of Rate Data for Dry Reforming of Methane. Ind. Eng. Chem. Res. 2007, 46, 5265–5270. [Google Scholar] [CrossRef]




| C | O | Fe | Ni | Ru | |
|---|---|---|---|---|---|
| Raw CNTs | 96.50 | 1.38 | 0.68 | 1.44 | - |
| Ru/CNTs | 83.97 | 7.28 | - | - | 8.75 |
| Species | Feed (Mole Fractions) | Equil (w/o Cs) (Mole Fracs) | Equil (w/Cs) (Mole Fractions) |
|---|---|---|---|
| CH4 | 0.0725 | 0.0227 | 0.0010 |
| CO2 | 0.0725 | 0.0160 | 0.0284 |
| CO | -- | 0.0930 | 0.0253 |
| H2 | -- | 0.0796 | 0.0802 |
| H2O | -- | 0.0067 | 0.0440 |
| He | 0.8550 | 0.7820 | 0.7497 |
| Cs | n/a | n/a | 0.0714 |
| SUM | 1.0000 | 1.0000 | 1.0000 |
| H2/CO | n/a | 0.856 | 3.17 |
| Reaction | Rate Expression ri | Appr. to Equil. ηi | Kpi |
|---|---|---|---|
| Dry Reforming CH4 + CO2 = 2CO + 2H2 | |||
| Reverse Water Gas Shift CO2 + H2 = CO + H2O | |||
| Methane Decomposition CH4 = Cs + 2H2 |
| PBR Balances Species j | Net Rates rj | Mole Fractions yj | Partial Pressures |
|---|---|---|---|
At W = 0, Fjo = value | Total molar rate includes inert gas | P = total pressure |
| Reaction i | Parameter Ai (mole, s, kg_cat, Pa) | Parameter Ei (J/mole) |
|---|---|---|
| 1 | 8.575 × 10−8 | 82,446 |
| 2 | 0.109 | 110,646 |
| 3 | 0.206 | 128,064 |
| XCH4 | XCO2 | YCO | YH2 | YCs | SCO | SH2 | SCs | H2/CO | |
|---|---|---|---|---|---|---|---|---|---|
| Ru/CNT | 0.084 | 0.099 | 0.180 | 0.076 | 0.0021 | 2.148 | 0.901 | 0.0255 | 0.838 |
| Pt_Pd/CNT | 0.075 | 0.091 | 0.164 | 0.066 | 0.0016 | 2.201 | 0.879 | 0.0213 | 0.798 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Chen, K.; Barat, R.; Mitra, S. Dry Reforming of Methane over a Ruthenium/Carbon Nanotube Catalyst. ChemEngineering 2020, 4, 16. https://doi.org/10.3390/chemengineering4010016
Zhu Y, Chen K, Barat R, Mitra S. Dry Reforming of Methane over a Ruthenium/Carbon Nanotube Catalyst. ChemEngineering. 2020; 4(1):16. https://doi.org/10.3390/chemengineering4010016
Chicago/Turabian StyleZhu, Yuan, Kun Chen, Robert Barat, and Somenath Mitra. 2020. "Dry Reforming of Methane over a Ruthenium/Carbon Nanotube Catalyst" ChemEngineering 4, no. 1: 16. https://doi.org/10.3390/chemengineering4010016
APA StyleZhu, Y., Chen, K., Barat, R., & Mitra, S. (2020). Dry Reforming of Methane over a Ruthenium/Carbon Nanotube Catalyst. ChemEngineering, 4(1), 16. https://doi.org/10.3390/chemengineering4010016






