Groundwater-Surface Water Interaction in the Nera River Basin (Central Italy): New Insights after the 2016 Seismic Sequence
Abstract
:1. Introduction
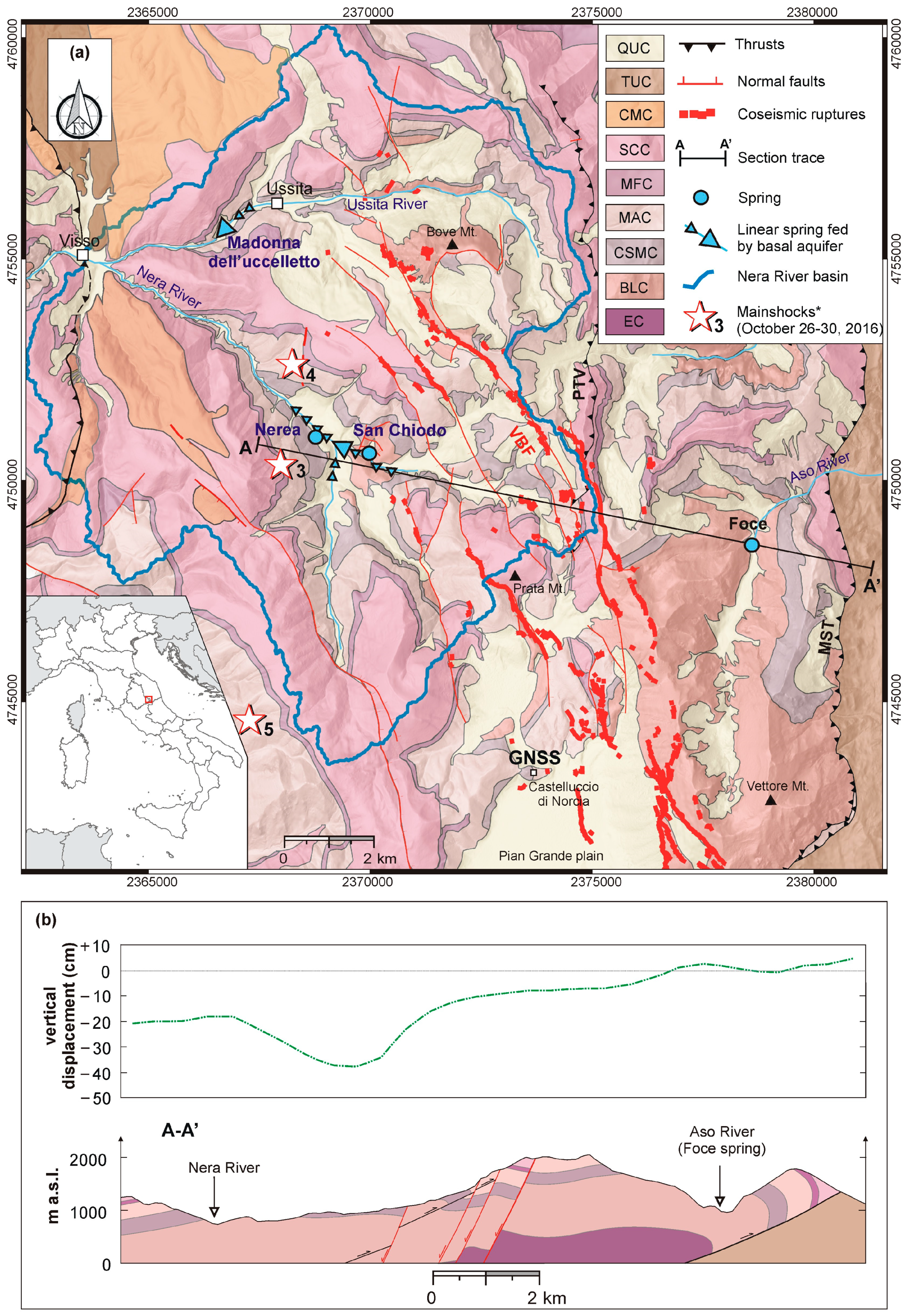
2. Study Area
2.1. Geological Structural and Hydrogeological Setting
2.2. Seismic Sequence and Co-Seismic Effects
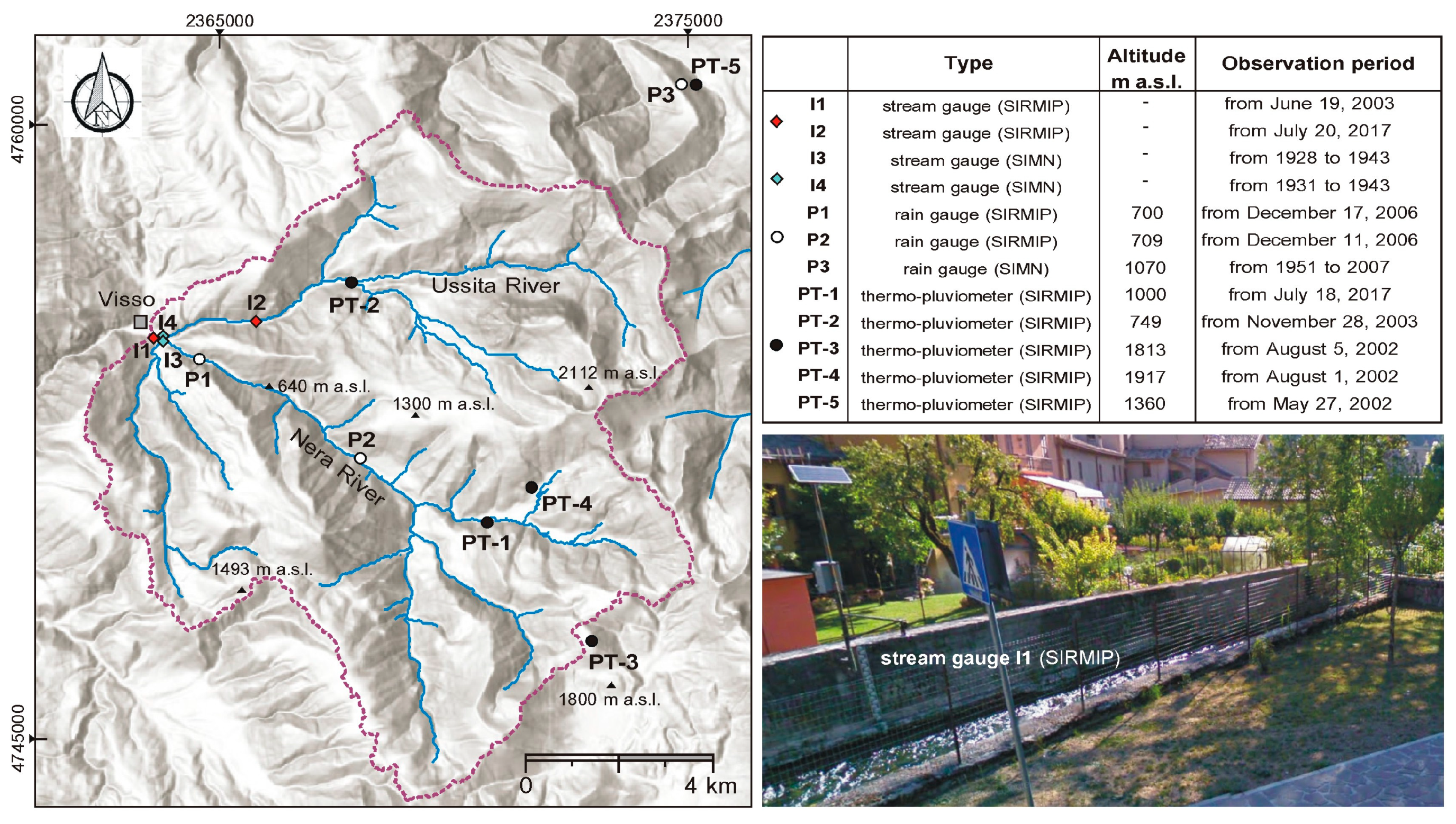
3. Materials and Methods
3.1. Meteorological Data and Techniques of Analysis
3.2. Discharge Data
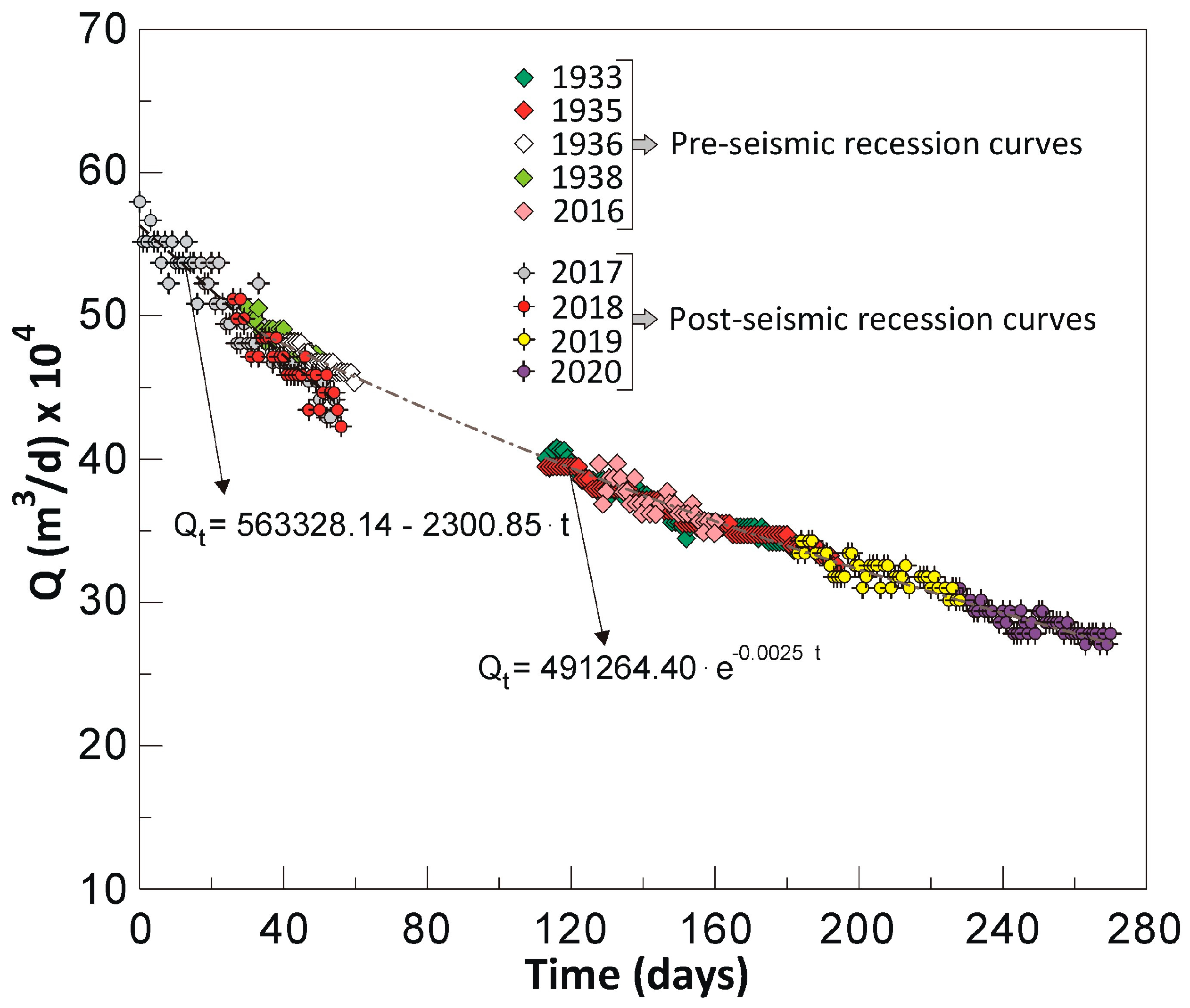
3.3. Models for River Recession Curves
4. Results
4.1. Rainfall-Water Surplus Analysis
4.2. River Hydrograph Analysis
5. Discussion
6. Conclusions
- −
- The peak river flow recorded in 2017 and 2018 did not occur in 2019 and 2020—the latter was preceded by a drought period well detected by the SPI, which affected the recharge of groundwater, and consequently the river discharge;
- −
- The rapid depletion observed during the 2017 and 2018 recession periods (straight line equation and turbulent flow) were not detected in 2019 and 2020, where both recession process and recession coefficients seem to be restored to the same as prior to the 2016 earthquake (exponential equation and Darcian flow);
- −
- Co-seismic effects on the hydrogeological system (e.g., increased aquifer permeability and pore water pressure) appear to have recovered after two years from the 2016 seismic sequence, as documented in other systems around the world affected by strong earthquakes.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Winter, T.C.; Harvey, J.W.; Franke, O.L.; Alley, W.M. Ground Water and Surface Water: A Single Resource; US Geological Survey: Denver, CO, USA, 1999; Volume 1139, pp. 1–88. Available online: https://pubs.usgs.gov/circ/circ1139 (accessed on 15 May 2021).
- Sophocleous, M. Interactions between groundwater and surface water: The state of the science. Hydrogeol. J. 2002, 10, 52–67. [Google Scholar] [CrossRef]
- Jolly, I.D.; McEwan, K.L.; Holland, K.L. A review of groundwater–surface water interactions in arid/semi-arid wetlands and the consequences of salinity for wetland ecology. Ecohydrology 2008, 1, 43–58. [Google Scholar] [CrossRef]
- Fleckenstein, J.H.; Krause, S.; Hannah, D.M.; Boano, F. Groundwater-surface water interactions: New methods and models to improve understanding of processes and dynamics. Adv. Water Res. 2010, 33, 1291–1295. [Google Scholar] [CrossRef]
- Li, M.; Liang, X.; Xiao, C.; Cao, Y. Quantitative Evaluation of Groundwater–Surface Water Interactions: Application of Cumulative Exchange Fluxes Method. Water 2020, 12, 259. [Google Scholar] [CrossRef] [Green Version]
- Guzman, P.; Batelaan, O.; Wyseure, G. Comparative analysis of base flow recession curves for different Andean catchments. Geophys. Res. Abs. 2012, 14, 8318. Available online: https://meetingorganizer.copernicus.org/EGU2012/EGU2012-8318.pdf (accessed on 18 May 2021).
- Berghuijs, W.; Woods, R.; Hrachowitz, M. A precipitation shift from snow towards rain leads to a decrease in streamflow. Nat. Clim. Chang. 2014, 4, 583–586. [Google Scholar] [CrossRef] [Green Version]
- Boni, C.; Bono, P.; Capelli, G. Schema idrogeologico dell’Italia Centrale. Mem. Soc. Geol. It. 1986, 35, 991–1012. (In Italian). Available online: https://www.idrogeologiaquantitativa.it/wordpress/wp-content/uploads/2009/11/Pubb_1986_Schema_Italia_Centrale.pdf (accessed on 18 May 2021).
- Cencetti, C.; Dragoni, W.; Nejad Massoum, M. Contributo alle conoscenze delle caratteristiche idrogeologiche del Fiume Nera (Appennino centro-settentrionale). Geol. Appl. Idrogeol. 1989, 24, 191–210. [Google Scholar]
- Preziosi, E.; Romano, E. From a hydrostructural analysis to the mathematical modelling of regional aquifers (Central Italy). It. J. Eng. Geol. Environ. 2009, 1, 183–198. [Google Scholar] [CrossRef]
- Boni, C.; Baldoni, T.; Banzato, F.; Cascone, D.; Petitta, M. Hydrogeological study for identification, characterization and management of groundwater resources in the Sibillini Mountains National Park (Central Italy). It. J. Eng. Geol. Environ. 2010, 2, 21–39. [Google Scholar] [CrossRef]
- Altava-Ortiz, V.; Llasat, M.C.; Ferrari, E.; Atencia, A.; Sirangelo, B. Monthly rainfall changes in Central and Western Mediterranean basins, at the end of the 20th and beginning of the 21st centuries. Int. J. Climatol. 2011, 31, 1943–1958. [Google Scholar] [CrossRef]
- Cambi, C.; Valigi, D.; Di Matteo, L. Hydrogeological study of data-scarce limestone massifs: The case of Gualdo Tadino and Monte Cucco structures (Central Apennines, Italy). Bollet. Geofisica Teorica Appl. 2010, 51, 345–360. [Google Scholar]
- Longobardi, A.; Villani, P. Trend analysis of annual and seasonal rainfall time series in the Mediterranean area. Int. J. Climatol. 2010, 30, 1538–1546. [Google Scholar] [CrossRef]
- Di Matteo, L.; Valigi, D.; Cambi, C. Climatic characterization and response of water resources to climate change in limestone areas: Some considerations on the importance of geological setting. J. Hydrol. Eng. 2013, 18, 773–779. [Google Scholar] [CrossRef] [Green Version]
- Diodato, N.; Büntgen, U.; Bellocchi, G. Mediterranean winter snowfall variability over the past millennium. Int. J. Climatol. 2019, 39, 384–394. [Google Scholar] [CrossRef] [Green Version]
- Gentilucci, M.; Barbieri, M.; Lee, H.S.; Zardi, D. Analysis of rainfall trends and extreme precipitation in the Middle Adriatic Side, Marche Region (Central Italy). Water 2019, 11, 1948. [Google Scholar] [CrossRef] [Green Version]
- Caporali, E.; Lompi, M.; Pacetti, T.; Chiarello, V.; Fatichi, S. A review of studies on observed precipitation trends in Italy. Int. J. Climatol. 2021, 41, E1–E25. [Google Scholar] [CrossRef]
- Caloiero, T.; Caroletti, G.N.; Coscarelli, R. IMERG-Based Meteorological Drought Analysis over Italy. Climate 2021, 9, 65. [Google Scholar] [CrossRef]
- Valigi, D.; Luque Espinar, J.A.; Di Matteo, L.; Cambi, C.; Pardo Iguzquiza, E.; Rossi, M. Analysis of drought conditions and their effects on Lake Trasimeno (Central Italy) levels. It. J. Groundwater 2016, 17–215, 39–47. [Google Scholar] [CrossRef]
- Diodato, N.; Bellocchi, G. Climate control on snowfall days in peninsular Italy. Theor. Appl. Climatol. 2020, 140, 951–961. [Google Scholar] [CrossRef]
- Petitta, M.; Mastrorillo, L.; Preziosi, E.; Banzato, F.; Barberio, M.D.; Billi, A.; Cambi, C.; De Luca, G.; Di Carlo, G.; Di Curzio, D.; et al. Water table and discharge changes associated with the 2016–2017 seismic sequence in central Italy: Hydrogeological data and a conceptual model for fractured carbonate aquifers. Hydrogeol. J. 2018, 26, 1009–1026. [Google Scholar] [CrossRef] [Green Version]
- Mastrorillo, L.; Saroli, M.; Viaroli, S.; Banzato, F.; Valigi, D.; Petitta, M. Sustained post-seismic effects on groundwater flow in fractured carbonate aquifers in Central Italy. Hydrol. Proc. 2020, 34, 1167–1181. [Google Scholar] [CrossRef] [Green Version]
- Di Matteo, L.; Dragoni, W.; Azzaro, S.; Pauselli, C.; Porreca, M.; Bellina, G.; Cardaci, W. Effects of earthquakes on the discharge of groundwater systems: The case of the 2016 seismic sequence in the Central Apennines, Italy. J. Hydrol. 2020, 583, 124509. [Google Scholar] [CrossRef]
- Valigi, D.; Fronzi, D.; Cambi, C.; Beddini, G.; Cardellini, C.; Checcucci, R.; Mastrorillo, L.; Mirabella, F.; Tazioli, A. Earthquake-induced spring discharge modifications: The Pescara di Arquata spring reaction to the august–october 2016 Central Italy earthquakes. Water 2020, 12, 767. [Google Scholar] [CrossRef] [Green Version]
- Fronzi, D.; Di Curzio, D.; Rusi, S.; Valigi, D.; Tazioli, A. Comparison between Periodic Tracer Tests and Time-Series Analysis to Assess Mid-and Long-Term Recharge Model Changes Due to Multiple Strong Seismic Events in Carbonate Aquifers. Water 2020, 12, 3073. [Google Scholar] [CrossRef]
- Manga, M.; Rowland, J.C. Response of Alum Rock springs to the October 30, 2007 earthquake and implications for the origin of increased discharge after earthquakes. Geofluids 2009, 9, 237–250. [Google Scholar] [CrossRef]
- Geballe, Z.M.; Wang, C.-Y.; Manga, M. A permeability-change model for water level changes triggered by teleseismic waves. Geofluids 2011, 11, 302–308. [Google Scholar] [CrossRef]
- Rojstaczer, S.; Wolf, S.; Michel, R. Permeability enhancement in the shallow crust as a cause of earthquake induced hydrological changes. Nature 1995, 373, 237–239. [Google Scholar] [CrossRef]
- Wang, C.Y.; Manga, M. New streams and springs after the 2014 Mw 6.0 South Napa earthquake. Nat. Commun. 2015, 6, 7597. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Binda, G.; Pozzi, A.; Michetti, A.M.; Noble, P.J.; Rosen, M.R. Towards the understanding of hydrogeochemical seismic responses in karst aquifers: A retrospective meta-analysis focused on the Apennines (Italy). Minerals 2020, 10, 1058. [Google Scholar] [CrossRef]
- Pierantoni, P.; Deiana, G.; Galdenzi, S. Stratigraphic and structural features of the Sibillini mountains (Umbria-Marche Apennines, Italy). It. J. Geosci. 2013, 132, 497–520. [Google Scholar] [CrossRef]
- Boscherini, A.; Checcucci, R.; Natale, G.; Natali, N. Carta Idrogeologica della Regione Umbria (scala 1:100.000). Giornale Geol. Appl. 2005, 2, 399–404. [Google Scholar] [CrossRef]
- Civita, M. Idrogeologia Applicata e Ambientale; Casa editrice ambrosiana: Milano, Italy, 2005; p. 800. (In Italian) [Google Scholar]
- Viaroli, S.; Mirabella, F.; Mastrorillo, L.; Angelini, S.; Valigi, D. Fractured carbonate aquifers of Sibillini Mts. (Central Italy). J. Maps 2021, 17, 140–149. [Google Scholar] [CrossRef]
- Valigi, D.; Cambi, C.; Checcucci, R.; Di Matteo, L. Transmissivity Estimates by Specific Capacity Data of Some Fractured Italian Carbonate Aquifers. Water 2021, 13, 1374. [Google Scholar] [CrossRef]
- Mazzoli, S.; Pierantoni, P.P.; Borraccini, F.; Paltrinieri, W.; Deiana, G. Geometry, segmentation pattern and displacement variations along a major Apennine thrust zone, central Italy. J. Struct. Geol. 2005, 27, 1940–1953. [Google Scholar] [CrossRef]
- Porreca, M.; Minelli, G.; Ercoli, M.; Brobia, A.; Mancinelli, P.; Cruciani, F.; Giorgetti, C.; Carboni, F.; Mirabella, F.; Cavinato, G.; et al. Seismic reflection profiles and subsurface geology of the area interested by the 2016–2017 earthquake sequence (Central Italy). Tectonics 2018, 37, 1116–1137. [Google Scholar] [CrossRef]
- Koopman, A. Detachment Tectonics in the Central Apennines, Italy. Ph.D. Thesis, Instituut voor Aardwetenschappen RUU, 1983. Available online: http://dspace.library.uu.nl/bitstream/handle/1874/216947/Koopman-Anton-30-1983.pdf?sequence=1&isAllowed=y (accessed on 31 May 2021).
- Centamore, E.; Adamoli, L.; Berti, D.; Bigi, G.; Bigi, S.; Casnedi, R.; Cantalamessa, G.; Fumanti, F.; Morelli, C.; Micarelli, A.; et al. Carta geologica dei bacini della Laga e del Cellino e dei rilievi carbonatici circostanti (Marche meridionali, Lazio nordorientale, Abruzzo settentrionale). Stud. Geol. Camert. 1992, 2, Tavola 1. Available online: http://193.204.8.201:8080/jspui/bitstream/1336/782/1/Vol.%2091-2%20Cap.%2018%20Allegato%202.pdf (accessed on 31 May 2021).
- Lavecchia, G.; Brozzetti, F.; Barchi, M.; Menichetti, M.; Keller, J.V. Seismotectonic zoning in east-central Italy deduced from an analysis of the Neogene to present deformations and related stress fields. GSA Bullet. 1994, 106, 1107–1120. [Google Scholar] [CrossRef]
- Porreca, M.; Fabbrizzi, A.; Azzaro, S.; Pucci, S.; Del Rio, L.; Pierantoni, P.P.; Giorgetti, C.; Roberts, G.P.; Barchi, M.R. 3D geological reconstruction of the M. Vettore seismogenic fault system (Central Apennines, Italy): Cross-cutting relationship with the M. Sibillini thrust. J. Struct. Geol. 2020, 131, 103938. [Google Scholar] [CrossRef]
- Tarragoni, C. Determinazione della “quota isotopica” del bacino di alimentazione delle principali sorgenti dell’alta Valnerina. Geol. Romana 2006, 39, 55–62. [Google Scholar]
- De Guidi, G.; Vecchio, A.; Brighenti, F.; Caputo, R.; Carnemolla, F.; Di Pietro, A.; Lupo, M.; Maggini, M.; Marchese, S.; Messina, D.; et al. Brief communication: Co-seismic displacement on 26 and 30 October 2016 (Mw = 5.9 and 6.5)–earthquakes in central Italy from the analysis of a local GNSS network. Nat. Hazards Earth Syst. Sci. 2017, 17, 1885–1892. [Google Scholar] [CrossRef] [Green Version]
- Valerio, E.; Tizzani, P.; Carminati, E.; Doglioni, C.; Pepe, S.; Petricca, P.; De Luca, C.; Bignami, C.; Solaro, G.; Castaldo, R.; et al. Ground Deformation and Source Geometry of the 30 October 2016 Mw 6.5 Norcia Earthquake (Central Italy) Investigated Through Seismological Data, DInSAR Measurements, and Numerical Modelling. Remote Sens. 2018, 10, 1901. [Google Scholar] [CrossRef] [Green Version]
- EMERGEO Working Group. Coseismic effects of the 2016 Amatrice seismic sequence: First geological results. Ann. Geophys. 2016, 59, Fast Track 5. [Google Scholar] [CrossRef]
- Aringoli, D.; Farabollini, P.; Giacopetti, M.; Materazzi, M.; Paggi, S.; Pambianchi, G.; Pierantoni, P.P.; Pistolesi, E.; Pitts, A.; Tondi, E. The August 24th 2016 Accumoli earthquake: Surface faulting and Deep-Seated Gravitational Slope Deformation (DSGSD) in the Monte Vettore area. Ann. Geophys. 2016, 59, Fast Track 5. [Google Scholar] [CrossRef]
- Lavecchia, G.; Castaldo, R.; de Nardis, R.; De Novellis, V.; Ferrarini, F.; Pepe, S.; Brozzetti, F.; Solaro, G.; Cirillo, D.; Bonano, M.; et al. Ground deformation and source geometry of the 24 August 2016 Amatrice earthquake (Central Italy) investigated through analytical and numerical modeling of DInSAR measurements and structural-geological data. Geophys. Res. Lett. 2016, 43, 389–398. [Google Scholar] [CrossRef]
- Livio, F.; Michetti, A.M.; Vittori, E.; Gregory, L.; Wedmore, L.; Piccardi, L.; Tondi, E.; Roberts, G.; and Central Italy Earthquake Working Grooup. Surface faulting during the August 24, 2016, Central Italy earthquake (Mw 6.0): Preliminary results. Ann. Geophys. 2016, 59, Fast Track 5. [Google Scholar] [CrossRef]
- Galli, P.; Galadini, F.; Pantosti, D. Twenty years of paleoseismology in Italy. Earth Sci. Rev. 2008, 88, 89–117. [Google Scholar] [CrossRef]
- Pucci, S.; De Martini, P.M.; Civico, R.; Villani, F.; Nappi, R.; Ricci, T.; Azzaro, R.; Brunori, C.A.; Caciagli, M.; Cinti, F.R.; et al. Coseismic ruptures of the24 August 2016, Mw 6.0 Amatrice earthquake (central Italy). Geophys. Res. Lett. 2017, 44. [Google Scholar] [CrossRef]
- Civico, R.; Pucci, S.; Villani, F.; Pizzimenti, L.; De Martini, P.M.; Nappi, R.; Wedmore, L. Surface ruptures following the 30 October 2016 Mw 6.5 Norcia earthquake, central Italy. J. Maps 2018, 14, 151–160. [Google Scholar] [CrossRef] [Green Version]
- Villani, F.; Pucci, S.; Civico, R.; De Martini, P.M.; Cinti, F.R.; Pantosti, D. Surface faulting of the 30 October 2016 Mw 6.5 central Italy earthquake: Detailed analysis of a complex coseismic rupture. Tectonics 2018, 37, 3378–3410. [Google Scholar] [CrossRef]
- Michele, M.; Chiaraluce, L.; Di Stefano, R.; Waldhauser, F. Fine-Scale Structure of the 2016–2017 Central Italy Seismic Sequence From Data Recorded at the Italian National Network. J. Geophys. Res. Solid Earth 2020, 125, e2019JB018440. [Google Scholar] [CrossRef]
- Barchi, M.R.; Carboni, F.; Michele, M.; Ercoli, M.; Giorgetti, C.; Porreca, M.; Azzaro, S.; Chiaraluce, L. The influence of subsurface geology on the distribution of earthquakes during the 2016-–2017 Central Italy seismic sequence. Tectonophysics 2021, 807, 228797. [Google Scholar] [CrossRef]
- Chiaraluce, L.; Di Stefano, R.; Tinti, E.; Scognamiglio, L.; Michele, M.; Casarotti, E.; Cattaneo, M.; De Gori, P.; Chiarabba, C.; Monachesi, G.; et al. The 2016 central Italy seismic sequence: A first look at the mainshocks, aftershocks, and source models. Seismol. Res. Lett. 2017, 88, 757–771. [Google Scholar] [CrossRef]
- Searcy, J.K.; Hardison, C.H. Double-Mass Curves; US Government Printing Office: Washington, DC, USA, 1960; Volume 1541, p. 66.
- Vernimmen, R.R.E.; Hooijer, A.; Aldrian, E.; Van Dijk, A.I.J.M. Evaluation and bias correction of satellite rainfall data for drought monitoring in Indonesia. Hydrol. Earth Syst. Sci. 2012, 16, 133–146. [Google Scholar] [CrossRef] [Green Version]
- Di Matteo, L.; Dragoni, W.; Maccari, D.; Piacentini, S.M. Climate change, water supply and environmental problems of headwaters: The paradigmatic case of the Tiber, Savio and Marecchia rivers (Central Italy). Sci. Total Environ. 2017, 598, 733–748. [Google Scholar] [CrossRef] [PubMed]
- Navarro, A.; García-Ortega, E.; Merino, A.; Sánchez, J.L.; Kummerow, C.; Tapiador, F.J. Assessment of IMERG precipitation estimates over Europe. Remote Sens. 2019, 11, 2470. [Google Scholar] [CrossRef] [Green Version]
- Saouabe, T.; El Khalki, E.M.; Saidi, M.E.M.; Najmi, A.; Hadri, A.; Rachidi, S.; Jadoud, M.; Tramblay, Y. Evaluation of the GPM-IMERG precipitation product for flood modeling in a semi-arid mountainous basin in Morocco. Water 2020, 12, 2516. [Google Scholar] [CrossRef]
- McKee, T.B.; Doesken, N.J.; Kleist, J. The relationship of drought frequency and duration to time scales. In Proceedings of the 8th Conference on Applied Climatology, Anaheim, CA, USA, 17–22 January 1993; Volume 17, pp. 179–183. [Google Scholar]
- WMO-World Meteorological Organization. Standardized precipitation index user guide. M.; Svoboda, M. Hayes and D. Wood. (WMO-No. 1090), Geneva. Available online: www.wamis.org/agm/pubs/SPI/WMO_1090_EN.pdf (accessed on 25 May 2021).
- Thornthwaite, C.W.; Mather, J.R. The water balance. Centerton: Drexel institute of technology, laboratory of climatology. Publ. Climatol. 1955, 8, 104. [Google Scholar]
- Shuttleworth, W.J. Putting the “vap” into evaporation. Hydrol. Earth Syst. Sci. 2007, 11, 210–244. [Google Scholar] [CrossRef] [Green Version]
- Čadro, S. Excel sheet for Potential Evapotranspiration (PET) and Soil Water Balance Calculation Based on Thornthwaite Method (1948). Available online: https://www.researchgate.net/profile/Sabrija_Cadro/publication/309740661_Thornthwaite_Potential_Evapotranspiration_PET_and_Water_Balance_1948/data/582187e808aeccc08af8d4eb/Thornthwaite-Evapotranspiration-PET-and-Water-Balance-1948.xlsx (accessed on 25 May 2021).
- D.R. 35/2018. Fiume Nera–Derivazione Dalle Opere di Captazione Presso la Sorgente San Chiodo. AATO 3 Marche Centro–Società Acquedotto del Nera (MC). Marche Region Law with Subsequent Modifications and Additions. Available online: http://www.ato3marche.it/assemblea-di-ambito/atti-e-documenti-assemblea-di-ambito/decreti-del-presidente/2018-2/1455-decreto-del-presidente-n-12-2018-del-08-06-2018/file (accessed on 25 May 2021).
- Boussinesq, J. Essai sur la théorie des eaux courantes du movement non permanent des eaux souterraines. Acad. Sci. Inst. Fr. 1877, 23, 252–260. [Google Scholar]
- Maillet, E. Essais D’hydraulique Souterraine et Fluviale; Librairie Sci.: Paris, France, 1905; p. 218. [Google Scholar]
- Scanlon, B.R.; Mace, R.E.; Barrett, M.E.; Smith, B. Can we simulate regional groundwater flow in a karst system using equivalent media models? Case study Barton springs Edwards Aquifer, USA. J. Hydrol. 2003, 276, 137–158. [Google Scholar] [CrossRef]
- Rehrl, C.; Birk, S. Hydrogeological characterisation and modelling of spring catchments in a changing environment. Aust. J. Earth Sci. 2010, 103, 106–117. [Google Scholar] [CrossRef] [Green Version]
- Dragoni, W.; Mottola, A.; Cambi, C. Modeling the effects of pumping wells in spring management: The case of Scirca spring (Central Apennines, Italy). J. Hydrol. 2013, 493, 115–123. [Google Scholar] [CrossRef]
- Coutagne, A. Les variations de dèbit en pèriode non influencèe par les prècipitations. In Le dèbit d’Inflitration (Corrèlations Fluviales Internes)–2me Partie, Meteorologie et Hydrologie; La Houille Blanche: Grenoble, France, 1948; pp. 416–436. [Google Scholar]
- Bonacci, O. Karst Hydrology With Special Reference to the Dinaric Karst; Springer: Heidelberg, Germany, 1987; p. 184. [Google Scholar]
- Rorabough, M.I. Estimating changes in bank storage and grounwater contribution to streamflow. Int. Assoc. Sci. Hydro. Publ. 1964, 63, 432–441. [Google Scholar]
- Kovács, A.; Perrochet, P.A. quantitative approach to spring hydrograph decomposition. J. Hydrol. 2008, 352, 16–29. [Google Scholar] [CrossRef]
- Barberio, M.D.; Barbieri, M.; Billi, A.; Doglioni, C.; Petitta, M. Hydrogeochemical changes before and during the 2016 Amatrice-Norcia seismic sequence (central Italy). Sci. Rep. 2017, 7, 11735. [Google Scholar] [CrossRef]
- Rosen, M.R.; Binda, G.; Archer, C.; Pozzi, A.; Michetti, A.M.; Noble, P.J. Mechanisms of earthquake-induced chemical and fluid transport to carbonate groundwater springs after earthquakes. Water Res. Res. 2018, 54, 5225–5244. [Google Scholar] [CrossRef]
- Fronzi, D.; Mirabella, F.; Cardellini, C.; Caliro, S.; Palpacelli, S.; Cambi, C.; Valigi, D.; Tazioli, A. The Role of Faults in Groundwater Circulation before and after Seismic Events: Insights from Tracers, Water Isotopes and Geochemistry. Water 2021, 13, 1499. [Google Scholar] [CrossRef]
- Singh, V.P. Hydrologic Systems: Watershed Modeling; Prentice-Hall: Denver, CO, USA, 1989; p. 448. [Google Scholar]
- Tallaksen, L.M. A review of baseflow recession analysis. J. Hydrol. 1995, 165, 349–370. [Google Scholar] [CrossRef]
- Posavec, K.; Parlov, J.; Nakić, Z. Fully automated objective-based method for master recession curve separation. Groundwater 2010, 48, 598–603. [Google Scholar] [CrossRef]
- Elkhoury, J.E.; Brodsky, E.E.; Agnew, D.C. Seismic waves increase permeability. Nature 2006, 441, 1135–1138. [Google Scholar] [CrossRef] [PubMed]
- Manga, M.; Beresnev, I.; Brodsky, E.E.; Elkhoury, J.E.; Elsworth, D.; Ingebritsen, S.E.; Mays, D.C.; Wang, C.Y. Changes in permeability caused by transient stresses: Field observations, experiments, and mechanisms. Rev. Geophys. 2012, 50, 1–24. [Google Scholar] [CrossRef]
- Aben, F.M.; Doan, M.L.; Gratier, J.P.; Renard, F. Experimental postseismic recovery of fractured rocks assisted by calcite sealing. Geophys. Res. Lett. 2017, 44, 7228–7238. [Google Scholar] [CrossRef] [Green Version]




| N° | Localization | Data | Mw | Hypocentral Depth (km) |
|---|---|---|---|---|
| 1 | Accumoli (42.70–13.70) | 24 August 2016 | 6.0 | 4.65 |
| 2 | Norcia (42.79–13.15) | 24 August 2016 | 5.4 | 4.87 |
| 3 | Castel Santangelo sul Nera (42.88–13.12) | 26 October 2016 | 5.4 | 3.46 |
| 4 | Visso (42.91–13.09) | 26 October 2016 | 5.9 | 2.47 |
| 5 | Norcia (42.83–13.11) | 30 October 2016 | 6.5 | 5.78 |
| 6 | Capitignano (42.56–13.29) | 18 January 2017 | 5.1 | 7.87 |
| 7 | Capitignano (42.55–13.28) | 18 January 2017 | 5.5 | 7.72 |
| 8 | Capitignano (42.52–13.29) | 18 January 2017 | 5.4 | 8.38 |
| 9 | Barete (42.48–13.28) | 18 January 2017 | 5.0 | 9.43 |
| Class | Condition | SPI Values |
|---|---|---|
| 1 | Extremely wet | SPI > 2 |
| 2 | Very wet | 1.5 ≤ SPI < 2 |
| 3 | Moderately wet | 1.0 ≤ SPI < 1.5 |
| 4 | Near normal | −1.0 ≤ SPI < 1.0 |
| 5 | Moderately dry | −1.5 ≤ SPI < −1.0 |
| 6 | Severely dry | −2.0 ≤ SPI < −1.5 |
| 7 | Extremely dry | SPI ≤ −2.0 |
| Year | Recession Period | Recession Model | Recession Coefficient |
|---|---|---|---|
| 1933 | 7 July–15 September | EF | −2.5 × 10−3 d−1 |
| 1935 | 4 June–23 August | EF | −2.2 × 10−3 d−1 |
| 1936 | 1 June–21 June | EF | −2.9 × 10−3 d−1 |
| 1938 | 22 June–12 July | EF | −3.2 × 10−3 d−1 |
| 2016 | 19 July–18 August | EF | −2.8 × 10−3 d−1 |
| 2017 | 13 June–6 August | SL | −2274 m3/d2 |
| 2018 | 27 June–27 July | SL | −2267 m3/d2 |
| 2019 | 16 August–29 September | EF | −2.1 × 10−3 d−1 |
| 2020 | 10 July–14 August | EF | −2.3 × 10−3 d−1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Matteo, L.; Capoccioni, A.; Porreca, M.; Pauselli, C. Groundwater-Surface Water Interaction in the Nera River Basin (Central Italy): New Insights after the 2016 Seismic Sequence. Hydrology 2021, 8, 97. https://doi.org/10.3390/hydrology8030097
Di Matteo L, Capoccioni A, Porreca M, Pauselli C. Groundwater-Surface Water Interaction in the Nera River Basin (Central Italy): New Insights after the 2016 Seismic Sequence. Hydrology. 2021; 8(3):97. https://doi.org/10.3390/hydrology8030097
Chicago/Turabian StyleDi Matteo, Lucio, Alessandro Capoccioni, Massimiliano Porreca, and Cristina Pauselli. 2021. "Groundwater-Surface Water Interaction in the Nera River Basin (Central Italy): New Insights after the 2016 Seismic Sequence" Hydrology 8, no. 3: 97. https://doi.org/10.3390/hydrology8030097
APA StyleDi Matteo, L., Capoccioni, A., Porreca, M., & Pauselli, C. (2021). Groundwater-Surface Water Interaction in the Nera River Basin (Central Italy): New Insights after the 2016 Seismic Sequence. Hydrology, 8(3), 97. https://doi.org/10.3390/hydrology8030097








