Effect of Plate Configuration in the Primary Stability of Osteotomies and Biological Reconstructions of Femoral Defects: Finite-Element Study
Abstract
:1. Introduction
2. Materials and Methods
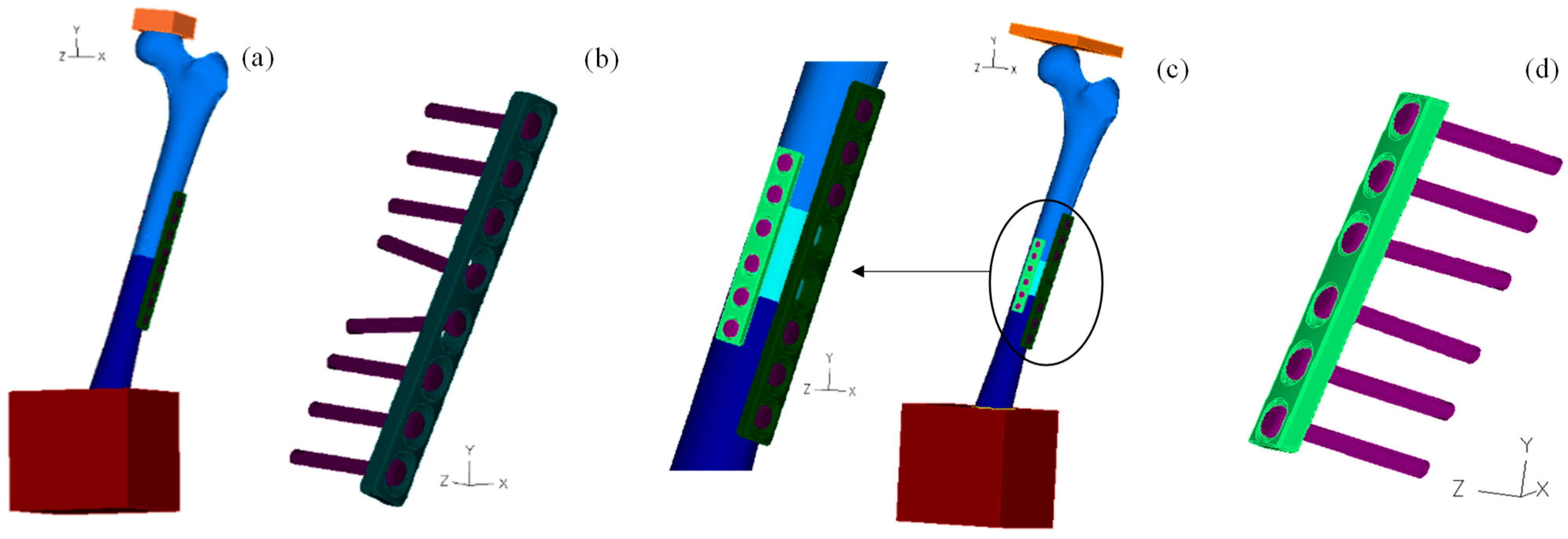
2.1. Geometrical Models
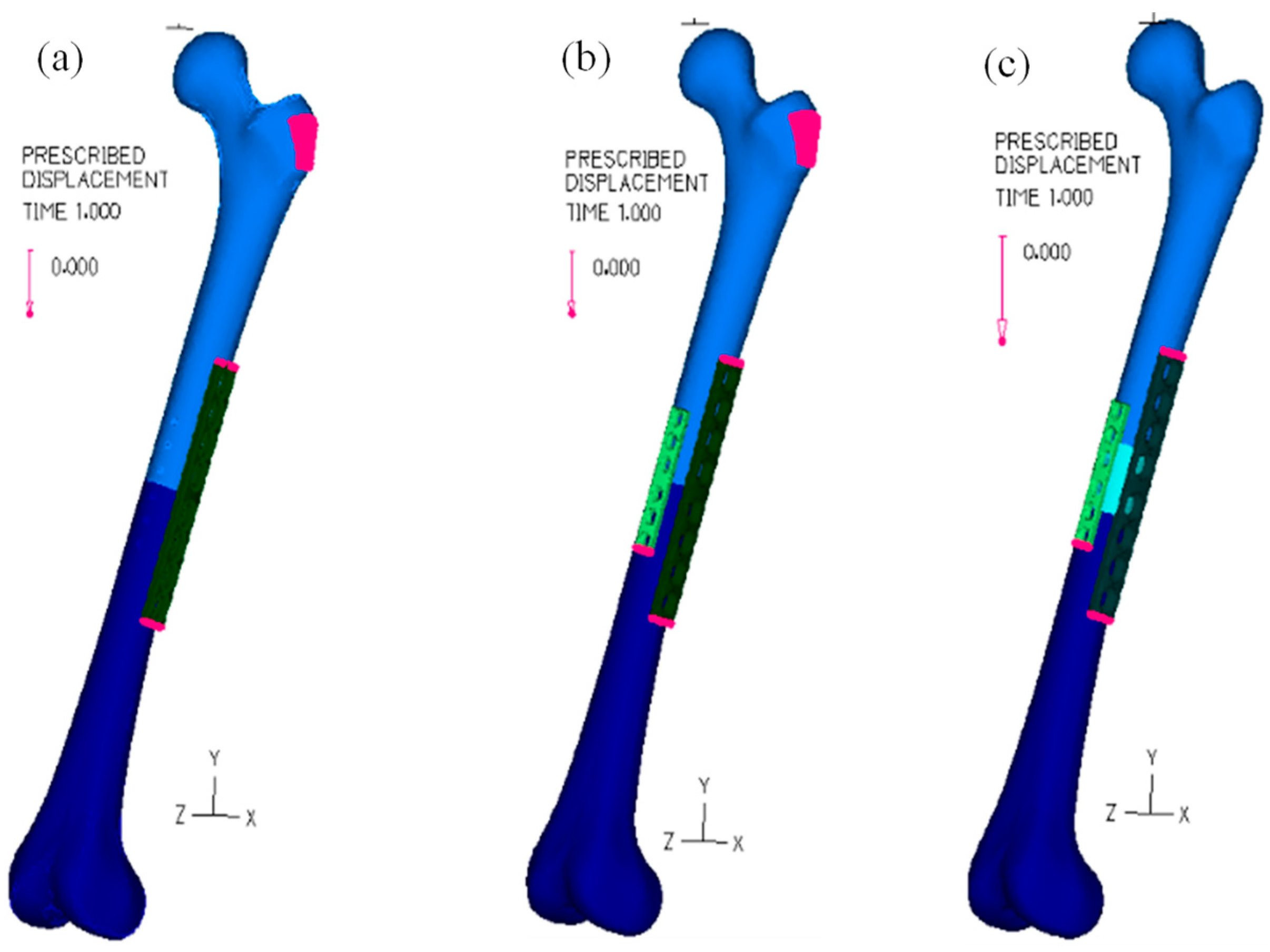
2.2. Numerical Models
2.3. Bolt Analysis
2.4. Loading Analysis
3. Results
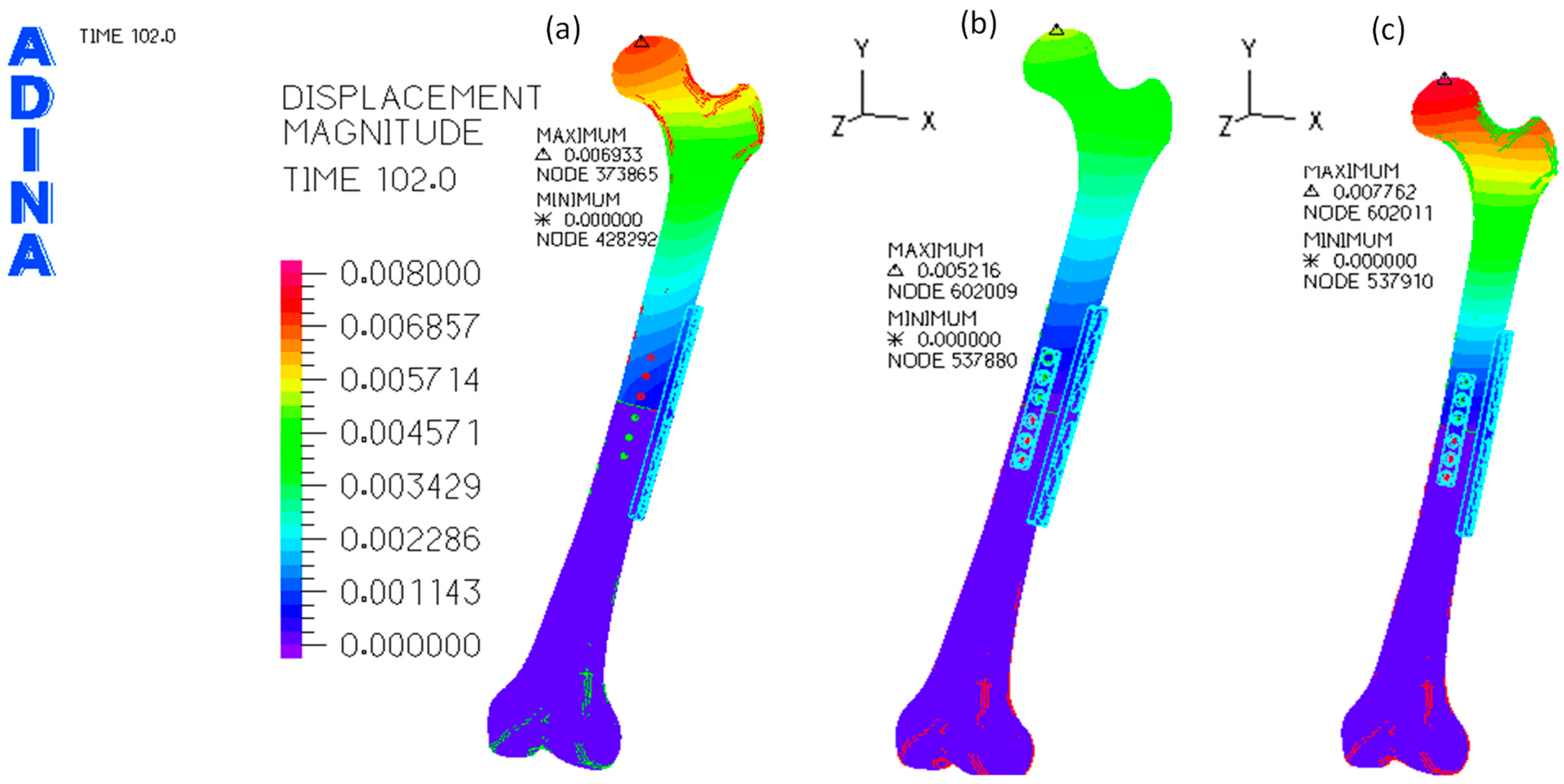
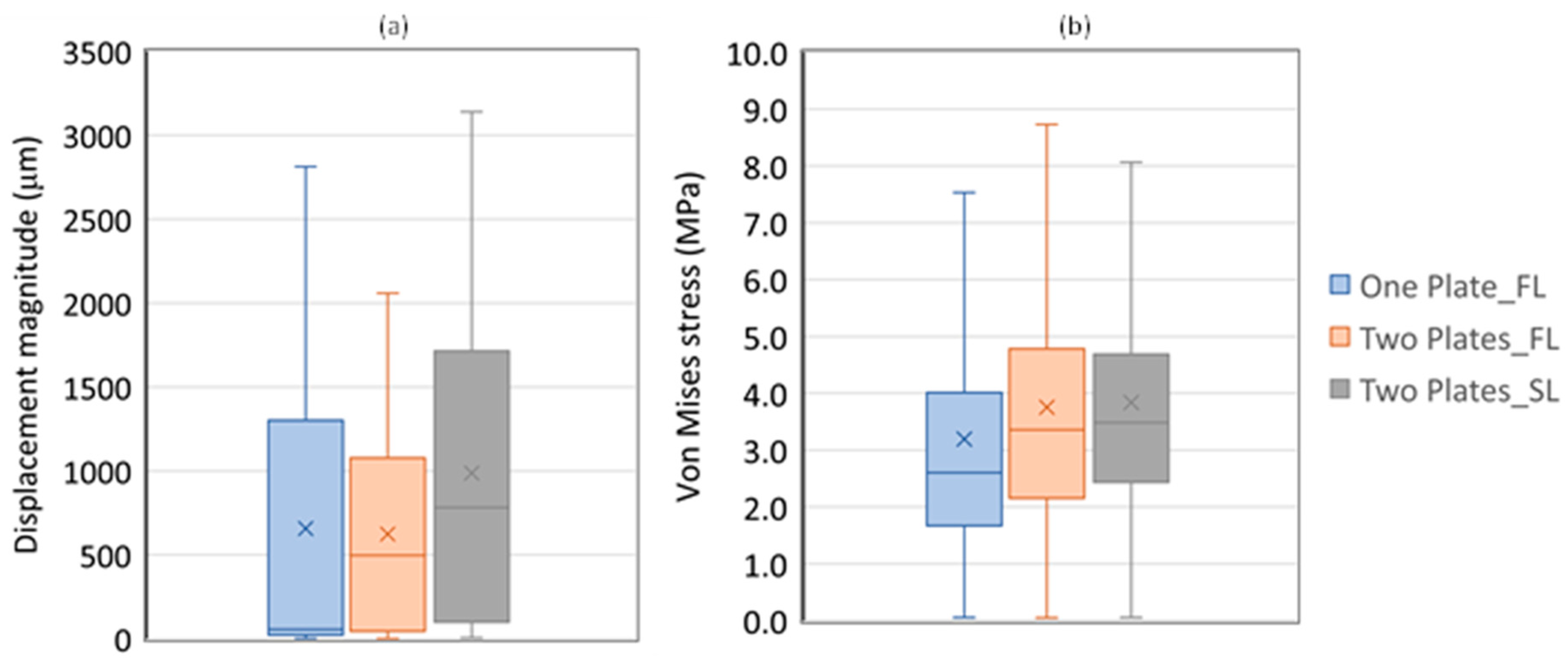
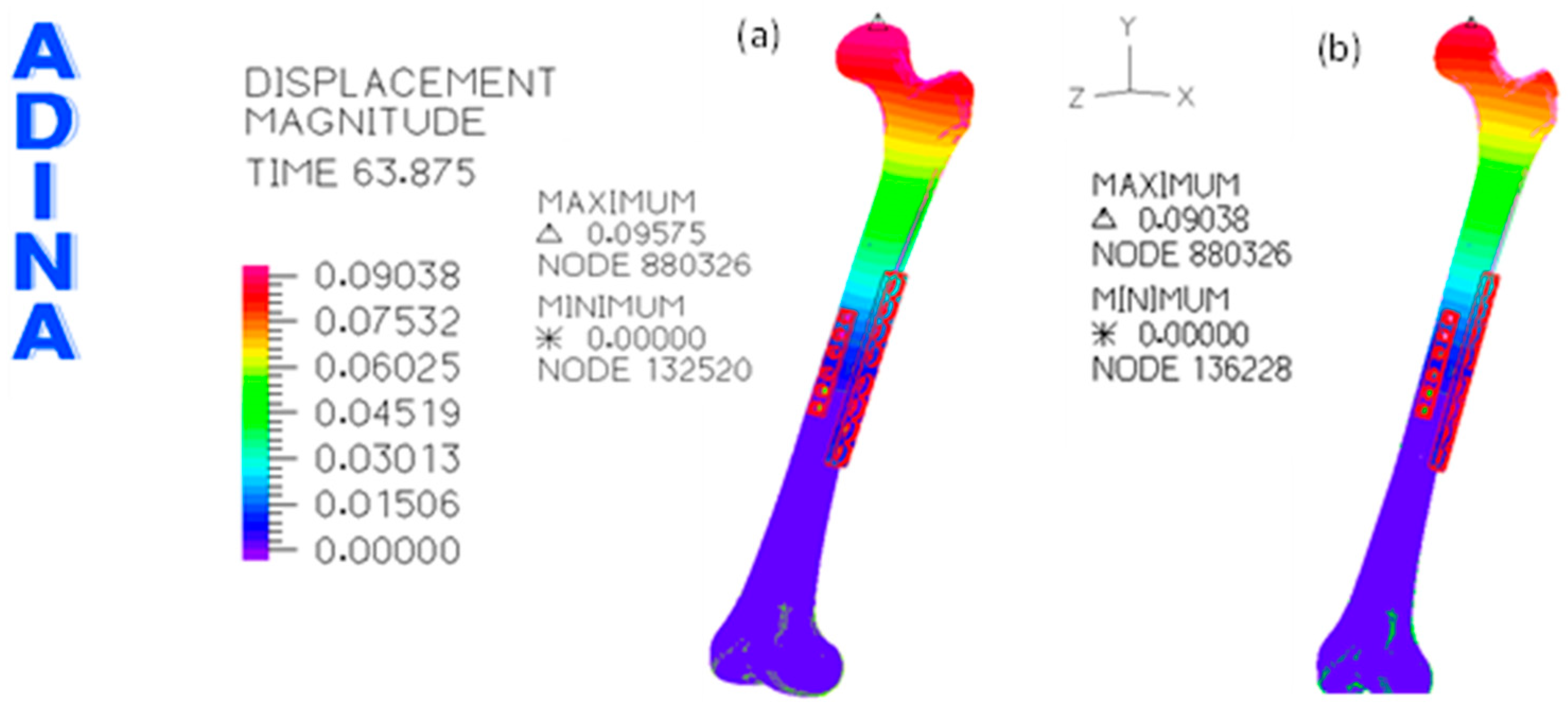
3.1. Without Allograft Bone
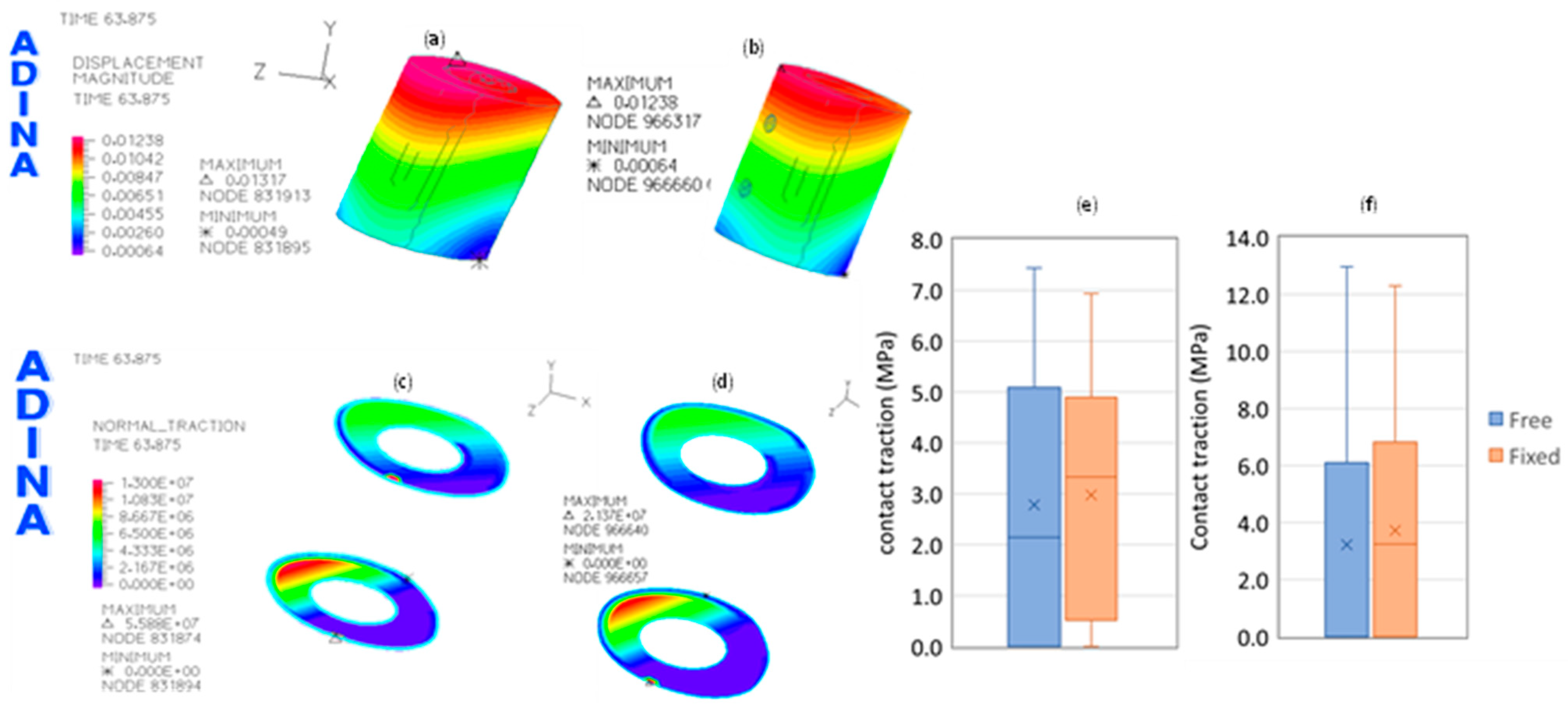
3.2. With Allograft Bone
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rathor, S.; Uddanwadiker, R.; Apte, A. Influence of Fixation Stabilization on Femur Diaphyseal Fracture Healing—A Finite Element Study Comparing Healing Outcomes of Nailing and Plating. J. Mech. Med. Biol. 2023, 23, 2350040. [Google Scholar] [CrossRef]
- Han, C.-S.; Chung, D.-W.; Lee, J.-H.; Jeong, B.-O. Lengthening of intercalary allograft combined with free vascularized fibular graft after reconstruction in pediatric osteosarcoma of femur. J. Pediatr. Orthop. B 2010, 19, 61–65. Available online: https://journals.lww.com/jpo-b/fulltext/2010/01000/lengthening_of_intercalary_allograft_combined_with.12.aspx (accessed on 20 March 2024). [CrossRef] [PubMed]
- Emori, M.; Kaya, M.; Irifune, H.; Takahashi, N.; Shimizu, J.; Mizushima, E.; Murahashi, Y.; Yamashita, T. Vascularised fibular grafts for reconstruction of extremity bone defects after resection of bone and soft-tissue tumours. Bone Jt. J. 2017, 99-B, 1237–1243. [Google Scholar] [CrossRef] [PubMed]
- Goldin, A.N.; Johnson, S.R.; Hajdu, K.S.; Kowalski, B.L.; Volkmar, A.J.; Moran, C.P.; Rekulapelli, A.; Lawrenz, J.M.; Halpern, J.L.; Schwartz, H.S.; et al. Surgical Fixation Method in Lower Extremity Intercalary Allograft Reconstruction after Oncologic Resection: A Comparison of Plates and Nails. JAAOS—J. Am. Acad. Orthop. Surg. 2023, 31, 881–892. Available online: https://journals.lww.com/jaaos/fulltext/2023/08150/surgical_fixation_method_in_lower_extremity.5.aspx (accessed on 20 March 2024). [CrossRef] [PubMed]
- Furtado, M.; Chen, L.; Chen, Z.; Chen, A.; Cui, W. Development of fish collagen in tissue regeneration and drug delivery. Eng. Regen. 2022, 3, 217–231. [Google Scholar] [CrossRef]
- Wang, J.; Huang, D.; Yu, H.; Cheng, Y.; Ren, H.; Zhao, Y. Developing tissue engineering strategies for liver regeneration. Eng. Regen. 2022, 3, 80–91. [Google Scholar] [CrossRef]
- Yang, S.; Wang, F.; Han, H.; Santos, H.A.; Zhang, Y.; Zhang, H.; Wei, J.; Cai, Z. Fabricated technology of biomedical micro-nano hydrogel. Biomed. Technol. 2023, 2, 31–48. [Google Scholar] [CrossRef]
- Nongdamba, H.; Bondarde, P.; Danish, V.; Maheshwari, V.; Karn, R.; Olkha, V.; Dhingra, M.; Vathulya, M. Functional audit of the use of megaprosthesis for limb reconstruction in musculoskeletal tumors—A retrospective single-center study. J. Orthop. 2024, 49, 123–127. [Google Scholar] [CrossRef]
- Henderson, E.R.; Groundland, J.S.; Pala, E.; Dennis, J.A.; Wooten, R.; Cheong, D.; Windhager, R.; Kotz, R.I.; Mercuri, M.; Funovics, P.T.; et al. Failure mode classification for tumor endoprostheses: Retrospective review of five institutions and a literature review. J. Bone Jt. Surg. 2011, 93, 418–429. [Google Scholar] [CrossRef]
- He, Z.; Huang, S.; Ji, T.; Tang, X.; Yang, R.; Guo, W. Plate configuration for biological reconstructions of femoral intercalary defect—A finite element evaluation. Comput. Methods Programs Biomed. 2022, 224, 107006. [Google Scholar] [CrossRef]
- Li, J.; Rai, S.; Ze, R.; Tang, X.; Liu, R.; Hong, P. The optimal choice for length unstable femoral shaft fracture in school-aged children: A comparative study of elastic stable intramedullary nail and submuscular plate. Medicine 2020, 99, e20796. Available online: https://journals.lww.com/md-journal/fulltext/2020/06190/the_optimal_choice_for_length_unstable_femoral.73.aspx (accessed on 20 March 2024). [CrossRef] [PubMed]
- Ramseier, L.E.; Janicki, J.A.; Weir, S.; Narayanan, U.G. Femoral Fractures in Adolescents: A Comparison of Four Methods of Fixation. J. Bone Jt. Surg. 2010, 92, 1122–1129. Available online: https://journals.lww.com/jbjsjournal/fulltext/2010/05000/femoral_fractures_in_adolescents__a_comparison_of.8.aspx (accessed on 20 March 2024). [CrossRef] [PubMed]
- Paul, R.W.; Abraham, J.A. Carbon fiber with reinforced polyetheretherketone (CFR-PEEK) plating and nailing for intercalary resection with tibial allograft reconstruction in two oncologic patients. J. Orthop. Rep. 2024, 3, 100263. [Google Scholar] [CrossRef]
- Liu, Q.; Long, F.; Zhang, C.; Liu, Y.; He, H.; Luo, W. Biological reconstruction of bone defect after resection of malignant bone tumor by allograft: A single-center retrospective cohort study. World J. Surg. Oncol. 2023, 21, 234. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Pan, Z.; Guo, H.; Fei, X.; Cheng, D.; Yang, Q. Long-Term Follow-Up of Biological Reconstruction with Free Fibular Graft after Resection of Extremity Diaphyseal Bone Tumors. J. Clin. Med. 2022, 11, 7225. [Google Scholar] [CrossRef] [PubMed]
- Lopes, V.M.M.; Neto, M.A.; Amaro, A.M.; Roseiro, L.M.; Paulino, M.F. FE and experimental study on how the cortex material properties of synthetic femurs affect strain levels. Med. Eng. Phys. 2017, 46, 96–109. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.-H.; Kim, S.-H.; Chang, S.-H. Estimation of the movement of the inter-fragmentary gap of a fractured human femur in the presence of a composite bone plate. J. Compos. Mater. 2011, 45, 1491–1498. [Google Scholar] [CrossRef]
- Kim, S.-H.; Chang, S.-H.; Jung, H.-J. The finite element analysis of a fractured tibia applied by composite bone plates considering contact conditions and time-varying properties of curing tissues. Compos. Struct. 2010, 92, 2109–2118. [Google Scholar] [CrossRef]
- Duda, G.N.; Mandruzzato, F.; Heller, M.; Goldhahn, J.; Moser, R.; Hehli, M.; Claes, L.; Haas, N.P. Mechanical boundary conditions of fracture healing: Borderline indications in the treatment of unreamed tibial nailing. J. Biomech. 2001, 34, 639–650. [Google Scholar] [CrossRef]
- ASM International. Properties and Selection: Nonferrous Alloys and Special-Purpose Materials; ASTM International: West Conshohocken, PA, USA, 1998; Volume 2. [Google Scholar]
- Feng, X.; Qi, W.; Wang, C.; Leung, F.; Chen, B. Effect of the screw tightening sequence on the stress distribution of a dynamic compression plate: A pilot finite element study. J. Orthop. Surg. 2019, 27, 2309499019876073. [Google Scholar] [CrossRef]
- El Beaino, M.; Morris, R.P.; Lindsey, R.W.; Gugala, Z. Biomechanical Evaluation of Dual Plate Configurations for Femoral Shaft Fracture Fixation. BioMed Res. Int. 2019, 2019, 5958631. Available online: https://api.semanticscholar.org/CorpusID:149662591 (accessed on 20 March 2024). [CrossRef] [PubMed]
- Heyworth, B.E.; Suppan, C.A.; Kramer, D.E.; Yen, Y.M. Management of pediatric diaphyseal femur fractures. Curr. Rev. Musculoskelet. Med. 2012, 5, 120–125. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Peng, Y.; Li, J.; Li, M.; Xiong, Y.; Xiao, J.; Zhang, L.; Tang, P. Spatial Bridge Locking Fixator versus Traditional Locking Plates in Treating AO/OTA 32-A3.2 Fracture: Finite Element Analysis and Biomechanical Evaluation. Orthop. Surg. 2022, 14, 1638–1648. [Google Scholar] [CrossRef] [PubMed]
- Wisanuyotin, T.; Paholpak, P.; Sirichativapee, W.; Sirichativapee, W.; Kosuwon, W. Effect of bone cement augmentation with different configurations of the dual locking plate for femoral allograft fixation: Finite element analysis and biomechanical study. J. Orthop. Surg. Res. 2023, 18, 405. [Google Scholar] [CrossRef] [PubMed]
- Wisanuyotin, T.; Sirichativapee, W.; Paholpak, P.; Kosuwan, W.; Kasai, Y. Optimal configuration of a dual locking plate for femoral allograft or recycled autograft bone fixation: A finite element and biomechanical analysis. Clin. Biomech. 2020, 80, 105156. [Google Scholar] [CrossRef] [PubMed]
- Vitiello, R.; Lillo, M.; Donati, F.; Masci, G.; Noia, G.; De Santis, V.; Maccauro, G. Locking plate fixation in pediatric femur fracture: Evaluation of the outcomes in our experience. Acta Biomed. Atenei Parm. 2019, 90, 110–115. [Google Scholar] [CrossRef] [PubMed]
- Hong, P.; Rai, S.; Tang, X.; Liu, R.; Li, J. Operative Choice for Length-Unstable Femoral Shaft Fracture in School-Aged Children: Locking Plate vs. Monolateral External Fixator. Front. Pediatr. 2022, 9, 799487. [Google Scholar] [CrossRef] [PubMed]
- Buecker, P.J.; Berenstein, M.; Gebhardt, M.C.; Hornicek, F.J.; Mankin, H.J. Locking Versus Standard Plates for Allograft Fixation After Tumor Resection in Children and Adolescents. J. Pediatr. Orthop. 2006, 26, 680–685. Available online: https://journals.lww.com/pedorthopaedics/fulltext/2006/09000/locking_versus_standard_plates_for_allograft.21.aspx (accessed on 20 March 2024). [CrossRef] [PubMed]
- Martins Amaro, A.; Paulino, M.F.; Roseiro, L.M.; Neto, M.A. The Effect of External Fixator Configurations on the Dynamic Compression Load: An Experimental and Numerical Study. Appl. Sci 2020, 10, 3. [Google Scholar] [CrossRef]
- Qi, F.; Gao, X.; Shuai, Y.; Peng, S.; Deng, Y.; Yang, S.; Yang, Y.; Shuai, C. Magnetic-driven wireless electrical stimulation in a scaffold. Compos. Part B Eng. 2022, 237, 109864. [Google Scholar] [CrossRef]
- Baleani, M.; Erani, P.; Blaise, M.; Fognani, R.; Palmas, M.; Manfrini, M. Intercalary reconstruction of long bones by massive allograft: Comparison of construct stability ensured by three different host-graft junctions and two types of fixations in a synthetic femur model. Front. Pediatr. 2022, 10, 868299. [Google Scholar] [CrossRef] [PubMed]
- Roseiro, L.M.; Neto, M.A.; Amaro, A.; Leal, R.P.; Samarra, M.C. External fixator configurations in tibia fractures: 1D optimization and 3D analysis comparison. Comput. Methods Programs Biomed. 2014, 113, 360–370. [Google Scholar] [CrossRef] [PubMed]


| Material | Density (kg/m3) | Young Modulus (GPa) | Coefficient of Poisson |
|---|---|---|---|
| Trabecular Bone, [19] | 300 | 1.1 | 0.30 |
| Cortical Bone, [19] | 1800 | 15.0 | 0.30 |
| screws and osteosynthesis plate (AISI 316L), [20] | 8027 | 200.0 | 0.27 |
| Aluminum loading plates | 2700 | 69 | 0.33 |
| Aver. | SD | Minimum | 1st Quartile | Median | 3rd Quartile | Maximum | Skewness | Kurtosis | |
|---|---|---|---|---|---|---|---|---|---|
| Displacement (µm) | |||||||||
| One Plate | 657.2 | 780.50 | 2.5 | 23.9 | 55.8 | 1301.1 | 2812.1 | 0.78 | −0.80 |
| Two Plates | 623.7 | 573.9 | 1.8 | 44.9 | 498.1 | 1077.0 | 2058.3 | 0.59 | −0.89 |
| Two Plates_SL | 987.7 | 897.3 | 5.9 | 98.7 | 781.4 | 1715.0 | 3139.2 | 0.59 | −0.94 |
| Von Mises (MPa) | |||||||||
| One Plate | 3.2 | 2.8 | 0.1 | 1.7 | 2.6 | 4.0 | 83.8 | 6.3 | 90.0 |
| Two Plates | 3.8 | 2.7 | 0.1 | 2.2 | 3.4 | 4.8 | 106.5 | 6.1 | 108.6 |
| Two Plates_SL | 3.8 | 2.9 | 0.1 | 2.4 | 3.5 | 4.7 | 154.8 | 12.6 | 353.7 |
| Aver. | SD | Minimum | 1st Quartile | Median | 3rd Quartile | Maximum | Skewness | Kurtosis | |
|---|---|---|---|---|---|---|---|---|---|
| Free | 3.7 | 2.3 | 0.2 | 1.6 | 3.5 | 5.3 | 32.5 | 1 | 4.4 |
| Fixed | 5.8 | 5.6 | 0.2 | 3.6 | 4.9 | 6.3 | 175.2 | 8.8 | 144.2 |
| Variation (%) | 58.6 | 145.5 | −2.7 | 131.9 | 42.0 | 19.1 | 438.4 | 785.5 | 3196.7 |
| Aver. | SD | Minimum | 1st Quartile | Median | 3rd Quartile | Maximum | Skewness | Kurtosis | |
|---|---|---|---|---|---|---|---|---|---|
| Displacement (µm) | |||||||||
| Free | 4723. | 5102. | 126.4 | 313.3 | 2771.0 | 8233.1 | 18,694.2 | 0.796 | −0.647 |
| Fixed | 4197. | 4210. | 115.5 | 349.9 | 3194.1 | 6108.8 | 17,649.1 | 1.03 | 0.180 |
| Von Mises (MPa) | |||||||||
| Free | 7.5 | 5.6 | 0.1 | 4.1 | 6.7 | 9.6 | 258.4 | 5.6 | 132.3 |
| Fixed | 6.4 | 5.0 | 0.2 | 3.6 | 5.2 | 8.1 | 248.4 | 6.0 | 138.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Neto, M.A.; Paulino, M.F.; Amaro, A.M. Effect of Plate Configuration in the Primary Stability of Osteotomies and Biological Reconstructions of Femoral Defects: Finite-Element Study. Bioengineering 2024, 11, 416. https://doi.org/10.3390/bioengineering11050416
Neto MA, Paulino MF, Amaro AM. Effect of Plate Configuration in the Primary Stability of Osteotomies and Biological Reconstructions of Femoral Defects: Finite-Element Study. Bioengineering. 2024; 11(5):416. https://doi.org/10.3390/bioengineering11050416
Chicago/Turabian StyleNeto, M. A., M. F. Paulino, and A. M. Amaro. 2024. "Effect of Plate Configuration in the Primary Stability of Osteotomies and Biological Reconstructions of Femoral Defects: Finite-Element Study" Bioengineering 11, no. 5: 416. https://doi.org/10.3390/bioengineering11050416
APA StyleNeto, M. A., Paulino, M. F., & Amaro, A. M. (2024). Effect of Plate Configuration in the Primary Stability of Osteotomies and Biological Reconstructions of Femoral Defects: Finite-Element Study. Bioengineering, 11(5), 416. https://doi.org/10.3390/bioengineering11050416









