Application of Fibre Bragg Grating Sensors in Strain Monitoring and Fracture Recovery of Human Femur Bone
Abstract
:1. Introduction
2. Methods
2.1. Theory of FBG Sensors
2.2. Instrumentation, Testing and FBG Fixation
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Beals, R.K.; Tower, S.S. Periprosthetic Fractures of the Femur. Clin. Orthop. Relat. Res. 1996, 327, 238–246. [Google Scholar] [CrossRef]
- Cooke, P.; Newman, J. Fractures of the femur in relation to cemented hip prostheses. J. Bone Jt. Surgery. Br. Vol. 1988, 70, 386–389. [Google Scholar] [CrossRef]
- Bethea, J.S.; DeAndrade, J.R.; Fleming, L.L.; Lindenbaum, S.D.; Welch, R.B. Proximal Femoral Fractures Following Total Hip Arthroplasty. Clin. Orthop. Relat. Res. 1982, 1982, 95–106. [Google Scholar] [CrossRef]
- Dennis, M.G.; A Simon, J.; Kummer, F.J.; Koval, K.J.; E DiCesare, P. Fixation of periprosthetic femoral shaft fractures occurring at the tip of the stem. J. Arthroplast. 2000, 15, 523–528. [Google Scholar] [CrossRef]
- Ebrahimi, H.; Rabinovich, M.; Vuleta, V.; Zalcman, D.; Shah, S.; Dubov, A.; Roy, K.; Siddiqui, F.S.; Schemitsch, E.H.; Bougherara, H.; et al. Biomechanical properties of an intact, injured, repaired, and healed femur: An experimental and computational study. J. Mech. Behav. Biomed. Mater. 2012, 16, 121–135. [Google Scholar] [CrossRef]
- E Johansson, J.; McBroom, R.; Barrington, T.W.; A Hunter, G. Fracture of the ipsilateral femur in patients wih total hip replacement. J. Bone Jt. Surgery-American Vol. 1981, 63, 1435–1442. [Google Scholar] [CrossRef]
- Zenni, E.J.; Pomeroy, D.L.; Caudle, R.J. Ogden Plate and Other Fixations for Fractures Complicating Femoral Endoprostheses. Clin. Orthop. Relat. Res. 1988, 1988, 83–90. [Google Scholar] [CrossRef]
- Berman, A.T.; Zamarin, R. The use of Dall-Miles cables in total hip arthroplasty. Orthopedics 1993, 16, 833–835. [Google Scholar]
- Brady, O.H.; Garbuz, N.S.; Masri, B.A.; Duncan, C.P. CLASSIFICATION OF THE HIP. Orthop. Clin. N. Am. 1999, 30, 215–220. [Google Scholar] [CrossRef]
- Brady, O.H.; Garbuz, N.S.; Masri, B.A.; Duncan, C.P. The reliability of validity of the Vancouver classification of femoral fractures after hip replacement. J. Arthroplast. 2000, 15, 59–62. [Google Scholar] [CrossRef]
- Duncan, C.P.; A Masri, B. The role of antibiotic-loaded cement in the treatment of an infection after a hip replacement. Instr. Course Lect. 1995, 44, 305–313. [Google Scholar]
- Serocki, J.H.; Chandler, R.W.; Dorr, L.D. Treatment of fractures about hip prostheses with compression plating. J. Arthroplast. 1992, 7, 129–135. [Google Scholar] [CrossRef]
- Tsiridis, E.; Haddad, F.S.; Gie, G.A. The management of periprosthetic femoral fractures around hip replacements. Injury 2003, 34, 95–105. [Google Scholar] [CrossRef]
- Wilson, D.; Masri, B.A.; Duncan, C.P. Periprosthetic fractures: An operative algorithm. Orthopedics 2001, 24, 869–870. [Google Scholar]
- Chandler, H.P.; King, D.; Limbird, R.; Hedley, A.; McCarthy, J.; Penenberg, B.; Danylchuk, K. The use of cortical allograft struts for fixation of fractures associated with well-fixed total joint prostheses. Semin. Arthroplast. 1993, 4, 99. [Google Scholar]
- Emerson, R.H.; I Malinin, T.; Cuellar, A.D.; Head, W.C.; Peters, P.C. Cortical strut allografts in the reconstruction of the femur in revision total hip arthroplasty. A basic science and clinical study. Clin. Orthop. Relat. Res. 1992, 1992, 35–44. [Google Scholar]
- Haddad, F.S.; Duncan, C.P.; Berry, D.J.; Lewallen, D.G.; Gross, A.E.; Chandler, H.P. Periprosthetic femoral fractures around well-fixed implants: Use of cortical onlay allografts with or without a plate. J. Bone Jt. Surgery-American Vol. 2002, 84, 945–950. [Google Scholar]
- Ogden, W.S. Fractures beneath hip prosthses: A special indication for Parham bands and plating. Orthop. Trans. 1978, 2, 70. [Google Scholar]
- Tadross, T.; Nanu, A.; Buchanan, M.; Checketts, R. Dall-Miles plating for periprosthetic B1 fractures of the femur. J. Arthroplast. 2000, 15, 47–51. [Google Scholar] [CrossRef]
- Tsiridis, E.; Haddad, F.S.; Gie, G.A. Dall-Miles plates for periprosthetic femoral fractures. A critical review of 16 cases. Injury 2003, 34, 107–110. [Google Scholar]
- Zdero, R.; Walker, R.; Waddell, J.P.; Schemitsch, E.H. Biomechanical Evaluation of Periprosthetic Femoral Fracture Fixation. J. Bone Jt. Surgery-American Vol. 2008, 90, 1068–1077. [Google Scholar] [CrossRef]
- Grassi, L.; Isaksson, H. Extracting accurate strain measurements in bone mechanics: A critical review of current methods. J. Mech. Behav. Biomed. Mater. 2015, 50, 43–54. [Google Scholar] [CrossRef] [Green Version]
- Väänänen, S.P.; Yavari, S.A.; Weinans, H.; Zadpoor, A.A.; Jurvelin, J.S.; Isaksson, H. Repeatability of digital image correlation for measurement of surface strains in composite long bones. J. Biomech. 2013, 46, 1928–1932. [Google Scholar] [CrossRef]
- Basso, T.; Klaksvik, J.; Syversen, U.; Foss, O.A. Biomechanical femoral neck fracture experiments—A narrative review. Injury 2012, 43, 1633–1639. [Google Scholar] [CrossRef]
- Fresvig, T.; Ludvigsen, P.; Steen, H.; Reikerås, O. Fibre optic Bragg grating sensors: An alternative method to strain gauges for measuring deformation in bone. Med Eng. Phys. 2008, 30, 104–108. [Google Scholar] [CrossRef]
- Fleming, B.; Beynnon, B.D. In vivo measurement of ligament/tendon strains and forces: A review. Ann. Biomed. Eng. 2004, 32, 318–328. [Google Scholar] [CrossRef]
- Othonos, A. Fiber Bragg gratings. Rev. Sci. Instrum. 1997, 68, 4309–4341. [Google Scholar]
- Al-Ahmad, O.; Ourak, M.; Van Roosbroeck, J.; Vlekken, J.; Poorten, E.B.V. Improved FBG-Based Shape Sensing Methods for Vascular Catheterization Treatment. IEEE Robot. Autom. Lett. 2020, 5, 1. [Google Scholar] [CrossRef]
- Beisenova, A.; Issatayeva, A.; Iordachita, I.; Blanc, W.; Molardi, C.; Tosi, D. Distributed fiber optics 3D shape sensing by means of high scattering NP-doped fibers simultaneous spatial multiplexing. Opt. Express 2019, 27, 22074–22087. [Google Scholar] [CrossRef]
- Beisenova, A.; Issatayeva, A.; Korganbayev, S.; Molardi, C.; Blanc, W.; Tosi, D. Simultaneous Distributed Sensing on Multiple MgO-Doped High Scattering Fibers by Means of Scattering-Level Multiplexing. J. Light. Technol. 2019, 37, 3413–3421. [Google Scholar] [CrossRef]
- Floris, I.; Madrigal, J.; Sales, S.; Calderón, P.A.; Adam, J. Twisting measurement and compensation of optical shape sensor based on spun multicore fiber. Mech. Syst. Signal Process. 2020, 140, 106700. [Google Scholar] [CrossRef]
- Talaia, P.; Ramos, A.; Abe, I.; Schiller, M.W.; Lopes, P.; Nogueira, R.; Pinto, J.L.; Claramunt, R.; Simões, J.A. Plated and Intact Femur Strains in Fracture Fixation Using Fiber Bragg Gratings and Strain Gauges. Exp. Mech. 2007, 47, 355–363. [Google Scholar] [CrossRef]
- Mishra, V.; Singh, N.; Rai, D.V.; Tiwari, U.; Poddar, G.; Jain, S.; Mondal, S.; Kapur, P. Fiber Bragg grating sensor for monitoring bone decalcification. Orthop. Traumatol. Surg. Res. 2010, 96, 646–651. [Google Scholar] [CrossRef] [Green Version]
- Abdul-Kadir, M.R.; Hansen, U.; Klabunde, R.; Lucas, D.; Amis, A. Finite element modelling of primary hip stem stability: The effect of interference fit. J. Biomech. 2008, 41, 587–594. [Google Scholar] [CrossRef]
- Götze, C.; Steens, W.; Vieth, V.; Poremba, C.; Claes, L.; Steinbeck, J. Primary stability in cementless femoral stems: Custom-made versus conventional femoral prosthesis. Clin. Biomech. 2002, 17, 267–273. [Google Scholar] [CrossRef]
- Dopico-González, C.; New, A.M.; Browne, M. Probabilistic finite element analysis of the uncemented hip replacement—effect of femur characteristics and implant design geometry. J. Biomech. 2010, 43, 512–520. [Google Scholar] [CrossRef] [Green Version]
- Schileo, E.; Taddei, F.; Cristofolini, L.; Viceconti, M. Subject-specific finite element models implementing a maximum principal strain criterion are able to estimate failure risk and fracture location on human femurs tested in vitro. J. Biomech. 2008, 41, 356–367. [Google Scholar] [CrossRef]
- Rothstock, S.; Uhlenbrock, A.; Bishop, N.; Laird, L.; Nassutt, R.; Morlock, N.L. Influence of interface condition and implant design on bone remodelling and failure risk for the resurfaced femoral head. J. Biomech. 2011, 44, 1646–1653. [Google Scholar] [CrossRef]
- Amstutz, H.C.; Campbell, P.A.; Le Duff, M.J. FRACTURE OF THE NECK OF THE FEMUR AFTER SURFACE ARTHROPLASTY OF THE HIP. J. Bone Jt. Surgery-American Vol. 2004, 86, 1874–1877. [Google Scholar] [CrossRef]
- Gerdesmeyer, P.D.L.; Gollwitzer, H.; Bader, R.; Rudert, M. Zugangswege zum Oberflächenersatz am Hüftgelenk. Der Orthopäde 2008, 37, 650–658. [Google Scholar] [CrossRef]
- Gupta, S.; New, A.M.; Taylor, M. Bone remodelling inside a cemented resurfaced femoral head. Clin. Biomech. 2006, 21, 594–602. [Google Scholar] [CrossRef]
- Long, J.P.; Santner, T.J.; Bartel, D.L. Hip resurfacing increases bone strains associated with short-term femoral neck fracture. J. Orthop. Res. 2009, 27, 1319–1325. [Google Scholar] [CrossRef] [Green Version]
- Pal, B.; Gupta, S.; New, A.M. A numerical study of failure mechanisms in the cemented resurfaced femur: Effects of interface characteristics and bone remodelling. Proc. Inst. Mech. Eng. Part H: J. Eng. Med. 2009, 223, 471–484. [Google Scholar] [CrossRef]
- Pal, B.; Gupta, S.; New, A.M.; Browne, M. Strain and micromotion in intact and resurfaced composite femurs: Experimental and numerical investigations. J. Biomech. 2010, 43, 1923–1930. [Google Scholar] [CrossRef]
- Taylor, M. Finite element analysis of the resurfaced femoral head. Proc. Inst. Mech. Eng. Part H: J. Eng. Med. 2006, 220, 289–297. [Google Scholar] [CrossRef]
- Grassi, L.; Schileo, E.; Taddei, F.; Zani, L.; Juszczyk, M.; Cristofolini, L.; Viceconti, M. Accuracy of finite element predictions in sideways load configurations for the proximal human femur. J. Biomech. 2012, 45, 394–399. [Google Scholar] [CrossRef]
- Zani, L.; Cristofolini, L.; Juszczyk, M.; Viceconti, M. IN-VITRO STRAIN DISTRIBUTION DURING SIDEWAYS FALL IN THE PROXIMAL HUMAN FEMUR. J. Biomech. 2012, 45, S351. [Google Scholar] [CrossRef]
- Zani, L.; Erani, P.; Grassi, L.; Taddei, F.; Cristofolini, L. Strain distribution in the proximal Human femur during in vitro simulated sideways fall. J. Biomech. 2015, 48, 2130–2143. [Google Scholar] [CrossRef]
- Cristofolini, L.; Varini, E.; Viceconti, M. In-vitro method for assessing femoral implant-bone micromotions in resurfacing hip implants under different loading conditions. Proc. Inst. Mech. Eng. Part H: J. Eng. Med. 2007, 221, 943–950. [Google Scholar] [CrossRef]
- Werneck, M.M.; Allil, R.C.S.B.; Ribeiro, B.A.; de Nazaré, F.V.B. A guide to fiber Bragg grating sensors: Current Trends in Short-and Long-period Fiber Gratings; InTech: Vienna, Austria, 2013. [Google Scholar]
- Ramos, A.; Schiller, M.W.; Abe, I.; Lopes, P.A.; Simões, J.A. Experimental Measurement and Numerical Validation of Bone Cement Mantle Strains of an In Vitro Hip Replacement Using Optical FBG Sensors. Exp. Mech. 2012, 52, 1267–1274. [Google Scholar] [CrossRef]
- Roriz, P.; Carvalho, L.R.; Frazão, O.; Santos, J.L.; Simões, J.A. From conventional sensors to fibre optic sensors for strain and force measurements in biomechanics applications: A review. J. Biomech. 2014, 47, 1251–1261. [Google Scholar] [CrossRef] [Green Version]
- Basso, T.; Klaksvik, J.; Syversen, U.; A Foss, O. A biomechanical comparison of composite femurs and cadaver femurs used in experiments on operated hip fractures. J. Biomech. 2014, 47, 3898–3902. [Google Scholar] [CrossRef]
- Heiner, A.D. Structural properties of fourth-generation composite femurs and tibias. J. Biomech. 2008, 41, 3282–3284. [Google Scholar] [CrossRef]
- McConnell, A.; Zdero, R.; Syed, K.; Peskun, C.; Schemitsch, E. The Biomechanics of Ipsilateral Intertrochanteric and Femoral Shaft Fractures: A Comparison of 5 Fracture Fixation Techniques. J. Orthop. Trauma 2008, 22, 517–524. [Google Scholar] [CrossRef]
- Papini, M.; Zdero, R.; Schemitsch, E.H.; Zalzal, P. The Biomechanics of Human Femurs in Axial and Torsional Loading: Comparison of Finite Element Analysis, Human Cadaveric Femurs, and Synthetic Femurs. J. Biomech. Eng. 2006, 129, 12–19. [Google Scholar] [CrossRef]
- Shah, S.; Kim, S.Y.R.; Dubov, A.; Schemitsch, E.H.; Bougherara, H.; Zdero, R. The biomechanics of plate fixation of periprosthetic femoral fractures near the tip of a total hip implant: Cables, screws, or both? Proc. Inst. Mech. Eng. Part H: J. Eng. Med. 2011, 225, 845–856. [Google Scholar] [CrossRef]
- Talbot, M.; Zdero, R.; Garneau, D.; Cole, P.A.; Schemitsch, E.H. Fixation of long bone segmental defects: A biomechanical study. Injury 2008, 39, 181–186. [Google Scholar] [CrossRef]
- Tosi, D. Review and Analysis of Peak Tracking Techniques for Fiber Bragg Grating Sensors. Sensors 2017, 17, 2368. [Google Scholar] [CrossRef]
- Yang, Y.; Wu, J.; Wang, M.; Wang, Q.; Yu, Q.; Chen, K.P. Fast demodulation of fiber Bragg grating wavelength from low-resolution spectral measurements using Buneman Frequency Estimation. J. Light. Technol. 2020, 1. [Google Scholar] [CrossRef]
- Bougherara, H.; Zdero, R.; Miric, M.; Shah, S.; Hardisty, M.; Zalzal, P.; Schemitsch, E.H. The biomechanics of the T2 femoral nailing system: A comparison of synthetic femurs withfinite element analysis. Proc. Inst. Mech. Eng. H. 2009, 223, 303–314. [Google Scholar]
- Cheung, G.; Zalzal, P.; Bhandari, M.; Spelt, J.; Papini, M. Finite element analysis of a femoral retrograde intramedullary nail subject to gait loading. Med. Eng. Phys. 2004, 26, 93–108. [Google Scholar] [CrossRef]
- Kuzyk, P.R.; Zdero, R.; Shah, S.; Olsen, M.; Waddell, J.P.; Schemitsch, E.H. Femoral Head Lag Screw Position for Cephalomedullary Nails. J. Orthop. Trauma 2012, 26, 414–421. [Google Scholar] [CrossRef]
- Frost, H.M. A 2003 update of bone physiology and Wolff’s Law for clinicians. Angle Orthod. 2004, 74, 3–15. [Google Scholar]
- Meireles, S.; Completo, A.; Simões, J.A.; Flores, P. Strain shielding in distal femur after patellofemoral arthroplasty under different activity conditions. J. Biomech. 2010, 43, 477–484. [Google Scholar] [CrossRef]
- Wille, H.; Ruess, M.; Rank, E.; Yosibash, Z. Uncertainty quantification for personalized analyses of human proximal femurs. J. Biomech. 2016, 49, 520–527. [Google Scholar] [CrossRef] [Green Version]
- Eberle, S.; Wutte, C.; Bauer, C.; Von Oldenburg, G.; Augat, P. Should extramedullary fixations for hip fractures be removed after bone union? Clin. Biomech. 2011, 26, 410–414. [Google Scholar] [CrossRef]
- Rosenblum, S.; Zuckerman, J.; Kummer, F.; Tam, B. A biomechanical evaluation of the Gamma nail. J. Bone Jt. Surgery. Br. Vol. 1992, 74, 352–357. [Google Scholar] [CrossRef]
- Allen, J.C.; Lindsey, R.W.; Hipp, J.A.; Gugala, Z.; Rianon, N.; Leblanc, A. The effect of retained intramedullary nails on tibial bone mineral density. Clin. Biomech. 2008, 23, 839–843. [Google Scholar] [CrossRef]
- Bråten, M.; Nordby, A.; Terjesen, T.; Rossvoll, I. Bone loss after locked intramedullary nailing: Computed tomography of the femur and tibia in 10 cases. Acta Orthop. Scand. 1992, 63, 310–314. [Google Scholar]
- Kröger, H.; Kettunen, J.; Bowditch, M.; Joukainen, J.; Suomalainen, O.; Alhava, E. Bone mineral density after the removal of intramedullary nails: A cross-sectional and longitudinal study. J. Orthop. Sci. 2002, 7, 325–330. [Google Scholar] [CrossRef]
- Nguyen, T.V.; Center, J.R.; Eisman, J.A. Femoral Neck Bone Loss Predicts Fracture Risk Independent of Baseline BMD. J. Bone Miner. Res. 2005, 20, 1195–1201. [Google Scholar] [CrossRef]
- Carvalho, P.; Abe, I.; Schiller, M.; Simões, J.; Lopes, P.; Pinto, J.L.; Carvalho, L.R. FEA and experimental FBG sensing system for the analysis of different dental implant concepts. J. Biomech. 2006, 39, S568. [Google Scholar] [CrossRef]
- Roriz, P.; Abe, I.; Schiller, M.; Gabriel, J.; Simoes, J. Ex Vivo Intervertebral Disc Bulging Measurement Using a Fibre Bragg Grating Sensor. Exp. Mech. 2011, 51, 1573–1577. [Google Scholar] [CrossRef]
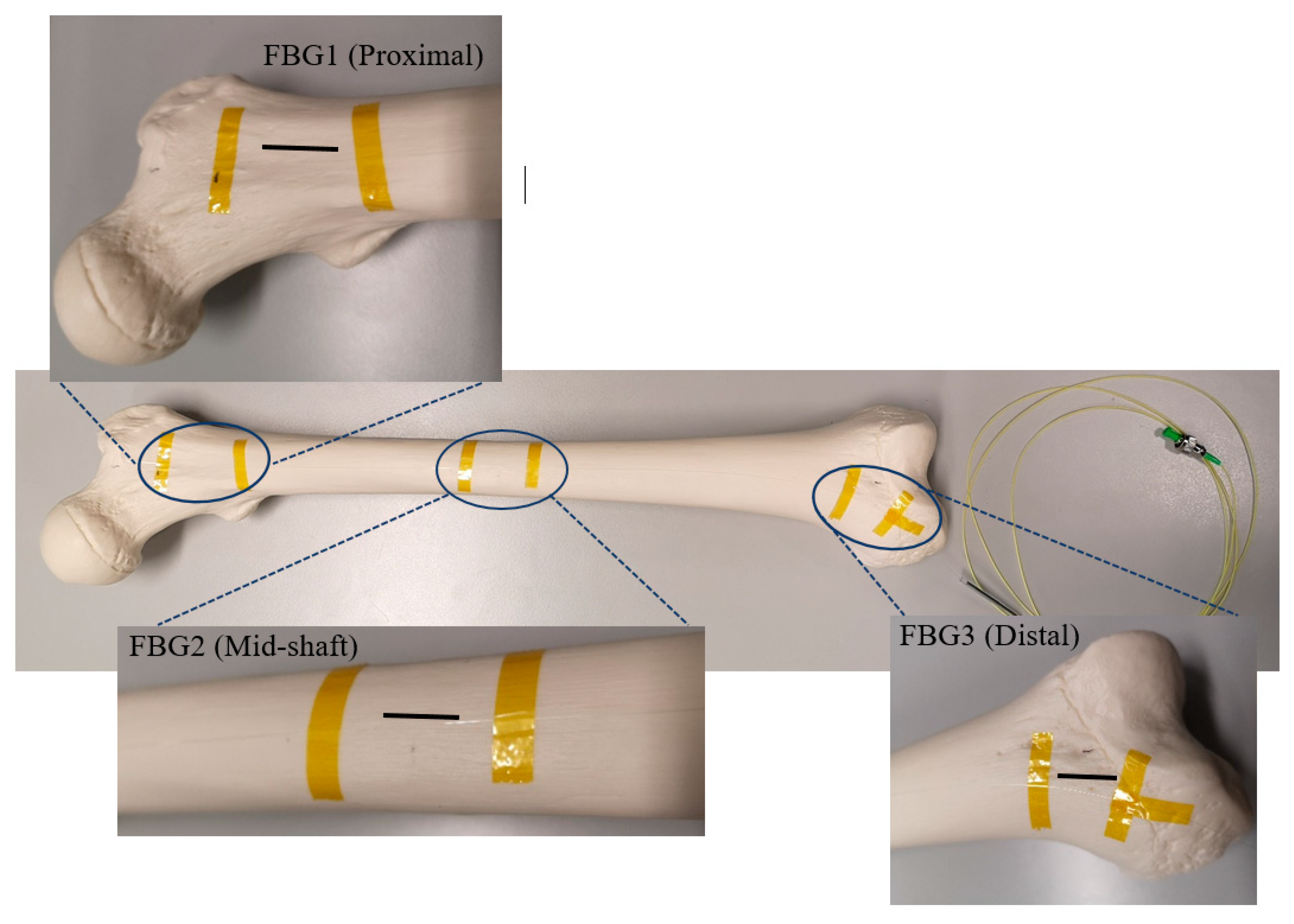
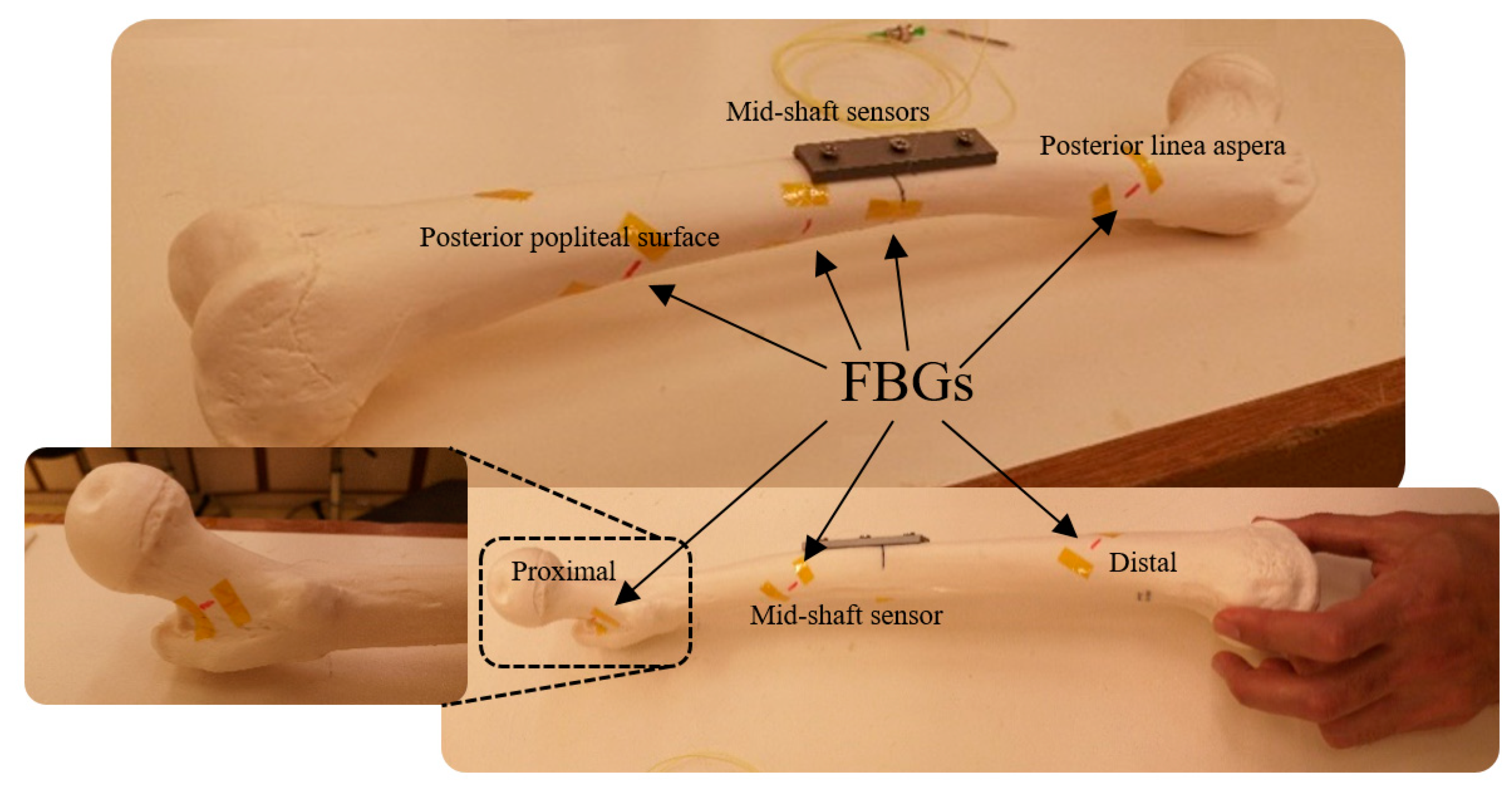
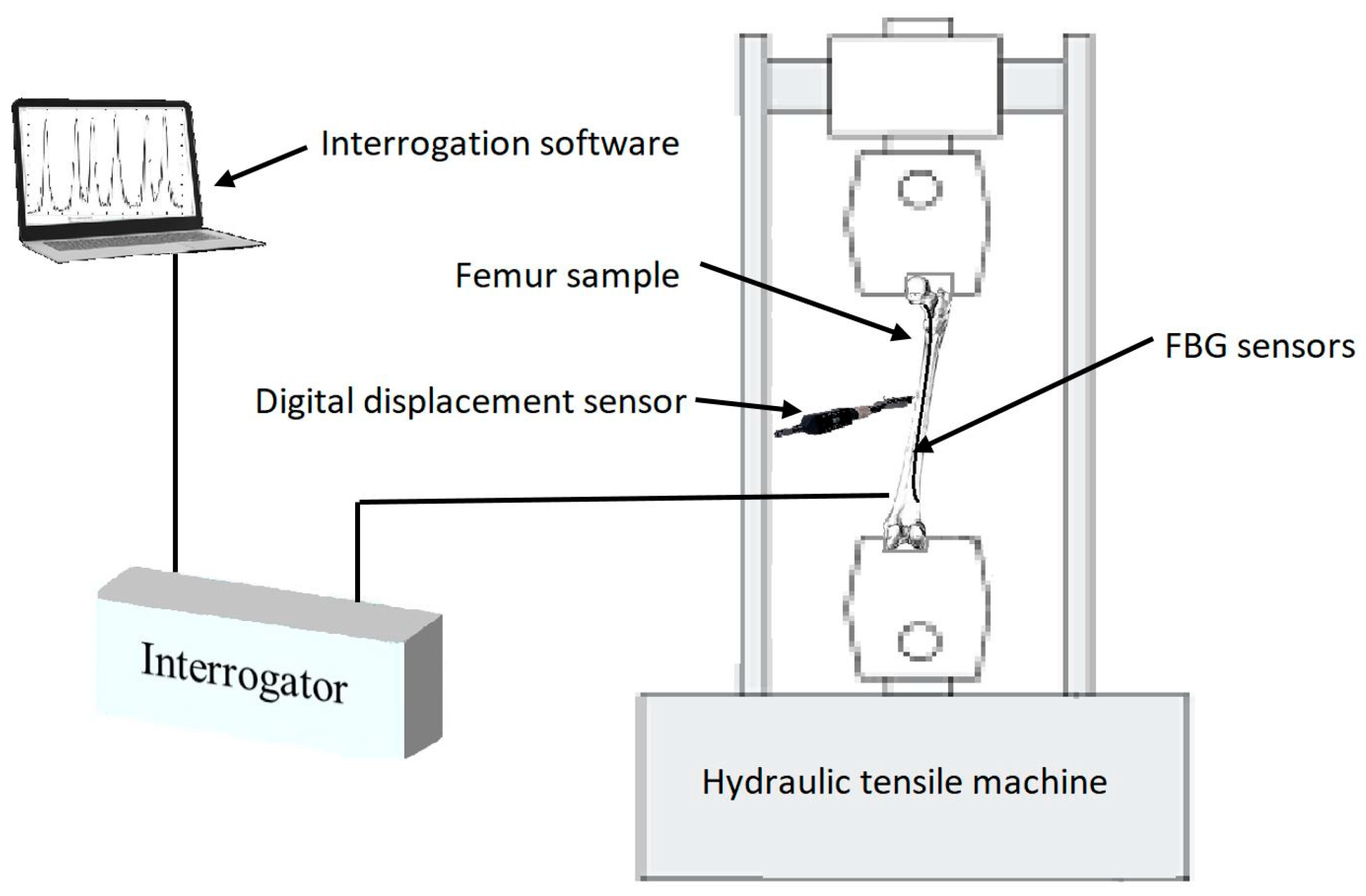



| Distance of Gratings on the Optical Fibre (cm) | ||||
|---|---|---|---|---|
| Intact | Plated | |||
| FBG Sensors | Longitudinal Fibre | Coiled Fibre | Longitudinal Fibre | Coiled Fibre |
| FBG1 | 2 | 2 | 2 | 2 |
| FBG2 | 20 | 18 | 18 | 9 |
| FBG3 | 38 | 20 | 20 | 15 |
| FBG4 | - | 22 | 22 | 20 |
| FBG5 | - | 32 | 38 | 23 |
| FBG6 | - | - | - | 29 |
| FBG7 | - | - | - | 32 |
| Femur Sawbones | FBG Array | Midshaft Displacement (mm) | Total Vertical Displacement (mm) | Loading Amount (kN) | Loading Time (s) |
|---|---|---|---|---|---|
| Intact Femur | Longitudinal Fibre | 1.01 | 0.414 | 0.098 | 24.88 |
| 2.03 | 0.689 | 0.185 | 41.38 | ||
| 3.01 | 0.944 | 0.250 | 56.68 | ||
| Coiled Fibre | 1.00 | 0.350 | 0.082 | 21.00 | |
| 2.02 | 0.670 | 0.155 | 40.20 | ||
| 3.08 | 0.955 | 0.215 | 57.30 | ||
| Plated Femur | Longitudinal Fibre | 1.05 | 0.311 | 0.071 | 18.70 |
| 2.10 | 0.625 | 0.118 | 37.50 | ||
| 3.02 | 0.945 | 0.166 | 56.70 | ||
| Coiled Fibre | 1.01 | 0.358 | 0.091 | 21.50 | |
| 2.03 | 0.690 | 0.183 | 41.45 | ||
| 3.11 | 0.976 | 0.275 | 58.60 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Najafzadeh, A.; Serandi Gunawardena, D.; Liu, Z.; Tran, T.; Tam, H.-Y.; Fu, J.; K. Chen, B. Application of Fibre Bragg Grating Sensors in Strain Monitoring and Fracture Recovery of Human Femur Bone. Bioengineering 2020, 7, 98. https://doi.org/10.3390/bioengineering7030098
Najafzadeh A, Serandi Gunawardena D, Liu Z, Tran T, Tam H-Y, Fu J, K. Chen B. Application of Fibre Bragg Grating Sensors in Strain Monitoring and Fracture Recovery of Human Femur Bone. Bioengineering. 2020; 7(3):98. https://doi.org/10.3390/bioengineering7030098
Chicago/Turabian StyleNajafzadeh, Ali, Dinusha Serandi Gunawardena, Zhengyong Liu, Ton Tran, Hwa-Yaw Tam, Jing Fu, and Bernard K. Chen. 2020. "Application of Fibre Bragg Grating Sensors in Strain Monitoring and Fracture Recovery of Human Femur Bone" Bioengineering 7, no. 3: 98. https://doi.org/10.3390/bioengineering7030098
APA StyleNajafzadeh, A., Serandi Gunawardena, D., Liu, Z., Tran, T., Tam, H.-Y., Fu, J., & K. Chen, B. (2020). Application of Fibre Bragg Grating Sensors in Strain Monitoring and Fracture Recovery of Human Femur Bone. Bioengineering, 7(3), 98. https://doi.org/10.3390/bioengineering7030098







