Data for Interaction Diagrams of Geopolymer FRC Slender Columns with Double-Layer GFRP and Steel Reinforcement
Abstract
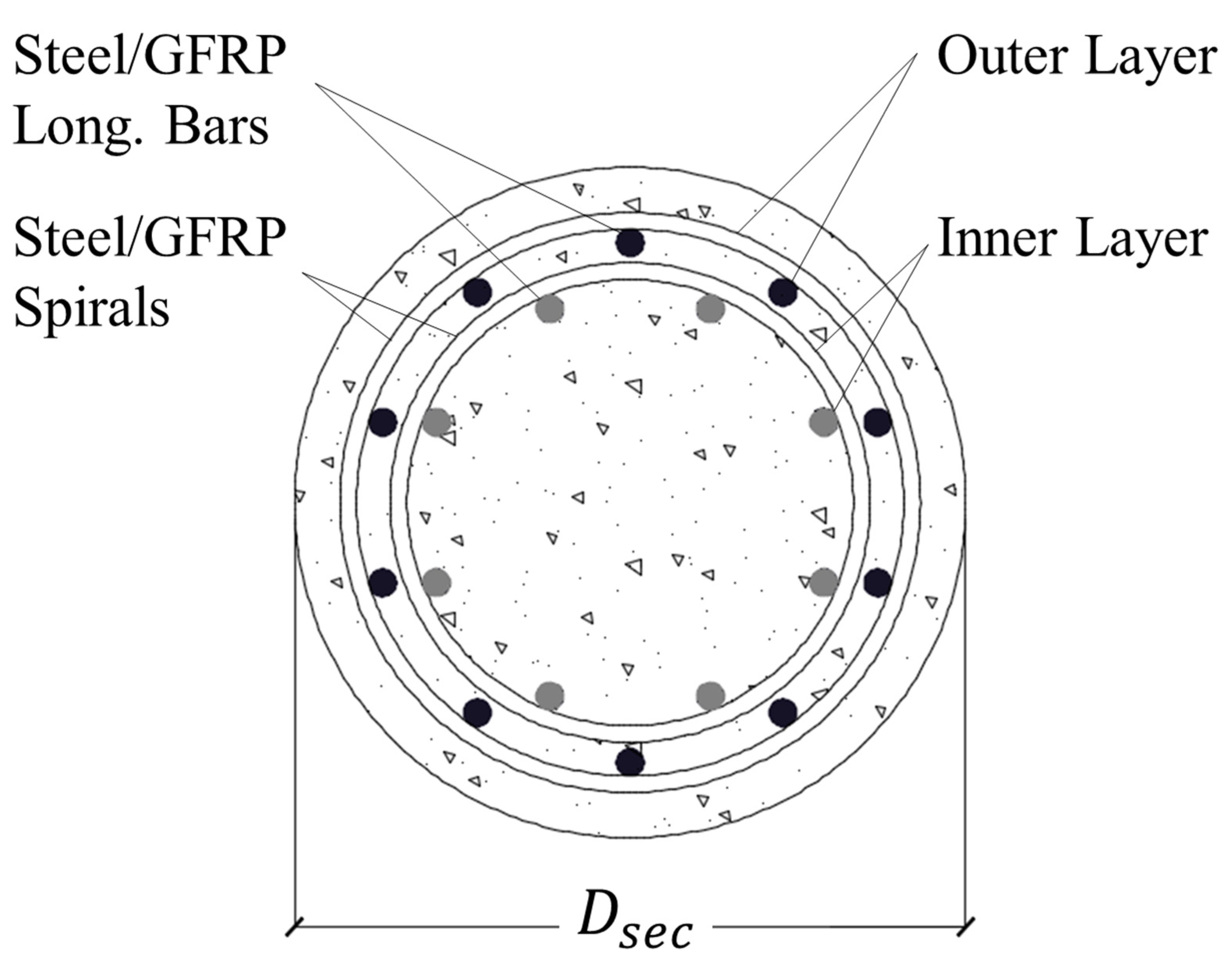
:1. Summary
2. Data Description
3. Methods
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hadhood, A.; Mohamed, H.M.; Benmokrane, B. Axial Load–Moment Interaction Diagram of Circular Concrete Columns Reinforced with CFRP Bars and Spirals: Experimental and Theoretical Investigations. J. Compos. Constr. 2017, 21, 04016092. [Google Scholar] [CrossRef]
- Farhan, N.A.; Sheikh, M.N.; Hadi, M.N. Load-Bending Moment (P-M) Interactions of Geopolymer Concrete Column Reinforced with and without Steel Fiber. ACI Struct. J. 2020, 117, 133–144. [Google Scholar] [CrossRef]
- Hales, T.A.; Pantelides, C.P.; Reaveley, L.D. Analytical buckling model for slender FRP-reinforced concrete columns. Compos. Struct. 2017, 176, 33–42. [Google Scholar] [CrossRef]
- Effect of Critical Test Parameters on Behavior of Glass Fiber-Reinforced Polymer-Reinforced Concrete Slender Columns under Eccentric Load. ACI Struct. J. 2020, 117, 127–142. [CrossRef]
- Noushini, A.; Hastings, M.; Castel, A.; Aslani, F. Mechanical and flexural performance of synthetic fibre reinforced geopolymer concrete. Constr. Build. Mater. 2018, 186, 454–475. [Google Scholar] [CrossRef]
- Kazmi, S.M.S.; Munir, M.J.; Wu, Y.-F.; Patnaikuni, I.; Zhou, Y.; Xing, F. Axial stress-strain behavior of macro-synthetic fiber reinforced recycled aggregate concrete. Cem. Concr. Compos. 2019, 97, 341–356. [Google Scholar] [CrossRef]
- Farhan, N.A.; Sheikh, M.N.; Hadi, M.N. Behaviour of Ambient Cured Steel Fibre Reinforced Geopolymer Concrete Columns Under Axial and Flexural Loads. Structures 2018, 15, 184–195. [Google Scholar] [CrossRef]
- V.ROD. Technical Data Sheets. Available online: https://www.fiberglassrebar.com/en/Fiberglass-reinforcement/Technical-data-sheets (accessed on 13 April 2021).
- Hadhood, A.; Mohamed, H.M.; Benmokrane, B.; Nanni, A.; Shield, C.K. Assessment of Design Guidelines of Concrete Columns Reinforced with Glass Fiber-Reinforced Polymer Bars. ACI Struct. J. 2019, 116, 193–207. [Google Scholar] [CrossRef]
- Proposed Flexural Stiffness of Slender Concrete Columns Reinforced with Glass Fiber-Reinforced Polymer Bars. ACI Struct. J. 2021, 118, 227–240. [CrossRef]
- Mander, J.B.; Priestley, M.J.N.; Park, R. Theoretical Stress-Strain Model for Confined Concrete. J. Struct. Eng. 1988, 114, 1804–1826. [Google Scholar] [CrossRef] [Green Version]
- Hales, T.A. Slender Concrete Columns Reinforced with Fiber Reinforced Polymer Spirals. Ph.D. Thesis, University of Utah, Salt Lake City, UT, USA, 2015. [Google Scholar]
- Hasan, H.A.; Karim, H.; Sheikh, M.N.; Hadi, M.N.S. Moment-Curvature Behavior of Glass Fiber-Reinforced Polymer Bar-Reinforced Normal-Strength Concrete and High-Strength Concrete Columns. ACI Struct. J. 2019, 116, 65–75. [Google Scholar] [CrossRef]
- Sarker, P.K. Analysis of geopolymer concrete columns. Mater. Struct. Constr. 2009, 42, 715–724. [Google Scholar] [CrossRef]
- Maranan, G.; Manalo, A.; Benmokrane, B.; Karunasena, W.; Mendis, P. Behavior of concentrically loaded geopolymer-concrete circular columns reinforced longitudinally and transversely with GFRP bars. Eng. Struct. 2016, 117, 422–436. [Google Scholar] [CrossRef]
- Wight, J.K. Reinforced Concrete: Mechanics and Design, 7th ed.; Pearson Education Limited: Edinburgh, UK, 2015. [Google Scholar]



| Group | Concrete Type | Longitudinal and Transverse Reinforcement Inner Layer | Longitudinal and Transverse Reinforcement Outer Layer | Data File |
|---|---|---|---|---|
| G1 | Steel-FRGPC | GFRP | GFRP | G1.xlsx |
| G2 | Steel-FRGPC | Steel | Steel | G2.xlsx |
| G3 | Steel-FRGPC | GFRP | Steel | G3.xlsx |
| G4 | Synthetic-FRGPC | GFRP | GFRP | G4.xlsx |
| G5 | Synthetic-FRGPC | Steel | Steel | G5.xlsx |
| G6 | Synthetic-FRGPC | GFRP | Steel | G6.xlsx |
| G7 | Plain-GPC | GFRP | GFRP | G7.xlsx |
| G8 | Plain-GPC | Steel | Steel | G8.xlsx |
| G9 | Plain-GPC | GFRP | Steel | G9.xlsx |
| Strength Level | GFRP Reinforcement | Steel Reinforcement | ||
|---|---|---|---|---|
| [MPa] | [GPa] | [MPa] | [GPa] | |
| 1 | 900 | 40 | 420 | 200 |
| 2 | 1000 | 50 | 550 | 200 |
| 3 | 1100 | 60 | 690 | 200 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
AlHamaydeh, M.; Amin, F. Data for Interaction Diagrams of Geopolymer FRC Slender Columns with Double-Layer GFRP and Steel Reinforcement. Data 2021, 6, 43. https://doi.org/10.3390/data6050043
AlHamaydeh M, Amin F. Data for Interaction Diagrams of Geopolymer FRC Slender Columns with Double-Layer GFRP and Steel Reinforcement. Data. 2021; 6(5):43. https://doi.org/10.3390/data6050043
Chicago/Turabian StyleAlHamaydeh, Mohammad, and Fouad Amin. 2021. "Data for Interaction Diagrams of Geopolymer FRC Slender Columns with Double-Layer GFRP and Steel Reinforcement" Data 6, no. 5: 43. https://doi.org/10.3390/data6050043
APA StyleAlHamaydeh, M., & Amin, F. (2021). Data for Interaction Diagrams of Geopolymer FRC Slender Columns with Double-Layer GFRP and Steel Reinforcement. Data, 6(5), 43. https://doi.org/10.3390/data6050043







