4. Heat Transfer Models
An important feature of the heat transfer processes in a metal foam is the departure from a condition of local thermal equilibrium between the solid phase, having a very large thermal conductivity, and the fluid phase which is significantly less conductive. The general point is that heat transfer in metal foams requires modeling the Local Thermal Non-Equilibrium (LTNE) [
3]. The usual model of LTNE in a fluid-saturated porous medium is through a two-temperature scheme where each phase, solid and fluid, has its own temperature field. Such temperature fields will be denoted as
and
, where
s and
f identify the solid and the fluid, respectively. At a given instant of time,
t, and position,
, the values of
and
differ in general. This means that, locally, there is an interphase heat transfer or, equivalently, a lack of local thermal equilibrium between the two phases. This model of LTNE presumes a macroscopic approach to the study of the fluid-saturated porous medium, where the adverb “locally” really means within a given Reference Elementary Volume (REV). A REV is that extremely small volume element where all physical fields (velocity, temperature, pressure) are assumed to be averaged [
6].
There is an evident conceptual difficulty in a theory based on a duality of temperature fields, namely the impossibility to measure the local values of either or . In fact, a thermometric probe such as a thermocouple placed anywhere inside the medium would unavoidably measure the local mean value of or . In other words, the phenomenological theory of LTNE based on the dual temperatures can be tested only by the correctness of its predictions rather than by the reliability of its basic assumptions.
The pair of temperature fields
satisfies the local energy balance equations [
6],
where the viscous dissipation effect is neglected, no internal heat sources are assumed to be present within the medium, and the properties of each phase are considered as temperature-independent. In Equations (3a) and (3b),
denotes the density of either the solid or the fluid,
and
the specific heats and the thermal conductivities of the two phases, while
h is the interphase heat transfer coefficient. The heat transfer between the two phases is expressed through the linear term
. Such a term embodies the condition of local thermal non-equilibrium and, in fact, it vanishes whenever the two fields
and
coincide,
. When this happens, we have the Local Thermal Equilibrium (LTE) and the temperature field
T satisfies the local energy balance equation,
Equation (4) is obtained by summing Equations (3a) and (3b), with
, and by defining
where
is the average thermal diffusivity of the fluid-saturated porous medium.
With LTNE, the local energy balance Equation (3) are coupled through the interphase heat transfer term. Following Vadasz [
9], we can resolve this coupling by determining
through Equation (3a),
and substituting it into Equation (3b), one obtains
where
and
Equation (7) is much more complicated than Equation (3a), being of higher order both in the time and in the spatial coordinates. On the other hand, Equation (7) has the advantage of displaying just one unknown temperature field,
. Moreover, Equation (7) includes a plethora of time and space lagging effects modulated by the relaxation times
and
as well as by the relaxation length
. Much in the same way, we can determine
by employing Equation (3b),
Then, we can substitute Equation (9) into Equation (3a) to obtain
Equations (7) and (10) are physically equivalent to Equations (3a) and (3b). However, unlike Equations (3a) and (3b), Equations (7) and (10) are uncoupled so that they can be solved separately, provided that either the boundary conditions or the initial conditions do not force a coupling between the fields and . Another interesting fact regarding the formulation given by Equations (7) and (10) is the focus on the time-relaxation and space-relaxation characteristics of LTNE, embodied by the parameters , and . These relaxation features do not emerge in Equations (3a) and (3b) where the nature of LTNE arises from the interphase heat transfer term, .
An important aspect of the two-temperature model of heat transfer in metal foams is the formulation of the temperature boundary conditions. There has been a thorough work on this subject carried out by Alazmi and Vafai [
23] and by Yang and Vafai [
24]. Two temperature fields have to be constrained at the boundaries, when such boundaries have either a given temperature distribution or a given heat flux distribution. A further complication may emerge if the boundary conditions are to describe external convection or finite thermal resistance, so that they must be expressed as Robin conditions. The simplest situation is when a boundary
is kept at a prescribed temperature distribution
. Then, the formal expression of the boundary conditions is
Equation (11) subsumes LTE at the boundary
. Modelling a prescribed heat input at
is much more tricky. While we can assume the validity of Fourier’s law for both fields
and
, it is unclear how the incoming heat flux is to be distributed among the solid medium and the fluid medium. Moreover, we have to produce two different boundary conditions as we have two local energy balance equations, i.e., Equations (3a) and (3b), which need to be conditioned at the boundary. The simplest case among the many envisaged by Vafai and coworkers [
23,
24] is one of an impermeable wall with a very large thermal conductivity. In this special case, the incoming heat flux distribution
yields the boundary conditions,
where
denotes the outward normal derivative. The rationale is that LTE holds at
and that the incoming heat flux distributes among the media
s and
f with weights given by the volume fractions of the two media,
and
. We do not go into further details for the alternatives to Equation (12). We refer the reader to the literature [
23,
24]. We just mention that Nield [
25] pointed out, in a short note, that some of these models of heat flux boundary conditions, as they stand, are conjectural and they need to be supported by experimental validation. Further considerations on this topic were made by Vadasz [
14]. A special case where there is no conceptual difficulty in the formulation of the heat flux boundary conditions is that of an adiabatic wall. In this case, the heat input at the boundary,
, is zero and this results into vanishing fluxes for both phases,
The use of Equations (7) and (10) instead of Equations (3a) and (3b) implies some further reflection regarding the boundary conditions. In fact, if Equations (3a) and (3b) are second-order PDEs in the coordinates
, Equations (7) and (10) are fourth-order. As a consequence, we have to set two constraints on
and two constraints on
at each boundary
. Let us assume the boundary conditions (11), then Equations (6) and (9) yield either
or
A similar argument can be claimed also for the initial conditions. In fact, Equations (3a) and (3b) are first-order in time so that one can state the initial conditions as
where
and
are prescribed functions expressing the temperature distributions at the initial time
. If we use the formulation of LTNE based on Equations (7) and (10), then the governing equations are second-order in time so that one needs two different initial conditions involving either
or
. Starting from Equation (16), this task can be accomplished by employing Equations (6) and (9). Then, we may write either
or
As noted by Vadasz [
9,
10,
11,
12], in the case of heat conduction
, Equations (7) and (10) are perfectly coincident, so that one can write
with
. Vadasz [
9,
12] also pointed out that the solutions for the fields
and
coincide if such fields are subjected to the same initial and boundary conditions. Physically, this means that LTE holds at
and at every boundary
, then LTE must hold at every time
and at every position
. Vadasz [
9,
12] suggests, by investigating a specific example, the apparently paradoxical nature of this finding when compared to the solution of Equations (3a) and (3b) subjected to the boundary conditions (11) and the initial conditions (16). An explanation of the apparent paradox is also proposed by the same author [
12].
A qualifying aspect of the LTNE model based on the dual temperature formulation is its experimental verification. There is not a wide literature reporting experimental tests of LTNE. As already pointed out above, a major difficulty in carrying out experiments is the direct measurement of the local values of
and
. Given this circumstance, the tests of LTNE are mainly focused on indirect measurements of the interphase heat transfer coefficient
h. The method adopted by Polyaev et al. [
26] is an indirect measurement of
h based on a solution for inverse heat transfer under unsteady conditions. The porous medium employed in such experiments is sintered stainless steel powder saturated by air. Results were reported in terms of an interphase Nusselt number,
, where
is the mean pore diameter. Values of
ranging from unity to approximately 14 were measured depending on the flow rate and on the porosity of the specimens [
26]. A thorough analysis of the literature on the experimental validations of LTNE can be found in Chapter 2 of the book by Nield and Bejan [
6].
5. Heat Transfer Model for Ideal Metal Foams
A metal foam is, as already stated, a porous medium that is widely employed for high-performance heat exchangers. The purpose of obtaining high heat transfer performances is achieved with more ease when the porous matrix has a high value of thermal conductivity. Particularly suitable for our analysis is pushing this configuration to the limit of an ideal case: a solid phase characterised by an extremely high value of thermal conductivity and a fluid phase characterised by a small, but finite, value of thermal conductivity. This limit yields that allows us to greatly simplify the heat transfer model (as presented later on in this section). Moreover, the assumption supports the choice of a two-temperature model especially when a heat exchanger undergoing forced convection is considered. A porous channel crossed by a forced flow with a temperature of the fluid different from the one of the solid, in fact, is expected to show an entrance region displaying local thermal non-equilibrium between the two phases.
With the aim of simplifying Equations (3a) and (3b), we employ the following relations to write the energy balance equations in a dimensionless form
where the tilde denotes the dimensionless quantities and
D is the hydraulic diameter. Equations (3a) and (3b) can thus be rewritten as
where the dimensionless parameters in Equation (22) are defined as follows:
The assumption of
just introduced yields
and
. This result allows us to neglect, in Equation (21a), the terms multiplied by
and
to obtain
The scaling defined by Equation (20) can now be employed to obtain a dimensional formulation of the energy balance equations, namely
Equation (24a) states that the porous matrix temperature is not affected by the presence of a fluid interacting with the solid. The porous matrix turns out to be a perfect heat source/sink for the fluid and this is, from the heat exchangers viewpoint, the ideal configuration.
When an entrance region in a porous channel with rectangular cross section is considered, one may obtain the temperature of the solid phase straight away by employing Equation (24a). We assume
x as the streamwise direction,
as the spanwise directions, and the following boundary conditions for the solid phase:
where
is the channel height and
is the channel width. A solution of the problem formed by Equations (24a) and (25) is a solid phase characterised by a constant temperature
. This result allows us to simplify Equation (24b) to
6. A CFD Analysis of Metal Foams with a Periodic Structure
Forced convection within metal foams can be studied numerically by solving the Navier-Stokes and Fourier equations in a computational domain represented by the region occupied by the fluid within the porous structure, under the assumption of constant temperature on the metal surface, as described in the previous section. The scope of the numerical approach is to obtain the velocity distribution within the flow domain and the heat transfer coefficient between the fluid and the metal surface. In general, the purpose is the validation of the volume-averaged model adopted to describe the momentum and energy transfer in the metal foam. Although the structure of typical metal foam, as shown in
Figure 1 is random and not easy to reproduce, some works evidenced a roughly periodic structure of the inner pores in many kinds of porous structures [
27]. A similarity between porous structures and some periodic structures such as triply periodic minimal surfaces (TPMS) has been recently suggested in the literature [
28]. These structures have a potential application in the field of metal foam heat exchangers also because they can be obtained by additive manufacturing 3D printing techniques. For these reasons, in this work a metal foam with a periodic structure has been considered. The porous structure has been built starting from a surface called gyroid, shown in
Figure 2, that is a combination of trigonometric functions such as:
where
a is the spatial periodicity of the function.
The fluid volume has been created starting from a fundamental surface unit shown in
Figure 3a, left. This unit has been copied and rotated by means of a computer aided design software (Siemens Nx) to obtain the periodic surface, as shown in
Figure 3a, right. Then, the periodic structure has been copied and translated to build the representative elementary volume employed for the volume-averaging of the physical fields (velocity, pressure, temperature). Such a representative elementary volume has a square section with dimensions
m and a length
m. The surface of the gyroid structure has been thickened to create a solid geometry representing the metal foam shown in
Figure 3b. The computational domain has been finally obtained by subtracting to the geometry of the representative elementary volume the metal foam volume (as shown in
Figure 3c).
The computational domain has been discretized to obtain an unstructured mesh with
elements by means of StarCCM+
software. The left surface in
Figure 3c is the inlet section of the flow, while the surfaces perpendicular to the inlet section has been set as symmetry planes. In the outlet section, perpendicular to the
x axis, a Neumann condition for the velocity and temperature, and a zero pressure condition are prescribed. The Navier-Stokes equations have been solved numerically for the laminar flow of water within the pores, together with the forced convection Fourier equation:
and
where
are the components of the velocity field,
T is the fluid temperature,
,
and
are respectively the density, dynamic viscosity and thermal diffusivity of the fluid. Six uniform velocities have been considered as inlet conditions (
0.001 m/s ÷ 0.006 m/s), each with three different fixed and uniform solid structure temperatures (
°C,
°C,
°C), while the inlet temperature of the flow has been set equal to 20 °C for each case. The Reynolds number ranges from 3.6 to 21.6, if the characteristic length is the spatial periodicity of the metal foam,
m.
6.1. CFD Results—Velocity Field
The cases considered represent a simultaneously-developing laminar flow.
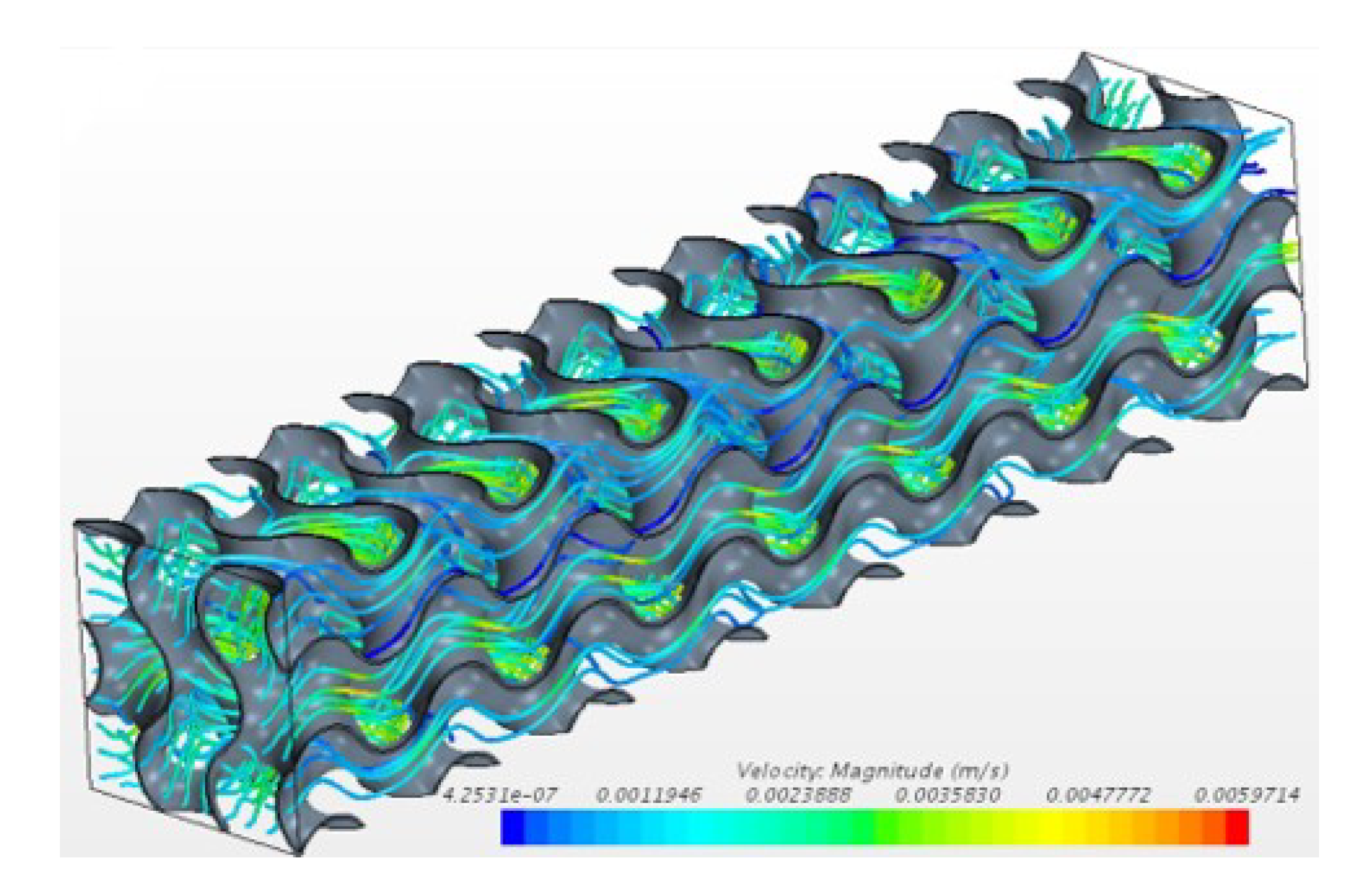
Figure 4 shows the streamlines obtained for the case
, coloured with the velocity magnitude. This case corresponds to a inlet velocity
m/s.
The figure shows winding paths within the pores, with a maximum velocity around
m/s, i.e., six times the inlet velocity.
Figure 5 shows the streamlines obtained for the same case on three planes at
,
and
.
The planes
and
cut the inner pores of the metal foam in the middle, while the plane
cuts the computational domain in the middle. The streamlines in the planes
and
evidence respectively separating and merging fluxes while wiggling in the direction
z, as shown by
Figure 6. The
Figure 5 and
Figure 6 evidence the symmetry of the velocity field under the exchange between the
y and
z coordinates.
Similar results are obtained for the cases with higher Re numbers, with a non-uniform velocity distribution that shows higher peaks as velocity increases, even in the developed flow region.
Figure 7 shows the velocity peaks obtained on different sections along the
x direction for the case
. This figure shows that the flow can be considered fully developed for
.
The pressure distribution is non-uniform as well.
Figure 8 shows the pressure obtained for the case
on the planes
,
and
.
By plotting the pressure gradient as a function of the inlet velocity one obtains
Figure 9.
Figure 9 shows that the trend is not linear. By fitting the data with the following function
where
K is the permeability and
is the Forchheimer coefficient one obtains
and
By using the permeability and the Forchheimer coefficient obtained from Equations (31) and (32) one obtains the following form drag coefficient
6.2. CFD Results—Temperature Field
The temperature distribution obtained reflects the velocity field shown in the previous section.
Figure 10 shows the temperature distribution obtained on the planes
and
for the case
. The temperature on the structure surface is
°C.
The figure shows that higher temperatures are reached where the velocity is lower. The computational data shows the observed mixing of the flow enhances the heat transfer within the metal foam. The temperature distribution on the plane
is shown in
Figure 11 for different inlet velocities and for a temperature on the porous structure
°C.
The figure shows that the length of the thermally developing region increases with the velocity. However, the Nusselt number shows that the flow is thermally developed in the region with
for all the cases. The interfacial heat transfer coefficient
(W/(m
2 K)) obtained in the thermally developed region and the volumetric heat transfer coefficient
h (W/(m
3 K)) described in Equation (26) are reported in
Table 1.
This result is in good agreement with the values reported in the literature for periodic structures such as Kelvin structure [
29].
Figure 12 shows the comparison of the volumetric heat transfer coefficient
h obtained in the present paper with the correlation obtained by Wu et al. for Kelvin structures [
30]:
where
is the cell characteristic length [
30],
is the fluid thermal conductivity and
is the porosity. In Equation (34), the Reynolds number has been evaluated with reference to the characteristic length
[
30].
These results show that periodic structures generated by a gyroid surface are characterised by heat transfer coefficients similar to those obtained for other periodic structures. The volumetric heat transfer coefficient obtained from local interface heat transfer coefficient gives a validation of the macroscopic averaged model, together with the LTNE assumption.