A Numerical Study of Spray Strips Analysis on Fridsma Hull Form
Abstract
:1. Introduction
2. Materials and Methods
2.1. Research Objects
2.2. Spray Strip Profile
2.3. Numerical Method
3. Results
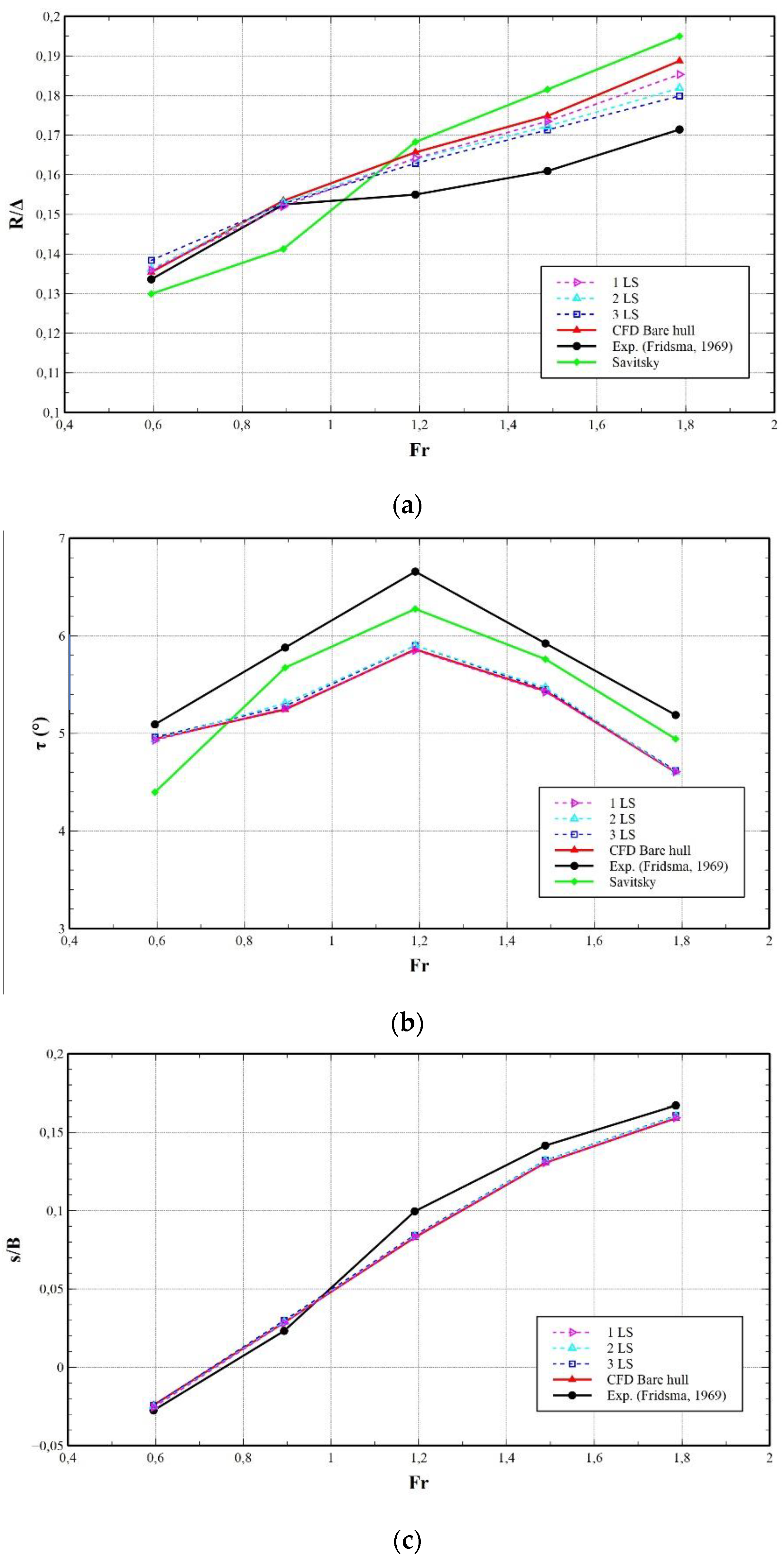
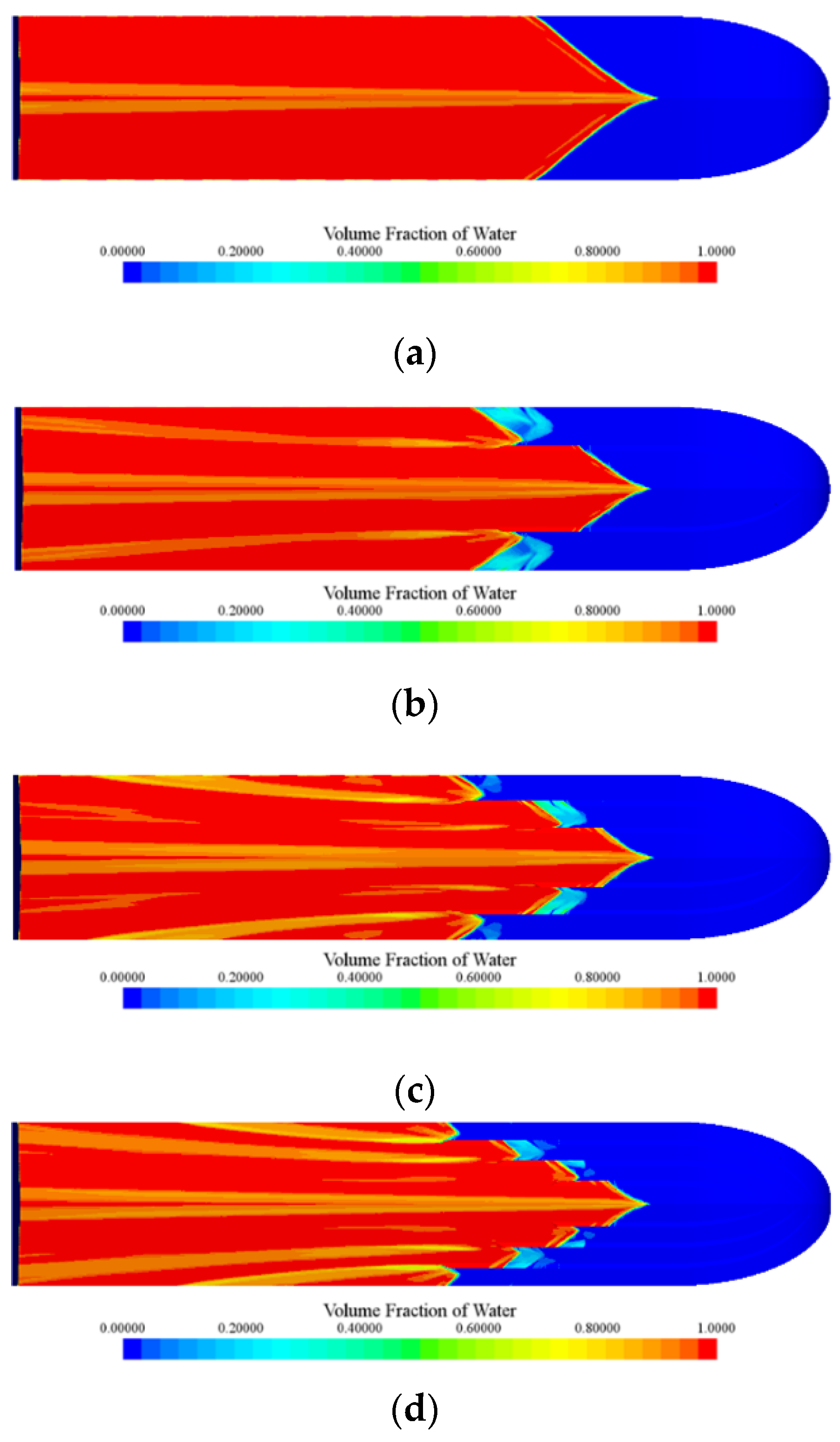
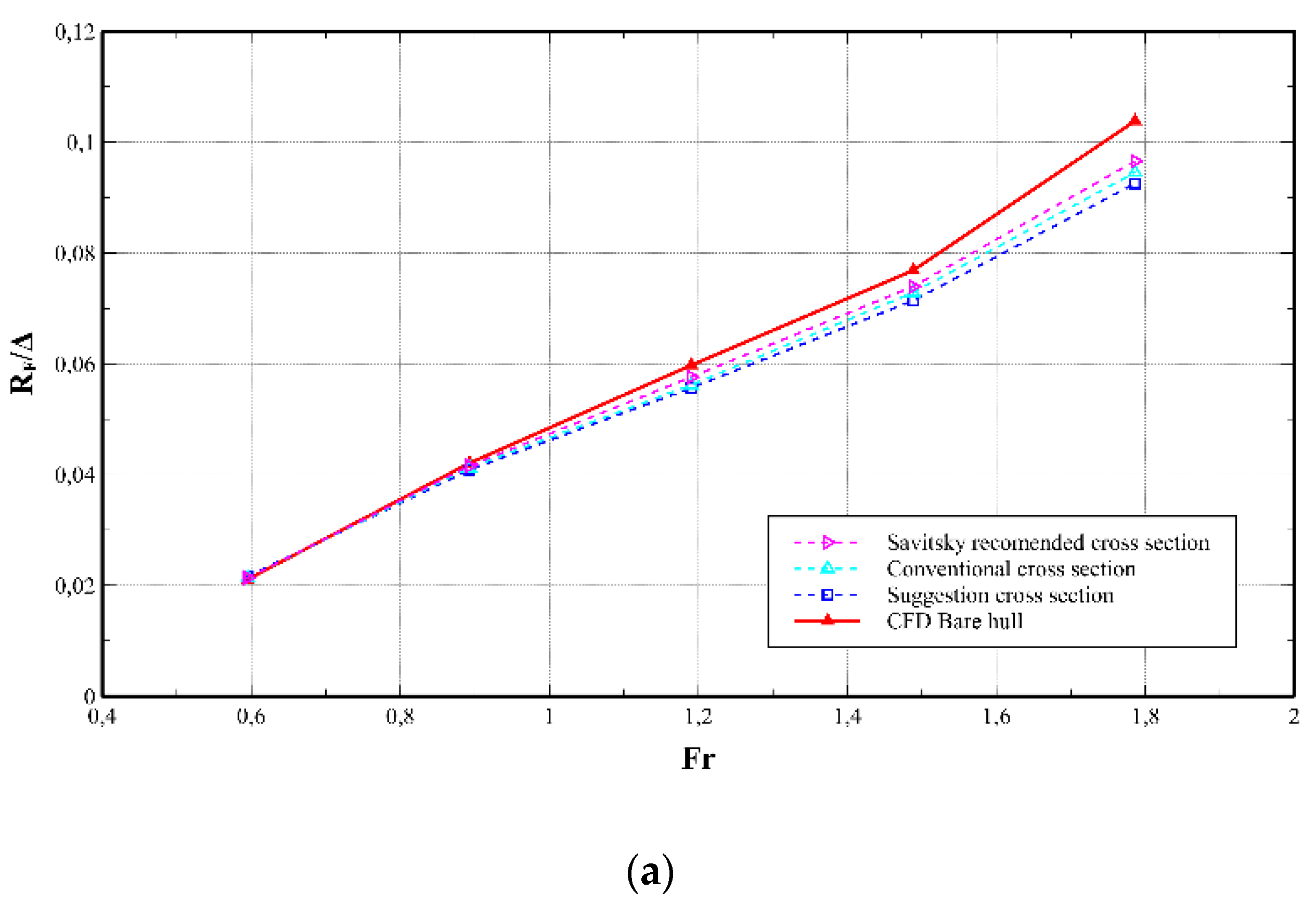
3.1. The Effect of the Number of Strips
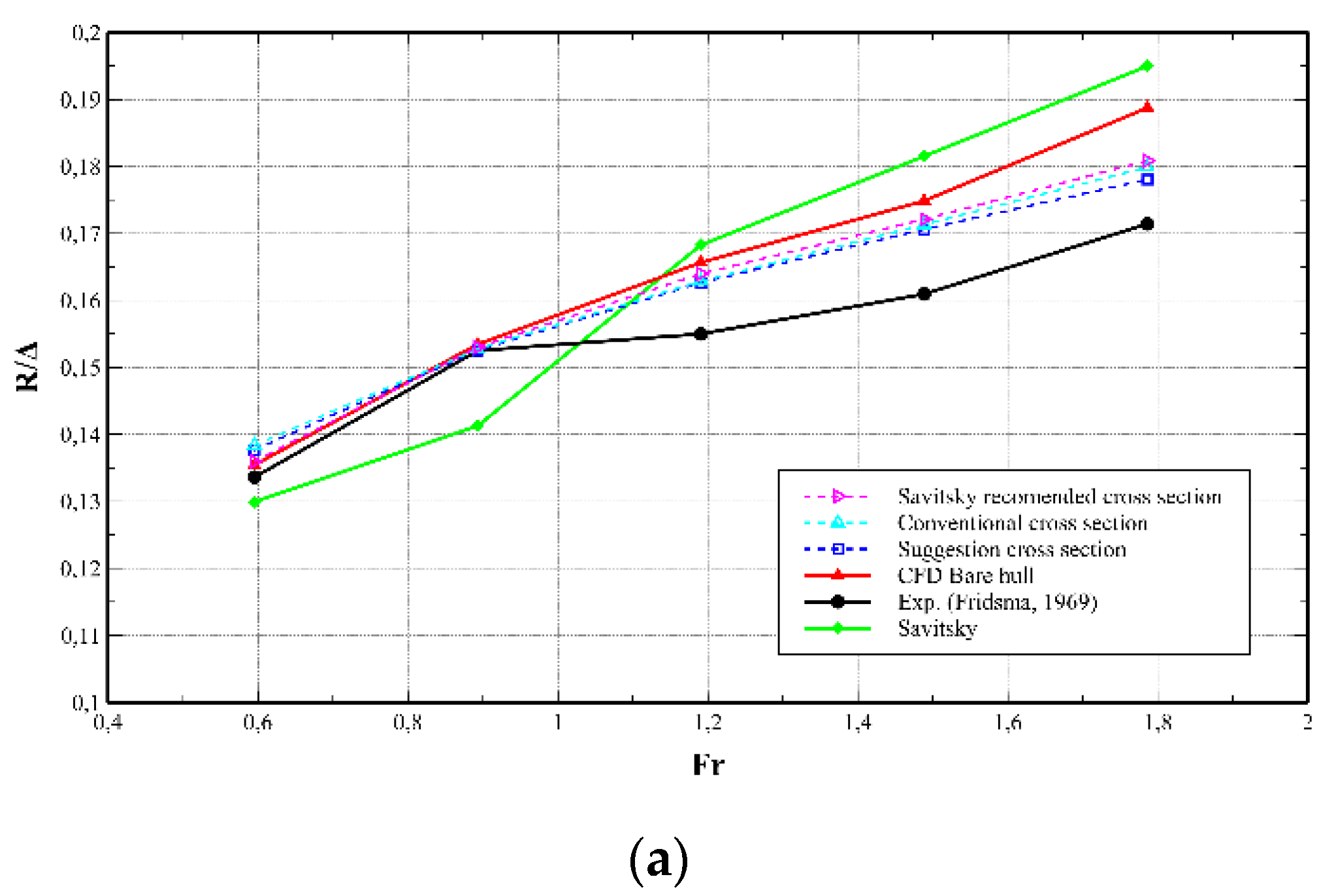
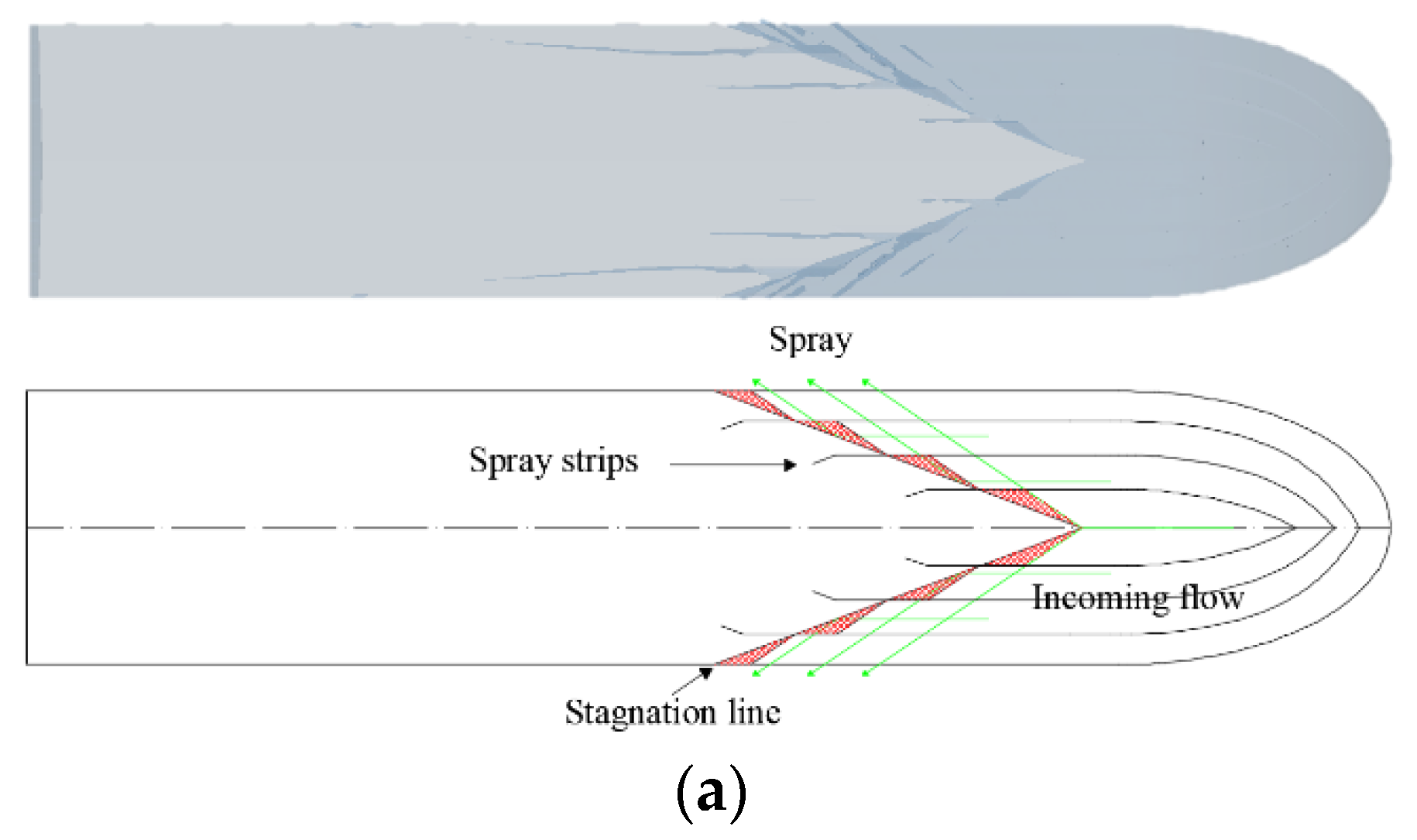
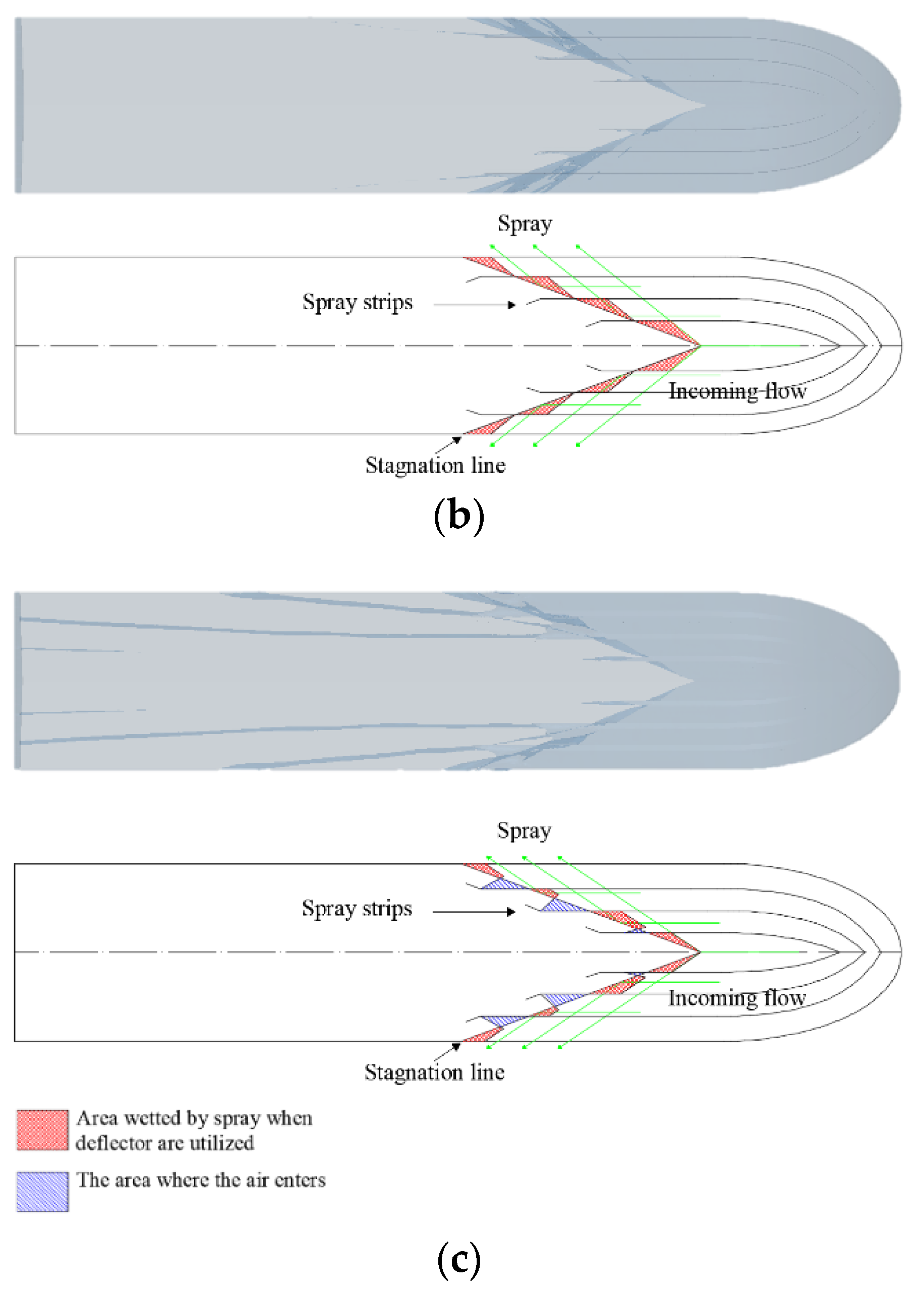
3.2. The Influence of Spray Strip Profile
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Savitsky, D.; DeLorme, M.F. Datla Inclusion of whisker spray drag in performance prediction method for high-speed planing hulls. Mar. Technol. 2007, 44, 35–56. [Google Scholar]
- Larsson, L. Ship Resistance and Flow; The Society of Naval Architects and Marine Engineers, the Principles of Naval Architecture Series: Jersey City, NJ, USA, 2010; ISBN 978-0-939773-76-3. [Google Scholar]
- Clement, E.P. Reduction of Planing Boat Resistance by Deflection of The Whisker Spray; Report No.1929; DTMB: Washington, DC, USA, 1964.
- Lakatos, M.; Sahk, T.; Andreasson, H.; Tabri, K.; Kõrgesaar, M. Spray Rail Performance in Off-Design Conditions. Sh. Boat Int. 2018, 5, 68–72. [Google Scholar]
- Molchanov, B.; Lundmark, S.; Fürth, M.; Green, M. Experimental validation of spray deflectors for high speed craft. Ocean Eng. 2019, 191, 1–9. [Google Scholar] [CrossRef]
- Castaldi, L.; Osmak, F.; Green, M.; Fürth, M.; Bonoli, J. The effect of spray deflection on the performance of high speed craft in calm water. Ocean Eng. 2021, 229. [Google Scholar] [CrossRef]
- Fridsma, G. A Systematic Study of The Rough-water Performance of Planning Boat; Report 1275; Davidson Laboratory, Stevens Institue of Technology: Hoboken, NJ, USA, 1969. [Google Scholar]
- Fu, T.C.; Brucker, K.A.; Mousaviraad, S.M.; Ikeda, C.M.; Lee, E.J.; O’Shea, T.T.; Wang, Z.; Stern, F.; Judge, C.Q. An Assessment of Computational Fluid Dynamics Predictions of the Hydrodynamics of High-Speed Planing Craft in Calm Water and Waves. In 30th Symposium on Naval Hydrodynamics; Hobart: Tasmania, Australia, 2014. [Google Scholar]
- Mousaviraad, S.M.; Wang, Z.; Stern, F. URANS studies of hydrodynamic performance and slamming loads on high-speed planing hulls in calm water and waves for deep and shallow conditions. Appl. Ocean Res. 2015, 51, 222–240. [Google Scholar] [CrossRef] [Green Version]
- Sukas, O.F.; Kinaci, O.K.; Cakici, F.; Gokce, M.K. Hydrodynamic assessment of planing hulls using overset grids. Appl. Ocean Res. 2017, 65, 35–46. [Google Scholar] [CrossRef]
- Samuel; Kim, D.J.; Fathuddiin, A.; Zakki, A.F. A Numerical Ventilation Problem on Fridsma Hull Form Using an Overset Grid System. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1096, 012041. [Google Scholar] [CrossRef]
- Avci, A.G.; Barlas, B. An experimental and numerical study of a high speed planing craft with full-scale validation. J. Mar. Sci. Technol. 2018, 26, 617–628. [Google Scholar] [CrossRef]
- Duan, X.; Sun, W.; Chen, C.; Wei, M.; Yang, Y. Numerical investigation of the porpoising motion of a seaplane planing on water with high speeds. Aerosp. Sci. Technol. 2019, 84, 980–994. [Google Scholar] [CrossRef]
- Savitsky, D. Hydrodynamic Design of Planing Hulls. Mar. Technolo SNAME 1964, 1, 71–95. [Google Scholar] [CrossRef]
- Savitsky, D.; Brown, P.W. Procedures for hydrodynamic evaluation of planing hulls in smooth and rough water. Mar. Technol. 1976, 13, 381–400. [Google Scholar] [CrossRef]
- Samuel; Iqbal, M.; Utama, I.K.A.P. An investigation into the resistance components of converting a traditional monohull fishing vessel into catamaran form. Int. J. Tech 2015, 6, 432–441. [Google Scholar] [CrossRef] [Green Version]
- Bilandi, R.N.; Dashtimanesh, A.; Tavakoli, S. Hydrodynamic study of heeled double-stepped planing hulls using CFD and 2D+T method. Ocean Eng. 2020, 196. [Google Scholar] [CrossRef]
- Tavakoli, S.; Niazmand Bilandi, R.; Mancini, S.; De Luca, F.; Dashtimanesh, A. Dynamic of a planing hull in regular waves: Comparison of experimental, numerical and mathematical methods. Ocean Eng. 2020, 217. [Google Scholar] [CrossRef]
- Bilandi, R.N.; Tavakoli, S.; Dashtimanesh, A. Seakeeping of double-stepped planing hulls. Ocean Eng. 2021, 236. [Google Scholar] [CrossRef]
- Olin, L.; Altimira, M.; Danielsson, J.; Rosén, A. Numerical modelling of spray sheet deflection on planing hulls. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2017, 231, 811–817. [Google Scholar] [CrossRef]
- Roshan, F.; Dashtimanesh, A.; Tavakoli, S.; Niazmand, R.; Abyn, H. Hull–propeller interaction for planing boats: A numerical study. Ships Offshore Struct. 2020, 1–13. [Google Scholar] [CrossRef]
- Bardina, J.E.; Huang, P.G.; Coakley, T.J. Turbulence Modeling Validation, Testing, and Development; Ames Research Center: California, CA, USA, 1997.
- Hosseini, A.; Tavakoli, S.; Dashtimanesh, A.; Sahoo, P.K.; Kõrgesaar, M. Performance prediction of a hard-chine planing hull by employing different cfd models. J. Mar. Sci. Eng. 2021, 9, 481. [Google Scholar] [CrossRef]
- Samuel. Fathuddiin Meshing Strategi untuk Memprediksi Hambatan Total pada Kapal Planing Hull. Rekayasa Mesin 2021, 12, 381–390. [Google Scholar]
- Wheeler, M.P.; Matveev, K.I.; Xing, T. Validation Study of Compact Planing Hulls at Pre-Planing Speeds. In Proceedings of the ASME 2018 5th Joint US-European Fluids Engineering Summer Conference, Montreal, QC, Canada, 15–20 July 2018; pp. 1–8. [Google Scholar]
- ITTC Practical Guidelines for Ship CFD Applications; 2011. Available online: https://ittc.info/media/1357/75-03-02-03.pdf (accessed on 31 August 2021).
- Star-CCM+ User guide star-CCM+. Version 13.02 2018. Available online: http://www.ata-plmsoftware.com/wp-content/uploads/2018/03/STAR-CCM_New_Features_List_v13.02_EN_smaller.pdf (accessed on 31 August 2021).
- Gray-Stephens, A.; Tezdogan, T.; Day, S. Strategies to minimise numerical ventilation in CFD simulations of high-speed planing hulls. Proc. Int. Conf. Offshore Mech. Arct. Eng. OMAE 2019, 2, 1–10. [Google Scholar] [CrossRef]
- Fotopoulos, A.G.; Margaris, D.P. Computational analysis of air lubrication system for commercial shipping and impacts on fuel consumption. Computation 2020, 8, 38. [Google Scholar] [CrossRef]
- Kim, D.J.; Kim, S.Y.; You, Y.J.; Rhee, K.P.; Kim, S.H.; Kim, Y.G. Design of high-speed planing hulls for the improvement of resistance and seakeeping performance. Int. J. Nav. Archit. Ocean Eng. 2013, 5, 161–177. [Google Scholar] [CrossRef] [Green Version]
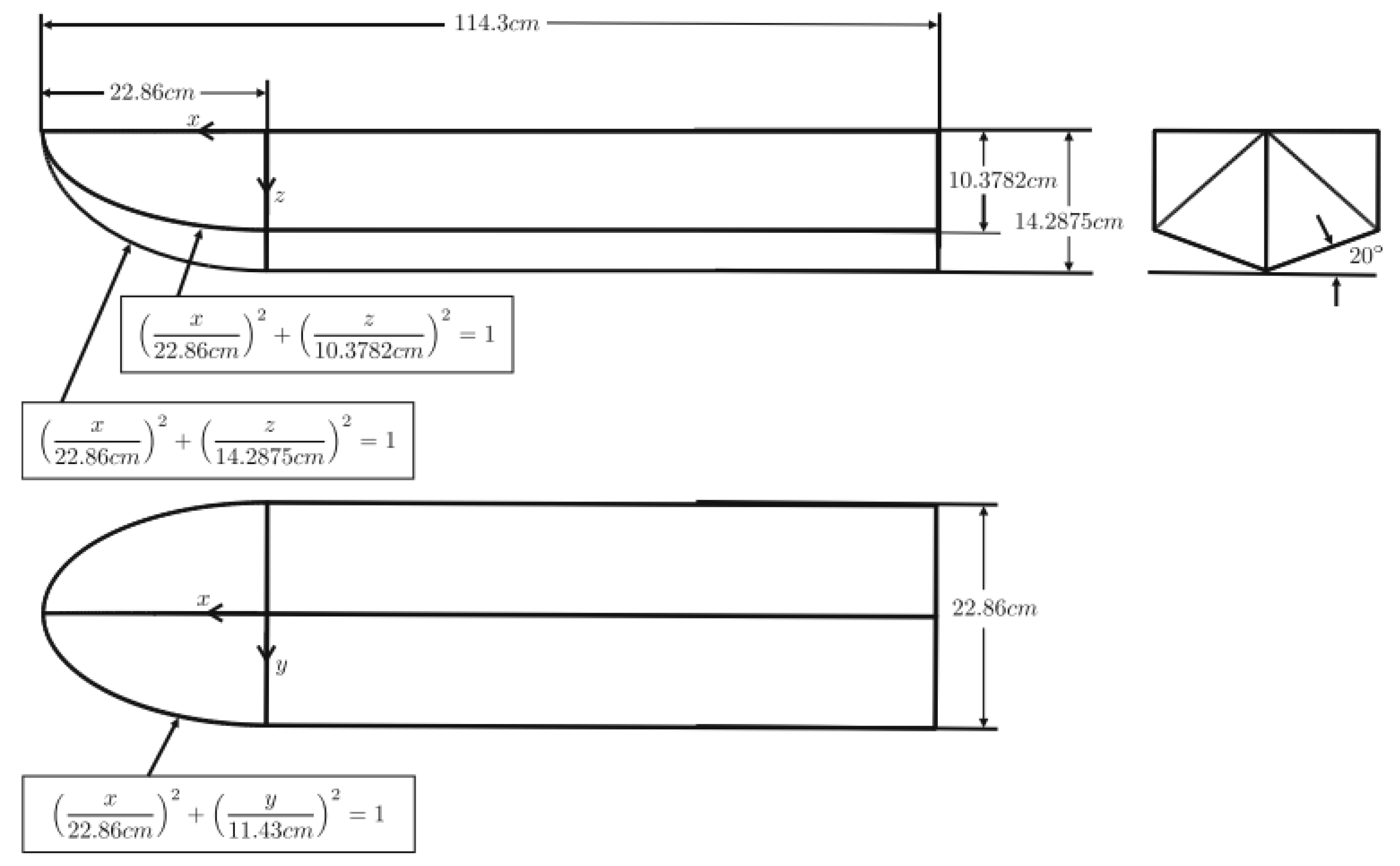


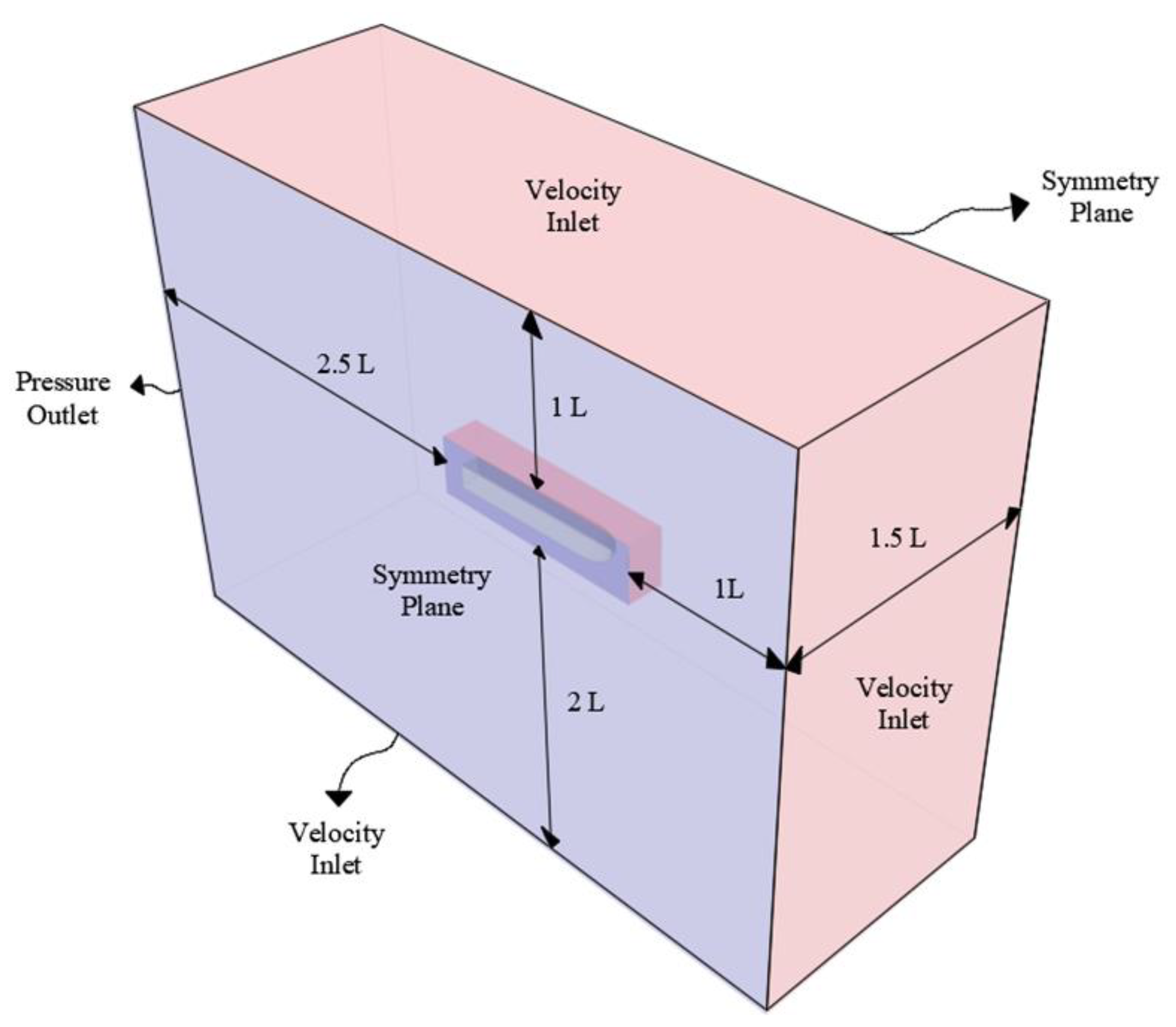
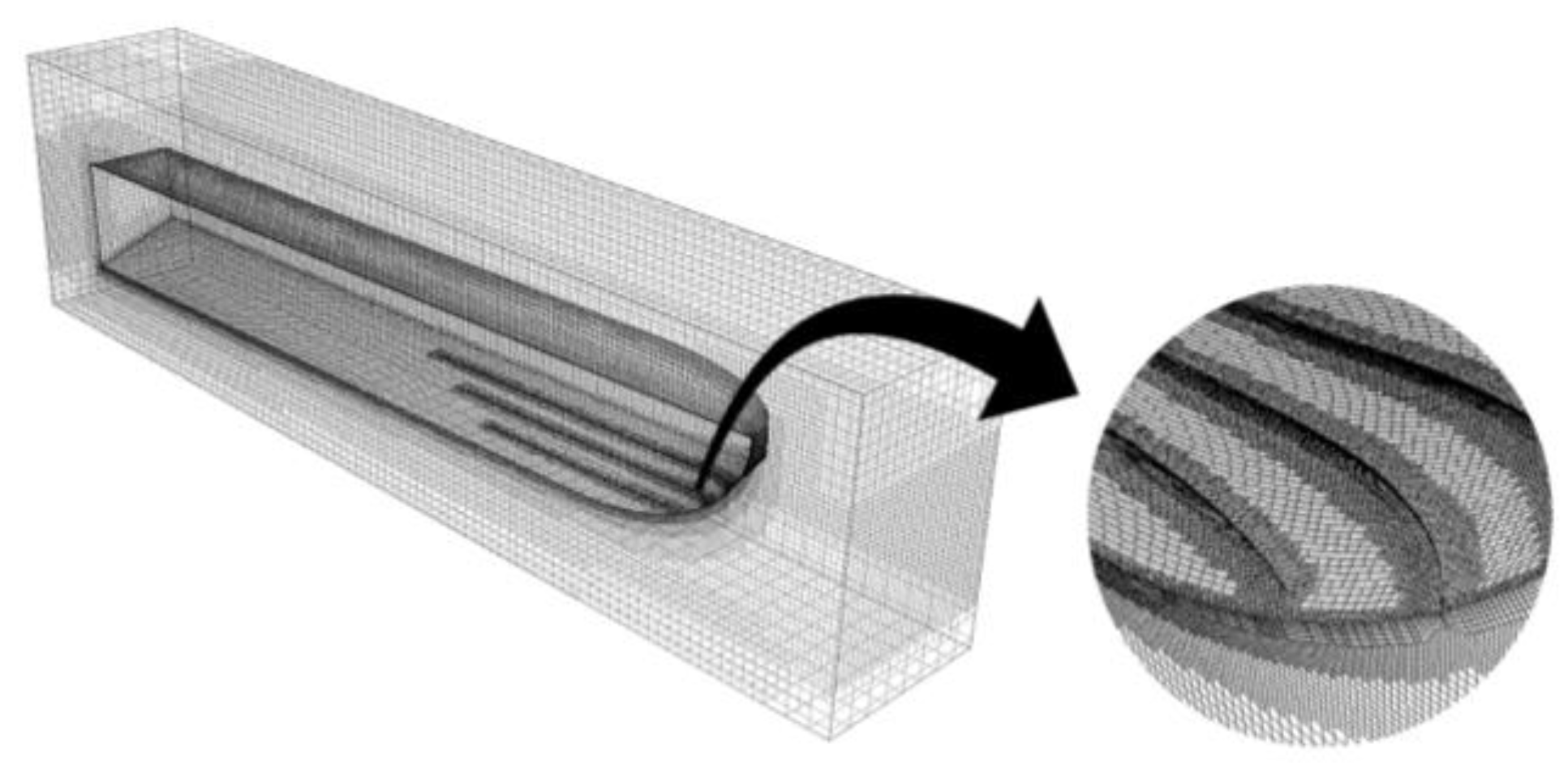
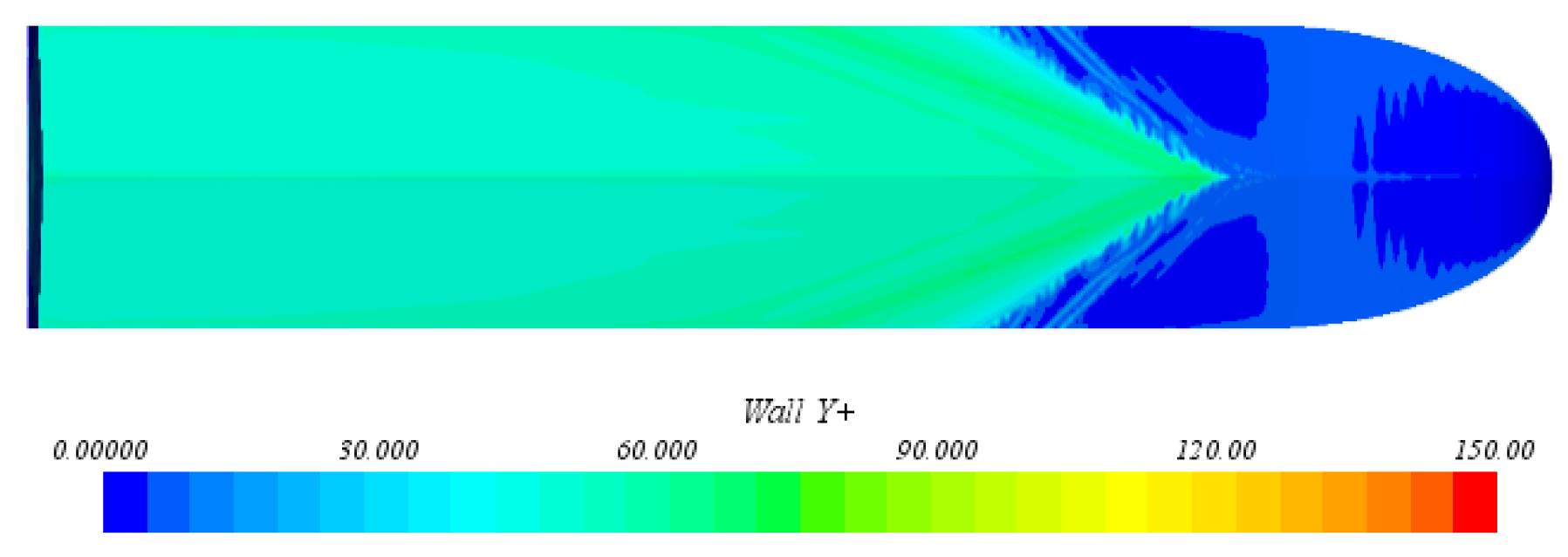






| Parameter | Unit | Value |
|---|---|---|
| L/B | - | 5 |
| L | m | 1.143 |
| B | m | 0.229 |
| TAP | m | 0.081 |
| LCG from AP | m | 0.457 |
| VCG from keel | m | 0.067 |
| τo | Degree | 1.569 |
| β | Degree | 20 |
| Δ | Kg | 10.890 |
| Iyy = Izz | Kg·m2 | 0.235 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samuel; Trimulyono, A.; Manik, P.; Chrismianto, D. A Numerical Study of Spray Strips Analysis on Fridsma Hull Form. Fluids 2021, 6, 420. https://doi.org/10.3390/fluids6110420
Samuel, Trimulyono A, Manik P, Chrismianto D. A Numerical Study of Spray Strips Analysis on Fridsma Hull Form. Fluids. 2021; 6(11):420. https://doi.org/10.3390/fluids6110420
Chicago/Turabian StyleSamuel, Andi Trimulyono, Parlindungan Manik, and Deddy Chrismianto. 2021. "A Numerical Study of Spray Strips Analysis on Fridsma Hull Form" Fluids 6, no. 11: 420. https://doi.org/10.3390/fluids6110420
APA StyleSamuel, Trimulyono, A., Manik, P., & Chrismianto, D. (2021). A Numerical Study of Spray Strips Analysis on Fridsma Hull Form. Fluids, 6(11), 420. https://doi.org/10.3390/fluids6110420






