Computational Approach for the Fluid-Structure Interaction Design of Insect-Inspired Micro Flapping Wings
Abstract
:1. Introduction
2. Fluid-Structure Interaction Design Approach
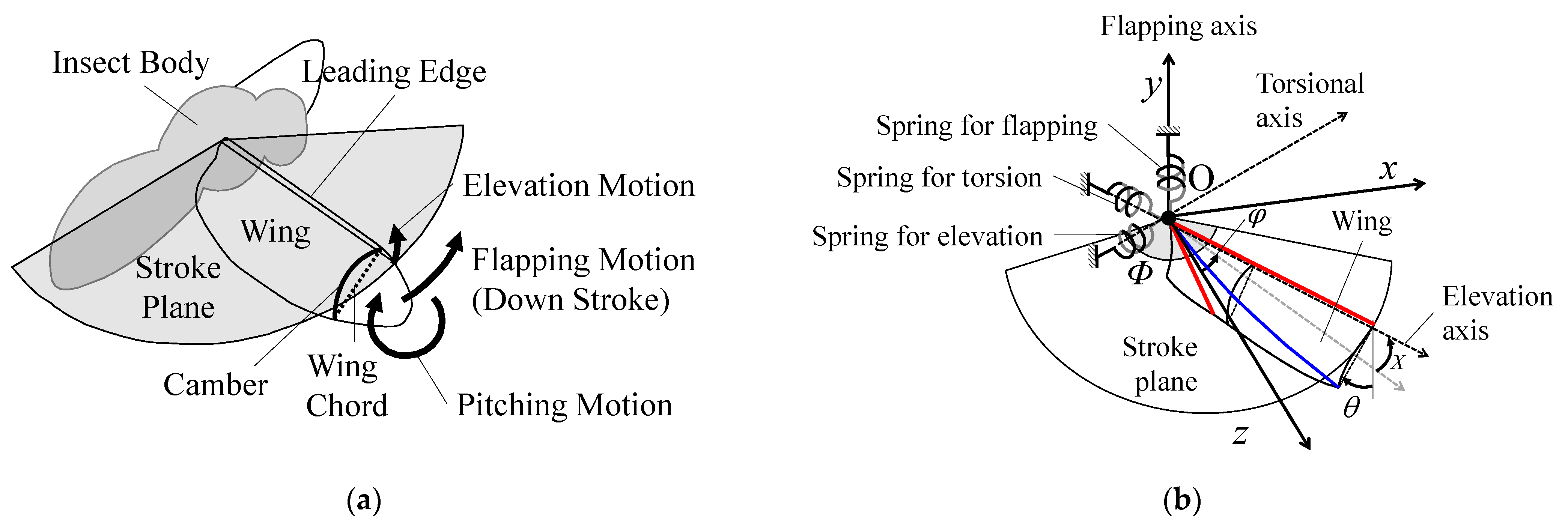
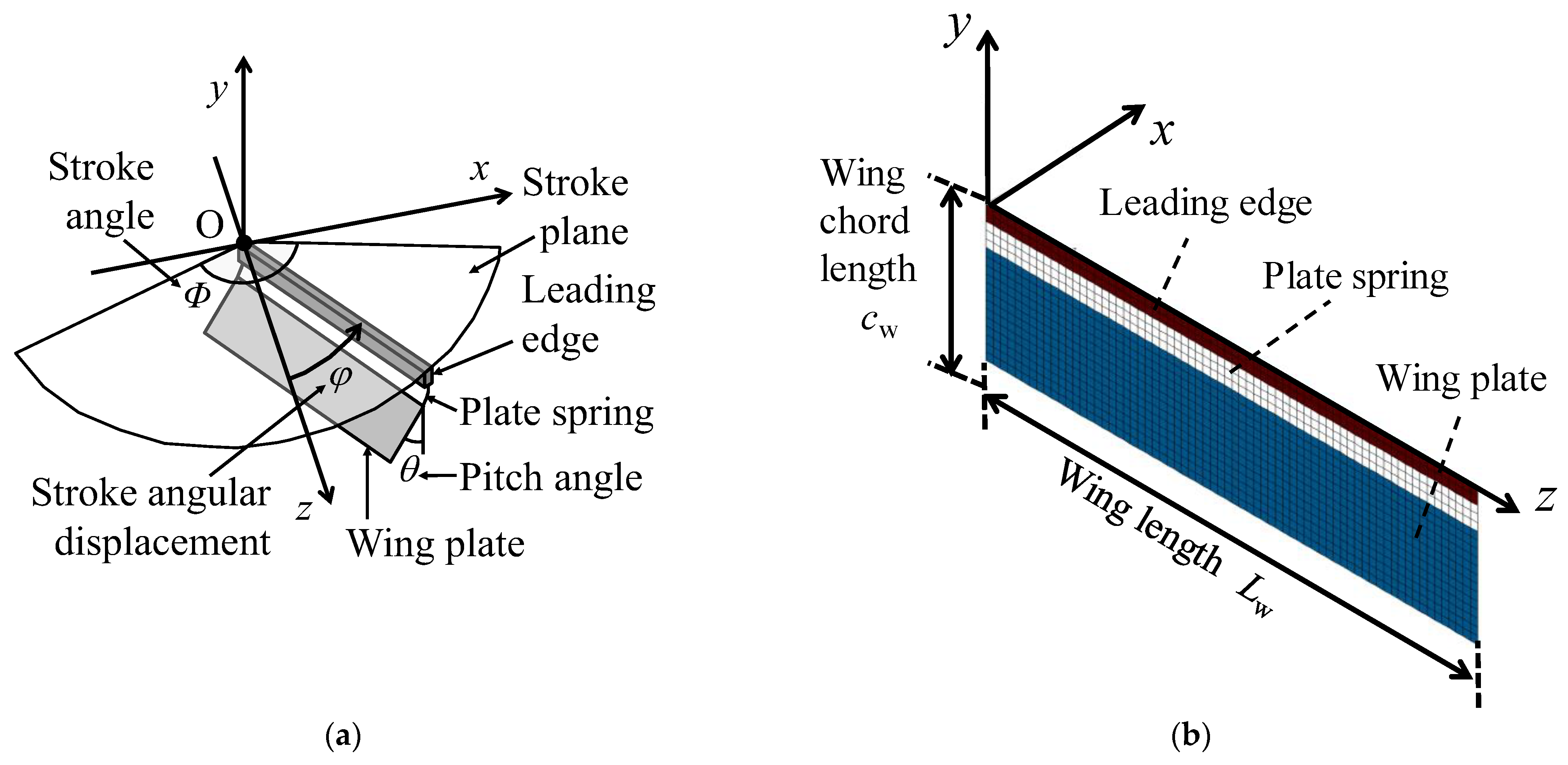
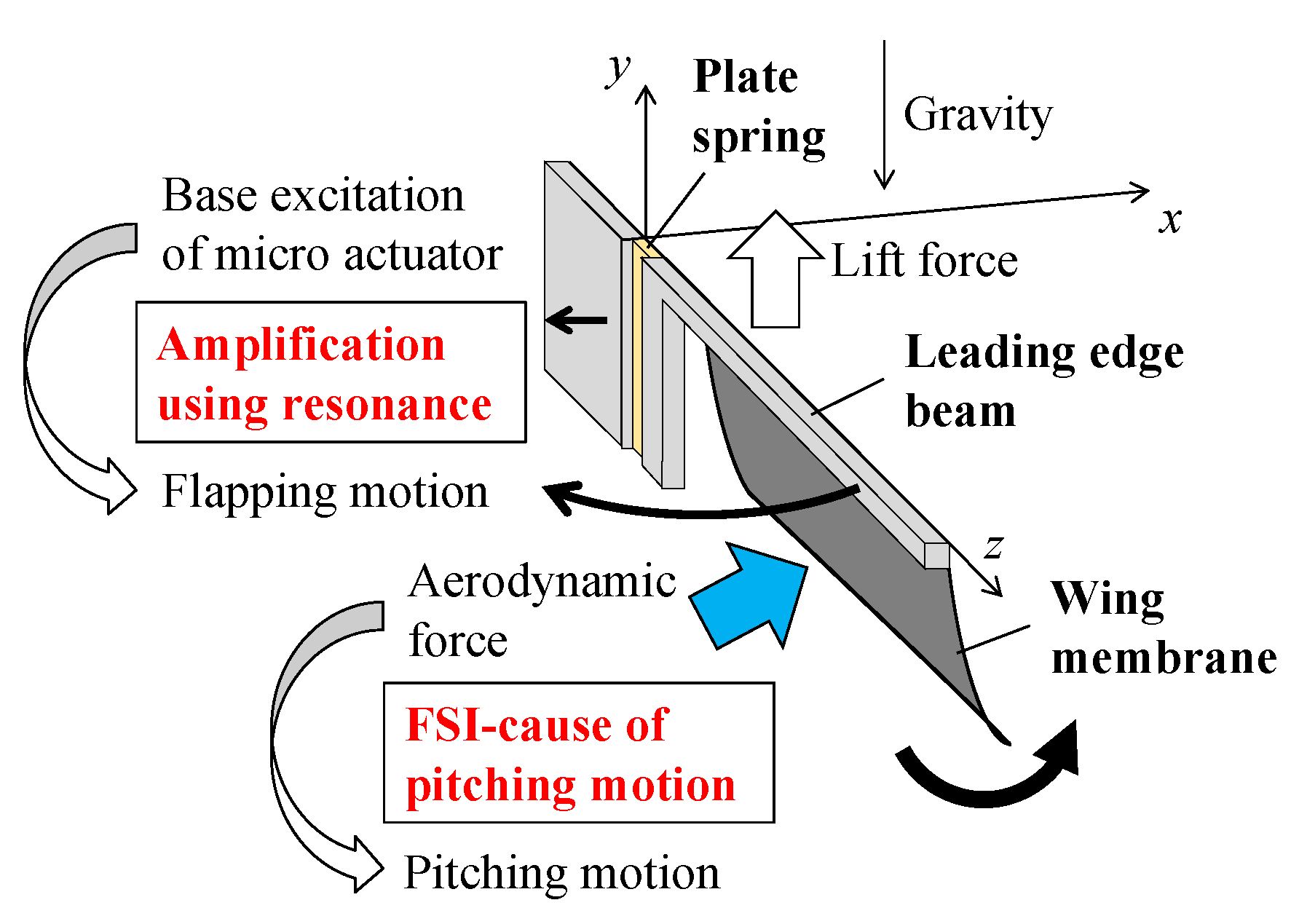
2.1. Model Wing for the Fluid–Structure Interaction Design
2.2. Fluid–Structure Interaction Analysis
2.2.1. Governing Equations for the FSI
2.2.2. Monolithic Equation System for the FSI
2.2.3. Projection Method Using the Algebraic Splitting
2.2.4. Parallel Computation
- Step 1. The matrix–vector product (15) is independently computed at Pi (i = 1, 2, …, Nd) using the element-by-element method aswhere A denotes the global matrix, p denotes the global vector, q denotes their matrix–vector product, A(e) and p(e) are their elemental counterparts, respectively, and e denotes the element number.
- Step 2. The nodal data of the matrix–vector product (16) on the interface between ΩFi and ΩFj (j ≠ i) computed at Pj is transferred to Pi and added to complete the corresponding nodal data computed in Step 1.
2.2.5. Shell Modeling of the Thin Elastic Wing
2.3. Dynamic Similarity Framework for the FSI
2.4. Design Window (DW) Search
3. Numerical Test for Dynamic Similarity Framework
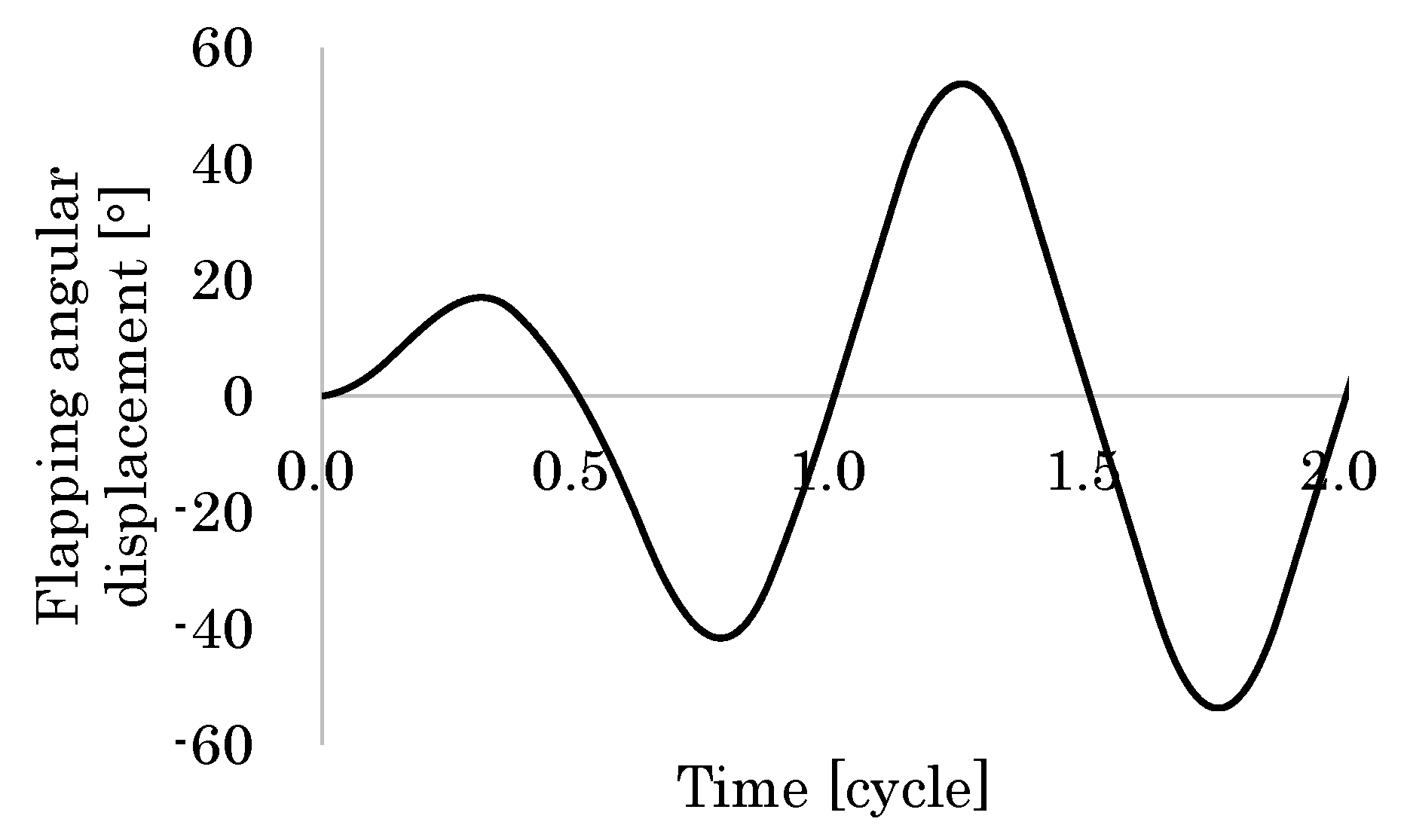
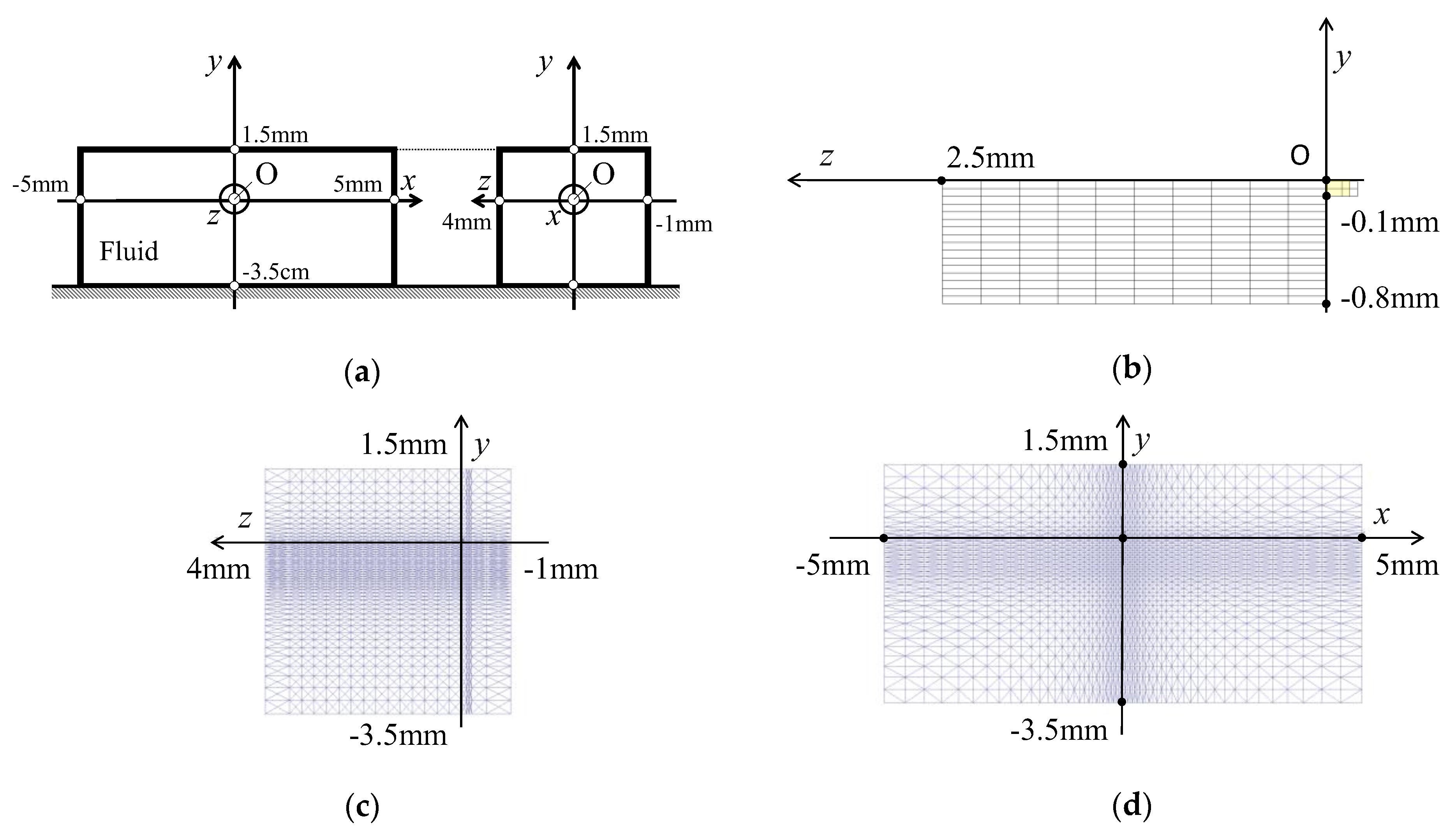
3.1. Problem Setup
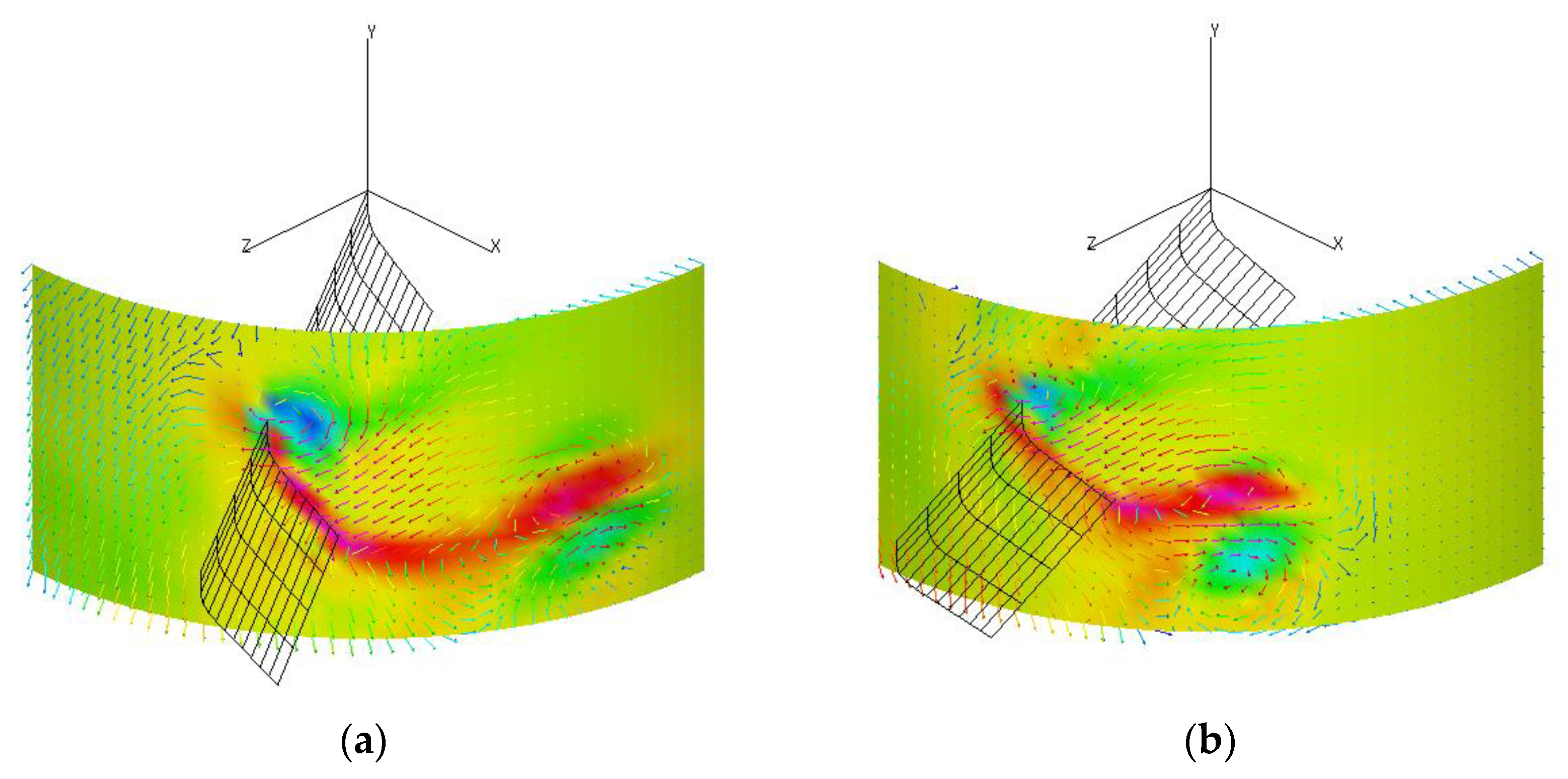
3.2. Results and Discussion
4. Effect of Flapping Frequency on Passive Wing Motion
4.1. Problem Setup
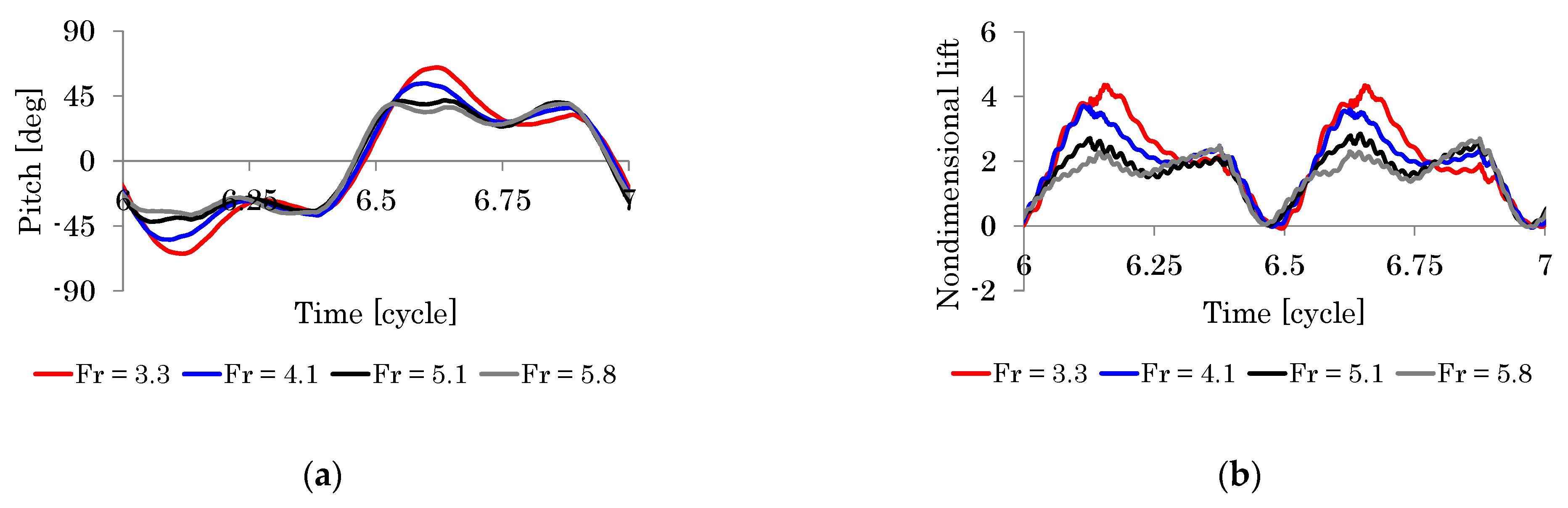
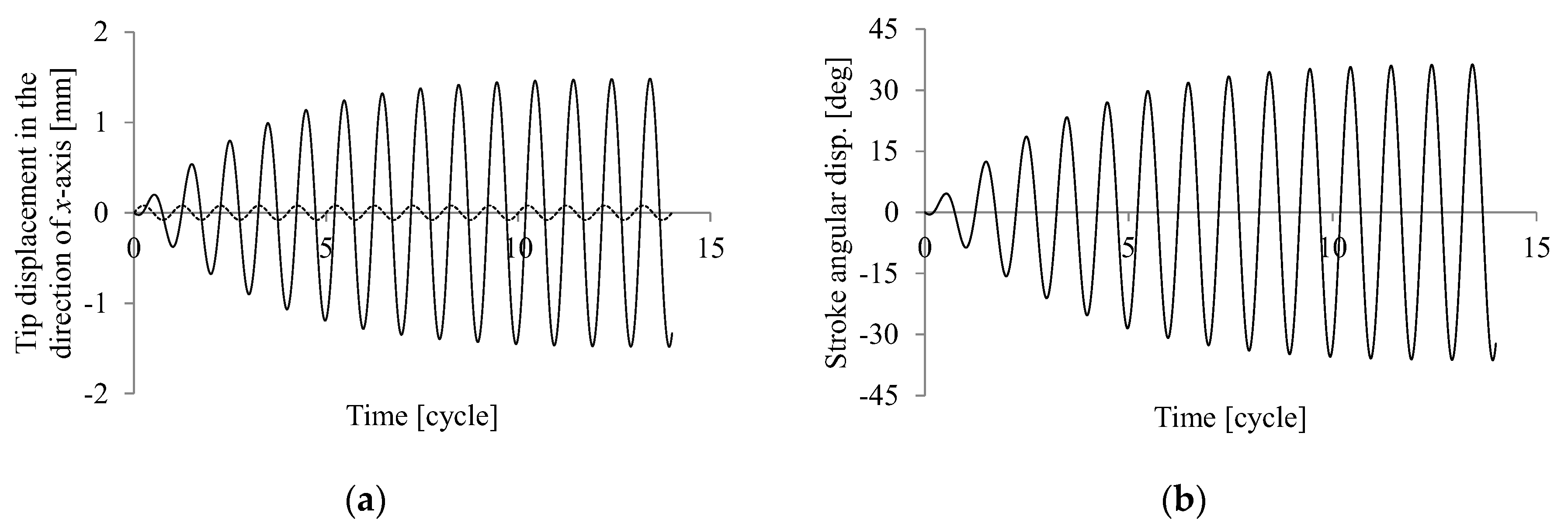
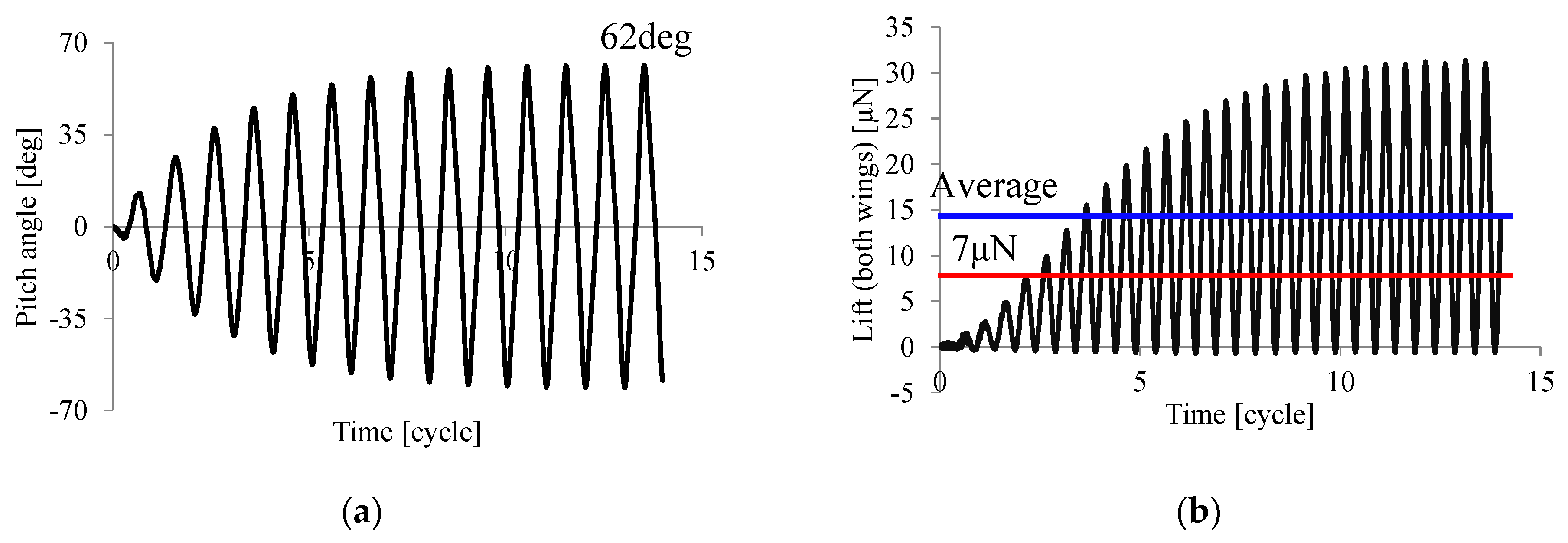
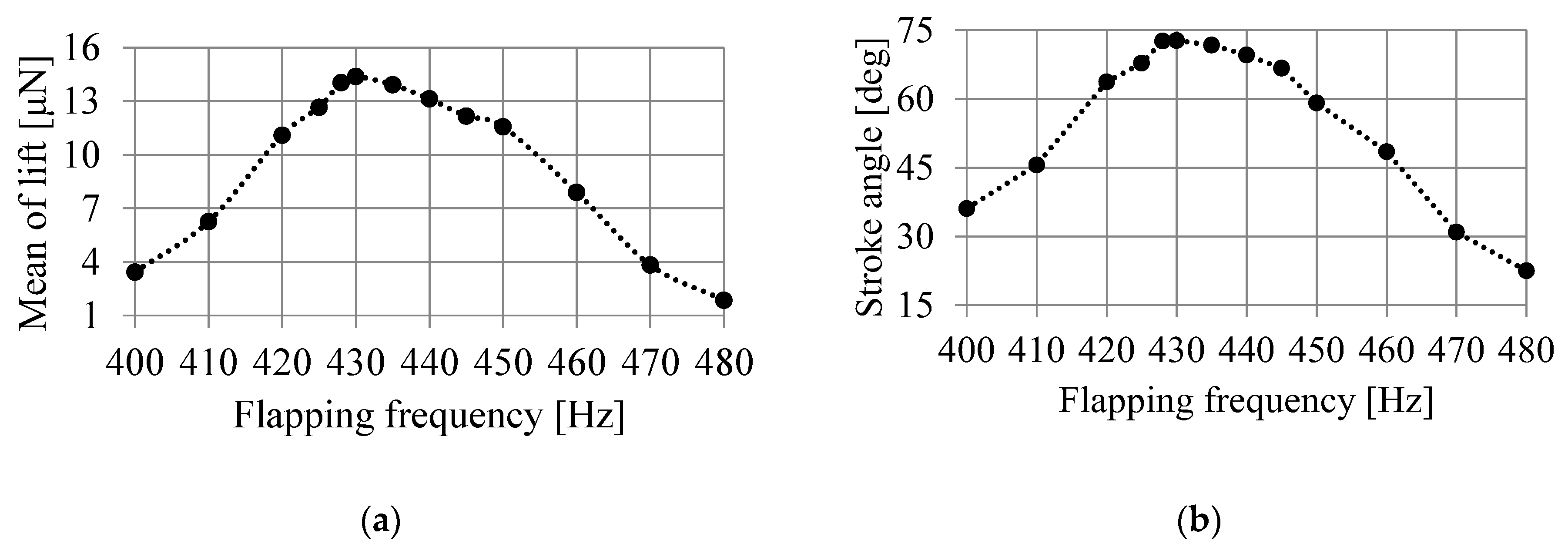
4.2. Results and Discussion
5. Micro Flapping Wing with 2.5-D Structure
5.1. Problem Setup
5.2. Results and Discussion
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Azuma, A. The Biokinetics of Flying and Swimming; AIAA Education Series; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2005. [Google Scholar]
- Dudley, R. The Biomechanics of Insect Flight: Form, Function, Evolution; Princeton University Press: Princeton, NJ, USA, 2002. [Google Scholar]
- Brodsky, A.K. The Evolution of Insect Flight; Oxford Science Publication: Oxford, UK, 1994. [Google Scholar]
- Liu, H.; Nakata, T.; Li, G.; Kolomenskiy, D. Biomechanics and biomimetics in flying and swimming. In Industrial Biomimetics; Jenny Stanford Publishing: Dubai, United Arab Emirates, 2019; pp. 29–80. [Google Scholar]
- Liu, H.; Ravi, S.; Kolomenskiy, D.; Tanaka, H. Biomechanics and biomimetics in insect-inspired flight systems. Philos. Trans. R. Soc. 2016, B371, 20150390. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.; Rossi, C. A review of compliant transmission mechanisms for bio-inspired flapping-wing micro air vehicles. Bioinspir. Biomim. 2017, 12, 025005. [Google Scholar] [CrossRef]
- Ishihara, D.; Murakami, S.; Ohira, N.; Ueo, J.; Takagi, M.; Urakawa, K.; Horie, T. Polymer micromachined transmission for insect-inspired flapping wing nano air vehicles. In Proceedings of the 15th Annual IEEE International Conference on Nano/Micro Engineered and Molecular Systems, San Diego, CA, USA, 27–30 September 2020; pp. 27–30. [Google Scholar]
- Rashmikant; Ishihara, D.; Suetsugu, R.; Ramegowda, P.C. One-wing polymer micromachined transmission for insect-inspired flapping wing nano air vehicles. Eng. Res. Express 2021, 3, 045006. [Google Scholar] [CrossRef]
- Pringle, J.W.S. Insect Flight; Cambridge University Press: Cambridge, UK, 1957. [Google Scholar]
- Ishihara, D.; Jeong, M.J.; Yoshimura, S.; Yagawa, G. Design window search using continuous evolutionary algorithm and clustering –its application to shape optimization of microelectrostatic actuator. Comput. Struct. 2002, 80, 2469–2481. [Google Scholar] [CrossRef]
- Ellington, C.P. The aerodynamics of hovering insect flight. III. Kinematics. Philos. Trans. R. Soc. 1984, B305, 41–78. [Google Scholar]
- Dickinson, M.H.; Lehmann, F.-O.; Sane, P.S. Wing rotation and the aerodynamic basis of insect flight. Science 1999, 284, 1954–1960. [Google Scholar] [CrossRef] [PubMed]
- Wootton, R.J. Support and deformability in insect wings. J. Zool. Lond. 1981, 193, 447–468. [Google Scholar] [CrossRef]
- Ennos, A.R. The importance of torsion in the design of insect wings. J. Exp. Biol. 1988, 140, 137–160. [Google Scholar] [CrossRef]
- Ennos, A.R. The inertial cause of wing rotation in Diptera. J. Exp. Biol. 1988, 140, 161–169. [Google Scholar] [CrossRef]
- Ennos, A.R. Inertial and aerodynamic torques on the wings of Diptera in flight. J. Exp. Biol. 1989, 142, 87–95. [Google Scholar] [CrossRef]
- Combes, S.A.; Daniel, T.L. Flexural stiffness in insect wings I. Scaling and the influence of wing venation. J. Exp. Biol. 2003, 206, 2979–2987. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Ma, T.; Ning, J.; Gorb, S. Structure and tensile properties of the forewing costal vein of the honeybee Apis mellifera. Soft Matter 2020, 16, 4057–4064. [Google Scholar] [CrossRef] [PubMed]
- Ning, J.G.; Ma, Y.; Ren, H.L.; Zhang, P.F. Investigation of span-chordwise bending anisotropy of honeybee forewings. Biol. Open 2017, 6, 619–624. [Google Scholar] [CrossRef] [Green Version]
- Ennos, A.R. The kinematics and aerodynamics of the free flight of some Diptera. J. Exp. Biol. 1989, 142, 49–85. [Google Scholar] [CrossRef]
- Miller, L.A.; Peskin, C.S. A computational fluid dynamics of ‘clap and fling’ in the smallest insects. J. Exp. Biol. 2005, 208, 195–212. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Young, J.; Walker, S.M.; Bomphery, R.J.; Taylor, G.K.; Thomas, A.L.R. Details of insect wing design and deformation enhance aerodynamic function and flight efficiency. Science 2009, 325, 1549–1552. [Google Scholar] [CrossRef] [PubMed]
- Du, G.; Sun, M. Effects of wing deformation on aerodynamic forces in hovering hoverflies. J. Exp. Biol. 2010, 213, 2273–2283. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zheng, L.; Hedrick, T.L.; Mittal, R. Time-varying wing-twist improves aerodynamic efficiency of forward flight in butterflies. PLoS ONE 2013, 8, e53060. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nakata, T.; Noda, R.; Liu, H. Effect of twist, camber and spanwise bending on the aerodynamic performance of flapping wings. J. Biomech. Sci. Eng. 2018, 13, 17–00618. [Google Scholar] [CrossRef] [Green Version]
- Ishihara, D.; Horie, T.; Denda, M. A two-dimensional computational study on fluid–structure interaction cause of wing pitch changes in dipteran flapping flight. J. Exp. Biol. 2009, 212, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Nakata, T.; Liu, H. A fluid–structure interaction model of insect flight with flexible wings. J. Comput. Phys. 2012, 231, 1822–1847. [Google Scholar] [CrossRef]
- Hua, R.; Zhu, L.; Lu, X. Locomotion of a flapping flexible plate. Phys. Fluids 2013, 25, 121901. [Google Scholar] [CrossRef]
- Ishihara, D.; Horie, T.; Niho, T. An experimental and three–dimensional computational study on the aerodynamic contribution to the passive pitching motion of flapping wings in hovering flies. Bioinspir. Biomim. 2014, 9, 046009. [Google Scholar] [CrossRef] [PubMed]
- Ishihara, D.; Horie, T. Passive mechanism of pitch recoil in flapping insect wings. Bioinspir. Biomim. 2017, 12, 016008. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shahzad, A.; Tian, F.-B.; Young, J.; Lai, J.C.S. Effect of hawkmoth-like flexibility on the aerodynamic performance of flapping on the performance of flapping wings with difference shapes and aspect ratios. Phys. Fluids 2018, 30, 091902. [Google Scholar] [CrossRef]
- Ishihara, D. Role of fluid-structure interaction in generating the characteristic tip path of a flapping flexible wing. Phys. Rev. E 2019, 98, 032411. [Google Scholar] [CrossRef]
- Xiao, Q.; Hu, J.; Liu, H. Effect of torsional stiffness and inertia on the dynamics of low aspect ratio flapping wings. Bioinspir. Biomim. 2014, 9, 016008. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ishihara, D.; Yamashita, Y.; Horie, T.; Yoshida, S.; Niho, T. Passive maintenance of high angle of attack and its lift generation during flapping translation in crane fly wing. J. Exp. Biol. 2009, 212, 3882–3891. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ishihara, D.; Ohira, N.; Takagi, M.; Murakami, S.; Horie, T. Fluid-structure interaction design of insect-like micro flapping wing. In Proceedings of the 7th International Conference on Computational Methods for Coupled Problems in Science and Engineering, Rhodes, Greece, 12–14 June 2017; pp. 870–875. [Google Scholar]
- Wood, R.J. The first takeoff of a biologically inspired at-scale robotic insect. IEEE Trans. Robot. 2008, 24, 341–347. [Google Scholar] [CrossRef]
- Bathe, K.J. Finite Element Procedures; Prentice-Hall: Hoboken, NJ, USA, 1996. [Google Scholar]
- Ishihara, D.; Yoshimura, S. A monolithic method for fluid–shell interaction based on consistent pressure Poisson equation. Int. J. Numer. Methods Eng. 2005, 64, 167–203. [Google Scholar] [CrossRef]
- Ishihara, D.; Horie, T. A projection method for the interaction of an incompressible fluid and a structure using new algebraic splitting. Comput. Modeling Eng. Sci. 2014, 101, 421–440. [Google Scholar]
- Murakami, S.; Ishihara, D.; Araki, M.; Horie, T.; Ohira, N.; Ito, T. Microfabrication of hybrid structure composed of rigid silicon and flexible polyimide membranes. Micro Nano Lett. 2017, 12, 913–915. [Google Scholar] [CrossRef]
- Tanaka, H.; Wood, R.J. Fabrication of corrugated artificial insect wings using laser micromachined molds. J. Micromech. Microeng. 2010, 20, 075008. [Google Scholar] [CrossRef] [Green Version]
- Wootton, R.J. Functional morphology of insect wings. Annu. Rev. Entomol. 1992, 37, 113–140. [Google Scholar] [CrossRef]
- Rees, C.J.C. Form and functions in corrugated insect wings. Nature 1975, 256, 200–203. [Google Scholar] [CrossRef]
- Sunada, S.; Zeng, L.; Kawachi, K. The relationship between dragonfly wing structure and torsional deformation. J. Theor. Biol. 1998, 193, 39–45. [Google Scholar] [CrossRef]
- Wang, X.S.; Li, Y.; Shi, Y.F. Effects of sandwich microstructures on mechanical behaviors of dragonfly wing vein. Compos. Sci. Technol. 2008, 68, 186–192. [Google Scholar] [CrossRef]
- Walker, S.M.; Thomas, A.L.R.; Taylor, G.K. Deformable wing kinematics in free-flying hoverflies. J. R. Soc. Interface 2010, 7, 131–142. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nalbach, G. The gear change mechanism of the blowfly (Calliphora erythrocephala) in tethered flight. J. Comp. Physiol. 1989, A165, 321–331. [Google Scholar] [CrossRef]
- Dickinson, M.H.; Tub, M.S. The function of dipteran flight muscle. Comp. Biochem. Physiol. 1997, 116A, 223–238. [Google Scholar] [CrossRef]
- Balint, C.N.; Dickinson, M.H. Neuromuscular control of aerodynamic forces and moments in the blowfly, Calliphora vicina. J. Exp. Biol. 2004, 207, 3813–3838. [Google Scholar] [CrossRef] [Green Version]
- Walker, S.M.; Thomas, A.L.R.; Taylor, G.K. Operation of the alula as an indicator of gear change in hoverflies. J. R. Soc. Interface 2012, 9, 1194–1207. [Google Scholar] [CrossRef] [Green Version]
- Wootton, R.J.; Herbert, R.C.; Young, P.G.; Evans, K.E. Approaches to the structural modelling of insect wings. Philos. Trans. R. Soc. Lond. 2003, B358, 1577–1587. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ishihara, D.; Yokota, J.; Onishi, M.; Niho, T.; Horie, T. A Shape simplification modeling of the cambering in insect’s flapping wings using beam and shell. Trans. Jpn. Soc. Comput. Eng. Sci. 2018, 2018, 20180018. [Google Scholar]
- Onishi, M.; Ishihara, D. Modeling the cambering of the flapping wings of an insect using rectangular shell finite elements. J. Adv. Simul. Sci. Eng. 2020, 7, 181–188. [Google Scholar] [CrossRef]
- Onishi, M.; Ishihara, D. Performance evaluation of the pixel wing model for the insect wing’s camber. J. Adv. Simul. Sci. Eng. 2021, 8, 163–172. [Google Scholar] [CrossRef]
- Kesel, A.B.; Philippi, U.; Nachtigall, W. Biomechanical aspects of the insect wing: An analysis using the finite element method. Comput. Biol. Med. 1998, 28, 423–437. [Google Scholar] [CrossRef]
- Herbert, R.C.; Young, P.G.; Smith, C.W.; Wootton, R.J.; Evans, K.E. The hind wing of the desert locust (Schistocerca Gregaria Forskal), III. A finite element analysis of a deployable structure. J. Exp. Biol. 2000, 203, 2945–2955. [Google Scholar] [CrossRef]
- Mengesha, T.E.; Vallance, R.R.; Barraja, M.; Mittal, R. Parametric structural modeling of insect wings. Bioinspir. Biomim. 2009, 4, 036004. [Google Scholar] [CrossRef] [PubMed]
- Bathe, K.J.; Dvorkin, E.N. A four-node plate bending element based on Mindlin/Reissner plate theory and a mixed interpolation. Int. J. Numer. Methods Eng. 1985, 21, 367–383. [Google Scholar] [CrossRef]
- Noguchi, N.; Hisada, T. Sensitivity analysis in post-buckling problems of shell structures. Comput. Struct. 1993, 47, 699–710. [Google Scholar] [CrossRef]
- Tezduyar, T.E.; Mital, S.; Ray, S.E.; Shih, R. Incompressible flow computations with stabilized bilinear and linear equal-order-interpolation velocity-pressure elements. Comput. Methods Appl. Mech. Eng. 1992, 95, 221–242. [Google Scholar] [CrossRef]
- Zhang, Q.; Hisada, T. Analysis of fluid-structure interaction problems with structural buckling and large domain changes by ALE finite element methods. Comput. Methods Appl. Mech. Eng. 2001, 190, 6341–6357. [Google Scholar] [CrossRef]
- Ellington, C.P.; Van den Berg, C.; Willmott, A.P.; Thomas, L.R. Leading-edge vortices in insect flight. Nature 1996, 384, 626–630. [Google Scholar] [CrossRef]
- Ellington, C.P. The aerodynamics of hovering insect flight. II. Morphological Parameters. Philos. Trans. R. Soc. Lond. 1984, B305, 17–40. [Google Scholar]








| Parameters | Insect | Dynamically Scaled |
|---|---|---|
| Wing length Lw [m] | 0.0113 | 0.226 |
| Wing chord length cw [m] | 0.00311 | 0.0622 |
| Density of the fluid ρf [kg/m3] | 1.205 | 955 |
| Viscosity of the fluid μf [Pa s] | 1.80 × 10−5 | 2.87 × 10−2 |
| Flapping frequency fφ [Hz] | 160.5 | 0.809 |
| Wing mass mw [kg] | 4.0332 × 10−9 | 2.5577 × 10−2 |
| Torsional compliance Cθ [1/(N m)] | 432 × 103 | 6.71 |
| Stroke angle Φ [deg] | 107.5 | |
| α | 0.127 | |
| Re | 824 | |
| Ca | 0.244 | |
| MR | 0.111 | |
| Case | Fr | α | fφ [Hz] | Φ [°] | MR | Re | Ca |
|---|---|---|---|---|---|---|---|
| 1 | 3.32 | 0.112 | 0.801 | 80 | 16 | 254 | 0.19 |
| 2 | 4.14 | 0.0892 | 0.641 | 100 | |||
| 3 | 5.10 | 0.0726 | 0.521 | 123 | |||
| 4 | 5.80 | 0.0637 | 0.458 | 140 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ishihara, D. Computational Approach for the Fluid-Structure Interaction Design of Insect-Inspired Micro Flapping Wings. Fluids 2022, 7, 26. https://doi.org/10.3390/fluids7010026
Ishihara D. Computational Approach for the Fluid-Structure Interaction Design of Insect-Inspired Micro Flapping Wings. Fluids. 2022; 7(1):26. https://doi.org/10.3390/fluids7010026
Chicago/Turabian StyleIshihara, Daisuke. 2022. "Computational Approach for the Fluid-Structure Interaction Design of Insect-Inspired Micro Flapping Wings" Fluids 7, no. 1: 26. https://doi.org/10.3390/fluids7010026
APA StyleIshihara, D. (2022). Computational Approach for the Fluid-Structure Interaction Design of Insect-Inspired Micro Flapping Wings. Fluids, 7(1), 26. https://doi.org/10.3390/fluids7010026






