Relative Influences of Inertia and Polymeric Viscoelastic Effects on Nusselt Numbers within Rotating Couette Flows
Abstract
:1. Introduction
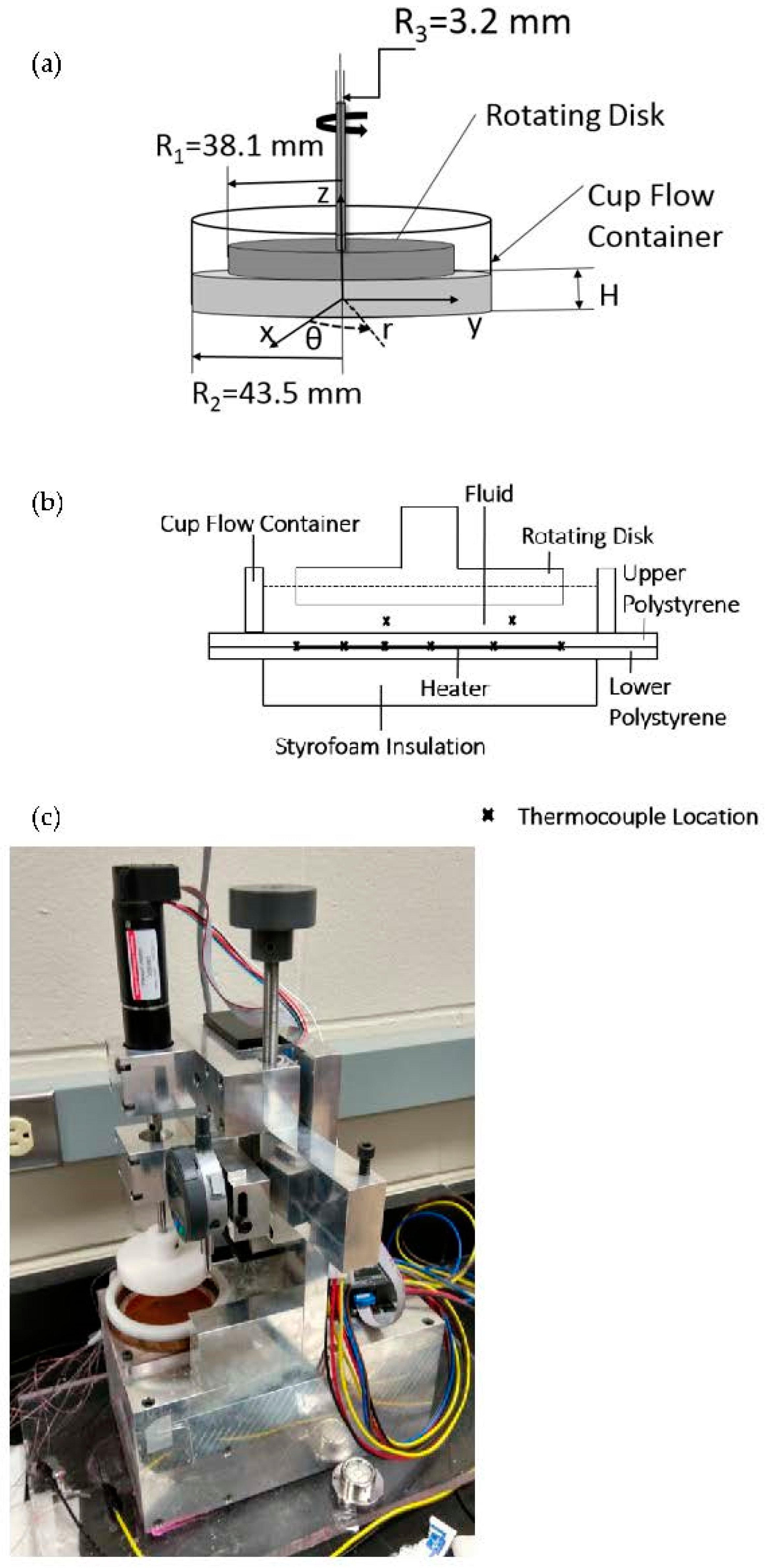
2. Experimental Apparatus and Procedures
2.1. Rotating Couette Flow (RCF) Experimental Apparatus
2.2. Polymer Solution Preparation
2.3. Heat Transfer and Nusselt Number Measurement Apparatus and Procedures
2.4. Experimental Uncertainty Magnitudes
3. Characterization of Inertial and Polymeric Viscoelastic Influences
4. Experimental Results and Discussion
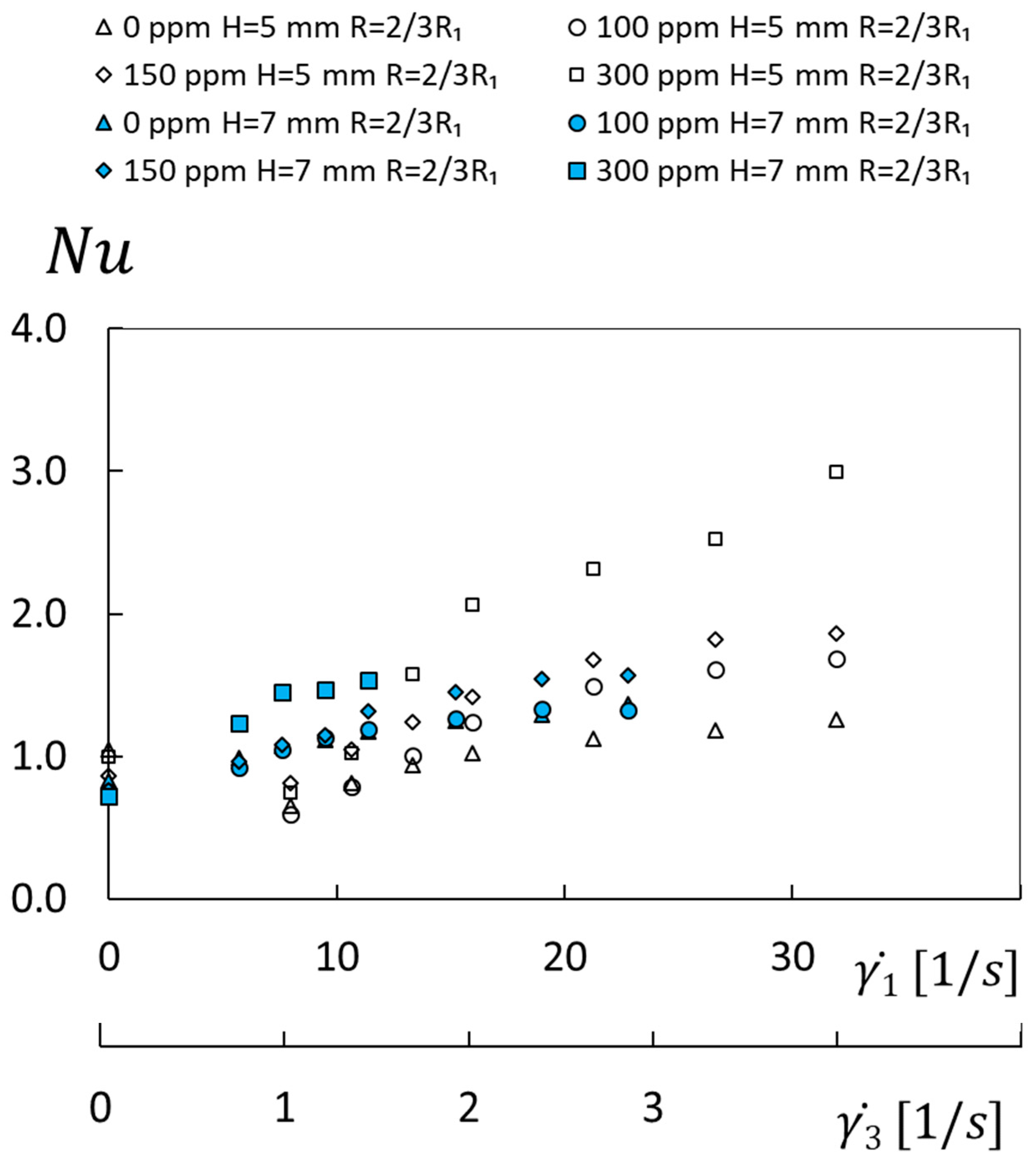
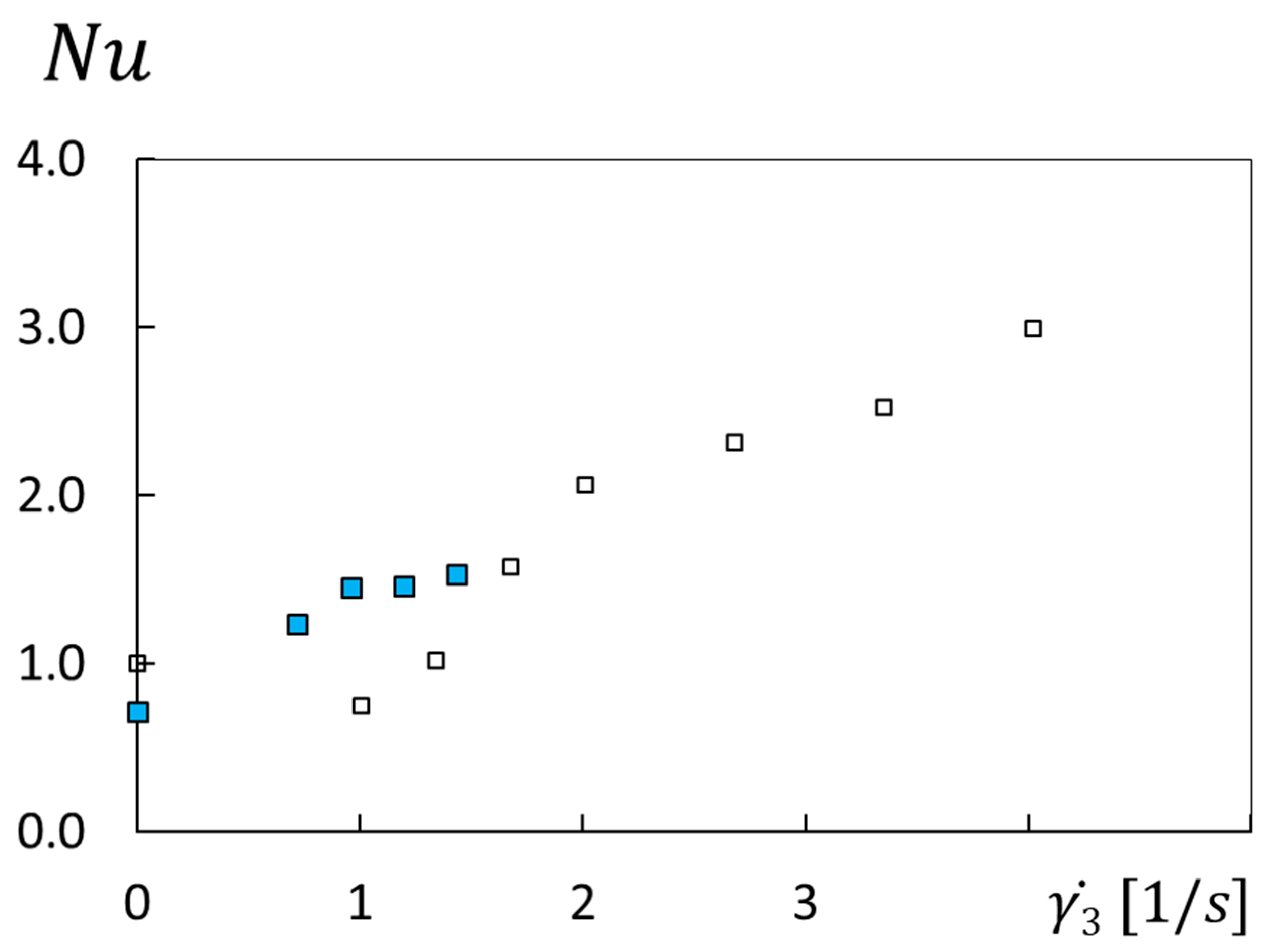
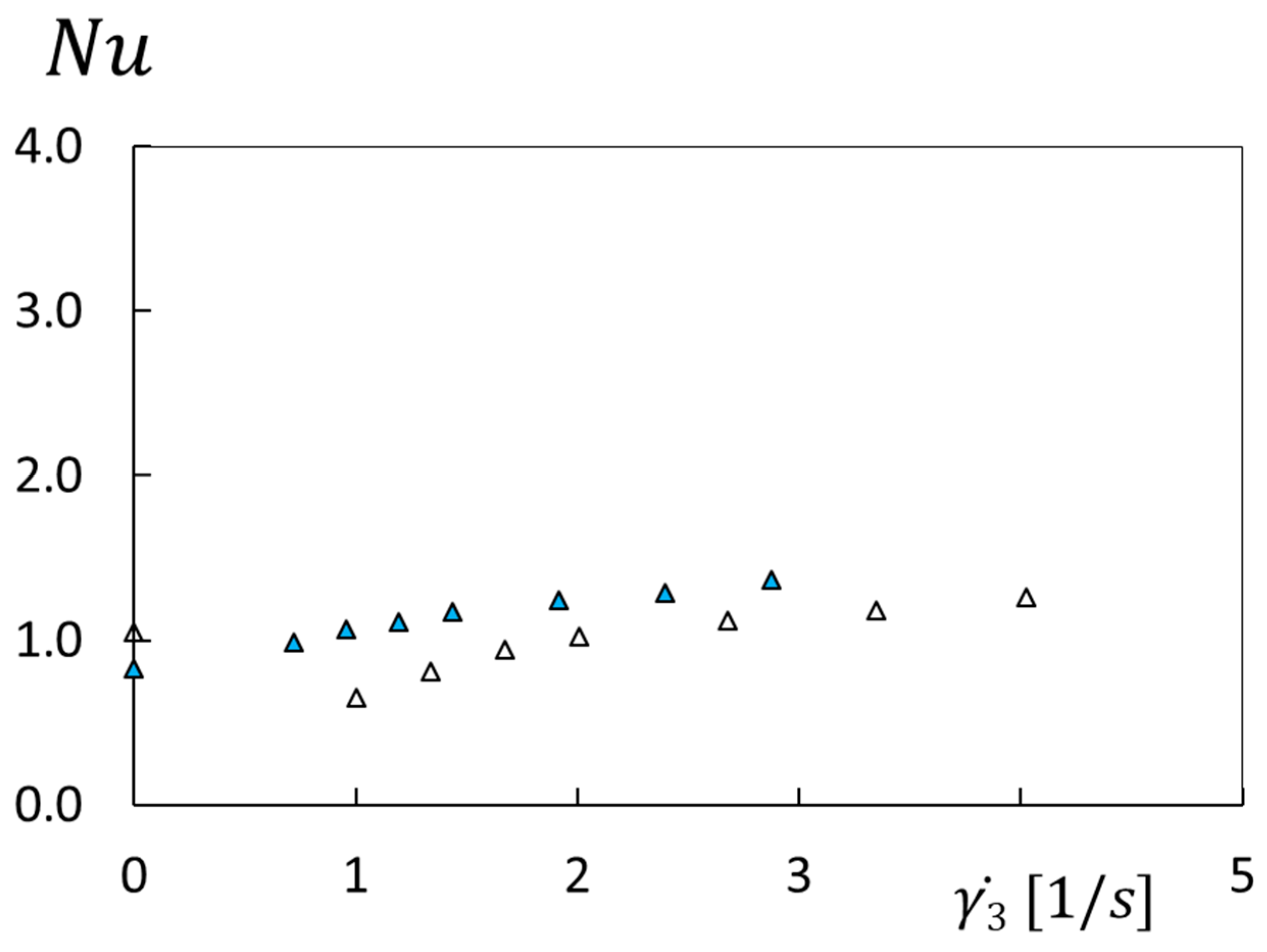
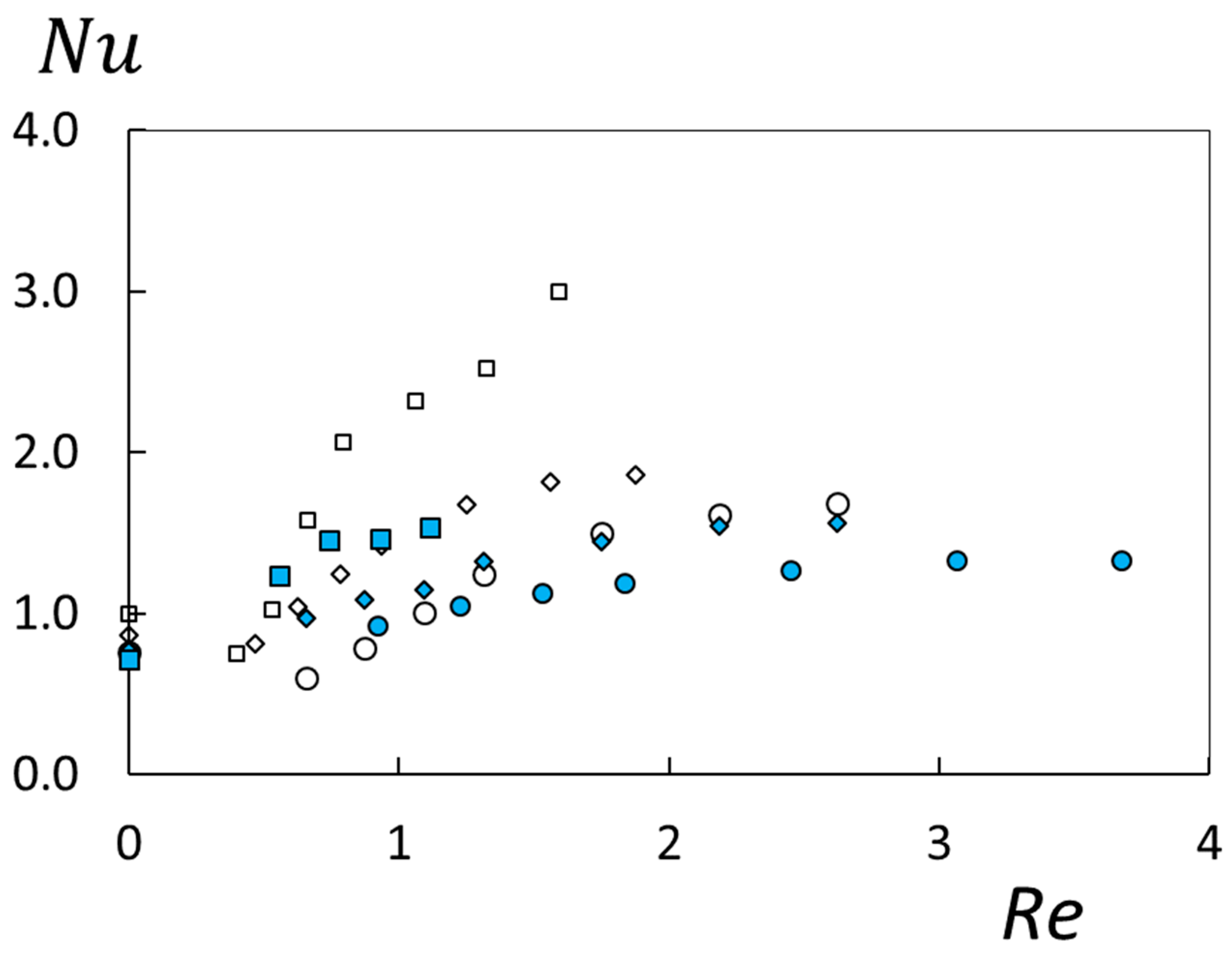
4.1. Nusselt Number Variations with Shear Rate
4.2. Nusselt Number Variations with Shear Rate and Reynolds Number When Dominated by Inertial Influences
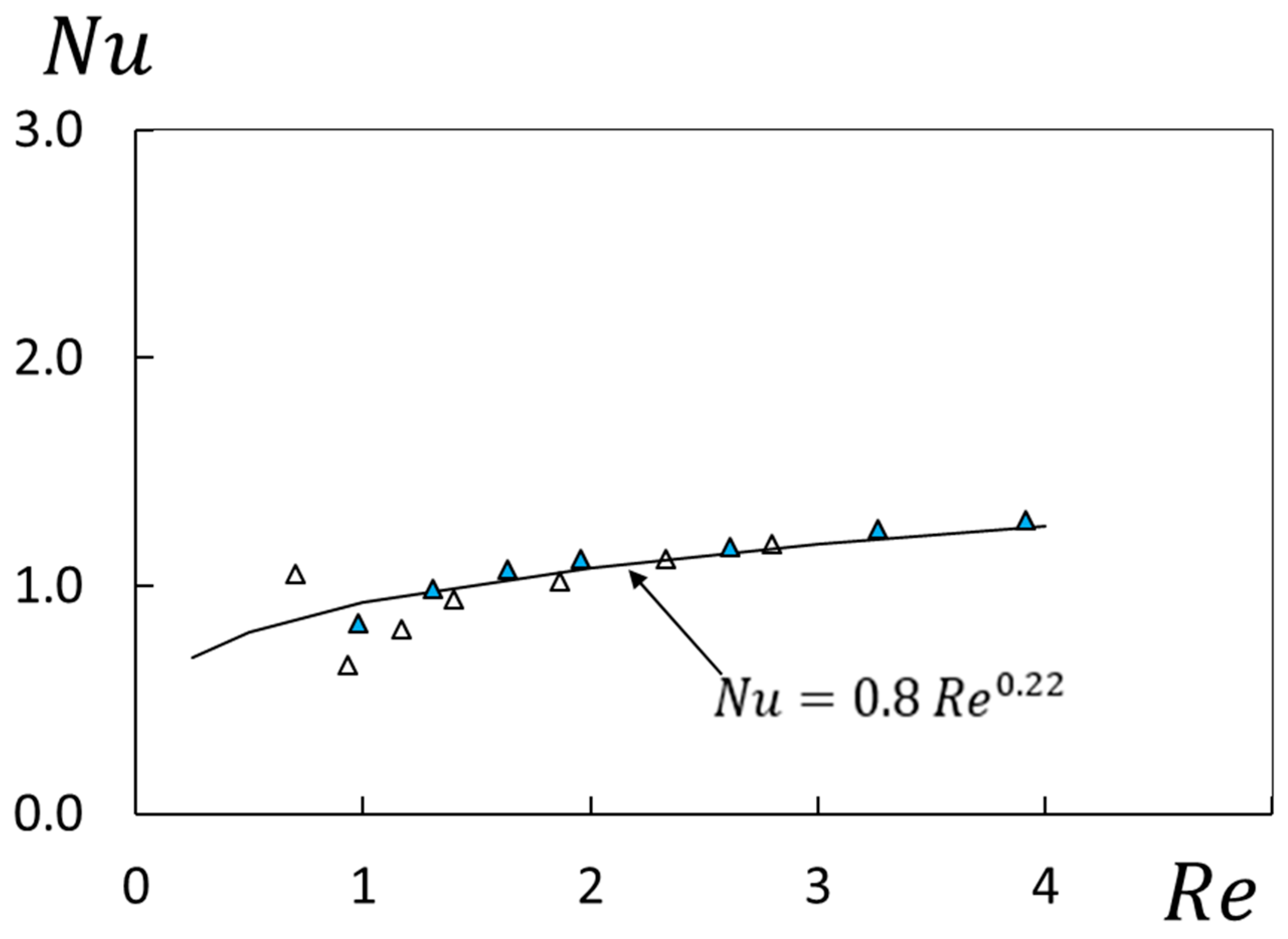
4.3. Nusselt Number Variations with Reynolds Number
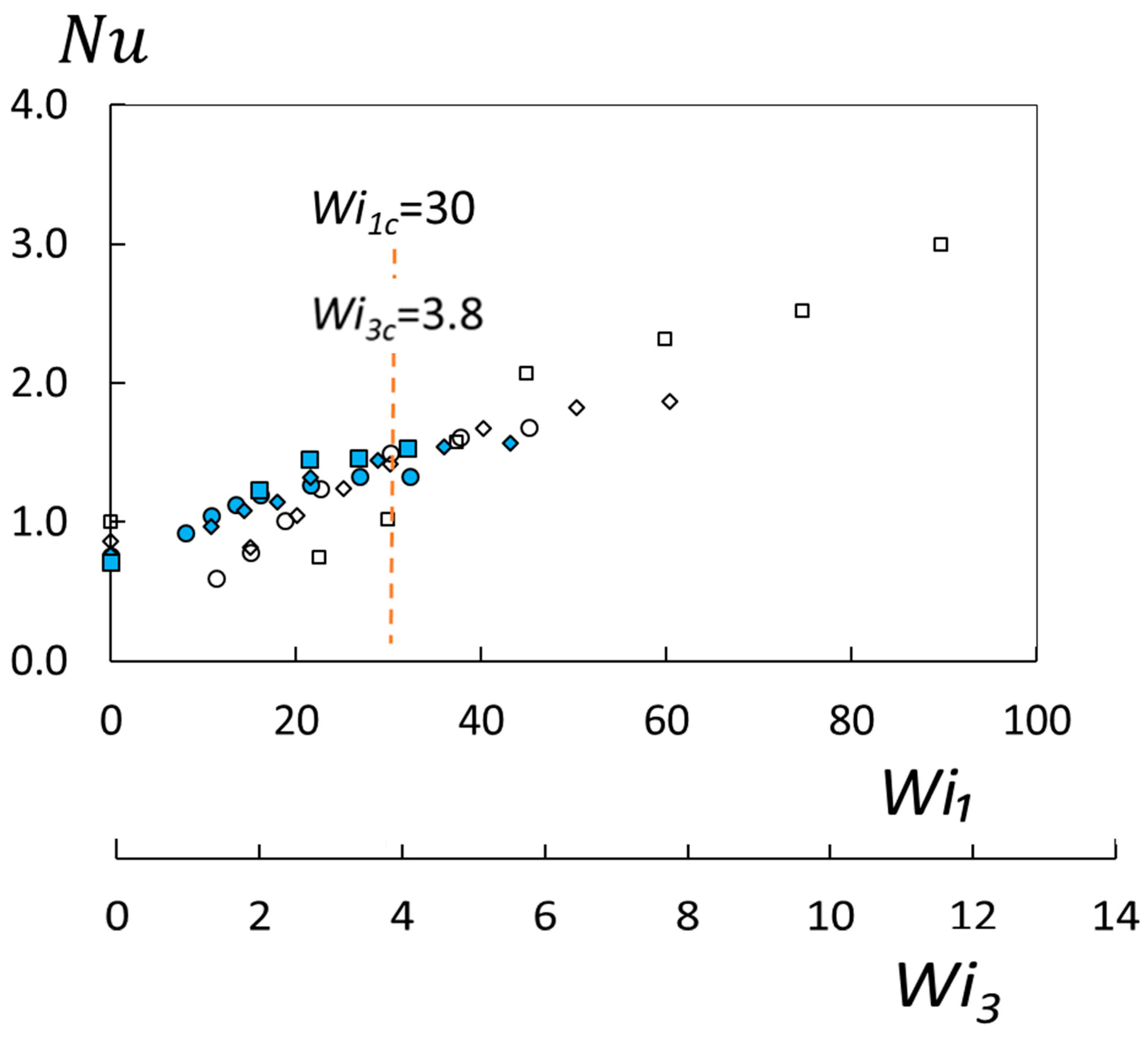
4.4. Nusselt Number Variations with Weissenberg Number
4.5. Nusselt Number Variations with a Modified P Parameter
5. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Cp | Specific heat (kJ/kg K) |
| h | Heat transfer coefficient (kW/m2K) |
| H | Flow passage height (m) |
| Kf | Thermal conductivity of the fluid (kW/mK) |
| Nu | Nusselt number |
| Nus | Nusselt number associated with sucrose solvent flow |
| r | Radial coordinate (m) |
| R | Radial location (m) |
| R1 | Outer radius of disk, and thermo-foil heater radius (m) |
| R2 | Inner radius of cup flow container (m) |
| R3 | Outer radius of rotating shaft (m) |
| Reynolds number | |
| Elastic instability Reynolds number | |
| Wi | Weissenberg number |
| x | First lateral coordinate (m) |
| y | Second lateral coordinate (m) |
| z | Normal coordinate (m) |
| Greek Symbols | |
| θ | Circumferential coordinate (m) |
| λ | Polymer relaxation time (s) |
| η | Absolute viscosity (kg/m s) |
| ηoo | Absolute viscosity at zero-shear rate (kg/m s) |
| η∞ | Absolute viscosity at infinite shear rate (kg/m s) |
| ηo | Absolute viscosity associated with solvent and polymer mixture flow (kg/m s) |
| ηs | Absolute viscosity associated with solvent flow (kg/m s) |
| ηp | Absolute viscosity associated with polymer flow (kg/m s) |
| ρ | Polymer concentration (ppm) |
| ρo | Fluid static density associated with solvent and polymer mixture flow (kg/m3) |
| σ11 | First normal stress difference in the direction of the flow (Pa) |
| Local fluid shear rate (1/s) | |
| ω | Angular rotation speed of disk (rad/s) |
References
- Larson, R.G. Instabilities in viscoelastic flows. Rheol. Acta 1992, 31, 213–263. [Google Scholar] [CrossRef]
- Pathak, J.A.; Ross, D.; Migler, K.B. Elastic flow instability, curved streamlines, and mixing in microfluidic flows. Phys. Fluids 2004, 16, 4028–4034. [Google Scholar] [CrossRef]
- Arratia, P.E.; Thomas, C.C.; Diorio, J.; Gollub, J.P. Elastic instabilities of polymer solutions in cross-channel flow. Phys. Rev. Lett. 2006, 96, 144502. [Google Scholar] [CrossRef] [PubMed]
- Pan, L.; Morozov, A.; Wagner, C.; Arratia, P.E. Nonlinear elastic instability in channel flows at low Reynolds numbers. Phys. Rev. Lett. 2013, 110, 174502. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.-N.; Li, F.-C.; Cao, Y.; Kunugi, T.; Yu, B. Direct numerical simulation of elastic turbulence and its mixing-enhancement effect in a straight channel. Chin. Phys. B 2013, 22, 024703. [Google Scholar] [CrossRef]
- Burghelea, T.; Segre, E.; Bar-Joseph, I.; Groisman, A.; Steinberg, V. Chaotic flow and efficient mixing in a microchannel with a polymer solution. Phys. Rev. E 2004, 69, 066305. [Google Scholar] [CrossRef] [PubMed]
- Groisman, A.; Steinberg, V. Elastic turbulence in a polymer solution flow. Nature 2000, 405, 53–55. [Google Scholar] [CrossRef] [PubMed]
- Groisman, A.; Steinberg, V. Efficient mixing at low Reynolds numbers using polymer additives. Nature 2001, 410, 905–908. [Google Scholar] [CrossRef] [PubMed]
- Qin, B.; Salipante, P.F.; Hudson, S.D.; Arratia, P.E. Flow resistance and structures in viscoelastic channel flows at low Re. Phys. Rev. Lett. 2019, 123, 194501. [Google Scholar] [CrossRef] [PubMed]
- Whalley, R.D.; Abed, W.M.; Dennis, D.J.C.; Poole, R.J. Enhancing heat transfer at the micro-scale using elastic turbulence. Theor. Appl. Mech. Lett. 2015, 5, 103–106. [Google Scholar] [CrossRef]
- Traore, B.; Castelain, C.; Burghelea, T. Efficient heat transfer in a regime of elastic turbulence. J. Non-Newton. Fluid Mech. 2015, 223, 62–76. [Google Scholar] [CrossRef]
- Abed, W.M.; Whalley, R.D.; Dennis, D.J.C.; Poole, R.J. Experimental investigation of the impact of elastic turbulence on heat transfer in a serpentine channel. J. Non-Newton. Fluid Mech. 2016, 231, 68–78. [Google Scholar] [CrossRef]
- Li, D.-Y.; Li, X.-B.; Zhang, H.-N.; Li, F.-C.; Qian, S.; Joo, S.W. Efficient heat transfer enhancement by elastic turbulence with polymer solution in a curved microchannel. Microfluid. Nanofluidics 2017, 21, 1846. [Google Scholar] [CrossRef]
- Ligrani, P.M.; Copeland, D.; Ren, C.; Su, M.; Suzuki, M. Heat transfer enhancements from elastic turbulence using sucrose-based polymer solutions. AIAA J. Thermophys. Heat Transf. 2018, 32, 51–60. [Google Scholar] [CrossRef]
- Ligrani, P.M.; Su, M.; Pippert, A.; Handler, R.A. Thermal transport of viscoelastic fluids within rotating Couette flows. AIAA J. Thermophys. Heat Transf. 2020, 34, 121–133. [Google Scholar] [CrossRef]
- Hietsch, V.; Ligrani, P.M.; Su, M. Characterization of effective diffusion within viscoelastic fluids with elastic instabilities. Fluids 2022, 7, 33. [Google Scholar] [CrossRef]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
- De Gennes, P.G. Coil-stretch transition of dilute flexible polymers under ultrahigh velocity gradients. J. Chem. Phys. 1974, 60, 5030. [Google Scholar] [CrossRef]
- Dubief, Y.; Terrapon, V.E.; Hof, B. Elasto-inertial turbulence. Annu. Rev. Fluid Mech. 2023, 55, 675–705. [Google Scholar] [CrossRef]

| ρ (ppm) | 0 | 100 | 150 | 300 |
|---|---|---|---|---|
| ρ0 (kg/m3) | 1311 | 1315 | 1315 | 1316 |
| kf (W/m·K) | 0.368 | 0.368 | 0.368 | 0.368 |
| cp (J/kg·K) | 2600 | 2600 | 2600 | 2600 |
| ηoo (Pa·s) | 0.187 | 0.287 | 0.345 | 0.439 |
| η∞ (Pa·s) | - | 0.200 | 0.280 | 0.330 |
| λ (s) | 0.000 | 1.415 | 1.893 | 2.809 |
| Concentration (ppm) | Time-Averaged Temperature | Nusselt Number |
|---|---|---|
| 0 (Sucrose Solution) | 8.4% | 5.1% |
| 100 | 6.6% | 6.3% |
| 150 | 5.9% | 5.5% |
| 300 | 5.4% | 5.1% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ligrani, P.; Hietsch, V.; Su, M. Relative Influences of Inertia and Polymeric Viscoelastic Effects on Nusselt Numbers within Rotating Couette Flows. Fluids 2023, 8, 258. https://doi.org/10.3390/fluids8100258
Ligrani P, Hietsch V, Su M. Relative Influences of Inertia and Polymeric Viscoelastic Effects on Nusselt Numbers within Rotating Couette Flows. Fluids. 2023; 8(10):258. https://doi.org/10.3390/fluids8100258
Chicago/Turabian StyleLigrani, Phil, Valerie Hietsch, and Mengying Su. 2023. "Relative Influences of Inertia and Polymeric Viscoelastic Effects on Nusselt Numbers within Rotating Couette Flows" Fluids 8, no. 10: 258. https://doi.org/10.3390/fluids8100258
APA StyleLigrani, P., Hietsch, V., & Su, M. (2023). Relative Influences of Inertia and Polymeric Viscoelastic Effects on Nusselt Numbers within Rotating Couette Flows. Fluids, 8(10), 258. https://doi.org/10.3390/fluids8100258






