Applicability of the Cox–Merz Relationship for Mayonnaise Enriched with Natural Extracts
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Formulation of Mayonnaise
2.3. Emulsion Stability
2.4. Rheological Analysis
2.4.1. Steady Shear Flow Rheology
2.4.2. Dynamic Oscillatory Rheology
2.5. Statistical Analysis
3. Results
3.1. Steady-State Behavior
3.2. Viscoelastic Properties
3.3. Applicability of the Cox–Merz Rule
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cox, W.P.; Merz, E.H. Correlation of Dynamic and Steady Flow Viscosities. J. Polym. Sci. 1958, 28, 619–622. [Google Scholar] [CrossRef]
- Bistany, K.L.; Kokini, J.L. Dynamic Viscoelastic Properties of Foods in Texture Control. J. Rheol. 1983, 27, 605–620. [Google Scholar] [CrossRef]
- Chamberlain, E.K.; Rao, M.A. Rheological Properties of Acid Converted Waxy Maize Starches in Water and 90% DMSO/10% Water. Carbohydr. Polym. 1999, 40, 251–260. [Google Scholar] [CrossRef]
- Park, S.; Chung, M.G.; Yoo, B. Effect of Octenylsuccinylation on Rheological Properties of Corn Starch Pastes. Starch—Stärke 2004, 56, 399–406. [Google Scholar] [CrossRef]
- Yaşar, K.; Kahyaoglu, T.; Şahan, N. Dynamic Rheological Characterization of Salep Glucomannan/Galactomannan-Based Milk Beverages. Food Hydrocoll 2009, 23, 1305–1311. [Google Scholar] [CrossRef]
- Augusto, P.E.D.; Ibarz, A.; Cristianini, M. Effect of High Pressure Homogenization (HPH) on the Rheological Properties of Tomato Juice: Viscoelastic Properties and the Cox–Merz Rule. J. Food Eng. 2013, 114, 57–63. [Google Scholar] [CrossRef]
- Razavi, S.M.A.; Cui, S.W.; Guo, Q.; Ding, H. Some Physicochemical Properties of Sage (Salvia Macrosiphon) Seedgum. Food Hydrocoll. 2014, 35, 453–462. [Google Scholar] [CrossRef]
- Zimeri, J.E.; Kokini, J.L. Rheological Properties of Inulin–Waxy Maize Starch Systems. Carbohydr. Polym. 2003, 52, 67–85. [Google Scholar] [CrossRef]
- Manero, O.; Bautista, F.; Soltero, J.F.A.; Puig, J.E. Dynamics of Worm-like Micelles: The Cox–Merz Rule. J. Nonnewton. Fluid Mech. 2002, 106, 1–15. [Google Scholar] [CrossRef]
- Riscardo, M.A.; Moros, J.E.; Franco, J.M.; Gallegos, C. Rheological Characterisation of Salad-Dressing-Type Emulsions Stabilised by Egg Yolk/Sucrose Distearate Blends. Eur. Food Res. Technol. 2005, 220, 380–388. [Google Scholar] [CrossRef]
- Davis, V.A.; Ericson, L.M.; Parra-Vasquez, A.N.G.; Fan, H.; Wang, Y.; Prieto, V.; Longoria, J.A.; Ramesh, S.; Saini, R.K.; Kittrell, C.; et al. Phase Behavior and Rheology of SWNTs in Superacids. Macromolecules 2004, 37, 154–160. [Google Scholar] [CrossRef]
- Dogan, M.; Kayacier, A.; Toker, Ö.S.; Yilmaz, M.T.; Karaman, S. Steady, Dynamic, Creep, and Recovery Analysis of Ice Cream Mixes Added with Different Concentrations of Xanthan Gum. Food Bioprocess Technol. 2013, 6, 1420–1433. [Google Scholar] [CrossRef]
- Karaman, S.; Yilmaz, M.T.; Kayacier, A. Mathematical Approach for Two Component Modeling of Salep-Starch Mixtures Using Central Composite Rotatable Design: Part II. Dynamic Oscillatory Shear Properties and Applicability of Cox-Merz Rule. Food Hydrocoll. 2013, 31, 277–288. [Google Scholar] [CrossRef]
- Mirzanajafi-Zanjani, M.; Yousefi, M.; Ehsani, A. Challenges and Approaches for Production of a Healthy and Functional Mayonnaise Sauce. Food Sci. Nutr. 2019, 7, 2471–2484. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.; Barbosa-Cánovas, G.V. Rheological Characterization of Mayonnaise. Part I: Slippage at Different Oil and Xanthan Gum Concentrations. J. Food Eng. 1995, 25, 397–408. [Google Scholar] [CrossRef]
- Figoni, P.I.; Shoemaker, C.F. Characterization of Time Dependent Flow Properties of Mayonnaise under Steady Shear. J. Texture Stud. 1983, 14, 431–442. [Google Scholar] [CrossRef]
- Kiosseoglou, V.D.; Sherman, P. Influence of Egg Yolk Lipoproteins on the Rheology and Stability of O/W Emulsions and Mayonnaise 1. Viscoelasticity of Groundnut Oil-In-Water Emulsions and Mayonnaise. J. Texture Stud. 1983, 14, 397–417. [Google Scholar] [CrossRef]
- Elliott, J.H.; Ganz, A.J. Salad Dressings—Preliminary Rheological Characterization. J. Texture Stud. 1977, 8, 359–371. [Google Scholar] [CrossRef]
- Chivero, P.; Gohtani, S.; Yoshii, H.; Nakamura, A. Assessment of Soy Soluble Polysaccharide, Gum Arabic and OSA-Starch as Emulsifiers for Mayonnaise-like Emulsions. LWT Food Sci. Technol. 2016, 69, 59–66. [Google Scholar] [CrossRef]
- Batista, A.P.; Raymundo, A.; Sousa, I.; Empis, J. Rheological Characterization of Coloured Oil-in-Water Food Emulsions with Lutein and Phycocyanin Added to the Oil and Aqueous Phases. Food Hydrocoll. 2006, 20, 44–52. [Google Scholar] [CrossRef]
- Guilmineau, F.; Kulozik, U. Influence of a Thermal Treatment on the Functionality of Hen’s Egg Yolk in Mayonnaise. J. Food Eng. 2007, 78, 648–654. [Google Scholar] [CrossRef]
- Katsaros, G.; Tsoukala, M.; Giannoglou, M.; Taoukis, P. Effect of Storage on the Rheological and Viscoelastic Properties of Mayonnaise Emulsions of Different Oil Droplet Size. Heliyon 2020, 6, e05788. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Q.; Fan, L.; Liu, Y.; Li, J. Mayonnaise-like High Internal Phase Pickering Emulsions Stabilized by Co-Assembled Phosphorylated Perilla Protein Isolate and Chitosan for Extrusion 3D Printing Application. Food Hydrocoll. 2023, 135, 108133. [Google Scholar] [CrossRef]
- Ma, L.; Barbosa-Cánovas, G.V. Rheological Characterization of Mayonnaise. Part II: Flow and Viscoelastic Properties at Different Oil and Xanthan Gum Concentrations. J. Food Eng. 1995, 25, 409–425. [Google Scholar] [CrossRef]
- Mancini, F.; Montanari, L.; Peressini, D.; Fantozzi, P. Influence of Alginate Concentration and Molecular Weight on Functional Properties of Mayonnaise. LWT 2002, 35, 517–525. [Google Scholar] [CrossRef]
- Dolz, M.; Hernández, M.J.; Delegido, J. Oscillatory Measurements for Salad Dressings Stabilized with Modified Starch, Xanthan Gum, and Locust Bean Gum. J. Appl. Polym. Sci. 2006, 102, 897–903. [Google Scholar] [CrossRef]
- Roshandel, Z.; Zibaei, R.; Abdolmaleki, K. Characteristics of Reduced-Fat Mayonnaise Prepared by Oleaster as a Fat Replacer and Natural Antioxidant. Food Sci. Nutr. 2023, 11, 3329–3338. [Google Scholar] [CrossRef]
- McClements, D.J. Food Emulsions: Principles, Practices, and Techniques, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Niknam, R.; Ghanbarzadeh, B.; Ayaseh, A.; Adun, P. Comprehensive Study of Intrinsic Viscosity, Steady and Oscillatory Shear Rheology of Barhang Seed Hydrocolloid in Aqueous Dispersions. J. Food Process Eng. 2019, 42, e13047. [Google Scholar] [CrossRef]
- Niknam, R.; Ghanbarzadeh, B.; Ayaseh, A.; Hamishehkar, H. Plantago Major Seed Gum Based Biodegradable Films: Effects of Various Plant Oils on Microstructure and Physicochemical Properties of Emulsified Films. Polym. Test 2019, 77, 105868. [Google Scholar] [CrossRef]
- Niknam, R.; Ghanbarzadeh, B.; Ayaseh, A.; Rezagholi, F. The Hydrocolloid Extracted from Plantago Major Seed: Effects on Emulsifying and Foaming Properties. J. Dispers. Sci. Technol. 2019, 41, 667–673. [Google Scholar] [CrossRef]
- di Mattia, C.; Balestra, F.; Sacchetti, G.; Neri, L.; Mastrocola, D.; Pittia, P. Physical and Structural Properties of Extra-Virgin Olive Oil Based Mayonnaise. LWT Food Sci. Technol. 2015, 62, 764–770. [Google Scholar] [CrossRef]
- Wu, Y.; Cui, W.; Eskin, N.A.M.; Goff, H.D. An Investigation of Four Commercial Galactomannans on Their Emulsion and Rheological Properties. Food Res. Int. 2009, 42, 1141–1146. [Google Scholar] [CrossRef]
- Niknam, R.; Ghanbarzadeh, B.; Ayaseh, A.; Rezagholi, F. The Effects of Plantago Major Seed Gum on Steady and Dynamic Oscillatory Shear Rheology of Sunflower Oil-in-Water Emulsions. J. Texture Stud. 2018, 49, 536–547. [Google Scholar] [CrossRef] [PubMed]
- Di Mattia, C.; Paradiso, V.M.; Andrich, L.; Giarnetti, M.; Caponio, F.; Pittia, P. Effect of Olive Oil Phenolic Compounds and Maltodextrins on the Physical Properties and Oxidative Stability of Olive Oil O/W Emulsions. Food Biophys. 2014, 9, 396–405. [Google Scholar] [CrossRef]
- Souilem, S.; Kobayashi, I.; Neves, M.A.; Sayadi, S.; Ichikawa, S.; Nakajima, M. Preparation of Monodisperse Food-Grade Oleuropein-Loaded W/O/W Emulsions Using Microchannel Emulsification and Evaluation of Their Storage Stability. Food Bioprocess Technol. 2014, 7, 2014–2027. [Google Scholar] [CrossRef]
- Depree, J.A.; Savage, G.P. Physical and Flavour Stability of Mayonnaise. Trends Food Sci. Technol. 2001, 12, 157–163. [Google Scholar] [CrossRef]
- Giacintucci, V.; Di Mattia, C.; Sacchetti, G.; Neri, L.; Pittia, P. Role of Olive Oil Phenolics in Physical Properties and Stability of Mayonnaise-like Emulsions. Food Chem. 2016, 213, 369–377. [Google Scholar] [CrossRef]
- Yoo, D.; Yoo, B. Rheology of Rice Starch-Sucrose Composites. Starch—Staerke 2005, 57, 254–261. [Google Scholar] [CrossRef]
- Bair, S.; Yamaguchi, T.; Brouwer, L.; Schwarze, H.; Vergne, P.; Poll, G. Oscillatory and Steady Shear Viscosity: The Cox-Merz Rule, Superposition, and Application to EHL Friction. Tribol. Int. 2014, 79, 126–131. [Google Scholar] [CrossRef]
- Franco, J.M.; Berjano, M.; Guerrero, A.; Muñoz, J.; Gallegos, C. Flow Behaviour and Stability of Light Mayonnaise Containing a Mixture of Egg Yolk and Sucrose Stearate as Emulsifiers. Food Hydrocoll. 1995, 9, 111–121. [Google Scholar] [CrossRef]
- Quintana, S.E.; Franco, J.M.; Garcia-Zapateiro, L. Physico-Chemical and Bromatological Characteristics of Arenca and Rheological Properties of Oil-in-Water Emulsions Containing Isolated Protein. Cienc. E Agrotecnologia 2015, 39, 634–641. [Google Scholar] [CrossRef]
- Guo, Q.; Zhu, X.; Zhen, W.; Li, Z.; Kang, J.; Sun, X.; Wang, S.; Cui, S.W. Rheological Properties and Stabilizing Effects of High-Temperature Extracted Flaxseed Gum on Oil/Water Emulsion Systems. Food Hydrocoll. 2021, 112, 106289. [Google Scholar] [CrossRef]
- Mieles-Gómez, L.; Lastra-Ripoll, S.E.; Torregroza-Fuentes, E.; Quintana, S.E.; García-Zapateiro, L.A. Rheological and Microstructural Properties of Oil-in-Water Emulsion Gels Containing Natural Plant Extracts Stabilized with Carboxymethyl Cellulose/Mango (Mangifera indica) Starch. Fluids 2021, 6, 312. [Google Scholar] [CrossRef]
- Rao, M.A.; Tattiyakul, J. Granule Size and Rheological Behavior of Heated Tapioca Starch Dispersions. Carbohydr. Polym. 1999, 38, 123–132. [Google Scholar] [CrossRef]
- Gunasekaran, S.; Ak, M.M. Dynamic Oscillatory Shear Testing of Foods—Selected Applications. Trends Food Sci. Technol. 2000, 11, 115–127. [Google Scholar] [CrossRef]
- Shende, T.; Niasar, V.J.; Babaei, M. An Empirical Equation for Shear Viscosity of Shear Thickening Fluids. J. Mol. Liq. 2021, 325, 115220. [Google Scholar] [CrossRef]
- Bengoechea, C.; López, M.L.; Cordobés, F.; Guerrero, A. Influence of Semicontinuous Processing on the Rheology and Droplet Size Distribution of Mayonnaise-like Emulsions. Food Sci. Technol. Int. 2009, 15, 367–373. [Google Scholar] [CrossRef]
- Håkansson, A.; Chaudhry, Z.; Innings, F. Model Emulsions to Study the Mechanism of Industrial Mayonnaise Emulsification. Food Bioprod. Process. 2016, 98, 189–195. [Google Scholar] [CrossRef]
- Juszczak, L.; Fortuna, T.; Kośla, A. Sensory and Rheological Properties of Polish Commercial Mayonnaise. Nahrung—Food 2003, 47, 232–235. [Google Scholar] [CrossRef]
- Laverse, J.; Mastromatteo, M.; Frisullo, P.; Del Nobile, M.A. X-ray Microtomography to Study the Microstructure of Mayonnaise. J. Food Eng. 2012, 108, 225–231. [Google Scholar] [CrossRef]
- Paredes, M.D.C.; Rao, M.A.; Bourne, M.C. Rheological Characterization of Salad Dressings 2: Effect of Storage. J. Texture Stud. 1989, 20, 235–250. [Google Scholar] [CrossRef]
- Yilmazer, G.; Carrillo, A.R.; Kokini, J.L. Effect of Propylene Glycol Alginate and Xanthan Gum on Stability of O/W Emulsions. J. Food Sci. 1991, 56, 513–517. [Google Scholar] [CrossRef]
- Kumar, Y.; Roy, S.; Devra, A.; Dhiman, A.; Prabhakar, P.K. Ultrasonication of Mayonnaise Formulated with Xanthan and Guar Gums: Rheological Modeling, Effects on Optical Properties and Emulsion Stability. LWT 2021, 149, 111632. [Google Scholar] [CrossRef]
- Worrasinchai, S.; Suphantharika, M.; Pinjai, S.; Jamnong, P. β-Glucan Prepared from Spent Brewer’s Yeast as a Fat Replacer in Mayonnaise. Food Hydrocoll. 2006, 20, 68–78. [Google Scholar] [CrossRef]
- Haddarah, A.; Bassal, A.; Ismail, A.; Gaiani, C.; Ioannou, I.; Charbonnel, C.; Hamieh, T.; Ghoul, M. The Structural Characteristics and Rheological Properties of Lebanese Locust Bean Gum. J. Food Eng. 2014, 120, 204–214. [Google Scholar] [CrossRef]
- Izidoro, D.; Sierakowski, M.R.; Waszczynskyj, N.; Haminiuk, C.W.I.; Scheer, A.D.P. Sensory Evaluation and Rheological Behavior of Commercial Mayonnaise. Int. J. Food Eng. 2007, 3, 1–15. [Google Scholar] [CrossRef]
- Yüceer, M.; Ilyasoǧlu, H.; Özçelik, B. Comparison of Flow Behavior and Physicochemical Characteristics of Low-Cholesterol Mayonnaises Produced with Cholesterol-Reduced Egg Yolk. J. Appl. Poult. Res. 2016, 25, 518–527. [Google Scholar] [CrossRef]
- Park, J.J.; Olawuyi, I.F.; Lee, W.Y. Characteristics of Low-Fat Mayonnaise Using Different Modified Arrowroot Starches as Fat Replacer. Int. J. Biol. Macromol. 2020, 153, 215–223. [Google Scholar] [CrossRef]
- Tudorache, M.; Bordenave, N. Phenolic Compounds Mediate Aggregation of Water-Soluble Polysaccharides and Change Their Rheological Properties: Effect of Different Phenolic Compounds. Food Hydrocoll. 2019, 97, 105193. [Google Scholar] [CrossRef]
- Quintana, S.E.; Llalla, O.; García-Zapateiro, L.A.; García-Risco, M.R.; Fornari, T. Preparation and Characterization of Licorice-Chitosan Coatings for Postharvest Treatment of Fresh Strawberries. Appl. Sci. 2020, 10, 8431. [Google Scholar] [CrossRef]
- Rao, A.M. Measurement of Flow and Viscoelastic Properties. In Rheology of Fluid, Semisolid, and Solid Foods—Food Engineering Series; Springer: Boston, MA, USA, 2014; pp. 63–159. ISBN 978-1-4614-9230-6. [Google Scholar]
- Mun, S.; Kim, Y.L.; Kang, C.G.; Park, K.H.; Shim, J.Y.; Kim, Y.R. Development of Reduced-Fat Mayonnaise Using 4αGTase-Modified Rice Starch and Xanthan Gum. Int. J. Biol. Macromol. 2009, 44, 400–407. [Google Scholar] [CrossRef] [PubMed]
- Ahmed, J.; Ramaswamy, H.S. Effect of Temperature on Dynamic Rheology and Colour Degradation Kinetics of Date Paste. Food Bioprod. Process. 2005, 83, 198–202. [Google Scholar] [CrossRef]
- Muñtoz, J.; Sherman, P. Dynamic Viscoelastic Properties of Some Commercial Salad Dressings. J. Texture Stud. 1990, 21, 411–426. [Google Scholar] [CrossRef]
- Ma, Z.; Boye, J.I. Advances in the Design and Production of Reduced-Fat and Reduced-Cholesterol Salad Dressing and Mayonnaise: A Review. Food Bioprocess Technol. 2013, 6, 648–670. [Google Scholar] [CrossRef]
- Alvarez-Sabatel, S.; De Marañón, I.M.; Arboleya, J.C. Impact of High Pressure Homogenisation (HPH) on Inulin Gelling Properties, Stability and Development during Storage. Food Hydrocoll. 2015, 44, 333–344. [Google Scholar] [CrossRef]
- Gabriele, D.; De Cindio, B.; D’Antona, P. A Weak Gel Model for Foods. Rheol. Acta 2001, 40, 120–127. [Google Scholar] [CrossRef]
- Akhtar, G.; Masoodi, F.A. Structuring Functional Mayonnaise Incorporated with Himalayan Walnut Oil Pickering Emulsions by Ultrasound Assisted Emulsification. Ultrason. Sonochem. 2022, 86, 106022. [Google Scholar] [CrossRef]
- Bhardwaj, M.; Sandhu, K.S.; Saxena, D.C. Experimental and Modeling Studies of the Flow, Dynamic and Creep Recovery Properties of Pearl Millet Starch as Affected by Concentration and Cultivar Type. Int. J. Biol. Macromol. 2019, 135, 544–552. [Google Scholar] [CrossRef]
- Yousefi, A.R.; Razavi, S.M.A. Dynamic Rheological Properties of Wheat Starch Gels as Affected by Chemical Modification and Concentration. Starch—Staerke 2015, 67, 567–576. [Google Scholar] [CrossRef]
- Sun, C.; Liu, R.; Liang, B.; Wu, T.; Sui, W.; Zhang, M. Microparticulated Whey Protein-Pectin Complex: A Texture-Controllable Gel for Low-Fat Mayonnaise. Food Res. Int. 2018, 108, 151–160. [Google Scholar] [CrossRef]
- Peressini, D.; Sensidoni, A.; De Cindio, B. Rheological Characterization of Traditional and Light Mayonnaises. J. Food Eng. 1998, 35, 409–417. [Google Scholar] [CrossRef]
- Mounsey, J.S.; O’Riordan, E.D. Characteristics of Imitation Cheese Containing Native Starches. J. Food Sci. 2001, 66, 586–591. [Google Scholar] [CrossRef]
- Rincón, F.; Muñoz, J.; Ramírez, P.; Galán, H.; Alfaro, M.C. Physicochemical and Rheological Characterization of Prosopis Juliflora Seed Gum Aqueous Dispersions. Food Hydrocoll. 2014, 35, 348–357. [Google Scholar] [CrossRef]
- García-Cruz, E.E.; Rodríguez-Ramírez, J.; Méndez Lagunas, L.L.; Medina-Torres, L. Rheological and Physical Properties of Spray-Dried Mucilage Obtained from Hylocereus Undatus Cladodes. Carbohydr. Polym. 2013, 91, 394–402. [Google Scholar] [CrossRef] [PubMed]
- Alvarez, M.D.; Fernández, C.; Canet, W. Rheological Behaviour of Fresh and Frozen Potato Puree in Steady and Dynamic Shear at Different Temperatures. Eur. Food Res. Technol. 2004, 218, 544–553. [Google Scholar] [CrossRef]
- Ahmed, J.; Ramaswamy, H.S. Viscoelastic and Thermal Characteristics of Vegetable Puree-Based Baby Foods. J. Food Process Eng. 2006, 29, 219–233. [Google Scholar] [CrossRef]
- Ishii, M.; Nakamura, H. Applicability of Modified Cox-Merz Rule to Concentrated Suspensions. J. Nonnewton. Fluid Mech. 2020, 282, 104322. [Google Scholar] [CrossRef]
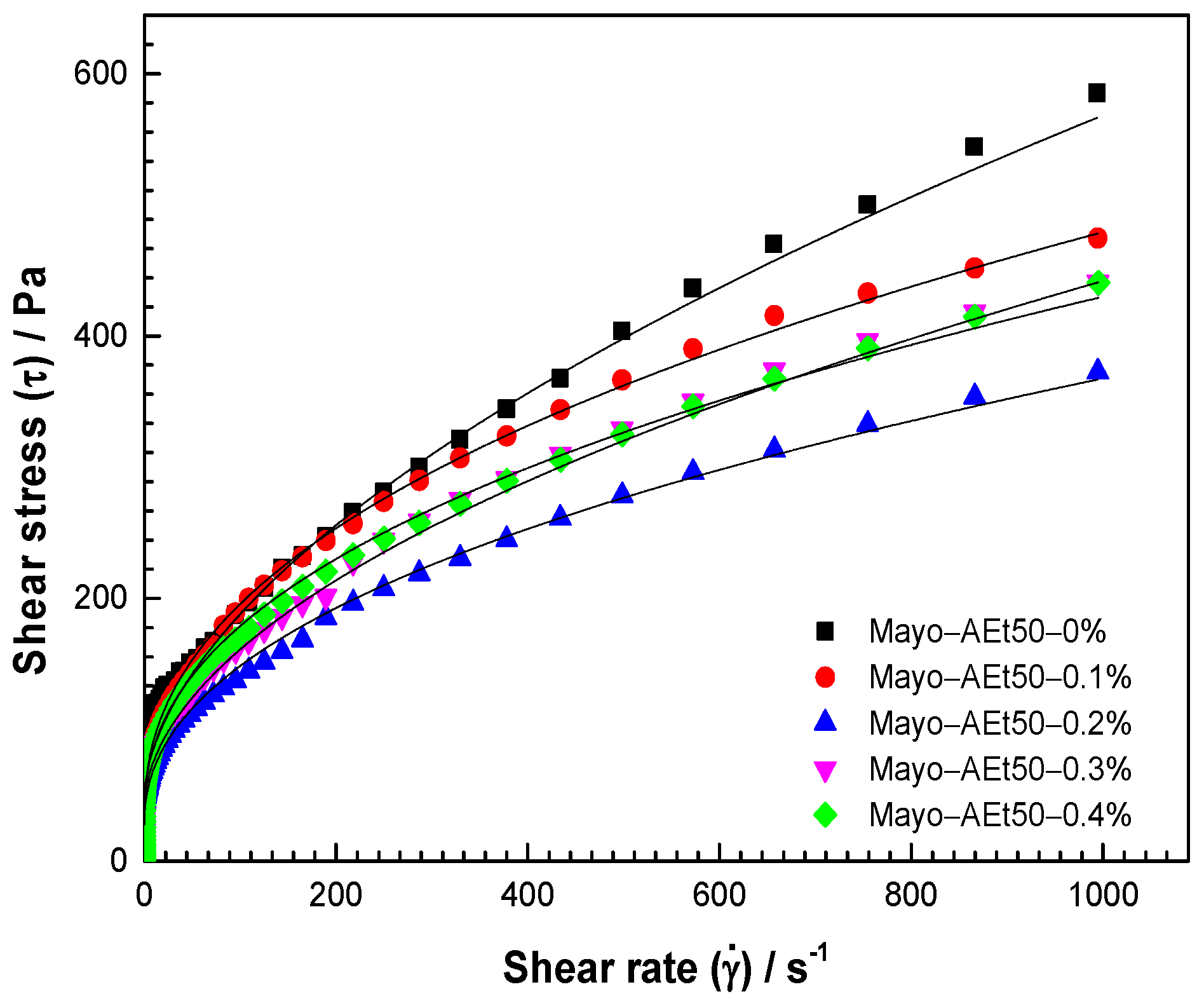
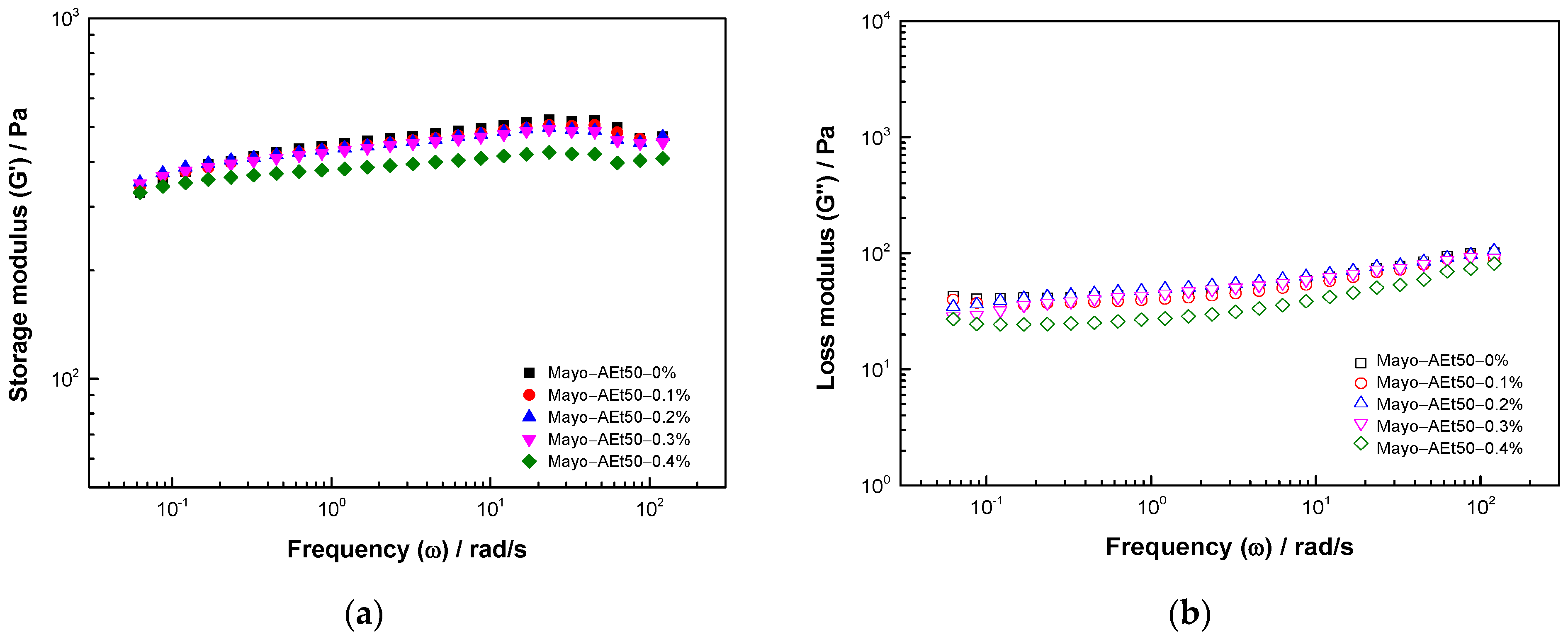
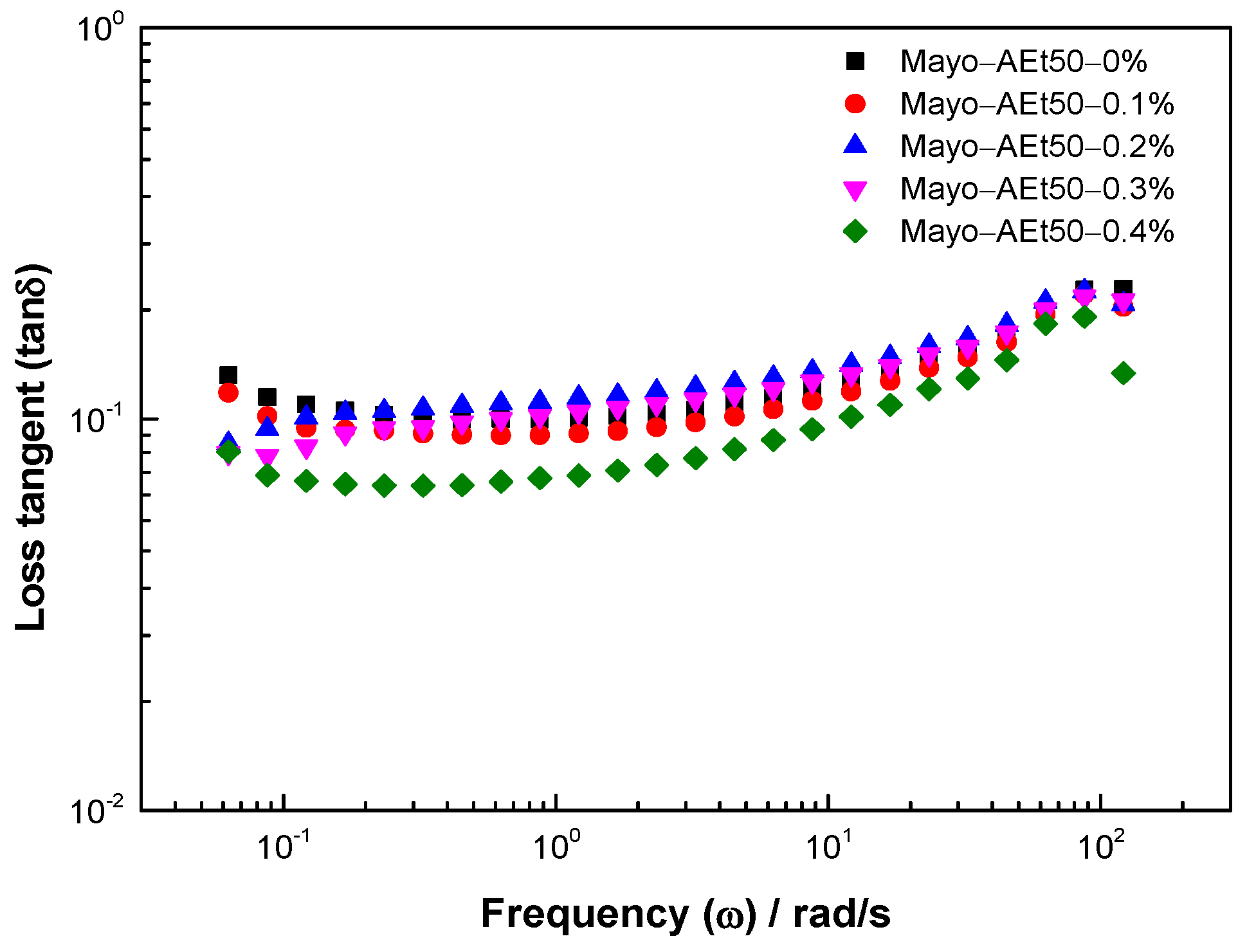


| Sample Code | Pa | K Pa·sn | n | R2 |
|---|---|---|---|---|
| 0% | 5.08 c | 1.79 a | 0.02 b | 0.96 |
| 0.1% | 1.82 b | 1.37 c | 0.01 a | 0.98 |
| 0.2% | 1.62 a | 1.19 b | 0.01 a | 0.98 |
| 0.3% | 1.94 b | 1.11 a | 0.01 b | 0.98 |
| 0.4% | 1.96 b | 1.48 c | 0.01 a | 0.98 |
| Sample Code | k′ Pa·sn′ | n′ | R2 | k″ Pa·sn″ | n″ | R2 |
|---|---|---|---|---|---|---|
| 0% | 5.92 b | 0.00 c | 0.731 | 1.20 c | 0.00 a | 0.921 |
| 0.1% | 4.64 b | 0.00 b | 0.781 | 1.26 b | 0.01 a | 0.909 |
| 0.2% | 4.24 b | 0.00 b | 0.757 | 0.66 c | 0.00 a | 0.976 |
| 0.3% | 4.27 b | 0.00 b | 0.761 | 0.45 b | 0.00 a | 0.988 |
| 0.4% | 2.47 a | 0.00 a | 0.812 | 1.09 a | 0.01 b | 0.920 |
| Sample Code | |||||
|---|---|---|---|---|---|
| R2 | R2 | ||||
| 0% | 1.013 a | 0.99 | 0.824 a | 1.025 a | 0.99 |
| 0.1% | 1.257 b | 0.99 | 1.263 c | 0.999 a | 0.99 |
| 0.2% | 1.606 c | 0.99 | 1.927 d | 0.997 a | 0.99 |
| 0.3% | 1.371 b | 0.99 | 0.928 b | 1.049 a | 0.99 |
| 0.4% | 1.083 a | 0.99 | 0.850 a | 1.029 a | 0.99 |
| Sample Code | ||||||
|---|---|---|---|---|---|---|
| A | B | R2 | A | B | R2 | |
| 0% | 0.995 a | 0.0 a | 0.99 | 0.995 a | 0.0 a | 0.99 |
| 0.1% | 0.998 a | 0.0 a | 0.99 | 0.998 a | 0.0 a | 0.99 |
| 0.2% | 0.999 a | 0.0 a | 0.99 | 0.999 a | 0.0 a | 0.99 |
| 0.3% | 0.999 a | 0.0 a | 0.99 | 0.999 a | 0.0 a | 0.99 |
| 0.4% | 0.997 a | 0.0 a | 0.99 | 0.994 a | 0.0 a | 0.99 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quintana, S.E.; Zuñiga-Navarro, M.; Ramirez-Brewer, D.; García-Zapateiro, L.A. Applicability of the Cox–Merz Relationship for Mayonnaise Enriched with Natural Extracts. Fluids 2023, 8, 287. https://doi.org/10.3390/fluids8110287
Quintana SE, Zuñiga-Navarro M, Ramirez-Brewer D, García-Zapateiro LA. Applicability of the Cox–Merz Relationship for Mayonnaise Enriched with Natural Extracts. Fluids. 2023; 8(11):287. https://doi.org/10.3390/fluids8110287
Chicago/Turabian StyleQuintana, Somaris E., Maria Zuñiga-Navarro, David Ramirez-Brewer, and Luis A. García-Zapateiro. 2023. "Applicability of the Cox–Merz Relationship for Mayonnaise Enriched with Natural Extracts" Fluids 8, no. 11: 287. https://doi.org/10.3390/fluids8110287
APA StyleQuintana, S. E., Zuñiga-Navarro, M., Ramirez-Brewer, D., & García-Zapateiro, L. A. (2023). Applicability of the Cox–Merz Relationship for Mayonnaise Enriched with Natural Extracts. Fluids, 8(11), 287. https://doi.org/10.3390/fluids8110287










